Patterns of interventions and the effect of coalitions and sociality on male fitness

Abstract

In group living animals, especially among primates, there is consistent evidence that high-ranking males gain a higher reproductive output than low-ranking males. Primate studies have shown that male coalitions and sociality can impact male fitness; however, it remains unclear whether males could potentially increase their fitness by preferentially supporting and socializing with females. Here we investigate patterns of male interventions and the effect of coalitions and sociality on male fitness in rhesus macaques (Macaca mulatta) with particular focus on male-female interactions. We combined behavioral collected on Cayo Santiago with genetic data analyzed for male reproductive output and relatedness. Our results revealed that the ten top-ranking males provided the majority of all male support observed. In contrast to other primates, male rhesus macaques mainly formed all-down coalitions suggesting that coalitions are less likely used to enhance male dominance. Males supporting females during and before their likely conception were not more likely to fertilize those females. We also found no evidence that males preferably support their offspring or other close kin. Interestingly, the most important predictor of male support was sociality, since opponents sharing a higher sociality index with a given male were more likely to be supported. Furthermore, a high sociality index of a given male-female dyad resulted in a higher probability of paternity. Overall, our results strengthen the evidence that sociality affects fitness in male primates, but also suggest that in species in which males queue for dominance, it is less likely that males derive fitness benefits from coalitions.

Introduction

Coalitions are one of the most impressive forms of cooperation observed in the animal kingdom. While intervening in an ongoing conflict between two opponents, the intervener provides support in favor of one opponent while simultaneously targeting the other. Coalitions thus include both cooperation and competition (compare de Waal 1992). The conditions favoring coalition formation are likely to differ across species, however, agonistic support has been reported in several species of mammals, among them African lions (Panthera leo) (Packer & Pusey 1982), African wild dogs (Lycaon pictus) (de Villiers et al. 2003), bottlenose dolphins (Tursiops sp.) (Connor et al. 1999), fallow deer (Dama dama) (Jennings et al. 2009), spotted hyenas (Crocuta crocuta) (Wahaj et al. 2004), and several species of primates, e.g., macaques (e.g., Kaplan 1977, 1978; Silk 1982, 1992a,b, 1993; Petit & Thierry 1994; Widdig et al. 2000; Bissonnette et al. 2009; Berghänel et al. 2010), baboons (e.g., Buchan et al. 2003; Silk et al. 2004) and chimpanzees (Pan troglodytes) (e.g., de Waal 1982; Langergraber et al. 2007). However, coalitions are not displayed equally often across species, and in addition a substantial sex difference regarding the frequency of intervention has been reported (Kaplan 1977; Bernstein & Ehardt 1985).

In primates, conditions favoring coalition formation among females have received much theoretical attention (Wrangham 1980; van Schaik 1989; Isbell 1991; Sterck et al. 1997). In most species the high level of contest competition leads female primates to stay in their natal group together with their relatives, forming strong bonds and frequently supporting each other in coalitions. Empirical evidence, mainly restricted to Cercopithecine primates (i.e., macaques and baboons), suggests that females preferentially support their close maternal kin, particularly offspring, as found in, e.g., Japanese macaques (Macaca fuscata) (Chapais et al. 1997), rhesus macaques (Kapsalis & Berman 1996a,b), and Savannah baboons (Papio cynocephalus) (Silk et al. 2004; but see also Cheney et al. 2010). Furthermore, females intervene on behalf of their kin even at a high risk of retaliation (Kaplan 1977; Silk 1982; Datta 1983a,b; Bernstein & Ehardt 1985; Widdig et al. 2006b).

Coalition formation among male primates presumably requires a different theoretical framework, as most males leave their natal group to breed elsewhere (Pusey 1987) which is likely to reduce their chances to interact with and support relatives (Chapais 1995). Nevertheless, some studies suggest that male primates also support their kin (e.g., bonnet macaques (Macaca radiata) (Silk 1992b), Barbary macaques (M. sylvanus) (Widdig et al. 2000), rhesus macaques (Bernstein & Ehardt 1985), Savannah baboons (Buchan et al. 2003)). A current theoretical model predicts a link between male coalitions and fitness (van Schaik et al. 2004, 2006). According to this model, coalitions are classified by comparing dominance relations between the allies and the target: (i) both allies being higher ranking than the target ('conservative' or 'all-down'), (ii) both allies being lower-ranking than the target ('revolutionary' or 'all-up') or (iii) target is ranked between both allies ('bridging') (Chapais 1995; van Schaik et al. 2004, 2006). The model further makes testable predictions for studies incorporating genetic data. First, for species in which male reproduction is skewed towards a few males, the model predicts that mid- and low-ranking males form all-up, rank-changing coalitions to increase their dominance rank and hence improve their reproductive output (van Schaik et al. 2004, 2006). Given that the majority of primate species studied so far show a pronounced male reproductive skew (reviewed in Widdig 2007) all-up, rank-changing coalitions are expected to be common. Second, the model predicts the occurrence of bridging coalitions where high-ranking males support their lower-ranking relatives (van Schaik et al. 2006), but this has rarely been tested due to limited data on male relatedness. Remarkably, the current theoretical framework does not consider male support of females, specifically the potential benefit that males could derive from forming coalitions or developing social bonds with females.

Only recently, studies on primates revealed that coalitions and/or sociality can be linked to fitness. For example, female baboons who formed strong social bonds with one another survive longer (Silk et al. 2010) and offspring of highly social females enjoy higher survival rates (Silk et al. 2003, 2009). These strong social bonds are preferentially formed among related individuals of the philopatric sex, yet there is also evidence of strong social bonds among unrelated individuals of the dispersing sex (female chimpanzees: Langergraber et al. 2009; Lehmann & Boesch 2009; male macaques (Macaca assamensis): Schülke et al. 2010). Furthermore, male macaques with stronger bonds form more coalitions with each other (Silk 1994; Schülke et al. 2010) and enjoyed enhanced future dominance success (Schülke et al. 2010) with the later study also demonstrating a link between male sociality and fitness.

While male coalition formation has been studied intensively in different species, studies on male sociality have emerged only recently and only one of them has yet looked at the effect of male coalitions and sociality on male fitness (Schülke et al. 2010). Therefore, the present study aims at investigating the effect of coalition formation and sociality on male fitness, with the specific focus on sociality within male and female dyads.

If male coalitions are linked to male fitness, this may occur over several paths. Firstly, males frequently intervening in conflicts of others may increase in rank, which in turn improves male access to conceptive females (e.g., Alberts et al. 2003) and hence paternity success (reviewed in Di Fiore 2003; Alberts & Altmann 2006). Secondly, after acquiring a higher rank, males might more frequently intervene in conflicts to stabilize their dominance position by predominantly targeting lower-ranking individuals (e.g., Kaplan 1978; Bernstein & Ehardt 1985; Silk 1993; Widdig et al. 2000). Thirdly, coalitions among males might increase access to mates without increasing dominance (“leveling”, cf. Pandit & van Schaik 2003) as shown for several primate species (e.g., Noë 1990; Duffy et al. 2007; Bissonnette 2009). For example, in species exhibiting at least partial female mate choice (Rhesus macaques: Manson 1992; Barbary macaques: Brauch et al. 2008), males might be more likely to bond and support sexually receptive females in order to increase their mating access. In addition, males can use coalitions to increase survival prospects of offspring and relatives. In general, it is difficult to determine fitness in male primates, as reproductive success can only be measured reliably when applying genetic paternity analyses (Ménard et al. 2001). To date, very few studies have been able to combine data on male support with male reproductive success and these studies suggested a link between male support and fitness (e.g., Pope 1990; Buchan et al. 2003; Schülke et al. 2010). However, despite their potential to enhance fitness, male coalitions appear to be puzzlingly rare across species (Cheney 2010).

Here we investigate coalition formation among non-natal male rhesus macaques a species in which male-male coalitions are not frequently observed as well as male interventions in conflicts of other age-sex classes (Kaplan 1977; Bernstein & Ehardt 1985; but see Higham & Maestripieri 2010). Rhesus macaques live in multi-male, multi-female groups characterized by female philopatry and male dispersal (Gouzoules & Gouzoules 1987). Genetic studies have shown that male reproduction is skewed towards few high-ranking males producing the majority of offspring (Berard et al. 1994; Bercovitch & Nürnberg 1996; Widdig et al. 2004). In addition to male dominance affecting male reproductive output, there is evidence for female attraction to novel males (Manson 1992; Dubuc et al. 2011). Male rhesus macaques most often enter the dominance hierarchy at the bottom (‘bottom entry’) and increase in rank through succession (hereafter: queuing), while overt dyadic aggression is rarely observed (Berard 1999).

The first objective of the present study was to investigate what causes male interventions by comparing dyadic conflicts with and without intervention by a third individual. Our second objective was to understand the patterns of coalition formation among male rhesus macaques. Finally, our last objective was to investigate the impact of coalitions and sociality on male fitness. Here, we tested a potential link between coalitions and male fitness by asking whether male coalitions are used (i) to achieve or maintain dominance, (ii) to increase mating access to sexually receptive females, or (iii) to increase survival prospects of offspring and relatives.

Methods

Study species and population

We studied rhesus macaques on Cayo Santiago, a 15.2 ha island off the coast of Puerto Rico. During our study period, Cayo Santiago was inhabited by approximately 800 rhesus macaques (see details in Rawlins & Kessler 1986). Rhesus macaques live in multi-male, multi-female groups characterized by female philopatry (Gouzoules & Gouzoules 1987) and male dispersal (Lindburg 1969; Colvin 1983). They breed on a seasonal basis (Drickamer 1974), with inter-birth intervals of approximately one year (Rawlins & Kessler 1986). During our study period, the mating season on Cayo Santiago lasted from May to October, followed by a birth season ranging from November to April (but see Hoffman et al. 2008 for shift of onset of mating season due to climatic changes). Our study focused on one social troop (group R) where we could visually recognize all individuals. During our study the group size ranged between 173 and 187 animals at any given time, adding up to a total of 191 different animals observed during the entire study period. During our study period group R contained 56 adult females and 29 to 41 adult males (these changes in numbers reflect male transfers between groups). The present study is restricted to interventions performed by adult males (at least 4 years of age). We included all adult males present for a minimum of 4 months during our study period based on the official census of the Caribbean Primate Research Center and born outside of our study group (hereafter: non-natal) leading to a total of 44 males used in our analyses (see Table S1, Supporting information).

Behavioral definitions

A 'dyadic conflict' is an interaction between two opponents, with an 'aggressor' directing an agonistic interaction towards a 'victim'. Agonistic interactions include aggressive behaviors (physical or non-physical) mostly followed by a submissive response. An 'intervention' was defined as the interference by a third animal in an ongoing dyadic conflict, therefore resulting in a triadic interaction. Consequently, the prerequisite of a potential intervention is the occurrence of a dyadic conflict. 'Coalition formation' was defined as the actual support of one opponent after intervention in a dyadic conflict (de Waal & Harcourt 1992). A 'triadic interaction' involves one 'supporter', one 'recipient' of support and one 'target' of support.

It is also possible that more than one animal intervenes in the original dyadic conflict (polyadic interaction) which we observed in 21.8 % of all interventions given by adult males and females. Following previous studies, we split such cases into triads (Watanabe 1979; Datta 1983a; de Waal 1984; Chapais et al. 1994; Widdig et al. 2000).

Behavioral data

The data of this study are part of a larger dataset collected by AW between May and December 1997 (Widdig et al. 2001, 2002, 2006a; Widdig 2002). Here we used data of focal animal sampling of 58 females (range 16.3–19.6 hrs. per focal) and all occurrence sampling recording grooming, mating, dyadic aggression and coalitions including all group members (nearly 1000 hrs.) (Altmann 1974; Martin & Bateson 1986). Comparing the frequency of occurrence of support, aggression and grooming in focal and all occurrence sampling revealed a clear correlation across subjects (Kendall's tau, support: r_T=0.82, p=0.002; aggression: r_T=0.435, p=0.001; grooming: r_T=0.430, p=0.001; N=58 focal female subjects). We therefore combined both datasets to increase the number of events observed per adult individual. Note, that data on male intervention and support were based on all occurrence sampling conducted on all group members. Coalitions are relatively long lasting and often noisy events (Altmann 1974). Because of the conspicuous nature of aggressive interactions, it is unlikely that we collected data on coalitions with a systematic bias. For each intervention in an ongoing dyadic conflict, we collected the following information whenever possible: (i) the date and the location of the event, (ii) the identity of the participants, (iii) the role of each participant in the interaction (aggressor and victim for the original dyadic conflict; supporter, receiver and target of support for the intervention), (iv) the kind of aggression observed in the original dyadic conflict.

Determination of paternity

Most of the genotypic data were available from previous studies (see Widdig et al. 2001, 2002, 2004, 2006a,b) and are part of the genetic data base of the Cayo Santiago population started in 1992. Briefly, nearly the entire population was systematically sampled for animals a) born between 1992 and 2000 (note that cohort 1999 had lower sampling success due to a hurricane) or b) born before 1992 if they survived until systematic sampling began in 1992. Newborns were sampled in the consecutive annual trapping season (Jan to Mar) if they survived their first year, which applied on average to 69.12% of the babies born between 1992 and 2010 (A. Widdig, unpublished data). Samples taken for this study were exclusively blood samples.

The data base analyzed consisted of 2290 animals typed at 14.62±2.44 loci on average (±SD) out of a panel of 21 STR markers (see Dubuc et al. 2011 and references therein). Efforts to fill in missing genotypes continue to this date and largely depend upon sample availability. The mean number of alleles per locus was 7.38±2.87, the mean observed heterozygosity across loci was 0.75±0.08, the mean expected heterozygosity was 0.74±0.07, and the mean polymorphic information content was 0.69±0.8 (all calculations performed with CERVUS 3.0; Kalinowski et al. 2007). There was no evidence of a null allele occurring at these loci and all but one locus were in Hardy-Weinberg equilibrium (HWE). Locus D20S206 deviation from HWE could be due to chance, mutation or typing errors. However, while the overall typing error rate derived from mother-offspring mismatches was 11% for the entire data set, this value decreased to 3% when considering only the group R individuals included in the analysis because of the increased effort in completing their genotypes.

Maternity derived from long-term field observations was first confirmed for 93.4% mother-infant pairs in this study using genotypic data and this information was subsequently used in paternity analyses. All sampled males older than 1250 days (based on earliest age at reproduction; Bercovitch et al. 2003) and present on the island at least 200 days before the actual birth of a given infant (mean days ±SD of gestation length of 166.5±7.4; Silk et al. 1993) were considered as potential sires for that infant. We included all genotyped males of the population who fulfilled these criteria in the paternity analyses in order to account for extra group paternity (Widdig et al. 2004). CERVUS 3.0 (Kalinowski et al. 2007) simulation settings for paternity analysis were: 10000 offspring, 215 candidate sires, 57% sampled candidate sires, 70% complete genotypes and 3% typing error, where the last four values were estimated given the subset of animals anlysed.

Our analysis included only those cases in which a given mother-father-offspring trio were genotyped on at least 12 common loci or when lacking a sample or genotypes of mothers were restricted, father-offspring duos had to be genotyped on at least 15 common loci. Paternity was determined for 142 of the 191 animals considered as group members during our study (74.3%) using a combination of exclusion and likelihood analyses as follows. In 129 cases, all males were excluded at a minimum of two loci, with the exception of the assigned sire, who matched the offspring-mother pair at all loci. Note that in two of these cases the mother's genotype was lacking. In seven cases, all males were excluded at one locus, with the exception of the assigned sire, who matched the offspring-mother pair at all loci, while four cases lacking the mother's genotype. To determine the current seasonal siring success per male we also assigned paternity of all 36 babies conceived during and born after the observational period was completed. For 33 babies all potential sires were excluded at two or more loci and, in three cases, all potential sires were excluded at one locus, while the assigned sire matched the offspring-mother pair at all loci.

Paternity assignments with exclusions at only one locus as well as the one case with one father-offspring mismatch were all supported at the 95% confidence level in favor of the male with the highest LOD score calculated by CERVUS 3.0 (Kalinowski et al. 2007).

Except for six animals, the unresolved cases of paternity involved animals born before 1992, the year when systematic sampling began. For these animals we could not resolve paternity either because of the reduced analytical power when lacking the mother's genotype and/or because all sampled potential sires were excluded by at least two confirmed mismatches, suggesting that the actual father was not sampled. For animals with unresolved paternities we were nevertheless able to exclude all 44 male subjects as potential fathers or paternal siblings, either because they mismatched these individuals at two or more loci or because they were too young to be a potential sire.

Determining kin relationship

We determined the number of close kin present for each study male. We defined close kin as (a) father-offspring dyad (degree of relatedness, r=0.5) or (b) half-sibling (r=0.25) considering a focal male as a maternal or paternal half-sibling of an adult group member present during our study. Maternal kinship and group membership were available from the long-term demographic data-base provided by the Caribbean Primate Research Center and paternal kinship (i.e., father and paternal half-siblings) was determined via pedigrees based on genetically determined paternities.

Variables used

(i) Age, sex, co-residency and tenure

Rhesus macaques can be assigned to non-overlapping birth cohorts even though infants from the same cohort may differ in age by up to six months. The dates of birth and sex of all subjects were extracted from the demographic data base provided by the Caribbean Primate Research Center (CPRC). In addition, we defined co-residency because the time spent in the same group is likely to increase the probability of social interaction of a given dyad. Co-residency was calculated as the total number of days both members of the dyad were present in the same group on Cayo. For the statistical analyses we standardized all dyadic co-residency measures, separately per potential supporter, to a range from zero (minimum co-residency) to one (maximum co-residency), in order to remove the correlation between co-residency and tenure (see below). Finally, we included male tenure to control for male presence in the study group calculated as the total number of days an individual was present in the study group at the date a given agonistic interaction. For two analyses we computed mean tenures over all conflicts per dyad.

(ii) Kin present

The available paternity data of the study group were used to identify offspring of any age sired by the 44 male subjects to evaluate whether males intervene in favor of their own offspring. We detected five males with offspring present, thereby resulting in eight father-offspring dyads (three sons, five daughters). Furthermore, we identified 14 males with adult brothers present, resulting in 12 dyads of maternal brothers and eight dyads of paternal brothers. We also detected three males with adult sisters present resulting in one maternal and three paternal sibling dyads. This amounted to a total of 29 close kin dyads out of a total of 4950 dyads (0.56%).

(iii) Current seasonal siring success

To assess whether coalition formation impacts the current seasonal siring success, we determined the number of offspring conceived during and born after our observation period (hereafter: ‘current seasonal siring success). Of the 36 newborns conceived during our study 31 were sired by 14 of the 44 study males (Table S1, Supporting information).

(iv) Dominance rank

To establish a dominance hierarchy, we extracted all dyadic agonistic interactions from focal and all occurrence sampling over the entire study period to determine the winner and loser for each interaction. Individuals won an agonistic interaction when their opponent gave submissive gestures after receiving an aggressive gesture. With the determined wins and losses we constructed a square matrix of interactions in which wins by the lower-ranking animal (entries below the diagonal) were few or zero.

(v) Composite sociality index (CSI)

Following previous studies (Sapolsky et al. 1997; Silk et al. 2003, 2006), we combined the frequencies of three social behaviors (friendly approaches, grooming and sharing of limited resources, such as food and water) to compute a composite sociality index (CSI) for each dyad present during the observational period. We calculated the index as an average measure over all these behaviors taken as the deviation of the dyad from the median of all adults' dyads over the entire study period:

$$CSI=\frac{{\displaystyle {\sum }_{i=1}^{\mathrm{a}}\frac{behaviouri}{Mediani}}}{3}$$

High CSI values indicate dyads which have more socio positive interactions than the median of all dyads, and low CSI values indicate dyads which have fewer such interactions than the median of all dyads.

(vi) Female receptive state

To investigate whether male support varied with female receptive state we calculated the time window of the likely conception per female by counting 166.5 ± 7.4 days back from the date her offspring was born (average and standard deviation of gestation days based on Silk et al. 1993). We could therefore distinguish conflicts occurring prior to likely conception (>174 days prior to birth of the offspring), during likely conception (159 to 174 days before birth) or after the likely conception of a given female (<159 days before birth). Since this was our only way to estimate time of conception we could calculate this solely for females who actually gave birth that season (N=39), as information about miscarriages was lacking.

Data analysis and statistical tests

For the purpose of the present study, we extracted all dyadic conflicts observed during the observational period, as well as all coalitions in which the 44 adult males intervened.

Model 1: Comparing dyadic conflicts with and without male intervention

In order to understand what drives males to intervene in an on-going dyadic conflict, we used a Generalized Linear Mixed Model (GLMM; Baayen 2008) with binomial error structure and logit link function. The data analyzed comprised all combinations of a single dyadic conflict with all potentially supporting males based on their presence, indicating whether the respective male acted as a supporter in the dyadic conflict or not. The dataset contained 3811 dyadic conflicts (resulting in 125,784 data points considering all present males as a potential supporter per dyadic conflict). In the GLMM we included the following variables for each supporter: age, rank and tenure at the day of the conflict. We used the following variables for describing the dyadic conflicts: whether kin of the potential supporter was involved, whether a female was involved; whether a female who was presumably sexually receptive was involved; the maximum of the rank of the two opponents involved; the maximum of the ages of the two opponents; the maximum co-residency of the two opponents and the maximum sociality index. We chose these maximum values as a putative measure of the overall importance of the conflict for the potential supporter. In addition, we included the number of females likely to be sexually receptive at the date of the conflict, and the location where the conflict occurred distinguishing locations where food competition was low vs. high.

We considered all these predictor variables as fixed effects, being covariates (i.e., continuous predictor variables) except for location. We checked all covariates for their distribution. Based on this we decided to square root transform tenure to achieve an approximately symmetrical distribution. In the next step we standardized all covariates (to a mean of 0 and a standard deviation of 1). In addition, we included identity of the potential supporter as well as the ID of the dyadic conflict as random effects into the model. Initially, we also included the identity of the aggressor and the victim of the conflict into the model, but we dropped them subsequently since they did not appear to explain any variance (likelihood ratio tests: χ²=0, df=2, p=1).

The data analyzed were likely to show temporal autocorrelation (i.e., residuals derived for the same supporter for conflicts observed closer to one another in time being more similar than residuals of conflicts more distant in time). Such autocorrelation leads to non-independent residuals and devalues the validity of the statistical model. To avoid this, we included a term explicitly accounting for autocorrelation into the model. We obtained this term as follows: first, we ran a model as described above and derived the residuals from it. For each data point, we then calculated the weighted average of the residuals of all other data points, with the weight equaling 1/(time lag to the other data points + 1). Only residuals of data points of the same potential supporter were considered. The time lag was measured in days plus fractions of a day for hours and minutes. We then included the result as an additional fixed effect in the final model ('autocorrelation-term'). The estimated coefficient and the significance (P-value) of this autocorrelation term will not be interpreted, since its sole aim was to control for temporal autocorrelation.

We fitted mixed models using the function 'lmer' provided by the package ’lme4' (Bates et al. 2008) for R 2.8.1 (R Development Core Team 2010). We tested for significance of covariates using z- and corresponding P-values provided by the function lmer. The influence of the random effects was tested with a likelihood ratio test (Dobson 2002) testing the full against the corresponding reduced model. In order to reveal reliable likelihood ratio tests we fitted the models using Maximum Likelihood (rather than Restricted Maximum Likelihood; Bolker et al. 2009). The autocorrelation term was generally that derived from the full model and included in all reduced models.

Model 2: Comparing recipient and target of male support including male and female participants

To investigate the question of who received male support, we reduced the original dataset to those dyadic conflicts in which an intervention by an adult male was actually observed. In the model we included as fixed effects rank, age, sex and role in the conflict (aggressor or victim) for both opponents of the dyadic conflicts, as well as the kin relationship, co-residency and sociality index of each opponent with the supporter. Based on our expectations taken from published data, we included several interactions between certain variables as fixed effects: the three-way interactions between sex of one opponent and sex of other opponent, on the one hand, and their roles, ranks, ages, kin and co-residency with the supporter, on the other hand. These three-way interactions account for the possibility that the preference to support, e.g., females may be mediated by the opponent's sex and, furthermore, vary with other properties of the two opponents (e.g., their roles in the conflict or their kin relationship with the potential supporter). We also included the two-way interactions (i) between age and co-residency and (ii) those between kin, on one hand, and co-residency, rank, role, and age; on the other hand because we assumed that the effects of age and kin-ship could overwrite the effects of the other factors. Furthermore, we included all two-way interactions comprised by the three way interactions and all main effects comprised by all interactions into the model. Finally, we included the identity of both opponents as random effects into the model (the identity of the supporter we did not include into the model, because only interactions with support were included into the data and, hence, all supporters had the same probability of intervening, by definition).

The dataset included in the model contained a total of 212 dyadic conflicts in which a male intervened. We standardized rank, age, co-residency and sociality index (to a mean of zero and a SD of one). For each intervention we included data for both the subject receiving (recipient) and the subject not receiving the support (target). Hence, the dataset had a 'repeated measures' structure with two corresponding entries per support event, and consequently, there was invariably one individual receiving and one not receiving support in a given conflict and the probability of support was identical for all conflicts. To nevertheless control for the non-independence of data we used a repeated random selection out of all events. To test significance we ran 1000 selections, each containing one randomly chosen data point per event. For each selection we used a GLMM (function "lmer"; see above) with binomial error structure and logit link function to determine the coefficients for fixed effects. Finally, we calculated the mean of the results for each coefficient (estimate; SE; z; P) as the result of the model. Furthermore, for each selection we tested the significance of the full model against the null model (without any fixed effects but including the random effects) using a likelihood ratio test (see model 1). In a last step we calculated the mean χ² and the mean P-value as the result of the likelihood ratio test for the model.

Model 3: Comparing recipient and target of male support in male-male conflicts

We ran a restricted model to test male support as a function of rank of the opponents and the potential supporter considering only conflicts among adult males. We used the same dataset and procedure as for the previous analysis, but included only male-male dyadic conflicts (N=35). Additionally, we incorporated the interactions between rank of the supporter, on the one hand, and ranks of the opponents, on the other. Note that, since we only considered coalitions with all participants being males, sex of opponents was removed from the model. Again, all continuous variables were transformed to a mean of zero and a SD of one and testing was done using the same random selection approach as in the prior analysis.

Model 4: Impact of male support towards females on current seasonal siring success

To understand whether or not male support had an influence on the probability of males siring an offspring in the ongoing mating season we prepared a dataset which contained each dyad comprising a present male and a female who gave birth to a surviving offspring in the subsequent birth season (N=36). For each male-female dyad, we calculated a) the probability of male support (as the proportion of conflicts a female had and into which the male intervened), for the period before, during and after the estimated receptive state of the female and b) the mean co-residency of this dyad. In addition, we included rank and age for each male and female, the sociality index of the dyad and mean male tenure across all support events. The final dataset comprised 1150 male-female dyads.

Based on the distribution of male tenure and female age we decided to square root transform these variables to achieve approximately symmetrical distributions. Finally, we standardized all variables to a mean of zero and a SD of one. We used a GLMM (function "lmer"; see model 1) with binomial error structure and logit link function. We also included the identity of the male and female as random effects into this model. The response variable was whether or not a given male sired a given female's offspring. To account for the varying numbers of days a given male-female dyad were together in the same group during the female's receptive period we included this duration as an offset variable in the model.

Model 5: Impact of the female's receptive state on the probability of male support

The data we used to analyze male support as a function of receptive state (before, during and after the estimated receptive state) contained all dyadic conflicts a female who gave birth to a surviving offspring in the consecutive birth season was involved in with all combinations of potentially supporting males. The estimated receptive state on the day the conflict occurred was included in the model as a fixed effect. The dataset contained 2770 dyadic conflicts resulting in 118,941 data points when including all potential male supporters. Like in previous models, we used a GLMM with binomial error structure and logit link function. In the model we included the following variables as fixed effects: male tenure per conflict, age and rank for males and females, co-residency, and sociality index for each dyad, and female receptive state. In addition, the identity of the potential supporter, the identity of the female involved in the dyadic conflict and the conflict ID were included as random effects. Initially, we included autocorrelation as described above to control for non-independent residuals (see model 1). However, since the derived term did not reveal significance (estimate=0.04217, P=0.520) we removed it from the final model. The influence of female receptive state was tested with a likelihood ratio test comparing the full model against the model which did not include the female phase but all other effects. Based on the distribution of the variables we decided to square root transform male tenure and co-residency and to log transform female age and the sociality index. Finally, we standardized all continuous variables to a mean of zero and a SD of one.

To check for the assumptions of each model we calculated the variance inflation factors (VIF; Quinn & Keough 2002). VIF for all models except one (Model 4) indicated collinearity to be no issue (largest VIF=2.18). The results revealed for Model 4 indicated that collinearity here was no problem either.

Results

During the study we observed 8589 dyadic conflicts resulting in 2505 triadic supports or, when considering only adult participants, we observed 3811 dyadic conflicts resulting in 878 triadic supports. The 44 study males were responsible for 356 out of 2505 interventions and for 212 out of 878 interventions observed among adults. The latter suggests that adult males on average intervened less often than adult females (24.1% vs 75.9% of all interventions including only adults). However, male interventions were predominantly performed by the top ten ranking males (87%) and in fact, over the study period, the ten top-ranking males intervened at similar rates to females (average frequency of interventions/animal: top-ranking males 32, females 30).

Comparing dyadic conflicts with and without male interventions (Model 1)

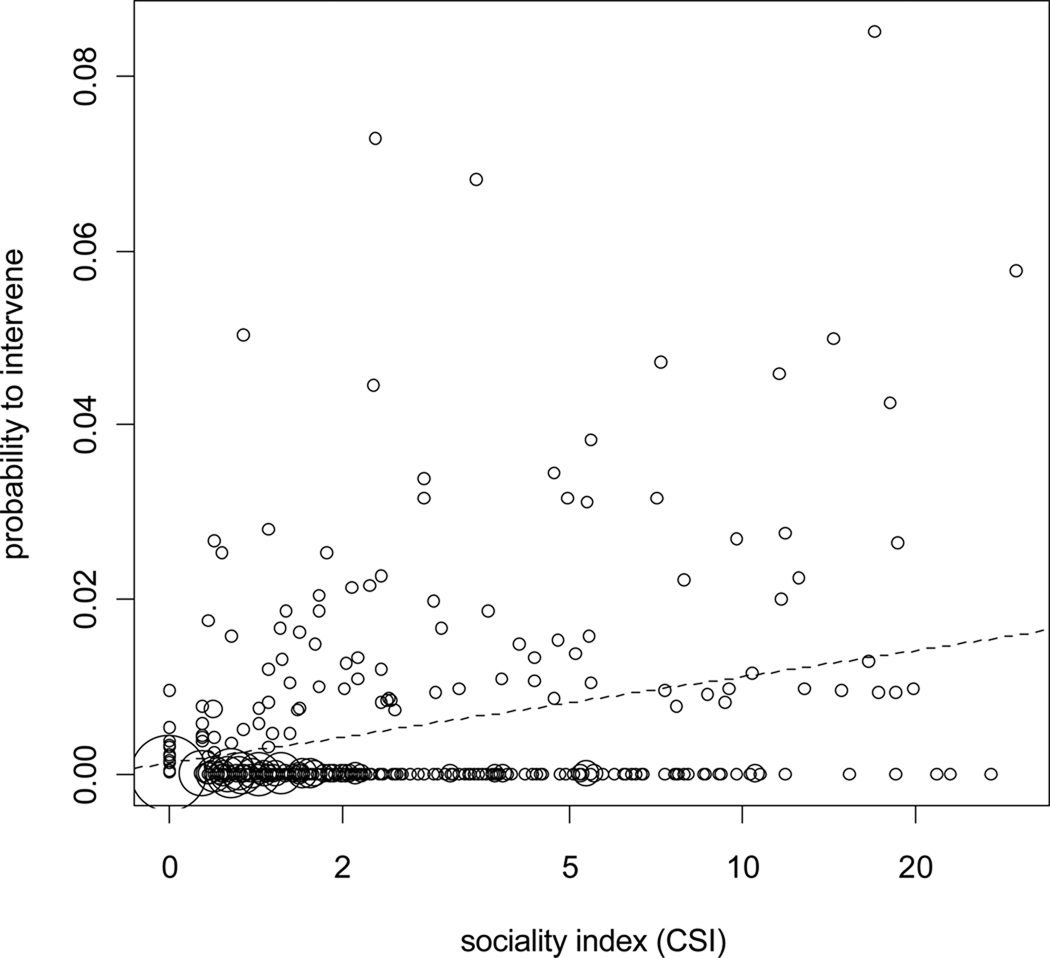
Overall, the results revealed that the set of predictor variables used had a clear influence on the probability of an intervention (likelihood ratio test comparing the fit of the full with the fit of the null model containing only the random effect and the autocorrelation term: χ²= 423.9, df=12, P<0.001). In more detail, males of higher rank were more likely to intervene (Table 1, Figure 1). In addition, the probability of a male to intervene in a conflict was higher for conflicts involving an individual sharing a high sociality index with the intervening male (Table 1, Figure 2). Furthermore, males were more likely to intervene in conflicts involving a close kin (Table 1). Our dataset included three non-natal high-ranking maternal brothers. In a control model we excluded them and the results revealed no kin effect (estimate=1.14; SE=0.97, z=1.17, P=0.24), suggesting that these brothers almost fully accounted for the kin effect. The raw data confirmed this clearly. Out of the 16 conflicts involving kin of the intervening male, the three brothers were involved in 14 of these conflicts.

Table 1.

Predictors of male interventions (Model 1). Note that the probability for a male to intervene in a dyadic conflict increased with its rank, with a higher sociality index with one of the two opponents and when a close kin was involved (but see text). Significant effects are marked in bold. Predictor variables with 'max' refer to the maximum of the respective variable among the two opponents.

Predictor variable	Estimate	SE	z	P
Intercept	−11.44	1.11
Age supporter	−0.10	0.13	−0.76	0.447
Rank supporter	1.93	0.24	7.91	<0.001
Male tenure at day of conflict	−0.01	0.11	−0.05	0.960
Kin involved (no=0; yes=1)	1.03	0.40	2.59	0.010
Female involved (no=0; yes=1)	−1.69	1.15	−1.48	0.139
Rank max	0.17	0.47	0.36	0.720
Age max	0.12	0.43	0.29	0.772
Co-residency max	−0.06	0.10	−0.58	0.560
Sociality index max	0.36	0.04	8.13	<0.001
N estrous females	−0.19	0.42	−0.45	0.656
Estrous female inv. (no=0; yes=1)	0.32	1.57	0.20	0.839
Location	−0.17	1.04	−0.17	0.868
autocorrelation-term	0.24	0.04	5.32	<0.001

Figure 1.

Impact of male rank on the probability of giving support in a conflict. Males with higher rank showed a higher probability of providing support. N indicates the number of possibilities to intervene in an on-going conflict within a rank category.

Figure 2.

Relationship between probability of male intervention and the higher sociality index of the two individuals involved in a conflict. Note, that a high sociality index between one opponent and the potential intervener significantly increased the probability of intervention. The area of dots is proportionate to the respective number of dyads.

Comparing recipient and target of male support including male and female participants (Model 2)

The full model including three-way interactions, as well as the reduced model containing only two-way interactions and main effects did not converge. This non-convergence was likely to be due to the application of a complex model to rather unbalanced data. Only the model which was reduced to the set of main effects (Table 2) converged. This revealed a clear influence on who received support (likelihood ratio test comparing the fit of the full with the fit of the null model comprising only the random effects: χ²= 95.5, df=8, P<0.001). Specifically, males were more likely to support the older of the two individuals involved in a dyadic conflict (Table 2). Furthermore, males more frequently supported individuals with whom they shared a higher sociality index (Figure 3). In addition, males who intervened gave more support to females than to males, and, hence, targeted males more often than females. Finally, the aggressor of the dyadic conflict received more support than the victim. In addition, Figure 4 suggests interactions between the three predictors (role and sexes of the two opponents) in addition to those revealed by the model. Specifically, it suggests that in conflicts involving both sexes, males biased their support towards the female rather than to the male opponent and that this difference was far more pronounced for conflicts with a female acting as aggressor than for conflicts with females acting as victims. In conflicts involving only females there was apparently no relationship between initiation of the dyadic conflict and the probability of receiving support, but in conflicts involving only males it was almost exclusively the aggressor who received support.

Table 2.

Predictors of male support (Model 2). Note that the probability of a subject to receive support increased with its age and the sociality index with the supporter. Furthermore, females received more support than males and males were more frequently targeted than females. The aggressor-role in in the dyadic conflict also increased the probability of getting support. Significant effects are marked in bold.

Predictor variable	Estimate	SE	z	P
Intercept	1.46	0.38
Age recipient	0.60	0.24	2.44	0.044
Rank recipient	−0.04	0.22	−0.21	0.544
Sociality index	1.62	0.43	3.71	0.001
Sex recipient (F=0, M=1)	−1.69	0.51	−3.34	0.005
Sex target (F=0, M=1)	1.78	0.44	4.09	<0.001
Recipient is kin (no=0, yes=1)	0.24	26.83	0.18	0.695
Co-residency	0.11	0.22	0.49	0.526
Role (aggressor=0, victim=1)	−2.29	0.45	−5.08	<0.001

Figure 3.

Relationship between probability of male support and the sociality index. The opponent with the higher sociality index towards to supporter had a significantly higher probability to receive support. The graph shows that most individuals sharing a low sociality index with the potential supporter (left side) had a probability of support below 50%. Sociality index values were binned and values at the x-axis show the midpoints of the respective bins.

Figure 4.

Impact of sex and role (aggressor or victim) of opponents in dyadic conflicts on their probability to receive male support. Males preferably supported females as compared to males and aggressors as compared to victims. However, the female preference seemed to be more pronounced when the female was an aggressor in the original conflict.

Comparing recipient and target of male support in male-male conflicts (Model 3)

In our limited dataset, we observed 35 events of males intervening in the 198 observed male-male conflicts (17.7%), most probably causing the model not to converge. The raw data however, revealed that adult males almost never targeted males ranking higher than themselves. In 29 coalitions (83%) both supporter and receiver of support were higher in rank than the target (conservative or all-down; cf. van Schaik et al. 2006). In five cases (14.3%) the rank of the target was between the supporter and the receiver rank (bridging coalitions). We observed only one case of a revolutionary coalition in which the supporter and the receiver of the support were both lower in rank than the target (all-up; cf. van Schaik et al. 2006). We observed no bridging or revolutionary coalitions among male kin.

Impact of male support towards females on current seasonal siring success (Model 4)

The set of predictor variables had a clear influence on reproductive success (likelihood ratio test comparing the fit of the full with the fit of the null model comprising only the random effects and the offset term: χ²=34.7, df=8, P<0.001). However, the probability of support before and during the receptive state had no significant influence on the current seasonal siring success (Table 3) even when we re-ran the model without the two top-ranking males, who did not reproduce (see Table 1). Interestingly, the sociality index as well as male tenure, rank and co-residency appeared to predict future reproductive success of males, i.e., a high sociality index of a given male-female dyad resulted in a higher probability of paternity.

Table 3.

Impact of male support towards females on current seasonal siring success including the periods before and during the receptive state of a given female (Model 4). The probability of support in favor of females had no impact on the reproductive success of males, but surprisingly a high sociality index of a given male-female dyad resulted in a higher probability of paternity

Predictor variable	Estimate	SE	z	P
Intercept	−7.69	0.48
Probability of support	0.14	0.09	1.58	0.114
Mean co-residency	1.09	0.52	2.12	0.034
Mean tenure	−1.46	0.58	−2.54	0.011
Male rank	1.52	0.52	2.92	0.004
Male age	−0.47	0.38	−1.24	0.214
Female rank	−0.18	0.23	−0.78	0.437
Female age	0.11	0.23	0.46	0.647
Sociality index	0.40	0.10	3.95	<0.001

Impact of the female’s receptive state on the probability of male support (Model 5)

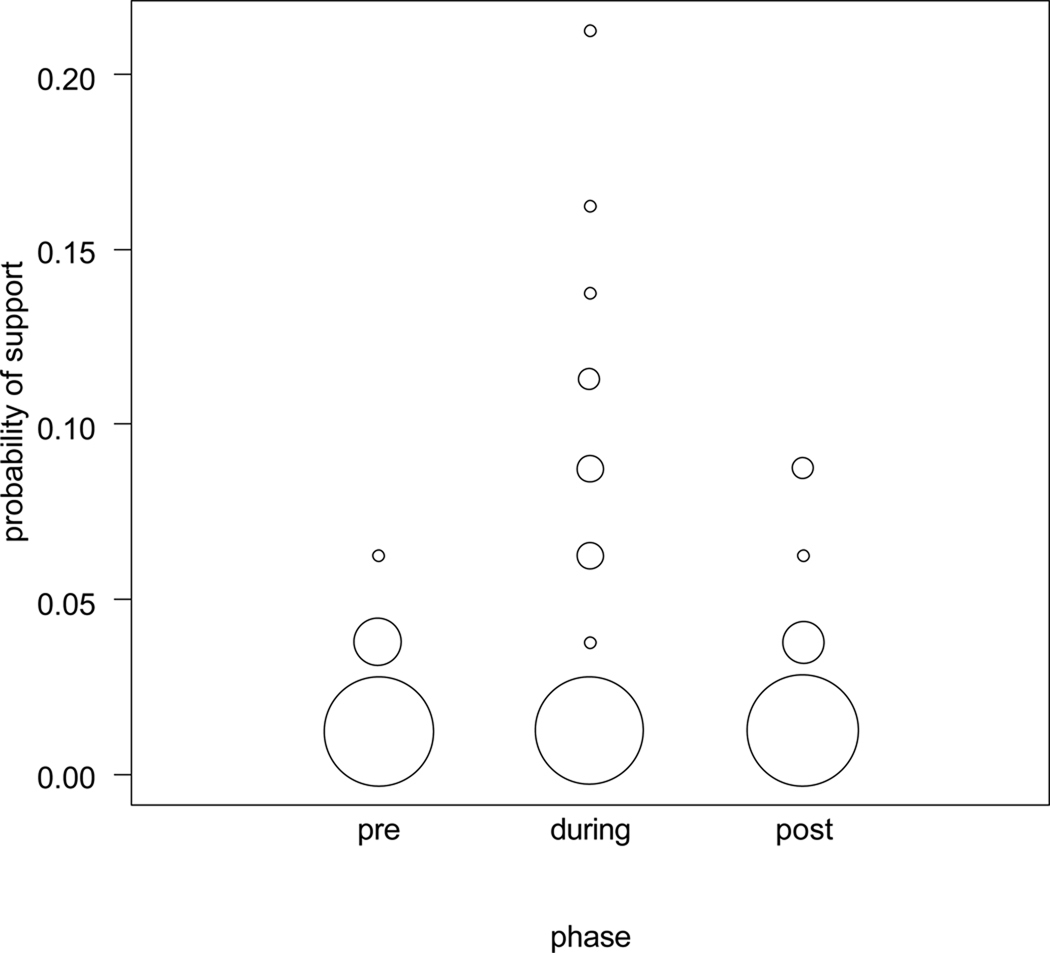
The likelihood ratio test of the full against the model reduced by the variable female receptive state revealed no obvious impact of the female receptive state on the probability of male support (χ²=4.3, df=2, P=0.119). However, Figure 5 suggests a weak tendency for increased male support during the estimated receptive state.

Figure 5.

Probability of male support in favor of females in different stages of their conceptive cycle. The figure suggests that males supported females during the period of likely conception more often than before or after likely conception. However, this result did not reveal statistical significance. The area of the circles corresponds to the respective number of dyads.

Discussion

The results of our study confirm previous findings that in adult rhesus macaques males are on average less frequently involved in coalition formation than females (Kaplan 1978; Bernstein & Ehardt 1985). Yet our results clearly show that the ten top-ranking males provide support at a similar rate to adult females. The rare interventions in dyadic conflicts are therefore mainly due to the lack of coalitions involving mid- and low-ranking males (Model 1).

One of our major findings is that there was no obvious link between male support and fitness in male rhesus macaques. Several lines of evidence strengthen this statement. Firstly, there was no association between support provided and current seasonal siring success (Model 4). Patterns of interventions in male primates seem to differ across species as a function of how males attain dominance, with dominance being assumed to translate into higher paternity success (Alberts et al. 2003). Male Savannah baboons, at least in their prime age, mainly enter the hierarchy at the top and produce a higher number of offspring than low-ranking males (when dominance is maintained for a sufficiently long period; Alberts et al. 2006) because they are able to monopolize the majority of matings by mate guarding females during their most likely conception days (Alberts et al. 2003). In such species, high-ranking males rarely form coalitions (Noë & Sluijter 1995) probably because they do not need them. Low- and mid-ranking males, however, gain the majority of their consortships by forming coalitions to aggressively taking over a consorted female (Bercovitch 1988; Noë & Sluijter 1995). In contrast, male rhesus macaques mainly enter the hierarchy at the bottom, queuing for dominance rather than fighting (Berard 1999). A recent study found that mating is shared among several males and that the alpha male did not have the highest mating access, leading to a relatively low mating skew in relation to male dominance rank (Dubuc et al. 2011). Furthermore, several paternity studies conducted on rhesus macaques reported an intermediate skew in male reproduction (Berard et al. 1993; Bercovitch & Nürnberg 1997; Widdig et al. 2004) with a relatively low reproductive skew in relation to male dominance (Widdig et al. 2002; Widdig unpublished data; this study; cf. Dubuc et al. 2011). Due to the queuing system in rhesus macaques, the alpha males are probably not the strongest males, and given the time it takes to reach dominance, they are not the most attractive males (Berard 1999; but see also Dubuc et al. 2011). In summary, in queuing systems dominance should probably be of lower importance with respect to male reproductive success.

Furthermore, our data support previous findings that male rhesus macaques form coalitions to maintain their dominance, but do not form rank-changing coalitions (Model 3) (Kaplan 1977; Bernstein & Ehardt 1985). Our results are in contrast to the theoretical model developed by van Schaik et al. (2004, 2006), which predicts that in species with intermediate male reproductive skew, mid- and low-ranking males should form all-up rank-changing coalitions. Our data therefore suggest that the contest level is not a strong predictor of this coalition type (cf. Berghänel et al. 2010). Interestingly, observations made in our study group 12 years later (i.e., composed of a different set of males, including an unusually high number of adult natal males) first reported that middle-ranking males formed revolutionary coalitions. This resulted in a change of the dominance hierarchy and in an expel from the group of high-ranking long-term resident males (Higham & Maestripieri 2010) emphasizing that rhesus' social system seems to be more flexible than previously thought (for further discussion of social flexibility see Schradin et al. THIS ISSUE).

Secondly, although males biased their support towards females when having the choice between a male and female opponent (Model 2), males frequently supporting a given female were not more likely to fertilize this female and, hence, did not increase their current seasonal siring success (Model 4). We expected that males would be more likely to support sexually receptive females in order to increase mating access, but our data revealed no significant evidence (Model 5). However, Figure 5 suggests that males support females more often during the period of likely conception. Note, our conservative estimate of a female’s reproductive state, being much larger than the actual period of conception, does not account for the fact that it can take a female more than one cycle to conceive and that females can have post-conceptive receptive periods too (Dubuc pers. communication). Therefore we cannot completely reject that males bias their support toward receptive females. Interestingly, male baboons have been shown to support lactating female ‘friends’ more often than other lactating females (Moscovice et al. 2010). In their study, Moscovice et al. (2010) showed that males monopolizing the largest proportion of a females’ total consort time were the father of the offspring and, if the father was present after parturition, he was very likely to become the ‘friend’ of the mother. However, this study did not test support in favor of females around the conception time.

Thirdly, males might increase their direct fitness by producing many offspring; yet, the survival of the offspring also critically impacts male fitness. In our study, we found no evidence that male rhesus macaques provide preferential support towards their offspring. Males potentially had the opportunity to support offspring of different ages (range: 0–12 years, mean 1.12 years) in 2371 out of all 8589 dyadic conflicts observed during the study period (9.2%), but we recorded only two interventions in favor of offspring by our study males. The lack of offspring support might suggest that male rhesus macaques can less accurately assess paternity probability and/or are unable to recognize their offspring directly using phenotype matching (Widdig 2007). However, further studies are needed to test this hypothesis. In contrast, previous studies on baboons reported that males bias their interventions towards genetic offspring in comparison to unrelated juveniles (Buchan et al. 2003). Male support of offspring might be more essential in baboons. Two studies support this hypothesis: First, mothers and infants have been shown to benefit significantly from male ‘friends’ (not necessarily the infants’ father) providing protection against harassment by others (Nguyen et al. 2009). Secondly, the presence of the father during the immature period was shown to accelerate maturation and, hence, offspring fitness in the same species (Charpentier et al. 2008). Furthermore, male baboons provided support even to unrelated infants of their female ‘friends’ (Nguyen et al. 2009), suggesting that males may invest preferentially in all infants they are likely to have sired, as the costs of care given to nonkin are low compared to the costs when refusing to aid kin (Moscovice et al. 2009).

A final line of evidence suggests that there is also no link between male support and indirect fitness. Our genetic data support previous studies on male-dispersing primate species with a lower average dyadic relatedness among adult males than among adult females within groups (Altmann et al. 1996; de Ruiter & Geffen 1998). However, this does not mean that males do not reside with relatives after migration, as groups of dispersing males can potentially be closely related (de Ruiter & Geffen 1998). Our genetic data also showed that non-natal males in our study group have some close kin available (avg. 0.73 per individual with a range of 0 to 3). Nevertheless, data suggest that males do not bias their actual support towards close kin (i.e., maternal and paternal half-siblings), despite the fact that we found significant kin bias with regard to male intervention. In other words, males intervened in conflicts involving kin, but they did not support their kin, thereby suggesting kin competition (see also Kappeler & Fichte THIS ISSUE). In addition, our data did not confirm recent theoretical models predicting a high occurrence of bridging coalitions among related males (van Schaik et al. 2006). In coalitions involving only male participants, we observed only 5 bridging coalitions (14.3%), and none of them involved related males.

Another major finding of our study is that the most important variable for the probability of male support was sociality (Models 1, 2, and 4) as opponents sharing a higher sociality index with a given male were more likely to be supported by this specific male (Model 2). Moreover, a high sociality index of a given male-female dyad resulted in a higher probability of paternity (Model 4). Recent studies on primate sociality, including humans, found that individuals who form strong social bonds with one another survive longer (Holt-Lunstad et al. 2010; Silk et al. 2010) and offspring of highly social females enjoy higher survival rates (Silk et al. 2003, 2009). These strong social bonds are preferentially formed among related individuals of the philopatric sex, yet they can also evolve among unrelated individuals of the dispersing sex (female chimpanzees: Langergraber et al. 2009; Lehmann & Boesch 2009; male macaques: Schülke et al. 2010). Male macaques with stronger social bonds form more coalitions with each other (Silk 1994; Schülke et al. 2010) and enjoy future dominance (Schülke et al. 2010). The later study also demonstrated a direct link between male sociality and fitness. To summarize, our results strengthen the existing evidence that high sociality positively affects fitness. However, in extension to previous studies looking at bonds among the same sex (female-female: Silk et al. 2010; male-male: Schülke et al. 2010), we here show for the first time that strong female-male bonds have a significant impact on male current seasonal siring success. Additionally, our data suggest that strong male-male or male-female bonds significantly increase the probability of receiving support by male rhesus macaques. Future studies should pay more attention to the proposed association between coalition formation, sociality and fitness in order to increase our understanding of the evolution of social behavior in dispersing male primates.