Pharmacological curve fitting to analyze cutaneous adrenergic responses

Abstract

Although dose-response curves are commonly used to describe in vivo cutaneous α-adrenergic responses, modeling parameters and analyses methods are not consistent across studies. The goal of the present investigation was to compare three analysis methods for in vivo cutaneous vasoconstriction studies using one reference data set. Eight women (22 ± 1 yr, 24 ± 1 kg/m²) were instrumented with three cutaneous microdialysis probes for progressive norepinephrine (NE) infusions (1 × 10⁻⁸, 1 × 10⁻⁶, 1 × 10⁻⁵, 1 × 10⁻⁴, and 1 × 10⁻³ logM). NE was infused alone, co-infused with NG-monomethyl-l-arginine (l-NMMA, 10 mM) or Ketorolac tromethamine (KETO, 10 mM). For each probe, dose-response curves were generated using three commonly reported analyses methods: 1) nonlinear modeling without data manipulation, 2) nonlinear modeling with data normalization and constraints, and 3) percent change from baseline without modeling. Not all data conformed to sigmoidal dose-response curves using analysis 1, whereas all subjects' curves were modeled using analysis 2. When analyzing only curves that fit the sigmoidal model, NE + KETO induced a leftward shift in ED₅₀ compared with NE alone with analyses 1 and 2 (F test, P < 0.05) but only tended to shift the response leftward with analysis 3 (repeated-measures ANOVA, P = 0.08). Neither maximal vasoconstrictor capacity (E_max) in analysis 1 nor %change CVC change from baseline in analysis 3 were altered by blocking agents. In conclusion, although the overall detection of curve shifts and interpretation was similar between the two modeling methods of curve fitting, analysis 2 produced more sigmoidal curves.

the cutaneous circulation is frequently used as a model to study microvascular function in humans (9). The skin is an ideal vascular bed to examine mechanisms of circulatory control due to its ease of accessibility, and because the mechanisms involved in cutaneous circulatory control often parallel those of other vascular beds (1, 4, 5, 8, 10–17). Intradermal microdialysis allows for vasoactive drug delivery to a precise local area (membranes are placed 0.3–0.9 mm beneath the stratum corneum, and drug diffusion distance is 2–3 mm from the membrane) with minimal systemic drug exposure. An additional advantage of the microdialysis technique is that, after insertion trauma has subsided, multiple doses of a drug can be delivered without causing either 1) an increase in local inflammatory mediators as occurs with intradermal injections or 2) membrane potential changes that occur with current changes induced via iontophoresis. When intradermal microdialysis is coupled with laser Doppler flowmetry (LDF), measurements of skin blood flow (SkBF) can explore mechanistic questions involved in regulation of the microcirculation that may only have been available in animal models (9). The primary issue we address here is how best to generate and compare in vivo dose-response data collected from studies examining cutaneous α-adrenergic responses in humans.

Differences in the α-adrenergic control of cutaneous blood flow have been examined to determine effects of aging (19, 20, 23), bed rest, thermal stress, and sex hormone perturbations (3, 18, 21, 22, 24, 25). The most commonly used approach to determine the α-adrenergic control of SkBF is to induce cutaneous vasoconstriction with stepwise increases in perfusate concentration of the α₁- and α₂-adrenergic agonist norepinephrine (NE), although selective agonists have also recently been used (26). During these stepwise NE infusion studies, SkBF-NE dose-response curves are generated to describe the in vivo cutaneous α-adrenergic sensitivity and responsiveness of the microvasculature.

Despite the common use of dose-response modeling techniques as an accepted method to interpret cutaneous vasoconstrictor responses, a clear and consistent rationale for analyzing the data has not been established. For example, the use of data transformations, normalizations, fixed vs. variable slopes, constraints placed on the model (e.g., maximal or minimal parameters of the curves), or the goodness of fit of the model are seldom reported. Furthermore, vasoconstrictor responses are normalized either to SkBF at the initial dose of NE administered (23) or to SkBF at a pre-NE infusion baseline value (19, 21, 24), and are expressed in a number of ways: as absolute values, percent of pre-infusion values, change from pre-infusion values, or percent change from pre-infusion values (18–24). As such, different analysis methods can yield different conclusions and hamper comparisons across investigations to determine cutaneous α-adrenergic responses in health and disease (2). To address this issue, and thereby clarify methods used to examine α-adrenergic responses in the skin, we re-analyzed previously published data (21) using three commonly reported analyses techniques in the literature. Two of the analysis techniques described herein apply nonlinear (sigmoidal) modeling of the SkBF-NE dose-response relationship to obtain parameters describing potency (ED₅₀), cooperativity (the interaction between one or more agonist or modulator and the receptor, in this context Hill slope), and maximal vasoconstrictor response (E_max; which is the bottom or minimum portion of the curve in this case), whereas the third analysis compared changes in SkBF across doses of NE using a repeated-measures ANOVA. The purpose of this study is to compare data analysis methods for in vivo cutaneous vasoconstrictor studies and to present a clear rationalization for implementing each analysis approach.

METHODS

We applied three commonly used techniques to analyze the SkBF responses to graded cutaneous microdialysis infusions of NE in eight healthy women (22 ± 1 yr, 24 ± 1 kg/m²) who participated in a study examining cutaneous adrenergic influence on orthostatic tolerance (21). We used the same data set generated from recently published data (21) for all three analyses. The protocol and procedures were approved by the Human Investigation Committee at Yale University School of Medicine and conformed to the principles of the Declaration of Helsinki. All data presented are from women with normal to high orthostatic tolerance (6, 7, 21). Since sex hormones are known to alter cutaneous vasoconstrictor responses (21), the analyses in the present study were generated from data when endogenous sex hormones were suppressed with a gonadotropin-releasing hormone (GnRH) antagonist.

Protocol and Measurements

Three microdialysis probes were placed in the intradermal space of the dorsal forearm under sterile conditions using 27-gauge needles as a guide. After placement, probes were perfused with 0.9% saline at 2 μl/min for 120 min to keep probes patent and to allow for recovery from trauma or inflammation that occurs with needle insertion. Laser Doppler probes (probe 457, Perimed) housed in local heaters to control skin temperature were placed on the surface of the skin directly over each microdialysis probe to measure red cell flux, an index of SkBF. Local skin temperature was controlled at 34°C during the experimental protocol (22), with room temperature controlled at 27°C. Beat-by-beat blood pressure was measured from the middle finger on the contralateral hand (Finometer, Finipres). Following baseline measurements, each microdialysis probe received one of the following: 1) saline (0.9%), 2) nitric oxide synthase inhibitor, N^G-monomethyl-l-arginine (l-NMMA; 10 mM), or 3) nonselective cyclooxygenase inhibitor ketorolac tromethamine (KETO; 10 mM) for 45 min. Following infusions of these pharmacological inhibitors, NE was progressively infused through the cutaneous microdialysis probes at the following concentrations: 1 × 10⁻⁸, 1 × 10⁻⁶, 1 × 10⁻⁵, 1 × 10⁻⁴, and 1 × 10⁻³ M. Each dose of NE was co-infused with one of the respective pharmacological agents listed above to maintain its inhibitory effect. All NE doses were infused at a rate of 5 μl/min for 15 min, with a 5-min saline washout between each concentration.

Data Analysis

Skin blood flow and mean arterial pressure (MAP) were averaged during the final 2 min of each NE concentration where a stable plateau was established and used for analyses. Cutaneous vascular conductance (CVC) was calculated as SkBF/MAP and expressed as a percent of baseline (% CVC_BL) (19–23) to normalize the data due to the heterogeneity of cutaneous perfusion across adjacent skin sites from differences in laser Doppler probe sample area and vascular perfusion at sites. Dose-response curves of the % CVC_BL-[NE] were generated using a four-parameter nonlinear regression with either fixed or variable slope (Prism, GraphPad, San Diego, CA). Dose-response relationships were analyzed by applying the following different analyses previously reported in the literature (18–24). Due to technical difficulties associated with a probe failure and subsequent leak, SkBF responses to KETO and l-NMMA were analyzed in seven subjects.

Analysis Method 1: Nonlinear Modeling with No Data Manipulation (18, 22–24)

Infused NE concentrations were log transformed, and % CVC_BL was plotted using nonlinear regression as defined above, initially with an unconstrained or variable Hill slope. With no data manipulations, the data from three subjects were excluded from the NE and NE + l-NMMA CVC_BL-[NE] curve analysis, and two subjects were excluded from the NE + KETO CVC_BL-[NE] curve analysis. These data were excluded because the CVC_BL-[NE] responses within each of these individual curves deviated from the acceptable sigmoidal dose-response curve to an extent that the curves were unable to be modeled. Performing analysis method 1 with an unconstrained Hill slope provided parameters of potency (ED₅₀), cooperativity (Hill slope), and maximal vasoconstrictor response (E_max) in the remaining subjects. For curves with fewer data points, the Hill slope is often standardized to a fixed value of 1. Using a fixed or constrained Hill slope value of 1, infused NE concentrations were log transformed, and % CVC_BL was plotted using nonlinear regression. Data from only one subject were excluded from the NE and NE + KETO CVC_BL-[NE] curve analysis, and data from two subjects were excluded from the NE + l-NMMA CVC_BL-[NE] curve analysis since these curves could not be modeled. Analysis method 1 with a fixed Hill slope provided parameters of potency (ED₅₀) and maximal vasoconstrictor response (E_max) in the remaining subjects with no index of cooperativity as the Hill slope was standardized.

Analysis Method 2: Nonlinear Modeling, Normalized with Top and Bottom Constraints (21)

Infused NE concentrations were log transformed, and % CVC_BL was normalized within each probe with the largest value of the data set at 100% and lowest value of the data set at 0% [vasoconstriction induced by the agonist (NE in this study)], and then plotted using nonlinear regression with a variable slope. Normalizing the % CVC_BL to a maximum value of 100% (no exogenous NE or inhibitors) and the minimum value 0% (highest dose of NE) enabled the comparison of the dose-response curves on a similar scale and is useful when comparing curve position. Furthermore, the top was constrained to 100, and the bottom was constrained to zero. Thus analysis method 2 provides parameters of ED₅₀ and Hill slope, but E_max cannot be assessed because of the constraining procedure. By using analysis method 2, data from all subjects conformed to the requirements for logistic curve modeling, resulting in no data exclusion.

Analysis Method 3: CVC % Change from Baseline with No Nonlinear Modeling (19, 20)

If the primary study interest is the vasoconstrictor response at a given dose or the maximal vasoconstricting capability, data can be expressed as a percent change from baseline and then plotted over concentration. The data were analyzed using a dose-by-treatment ANOVA for repeated measures. Thus analysis method 3 does not provide parameters of ED₅₀ or Hill slope but does provide the maximal vasoconstrictor capacity (similar to the modeled E_max) without the need to satisfy logistic modeling requirements.

Analysis of the Mean Individual ED₅₀ or the ED₅₀ Generated by the Group Dose-Response Curve as a Whole

It is unclear from previously reported methods analyzing α-adrenergic % CVC_BL dose-response curves (18, 22–24) whether the ED₅₀ values reported were the averaged ED₅₀ values from each individual dose-response curve or were generated from the ED₅₀ of the overall dose-response curve generated by each group. These values can be quite different depending on the variability within the data. In the present analysis, we used analysis methods 1 and 2 to calculate both ED₅₀ terms (Prism software).

Comparisons of Dose-Response Curves

A simple approach to comparing vasoconstrictor responses is to use ANOVA (2), followed by post hoc testing when warranted (analysis method 3). Alternatively, the ED₅₀, Hill slope, and E_max of the modeled dose-response curves can be compared using an F test (Prism), which takes into account all points over the entire curves as opposed to each specific dose (2). After generating the dose-response curves using analysis methods 1 and 2, we used the F test to detect differences in the modeled parameters of the mean group curves. Individual ED₅₀ from analysis methods 1 and 2 were compared using t-tests. t-tests were also used to compare E_max from analysis method 1 and maximal vasoconstrictor capacity from analysis method 3. Data are presented as means ± SE, and parameters were considered significantly different at P < 0.05.

RESULTS

Dose-Response Curves

Analysis method 1.

When performing the analysis with an unconstrained or variable Hill slope, three subjects were excluded from the % CVC_BL-NE dose-response analysis, two subjects excluded from the % CVC_BL-NE + KETO analysis, and three subjects excluded from the % CVC_BL-NE + l-NMMA analysis. These individual subject's dose-response curves deviated from the acceptable sigmoidal dose-response curve to an extent that the curves could not be modeled using the standard sigmoidal curve procedure for nonlinear analysis. By using the remaining subjects' data, the addition of KETO significantly shifted the ED₅₀ to the left compared with NE alone when comparing dose-response curves between treatments using analysis method 1 with an unconstrained or variable Hill slope (Fig. 1A; Table 1). All other parameters of the dose-response curve (Hill slope and minimum/E_max) were similar between NE and NE + KETO curves (Table 1). Despite a separation of the curves, there were no statistical differences between NE and NE + l-NMMA curves (P > 0.40; Fig. 2A; Table 1). This was likely because of the low subject number in this analysis method, which is reflected in the wide and overlapping 95% confidence intervals for ED₅₀, Hill slope, and E_max (Table 1).

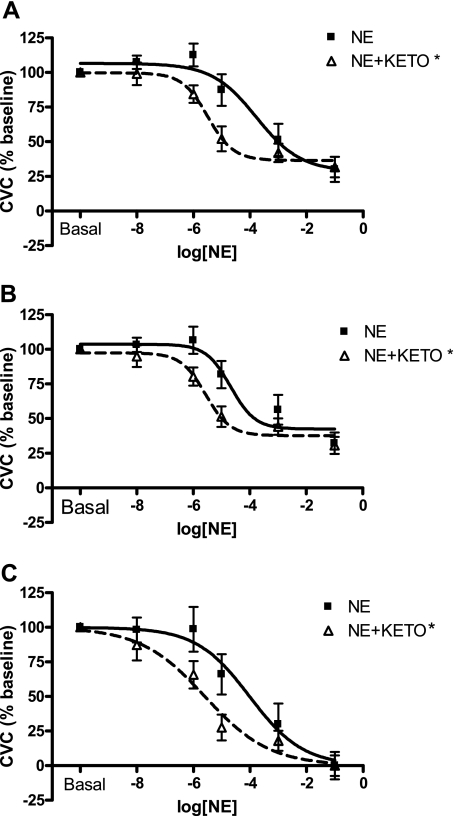
Fig. 1.

Mean norepinephrine (NE) and NE plus Ketorolac tromethamine (KETO) dose-response curves with no data manipulation (A; analysis method 1), after constraining the Hill slope (B; analysis method 1 with fixed slope), and after normalizing and constraining the top and bottom of the curve (C; analysis method 2). Ketorolac shifts the ED₅₀ to the left with all analyses (A–C), indicating enhanced cutaneous adrenergic responsiveness with prostaglandin inhibition. Goodness of fit for curve fitting was unaffected by constraining the Hill slope (slope = 1) for NE alone (r² = 0.60 and 0.63, unconstrained and constrained, respectively) or NE + KETO (r² = 0.74 and 0.76, unconstrained and constrained, respectively). Parameters of the model curves are listed in Tables 1–3. P < 0.05.

Table 1.

Analysis method 1 with variable slope

	NE (n = 5)	NE + KETO (n = 5)	NE + l-NMMA (n = 4)
Maximum, top [95% CI]	106.6 ± 6.1 [94.1 to 119.1]	99.7 ± 4.9 [89.6 to 109.8]	105.7 ± 10.2 [84.4 to 127.1]
Minimum, Emax [95% CI]	28.6 ± 11.2 [5.5 to 51.7]	36.6 ± 4.9 [26.5 to 46.6]	30.7 ± 11.5 [6.7 to 54.7]
Slope [95% CI]	−0.55 ± 0.25 [−1.07 to −0.03]	−0.96 ± 0.38 [−1.75 to −0.17]	−0.34 ± 0.20 [−0.76 to 0.09]
ED50, logM [95% CI]	−3.77 ± 0.52 [−4.84 to −2.71]	−5.49 ± 0.23* [−5.96 to −5.03]	−5.31 ± 0.62 [−6.61 to −4.02]
Mean maximum	107.6 ± 3.1	99.9 ± 3.3	105.0 ± 3.1
Mean minimum, Emax	31.7 ± 7.1	35.5 ± 5.1	24.8 ± 9.2
Mean slope	−2.72 ± 0.69	−1.04 ± 0.16	−0.78 ± 0.23
Mean ED50, logM	−4.34 ± 0.38	−5.41 ± 0.19	−4.98 ± 0.58

Data are means ± SE. NE, norepinephrine; NE + KETO, noradrenaline combined with Ketorolac tromethamine, a nonselective cyclooxygenase inhibitor; NE + l-NMMA, noradrenaline combined with N^G-monomethyl-l-arginine, a nitric oxide synthase inhibitor.

^*Significant difference compared with NE (P < 0.05).

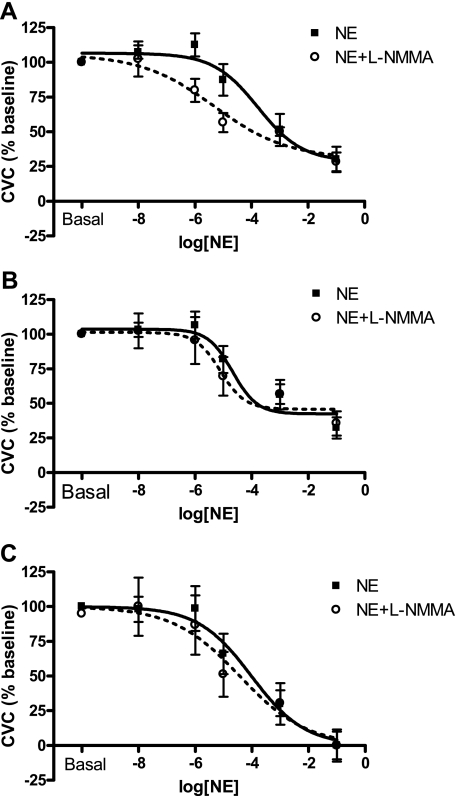
Fig. 2.

Mean NE and NE plus N^G-monomethyl-l-arginine (l-NMMA) dose-response curves with no data manipulation (A; analysis method 1), after constraining the Hill slope (B; analysis method 1 with fixed slope), and after normalizing and constraining the top and bottom of the curve (C; analysis method 2). No statistical effect of l-NMMA was detected using any analyses. Parameters of the model curves are listed in Tables 1–3.

Analysis method 1 was also performed using a fixed Hill slope (Hill slope = 1). One subject was excluded from the % CVC_BL-NE dose-response analysis, one subject was excluded from the % CVC_BL-NE + KETO analysis, and two subjects were excluded from the % CVC_BL-NE + l-NMMA analysis. By constraining the slope of the curve, the error associated with the curve was reduced as demonstrated by the more narrow 95% confidence intervals for the ED₅₀ for all curves (NE, NE + KETO, and NE + l-NMMA; Table 2), reflecting the lower variability associated with these curves. In this case, the addition of KETO significantly shifted the ED₅₀ to the left compared with NE alone (Fig. 1B; Table 2). All other parameters of the dose-response curve were similar between NE and NE + KETO curves (Table 2). Similar to the previous analysis, there were no differences between NE and NE + l-NMMA curves (P > 0.37; Fig. 2B; Table 2). Goodness of fit for curve fitting was unaffected by constraining the Hill slope (slope = 1) compared with unconstrained curves for NE alone (r² = 0.60 and 0.63, unconstrained and constrained, respectively) or NE + KETO (r² = 0.74 and 0.76, unconstrained and constrained, respectively).

Table 2.

Analysis method 1 with fixed slope

	NE (n = 7)	NE + KETO (n = 6)	NE + l-NMMA (n = 5)
Maximum, top [95% CI]	103.7 ± 4.9 [93.6 to 113.7]	97.4 ± 4.3 [88.6 to 106.1]	101.3 ± 7.3 [86.4 to 116.2]
Minimum, Emax [95% CI]	42.4 ± 6.1 [30.2 to 54.7]	37.7 ± 4.2 [29.1 to 46.2]	45.8 ± 7.7 [29.9 to 61.6]
Slope [95% CI]	−1.0	−1.0	−1.0
ED50, logM [95% CI]	−4.68 ± 0.30 [−5.28 to −4.07]	−5.57 ± 0.21* [−6.00 to −5.13]	−5.11 ± 0.38 [−5.89 to −4.32]
Mean maximum	105.2 ± 3.6	97.9 ± 3.3	105.9 ± 5.6
Mean minimum, Emax	35.7 ± 8.0	37.3 ± 4.8	41.2 ± 6.4
Mean slope	−1.0	−1.0	−1.0
Mean ED50, logM	−4.49 ± 0.38	−5.61 ± 0.15*	−4.91 ± 0.55

Data are means ± SE.

^*Significant difference compared with NE (P < 0.05).

Analysis method 2.

After normalizing and constraining both the maximal (top) and minimum (bottom) portions of the dose-response curves (max = 100, min = 0), no subjects were excluded from this analysis method. By constraining the maximal and minimal aspects of the curve, the error associated with the curve was reduced as demonstrated by the more narrow 95% confidence intervals for both the ED₅₀ and the Hill slope for all curves (NE, NE + KETO, and NE + l-NMMA; Table 3), reflecting the lower variability associated with these curves. Using this analysis method to compare the curves, the addition of KETO significantly shifted the ED₅₀ to the left compared with NE alone (Fig. 1C; Table 3). All other parameters of the dose-response curve were similar between NE and NE + KETO curves (Table 3), and there were no differences between NE and NE + l-NMMA curves (P > 0.50; Fig. 2C; Table 3).

Table 3.

Analysis method 2

	NE (n = 8)	NE + KETO (n = 7)	NE + l-NMMA (n = 7)
Maximum, top [95% CI]	100	100	100
Minimum, Emax [95% CI]	0	0	0
Slope [95% CI]	−0.46 ± 0.15 [−0.75 to −0.17]	−0.38 ± 0.09 [−0.57 to −0.19]	−0.37 ± 0.14 [−0.65 to −0.09]
ED50, logM [95% CI]	−4.02 ± 0.39 [−4.81 to −3.23]	−5.60 ± 0.26* [−6.13 to −5.07]	−4.43 ± 0.49 [−5.43 to −3.43]
Mean maximum	100	100	100
Mean minimum, Emax	0	0	0
Mean slope	−0.91 ± 0.39	−0.56 ± 0.14	−0.34 ± 0.07
Mean ED50, logM	−4.87 ± 0.44	−5.85 ± 0.28*	−5.46 ± 0.34

Data are means ± SE.

^*Significant difference compared with NE (P < 0.05).

To determine whether analysis method 2 was in fact preserving statistical power by reducing the number of subjects excluded as opposed to altering the functional parameters of the dose-response curve, we also performed analysis method 2 only on the five subjects included in analysis method 1. Consistent with prior analyses, the addition of KETO significantly shifts the ED₅₀ to the left compared with NE alone (NE, −4.04 ± 0.33 logM; NE+KETO, −5.40 ± 0.18 logM; P < 0.05) but did not alter the Hill slope (NE, −0.50 ± 0.14 logM; NE + KETO, −0.82 ± 0.29 logM; P = 0.43). Furthermore, the addition of l-NMMA did not alter the ED₅₀ (−5.05 ± 0.35 logM) or Hill slope (−0.33 ± 0.09 logM) compared with NE alone, as demonstrated in prior analyses.

Analysis method 3.

For this final analysis, CVC was calculated as a percent change from baseline and plotted across NE concentrations without generating a dose-response curve. Because no stipulations of logistic modeling needed to be met, all data were included in the analysis. Comparisons were made using a two-factor repeated-measures ANOVA (drug by dose). As expected, progressive NE infusions decreased %Δ CVC compared with baseline (Fig. 3; ANOVA, P < 0.05). There was also a decrease in %Δ CVC during NE + l-NMMA infusions (Fig. 3A; ANOVA, P < 0.05) and NE + KETO infusions (Fig. 3B; ANOVA, P < 0.05). The vasoconstrictor responses were similar between NE and NE + l-NMMA infusions (Fig. 3A), but there was a trend toward an enhanced adrenergic response with KETO (Fig. 3B; P = 0.08). Consistent with analysis method 1, there were no differences in maximal vasoconstrictor response (at 1 × 10⁻³: NE, −70.1 ± 8.1, NE + l-NMMA −62.9 ± 7.2, NE + KETO −68.7 ± 5.4%ΔCVC_BL).

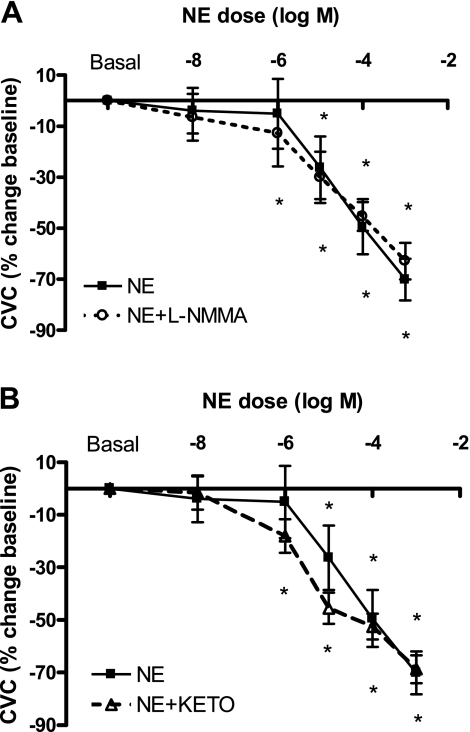
Fig. 3.

Mean skin blood flow response expressed as a percent change from baseline cutaneous vascular conductance (%ΔCVC) during infusions of NE, NE + l-NMMA, and NE + KETO. All infusions resulted in a significant decrease in %ΔCVC (*P < 0.05 compared with baseline), with no differences in maximal vasoconstrictor capacity (at 1 × 10⁻³: NE −70.1 ± 8.1, NE + l-NMMA −62.9 ± 7.2, NE + KETO −68.7 ± 5.4%ΔCVC_BL). Co-infusions of l-NMMA (A) or KETO (B) did not alter %ΔCVC compared with NE alone, although the KETO intervention resulted in a trend toward a greater adrenergic response (P = 0.08).

DISCUSSION

The goal of the current paper was to compare three commonly used analyses of vasoconstrictor dose-response curves and to present a clear rationale for implementing each analysis approach. To make valid comparisons across populations, drug manipulations, and other experimental interventions, it is important to have a consistent approach when analyzing the data. Ultimately, the choice of analysis will depend on the research question at hand. For example, if the primary research question involves discerning the differences in the cooperativity of the overall dose-response relationship, an analysis that provides ED₅₀/Hill slope is preferred (analysis method 2). Alternatively, if the primary research question is the extent of maximal vasoconstrictor capability, an analysis that provides E_max is essential (analysis method 1). Finally, if information on vasoconstriction capacity at a specific dose is of interest, the third method provides an easy to calculate method (analysis method 3).

We used CVC as percent of baseline with locally heated skin to 34°C in a 27°C room to normalize our CVC data because cutaneous perfusion is heterogeneous across adjacent skin sites due to factors such vascularity and laser-Doppler sample area. Traditionally, cutaneous vasoconstrictor studies use this type of normalization. We recognize that normalizing to baseline is not ideal because, for example, low baseline blood flow can exaggerate the relative decrease in flow to a vasoconstrictor stimulus, whereas high baseline flow could minimize such changes during a vasodilator stimulus. To avoid this problem during vasodilation studies, CVC or SkBF is often normalized to maximum flow induced by local heating or administration of sodium nitroprusside. This vasodilatory approach works less well with vasoconstrictor agonists because it is difficult to override the vasoconstriction induced by the final high NE dose to achieve maximum flow. In this study, baseline blood flow was not different within women or across drug treatment sites [data by Wenner et al. (21)].

When the primary research question is to determine the potency (ED₅₀) and/or cooperativity (Hill slope) of cutaneous α-adrenergic response, then analysis method 2 (i.e., normalizing the data and constraining parameters of dose-response curves) is a powerful approach. Analysis method 2 enabled us to model a greater portion of the data. This not only avoids the sensitive decision to exclude data but also increases statistical power. This is important because excluding subjects for reasons other than those indicated in the initial design of the study could bias results. The normalization of the upper and lower area of the curves decreases error and provides interpretable sigmoidal curves, and this can be especially important in in vivo human experiments in which there are few infused concentrations of the vasoconstrictor. Our ability to administer multiple concentrations during microdialysis studies in humans is limited. Each dose is time consuming, and at the end of the study we truly reach the limits of subject tolerance (for example, attention span and bladder control for our subjects). Thus the use of these constraint parameters can prove quite useful for in vivo human studies.

Normalizing and constraining the data in this way also has limitations. Zero in this study refers to the maximal amount of vasoconstriction that can be induced by the exogenous administration of norepinephrine. It is acknowledged that this level of vasoconstriction, although maximal, does not represent an absence of flow. With reference to this study, the analysis is focused on vasoconstriction and not flow, so the use of zero is reasonable. Normalizing and constraining the maximal and minimal responses to 100% and 0% also does not permit the determination of E_max or maximal vasoconstrictor capability. Therefore, either analysis method 1 or 3 is preferred if E_max or maximal vasoconstrictor capability is of primary interest to the investigator. Importantly, both analysis methods utilizing curve modeling (analyses methods 1 and 2) generated similar conclusions with this data set regarding the effects of blocking agents (i.e., treatment effect), whereas analysis method 3 trended in the same direction. Thus the effect of cyclooxygenase inhibition on cutaneous α-adrenergic responses can be seen with all analysis methods.

When the research question is to determine the maximal vasoconstrictor capability while retaining the ability to identify other sensitivity parameters (e.g., ED₅₀), analysis method 1 appears to be the best methodological choice. This analysis method of curve fitting determines the top and bottom parameters of the curve to compare the maximal constrictor response (22). However, because of the stringency of the data needed to model this relation, statistical power can be reduced because of data that lie outside the model. Excluding data that are unable to be modeled may be minimized by having duplicate or triplicate probes infusing the same substance. This increases confidence in the model but may be experimentally difficult in some studies because these additional probes can reduce the number of research questions that can be addressed and can be more traumatic for the subject. Another solution may be to fix or constrain the Hill slope (as shown with analysis method 1), which increases the number of curves that can be modeled and is particularly useful if there are a small number of data points within a particular dose-response relationship. Of course, we lose the ability to determine the sensitivity or cooperativity of the slope when restraining the Hill slope. Guidelines for determining the use of a variable or fixed slope are typically determined by the number of data points within the curve and whether minimum and maximum plateaus are achieved. If the curve has many data points with observed plateauing, then a variable slope analysis may provide additional information about the relations between agonist and receptor. However, if data points are limited and there is less clarity concerning curve plateauing, an equation with a standard slope (fixed Hill slope) is normally suggested. This assumes a one-to-one relationship between agonist and receptor, which is a reasonable assumption for norepinephrine and α-adrenergic receptor. In the presented data, there were no differences in interpretation whether a fixed or variable Hill slope curve was modeled.

If significant vasoconstriction occurs with the initial dose of NE, it may not be possible to generate dose-response curves because of the lack of an established top plateau region (20). In this case, if re-dosing is not an option, calculating a percent change in CVC from baseline (analysis method 3) may be a viable alternative to analysis method 1 as this method will still allow the identification of maximal vasoconstrictor capability (20), even though ED₅₀ or Hill slope will not be generated.

After choosing an analysis method, another consideration is choosing the appropriate statistical approach. A simple t-test or ANOVA is used to compare responses at each dose to determine maximal vasoconstrictor capability (2). However, if the question is to compare differences in dose-response curves themselves (sensitivity parameters), then the statistical comparison can be made across all data points simultaneously, encompassing the entire curve (2). Because of the large variability and the moderate shifts that are typically seen with dose-response curves, it can be challenging to show statistical significance despite a clear difference in the response if averaging individual subjects curve parameters, a complication that can lead to type II error (2). Statistical comparisons on all parameters of the dose response curve using the F test compare the sum of squares and degrees of freedom of each curve model to determine whether a specific intervention altered one or all parameters of the curve. However, when using nonlinear regression, the data are usually not normally distributed because there are few data points at the plateau regions with most points around the linear portion of the curve. Therefore, it can be appropriate to use nonparametric tests, such as Friedman's test or Wilcoxon matched pairs test (21) to compare the effects of treatment on dose-response curves.

In summary, the analysis and statistical methods used to examine and compare dose-response vasoconstrictor curves primarily depend on the posed research question. With our data, although the overall interpretation was similar between the two modeling methods of curve fitting, normalizing and constraining parameters increased statistical power and minimized the need to exclude data because of poor curve fitting. Although the other analysis methods were able to quantify the maximal vasoconstrictor responses, these were not altered by KETO or l-NMMA; rather, the ED₅₀ was affected by prostaglandin inhibition. Because of detectable differences between the ED₅₀ of the group mean curve and the mean from individual curves, our data support using the former because it takes into account the overall variability inherent in the ED₅₀, and this method provides information on the general microvascular response throughout the curve. Analysis method 3 is a good method to compare responses to a specific dose, including the maximal dose, but was less powerful in determining difference across doses. Regardless of the methods chosen, it is essential for researchers to provide a precise and detailed description of the analysis method used, along with the reasoning behind these analyses, to enhance cross-study data comparisons and interpretations.

GRANTS

This research was supported by National Heart, Lung, and Blood Institute Grant R01 HL-071159 (awarded to N. Stachenfeld).

DISCLOSURES

No conflicts of interest, financial or otherwise, are declared by the author(s).