A review of methods for quantitative evaluation of spinal curvature

Abstract

The aim of this paper is to provide a complete overview of the existing methods for quantitative evaluation of spinal curvature from medical images, and to summarize the relevant publications, which may not only assist in the introduction of other researchers to the field, but also be a valuable resource for studying the existing methods or developing new methods and evaluation strategies. Key evaluation issues and future considerations, supported by the results of the overview, are also discussed.

Introduction

Quantitative evaluation of spinal curvature is valuable for planning of orthopedical surgical procedures, monitoring the progression and treatment of spinal deformities, and for determining reference values in normal and pathological conditions. Spinal curvature is one of the most significant spine parameters [86]; however, the human capability of its quantitative evaluation from medical images is limited due to our non-systematic search patterns, similar characteristics of normal and pathological conditions, and by the natural biological variability of human anatomy. On the other hand, technical limitations, such as the presence of image noise, distinctive characteristics of imaging techniques and variable positioning of the patient during image acquisition, also represent a major source of variability that may conceal the actual geometrical relationship between anatomical structures and introduce evaluation errors. Although two-dimensional (2D) images are still widely used in clinical examination, advances in medical technology led to the development of new three-dimensional (3D) imaging techniques that have become important clinical tools in modern health care. Especially 2D radiographic (X-ray) images are persistently being replaced by 3D images, acquired by computed tomography (CT) and magnetic resonance (MR) imaging. With a continuously increasing number of medical images, the methods for quantitative evaluation of medical images are most valuable when they are completely automated or semiautomated, i.e., require minimal manual intervention. However, to recognize their medical significance and potential use, techniques for verifying the accuracy and reliability have to be provided. It is therefore crucial to use specially designed methods for quantitative evaluation of spinal curvature that may improve medical diagnosis, treatment and management of spinal disorders.

The aim of this paper is to provide a complete overview of the existing methods for quantitative evaluation of spinal curvature from medical images. By outlining, analyzing and categorizing each method we summarize the relevant publications, which may not only assist in the introduction of other researchers to the field, but also be a valuable resource for studying the existing methods or developing new methods and evaluation strategies. Let us first discuss three important considerations of this review:

1. An arbitrary 3D spinal deformity can be described by a combination of the deformities in three spatial planes, i.e., in the coronal (frontal), sagittal (lateral) and axial (transverse) plane, each characterized by the corresponding spinal curvature and vertebral rotation. However, as the spine is an articulated yet connected anatomical structure, the spinal curvature and vertebral rotation are related features. For example, the deformity of the spine in the coronal plane can be viewed as a curve in the coronal plane (coronal spinal curvature), which also means that the vertebrae are rotated around the anteroposterior axis (coronal vertebral rotation). However, the anatomical and structural properties as well as human perception of the spine established the concept of referring to a 3D spinal deformity as a combination of the coronal spinal curvature, sagittal spinal curvature and axial vertebral rotation. For the latter, the reader is referred to our accompanying review of methods for quantitative evaluation of axial vertebral rotation [105].

2. The methods for quantitative evaluation of medical images are most valuable when they are completely automated or require minimal manual intervention. Each method in this review was therefore assigned a degree of automation that ranged from zero to five. The description of the six degrees of automation is given in Table 1.

3. A single quantitative measurement of a spine parameter depends on the unknown true value, the inability of the observer or method to repeat multiple measurements (i.e., repeatability, reproducibility or intra-observer variability), and the bias of the observer or method (i.e., reliability or inter-observer variability). However, different studies reported different statistical measures for intra- and/or inter-observer variability of the measurements (Table 2), but not always was enough information given to convert between different measures and further compare different studies. As a result, the accuracy and precision of the reviewed methods are reported according to the original publications.

Table 1.

Degrees of automation, assigned to quantitative evaluation methods

Degree	Description
0	Visual inspection: such an approach is subjective, unreliable and inconsistent for quantitative evaluation
1	Manual measurement: the observer evaluates the relationship between the manually identified geometrical constructs in the image (i.e., the “ruler and pencil” approach)
2	Computer-assisted measurement: the computer evaluates the relationship between the geometrical constructs, obtained by digital reconstruction of manually identified anatomical landmarks
3	Computerized image processing: the computer evaluates the relationship between the geometrical constructs, obtained or enhanced by image processing techniques (e.g., edge detection, filtering)
4	Computerized image analysis: the computer evaluates the relationship between the geometrical constructs, obtained by image analysis techniques (e.g., segmentation, registration)
5	Computerized image understanding: such an approach results in an automated diagnosis, which is the primary objective when developing automated methods

Table 2.

Statistical measures for intra- and/or inter-observer variability of the methods

Measure	Description	Definition*
Root-mean-square error	The estimated accuracy of the measurements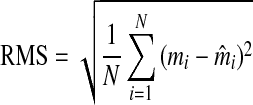
Mean absolute difference	The mean absolute difference between the measurements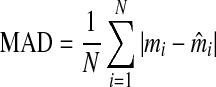
Standard deviation	The estimated precision of the measurements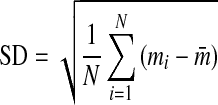
Correlation coefficient	The strength of a linear relationship between two sets of measurements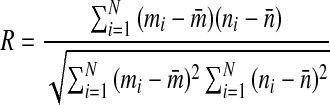
Coefficient of variation	The estimated normalized precision of the measurements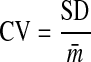
Intra- or inter-class correlation coefficient	The ratio between intra- or inter-observer variability and the total variability of the measurements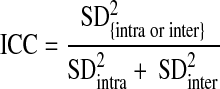
Standard error of measurement	The estimated error of the measurements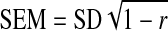

* N number of measurements, m_i, n_i measurement values, 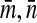mean measurement values, 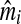reference values, r the reliability coefficient that estimates the amount of consistency

The review is organized according to the type of evaluated images and curvature planes. Sect. “Evaluation of coronal spinal curvature in 2D images” summarizes the methods for evaluation of coronal spinal curvature in 2D images, Sect.  “Evaluation of sagittal spinal curvature in 2D images” the methods for evaluation of sagittal spinal curvature in 2D images, and Sect. “Evaluation of spinal curvature in 3D images”, the evaluation of spinal curvature in 3D images. The article concludes with Sects. “Discussion” and “Conclusion”.

Evaluation of coronal spinal curvature in 2D images

The evaluation of spinal curvature in the coronal (frontal) plane is primarily focused on the measurement of scoliosis, as coronal cross-sections display the most significant component of a scoliotic deformity. The methods for evaluation of spinal curvature were developed first for coronal radiographic images. The reviewed methods with the assigned degrees of automation and reported reliability and/or reproducibility are summarized in Table 3.

Table 3.

Evaluation of coronal spinal curvature in 2D images

Method [ref.]	Degreea	Reported variability [ref.]b
Ferguson [31]	1	SD: 5.6° (intra), 6.6° (inter) [24]
Cobb [19]	1 and 2	SD: 4.2° (inter) [8] • 3.6° (intra), 2.8° (inter) [62] • 1.5° (intra), 2.3° (inter) [37] • 1.9° (intra), 2.5° (inter) [34] • 2.8° (intra), 1.0° (inter) [13] • 2.4° (intra), 3.6° (inter) [61] • 1.7° (inter) [7] • 2.0° (inter) [73] • 4.8° (intra), 5.9° (inter) [56] • 1.5° (intra), 3.9° (inter) [30] • 3.1° (intra), 3.7° (inter) [55] • 4.4° (intra) [76] • 8.5° (intra), 8.8° (inter) [24] • 3.3° (man), 1.7° (comp) [51] • 6.5° (man), 2.2° (comp) [29] • 2.4° (man), 2.0° (comp) [81] • 2.6° (man), 2.1° (comp) [108] • 1.6° (comp, 1), 1.1° (comp, 2) [17] • 2.0° (comp, intra), 2.6° (comp, inter) [89] • 1.6° (man, intra), 3.6° (man, inter) [21] • 3.3° (intra), 3.8° (inter) [1] • 0.65–5.35° [93] SEM: 1.9° (man), 1.4° (comp) [18] • 2.2° (man, intra), 2.0° (comp, intra), 3.2° (man, inter), 2.4° (comp, inter) [3] ICC: 0.9701 (man, intra), 0.9692 (man, inter), 0.9647 (comp, intra), 0.9317 (comp, inter) [38] • 0.93 (man, inter), 0.97 (comp, inter), 0.95 (comp, intra) [60] MAD: 2.5° (intra), 2.7° (inter) [15]
Greenspan index [36]	1 and 2	SD: 13.67–33.6 mm (comp) [93]
Diab et al. [24]	1	SD: 6.1° (intra), 6.5° (inter) [24]
Centroid [15]	2	MAD: 1.7° (intra), 1.7° (inter) [15]

^aDegree of automation

^bMan manual measurement, comp computerized measurement, intra intra-observer, inter inter-observer

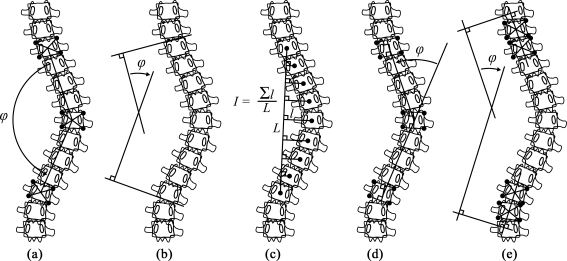
One of the earliest methods was proposed by Ferguson [31]. The method evaluates the deformity by the angle between the two straight lines that connect the centers of the end vertebrae with the center of the apical vertebra (Fig. 1a). A similar method was proposed by Cobb [19], where the deformity is measured by the angle between the two straight lines that are tangent to the superior and inferior endplate of the superior and inferior end vertebra, respectively (Fig. 1b). As both Cobb and Ferguson methods are based on manual identification of the end vertebrae, their variability and unreliability are relatively high. The Cobb angle reflects changes in the end vertebrae inclination rather than changes within the spinal curvature; moreover, it neglects the translation of the apical vertebra, which occurs in scoliosis. Although the latter is taken into account by the Ferguson method, the Cobb method was preferred because of its better reproducibility, easier application and measurement of larger angles for more severe spinal curvatures. In 1966 it was adopted by the Scoliosis Research Society (SRS) as the standard method for quantification of scoliotic deformities, resulting in being nowadays still the most common method for the evaluation of spinal curvature. Although poorly documented, the Greenspan index [36] allows to measure the deformity at individual vertebrae and is therefore valuable for measuring short-segment or small spinal curvatures. The centers of the end vertebrae are connected to form the spinal line, orthogonally to which additional lines are drawn from the center of each vertebra in the spine curve (Fig. 1c). The sum of the lengths of these additional lines divided by the length of the spinal line represents the index of the deformity, which should be zero for normal coronal curvatures.

Fig. 1.

Evaluation of coronal spinal curvature in 2D images. a Ferguson method [31]. b Cobb method [19]. c Greenspan index [36]. d Diab et al. method [24]. e Centroid method [15]

Since the Cobb method has been standardized, a number of studies tested its reproducibility and/or reliability in measurement of idiopathic and congenital scoliosis [8, 13, 30, 34, 37, 55, 56, 60–62, 73, 76]. The accepted standard for a measured change to represent a true change in spinal curvature is considered to be 5°. Goldberg et al. [34] reported that the variability of the Cobb angle tends to increase in measuring smaller curvatures and concluded that it can be considered as an accurate classifier of adolescent idiopathic scoliosis for clinical purposes. Carman et al. [13] reported that the Cobb angle measurements were 5°, 8° and 10° accurate with a confidence of 30, 90 and 95%, respectively, with the main source of error being the intra-observer error. However, this was not in agreement with the study of Rosenfeldt et al. [76], who reported the intra-observer error to be lower than the inter-observer error. For Morrissy et al. [61], there was a 95% confidence that the measurement error was less than 3°. Moreover, they considered experience not to be a key factor in error minimization, although such findings were supported by Facanha-Filho et al. [30]. Stokes et al. [88] compared the Cobb and Ferguson angle measurements of idiopathic scoliosis and reported high correlation between the two methods, with the Cobb angle overvaluing the Ferguson angle. The selection of different end vertebrae was the largest source of error for both methods. Scholten and Veldhuizen [79] presented a mathematical model for the Cobb and Ferguson angle, and reported a 3° error in the Cobb angle when the plane of scoliotic deformity was not parallel to the radiographic projection plane. Moreover, they reported the Cobb angle to be always larger and the Ferguson angle always smaller than the true spatial angle, and attributed the cause of both findings to axial vertebral rotation. Diab et al. [24] developed a new method for measuring coronal curvature in radiographs and compared it to the Cobb and Ferguson method. The new method consisted of identifying the four vertebral body corners of the apical and end vertebrae. In contrast to the Ferguson method, the centers of vertebral bodies were found at the intersection of lines orthogonal to the superior and inferior endplates. The centers of both end vertebrae were then connected with the center of the apical vertebra, forming two intersecting lines that defined the angle of the deformity (Fig. 1d). The reported high variability of the new method was probably due to the large range of spinal curvatures included in the study, i.e., from 7° up to 80°. Chen et al. [15] obtained the centers of vertebral bodies (i.e., centroids) by connecting the opposite corners of two vertebral bodies at both ends of the measured spine curve. The curvature angle was then defined as the angle between the straight lines through the two top and through the two bottom vertebral centroids (Fig. 1e). The analysis of the measurements in digitized radiographs showed that the centroid angle revealed smaller curvatures and was strongly correlated with the Cobb angle (R ≈ 0.97). However, as it resulted in equal or less intra- and inter-observer variability, the authors suggested that the centroid method, although originally developed for evaluating sagittal spinal curvatures [14], may represent an alternative in assessing scoliosis for clinical diagnosis and treatment decision.

In order to improve the reliability and accuracy of the Cobb angle measurements, methods assisted by computer algorithms were developed. Jeffries et al. [51] digitized the manually identified centers of concavities at each lateral vertebral body wall on anteroposterior radiographs and formed a polygonal arc that approximated the scoliotic curve. Lines tangent to the curve were generated at the apical and at the end vertebrae, which were localized at curve inflection points that corresponded to the first two derivatives of the polygonal arc. Dutton et al. [29] reported high correlation between manual and computerized Cobb angle measurements; however, the reliability and repeatability of computerized measurements were significantly better. Shea et al. [81] replaced the manual with computerized drawing of lines and reported that it can reliably measure the Cobb angle with lower or at least equal intra-observer variability. The same computerized approach but with pre-selected end vertebrae was recently used by Wills et al. [108], who reported approximately the same variability of the method. On the other hand, Gstoettner et al. [38] reported no significant difference in the reliability between manual and computerized Cobb angle measurements in plain analog and digital radiographs, respectively. A different computerized measurement of the Cobb angle was presented by Chockalingam et al. [18]. A radiograph was first divided by lines into cranial-to-caudal segments, and for each line, the points that intersected the spine were manually identified. Cheung et al. [17] studied the accuracy of the determination of anatomical landmarks, specifically the vertebral body corners, in digital radiographs. They reported a standard deviation of 0.44 mm in horizontal and 0.63 mm in vertical positioning of the landmarks. The same anatomical landmarks were used by Stokes and Aronsson [89], who concluded that computerized algorithms may improve the reliability of Cobb angle measurements and classification of spinal deformities by reducing technical errors and errors in interpretation and display of data. Very recently, an active shape model approach to the Cobb angle measurement was presented by Allen et al. [3]; however, manual identification of five distinctive anatomical landmarks between vertebrae T4 and L4 was still required. A computerized version of the Greenspan index was proposed by Tang et al. [93], who reported a moderate correlation to the Cobb angle (R = 0.41–0.92). Unfortunately, the authors did not provide any description of the automated extraction of the spinal curvature from the radiographs.

Although scoliosis is the most frequently evaluated spinal deformity, only few methods for quantitative evaluation of coronal spinal curvature exist. Publications have rather been concentrating on the Cobb method, studying its unreliability or improving its performance. The evaluation of the Cobb angle is affected by many factors that can increase the variability of the measurements (e.g., radiographic markers of wide diameter, selection of end vertebrae, bias of different observers, inaccurate protractors, image acquisition techniques, patient positioning, acquisition time, image size). De Smet et al. [22] reported a 2.4° difference between Cobb angles measured in posteroanterior and anteroposterior radiographs that was not related to the angle magnitude. Torell et al. [95] reported an average decrease of 9° between Cobb angles measured in standing and supine positions. Wessberg et al. [107] studied the Cobb angles in standing radiographs and supine MR images and obtained the same results when a supine axial load was applied to patients in the MR scanner; however, the variation between both methods was still 3.4°. On the other hand, Adam et al. [1] performed the Cobb angle measurements manually in reformatted coronal CT cross-sections, and reported that the measurements in supine position were comparable to those in standing position. Beauchamp et al. [7] reported an average increase of 5.2° from morning to afternoon acquisition of the radiograph of the same patient. Pruijs et al. [73] reported that a 2.2° error may be induced during the acquisition of radiographs. De Carvalho et al. [21] recently showed that no significant variations in measurements occur due to the size of the radiographs (large plain analog vs. small digital).

Evaluation of sagittal spinal curvature in 2D images

The evaluation of spinal curvature in the sagittal (lateral) plane refers to the measurement of cervical lordosis, thoracic kyphosis and lumbar lordosis, and to the segmental and reciprocal angulation (i.e., inclination of individual vertebrae and between adjacent vertebrae, respectively). Table 4 summarizes the reviewed methods for the evaluation of sagittal spinal curvature in 2D images.

Table 4.

Evaluation of sagittal spinal curvature in 2D images

Method [ref.]	Degreea	Reported variability [ref.]b
Modified Cobb [19]	1 and 2	SEM: 2.0° (man) [47] • 0.65° (comp) [100] • 2.0° (comp) [107] MAD: 5° [32] • 3° [98] • 2.2° [101] • 4.5° [84] • 6.5° [14] • 7.3° [11] • 2.5° [40] • 1.5° [42] • 3.3°–4.5° [68] • 4.3° (intra) [91] SD: 3.4° (man) [13] • 12.4° (man) [16] • 2.5° (man), 2.7° (comp) [80] • 2.2° (intra), 4.2° (inter) [82] • 2.2°–3.2° (comp) [75] • 3.4° (inter) [91] • 2.9° (intra), 2.7° (inter) [2] ICC: 0.94–0.96 [20] • 0.98 [47] • 0.97 [33] • 0.89 (intra), 0.87 (inter) [69] • 0.95 (intra), 0.92 (inter) [43] R: 0.67–0.95 (intra), 0.64–0.92 (inter) [39] CV: 0.015 [63]
Ishihara index [49]	1	CV: 0.083 [63]
Index of kyphosis/lordosis [101]	1	MAD: 2.4° [101]
Polynomial angle [84]	3	MAD: 2.6° [84] R: 0.98 [11]
Mean radius of curvature [83]	2	ICC: 0.95 [33]
TRALL [16]	1	MAD: 0.8° [42] SD: 7.5° [16]
Centroid [14]	1	MAD: 1.1°–2.9° [14] • 2.8° [11] • 1.0° [40] • 0.8° [42] CV: 0.04 [63]
Best-fit ellipses [41, 50]	3	RMS: 1.1 mm [50] • 1.0 mm [44] • 0.5–1.1 mm [45]
Posterior tangents [46, 96]	1 and 2	SEM: 0.7°–1.0° [46] MAD: 1.8° [40] • 2.0° [42] SD: 2.4° (man), 3.0° (comp) [80] • 1.9° (intra), 3.7° (inter) [82] ICC: 0.97 (intra), 0.94 (inter) [43] CV: 0.029 [63]
Anterior tangents [80]	1 and 2	SD: 2.3° (man), 3.0° (comp) [80]
Tangent circles [97]	2	MAD: 4.0°–5.3° [68]
Area under the curve (AUC) [109]	2	ICC: 0.986 (intra), 0.971 (inter) [109]
Vertebral wedge ratio [94]	1	ICC: 0.94 (intra), 0.88 (inter) [94]

^aDegree of automation

^bMan manual measurement, comp computerized measurement, intra intra-observer, inter inter-observer

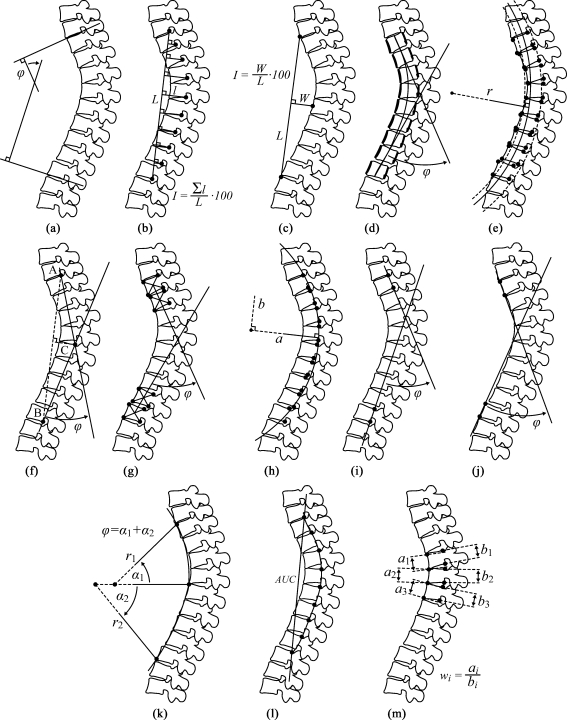
Although originally developed for the evaluation of spinal deformities in the coronal plane, the Cobb method [19] was one of the first methods for the evaluation of sagittal spinal curvature (Fig. 2a). The use of vertebral endplate lines to construct angles on sagittal radiographs is often termed “the modified Cobb method” [40], and was used to evaluate cervical lordosis [20, 39], thoracic kyphosis [2, 11, 13, 32, 57, 84, 91, 98, 101] and lumbar lordosis [47, 57, 98, 101], and to perform segmental angulation analysis [9, 53, 85, 106]. The reported limitations were similar to those present in the measurement of coronal curvature, mainly concentrated around the fact that the Cobb angle predominantly reflects endplate tilt and endplate architecture [69], thus not revealing regional curvature changes. As a result, two spinal curvatures of different magnitude may result in the same Cobb angle [101]. On the other hand, it was also reported that the Cobb angle measurements of thoracic kyphosis [91] and lumbar lordosis [47] may be highly reliable when standardized measurement procedures are used. According to Mac-Thiong et al. [57], the Cobb angle measurements of thoracic kyphosis and lumbar lordosis are mainly influenced by the deformity in the coronal plane and by the sagittal alignment of the pelvis, respectively. Although the Cobb method has become the standard for measuring kyphotic and lordotic curvatures in sagittal radiographs [42, 43], a large number of alternative methods were proposed to overcome its limitations.

Fig. 2.

Evaluation of sagittal spinal curvature in 2D images. a Modified Cobb method [19]. b Ishihara index [49]. c Index of kyphosis/lordosis [101]. d Polynomial angle [84]. e Mean radius of curvature [83]. f TRALL method [16]. g Centroid method [14]. h Best-fit ellipses [41, 50]. i Posterior tangents [46, 96]. j Anterior tangents [80]. k Tangent circles [97]. l Area under the curve (AUC) [109]. m Vertebral wedge ratio [94]

The Ishihara index [49] consists of forming a spinal line by connecting the posteroinferior corners of the bodies of end vertebrae and constructing additional lines orthogonally to the spinal line to the posteroinferior corners of the remaining vertebral bodies in the spine curve (Fig. 2b). The sum of the lengths of these additional lines against the length of the spinal line represents the Ishihara index, which is similar to the Greenspan index [36] for coronal curvature. A similar approach was adopted by Voutsinas and MacEwen [101] for the index of kyphosis/lordosis, where the spinal line was always constructed on the concave side of the curve, while the sum of the lengths of Ishihara additional lines was replaced by the maximal orthogonal distance between the spine curve and the obtained spinal line (Fig. 2c). The index of kyphosis/lordosis was also compared to the Cobb angle, which resulted in similar patterns but a relatively low correlation (R ≈ 0.65). The method was recently also used for predicting vertebral deformities from the measurements of thoracic kyphosis [72].

One of the first documented mathematical models for sagittal spinal curvature was presented by Singer et al. [84]. The thoracic curvature was represented by a polynomial function of the sixth degree that was aligned by a computerized algorithm with the manually identified anterior and posterior vertebral body contours (Fig. 2d). The polynomial angle of kyphosis was obtained from the first derivative (i.e., tangents to the curve), while the inflection point between the kyphotic and lordotic segment was obtained from the second derivative of the polynomial. The comparison to the Cobb method revealed a significant correlation (R = 0.91) and an improved intra-observer variability. A later study [83] reported high inter-observer reliability for a similar computerized method, where the measure of the deformity was derived from two arcs that approximated the manually identified anterior and posterior contours of the vertebral bodies (Fig. 2e). The mean radius of curvature demonstrated a relatively high correlation with the Cobb angle (R = 0.91) and proved superior in overcoming the errors induced by the poor quality of radiographic images [33]. However, the number of anatomical landmarks that had to be manually identified was relatively high.

Chernukha et al. [16] developed a method based on tangential radiologic assessment of lumbar lordosis (TRALL). The posterosuperior corner of the superior end vertebra and the posteroinferior corner of the inferior end vertebra were identified as points A and B, respectively. The point C was determined as the point with the maximal orthogonal distance from the spine to the straight line AB. The TRALL angle was then defined between the straight lines AC and BC (Fig. 2f). When compared to the Cobb angle, the TRALL angle was reported to be less variable and equally reproducible, which probably resulted from easier identification of posterior vertebral body corners than of vertebral endplates. However, when used to assess lumbar lordosis, the method inappropriately added a substantial part of the sacrum to the measurements [87]. A method based on vertebral centroid measurement of lumbar lordosis (CLL) was presented by Chen [14]. The curvature angle was defined by two straight lines that passed through two vertebral centroids at both ends of the measured spine curve (Fig. 2g). The CLL method proved to be more reliable than the Cobb method, as the measurements were not affected by the endplate architecture and the apex of the spine curve did not have to be identified. Briggs et al. [11] used three different methods to study the thoracic kyphosis in osteoporotic spines, where vertebral deformities are common and endplate tilt is difficult to identify accurately. A computerized centroid method, based on manual identification of the vertebral body corners from T1 to T12, was used to overcome this limitation. They obtained the curvature at each vertebral level by computing the gradient of the cubic polynomial function that was aligned with the reconstructed T1–T12 vertebral centroids, and concluded that greater consistency of measurements may be achieved by using the centroid instead of the Cobb method.

Harrison et al. [46] presented a circular geometrical model for predicting cervical curvatures in sagittal radiographs. The model was later extended to an elliptical form and proved useful for describing lumbar lordosis [41, 50], thoracic kyphosis [45] and cervical lordosis [44]. Elliptical modeling was obtained by aligning an elliptical arc to the manually identified posterior corners of vertebral bodies using the least-squares technique (Fig. 2h). The posterior tangent method, although already mentioned in an early study of Gore et al. [35], was mainly studied by Harrison et al. [41, 43, 46]. The angle of sagittal curvature was defined between two straight lines, drawn tangentially to the posterior vertebral body wall of the end vertebrae (Fig. 2i). The absolute rotation angle (ARA) was measured on the whole spine curve, while the relative rotation angle (RRA) was measured between adjacent vertebrae (i.e., segmental angulation). The authors emphasized that the posterior tangent lines were equal to the slope of the spine curve, and could be thus obtained from the first derivative of the mathematical curve model. The posterior tangent method was extensively used in a number of comparative studies by the same authors and/or their collaborators. When compared to the Cobb angle, the method was reported to be superior in terms of measurement error and accuracy in the evaluation of cervical lordosis [43]. In a similar study, the centroid, Cobb and posterior tangent method were compared by evaluating the thoracic kyphosis [40]. Although the differences in reliability and measurement error were relatively small, the posterior tangent method was reported to be the most reliable. The evaluation of lumbar lordosis with the centroid, Cobb, TRALL and posterior tangent method [42] also contributed to a better understanding of different methods. The Cobb angle was not considered a correct measurement because the endplate lines were not orthogonal to the posterior contour of the lumbar spine, while the TRALL method was not recommended for the sole reason of not allowing segmental angulation. Although the authors admitted that the centroid method is very similar to the posterior tangent method, they recommended using the latter because it is defined by the slope of the spine curve. Schuler et al. [80] compared the Cobb, anterior tangent, posterior tangent and a combined Cobb-posterior tangent method. The anterior tangent was similar to the posterior tangent method, with the difference that the curvature angle was measured between lines tangent to the anterior and not to the posterior vertebral body wall (Fig. 2j). The combined Cobb-posterior tangent method used the endplate line and the posterior tangent line at different ends of the spine curve. Each method was evaluated on the basis of manual and computerized measurements, obtained from manually identified anatomical landmarks. When compared to manual evaluation, the computerized measurements resulted in higher intra-observer variability but lower inter-observer variability. Silber et al. [82] studied the variability of the posterior tangent and Cobb method in assessing the cervical curvature. They recommended the use of the posterior tangent method, preferably with a single observer performing sequential measurements over time. The reliability of the Ishihara index, Cobb, centroid, and posterior tangent method in assessing the cervical curvature was also evaluated by Ohara et al. [63]. Strong correlation among all evaluated methods was reported when cervical lordosis was maintained. On the other hand, when the cervical curvature was small, sigmoid or kyphotic, the correlation was weak. The Cobb method was surprisingly reported to be the most reliable, while the Ishihara index was reported to be the least reliable, especially when the cervical curvature was small.

A method for modeling the sagittal spinal curvature with tangent circles was proposed by Vaz et al. [97]. The spine curve was modeled by two circular arcs that were tangent to the apices of the thoracic kyphosis and lumbar lordosis, respectively. The curvature angle was defined between the straight lines that connected the centers of the circular arcs with the corners of vertebral bodies at both ends of the spine curve, and the reference horizontal line (Fig. 2k). The authors stated that the method was valuable for the evaluation of global sagittal geometry, especially when there was limited visibility on the radiographs. Pinel-Giroux et al. [68] concluded that tangent circles may represent a good alternative to the Cobb method, as a relatively strong correlation between methods was demonstrated. Yang et al. [109] presented a mathematical model of the sagittal spine curve that was based on cubic spline functions. Spline interpolation allowed the obtained spine curve to pass exactly through each of the manually identified posterosuperior vertebral body corners. For a given cubic spline model, the area under the curve (AUC) could be computed analytically and was therefore proposed as a descriptor of sagittal curvature (Fig. 2l). In a recent study of the reliability of radiographic assessment of sagittal spinal deformities, Tayyab et al. [94] proposed to measure the vertebral wedge ratio, defined by the quotient of the manually obtained anterior and posterior vertebral body heights (Fig. 2m). However, only the apical and its adjacent cephalic and caudal vertebrae in the spine curve were measured, resulting in a relatively high intra- and inter-observer reliability.

Rajnics et al. [75] evaluated a dedicated software for measuring a range of spine variables, including sagittal spinal curvature. In the “SpineView” software, the user had to manually identify a number of anatomical landmarks, among them the anterior contour of each observed vertebral body. The thoracic kyphosis and lumbar lordosis were then computed as the angle between the two straight lines that were orthogonal to the anterior contours of the selected vertebral bodies. The same software was used by Vialle et al. [100] to measure the sagittal spinal curvature and study their relationships to other parameters, such as the inclination of the pelvis. A similar software [77] required manual identification of the T1 and L5 vertebral body corners, the apices of thoracic kyphosis and lumbar lordosis, and the inflection point between both apices. The spine curve was further divided above and below the inflection point into two separate circular arcs and measured by the tangent circles method. Recently, a computerized method similar to the tangent circles [97] was reported by Dimar et al. [25] as a part of the “SagittalSpine” software. The sagittal spine was represented with circular arcs that best fit the manually identified anatomical landmarks at the anterior vertebral body walls.

Evaluation of spinal curvature in 3D images

The main source of variability of the methods for quantitative evaluation of spinal curvature from 2D images originates from the fact that the curvature of the spine, which is a relatively complex 3D anatomical structure, is evaluated by a relatively simple measurement in a single 2D coronal or sagittal plane. However, the development of 3D imaging techniques stimulated the design of methods that attempted to extract spatial information of the spine from 3D images (Table 5).

Table 5.

Evaluation of spinal curvature in 3D images

Method [ref.]	Degreea	Reported variability [ref.]b
3D reconstruction methods	2	SEM: (x, y, z) = (0.8, 0.5, 2.7) mm [78] MAD: 2.3 mm [74] • 1.4–2.4 mm [59] • 0.8–1.9 mm [58] RMS: 1 mm [65] • 2.2 mm [4] SD: (x, y, z) = (0.9, 3.8, 1.5) mm [90] • (x, y, z) = (0.6, 1.6, 6.3) mm [5] • (x, y, z) = (0.5, 0.5, 3.0) mm [54] • 2.4 mm [6] • 2 mm [28]
Modeling with harmonic functions [26, 27, 48, 70, 90]	2, 4	RMS: 0.9 mm [48] SD: 0.9–2.0 mm [26, 27]
Modeling with spline functions [10, 52, 64, 99]	2, 4	MAD: 0.8–3.4 mm [64]
Modeling with polynomial functions [66, 102–104]	2, 4	RMS: 1.1 mm (man), 2.1 mm (comp) [103] SD: 1.7 mm [102] • 0.6 mm (man), 1.4 mm (comp) [103]

^aDegree of automation

^bMan manual measurement, comp computerized measurement, intra intra-observer, inter inter-observer

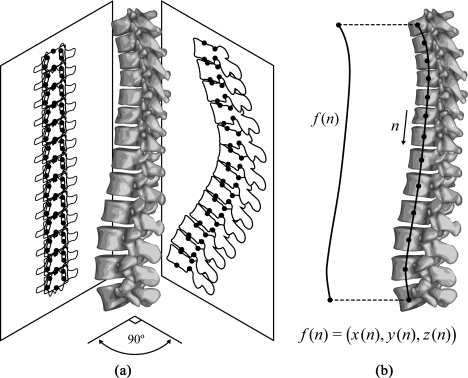
Spatial representation of the spine was first approached by stereophotogrammetric reconstruction in 3D, which was based on computerized identification of corresponding anatomical landmarks in different radiographic images of the same spine [78, 92]. The methods based on biplanar orthogonal radiography performed such identification in coronal and sagittal radiographs [65, 67, 74], while the methods based on biplanar oblique or multiplanar radiography performed such identification in two or multiple oblique radiographs [4, 5, 23, 54, 90]. However, biplanar and multiplanar radiographs are often termed two-and-a-half-dimensional (2.5D) images, since the radiographs are actually 2D, but their combination allows the extraction of 3D structural information. When it was possible to identify the corresponding anatomical landmarks in all available radiographs, the resulting model was stereocorresponding. The centers of vertebral endplates and the superior and inferior tips of pedicles (Fig. 3a) proved to be appropriate landmarks for a six-point stereocorresponding model [12], and for which reconstruction errors of up to 8 and 15 mm were reported for well and poorly visible landmarks, respectively [4, 5]. André et al. [4] attempted to improve the reconstruction accuracy by using three or more radiographs but did not recommend it due to additional patients’ exposure to radiation. Instead of using the centers of vertebral endplates, Verdonck et al. [99] used the corners of vertebral bodies, as they can be better determined in the case of poor visibility of the structures. By using non-stereocorresponding landmarks [58], i.e., landmarks identified in one radiograph without a corresponding pair in any other radiograph, the reconstruction error was reduced to 1.1 mm [59].

Fig. 3.

Evaluation of spinal curvature in 3D images. a Biplanar orthogonal radiographic reconstruction method [10, 65, 67, 74]. b Modeling with mathematical functions, e.g., harmonic [26, 27, 48, 70, 90], spline [10, 52, 64, 99] or polynomial [66, 102–104] functions

Due to the continuous course of the spinal curvature, a number of studies attempted to model the spinal curvature with a mathematical curve in multiplanar radiographic, CT or MR spine images (Fig. 3b). Different functions, such as harmonic functions (i.e., sine and cosine, or Fourier series) [26, 27, 48, 70, 90], splines [10, 52, 64, 99] and polynomials [66, 102–104], and also statistical interpolation techniques, such as kriging [71], were used for modeling. By computerized least-squares aligning of a parametric sine function to the stereographically reconstructed landmarks, Stokes et al. [90] measured the Cobb angle between the normals to the obtained curve at inflection points in the coronal and sagittal plane, and in the plane of maximal curvature. Drerup and Hierholzer [26, 27] also considered the sine function appropriate, as it most resembled the appearance of the curves in idiopathic scoliosis. On the other hand, Patwardhan et al. [64] justified the use of spline functions by stating that splines are used to describe geometries with continuously changing curvature, such as scoliotic spines. In their framework for spine segmentation from CT images, Kaminsky et al. [52] used spline functions because they proved appropriate to describe both the anatomical shape and the scoliotic deformations of the spine. Recently, Berthonnaud and Dimnet [10] constructed the spine curve separately in coronal and sagittal projections by computing the average of two spline functions that connected the anatomical landmarks on vertebral body walls. Polynomial functions were used to model both normal and pathological spine curves in CT images by Vrtovec et al. [102]. The spine curve was automatically determined by aligning the polynomial function with the centers of vertebral bodies in 3D, obtained by maximizing the distance from the edges of vertebral bodies. The same authors also developed a method for MR images [104], where the center of vertebral body was first automatically detected in each axial cross-section by maximizing the entropy of image intensities inside a circular region. The detected centers of vertebral bodies in 3D were then joined by a polynomial function using the robust least-trimmed-squares regression. Polynomial functions were also used by Peng et al. [66] to detect and segment the vertebrae from MR images using vertebral disc templates. Besides modeling the spine curve in 3D, different geometrical descriptors of spinal curvature were derived from mathematical functions. Poncet et al. [70] proposed the geometrical torsion, which describes the rate of rotation of a plane tangent to the spine curve, as a measure for classifying spinal deformities. Vrtovec et al. [103] showed that clinically relevant features of the spine can be identified in 3D by observing the geometric curvature and curvature angle, both derived from polynomial functions describing the spine curves in CT images of normal spines.

Discussion

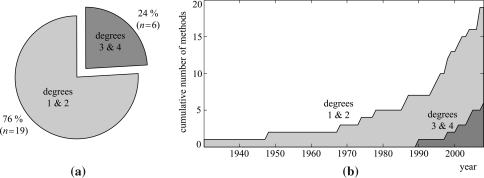
The spinal curvature is among the most important parameters for the evaluation of spinal deformities [86], providing support to various spine-related clinical measurements and image processing techniques. As 2D spine images represent only projections of the actual 3D spinal anatomy onto a chosen plane, an exact description of spinal curvature can only be determined from 3D spine images. However, the extraction of information from 3D images is far more difficult than from 2D images. First of all, the amount of available image information increases to the power of image dimension, moreover, manual measurement in 3D requires navigation through a 3D image, which may be time-consuming and difficult to interpret. The main limitation of some of the reviewed methods is that they were developed for 2D images and then without any modification transferred to 3D images. On the other hand, models defined in 3D, such as mathematical functions that follow the spine curve, are only 3D representations of spinal anatomy and do not provide clinically useful information. Although the evaluation in 3D is generally recognized to be the most accurate, clinically relevant measures for the evaluation of spinal curvature in 3D have yet to be developed. Manual measurements are nowadays considered unreliable, as they are time-consuming, often too complex for routine clinical use, and subjected to relatively high inter- and intra-observer variability. On the other hand, computerized measurements reduce human variability and make the interpretation of images more objective, thus increasing the reliability and repeatability of the evaluation. However, errors may still be introduced when the initial settings of an automated analysis are determined manually. Avoiding manual settings determination is currently the most challenging task in the development of computerized methods. For the evaluation of spinal curvature, solutions to this problem were already approached by image processing and analysis techniques [52, 66, 70, 71, 102, 104]. Figure 4a reveals that most of the reviewed methods can be identified as manual methods (degree of automation 1 and 2), mostly because the oldest manual method in the review dates back to 1930, while the first automated method was proposed in 1990. An interesting observation is that although more automated methods (degree of automation 3 and 4) have become available, the number of manual methods has been increasing even in the last two decades, as shown in Fig. 4b. This may indicate that the possibilities of quantitative evaluation of spinal curvature have not been entirely explored yet, thus still leaving room for further improvements.

Fig. 4.

Summary of the reviewed methods. a The number of manual methods (degree 1 & 2) versus the number of automated methods (degree 3 & 4). b A timeline showing the cumulative number of the proposed manual (degree 1 & 2) and automated methods (degree 3 & 4)

Although a relatively large number of manual and automated methods exist, many methods proved too complex and because of their variability, they were not appropriate for routine clinical use. Moreover, different studies apply different statistical measures of the reliability and reproducibility of a method (Table 2), often hampering the conversion between different measures and further comparison of different studies. In order to develop an accurate, clinically useful and meaningful measure for the evaluation of spinal curvature, generally accepted standards should be adopted. For instance, the research community would benefit immensely from a publicly available database, which would consist of anonymized 2D (e.g., radiographic) and 3D (e.g., CT and MR) images of normal spinal anatomy, most common spinal deformities and also specific cases of spinal pathology. By annotating these images with generally accepted reference manual measurements representing the “gold standard” and by applying reference statistical measures, the researchers would be able to compare between existing methods and test new methods. However, other considerations would arise in the production of such image database, especially in the determination of the reference “gold standard” measurements. The spine is a relatively complex and articulated anatomical structure, and due to the natural biological variability, an infinite range of spinal anatomies exists. The question arises, which are the best anatomical properties that would define the “gold standard” spinal curvature. For example, if the spine curve is defined to pass through the corresponding anatomical landmarks on different vertebrae, which landmarks do we choose and how do we define them? The definition of such landmarks may be ambiguous, especially in 3D. The above-mentioned limitations can be united under the problem of the definition of a spine-based coordinate system. Such a coordinate system should be invariant to rigid body transformations and scaling, and at the same time it should be simple, intuitive, easy to interpret, and explicitly provide clinically relevant information of spinal anatomy.

Conclusion

Despite all of the reported limitations, modern imaging techniques help clinicians in making more accurate diagnosis and planning more effective treatment strategies for spinal disorders. Increasing the efficiency of interpretation, reducing human variability and error, and making the interpretation more quantitative are among the most important motivations for developing systems for computer-assisted diagnosis. Computerized quantitative evaluation of spinal curvature in 3D therefore remains a challenging task in the field of medical image processing, analysis and understanding.