Abstract
Aim
To calculate for which combinations of age and perimetric disease stage glaucoma patients are unlikely to become visually impaired during their lifetime.
Methods
We used residual life expectancy data (life expectancy adjusted for the age already reached) as provided by Statistics Netherlands and rates of progression as derived from published studies. We calculated the baseline mean deviation (MD) for which an individual would reach a MD of −20 dB at the end of life as a function of age and rate of progression. For situations in which the individual rate of progression is unknown, we used the 90th percentiles of rate of progression and residual life expectancy. For situations in which the individual rate of progression is known, we used the 95th percentile of the residual life expectancy.
Results
An easily applicable graphical tool was developed that enables an accurate estimate of the probability of becoming visually impaired during lifetime, given age, current glaucomatous damage, and—if available—the individual rate of progression.
Conclusions
This novel tool enables the clinician to incorporate life expectancy in glaucoma care in a well-founded manner and may serve as a starting point for personalized decision making.
Keywords: glaucoma, ageing, perimetry, rate of progression, healthy ageing
Introduction
Glaucoma is mainly a disease of the elderly. As it tends to have a low speed of progression, some patients will not become visually impaired during their lifetime.1 If patients with a near-zero risk of visual impairment could be identified, a source of excess treatment and excess monitoring—with all its side effects and costs—could be identified as well. The occurrence of future visual impairment (depicted as a certain amount of visual field loss) can be predicted from the current visual field loss of a patient together with his or her rate of progression.2, 3 If the predicted future field loss does not exceed a certain amount before the end of life, a less scrutinized glaucoma care could be the preferred approach.
This seemingly attractive concept has at least two major limitations. First, a reliable measurement of the rate of progression is required. This measurement, however, takes at least 5 years.4 As a result, this information will not be available at the time of the initial decision making, and may never become available in the ageing patient. Hence, initial decision making is necessarily based on general knowledge of rates of progression as found in observational clinical studies and trials. Second, the remaining number of years of life has to be known. This number is usually approximated by the difference between the current age and the median life expectancy at birth, the latter being in between 80 and 85 years of age in the western world. This approximation, however, is unsuitable for estimating life expectancy in the elderly, as the average age-of-dying becomes higher with age. Moreover, it does not take into account variability in survival. As a consequence, many glaucoma patients are diagnosed, monitored, and treated at an age amply beyond the age corresponding to their median life expectancy at birth. To make a proper estimate of the life expectancy of glaucoma patients, life expectancy should be adjusted for the age already reached: residual life expectancy. Moreover, to take into account variability in survival, not the median residual life expectancy should be used but rather some estimate of the upper limit.
The aim of this study was to calculate for which combinations of age and perimetric disease stage glaucoma patients are unlikely to become visually impaired during lifetime. For this purpose, we calculated age and gender-adjusted life expectancy values using data from Statistics Netherlands. With these residual life expectancy values, we calculated the probability of dying without visual impairment. These calculations were performed (1) with rates of progression of untreated and treated glaucoma patients from the literature and (2) with individual rates of progression incorporated. The former yields a tool for initial decision making; the latter yields a tool that can be used in patients with a perimetric follow-up of at least 5 years.
Materials and methods
Residual life expectancy
Residual life expectancy was calculated by using data from Statistics Netherlands (http://www.cbs.nl/; accessed 25 September 2008). This public institute has data available on the chances of dying within a 1-year period for a western population. For example, a male individual who has reached the age of 70 has a chance of dying of 2.5% before reaching the age of 71. The corresponding 1-year survival chance is 97.5%. Likewise, the 1-year survival chance of a male aged 71 is 97.3%.
The chance of surviving from age A to age B can be calculated by multiplying the 1-year survival chances of age A up to age B-1. If this chance equals 50%, then the difference between age A and age B is considered the median residual life expectancy belonging to age A. Similarly, a chance of surviving of 10% corresponds to the 90th percentile of the residual life expectancy belonging to age A. In our example, the chances of surviving for a male individual from age 70 to age 72 can be calculated to be 95% (97.5 of 97.3%). Therefore, the 5th percentile of the residual life expectancy for men aged 70 is 2 years.
Probability of dying without becoming visually impaired
For all ages and both genders, the amount of baseline visual field loss—expressed as a mean deviation (MD) value—above which an eye has a low risk (see below) of becoming visually impaired during lifetime was calculated. This amount, referred to as critical baseline loss, was calculated by subtracting the product of the residual life expectancy and the rate of progression (change of MD in dB/year) from a pre-defined MD level of −20 dB, arbitrarily chosen to represent visual impairment (see Discussion).
For the situation in which the individual rate of progression is unknown, we performed these calculations using the 90th percentile of the rate of progression distribution—both for treated and for untreated patients—and the 90th percentile of the residual life expectancy. The 90th percentile of the rate of progression was assumed to be −2.5 dB/year for untreated glaucoma and −1.0 dB/year for treated glaucoma.5, 6 The critical baseline losses for untreated and treated glaucoma were subsequently plotted with age along the x axis and MD along the y axis. In this way, the age–MD plane was divided into three areas, depicting different probabilities of becoming visually impaired before dying.
For patients for whom the individual rate of progression is known, we performed these calculations for rates of progression of −0.25, −0.5, −1.0 and −1.5 dB/year, and we used the 95th percentile of the residual life expectancy (see Discussion). The critical baseline losses for these four rates were subsequently plotted with age along the x axis and MD along the y axis.
For the situation in which the individual rate of progression is unknown, a computer simulation was used to calculate the percentage of patients who would become visually impaired before the end of life despite an apparently favourable baseline combination of age and MD. For each possible combination of age, gender, and treatment condition, a set of 1000 patients was simulated, all with critical baseline loss. A random survival and rate of progression were assigned to each patient, using the appropriate distributions. The outcome of the simulation was the percentage of patients with an MD below −20 dB, which happened to be 2.5%. For the situation in which the individual rate of progression is known, the use of the 95th percentile of the residual life expectancy implies a 5% risk of becoming visually impaired before dying if a patient has an age–MD combination that coincides with the critical baseline loss corresponding to his or her rate of progression. All analyses were performed using SPSS (version 16.0; SPSS Inc., Chicago, IL, USA).
Results
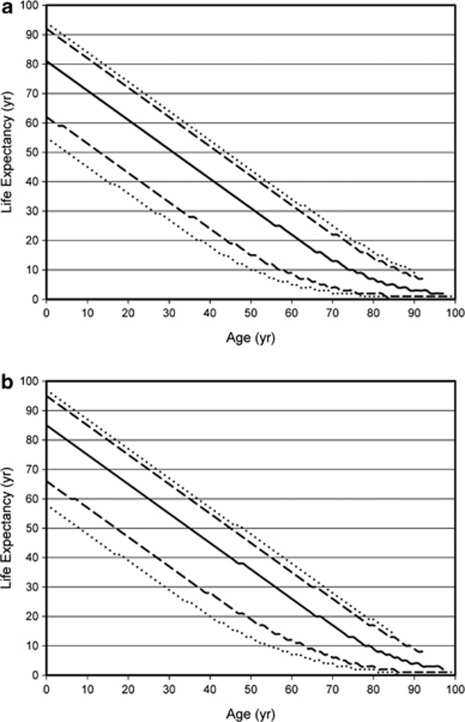
Figure 1 shows the residual life expectancy for men (a) and women (b) as a function of age. The median life expectancy at birth is 81 years for men and 85 years for women. If a man has reached the age of 81 or a woman has reached the age of 85, they both have on average another 6 years to live. For every year a person becomes older, the residual life expectancy decreases by less than a year. This phenomenon becomes more pronounced with age.
Figure 1.
Residual life expectancy (life expectancy adjusted for the age already reached) for men (a) and women (b) as a function of age. Lines depict the median residual life expectancy along with the 5th, 10th, 90th, and 95th percentiles.
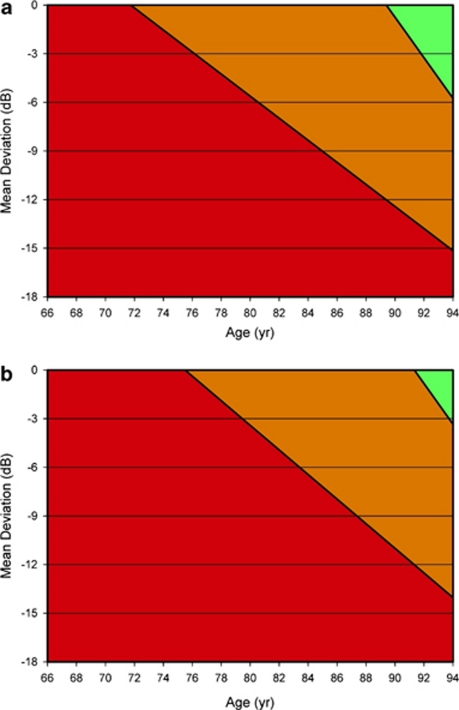
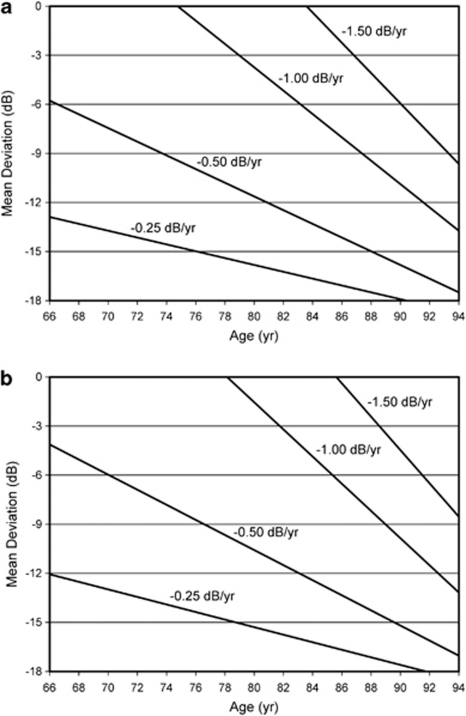
Figure 2 presents the probability of becoming visually impaired before dying for the situation in which an individual rate of progression is unknown, for men (a) and women (b). Figure 3 shows the corresponding results for the situation in which an individual rate of progression is known. The interpretation of the Figures 2 and 3 is explained in the legends.
Figure 2.
Probability of becoming visually impaired before dying for the situation in which an individual rate of progression is unknown, for men (a) and women (b). If the combination of age and current mean deviation brings the patient in the red area, the probability of becoming visually impaired before dying exceeds 2.5%, even if treated. In the orange area, this probability is <2.5% if treated but >2.5% if untreated. In the green area, this probability is <2.5% even if untreated. Visually impaired was defined as a mean deviation of −20 dB.
Figure 3.
Probability of becoming visually impaired before dying for the situation in which an individual rate of progression is known (patients with at least 5 years of perimetric follow-up), for men (a) and women (b). Patients with an age and current mean deviation combination that brings them above their individual rate of progression line have a probability of <5% of becoming visually impaired before dying; below this line, this probability is >5%. Visually impaired was defined as a mean deviation of −20 dB.
Discussion
This novel tool, condensed into two easily applicable graphs that could be used stand-alone in the consulting room, should help the clinician with the decision-making process in glaucoma in the elderly. The first graph (Figure 2) enables a well-founded estimate of the probability of visual impairment if only the age and the current amount of glaucomatous damage are known; the second graph (Figure 3) offers a refinement of the estimate once the individual rate of progression becomes available.
Patients in the green area of Figure 2 have a probability of <2.5% to become visually impaired during their lifetime, even if untreated; watchful waiting would seem to be the appropriate initial approach. On the other hand, patients in the red area are at risk of visual impairment, even if treated. Therefore, monitoring and treatment according to current standards would be obligatory in these patients. For patients in the orange area, an intermediate approach can be followed. A patient with a glaucomatous optic disc but without visual field loss may be considered to have an MD of 0 dB. This approach is on the safe side, as such a patient could be years away from actually developing visual field loss. If a perimetric follow-up was deemed needed, the individual rate of progression can be determined after 5 years, and the glaucoma care can be fine-tuned using Figure 3. For example, an 84-year-old male patient with an MD of −9 dB has a rate of progression of −1.0 dB/year despite optimized topical treatment and laser surgery. In this patient, Figure 3 denotes a non-negligible risk of blindness and may guide the clinician towards glaucoma surgery. If the same patient would have a rate of progression of −0.5 dB/year, a more conservative approach might be preferred.
Our tool should not be considered as a rule that dictates the need of treatment and/or follow-up in individual patients. Rather, it helps the clinician to estimate the prior probability of future visual impairment in a reproducible way, which may serve as a starting point of the decision-making process. Before making a decision, the clinician should compare his/her patient with the characteristics of the populations on which the analyses presented in this study were based (see below). The patient may have a higher risk than addressed in the analyses if pseudoexfoliation is present, if it concerns a secondary glaucoma, or in the case of African descent. Also, the clinician should try to make a personalized estimate of the life expectancy. No signs of any chronic illness along with a family history of longevity may denote one end of the spectrum and a long drug list the other end. Moreover, the clinician should take into consideration personal visual needs and the location of the initial defect. Generally speaking, quality of life is better with two functional eyes,7 indicating that the tool should be applied to the worse eye. However, in some situations, it is more realistic to concentrate on the better eye, depending on what is considered acceptable or possible given individual patient's circumstances, or on both eyes separately (for example, the decision might be a surgery in a functional eye with advanced disease along with watchful waiting in a fellow eye with little damage). Finally, the impact of the decision (prescribing drops or doing a laser trabeculoplasty may be more easily initiated than performing filtration surgery or implanting a drainage device) and the preferences of the patient (who may prefer ‘to be on the safe side' or to refrain from treatment as much as possible) should be taken into account. This is especially important if the individual combination of MD and age, and rate of progression if available, is close to a decision cutoff point.
When applying Figure 2, the probability of a poor outcome (that is, an MD below −20 dB at the end of life) is 2.5%. This seems to be a clinically realistic choice. A much higher probability would be undesirable from an ethical point of view whereas a much lower probability would result in the unrealistic need to monitor and treat all patients until death. Moreover, some risk can be accepted more easily in a slowly progressing disease like glaucoma where an initial policy can be adjusted later. When applying Figure 3, the probability of a poor outcome is 5%. We selected this probability because data needed to make calculations for a probability of 2.5% were not available for the very old.
For untreated glaucoma, we used rate-of-progression data from the early manifest glaucoma trial (EMGT; 90th percentile −2.5 dB/year in patients without pseudoexfoliation).5 Patients with a mean IOP above 30 mm Hg or any IOP above 35 mm Hg were excluded from the EMGT.8 For treated glaucoma, data from the Groningen longitudinal glaucoma study (GLGS; 90th percentile −1.0 dB/year) were used. In the GLGS, the mean IOP during follow-up was 14.9 mm Hg with a SD of 2.9 mm Hg.6 The rate of progression distribution was very similar to that of the treated arm of the EMGT.9 Interestingly, the same rate of progression (90th percentile −1.0 dB/year) was found in untreated normal tension glaucoma patients.10 The participants in the EMGT and GLGS were predominantly Caucasians with primary open-angle glaucoma. Obviously, our tool should not be used without caution in patients with characteristics different from those of the participants in these studies, as listed in this paragraph.
Strength of our study is that, we implemented an upper limit of residual life expectancy rather than the median life expectancy at birth—which would have been much easier from a computational point of view. The median life expectancy at birth is 81 years for men and 85 years for women (Figure 1). Many patients in the consulting room have already passed or will pass this age. Hence, similar but simplified tools using the median life expectancy at birth will dangerously underestimate the probability of visual impairment.
We used linear modelling, that is, we assumed MD to decay at a constant rate. Although both episodic and continuous progression types appear to exist,11, 12 this linear approach seems to be the most appropriate choice if it comes to modelling.13 The debate is ongoing as to whether or not the rate of progression increases with disease severity.14 In the EMGT, such an increase was not observed.15 Patients with an MD below −16 dB at baseline, however, were excluded from the EMGT and thus the increase might still occur below −16 dB. As −16 dB is close to our endpoint of −20 dB, disease acceleration may be ignored in our analyses.
The MD is not only influenced by glaucoma but also by cataract. There are perimetric indices that are less influenced by cataract, such as the pattern standard deviation (PSD) and the visual field index (VFI).13, 16 The PSD cannot be used because it does not change monotonically with time. The VFI seems promising, but currently there are no normative data for its rate of progression. The same is the case for other staging systems like the Hodapp–Parrish–Anderson method, the Mills' classification, the AGIS scoring system, or the Glaucoma Staging System 2.17, 18, 19, 20, 21 For these reasons, we confined our study to the MD. From the point of view of safety this is an appropriate choice, because cataract, if present, will result in an overestimation of the amount of glaucomatous damage.
We defined visual impairment as an MD of −20 dB. This corresponds to the definition of end-stage disease as used in the Advanced Glaucoma Intervention Study19 and was also adopted in the NICE guidelines (http://www.nice.org.uk/CG85; assessed 12 May 2009). Visual impairment and loss of quality of life are related to the MD,22, 23, 24, 25, 26, 27 but also to the location of visual field loss. In most glaucoma patients, the field loss starts superiorly in the Bjerrum area or in the nasal periphery. In some patients, however, the field loss starts adjacent to fixation. These patients are more likely to lose fixation in an early stage and to perceive disability due to overlapping binocular field loss,28 especially if located inferiorly. For that reason, our tool should not be used in these patients.
We assumed the residual life expectancy of glaucoma patients to be equal to that of the general population. Some studies have reported a decreased life expectancy in glaucoma patients, whereas others have not. Based on a recent review that denied a decreased life expectancy in glaucoma patients,29 we decided not to adjust residual life expectancy for the presence of glaucoma.
Finally, clinicians dealing with elderly patients with a chronic disease must incorporate life expectancy in some way in their decision making, and it is part of the physicians duty to ‘set limits' by not ordering tests and treatments of zero or very marginal utility.30 We hope that our tool is helpful in this respect.
Acknowledgments
Financial support was provided by the University Medical Center Groningen.
The authors declare no conflict of interest.
Footnotes
Meeting presentation: This work has been presented at the EVER meeting 2009, Portoroz, Slovenia, and at the WOC 2010, Berlin, Germany.
References
- Ang GS, Eke T. Lifetime visual prognosis for patients with primary open-angle glaucoma. Eye (Lond) 2007;21:604–608. doi: 10.1038/sj.eye.6702284. [DOI] [PubMed] [Google Scholar]
- Chauhan BC, Garway-Heath DF, Goni FJ, Rossetti L, Bengtsson B, Viswanathan AC, et al. Practical recommendations for measuring rates of visual field change in glaucoma. Br J Ophthalmol. 2008;92:569–573. doi: 10.1136/bjo.2007.135012. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Holmin C, Krakau CE. Regression analysis of the central visual field in chronic glaucoma cases. A follow-up study using automatic perimetry. Acta Ophthalmol. 1982;60:267–274. doi: 10.1111/j.1755-3768.1982.tb08381.x. [DOI] [PubMed] [Google Scholar]
- Jansonius NM. On the accuracy of measuring rates of visual field change in glaucoma. Br J Ophthalmol. 2010;94:1404–1405. doi: 10.1136/bjo.2009.164897. [DOI] [PubMed] [Google Scholar]
- Heijl A, Bengtsson B, Hyman L, Leske MC. Natural history of open-angle glaucoma. Ophthalmology. 2009;116:2271–2276. doi: 10.1016/j.ophtha.2009.06.042. [DOI] [PubMed] [Google Scholar]
- Wesselink C, Heeg GP, Jansonius NM. Glaucoma monitoring in a clinical setting: glaucoma progression analysis vs nonparametric progression analysis in the Groningen longitudinal Glaucoma Study. Arch Ophthalmol. 2009;127:270–274. doi: 10.1001/archophthalmol.2008.585. [DOI] [PubMed] [Google Scholar]
- Brown MM, Brown GC, Sharma S, Busbee B, Brown H. Quality of life associated with unilateral and bilateral good vision. Ophthalmology. 2001;108:643–648. doi: 10.1016/s0161-6420(00)00635-7. [DOI] [PubMed] [Google Scholar]
- Leske MC, Heijl A, Hyman L, Bengtsson B. Early Manifest Glaucoma Trial: design and baseline data. Ophthalmology. 1999;106:2144–2153. doi: 10.1016/s0161-6420(99)90497-9. [DOI] [PubMed] [Google Scholar]
- Heijl A, Leske MC, Bengtsson B, Hyman L, Bengtsson B, Hussein M. Reduction of intraocular pressure and glaucoma progression: results from the Early Manifest Glaucoma Trial. Arch Ophthalmol. 2002;120:1268–1279. doi: 10.1001/archopht.120.10.1268. [DOI] [PubMed] [Google Scholar]
- Anderson DR, Drance SM, Schulzer M. Natural history of normal-tension glaucoma. Ophthalmology. 2001;108:247–253. doi: 10.1016/s0161-6420(00)00518-2. [DOI] [PubMed] [Google Scholar]
- McNaught AI, Crabb DP, Fitzke FW, Hitchings RA. Modelling series of visual fields to detect progression in normal-tension glaucoma. Graefes Arch Clin Exp Ophthalmol. 1995;233:750–755. doi: 10.1007/BF00184085. [DOI] [PubMed] [Google Scholar]
- Mikelberg FS, Schulzer M, Drance SM, Lau W. The rate of progression of scotomas in glaucoma. Am J Ophthalmol. 1986;101:1–6. doi: 10.1016/0002-9394(86)90457-5. [DOI] [PubMed] [Google Scholar]
- Bengtsson B, Heijl A. A visual field index for calculation of glaucoma rate of progression. Am J Ophthalmol. 2008;145:343–353. doi: 10.1016/j.ajo.2007.09.038. [DOI] [PubMed] [Google Scholar]
- Wesselink C, Marcus MW, Jansonius NM.Risk factors for visual field progression in the Groningen longitudinal glaucoma study: a comparison of different statistical approaches J Glaucoma 2011. e-pub ahead of print 22 June 2011; doi: 10.1097/IJG.0b013e31822543e0 [DOI] [PubMed]
- Heijl A, Leske MC, Bengtsson B, Bengtsson B, Hussein M. Measuring visual field progression in the early manifest glaucoma trial. Acta Ophthalmol Scand. 2003;81:286–293. doi: 10.1034/j.1600-0420.2003.00070.x. [DOI] [PubMed] [Google Scholar]
- Bengtsson B, Lindgren A, Heijl A, Lindgren G, Asman P, Patella M. Perimetric probability maps to separate change caused by glaucoma from that caused by cataract. Acta Ophthalmol Scand. 1997;75:184–188. doi: 10.1111/j.1600-0420.1997.tb00121.x. [DOI] [PubMed] [Google Scholar]
- Brusini P. Monitoring glaucoma progression. Prog Brain Res. 2008;173:59–73. doi: 10.1016/S0079-6123(08)01106-0. [DOI] [PubMed] [Google Scholar]
- Brusini P. Clinical use of a new method for visual field damage classification in glaucoma. Eur J Ophthalmol. 1996;6:402–407. doi: 10.1177/112067219600600411. [DOI] [PubMed] [Google Scholar]
- AGIS investigators Advanced Glaucoma Intervention Study. 2. Visual field test scoring and reliability. Ophthalmology. 1994;101:1445–1455. [PubMed] [Google Scholar]
- Hodapp E, Parrish I, Anderson DR. Clinical Decisions in Glaucoma. Mosby: St Louis, MO; 1993. pp. 52–61. [Google Scholar]
- Shin YS, Suzumura H, Furuno F, Harasawa K, Endo N, Matsuo H.Classification of glaucomatous visual field defects using the humphrey field analyzer box plotsIn: Mills RP, Heijl A (eds).Perimetry Update 1990/91 Kugler Publ: Amsterdam, New York; 1991235–243. [Google Scholar]
- Gutierrez P, Wilson MR, Johnson C, Gordon M, Cioffi GA, Ritch R, et al. Influence of glaucomatous visual field loss on health-related quality of life. Arch Ophthalmol. 1997;115:777–784. doi: 10.1001/archopht.1997.01100150779014. [DOI] [PubMed] [Google Scholar]
- Lin JC, Yang MC. Correlation of visual function with health-related quality of life in glaucoma patients. J Eval Clin Pract. 2010;16:134–140. doi: 10.1111/j.1365-2753.2009.01135.x. [DOI] [PubMed] [Google Scholar]
- Magacho L, Lima FE, Nery AC, Sagawa A, Magacho B, Avila MP. Quality of life in glaucoma patients: regression analysis and correlation with possible modifiers. Ophthalmic Epidemiol. 2004;11:263–270. doi: 10.1080/09286580490515251. [DOI] [PubMed] [Google Scholar]
- Nah YS, Seong GJ, Kim CY. Visual function and quality of life in Korean patients with glaucoma. Korean J Ophthalmol. 2002;16:70–74. doi: 10.3341/kjo.2002.16.2.70. [DOI] [PubMed] [Google Scholar]
- van Gestel A, Webers CA, Beckers HJ, van Dongen MC, Severens JL, Hendrikse F, et al. The relationship between visual field loss in glaucoma and health-related quality-of-life. Eye (Lond) 2010;24:1759–1769. doi: 10.1038/eye.2010.133. [DOI] [PubMed] [Google Scholar]
- Wren PA, Musch DC, Janz NK, Niziol LM, Guire KE, Gillespie BW. Contrasting the use of 2 vision-specific quality of life questionnaires in subjects with open-angle glaucoma. J Glaucoma. 2009;18:403–411. doi: 10.1097/IJG.0b013e3181879e63. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Viswanathan AC, McNaught AI, Poinoosawmy D, Fontana L, Crabb DP, Fitzke FW, et al. Severity and stability of glaucoma: patient perception compared with objective measurement. Arch Ophthalmol. 1999;117:450–454. doi: 10.1001/archopht.117.4.450. [DOI] [PubMed] [Google Scholar]
- Akbari M, Akbari S, Pasquale LR. The association of primary open-angle glaucoma with mortality: a meta-analysis of observational studies. Arch Ophthalmol. 2009;127:204–210. doi: 10.1001/archophthalmol.2008.571. [DOI] [PubMed] [Google Scholar]
- Callahan D. Setting Limits: Medical Goals in an Aging Society. Simon & Schuster: New York; 1987. [PubMed] [Google Scholar]