Abstract
Accurate modeling of arterial response to physiological or pathological loads may shed light on the processes leading to initiation and progression of a number of vascular diseases, and may serve as a tool for prediction and diagnosis. In this study, a micro-structure based hyperelastic constitutive model is developed for passive media of porcine coronary arteries. The most general model contains twelve independent parameters representing the three-dimensional (3D) inner fibrous structure of the media, and includes the effects of residual stresses and osmotic swelling. Parameter estimation and model validation were based on mechanical data of porcine left anterior descending (LAD) media under radial inflation, axial extension, and twist tests. The results show that a reduced four parameter model is sufficient to reliably predict the passive mechanical properties. These parameters represent the stiffness and the helical orientation of each lamellae fibers, and the stiffness of the inter-lamellar struts interconnecting these lamellae. Other structural features, such as orientational distribution of helical fibers and anisotropy of the inter-lamellar network, as well as possible transmural distribution of structural features, were found to have little effect on the global media mechanical response. It is shown that the model provides good predictions of the LAD media twist response based on parameters estimated from only biaxial tests of inflation and extension. In addition, good predictive capabilities are demonstrated for the model behavior at high axial stretch ratio based on data of law stretches.
Keywords: Arteries, Media, Mechanical Properties, Finite Deformations, Microstructure, Constitutive Equations, Non-Linear Continuum Mechanics
1 Introduction
Mechanical properties of arteries are of central importance in understanding hemodynamics. They play a pivotal role in blood wave propagation along the arterial tree and determine the stress imposed on the vessel wall cells which is converted in turn via signal transduction into biological and biochemical response. These processes may lead to pathologies such as hypertension, atherosclerosis, and aneurysms. Hence, understanding of the mechanical behavior of blood vessels and their underlying determinants is of essential importance in developing preventative and diagnostic measures and new treatment strategies. In particular, a model based on the tissue microstructure will provide a more reliable evaluation of the mechanical environment to which tissue cells and fibers are exposed, and to which they respond biologically.
The wall tissue is mechanically nonlinear, anisotropic and heterogeneous [1–5]. Like other soft tissues, blood vessels are also nearly incompressible [6, 7]. Unloaded arteries are not stress-free. In rings cut from blood vessels, Fung and coworkers [8–11] showed that following a radial cut the ring opens to a circular section with an opening angle (OA), a measure of the residual strain. It was recently found that osmolarity-induced swelling is likely to affect the zero-stress state in addition to its effect on other mechanical properties [12, 13]. These findings are supported by similar observations of myocardial swelling effects in the rat left ventricle [14] and in the aorta [15].
Most of previous constitutive models of arteries are phenomenological [16–20]. As such, they consider average properties of the wall tissue and cannot predict the microscopic mechanical environment of cells and fibers, since their parameters are not directly related to wall microstructure. The mechano-structure link in arteries was first proposed and demonstrated by [21]. Oka and co-workers [22–24] examined the rheological properties of arteries and veins in terms of idealized networks of collagen, elastin, and smooth muscle cells. These models, however, used extremely simplified wall structures, neglecting some essential features. Holzapfel et al. [5, 25–27] employed an invariant based constitutive modeling, which includes the contributions of an isotropic non-collagenous neo-Hookean matrix (consisting of elastin and smooth muscle cells), and two anisotropic 2D families of helical wavy collagen fibers. This approach has been used by other researchers in the field for examining possible mechanisms of tissue growth and remodeling [28–32]. An extension of that basic invariant model to include orientation dispersion of fibers, composing circumferential lamellae, has also been given by [33]. Although these models have a structural basis, the structure they consider is solely that of the collagen. In addition, the use of a neo-Hookean isotropic matrix, which can sustain compressive loads, disregards the possibility of fiber buckling under compression (inter-lamellar fibers with a radial component may buckle as the vessel inflates). A 2D mixture formulation for arterial growth and remodeling was introduced in [34, 35]. This model did not include a phenomenological component, but used expressions of average stresses, thus disregarding transmural stress and strain distributions.
The torsion properties of blood vessels were experimentally measured by [36] and [37]. Previous models were analytically examined under shear [3, 25], but did not include experimental validation against shear data. Although blood vessels in the body are mainly subjected to internal pressure and axial stretch, some may also twist. The coronaries, for example, twist under normal contraction of the heart. For other vessels, characterization of twist is important for the purpose of model validation under more general information.
The blood vessel wall has three distinct layers: intima, media, and adventitia. In healthy vessels, the intima contributes negligibly to the tissue mechanics. The media is made up of a 3D elastin scaffold in the shape of thick fenestrated concentric laminae, inter-connected by a network of thin and short inter-lamellar struts [38–41]. Although the organized lamellar structure has been observed mainly for elastic arteries, it was also noticed for muscular vessels like the coronaries [39]. The elastin scaffold embeds vascular smooth muscle cells (VSMC), which are responsible for the vasoactive properties. The media also contains bundles of thick helically oriented wavy collagen fibers dispersed between the concentric laminae [40,42].
To summarize, although previous models contributed significantly to our understanding of the mechanical response of arterial mechanics, their reliability has not been validated against 3D data. The goal of the present study was to develop a micro-structure based constitutive model for the passive LAD media, and to validate the model against 3D data of inflation, extension, and twist tests. This structural approach has been developed and validated for a number of soft tissues including tendons [43, 44], heart [45–47] and heart valves [48, 49], skin [50], and cartilage [51]. It is hypothesized that a model based on the detailed media microstructure which includes the effects of swelling and residual stress, provides for a realistic representation of the media mechanical properties.
2 Methods
The present media model was contrasted and validated with data of inflation, extension, and twist tests, under internal pressure Pi, external axial load F, and external torque M. The media tube is initially loaded by residual stress (RS) and by tissue osmotic swelling. For these protocols, the media was considered as a hollow cylindrical tube, and the following kinematical assumptions were adopted: 1) deformations are axis-symmetric and independent of axial position [52,53]; 2) incompressible vessel media; 3) transverse sections remain planar (no warping); 4) both the twist angle and axial displacement are independent of radial position; 5) quasi-static and elastic response; 6) no luminal flow and associated shear stress; and 7) there is a unique stress-free reference configuration, which can be obtained through a combination of cutting the tissue radially and unswelling it by immersion in a hyper-osmotic solution [8,10,12,13,15].
2.1 Kinematics
Following these assumptions, the deformation field is
| (1) |
where (r,θ,z) and (R,Θ,Z) are the radial, tangential, and axial cylindrical coordinates in the deformed and reference configurations, respectively.
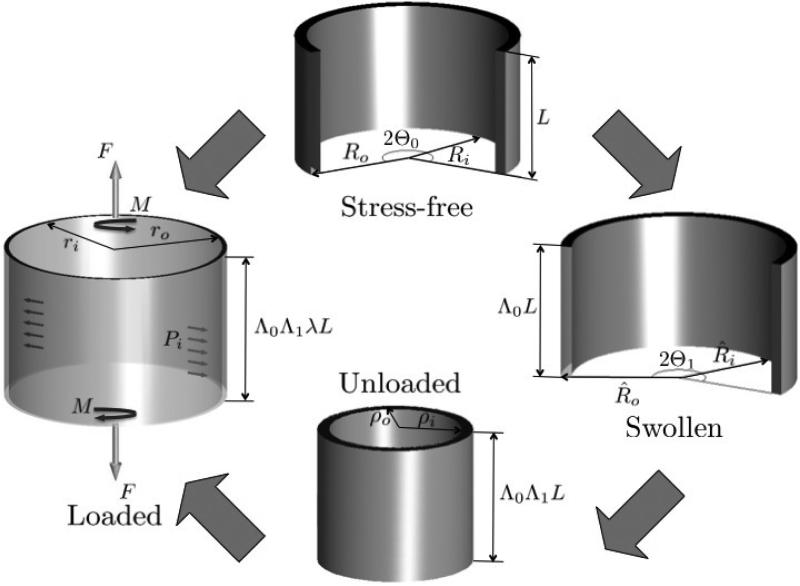
Both media swelling and RS affect the wall response and must be considered in evaluating the accurate state of strain and stress in the tissue. To this end, four distinct configurations were considered (Fig. 1). In the true reference state the vessel is open, unswollen, and stress-free (SF) with inner and outer radii, Ri and Ro, length L and an opening angle Θ0. In the swollen state (SW) it is open but not stress free, due to balance between the fluid-like matrix osmotic pressure and tissue solid fibers. The corresponding dimensions in this configuration are R̂i, R̂o, Λ0L, with Λ0 as the SF–SW transformation stretch ratio, and an opening angle Θ1. The third configuration is closed and unloaded (UL), with no further swelling, and dimensions ρi, ρo, and Λ0Λ1L, with Λ1 as the SW-UL stretch ratio. In the loaded (L) configuration the closed vessel dimensions are: inner and outer radii ri and ro, length λΛ0Λ1L, with λ as the UL-L stretch ratio, and twist per unit length γ. The combined mapping between stress-free and loaded configurations (SF-L) is ( [3,52])
| (2) |
An intermediate mapping of special importance is the one between stress-free and swollen configurations (SF-SW), which is given by
| (3) |
where are the radial, tangential, and axial coordinates of the swollen configuration. By incompressibility, det F = 1 for the intermediate mappings SW-UL and UL-L, where F is the deformation gradient. For the mapping SF-SW an input volume ratio JSW is subscribed. It follows that the radial mappings between SF-L and SF-SW configurations are
| (4) |
Fig. 1.
A schematic description of the mappings from the open stress-free (SF) sector configuration, through the open sector swollen (SW) and closed unloaded (UL) states, to the loaded (L) configuration.
2.2 Statics
The radial component of the vectorial force equilibrium equation (∇ · σ = 0) imposed on the loaded configuration is
| (5) |
with σ as the Cauchy stress tensor. The boundary conditions associated with (5) are
| (6) |
The total axial force F and torque M required to maintain equilibrium in the loaded state are related to the stress by
| (7) |
In Eq. (7), the term is subtracted from F to account for the internal pressure in the closed tube during the test. The circumferential and axial equilibrium equations under no flow yield σrθ = σrz = 0 for all ri ≤ r ≤ ro.
Radial equilibrium equations, similar to 5, were solved for the SW and UL configurations as well, under boundary conditions of zero external loads. For the SW configuration both the resultant force f and bending moment m on the cut edges (associated with the circumferential stress component ) vanish for every radial cut (Fig. 2). The first condition (f = 0) is identically satisfied from the radial equilibrium equation. The condition m = 0 implies the following independent boundary condition [14]
| (8) |
Fig. 2.
Transverse section of the cut open swelled configuration. Distribution of circumferential stress is indicated along with its resultant force f and bending moment m.
Solution of equilibrium equations for each configuration is subjected to compliance with the relevant boundary conditions: the luminal pressure Pi, the loaded stretch ratio λ, the twist angle per unit length γ, and the SW configuration geometry characterized by R̂i, R̂o, and Θ1 (measured experimentally). A value of JSW = 1.35 [54] was assigned to the SW-SF volume ratio. The unknowns to be calculated are the SF-SW and SW-UL stretches Λ0 and Λ1, the stress-free opening angle Θ0, the inner radii of the SF and UL configurations, Ri and ρi, and the loaded configuration outer radius ro, axial force F, and torque M. All unknowns are computed for a set of model parameters, whereas the last three (r0,F,M) are best fitted to experimental data.
2.3 Hyperelastic constitutive formulation
The passive media is considered as an hyperelastic solid, characterized by a strain energy function (SEF) W(E), which is a function of the Green-Lagrange strain tensor E = (FT · F – I)/2. Due to incompressibility, the Cauchy stress tensor is obtained by the relation , with p being the matrix hydrostatic pressure, which acts as a Lagrange multiplier. Using the deformation mapping (2) and recalling the representation of F in cylindrical coordinates [3, 55], the expressions for the non-vanishing Cauchy stress components in the loaded configuration are
| (9a) |
| (9b) |
| (9c) |
| (9d) |
2.3.1 Micro-structural modeling
In the micro-structural approach [56], W reflects the tissue inner micro-structure and associated changes during deformation. The basic assumptions are [46,48,50,56]: 1) the passive tissue total strain energy is the sum of the strain energies of its fibrous constituents, elastin and collagen (mixture theory); 2) the fibers deform as if they are parts of a continuum (affine deformation); and 3) the fluid-like matrix (the ground substance) in which the fibers are embedded contributes hydrostatic pressure. For the present case of arterial media, the following specific assumptions were made:
The elastin network forms a 3D continuous scaffold made of concentric thick lamellae (including the IEL and EEL at the internal and external media boundaries) that are interconnected by a network of thin elastin fibrils (struts).
The thin inter-lamellar struts are uniformly dispersed over orientation space with a mean radial direction [39].
The concentric lamellae are composed by two families of helical fibers with a mean symmetric pitch angle [42, 57, 58]. The fibers’ orientations are distributed around the mean symmetric angle. The first family consists of straight elastin fibers, and the other, of wavy collagen fibers arranged in parallel with the elastin fibers.
All fibers are thin and long and have negligible compressive and bending rigidities compared to their tensile one [56].
With stretch, wavy collagen fibers become gradually straight and mechanically active, thereby increasing the tissue stiffness and inducing non-linear stress-strain relationship even if the fibers’ mechanical response is linear [43].
A main micro-structural characteristic is the fibers orientation density distribution function for each type of fibers i, defined such that is the area fraction over a unit sphere surface of i-fibers oriented between the polar angles (φ,θ) and (φ + dφ,θ + dθ) [56]. In the sequel we will use for simplicity a modified density function . Each parallel fiber bundle has its own energy wi(e), which depends on the uniaxial fiber strain e. Following the affine deformation assumption, e can be derived from the global strain tensor E using the projection e(E,N) = E : NN, where N is the fiber reference unit orientation vector. In this approach, W(E) is expressed as the sum of strain energies in all directions, i.e.,
| (10) |
where is the reference (stress-free) volume fraction of type i fibers. Differentiating W in (10) with respect to E, and using the above relation for e(E,N), yields the volume averaged of the tensor ∂W/∂E
| (11) |
2.4 Model realization
The inter-lamellar (IL) 3D elastin strut network interconnects adjacent media lamellae (Fig. 3). In this family a fiber has a reference orientation Nil = cosαil cosβileR + sinαil cosβileΘ + sinβileZ, where the orthogonal triad (eR,eΘ,eZ) defines the three unit vectors in the reference configuration. Motivated by the observations in [39], on the anisotropic nature of the IL network, the azimuthal and polar angles αil and βil were assumed to be distributed by a 3D beta function given by
| (12) |
where is defined as
| (13) |
with B(k) = [Γ(k)]2/Γ(2k) (k > 1), and m, n, and c0 as material constants. The distribution in (12) and (13) is appropriately bounded and satisfies the normalization criterion
| (14) |
From Eq. (10), the total strain energy function of the IL network is written as
| (15) |
with as the reference volume fraction of the IL fibers. For the IL network, a linear elastin fiber response was assumed, namely, ∂wil/∂eil = kileil, with kil as a material parameter. This choice is based on the nearly linear nature of the mechanical response of elastin. In order to account for the negligible compressive fiber rigidity, the condition ∂wil(eil)/∂eil = 0 for eil ≤ 0 was imposed. By the assumed affine deformation, the IL fiber strain eil is given by
| (16) |
where Eij (i, j = R,Θ,Z) are components of the Green-Lagrange strain tensor E.
Fig. 3.
A scheme of the vessel wall micro-structure including the lamellae helical elastin-collagen fibers, the inter-lamellar strut networks, and the smooth muscle cells.
The second family of fibers is assumed to be composed of elastin and collagen fibers aligned in parallel to each other, forming concentric lamellae. While this consecutive structure has been observed mostly in elastic arteries, it was also noticed for muscular vessels like the coronaries [39]. The fibers in this network are aligned in two groups with symmetrical polar angles (Fig. 3). This bi-polar arrangement follows observations made by [42, 57, 58], and also discussed in [26]. The two primary directions of fibers within this family are , with as the symmetrical polar angle. The polar orientation of fibers in each group is dispersed around the mean by a standard symmetric beta distribution function; namely
| (17) |
which satisfies the normalization criterion . In (17) the symmetric beta function exponent is denoted by mh and the range of orientations is denoted by Δβh; both are additional material parameters. An equivalent orientational distribution of medial helical fibers was considered by [33], who used the von Mises distribution function.
The strain energy function W(E) for the helical network is, therefore,
| (18) |
with and wh(eh) as the reference volume fraction and the helical network fiber strain energy function, where the fiber strain eh(βh) = cos2βhEθθ + 2cosβh sinβhEθZ + sin2βhEZZ. For the helical fibers, a combination of linear and nonlinear power law for the fiber response is assumed, namely,
| (19) |
where is the stiffness related to the helical elastin fibers, and Nh are related to the nonlinear collagen fiber bundle response due to the fiber gradual recruitment with strain, and e01 and e02 are collagen straightening strain limits, such that bellow e01 all collagen fibers are undulated, while above e02 all collagen fibers are stretched.
Solution of vessel kinematics for a known set of parameters, is performed using an inverse method, in which the vessel radius is iteratively updated until static equilibrium and fulfillment of boundary conditions are met. The SUNDIALS ODE solver [59] was applied to obtain the solution in a C-code based program.
2.5 Model Testing
2.5.1 Experimental database
Model validation was carried out based on triaxial mechanical data [37, 60] of porcine coronary arteries. The database includes 5 left anterior descending (LAD) specimen. The cylindrical arteries were cannulated to a triaxial machine and pre-conditioned prior to mechanical testing. Measurements were made of the outer vessel radius, axial force, and torque, in response to a series of applied stepwise luminal pressures (0 to 130 mmHg), axial stretches (1.2 to 1.4), and twist (–25° to 25°, which reflects a range of twist angle per unit length γ = ±0.04rad/mm). In addition, stress-free geometric data were recorded (although hitherto unpublished) for each vessel.
2.5.2 The torsional stiffness
For the experimentally used twist range, recorded data shows [36,37] that M and γ are linearly proportional. This implies
| (20) |
where μ* is the vessel torsional stiffness. Unlike linear elasticity, μ* is considered here a function of the inner pressure and axial stretch, and can be evaluated by differentiating M in (7) with respect to γ, to yield
| (21) |
The derivative of σθz with respect to γ is calculated from (9d) as
| (22) |
2.5.3 Model adjustments to database experiments
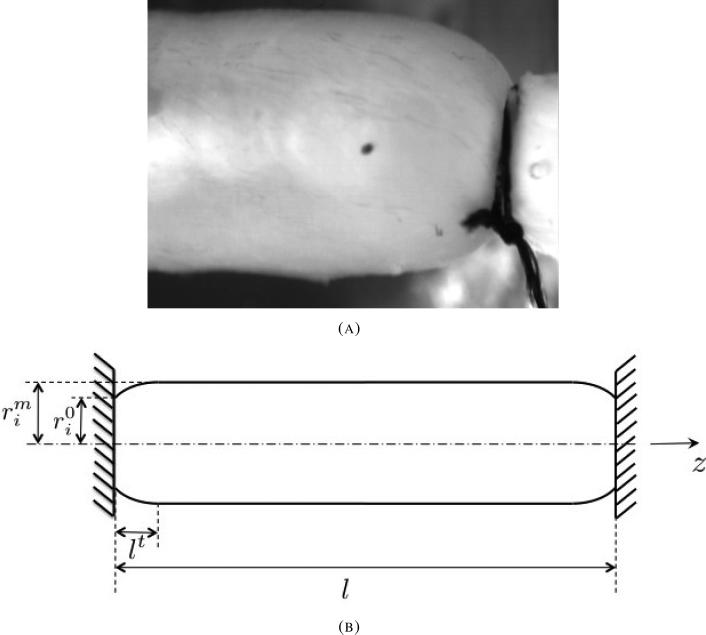
Observations show that during inflation, especially under medium and high internal pressures, the media tube of coronary arteries bulges since its ends are rigidly fixed to cannulae. The bulging occurs along short transition zones near both cannulae, while in between, the tube region is cylindrical (Fig. 4). Hence, the first kinematic assumption regarding independence of axial position is violated during inflation tests. Due to bulging, both the vessel axial force and effective torsional stiffness are in reality smaller than their predicted levels from Eqs. (7) and (21). To compare prediction to data in an accurate manner, the model realization was adjusted to account for the tube bulging. A simple correction was introduced as follows: the twist angle per unit length γ(z) and axial stretch ratio λ(z) were taken to vary with the loaded axial coordinate z. For each axial segment
| (23) |
The torque M can also be written in terms of the effective torsional stiffness μ as follows
| (24) |
where l is the loaded vessel's length and θ(l) is the absolute (measured) twist angle at z = l. By integrating (23) and using (24) the expression for μ is given by
| (25) |
The distribution of the local axial stretch ratio λ(z) = ∂z/∂ζ needs to satisfy the global kinematic constraint of measured deformed vessel length, namely
| (26) |
with LUL as the vessel unloaded length. In addition, it has to satisfy axial equilibrium at each axial position, namely
| (27) |
Fig. 4.
(A) Axial profile of pig RCA media, inflated by luminal pressure of 100mmHg. The vessel radius is constant throughout most of vessel length. A short transition zone, over which the vessel radius decreases from its mid-length level (left) to the cannula radius (right), is seen. (B) Scheme of the loaded vessel axial profile inner diameter.
Along the short transition zones at the tube ends, the tube internal radius changes between the rigid cannula radii () and the radius measured at the vessel mid-section () (Fig. 4B). A parabolically axial profile of the inner radius was assumed between the two radii and over a transition zone of length lt. Similar axial profile was obtained theoretically by [61] for internally pressurized rubber-like shells of revolution.
The radial equilibrium equation (5) must be generalized to include the contribution of the shear stress component σrz, resulting from the vessel bulging
| (28) |
Since the assumed parabolic profile is very close to the real axial profile ( [61] and Fig. 4), the length of the transition zone lt was determined as its value under which the integral of square root deviations from (28) attains its lowest value; i.e.
| (29) |
A simple implementation of the one dimensional Brent's method [62] was used to find lt value, which approximately satisfied equation (29). Following solution of lt, the new effective torsional stiffness μ was computed from equations (21), (22), and (25). The axial force F was recalculated accordingly, but was found to be only slightly affected by the tube bulging, since the local stretch ratio at the vessel mid-section did not deviate significantly from the average axial ratio calculated from the cannulae displacements.
2.5.4 Parameter Estimation
Parameters were optimized by least squares fit to the data by minimizing an objective function consisting of the sum of squared residuals (SSE) between model predictions and experimental data. The objective function incorporates the averaged normalized data of all three protocols and was defined as
| (30) |
where n is the number of data points, (•)i and are the i'th point model prediction and measured data respectively, and are the standard deviations of the experimental data for the three protocols. The search for optimal parameter set was carried out using the Genetic Algorithm (GA) method [63], using an MPI parallel computation based version of the C-code GAUL package [64].
2.5.5 Model Structure Analysis
The general model realization involves 12 parameters: , , Nh, mh, , Δβh, kil, m, n, c0, e01, and e02, summarized in table 1. Following a number of preliminary estimation runs it has been established that the two straightening strains e01 and e02 have a negligible effect, and were therefore excluded from the model (leaving a 10 parameter model) by setting in (19) e01 = 0 and e02 ≫ 1. The structural meaning of excluding these parameters is that within the physiological range of loading not all collagen fibers are fully stretched; at every combination of inner pressure, axial stretch, and twist angle a portion of the fibers are stretched while others are wavy.
Table 1.
A summary of model parameters. Underlined are the parameters found by parsimony analysis to be most significant.
| Parameter | Physical meaning |
|---|---|
| Helical elastin stiffness | |
| Helical collagen gradual recruitment power law response | |
| e01, e02 | Collagen lowest and highest straightening strains |
| Orientational distribution of helical fibers | |
| Inter-lamellar struts stiffness | |
| m, n, c0 | Anisotropic inter-lamellar network |
A further parsimony analysis was conducted on the 10 remaining parameters in order to verify that the model contains a minimum set of parameters, i.e., that each parameter contributes significantly to its descriptive and predictive capabilities. The effect on the goodness of fit of each parameter was tested by sensitivity analysis based on the objective function value. The features tested were: the anisotropy of the IL network (the parameters m, n, and c0); the helical elastin (the parameter ); the orientational distribution within the helical network (the parameters mh, and Δβh); the nonlinear mechanical response of collagen fibers (the parameter Nh); and the level of helicity of the helical fibers (the parameter ). In addition, the significance of swelling in evaluating tissue mechanical behavior was tested for a representative sample, by eliminating tissue swelling, i.e., performing parameter estimation while setting JSW to 1.
2.5.6 Model Predictive Power
Model predictive power was examined in two different manners. First, parameters were estimated from the full data (inflation, extension, and torsion) under two axial stretches (1.2 and 1.3) and then used to simulate response under axial stretch of 1.4. In the second, the model parameters were estimated using only inflation-extension data. Predictive power was examined by comparing parameters estimated from the full data to those estimated from the partial data, and by comparing model predictions (in terms of SSEs) based on partial and full data sets.
2.5.7 Data Structure Analysis
With the goal of determining the minimal sets of test protocols required for reliable parameter estimation, two additional parameter estimations were conducted, based in turn on inflation-torsion (ro, μ) and on extension-torsion (F, μ) data. The results were compared with those based on inflation-extension (ro, F) data, and those based on the full 3D data set, in terms of parameter estimates and model prediction fit to the data.
3 Results
3.1 Model Descriptive Power
Estimation results of the full 10 parameter model are summarized in Table 2A for all LAD media specimen. The values for the parameters , , and kil incorporate the values of their perspective volume fractions. Table 2A also lists the SSE values for each of the measures ro, F, and μ, and the total SSE, as defined in (30). The 3D beta distribution of the IL family (12) is exemplified in Fig. 5(a) for the mean values m = 9.89, n = 6.19, and c0 = 15.50 (Table 2A). The 2D beta distribution of the helical fibers (17) is demonstrated in Fig. 5(b) for the mean values of , mh = 7.40, and Δβh = 0.25.
Table 2.
Parameter estimates and their dispersion (as mean ± standard error of the mean) for LAD media samples based on the full database of inflation, extension, and twist tests: A – estimates for the ten parameter models; B – estimates for the four parameter model.
| A: Full model estimation (10 parameters) | |||||||
|---|---|---|---|---|---|---|---|
| Sample | 1 | 2 | 3 | 4 | 5 | Mean±SEM | |
| [KPa] | 0.79 | 1.03 | 8.29 | 1.21 | 0.54 | 2.37±0.83 | |
| [102 KPa] | 4.68 | 7.52 | 3.25 | 2.56 | 2.68 | 4.14±0.52 | |
| Nh | [-] | 6.55 | 6.74 | 5.78 | 6.96 | 9.45 | 7.10±0.35 |
| mh | [-] | 15.85 | 3.86 | 4.98 | 6.65 | 5.64 | 7.40±1.21 |
| [rad] | 0.64 | 0.61 | 0.72 | 0.71 | 0.62 | 0.66±0.01 | |
| Δ β h | [-] | 0.08 | 0.32 | 0.34 | 0.30 | 0.23 | 0.25±0.03 |
| kil | [101 KPa] | 8.12 | 11.08 | 5.15 | 8.07 | 11.68 | 8.82±0.66 |
| m | [-] | 1.09 | 17.69 | 1.14 | 1.37 | 28.14 | 9.89±12.45 |
| n | [-] | 18.75 | 2.40 | 3.27 | 5.38 | 1.16 | 6.19±1.80 |
| c 0 | [-] | 5.06 | 14.74 | 2.50 | 21.36 | 33.85 | 15.50±3.19 |
|
SSE | |||||||
| ro | [%] | 2.13 | 2.63 | 2.92 | 1.16 | 3.07 | 2.38±0.19 |
| F | [%] | 1.61 | 0.92 | 1.46 | 0.59 | 0.66 | 1.05±0.12 |
| μ | [%] | 0.63 | 1.21 | 0.95 | 0.81 | 0.42 | 0.80±0.08 |
| Total | [%] | 4.36 | 4.76 | 5.33 | 2.55 | 4.15 | 4.23±0.26 |
| B: Reduced model estimation (4 parameters) | |||||||
|---|---|---|---|---|---|---|---|
| Sample | 1 | 2 | 3 | 4 | 5 | Mean±SEM | |
| [102 KPa] | 4.64 | 5.69 | 3.40 | 2.51 | 2.34 | 3.72±0.36 | |
| Nh | [-] | 6.72 | 6.09 | 5.85 | 7.05 | 9.68 | 7.08±0.38 |
| [rad] | 0.63 | 0.56 | 0.70 | 0.68 | 0.59 | 0.63±0.01 | |
| kil | [101 KPa] | 7.99 | 11.06 | 4.73 | 8.05 | 12.02 | 8.77±0.72 |
|
SSE | |||||||
| ro | [%] | 1.93 | 2.59 | 2.80 | 1.23 | 3.08 | 2.33±0.19 |
| F | [%] | 1.63 | 0.79 | 2.03 | 0.75 | 0.58 | 1.16±0.16 |
| μ | [%] | 1.03 | 1.81 | 1.36 | 0.77 | 0.50 | 1.09±0.13 |
| Total | [%] | 4.59 | 5.20 | 6.19 | 2.74 | 4.16 | 4.58±0.32 |
Fig. 5.
Fiber orientation beta distribution functions: (A) The 3D distribution of the inter-lamellar fibers (12); (B) the 2D distribution of the helical fibers (17).
3.2 Model Structure Analysis
The results of the parsimony analysis are given in Table 3. The elimination of parameters representing the anisotropy of the inter-lamellar network, the helical elastin, and orientational distribution of helical fibers, only slightly affects the model descriptive capabilities of the data, as can be deduced from their SSE values. Further elimination of structural features such as waviness of collagen fibers, and the helicity level of the helical fibers, however, causes a substantial SSE increase (SSE ratios are for a representative tissue sample). This implies that although the original model includes 10 parameters representing different structural features, the model complexity for the LAD media in the database loading range can be reduced to include only 4 parameters: , Nh, , and kil (underlined in Table 1). Table 3 also lists the SSE of the estimation result for the case where JSW was set to 1. It is apparent that without swelling, the model descriptive capability deteriorates significantly.
Table 3.
Summary of model parsimony examination. Each line lists the partial to full model SSE ratio. Values are given for a representative tissue (sample #1 in Table 2).
| Model | |
|---|---|
| Full model (10 parameters) | 1.00 |
| Isotropic inter-lamellar network | 1.01 |
| No helical elastin | 1.02 |
| No orientational distribution of helical fibers (Reduced model – 4 parameters) | 1.05 |
| No helical collagen waviness | 11.38 |
| Zero helicity | 7.53 |
| No swelling | 9.10 |
Estimation results for the four parameter model are listed in Table 2B. As can be observed, the SSE values for the 4 parameter estimations are close in most cases to the SSEs of the 10 parameter model. Also, the values of the 4 significant parameters vary only slightly between the two models, and their estimates for all samples are mutually close, with a similar small scatter.
A visual demonstration of the goodness of fit is exemplified graphically in Fig. 6 for a representative LAD tissue (sample #1 in table 2), from which it is observed that the model predictions follow closely the experimental data for all three-dimensional protocols. Model to data comparison between average circumferential and axial Cauchy stresses vs. inner luminal pressure are demonstrated in Fig. 7. For the same tissue sample transmural stress distributions for the three Cauchy components σrr, σθθ, and σzz are plotted in Fig. 8.
Fig. 6.
Model descriptive power: predictions (lines) compared with experimental data (symbols) of (A) outer radius ro, (B) axial force F, and (C) torsional stiffness μ, vs. inner luminal pressure Pi, under three axial stretch ratios (λ).
Fig. 7.
Model descriptive power: predictions (lines) compared with experimental data (symbols) of (A) average circumferential Cauchy stress, and (B) average axial Cauchy stress, vs. inner luminal pressure Pi, under three axial stretch ratios (λ).
Fig. 8.
Transmural distribution of (A) radial, (B) circumferential, and (C) axial Cauchy stresses at fix luminal pressure of 12KPa, under three axial stretch ratios (λ).
In order to assess the significance of the redundant parameters at supra-physiological deformation regions not covered by the data, comparative simulations were performed at regions of higher luminal pressures and stretch ratios, and at regions of low luminal pressure, while using parameters estimated from the available data. In the physiological range of loading, examination of the helical elastin (Figs. 9A–9C) shows an insignificant effect of this feature on the response of the vessel outer radius, axial force, and torsional stiffness (calculated without the aforementioned bulge correction). In high supra-physiological pressures, however, the influence of helical elastin is significant, mostly for the torsional stiffness and axial force, for axial stretch ratio beyond the one tested. In addition, in the low pressure domain, where, due to experimental difficulties, the number of data points is small, helical elastin is predicted to have significant effect on the outer radius (Fig. 9A). Examination of the influence of the orientational distribution of helical fibers (parameters mh and Δβh) revealed that the significance of this feature on the outer radius and torsional stiffness responses is very similar to that of the helical elastin fibers. For the axial force, this orientational distribution is predicted to affect the mechanical response within the database range of stretch ratios, an effect which significantly increases at higher pressures (Fig. 9D). No significant effect of the anisotropy of the inter-lamellar network (represented by the three parameters m, n, and c0) was observed, not within and not outside the database range of physiological pressures and axial stretches (results not shown).
Fig. 9.
Comparative model simulations beyond the range of pressures and axial stretches of the experimental database. In (A), (B), and (C) the influence of helical elastin on the behavior of outer radius, axial force, and torsional stiffness is demonstrated. The influence of orientational distribution on the behavior of axial force in demonstrated in (D). In all subfigures solid and dash lines are the simulations with and without the examined structural feature.
3.3 Model Predictive Power
Parameter estimates (mean±SEM) and model to data fit (SSE), based on partial database are summarized in table 4. The model predictive power is demonstrated for a representative sample in Fig. 10. Results show (Figs. 10A–10C) good prediction of the model under all stretch ratios, including the stretch ratio not used in the estimation process (λ = 1.4). In addition, the results show (Fig. 10D) good prediction of torsional stiffness when torsional stiffness data was not used as part of the estimation process.
Table 4.
Model (four parameter version) estimates (mean± SEM) and SSE values based on partial and full database. Table columns: left – data of stretch ratios 1.2 and 1.3; middle – data of inflation and extension protocols; right – full database.
| Three protocols under two axial stretches | Inflation-extension under three axial stretches | Full database | ||
|---|---|---|---|---|
| [102 KPa] | 3.04±0.54 | 3.22±0.37 | 3.72±0.36 | |
| Nh | [-] | 6.57±0.51 | 6.28±0.38 | 7.08±0.38 |
| [rad] | 0.67±0.02 | 0.63±0.01 | 0.63±0.01 | |
| kil | [101 KPa] | 7.75±0.73 | 8.73±0.69 | 8.77±0.72 |
|
SSE | ||||
| ro | [%] | 2.34±0.18 | 2.37±0.19 | 2.33±0.19 |
| F | [%] | 1.81±0.41 | 1.16±0.12 | 1.16±0.16 |
| μ | [%] | 1.56±0.21 | 1.76±0.33 | 1.09±0.13 |
| Total | [%] | 5.71±0.62 | 5.29±0.48 | 4.58±0.32 |
Fig. 10.
Model predictive power. (A), (B), and (C) – comparison of data (symbols) with predictions of the model with parameters estimated from partial data of stretch ratios 1.2 and 1.3 (solid lines) and compared to data of 1.4 (dashed line). (D) – predictions of torsional stiffness vs. internal pressure with parameters estimated from inflation-extension data.
3.4 Data structure analysis
The results of the four parameter model estimates and of model-to-data fit using only partial data of inflation-extension (ro, F), inflation-torsion (ro, μ), and extension-torsion (F, μ) are summarized in Table 5 (average values over all samples). Results show that only the combination of inflation-extension data is sufficient for reliable estimation. Estimation based on the other two types of partial protocol estimations yielded higher SSE values and unacceptable predictions. An illustration of the unsatisfactory model prediction, estimated against inflation-torsion data, is presented in Fig. 11A. The poor model prediction of axial force F for this case is readily observed. Prediction of outer radius ro response from estimation of extension and torsion (Fig. 11B) yields smaller radii than the experimented values for all pressure region.
Table 5.
Data structure analysis. Parameter estimates (mean±SEM) based solely on inflation-torsion or extension-torsion data, compared to those estimated from inflation-extension and from data of all protocols.
| Partial protocols | Inflation-torsion | Extension-torsion | Inflation-extension | All protocols | |
|---|---|---|---|---|---|
| [102 KPa] | 5.52±0.82 | 5.42±0.94 | 3.22±0.37 | 3.72±0.36 | |
| Nh | [-] | 8.31±0.31 | 7.12±0.25 | 6.28±0.38 | 7.08±0.38 |
| [rad] | 0.66±0.02 | 0.63±0.02 | 0.63±0.01 | 0.63±0.01 | |
| kil | [101 KPa] | 12.16±0.71 | 8.71±0.76 | 8.73±0.69 | 8.77±0.72 |
|
SSE | |||||
| ro | [%] | 2.23±0.18 | 4.21±0.45 | 2.37±0.19 | 2.33±0.19 |
| F | [%] | 17.25±5.69 | 1.02±0.15 | 1.16±0.12 | 1.16±0.16 |
| μ | [%] | 0.54±0.09 | 1.11±0.13 | 1.76±0.33 | 1.09±0.13 |
| Total | [%] | 20.02±5.70 | 6.33±0.57 | 5.29±0.48 | 4.58±0.32 |
Fig. 11.
Data structure analysis: predictions (lines) compared with experimental data (symbols) of (A) axial force from inflation extension data, and (B) outer radius from extension torsion estimation, under three axial stretch ratios (λ).
4 Discussion
A constitutive model has been developed for the passive coronary arterial media, based on its reported 3D morphology. The model micro-structural patterns were taken to mimic observed morphological features while the parameters were evaluated to best fit triaxial mechanical LAD data of radial inflation, axial extension, and twist tests. The model was then tested against these data in terms of its descriptive and predictive capabilities and its structure parsimony. The key result is that a model based on reported wall histology, provides a reliable representation of the LAD media 3D mechanical response.
4.1 Model Descriptive Power
Although the general media model has twelve parameters representing a number of observed histological features, it was found that good descriptive power could be achieved for the LAD media in the physiological range of loadings [37], by a reduced model having only four significant parameters, representing the stiffness and the helical orientation of each lamellae fibers, and the stiffness of the inter-lamellar struts interconnecting these lamellae. Goodness of fit is demonstrated for the direct measured data (Fig. 6) as well as for data of average wall stresses (Fig. 7). Examinations of additional structural characteristics, such as orientational distribution of helical fibers, anisotropy of inter-lamellar network, and the presence of helical elastin, showed little improvement of model predictions.
It should be stressed, however, that these additional model parameters are not insignificant under all circumstances. They are predicted to have a significant impact on the tissue response under pressures and stretch ratios outside the physiological range tested (Fig. 9). The helical elastin fibers, for example, are aligned in parallel to the helical collagen, so that their separate characterization by parameter estimation based on data in the physiological range cannot provide reliable estimates (excessively high variance). Interestingly, their impact on the tissue behavior is predicted to be more significant for the outer radius response at low pressures (Fig. 9A), and for the axial force and torsion stiffness at high supra-physiological stretch ratios (Figs. 9B and 9C). In addition, the orientation distribution of helical fibers is predicted to influence the axial force response at high pressures (Fig. 9D).
4.2 Model Predictive Power
The predictive capabilities of the proposed model were tested and demonstrated over a range of mechanical testing protocols. In these tests, partial data was used for estimation, whereas simulations were performed for missing data. The results show that the model can reliably predict a response which was not used for the estimation. The model accurately predicted the tissue 3D mechanical response at stretch ratio of 1.4, while its parameters were estimated from data which included only two stretch ratios (1.2 and 1.3). In addition, good predictions of twist response were obtained from estimations which included data of inflation-extension only.
4.3 Data structure analysis
The data used in this work is composed of a combined triaxial mechanical response. Although it was shown that reliable model estimation requires all three protocols, two dimensional protocols of inflation and extension may suffice. However, a combination of inflation and torsion (without extension) and of extension and torsion (without inflation) are insufficient for reliable estimations. This result is related to the structural nature of the model. The model considers the tissue fibers. Hence, data of any sets of tests in which all the fibers considered participate in the global tissue response should be sufficient to provide for reliable estimation of the properties and structure of all the fibers. It can be readily envisioned that this is the case for the inflation-extension test combination but not for the other protocol combinations.
4.4 Comparison with Previous Models
Model Nature
The present model is the first purely structural 3D model for arterial media. As such it represents a realistic description of the wall internal structure and its mechanical response to loading, and facilitates reliable evaluation of wall stress and strain fields and the consequent traction exerted on the wall cells. Previous blood vessel constitutive models were either phenomenological [16–20] or structurally motivated [26, 33, 65], but not purely structural in the sense that some of the 3D wall structural features were not considered, but rather phenomenologically modeled (elastin scaffold). The importance of considering the full wall structure can be clearly seen for instance in the models of [27, 65] who treated the non collagenous tissue constituents as a neo-Hookean isotropic solid which can sustain compressive loads. In so doing, they do not take into account possible inter-lamellar fibers buckling during vessel inflation. In addition, the mathematical structure of the tensor ∂W(E)/∂E is different for the neo-Hookean matrix and its inter-lamellar strut equivalent system, proposed in this work. For the neo-Hookean matrix ∂W(E)/∂E = 2μI, with μ as the neo-Hookean material constant, and I as the identity tensor. In the present model ∂W(E)/∂E is a symmetric tensor, including deviatory terms, with non-equal and non-constant components, as can be deduced by differentiating Wil in (15) with respect to E. In order to obtain a symmetric tensor using the invariant approach, one has to consider a strain energy function which depends on both the first and second invariants (e.g. Mooney-Rivlin), resulting in additional material constants. A different model for the elastin matrix was proposed by [28]. They considered SEF of the form W(I1) = c(I1 –3)3/2, for which ∂W(E)/∂E is yet still a diagonal tensor with mutually equal terms.
Osmotic induced swelling was found to influence the blood vessel wall mechanical behavior [12,13,15], as well as that of other soft tissues like the myocardium [14]. Taking into account the osmotic balance between the fluid-like matrix and inter-lamellar fibers is essential for a reliable representation of the tissue structure, and improves its mechanical realism. In this respect the present model is the first to account for the osmotic balance between the fluid-like matrix and inter-lamellar fibers in arteries. The response to tension is taken by the fibers, which buckle under compression. The compressive loads are carried by a fluid-like ground substance matrix, which contributes hydrostatic pressure. An example of the impact tissue swelling has on the tissue residual stress is demonstrated in Fig. 12, in which stress distributions at pressure of 12KPa are plotted for both normal swelling and no swelling cases. As can be seen, swelling reduces transmural gradients of both circumferential and axial stresses.
Fig. 12.
Transmural distribution of (A) circumferential and (B) axial stresses at fix luminal pressure of 12KPa and axial stretch λ = 1.4 for a model with and without tissue swelling
Significant Response Determinants
The present model was extensively tested as to the contribution of each major structural component and of its features (e.g., fibers orientation distribution). It was verified which of these features are more significant to the tissue mechanical response, within the physiological loading region. Previous structurally motivated models a-priori assumed which were the major features but had not verified that other ones were insignificant. For instance, in [27] no proof was provided on the negligible influence of the helical fiber orientation distribution, and of the anisotropy of the inter-lamellar strut network. The effect of fiber orientational distribution of collagen fibers was considered by [33]. However, they did not experimentally validate this effect.
Validation Database
The present model is the first to be validated against shear (twist) data, in addition to data of inflation and extension. Previous studies used only inflation-extension data. Some of the previous validation data relates to response of complete cylindrical vessels, but most previous studies used biaxial stretch data of rectangular strips cut from the vessel wall. The process of cutting these rectangular strips relieves residual stresses which existed in the original vessel, and introduces new stresses due to the flattening of the strips during the experiment.
Number of Model Parameters
The highest number of the present model parameters is twelve. Since media of other arteries have the same general internal structures as considered in the present model, it is expected that future models of other arterial medias will have at most twelve parameters. But the number can be considerably smaller. In the present study, it was found that only four parameters are needed for the LAD media in the physiological range of the available database. In comparison, phenomenological models were found to require up to seven parameters [18,60] using Fung exponential model. In general there are no inter-relationships between parameters of phenomenological and structural models, so that parameter values of the latter cannot be deduced from those estimated for the former.
Plausibility and Stability of the Strain Energy Function
To ensure physical plausibility and stability, the strain energy function must be strongly elliptical. As previously shown [66] fully structural models based on tissues fibrous networks have an important advantage – they are inherently strongly elliptical since the fibers stress-strain relationship are convex. In contrast, as shown by [25], phenomenological models such as the exponential ones, must be checked for ellipticity, which is parameter dependent.
4.5 Model limitations
The model presented in this work is based on structural features observed in histological studies. The quantitative estimation of the model structural parameters was based however on mechanical response data, rather than on direct data of tissue morphology. Although model validation based on histological data is preferred, such quantitative data are scarce. In view of this, a similar approach to the present one has been adopted by previous studies regarding the helical collagen fibers. The 3D orientation distribution of inter-lamellar elastin struts has, to the best of our knowledge, not been studied, and a reliable methodology for studying and quantifying this 3D feature is not yet available. This reliance of estimation on mechanical data is not expected to affect the study results since based on our and others previous experience with a variety of tissues (e.g., skin, myocardium, cartilage, pericardium, heart valves) estimation based on appropriate and sufficient mechanical data provides for good descriptive and predictive model capabilities. Another limitation of the model is related with the value of the swelling ratio, which was taken from the literature rather than being measured per sample.
4.6 Utility of the Model
The present 3D model can be applied to solve general boundary value problems using the finite element method. To that end, a structural finite element which realizes the present media structural model can be readily formulated, as was previously done for the 3D model of the myocardium [67]. Such an element can be incorporated into any commercial FEM package.
Acknowledgement
This research is supported in part by the National Institute of Health-National Heart, Lung, and Blood Institute Grant HL-087235.
Contributor Information
Yaniv Hollander, Faculty of Aerospace Engineering Technion - Israel Institute of Technology Haifa 32000 Israel.
David Durban, Faculty of Aerospace Engineering Technion - Israel Institute of Technology Haifa 32000 Israel.
Xiao Lu, Department of Biomedical Engineering, Surgery, Cellular, and Integrative Physiology Indiana University Purdue University at Indianapolis Indianapolis, Indiana 46202.
Ghassan S. Kassab, Department of Biomedical Engineering, Surgery, Cellular, and Integrative Physiology Indiana University Purdue University at Indianapolis Indianapolis, Indiana 46202
Yoram Lanir, Faculty of Biomedical Engineering Technion -Israel Institute of Technology Haifa 32000 Israel.
References
- 1.Doyle JM, Dobrin PB. “Finite deformation analysis of the relaxed and contracted dog carotid artery.”. Microvasc Res. 1971;3(4):400–415. doi: 10.1016/0026-2862(71)90042-2. [DOI] [PubMed] [Google Scholar]
- 2.von Maltzahn WW, Warriyar RG, Keitzer WF. “Experimental measurements of elastic properties of media and adventitia of bovine carotid arteries”. Journal of biomechanics. 1984;17(11):839–847. doi: 10.1016/0021-9290(84)90142-8. [DOI] [PubMed] [Google Scholar]
- 3.Humphrey JD. Cardiovascular solid mechanics : cells, tissues, and organs. Springer; New York: 2002. [Google Scholar]
- 4.Vito RP, Dixon SA. “Blood vessel constitutive models-1995-2002.”. Annu Rev Biomed Eng. 2003;5:413–439. doi: 10.1146/annurev.bioeng.5.011303.120719. [DOI] [PubMed] [Google Scholar]
- 5.Holzapfel GA, Sommer G, Gasser CT, Regitnig P. “Determination of layer-specific mechanical properties of human coronary arteries with nonatherosclerosis intimal thickening and related constitutive modeling”. Am J Physiol Heart Circ Physiol. 2005;289:2048–2058. doi: 10.1152/ajpheart.00934.2004. [DOI] [PubMed] [Google Scholar]
- 6.Carew TE, Vaishnav RN, Patel DJ. “Compressibility of the arterial wall”. Circ. Res. 1968;23:61–68. doi: 10.1161/01.res.23.1.61. [DOI] [PubMed] [Google Scholar]
- 7.Chuong CJ, Fung YC. “Compressibility and constitutive equation of arterial wall in radial compression experiments.”. J Biomech. 1984;17(1):35–40. doi: 10.1016/0021-9290(84)90077-0. [DOI] [PubMed] [Google Scholar]
- 8.Chuong CJ, Fung YC. “On residual stresses in arteries.”. J Biomech Eng. 1986;108(2):189–192. doi: 10.1115/1.3138600. [DOI] [PubMed] [Google Scholar]
- 9.Omens JH, Fung YC. “Residual strain in rat left ventricle”. Circ Res. 1990;66(1):37–45. doi: 10.1161/01.res.66.1.37. [DOI] [PubMed] [Google Scholar]
- 10.Fung YC. “What are the residual stresses doing in our blood vessels?”. Ann Biomed Eng. 1991;19(3):237–49. doi: 10.1007/BF02584301. [DOI] [PubMed] [Google Scholar]
- 11.Fung YC, Liu SQ. “Strain distribution in small blood vessels with zero-stress state taken into consideration.”. Am J Physiol. 1992;262(2 Pt 2):H544–52. doi: 10.1152/ajpheart.1992.262.2.H544. [DOI] [PubMed] [Google Scholar]
- 12.Guo X, Lanir Y, Kassab GS. “Effect of osmolarity on the zero-stress state and mechanical properties of aorta.”. Am J Physiol Heart Circ Physiol. 2007;293(4):H2328–34. doi: 10.1152/ajpheart.00402.2007. [DOI] [PubMed] [Google Scholar]
- 13.Lanir Y. “Mechanisms of residual stress in soft tissues”. J Biomech Eng. 2009;131(4):044506. doi: 10.1115/1.3049863. [DOI] [PubMed] [Google Scholar]
- 14.Lanir Y, Hayam G, Abovsky M, Zlotnick Y, Uretzky G, Nevo E, Ben-Haim SA. “Effect of myocardial swelling on residual strain in the left ventricle of the rat”. Am J Physiol Heart Circ Physiol. 1996;39:H1736–H1743. doi: 10.1152/ajpheart.1996.270.5.H1736. [DOI] [PubMed] [Google Scholar]
- 15.Azeloglu EU, Albro MB, Thimmappa VA, Ateshian GA, Costa KD. “Heterogeneous transmural proteoglycan distribution provides a mechanism for regulating residual stresses in the aorta.”. Am J Physiol Heart Circ Physiol. 2008;294(3):H1197–205. doi: 10.1152/ajpheart.01027.2007. [DOI] [PubMed] [Google Scholar]
- 16.Vaishnav RN, Young JT, Janicki JS, Patel DJ. “Nonlinear anisotropic elastic properties of the canine aorta.”. Biophys J. 1972;12(8):1008–1027. doi: 10.1016/S0006-3495(72)86140-X. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 17.Fung YC, Fronek K, Patitucci P. “Pseudoelasticity of arteries and the choice of its mathematical expression”. Am J Physiol Heart Circ Physiol. 1979;237:H620–H631. doi: 10.1152/ajpheart.1979.237.5.H620. [DOI] [PubMed] [Google Scholar]
- 18.Chuong CJ, Fung YC. “Three-dimensional stress distribution in arteries.”. J Biomech Eng. 1983;105(3):268–274. doi: 10.1115/1.3138417. [DOI] [PubMed] [Google Scholar]
- 19.Takamizawa K, Hayashi K. “Strain energy density function and uniform strain hypothesis for arterial mechanics.”. J Biomech. 1987;20(1):7–17. doi: 10.1016/0021-9290(87)90262-4. [DOI] [PubMed] [Google Scholar]
- 20.Humphrey JD. “Mechanics of the arterial wall: review and directions”. Crit Rev Biomed Eng. 1995;23(1-2):1–162. [PubMed] [Google Scholar]
- 21.Roach MR, Burton AC. “The reason for the shape of the distensibility curves of arteries”. Can J Biochem Physiol. 1957;35(8):681–90. [PubMed] [Google Scholar]
- 22.Oka S, Azuma T. “Physical theory of tension in thick-walled blood vessels in equilibrium.”. Biorheology. 1970;7(2):109–117. doi: 10.3233/bir-1970-7203. [DOI] [PubMed] [Google Scholar]
- 23.Azuma T, Oka S. “Mechanical equilibrium of blood vessel walls.”. Am J Physiol. 1971;221(5):1310–1318. doi: 10.1152/ajplegacy.1971.221.5.1310. [DOI] [PubMed] [Google Scholar]
- 24.Azuma T, Hasegawa M. “A rheological approach to the architecture of arterial walls.”. Jpn J Physiol. 1971;21(1):27–47. doi: 10.2170/jjphysiol.21.27. [DOI] [PubMed] [Google Scholar]
- 25.Holzapfel GA, Gasser TC, Ogden RW. “A new constitutive framework for arterial wall mechanics and comparative study of material models”. Journal of Elasticity. 2000;61:1–48. [Google Scholar]
- 26.Holzapfel GA, Gasser TC, Stadler M. “A structural model for the viscoelastic behavior of arterial walls: Continuum formulation and finite element simulation”. European Journal of Mechanics A: Solids. 2002;21(3):441–463. [Google Scholar]
- 27.Holzapfel GA, Gasser TC, Ogden RW. “Comparison of a multi-layer structural model for arterial walls with a fung-type model, and issues of material stability”. Journal of Biomechanical Engineering. 2004;126:264–275. doi: 10.1115/1.1695572. [DOI] [PubMed] [Google Scholar]
- 28.Zulliger MA, Fridez P, Hayashi K, Stergiopulos N. “A strain energy function for arteries accounting for wall composition and structure”. J Biomech. 2004;37(7):989–1000. doi: 10.1016/j.jbiomech.2003.11.026. [DOI] [PubMed] [Google Scholar]
- 29.Zulliger MA, Stergiopulos N. “Structural strain energy function applied to the ageing of the human aorta”. J Biomech. 2007;40(14):3061–9. doi: 10.1016/j.jbiomech.2007.03.011. [DOI] [PubMed] [Google Scholar]
- 30.Tsamis A, Stergiopulos N. “Arterial remodeling in response to hypertension using a constituent-based model”. Am J Physiol Heart Circ Physiol. 2007;293(5):H3130–9. doi: 10.1152/ajpheart.00684.2007. [DOI] [PubMed] [Google Scholar]
- 31.Alford PW, Humphrey JD, Taber LA. “Growth and remodeling in a thick-walled artery model: effects of spatial variations in wall constituents”. Biomech Model Mechanobiol. 2008;7(4):245–62. doi: 10.1007/s10237-007-0101-2. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 32.Tsamis A, Stergiopulos N, Rachev A. “A structure-based model of arterial remodeling in response to sustained hypertension”. J Biomech Eng. 2009;131(10):101004. doi: 10.1115/1.3192142. [DOI] [PubMed] [Google Scholar]
- 33.Gasser TC, Ogden RW, Holzapfel GA. “Hyperelastic modelling of arterial layers with distributed collagen fibre orientations”. Journal of the Royal Society Interface. 2005;3:15–35. doi: 10.1098/rsif.2005.0073. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 34.Gleason RL, Humphrey JD. “A mixture model of arterial growth and remodeling in hypertension: altered muscle tone and tissue turnover”. J Vasc Res. 2004;41(4):352–63. doi: 10.1159/000080699. [DOI] [PubMed] [Google Scholar]
- 35.Gleason RL, Jr, Humphrey JD. “A 2d constrained mixture model for arterial adaptations to large changes in flow, pressure and axial stretch”. Math Med Biol. 2005;22(4):347–69. doi: 10.1093/imammb/dqi014. [DOI] [PubMed] [Google Scholar]
- 36.Deng SX, Tomioka J, Debes JC, Fung YC. “New experiments on shear modulus of elasticity of arteries.”. Am J Physiol. 1994;266(1 Pt 2):H1–10. doi: 10.1152/ajpheart.1994.266.1.H1. [DOI] [PubMed] [Google Scholar]
- 37.Lu X, Yang J, Zhao JB, Gregersen H, Kassab GS. “Shear modulus of porcine coronary artery: contributions of media and adventitia”. Am J Physiol Heart Circ Physiol. 2003;285:1966–1975. doi: 10.1152/ajpheart.00357.2003. [DOI] [PubMed] [Google Scholar]
- 38.Wolinsky H, Glagov S. “A lamellar unit of aortic medial structure and function in mammals”. Circ. Res. 1967;20:99–111. doi: 10.1161/01.res.20.1.99. [DOI] [PubMed] [Google Scholar]
- 39.Wasano K, Yamamoto T. “Tridimensional architecture of elastic tissue in the rat aorta and femoral artery - a scanning electron microscope study”. Journal of Electron Microscopy. 1983;32(1):33–44. [PubMed] [Google Scholar]
- 40.Clark JM, Glagov S. “Transmural organization of the arterial media. The lamellar unit revisited”. Arteriosclerosis. 1985;5:19–34. doi: 10.1161/01.atv.5.1.19. [DOI] [PubMed] [Google Scholar]
- 41.O'Connell MK, Murthy S, Phan S, Xu C, Buchanan J, Spilker R, Dalman RL, Zarins CK, Denk W, Taylor CA. “The three-dimensional micro- and nanostructure of the aortic medial lamellar unit measured using 3d confocal and electron microscopy imaging”. Matrix Biology. 2008;27(3):171–181. doi: 10.1016/j.matbio.2007.10.008. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 42.Dahl SLM, Vaughn ME, Niklason LE. “An ultrastructural analysis of collagen in tissue engineered arteries”. Annals of Biomedical Engineering. 2007;35(10):1749–1755. doi: 10.1007/s10439-007-9340-8. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 43.Sverdlik A, Lanir Y. “Time-dependent mechanical behavior of sheep digital tendons, including the effects of preconditioning”. Journal of Biomechanical Engineering. 2002;124:78–84. doi: 10.1115/1.1427699. [DOI] [PubMed] [Google Scholar]
- 44.Raz E, Lanir Y. “Recruitment viscoelasticity of the tendon”. Journal of Biomechanical Engineering. 2009;131(11):111008. doi: 10.1115/1.3212107. [DOI] [PubMed] [Google Scholar]
- 45.Horowitz A, Lanir Y, Yin FC, Perl M, Sheinman I, Strumpf RK. “Structural three-dimensional constitutive law for the passive myocardium”. Journal of Biomechanical Engineering. 1988;110(3):200–207. doi: 10.1115/1.3108431. [DOI] [PubMed] [Google Scholar]
- 46.Nevo E, Lanir Y. “Structural finite deformation model of the left ventricle during diastole and systole”. Journal of Biomechanical Engineering. 1989;111(4):342–349. doi: 10.1115/1.3168389. [DOI] [PubMed] [Google Scholar]
- 47.Nash MP, Hunter PJ. “Computational mechanics of the heart”. Journal of Elasticity. 2000;61(1-3):113–141. [Google Scholar]
- 48.Billiar KL, Sacks MS. “Biaxial mechanical properties of the native and glutaraldehyde-treated aortic valve cusp: Part ii—a structural constitutive model”. Journal of Biomechanical Engineering. 2000;122:327–335. doi: 10.1115/1.1287158. [DOI] [PubMed] [Google Scholar]
- 49.Sacks MS. “Incorporation of experimentally-derived fiber orientation into a structural constitutive model for planar collagenous tissues”. Journal of Biomechanical Engineering. 2003;125:280–287. doi: 10.1115/1.1544508. [DOI] [PubMed] [Google Scholar]
- 50.Lokshin O, Lanir Y. “Micro and macro rheology of planar tissues”. Biomaterials. 2009;30(17):3118–27. doi: 10.1016/j.biomaterials.2009.02.039. [DOI] [PubMed] [Google Scholar]
- 51.Farquhar T, Dawson PR, Torzilli PA. “A microstructural model for the anisotropic drained stiffness of articular cartilage”. Journal of Biomechanical Engineering. 1990;112(4):414–425. doi: 10.1115/1.2891205. [DOI] [PubMed] [Google Scholar]
- 52.Green AE, Adkins JE. Large Elastic Deformations. 2nd ed. Oxford University Press; 1970. [Google Scholar]
- 53.Humphrey JD, Strumpf RK, Yin FC. “A theoretically-based experimental approach for identifying vascular constitutive relations”. Biorheology. 1989;26(4):687–702. doi: 10.3233/bir-1989-26402. [DOI] [PubMed] [Google Scholar]
- 54.Fung YC. Biomechanics - Mechanical Properties of Living Tissues. 2nd ed. Springer; 1993. [Google Scholar]
- 55.Spencer AJM. Continuum Mechanics. Longman Group Limited; 1980. [Google Scholar]
- 56.Lanir Y. “Constitutive equations for fibrous connective tissues”. Journal of Biomechanics. 1983;16(1):1–12. doi: 10.1016/0021-9290(83)90041-6. [DOI] [PubMed] [Google Scholar]
- 57.Canham PB, Finlay HM, Dixon JG, Boughner DR, Chen A. “Measurements from light and polarised light microscopy of human coronary arteries fixed at distending pressure.”. Cardiovasc Res. 1989;23(11):973–982. doi: 10.1093/cvr/23.11.973. [DOI] [PubMed] [Google Scholar]
- 58.Canham PB, Finlay HM, Boughner DR. “Contrasting structure of the saphenous vein and internal mammary artery used as coronary bypass vessels.”. Cardiovasc Res. 1997;34(3):557–567. doi: 10.1016/s0008-6363(97)00056-4. [DOI] [PubMed] [Google Scholar]
- 59.Hindmarsh AC, Brown PN, Grant KE, Lee SL, Serban R, Shumaker DE, Woodward CS. “Sundials: Suite of nonlinear and differential/algebraic equation solvers”. ACM Transactions on Mathematical Software. 2005;31:363–396. [Google Scholar]
- 60.Wang C, Garcia M, Lu X, Lanir Y, Kassab GS. “Three-dimensional mechanical properties of porcine coronary arteries: a validated two-layer model.”. Am J Physiol Heart Circ Physiol. 2006;291(3):H1200–9. doi: 10.1152/ajpheart.01323.2005. [DOI] [PubMed] [Google Scholar]
- 61.Su FC, Taber LA. “Torsional boundary layer effects in shells of revolution undergoing large axisym-metric deformation”. Computational Mechanics. 1992;10(1):23–37. [Google Scholar]
- 62.Press WH, Teukolsky SA, Vetterling WT, Flannery BP. Numerical Recipes in C. 2nd ed. Cambridge University Press; 2002. [Google Scholar]
- 63.Goldberg DE. Genetic Algorithms in Search, Optimization, and Machine Learning. Addison-Weseley; 1989. [Google Scholar]
- 64.Adcock S. Gaul, the genetic algorithm utility library. 2004 ( http://gaul.sourceforge.net)
- 65.Holzapfel GA, Sommer G, Regitnig P. “Anisotropic mechanical properties of tissue components in human atherosclerosis plaques”. Journal of Biomechanical Engineering. 2004;126:657–665. doi: 10.1115/1.1800557. [DOI] [PubMed] [Google Scholar]
- 66.Lanir Y. “Plausibility of structural constitutive equations for swelling tissues – implications of the c-n and s-e conditions”. Journal of Biomechanical Engineering. 1996;118:10–16. doi: 10.1115/1.2795935. [DOI] [PubMed] [Google Scholar]
- 67.Horowitz A, Sheinman I, Lanir Y. “Nonlinear incompressible finite element for simulating loading of cardiac tissue–part ii: Three dimensional formulation for thick ventricular wall segments”. J Biomech Eng. 1988;110(1):62–8. doi: 10.1115/1.3108407. [DOI] [PubMed] [Google Scholar]