Force Spectroscopy Reveals the Effect of Different Ions in the Nanomechanical Behavior of Phospholipid Model Membranes: The Case of Potassium Cation

Abstract

How do metal cations affect the stability and structure of phospholipid bilayers? What role does ion binding play in the insertion of proteins and the overall mechanical stability of biological membranes? Investigators have used different theoretical and microscopic approaches to study the mechanical properties of lipid bilayers. Although they are crucial for such studies, molecular-dynamics simulations cannot yet span the complexity of biological membranes. In addition, there are still some experimental difficulties when it comes to testing the ion binding to lipid bilayers in an accurate way. Hence, there is a need to establish a new approach from the perspective of the nanometric scale, where most of the specific molecular phenomena take place. Atomic force microscopy has become an essential tool for examining the structure and behavior of lipid bilayers. In this work, we used force spectroscopy to quantitatively characterize nanomechanical resistance as a function of the electrolyte composition by means of a reliable molecular fingerprint that reveals itself as a repetitive jump in the approaching force curve. By systematically probing a set of bilayers of different composition immersed in electrolytes composed of a variety of monovalent and divalent metal cations, we were able to obtain a wealth of information showing that each ion makes an independent and important contribution to the gross mechanical resistance and its plastic properties. This work addresses the need to assess the effects of different ions on the structure of phospholipid membranes, and opens new avenues for characterizing the (nano)mechanical stability of membranes.

Introduction

Cells can be thermodynamically defined as open systems that continuously exchange mass, energy, and information with their medium. For that purpose, the cell membrane is a key structure that defines the limits of the cell and some of its internal compartments. The membrane is essential as a structural part of the cell and also provides a support matrix for many types of proteins that are inserted into it (1). Biological membranes are electrostatically charged entities that physiologically coexist with electrolyte solutions. Thus, specific interactions with ions are a matter of considerable interest (2, 3, 4, 5, 6, 7). The distribution of ions in the solution and their interaction with the membranes are factors that substantially modify the structure and dynamics of the cell membranes (8, 9, 10). Furthermore, signaling processes are modified by the membrane's ability to retain ions (11). Supported planar bilayers (SPBs) are a versatile tool for investigating phospholipid membranes that mimic biological surfaces. Such lipid bilayers are planar, well organized, reproducible, composition-tunable, and easy to obtain. They have been shown to be viable model systems for the plasma membrane, as well as robust components of biotechnological nanodevices (12).

The specific chemical environment strongly modifies both the thermodynamic and structural behaviors of SPBs (13, 14). The first experimental approach in this field was performed by Evans (15), who obtained valuable information about the membrane elasticity, shear, and bending moduli by means of a micropipette aspiration technique. Regarding the influence of the electrolyte solution on the membrane, researchers have reported specific effects for different ions of the same charge that in many cases follow a trend as a function of the ion size (10, 16, 17, 18, 19, 20). Studies have shown that ion binding to lipid bilayers provokes an increased ordering in the membrane structure, and it was reported that ions reduce the area per lipid for both negatively charged and zwitterionic phospholipids (6, 21). Detailed information about the location of ions with respect to the polar headgroups and hydrocarbon chains of the phospholipid membranes was obtained from molecular-dynamics (MD) simulations (4, 6, 22, 23, 24, 25, 26, 27), which showed that ion binding modifies the area per lipid, lipid ordering, orientation of the lipid head dipole, and the charge distribution along the system, among other effects. One of the most studied ions, partly because of its biological relevance, is K⁺, which has been a subject of controversy in the literature. MD simulations showed that Na⁺ ions have a strong effect on phosphatidylcholine (PC) bilayers, increasing the lateral interaction between phospholipid molecules. The binding of K⁺ was found to be much weaker, mainly due to the larger size of K⁺ compared with Na⁺ (26). However, these results were not conclusive due to the uncertainty in the estimation of the strength of the different force fields used (27, 28). In addition, it was pointed out that the size of the K⁺ ion may have been exaggerated in some of the simulations (28). Another drawback of MD simulations is that, despite the current computational resources, simulations of ions within a lipid bilayer cannot be of sufficient duration to fully elucidate the interaction between the ion and the surrounding phospholipids. Nevertheless, differences in the residence times and binding behaviors of different ion species have been observed (8, 26, 27).

Atomic force microscopy (AFM) has proved to be a powerful experimental technique not only for imaging surfaces with subnanometer resolution but also for testing the nanomechanical response of a wide variety of samples, both in air and in a liquid environment, by means of the force spectroscopy (FS) mode. FS is a unique technique in terms of spatial accuracy and force resolution when it comes to testing the response of phospholipid membranes under compression. In a typical FS experiment (also called force curve), the AFM tip approaches the surface until a mechanical contact with the lipid bilayer is established. Then, the bilayer is elastically deformed by the AFM probe until its tip ruptures the membrane, thereby coming into contact with the substrate. This scenario is exemplified in Fig. 1 B, where a jump can be observed on the approaching force curve. This is interpreted as the penetration of the AFM tip through the SPB (29). The vertical force (F) at which this jump happens is the maximum force (F_y) the bilayer is able to withstand before breaking. Because of the reproducibility of its value, F_y can be considered as a fingerprint of the bilayer stability under the experimental conditions in which the measurements are performed. Different variables have been reported to affect the F_y-value, including the temperature, tip and phospholipid chemistry, tip approaching velocity, pH, and ionic strength (2, 13, 30). For example, it was experimentally determined that the ionic strength increases the mechanical stability of lipid bilayers by increasing the electrostatic interaction between phospholipid headgroups due to a charge screening effect, leading to a higher proximity of the hydrophobic phospholipid tails and a consequent increase of van der Waals interactions (31). A pioneer study about the effect of ionic strength on the mechanical stability of lipids was performed by Garcia-Manyes et al. (2), who showed that high ionic strength induces a better and faster deposition of PC bilayers onto mica and an increase of the F_y-value, for both Na⁺ and Mg²⁺ addition. It is well established that electrostatic interactions have a pivotal role in the structural and dynamic properties of lipid bilayers (2, 13, 32, 33, 34, 35, 36). This is the case for Na⁺ or Ca²⁺, which strongly interact with the carbonyl oxygen groups of PC polar moieties while changing the orientation of the phospholipids and their molecular packing (5, 6, 7, 37, 38). Interestingly, this increment in the lipid order also influences the brittleness of the lipid bilayer. Oncins et al. (31) studied the friction properties of PC bilayers and concluded that the cohesion of the film and the F_y-value are reduced in the absence of NaCl.

Figure 1.

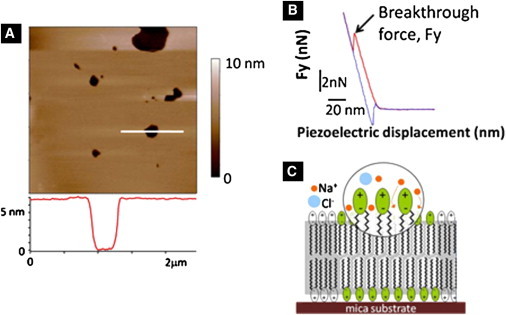
Plastic penetration of an AFM tip through a lipid bilayer serves as a fingerprint of the mechanical stability of the membrane. (A) A 5 × 5 μm² AC mode image of a supported lipid bilayer of DPPC in a liquid environment (the section profile enclosed reveals the characteristic thickness of ∼7 nm). (B) Typical force curve of a lipid bilayer. As the AFM tip approaches the surface (red trace, from right to left), the lipid bilayer is deformed until a sudden penetration occurs. (C) Schematics of the system studied. Surface planar bilayers are formed on a hydrophilic surface in the presence of the electrolyte solution. Ions present in solution specifically interact with the zwitterionic headgroups of membranes, altering their compactness and their mechanical stability.

In this study, we kept parameters such as temperature, pH, and ionic strength constant and examined the effects of different alkali and alkaline earth electrolytes. From a quantitative point of view, it is fundamentally important to understand the effect of mechanical stress in order to establish a relation between different chemical experimental environments and the resulting structure of lipid membranes. To that end, we studied the influence of electrolytes on both a liquid-like and a solid-like one-component PC bilayer on the nanomechanical FS response of SPBs. To discern the electrostatic contributions of the AFM tip and the sample to the overall mechanical deformation of the SPBs, we experimentally assessed the charge density of the AFM probe and the sample.

Materials and Methods

Materials

For the experiments, 1,2-dilauroyl-sn-glycero-3-phosphocholine (DLPC) and 1,2-dipalmitoyl-sn-glycero-3-phosphocholine (DPPC; specified as 99% pure) were purchased from Avanti Polar Lipids (Alabaster, AL) and used without further purification. EtOH, CHCl₃, MetOH, NaCl, KCl, CsCl, LiCl, tetraethylammonium chloride (TEA), CaCl_2, MgCl_2, SrCl₂, NaOH, LiOH, KOH, and CsOH were all purchased from Sigma-Aldrich (Madrid, Spain).

Sample preparation

The buffer solutions consisted of a 20 mM Hepes and 150 mM XCl solution, where X = Li⁺, Na⁺, K⁺, Cs⁺, and TEA. All buffer solutions used in this work were set at pH = 7.4, using XOH, with X being the monovalent ion present in the buffer. In the TEA solution, the pH was adjusted with NaOH. All buffers were prepared with ultrapure water (MilliQ reverse osmosis system), with 18.3 mΩ·cm resistivity, and filtered before use with an inorganic membrane filter (0.2 μm pore size; Whatman International Ltd., Maidstone, UK).

To prepare liposomes in solution, we dissolved phospholipids in chloroform/methanol (3:1) to obtain a final phospholipid concentration of 3 mM. An aliquot was poured into a glass vial and evaporated to dryness with a nitrogen flow. The resulting thin lipid film was kept under reduced pressure overnight to ensure the absence of organic solvent traces. Then, multilamellar vesicles were obtained by hydration with buffer to obtain a final phospholipid concentration of 500 μM, with the vial subjected to 30-s cycles of vortexing and temperature. The solutions were finally sonicated for 25 min, always protected from light, to obtain unilamellar vesicles. Circular mica surfaces (Ted Pella, Redding, CA) were used as a substrate for the AFM experiments. Before use, the mica surfaces were glued onto Teflon discs with a water-insoluble mounting wax. A 50 μL of the corresponding liposome suspension with the specific halide salt were applied to cover the freshly cleaved mica for a deposition time of 35 min. The mica was then rinsed four times with 100 μL of the corresponding ionic aqueous solution.

ξ-Potential measurements

The ξ-potential values were measured with a Zetasizer Nano ZS (Malvern Instruments Ltd., Worcestershire, UK). We performed ξ-potential measurements three times for each sample and calculated the standard deviation. The equipment measures the autocorrelation function of the scattered light, with the electrophoretic mobility and the ξ-potential obtained through the Henry equation.

AFM imaging and FS measurements

AFM images and FS plots were obtained with the use of an MFP-3D (Asylum Research, Santa Barbara, CA). We performed all of the measurements at constant temperature (25°C ± 0.1°C) using an in-house-made heater controller. Basically, the temperature setup consisted of a resistor placed beneath the sample holder. We measured the real temperature in the sample solution using a thermocouple thermometer (Cole-Parmer, Vernon Hills, IL). We then measured the temperature difference between the resistor and the sample surface, and calibrated the system. This step proved to be very important because the temperature can drop several degrees from the resistor to the mica surface due to the Teflon epoxy layers. The tips used were V-shaped Si₃N₄ (OTR8; Veeco, Camarillo, CA) with a nominal spring constant of 0.57 N·m⁻¹. For the FS experiments, we calibrated individual spring constants using the equipartition theorem (thermal noise routine (39)) after we correctly measured the piezo sensitivity (V·m⁻¹) by measuring it at high voltages after several minutes of performing force plots on a clean mica surface to avoid hysteresis. We used the same constant velocity (1 μm·s⁻¹) in all of the experiments. To measure the surface charge density of the silicon nitride tips, we obtained force curves with a silicon nitride tip. The force curves were acquired on the uncoated side of another cantilever that came from the same batch that was previously glued with epoxy resin to a Teflon sheet (40). Both tips were thoroughly rinsed with milliQ water and then kept for 2 h in a UV ozone generator (PSD-UV; Novascan Technologies, Ames, IA) immediately before use. The buffer used was 150 mM NaCl, and pH was set at 7.4 with 20 mM Hepes/NaOH. We fitted the experimental force curves using the Derjaguin, Landau, Verwey, and Overbeek (DLVO) theoretical model, assuming that both tips had the same surface charge density.

Results and Discussion

SPBs mechanics as a combination of forces

To understand the mechanical interaction between the AFM probe and the lipid bilayer, one must take into account the nature of the different forces that arise in an FS experiment. At this length scale (<100 nm), and before contact is made between the tip and the sample, the main interactions are electrostatic and van der Waals forces, i.e., DLVO interactions. Furthermore, hydration and steric forces have also been described as being responsible for short-range interactions in the membranes (41). In this work, we experimentally assessed and compared the effects of different cations on the mechanical response of the membrane. For that purpose, we independently measured the DLVO forces that arose between the studied samples and the AFM probes used in our experiments.

Surface charge density (σ) of the AFM tip

To calculate the interaction forces between the sample and the tip, we experimentally calculated the σ-value of the probe. To that end, we obtained force curves on the back of a silicon nitride chip that came from the same batch as the tested AFM probe. Considering that the σ-values of both surfaces in contact are the same, and that the elastic deformation due to contact is negligible, the application of the DLVO theory is straightforward. Experimental details of this method can be found in Yin and Drelich (40). The theoretical DLVO model for a conical tip-flat substrate geometry was described by Drelich et al. (42). The tip σ-value (σ_tip) measurement must be performed at the same pH and ionic strength at which the final FS experiment will be conducted (in this case, pH = 7.4 and I = 0.15 M). Equations and further experimental details are provided in the Supporting Material. After fitting the experimental data with a DLVO theoretical model, we calculated the effective σ-value (Fig. 2), which resulted in a value of −0.040 C·m⁻². As can be seen in the graph, the theoretical force curves fit well with the experimental ones. This is a satisfactory value for a silicon nitride tip, as powder silicon nitride is known to be slightly negatively charged (40). This experimental value is close to those reported in a study by Zhmud et al. (43), in which Si₃N₄ was etched with HF, HCl, or KOH to remove the oxide layer, and is also in good agreement with results reported by Yin and Drelich (40) (∼−0.01 C·m⁻² for 1 mM KCl solution and pH ∼7.5).

Figure 2.

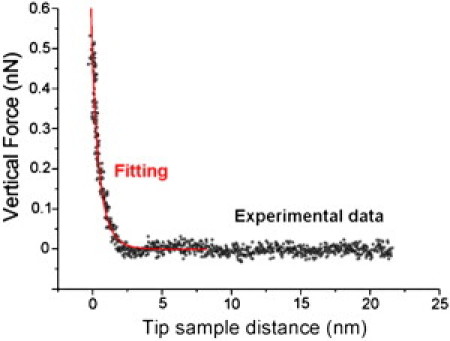
Surface charge density of an AFM tip calculation. We measure the interaction between the silicon nitride tip and an identical cantilever tip that serves as a surface under the same measuring buffer solution. To perform this calculation, we apply DLVO theory, considering that the σ-values of both surfaces in contact are the same, and that the elastic deformation due to contact is negligible. We obtain a value for a slightly negatively charged tip of −0.040 C·m⁻², which is in good agreement with other values from the literature.

Surface charge density of lipid membranes

To study the adsorption of different ions in the membrane and to calculate its σ-value, we first measured the liposome mobility in an electrophoretic field and hence the ξ-potential. Table S1 shows the experimental ξ-potential values for both DPPC and DLPC liposome solutions with the different cations in the medium. All of the studied electrolytes increase the net ξ-potential values with respect to the blank (distilled water), indicating that positive cations adsorb in the polar head of the phospholipid molecule to a greater extent than do negative ions. PC phospholipid heads are zwitterionic, so they are theoretically uncharged at neutral pH, but they actually are negatively charged when they are in solution. This is mainly due to the orientation of the phospholipid headgroups and the hydration layers that form around the headgroup-water interface (44, 45). Then, the presence of positive cations, which can enter the polar headgroups up to the phosphate moiety (6), renders a final ξ-potential value much closer to zero than in the case of cation-free solutions. In fact, divalent cations such as CaCl₂ and MgCl₂ can invert the sign of the ξ-potential and provide the liposomes with a positive surface charge. To calculate the σ-values of the liposomes from the experimentally measured ξ-potential values, the simplified Grahame equation for low potentials can be applied (46) as explained in the Supporting Material.

DLVO forces make a slight contribution to the total tip-sample interaction

According to the DLVO theory, and using the calculated σ-values of the AFM tip (σ_tip) and the σ of the SPB (σ_sample), one can calculate the DLVO interaction between the two surfaces as the tip approaches the bilayer by summing the van der Waals forces with the electrostatic forces. The theoretical model used in this study was developed by Drelich et al. (42), and allows calculation of electrostatic and van der Waals forces for a conical tip-flat substrate system. The final equations used and experimental details about the geometry of the system can be found in Fig. S1. Fig. 3 shows a set of data corresponding to the force curves obtained for DPPC and DLPC. The estimated DLVO interaction, considering our experimental data for σ_tip and σ_lipid, is shown in the inset. Whereas the experimental force values reach up to tens of nanonewtons, the calculated interaction is a slight repulsion in the piconewton range for both DLPC and DPPC for all of the studied electrolyte solutions. At these high-ionic-strength conditions, the surface charges of both the SPB and AFM tip are highly screened, so the tip-sample interaction is mostly mechanical. Therefore, it seems that experimentally measured breakthrough force values and tip-sample interactions in the contact region should be discussed in terms of mechanical deformation rather than electrostatic interaction.

Figure 3.

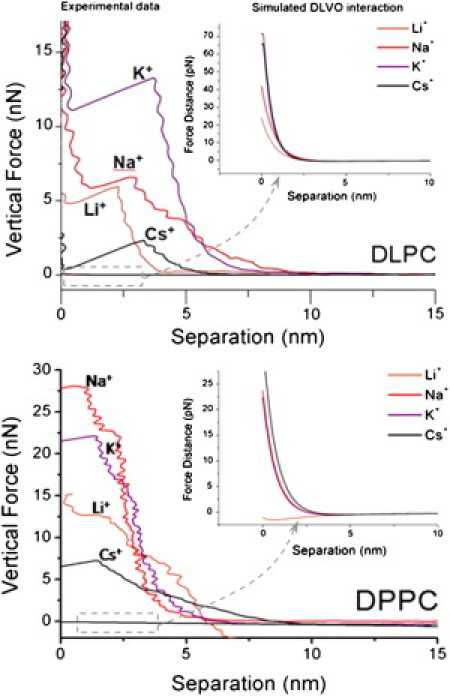
F_y versus separation curves obtained on DLPC bilayers (upper graph) and DPPC bilayers (lower graph) immersed in different buffer solutions. The X axis corresponds to sample deformation under compression. Separation = 0 nm corresponds with a hard contact between the tip and the mica substrate. The abrupt changes in separation at a certain F_y-value correspond to the tip puncturing the membrane, and the whole region between the point of F_y ∼0 nN up to the breakthrough event corresponds to the interaction between the SPB and the tip. The insets correspond to the simulated DLVO interactions considering the experimentally measured σ_tip- and σ_sample-values.

FS on lipid model membranes: DPPC and DLPC

SPBs were imaged in AC mode to ensure the presence of continuous, widespread bilayer patches. FS measurements were performed on the central part of these patches to avoid rim effects (Fig. 1 A). In all cases, force mapping or an XY closed-loop positioner was used to avoid piercing the membrane consecutively in the same location and biasing the F_y distribution. As can be seen in the section profile, the DPPC is ∼7 nm thick and the shorter DLPC molecule is ∼5 nm thick. Force curves obtained in lipid bilayers exhibit a discontinuity in the approaching force curve that is interpreted as the penetration of the AFM tip through the lipid bilayer (Fig. 1 B). The force at which this jump happens, F_y, is the maximum force the bilayer is able to withstand before breaking, and it can be regarded as the fingerprint of the bilayer stability (47), just as force is the fingerprint of a protein to be unfolded (48) or for a hard material surface to be indented (49). We performed FS experiments for both DLPC and DPPC SPBs, which differ only in the number of –CH₂ groups in the fatty acid chain. DPPC (16 –CH₂ groups per chain) has a liquid-gel transition temperature (T_m) of 42°C, so it was tested in its gel phase at room temperature. DLPC (12–CH₂ groups per chain) has a T_m = −2°C, so it was tested in its liquid phase. These two phospholipids were chosen to further elucidate the effect of electrolytes on membranes in gel and liquid-like phases.

We used the continuum nucleation model to further investigate the microscopic parameters that define the physicochemical state of the SPB. Butt and Franz (50) and Loi et al. (51) described a theoretical model that relates the force of film rupture to microscopic properties of the bilayer. According to this model, the rupture of the membrane is an activated process, with an associated energy barrier that follows the Arrhenius law. The distribution of forces necessary to create a hole under the AFM tip is closely related to the line tension (Γ), which represents the free energy associated with the unsaturated bonds of the molecules at the edge of the hole, and with the effective spreading pressure (S), which is used to quantify the tendency of the film to spread into the gap between the tip and the substrate. Even though this model simplifies the molecular nature of the membrane (which is considered a fluid in the lateral dimension), it has been shown to provide realistic quantitative numbers to explain film indentation on supported substrates under a wide variety of conditions (47, 52, 53). Fig. S2 A and Fig. S2 B show the variation of Γ and S as a function of the cation present in the buffer solution, respectively. Whereas the values for Γ do not significantly vary with the cation species, the S-values change slightly, suggesting that certain buffer solutions modify the mesoscopic behavior of the film, especially in the case of DPPC.

Alkali cations

The F_y-value is known to be a reflection of the lateral forces that bind the phospholipid molecules together (14). The challenge then is to quantify the F_y-value depending on the presence of different alkali cations in the solution, which are thought to change the lateral interactions between molecules via cohesive electrostatic interactions (Fig. 1 C). To that end, we tested DPPC and DLPC bilayers in the presence of different electrolyte solutions: NaCl, KCl, LiCl, CsCl, TEA (150 mM), and a blank (pure water). Sets of at least 500 force curves over different places of the sample were acquired. Data obtained from at least three different samples with different tips were considered to be representative results and to ensure reproducibility. At this high ionic strength, it is common to observe double jumps (∼10% of the force curves), which are commonly interpreted as the rupture of both the SPB and the bilayer formed on the surface of the AFM tip (54). This datum was excluded from results presented here. Histograms of F_y-values were fitted to a continuum nucleation model for each electrolyte solution (Fig. S3 corresponds to DPPC histograms, and Fig. S4 corresponds to DLPC histograms). The mean values of these histograms are shown in Fig. 4. One can see that the F_y-value is always higher in the case of DPPC; solid-like phases are known to show a higher F_y-value than liquid phases (19) because they show a smaller intermolecular distance. This increases the van der Waals interactions between hydrocarbon chains and also draws the polar headgroups closer, which in turn greatly increases the electrostatic attractive interactions.

Figure 4.

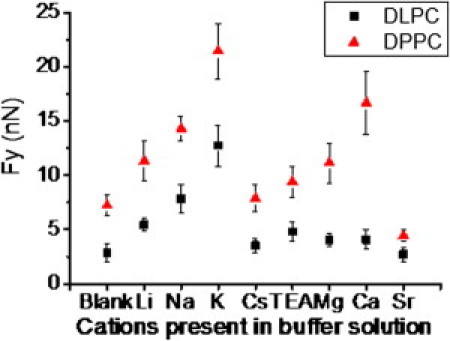
F_y-values versus ionic composition for the obtained force plots on DPPC and DLPC bilayers. Each point in the graph corresponds to the mean value of the data shown in Fig. S3 and Fig. S4. Error bars stand for standard deviation.

The F_y-value, as a hallmark of the bilayer mechanical stability, is considerably sensitive to the nature of the cations present in the electrolyte solution. This is because the cations have a strong effect on the mechanical stability of the membranes due to a reduction in the intermolecular distances in the bilayer promoted by polar headgroup screening enhancement (2). In the case of DPPC, a gel phase SPB, the maximum observed F_y-value corresponds to the presence of K⁺ (21.4 nN ± 2.6 nN), and the minimum corresponds to the presence of Cs⁺ (7.9 nN ± 1.3 nN). In the case of DLPC, a liquid-like phase, the maximum F_y-value also corresponds to the presence of K⁺ (12.7 nN ± 1.9 nN) and the lowest F_y-value corresponds to the presence of Cs⁺ (3.5 nN ± 0.6 nN). These results show that monovalent ions have an important role in bilayer ordering, i.e., they modify the mechanical properties by affecting the bilayer packing. It must be pointed out that in the case of a gel phase, the F_y-value can increase more than threefold depending on the cation present in the solution, illustrating the remarkable effect of different monovalent cations in the overall structure of the membranes. Experiments performed in pure water as a blank rendered F_y-values of 7.2 nN ± 1.0 nN for DPPC and 2.9 nN ± 0.8 nN for DLPC. To further investigate the location of these alkali cations when they interact with the lipid membranes, we also tested a cation with a significant steric hindrance, as is the case for TEA, and obtained F_y-values comparable to those reported for pure water. On the basis of these results, we propose that cation size plays a key role in adsorption between the phospholipid polar headgroups and consequently in the membrane mechanics. In fact, small ions (e.g., Li⁺) increase the SPB F_y-value with respect to pure water, but higher F_y-values are obtained for bigger ions (e.g., K⁺ for both DPPC and DLPC). In the case of extremely big ions (e.g., TEA), the F_y-value decreases again (9.4 nN ± 1.4 nN for DPPC and 4.8 nN ± 0.8 nN for DLPC). From these results, it seems clear that the cation's size and consequently its ability to enter the membrane and interact with the polar moieties play an important role in the mechanical strength of the membrane.

Our results quantitatively agree with data from a previous work that showed how Na⁺ cations adsorb more efficiently into PC membranes than other alkali cations (28), being preferentially located at the phosphate region and enabling the adsorption of Cl⁻ ions at the choline region. The influence of the cations has often been explained by means of the classical Gouy-Chapman theory, which predicts the same behavior for ions with the same valence (55). The results presented in this work undoubtedly show that same-valence cations have a remarkably different effect on the structure of the SPBs, and we discuss this taking into account both the ionic radius and the compactness of the SPB. In the case of DLPC, the F_y-value is increased more than fourfold in the presence of K⁺, whereas it is increased threefold in the case of DPPC. In reference to the compactness of the phospholipid bilayer, DPPC has a smaller area per molecule (0.63 nm² (26)) than DLPC (0.68–0.70 nm² (56)), so we propose that K⁺, being bigger than Na⁺, accommodates better between the polar headgroups when a larger area per molecule is available, as in the case of DLPC. Furthermore, although the ionic radius is important for the formation of an electrostatic network among cations, phosphodiester groups, and carbonyl oxygens in the polar moiety of PC lipids, it has to be taken into account that these hydrated cations in solution lose one or more water molecules from their coordination shells when they bind to the membrane. For example, Na⁺ ions in the interfacial region of the bilayer lose one to three hydration waters (out of approximately six) in DPPC membranes (7). With this criterion in mind, the real ionic radius of cations (i.e., the cation plus a certain number of coordinated water molecules) should be considered to have a size between that of the ionic radius and the hydrated radius of the cation. In this case, and for the series of studied alkali cations, Li⁺ and Cs⁺ do not significantly modify the mechanical properties of PC SLBs, unlike Na⁺ and K⁺. Therefore, it seems that Li⁺ and Cs⁺ do not have a specific adsorption to the bilayer polar moieties. On the basis of our experimental data, we propose that a preferential binding of K⁺ in DLPC and DPPC is due to an optimal relationship between the real cationic size and the distance between polar headgroups.

The specific adsorption of K⁺ in membranes has been widely discussed in the literature. Although some MD simulations indicate that K⁺ cations do not bind to the lipid headgroup oxygens (26), others show that K⁺ cations bind less to PC membranes than Na⁺ due to their larger radius (27), with Cs⁺ (the larger one) exhibiting the lowest interaction with the membrane (37). However, it was also reported that the K⁺ binding behavior is dependent on the force-field parameters chosen in MD simulations (28, 37). Gurtovenko and Vattulainen (28) studied the effects of NaCl and KCl in PC and phosphatidylethanolamine (PE) membranes by means of MD simulations, and concluded that the binding of KCl was much weaker than that of NaCl for PC membranes and negligible for PE membranes. Furthermore, they also noted that the effect of K⁺ was force-field-dependent, and pointed out that Gromacs force-field parameter seemed to exaggerate the size of the K⁺ ion. FS experiments can provide a new perspective regarding the effect of K⁺ in zwitterionic membranes. If we compare the mechanical stabilities of DPPC and DLPC membranes in the presence of K⁺, we can see that K⁺ promotes a variation in the lateral interactions at the molecular level. This is shown experimentally by an increase of the F_y-value in the case of DPPC (21.4 nN ± 2.6 nN) with respect to the blank test, whereas in the case of DLPC the presence of K⁺ in the solution resulted in a still further increase in the mechanical stability of the membrane (12.7 nN ± 1.9 nN).

It is well established that the F_y-value depends on the loading rate (29, 50, 51, 57). Because different cations give rise to different F_y-values at a fixed tip velocity, it is conceivable that the presence of different ions would result in a different kinetic rate for the rupture process. In a recent work, Sullan et al. (57) used the loading-rate dependence of the F_y-value to calculate differences in the activation energy for the rupture process as a function of the cholesterol content. To test the rate dependence of the F_y-value, we measured the experimental F_y-value as a function of three different loading rates (0.1, 1, and 10 μm·s⁻¹; Fig. S5). We provide data for both DPPC and DLPC in the presence of Na⁺ and K⁺. In both cases, the F_y rate dependence is flatter for Na⁺ than for K⁺. These results confirm that both Na⁺ and K⁺ modify the kinetics of the rupture process, leading to different activation energies. This is in accordance with the continuum nucleation model, where the lipid bilayer rupture is described as a two-state process with a single energy barrier.

Alkaline earth cations: Ca²⁺ and Mg²⁺

We also performed SPB FS experiments in Ca²⁺, Mg²⁺, and Sr²⁺ solutions containing 50 mM MgCl₂, 50 mM of CaCl₂, and 50 mM SrCl₂, respectively, so as to match the ionic strength present in the experiments with alkaline cations and enable comparison with the nanomechanical results. As before, we studied two phospholipidic systems: DPPC and DLPC. The corresponding histograms of F_y-values are shown in Fig. S3 and Fig. S4, and summarized in Fig. 4. It can be observed, as in the case of the studied alkaline series, that higher F_y-values are always obtained for the solid-like system (DPPC) than for the liquid-like phase (DLPC).

In the case of DPPC, the maximum mean F_y-value is achieved in the presence of CaCl₂ (16.7 nN ± 2.9 nN), followed by MgCl₂ (11.1 nN ± 1.8 nN) and SrCl₂ (4.4 nN ± 0.6 nN). Concerning the DLPC bilayers, the maximum mean F_y-value corresponds to 4.1 nN ± 0.9 nN in the presence of CaCl₂, followed by a very close value for Mg²⁺-containing buffer (4.0 nN ± 0.6 nN) and finally 2.7 nN ± 0.7 nN for the SrCl₂ solution. Sr²⁺ makes a negligible contribution to the F_y-value, similar to that of Cs⁺, TEA, or pure water. Because Sr²⁺ is larger than Cs⁺, it is expected to have a minimal influence on the nanomechanical behavior of the lipid membranes. Hence, it seems that Sr²⁺ has a practically null adsorption in the phospholipid bilayer. Concerning Ca²⁺ and Mg²⁺ cations, specific effects may be noteworthy because of their physiological relevance. Interestingly, Ca²⁺ makes a greater contribution to the lateral cohesive forces involved in lipid membranes than does Mg²⁺ in the case of DPPC. In the case of DLPC, both cations make virtually the same contribution to the mechanics of the SPB. Keeping our discussion in terms of cations having an optimal radius to sterically fit in each lipid membrane, the Ca²⁺ cations, with an intermediate size between those of Na⁺ and K⁺, properly fit in both DPPC and DLPC intermolecular distances. Nevertheless, Mg²⁺, being smaller than Ca²⁺, provides a weak improvement of the lateral cohesion in DPPC bilayers. It is worth noting that Mg²⁺ has an ionic radius even smaller than that of Li⁺. As discussed above, Li⁺ contributes weakly to the stability of the studied lipid membrane, demonstrating that the effect of the cations depends not only on their specific radius but also on their electric charge.

It was previously noted that the F_y-value has a strong dependence on the ionic strength (2) (i.e., the higher the ionic strength, the higher the F_y-value). To compare the effects of the studied monovalent and divalent cations, we tested DPPC and DLPC in the presence of 150 mM of Ca²⁺, Mg²⁺, and Sr²⁺, which is the same salt concentration used to study the effect of monovalent cations (Fig. 5). In the case of Mg²⁺ and Ca²⁺, higher F_y-values are observed for both DPPC and DLPC membranes compared with monovalent cations. This is an expected result because of the increase in lateral interactions promoted by divalent cations (i.e., a higher phospholipid-phospholipid interaction, and decrease in area per lipid). Even though the F_y-values are always higher, the tendency for this high ionic strength is the same as in the case of 50 mM electrolyte: for DPPC, the maximum value is in the presence of Ca²⁺ (29.8 nN ± 1.7 nN), followed by Mg²⁺ (17.8 nN ± 3.1 nN). In the case of DLPC, the maximum force is observed in the presence of Mg²⁺ (10.7 nN ± 0.9 nN), followed by Ca²⁺ (7.4 nN ± 0.9 nN). Regarding the Sr²⁺ cation, we can see that its interaction with the lipid bilayers is minimal. Although higher F_y-values are achieved in the presence of a 150 mM SrCl₂ solution, this does not imply a significant contribution to the overall mechanical resistance of the membrane. Taken together, these results suggest a picture in which the biological relevance of Mg²⁺ and Ca²⁺ is related to the peculiarity conferred by their ionic radius, electric charge, and ionic strength.

Figure 5.

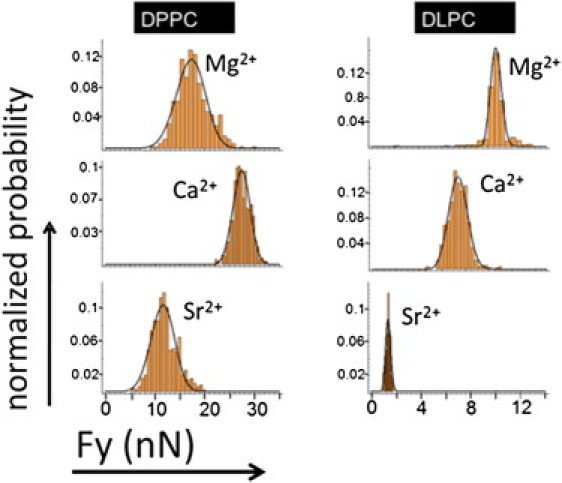
Histograms corresponding to the F_y-values for DPPC bilayers (left column) and DLPC (right column) in the presence of 150 mM Mg²⁺, Ca²⁺, and Sr²⁺. The black solid line represents the fitting to the continuum nucleation model.

Conclusions

In this work, we performed a detailed, experimental, quantitative FS study of the effect of monovalent and divalent cations on the nanomechanics of model PC membranes. We also examined the effect of these cations on the internal structure of phospholipid membranes, and to our knowledge obtained new data to characterize the interplay between ordering and (nano)mechanical stability. We experimentally proved that the presence of ions has a noticeable effect on the vertical force needed to puncture PC SPBs with an AFM probe. In the context of the DLVO theory, interaction forces arising between the SPB surface and the AFM tip have been experimentally shown to be mainly due to the mechanical resistance of the lipid bilayer, whereas the contribution of the direct electrostatic interaction between both interfaces is almost negligible.

Our results indicate that cations of different charge and size modify the nanomechanical properties of zwitterionic phospholipid bilayers, suggesting that these two parameters are crucial to explain the resilience of membranes. In the case of alkali cations, the F_y-value is maximal in the presence of K⁺ for DPPC (increased threefold) and DLPC (increased more than fourfold) SPBs. We propose that high F_y-values correspond to a tight phospholipid packing. Accordingly, the penetration of the cations on the polar moiety of the membrane and the consequent decrease in intermolecular distances are controlled by the cations' charge density and hydration, as well as by the initial distance between the SPB phospholipids. We have experimentally proved that K⁺ contributes to the overall mechanical stability in both DPPC and DLPC bilayers, an issue that has been somewhat controversial in the literature.

In the case of divalent cations, Ca²⁺, with an ionic radius between those of Na⁺ and K⁺, greatly increases the F_y-value for DPPC SPBs, whereas Mg²⁺ is too small to effectively reduce the intermolecular distances between individual phospholipids. In the case of DLPC SPBs, all of the tested divalent cations proved to be too small to modify the resilience of the membrane at the same ionic strength as the monovalent ions. When the ionic strength of these divalent cations was further increased to 150 mM (i.e., the same concentration as the studied monovalent cations, but with a fourfold higher ionic strength), the nanomechanical stability of both gel and liquid-like membranes was greatly increased in the presence of both Ca²⁺ and Mg²⁺. For DPPC, the maximum F_y-value was obtained in the presence of Ca²⁺, whereas in the case of DLPC the maximum F_y-value was obtained in the presence of Mg²⁺. This confirms the idea that ionic strength, ionic radius, and electric charge are decisive factors in the mechanics of bilayers. Together, our results confirm the notion that there are several physicochemical mechanisms that contribute to determine ion-specific adsorption to SPBs. The nature, size, and charge density of the metallic cation directly influence the penetration of the ions into the polar region of PC membranes. In addition, the intermolecular distance of phospholipids in the bilayer contributes to a preferential ionic adsorption according to the real size of the cations in solution.

Editor: Lukas K. Tamm.