Dosing algorithm to target a predefined AUC in patients with primary central nervous system lymphoma receiving high dose methotrexate

Abstract

AIM

There is no consensus regarding optimal dosing of high dose methotrexate (HDMTX) in patients with primary CNS lymphoma. Our aim was to develop a convenient dosing algorithm to target AUC_MTX in the range between 1000 and 1100 µmol l⁻¹ h.

METHODS

A population covariate model from a pooled dataset of 131 patients receiving HDMTX was used to simulate concentration–time curves of 10 000 patients and test the efficacy of a dosing algorithm based on 24 h MTX plasma concentrations to target the prespecified AUC_MTX. These data simulations included interindividual, interoccasion and residual unidentified variability. Patients received a total of four simulated cycles of HDMTX and adjusted MTX dosages were given for cycles two to four.

RESULTS

The dosing algorithm proposes MTX dose adaptations ranging from +75% in patients with MTX C₂₄ < 0.5 µmol l⁻¹ up to −35% in patients with MTX C₂₄ > 12 µmol l⁻¹. The proposed dosing algorithm resulted in a marked improvement of the proportion of patients within the AUC_MTX target between 1000 and 1100 µmol l⁻¹ h (11% with standard MTX dose, 35% with the adjusted dose) and a marked reduction of the interindividual variability of MTX exposure.

CONCLUSIONS

A simple and practical dosing algorithm for HDMTX has been developed based on MTX 24 h plasma concentrations, and its potential efficacy in improving the proportion of patients within a prespecified target AUC_MTX and reducing the interindividual variability of MTX exposure has been shown by data simulations. The clinical benefit of this dosing algorithm should be assessed in patients with primary central nervous system lymphoma (PCNSL).

WHAT IS ALREADY KNOWN ABOUT THIS SUBJECT

• High dose methotrexate (HDMTX) is the most effective drug in treating primary central nervous system lymphoma (PCNSL).

• While interoccasion variability of MTX elimination is moderate, interindividual variability is considerable and unpredictable.

• MTX dose intensity is important in patients with PCNSL to allow for an optimal clinical outcome.

• No dosing algorithm has yet been defined to individualize HDMTX dose and allow for targeting a prespecified dose intensity of the drug in patients with PCNSL.

WHAT THIS STUDY ADDS

• The present simulation study shows that a simple and practical dosing algorithm is able to improve the proportion of patients within a prespecified target AUC_MTX.

• Using this dosing algorithm, 71% of the patients received a MTX dose that was higher than the standard (500 mg m⁻² over 15 min followed by 3000 mg m⁻² over 3 h), while 11% of the patients received a dose that was lower than standard.

Introduction

High dose methotrexate (HDMTX) is widely accepted as the most effective anticancer drug for either upfront [1] or relapsed [2] primary central nervous system lymphoma (PCNSL). However, various dosing strategies have been adopted in clinical trials, highlighting the general lack of a consensus with regards to the optimal dosing of HDMTX in this group of patients. As a result, available reports in the literature describe the use of MTX at doses ranging from 1 to 8 g m⁻²[3–11], and this is basically a result of the lack of specific MTX dose-finding studies in patients with PCNSL [9, 11]. The pharmacology of MTX is characterized by rapid distribution after i.v. administration, prolonged penetration to the CNS and mainly renal elimination with a terminal half-life of 8 to 15 h with high doses of the drug. Although excretion of MTX can exhibit some nonlinearity at lower doses or in the case of third-space accumulation [12], MTX excretion is essentially linear at medium or high doses [13–16]. Furthermore, treatment with HDMTX is complicated by marked interindividual variability in drug elimination, partly related to renal function [17], third-space accumulation of the drug [12] and drug–drug interactions [17], justifying therapeutic drug monitoring (TDM) to guide individual leucovorin rescue [18]. Over the last years, data from two retrospective clinical studies have suggested that MTX dose intensity in patients with PCNSL may be important to improve treatment activity. More specifically, a target area under the concentration–time curve of MTX (AUC_MTX) of at least 1000 µmol l⁻¹ h has been suggested to allow for optimal clinical outcome [19, 20]. Apart from the fact that there are also clinical studies that have questioned the predictive value of AUC_MTX for clinical outcome [21], any reduction of the substantial interindividual variability of MTX elimination (fast and slow MTX eliminators) would be beneficial, as it would be expected to result in less unpredictable toxicity and avoid treatment failure due to inadequate CNS penetration of the drug.

The use of Monte Carlo like data simulations based on previously developed population PK models is a widely accepted and useful method to test new dosing regimens of a certain drug [22–24], as has been previously described for gentamicin in elderly patients [25] or paclitaxel in patients with liver dysfunction [26]. The results of such population analyses provide the information required to individualize dosing regimens or assess the quantitative impact of changes in covariates on the pharmacology of the respective drug. The aim of this study was to build a population PK model of HDMTX in a pooled dataset, build a simple and practical dosing algorithm and use extensive data simulations to test the efficacy of the latter to target a prespecified AUC_MTX and lower the interindividual variability of drug exposure. To be easily implemented in daily clinical practice, the dose adaptation strategy should preferably be based on the existing TDM sampling strategy to guide leucovorin rescue. Furthermore, a simple nomogram or dosing table would be preferred over more sophisticated Bayesian prediction algorithms.

Methods

Patients and sampling procedure

Methotrexate concentration–time data of 55 patients with PCNSL from the International Extranodal Lymphoma Study Group (IELSG) no. 20 trial, randomized to HD-MTX (n = 30) or HDMTX and high dose cytarabine (n = 25), underwent population pharmacokinetic (PK) and pharmacodynamic analysis as described previously [20]. The study was conducted according to the Declaration of Helsinki and Good Clinical Practice guidelines and was approved by each participating institution's ethics review board. All patients gave written informed consent. In the IELSG no. 20 study, patients were sampled for MTX serum concentrations every 24 h until the MTX concentration fell under the threshold concentration of 0.05 µmol l⁻¹. These data were pooled with MTX concentration–time data of a second observational study in 76 cancer patients receiving HDMTX [17], resulting in a final study population of 131 patients. Twenty-one out of the 76 patients from the latter study underwent extensive sampling at the end of the MTX infusion (C_max), 6, 8 and 12 h after the start of the MTX infusion [17]. In all patients, leucovorin rescue 15 mg m⁻² i.v. push every 6 h was started 24 h after the start of HDMTX infusion, and repeated for 12 times or until MTX serum concentrations were undetectable. After 48 h from the MTX infusion, leucovorin rescue was modified according to MTX serum concentrations.

Population pharmacokinetic analysis

Population PK analysis was performed using the nonlinear mixed-effects modelling program (NONMEM) version VII (double precision, level 1.1) [27]. Firstly, a basic PK model was developed for log-transformed MTX concentration–time data. Model selection was based on the minimum value of objective function, the precision of parameter estimates and the fit of the model to the data as approached by graphical plots. Interindividual and interoccasion variability was estimated using a proportional error model and residual unidentified variability was modelled using an additive error model. Secondly, a covariate model was assessed by testing the following covariates for their relationship with MTC clearance (CL_MTX): patient gender, age, body surface area (BSA), creatinine clearance (CL_cr) according to the Cockroft–Gault formula, assessed before the start of MTX infusion and capped at 140 ml min⁻¹ and co-medication with anticonvulsant drugs and steroids. Forward selection and backward elimination were used for covariate testing, with the significance level set at P < 0.01. The first order conditional estimation (FOCE) method was used throughout data analysis.

Data simulations to build a dosing algorithm for targeting AUC_MTX

The final covariate model of MTX concentration–time data from the dataset of 131 cancer patients was used to simulate MTX concentration–time curves for a total of 10 000 patients. These data simulations included the previously defined interindividual, interoccasion and residual unidentified variability, as well as the physiological variability for the covariates on CL_MTX. The generated MTX concentration–time curves were used to assess dosing algorithms that were based on individual MTX plasma concentrations 24 h after the start of MTX infusion (MTX C₂₄). Methotrexate dose adaptations were calculated to achieve a target AUC_MTX between 1000 and 1100 µmol l⁻¹ h as derived from two previous clinical studies [19, 20]. This target range was suggested to be a reasonable compromise, as the two latter studies suggested a minimal threshold for AUC_MTX in the range of 1000 and 1100 µmol l⁻¹ h, with the potential for increased toxicity with AUC_MTX values above 1200 µmol l⁻¹ h. For this purpose, MTX C₂₄ was used to predict the individual AUC_MTX and MTX C₂₄ was subsequently used to calculate the individual adjusted dose of MTX in the following treatment cycle. Patients received a total of four simulated cycles of HDMTX and adjusted MTX dosages were given for cycles two to four. For the unadjusted cycle one, MTX standard dose was used (500 mg m⁻² over 15 min followed by 3000 mg m⁻² over 3 h). The efficacy and potential benefit of MTX dose adaptations was assessed by comparing the following parameters between the unadjusted HDMTX cycle one and dose adaptations in cycles two to four: Median AUC_MTX with the 95% confidence interval, proportion of patients with an AUC_MTX within the range of 1050 ± 100 µmol l⁻¹ h and the proportion of patients with an AUC_MTX <850 or >1150 µmol l⁻¹ h. All tests of significance were two-sided; P < 0.05 was considered statistically significant (with the exception of the covariate model building, where a significance level of P < 0.01 was used to compensate for multiple testing). Statistical analyses were performed using STATA version 11.0 (STATA Corp, College Station, Texas, U.S.).

Results

Basic and covariate methotrexate population model

A two compartment population PK model for MTX concentration–time data was developed on the pooled dataset of 131 patients (55 patients with PCNSL from the IELSG study no. 20 trial [20] and 76 patients with solid tumours [17]). The final covariate model for CL_MTX included CL_cr as defined above and BSA, as outlined in Equation 1:

(1)

According to the final population model, interindividual variability in CL_MTX was 33.9% (Table 1). Interoccasion variability (IOV) for CL_MXT was 13.3%. Body weight, patient age, gender or liver function did not explain interindividual variability and these parameters were not included in the final population model. Precision of final parameter estimates (Table 1) and goodness-of-fit plots (Figure 1) support the adequate data fit of the final model. Subsequently, parameter estimates from this basic model were used to simulate MTX concentration–time curves for 10 000 patients as outlined above. Simulations included randomized creatinine clearance (median 95 ml min⁻¹, variance 10 ml min⁻¹) and BSA (median 1.75 m², variance 0.11 m²), both using a normal distribution.

Table 1.

Final population pharmacokinetics

Parameter	Estimate	RSE	Shrinkage (%)*
Basic pharmacokinetic parameter
CLMTX (l h−1)	10.8	0.92
V1 (central MTX compartment) (l)	34.0	5.4
V2 (peripheral MTX compartment) (l)	6.3	1.6
		1.6
Q (intercompartmental clearance) (l h−1)	0.35	0.11
Interindividual variability
ω CLMTX (%)	33.9	11.0	8.8
ω V1 (%)	–	–
ωV2 (%)	35.8	19.3	16.1
ω Q (%)	–	–
Interoccasion variability Residual variability (%)	13.3	4.3
σ MTX (%)	31.8	21.8	12.7

^*Parameter shrinkage to the mean, defined as parameter standard deviation divided by ω. ω, interindividual variability; σ, residual variability; CL_MTX, clearance of methotrexate; RSE, relative standard error.

Figure 1.

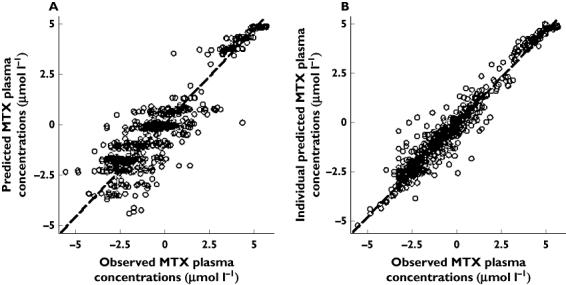
Goodness-of-fit plots of the final population pharmacokinetic model (all data log-transformed, drug concentration as µmol l⁻¹). Observed MTX concentrations vs. model predictions (A) and vs. individual Bayesian predictions (B)

Dosing algorithm based on MTX C₂₄

Correlation analysis found a strong and significant correlation between AUC_MTX and MTX C₂₄ (r = 0.81, P < 0.001) (Figure 2) as a basis for using MTX C₂₄ as a surrogate for AUC_MTX. The optimal dosing algorithm as derived from the association between AUC_MTX and MTX C₂₄ gives separate dosing recommendations for 10 categories of MTX C₂₄ (Table 2). Methotrexate dose adaptations ranged from +75% in patients with MTX C₂₄ of <0.5 µmol l⁻¹ up to −35% in patients with MTX C₂₄ > 12 µmol l⁻¹. According to this categorization, 24% of the patients had an MTX C₂₄ of 1.0 to 2.0 µmol l⁻¹, followed by 0.5 to 1.0 µmol l⁻¹ in 17.5% of the patients and 2.0 to 3.0 µmol l⁻¹ in 14.9% of the patients.

Figure 2.

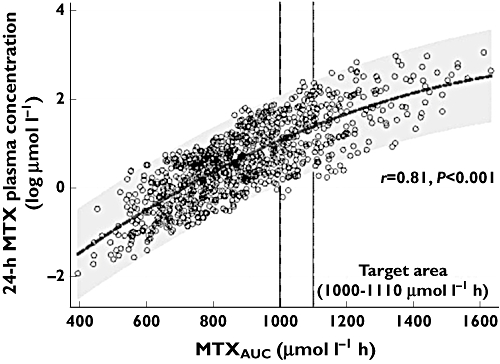
Relationship between MTX_AUC and MTX plasma concentrations at 24 h after MTX infusion

Table 2.

MTX dosing algorithm based on 24 h MTX plasma concentrations

MTX AUC (µmol l−1 h)	C24 MTX (µmol l−1)	Dose adaptation (%)	Proportion of patients (%)
C3	C4
<600	<0.5	+75	4.6
600–700	0.5–1.0	+55	17.5
700–800	1.0–2.0	+35	24.0
800–900	2.0–3.0	+15	14.9
900–1.000	3.0–4.0	+5	10.4
1.000–1.100	4.0–5.0	unchanged	7.5
1.100–1.200	5.0–7.0	–5	10.1
1.200–1.300	7.0–9.0	–15	6.1
1.300–1.400	9.0–12.0	–25	3.7
>1.400	>12.0	–35	1.2

C, cycle; AUC, area under the concentration–time curve.

Efficacy of the dosing algorithm to target AUC_MTX

Extensive data simulations based on the final population covariate model and applying MTX dose adjustments in treatment cycles two to four showed a marked reduction of the interindividual variability of AUC_MTX and a significant increase of the proportion of patients achieving the prespecified AUC_MTX target between 1000 and 1100 µmol l⁻¹ h (from 11% with MTX standard dose to 35% with the adjusted dose) (Table 3). While the median AUC_MTX was increased from an initial of 876 to 1008 µmol l⁻¹ h in cycle two, the proportion of patients with an AUC_MTX lower than 900 or higher than 1200 µmol l⁻¹ h decreased significantly from 67% with MTX standard dose to 11% with the adjusted dose. In Figure 3, the variability of AUC_MTX (3A) and MTX C₂₄ (3B) is shown over the four treatment cycles, from the first unadapted HDMTX cycle up to cycle four (MTX dose adaptations in cycles two to four). Interindividual variability of AUC_MTX markedly improved from the unadjusted cycle one to the adjusted cycles two to four (Table 3). At the same time, mean MTX C₂₄ decreased from 3.6 µmol l⁻¹ with MTX standard dose in cycle one to 3.1 µmol l⁻¹ with the adjusted dose, while the median MTX C₂₄ was unchanged at 2.2 µmol l⁻¹. Accordingly, the proportion of patients with MTX C₂₄ > 7.5 µmol l⁻¹ decreased from 12.7% with MTX standard dose to 6.8% with the adjusted dose, and the right-skewed distribution of MTX C₂₄ with standard dose converted to a more normal distribution with the adjusted dose (Figure 3B). Methotrexate dose adjustments for cycles two to four were all based on MTX standard dose, as the model did not support repeated dose adjustments in the individual patient over the cycles. The mean final dose of MTX for the 3 h infusion was 3640 mg m⁻² as compared with the standard MTX dose of 3000 mg m⁻² used in cycle one. With individualized dosing, 71% of the patients received a final MTX dose that was higher than the initial 3000 mg m⁻² over 3 h, while 11% of the patients received a dose that was lower than 3000 mg m⁻² and 7.5% of the patients received 3000 mg m⁻².

Table 3.

Results of individual Bayesian dose adjustments of HDMTX from cycle one to cycle four (MTX dosing adaptations in cycles two to four), assuming conventional daily therapeutic drug monitoring of MTX plasma concentration

Parameter	C1	C2	C3	C4
Simulated number of patients n	10 000	10 000	10000	10 000
Median AUCMTX (µmol l−1 h)	876	1 008	1013	1 012
5% percentile (µmol l−1 h)	559	877	873	861
95% percentile (µmol l−1 h)	1 387	1 164	1164	1 170
AUCMTX 1.000–1.100 µmol l−1 h (%)	11	35	34	35
AUCMTX <900 or >1.200 µmol l−1 h (%)	67	11	11	10

C, cycle; AUC, area under the concentration–time curve; MTX, methotrexate.

Figure 3.

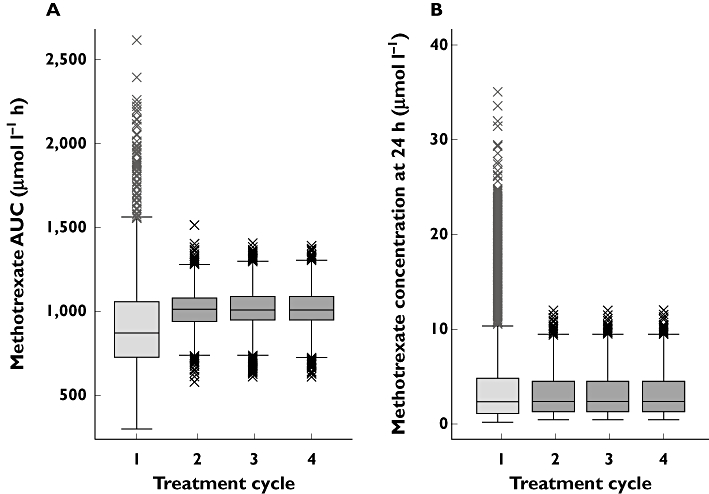
Distribution of AUC_MTX with standard MTX dosing (cycle one) and adapted MTX based on MTX 24 h plasma concentrations (cycles two to four)

Discussion

Historically, MTX is one of the few drugs in oncology where therapeutic drug monitoring is usually performed when administering MTX at high doses. Therapeutic drug monitoring in the case of HDMTX has classically been used to avoid severe toxicity by applying leucovorin rescue in patients with prolonged elimination of MTX, and so far there is no value in therapeutic drug monitoring to detect patients with fast renal elimination of MTX at risk of inferior clinical outcome [9]. Leucovorin rescue, usually started 24 h after the infusion of HDMTX, improves the therapeutic index of MTX [28], and is an important therapeutic tool to avoid severe MTX-associated toxicity such as renal dysfunction, neutropenia and mucositis [29, 30]. Although HDMTX is widely used as the backbone for treating patients with PCNSL [1, 2, 6–8, 10, 31], there are still some open issues, including the lack of a uniform dosing regimen [9], the marked interindividual variability in the elimination of MTX [17] and the fact that two retrospective clinical studies have suggested that MTX dose intensity in patients with PCNSL may be important to improve treatment activity [19, 20]. Preliminary evidence for the clinical importance of MTX pharmacokinetics came from a retrospective IELSG study of 45 patients with PCNSL, showing a minimum AUC_MTX of 1100 µmol l⁻¹ h to be significantly associated with improved overall survival [19]. In a post hoc analysis of the IELSG trial number 20, time–concentration data of MTX were submitted to population analysis to estimate pharmacokinetic parameters, random variability and covariate effects [20]. The main findings were a marked interindividual variability in drug eliminination and subsequently AUC_MTX (median 931 µmol l⁻¹ h, range 486–1710 µmol l⁻¹ h), a correlation between creatinine clearance and elimination of MTX and a gradual improvement of clinical outcome in patients with increasing drug exposure (hazard ratio of 0.82 for event-free survival per 100 µmol l⁻¹ h increase in AUC_MTX and hazard ratio of 0.73 for overall survival per 100 µmol l⁻¹ h increase in AUC_MTX). These findings were independent of study treatment or clinical risk factors and suggested that patients with rapid MTX elimination are at risk for having an inferior clinical outcome and might be good candidates for dose adjustments to increase MTX exposure. However, the relevancy of AUC_MTX as a predictive marker for clinical outcome has recently been questioned by a retrospective study of Blasco and colleagues, showing that individual AUC_MTX was not significantly lower in early non responders [21]. As a note of caution, MTX was given as an infusion over 6 to 24 h in the study by Blasco and colleagues, a regimen that is inferior to the 3 h MTX infusion and is suboptimal for CNS penetration [32]. Accordingly, a 3 h MTX infusion has been recommended by the British Committee for Standards in Haematology [33].

This study suggests that the presented simple and practical dosing algorithm based on 24 h MTX plasma concentrations is able to improve significantly the proportion of patients within a prespecified AUC_MTX target between 1000 and 1100 µmol l⁻¹ h and simultaneously reduce the proportion of patients with high MTX 24 h plasma concentrations and improve the high interindividual variability of drug exposure. The data simulations used to evaluate the proposed dosing algorithm are based on a previously described two compartimental population model [31] that has been applied to a pooled dataset of 131 cancer patients receiving HDMTX. Importantly, this result was achieved with taking into account interindividual, interoccasion and residual unexplained variability. For dose adaptations, individual MTX C₂₄ was preferred over AUC_MTX, as MTX C₂₄ is an unbiased parameter that is routinely assessed in clinical practice. On the contrary, estimation of individual AUC_MTX by using population analysis is inherently biased, as it is subject to ‘shrinkage’ towards the mean of the population, resulting in overestimation of the success rate of any dose adaptation based on AUC_MTX alone. The validity of MTX dose individualization depends on interoccasion variability that must not be markedly higher than interindividual variability. This condition is fulfilled in the case of MTX, as interoccasion variability was found to be 13%, lower than what has been described for interindividual variability (34%). A different approach has been taken by Iliadis and colleagues, who used Bayesian estimations to individualize treatment with HDMTX [34]. Bayesian estimation combines information about population characteristics and those of individuals based on previously measured plasma concentrations as the prior information. The Bayesian approach was suggested to have a high predictive value for individual elimination of MTX, but depends on local expertise for sophisticated dosing calculations. In a recent publication, Dombrowsky and colleagues describe another Bayesian forecasting algorithm that predicts individual MTX plasma concentrations in children with high precision [35]. The approach as described in the present study is different, as the proposed dosing algorithm can be applied without the need for inputing prior information other than MTX C₂₄ and without the need for maximum likelihood approximations. Furthermore, no additional sampling is necessary, since MTX C₂₄ is routinely monitored to guide leucovorin rescue.

The strengths of the present study lie in the fact that a previously described population PK model of HDMTX has been further developed in a fairly large dataset of 131 cancer patients and that extensive data simulations were used to assess the potential benefit of the developed dosing algorithm. Major limitations of this study are its retrospective and data simulation character and the fact that time-varying covariates, such as renal function, comedication and the clinical status of patients, were not taken into account, which may not reflect clinical reality. Although available data are limited for defining the optimal target AUC_MTX in patients with PCNSL, the proposed AUC_MTX target of between 1000 and 1100 µmol l⁻¹ h is supported by results of two clinical studies [19, 20].

In conclusion, a simple and practical dosing algorithm for HDMTX has been developed based on MTX 24 h plasma concentrations, and its potential efficacy in improving the proportion of patients within a prespecified target AUC_MTX and reducing the interindividual variability of MTX exposure has been shown by data simulations. The clinical benefit of this dosing algorithm should be assessed in patients with PCNSL.

Competing Interests

There are no competing interests to declare.