A Practical Multinuclear Transceiver Volume Coil for in-vivo MRI/MRS at 7T

Abstract

A practical multinuclear transceiver RF volume coil with improved efficiency for in-vivo small animal ¹H/¹³C/²³Na MR applications at the ultrahigh magnetic field of 7T is reported. In the proposed design, the coil’s resonance frequencies for ¹H and ¹³C are realized by using a traditional double-tuned approach while the resonant frequency for ²³Na, which is only some 4-MHz away from the ¹³C frequency, is tuned based upon ¹³C channel by easy-operating capacitive “frequency switches”. In contrast to the traditional triple-tuned volume coil, the volume coil with the proposed design possesses less number of resonances, which helps improve the coil efficiency and alleviate the design and operation difficulties. This coil design strategy is advantageous and well-suitable for multinuclear MR imaging and spectroscopy studies, particularly in the case where Larmor frequencies of nuclei in question are not separate enough. The prototype multinuclear coil was demonstrated in the desired unshielded design for easy construction and experiment implementation at 7T. The design method may provide a practical and robust solution to designing multinuclear RF volume coils for in-vivo MR imaging and spectroscopy at ultrahigh fields. FDTD simulations for evaluating the design and 7T MR experiment results acquired using the prototype coil are presented.

INTRODUCTION

In vivo MR imaging (MRI) and spectroscopic imaging (MRSI) at ultrahigh static magnetic fields (7T and above) is proven to be advantageous due to its intrinsically high sensitivity and improved spectral dispersion [1–10]. ¹³C and ²³Na MRSI combined with proton (¹H) imaging is a promising tool to depict metabolism process and intercellular information [11–18]. To implement this multinuclear MR technology to ultrahigh field MR for improved sensitivity, a multinuclear RF coil is essential and critical for efficient MR signal excitation and reception of multinuclei involved, ultimately realizing the ultrahigh field advantages and ensuring correct spatial co-registration of signals from different nuclei without changing coils during imaging and spectroscopy examinations.

Birdcage coil is a mature and well-established volume coil structure for in vivo MR applications at relatively low field strengths (1.5T or below) [19]. Based on this design, a variety of design approaches of double-tuned birdcage coils have been proposed for multinuclear MRI and MRSI studies. These approaches include inserting band-pass/band-stop filters [20] or LC trap circuit [21–23] into a the rungs and/or the end rings of the birdcage coil. A transformer coupled birdcage coil which consists of two coaxial birdcages has been developed as a quadrature double-tuned birdcage [24], which provides nearly ideal quadrature performance in the low frequency mode. In order to achieve simultaneous, quadrature operation for both low and high frequencies, two four-ring birdcage configurations have been presented [25]. Another method to double-tuned birdcage coil design is to tune the alternate rungs to alternate frequencies [26–28], which can be treated as two imbricate coupled low-pass birdcages.

At ultrahigh magnetic fields, the required high frequency for proton imaging poses considerable technical challenges [2,7,8,29–33] in designing RF coils based on the conventional birdcage-coil technology. Despite the cumbersome structure and some degree of design/construction difficulties, RF shielding is a commonly used approach to alleviate the low quality factors (Q-factors) and low B₁ efficiency of volume coils due to the high resonance frequency. In the design of multiple-tuned volume coils required for multinuclear MR studies at ultrahigh fields, increased interaction between proton channel and non-proton channels, ultimately degrading the efficiency on MR sensitivity for both proton and non-proton nuclei, makes the design of such multi-modal volume coil more challenging. The design of multiple-tuned multi-modal volume coil becomes even more problematic in the case where Larmor frequencies of nuclei involved, e.g. ¹H/¹⁹F and ¹³C/²³Na, are not separate enough for the volume coil to establish clearly defined multi-modal resonance for each nucleus.

In this work, we explored the feasibility of designing a multinuclear volume coil based on the birdcage-coil design for rat ¹H/¹³C/²³Na MR studies at the ultrahigh field of 7T. The proposed multinuclear volume coil was first designed to operate at 7T ¹H and ¹³C frequencies based on the alternative rung double-tuned birdcage coil technology. The third frequency for ²³Na is achieved by simply changing the capacitance on each ¹³C rung of the volume coil. This approach is feasible because Larmor frequency of ¹³C is close enough to the frequency of ²³Na, only some 4 MHz difference at 7T, and therefore the third resonance frequency for ²³Na is easy to be reached from ¹³C frequency by tuning commercial-available trimmer capacitors. The Finite difference time domain method (FDTD) was employed to predict the resonance characteristics and the B₁ field distribution. Bench test, phantom MRI and MRSI experiments were carried out to investigate the coil performance. The results demonstrated the proposed strategy for designing multinuclear volume coils for in vivo MR is advantageous in terms of MR sensitivity because it diminishes the signal losses caused by conventional multiple-tuned coils. In addition, it makes the multimodal volume coil design convenient in multinuclear MR applications in which Larmor frequencies in question are not separate enough.

METHODS

1. Design and construction of the 7T multinuclear volume coil

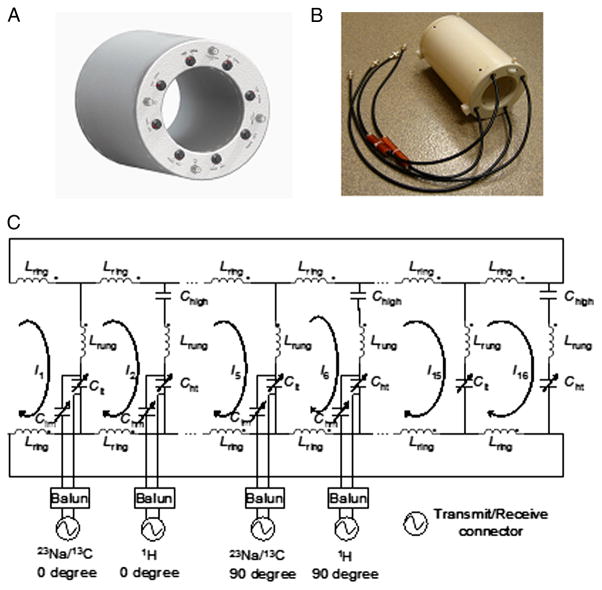
A multinuclear volume coil (Diameter: 10.16 cm, Length: 12.7 cm) showed in Fig. 1 was built with 16 copper tubes (OD: 6.35 mm, Length: 12.7 cm, 8 for each frequency). The inner diameter of the coil housing was 7.4 cm, which was the maximum usable diameter for the coil. Compared with copper foil, copper tubes would provide larger cross section area of the rungs in the limited space, which may potentially reduce the coil resistance and increase the Q factors of the coil. Whereas the copper tubes may cause certain distortions in B₀ fields, the previous works showed the distortions may not be observable in practice [2,26,34–36]. Trimmer capacitors (Voltronics, Denville, NJ) were placed between copper tubes and end-rings to facilitate fine tuning of the coil. Fixed capacitors (American Technical Ceramics, Huntington Station, NY) were placed on the other end of each element. Alternate struts were tuned to the alternate coil frequencies. The coil was driven in quadrature at both frequencies. Instead of inductive driving, in which magnetic fields generated by driving loops can disturb the B₁ field generated by RF volume coil, capacitive driving was employed in this design. Each port was matched to 50Ω while loaded with a cylindrical-shaped corn oil phantom (Diameter: 6.35 cm, Length: 15.24 cm) by matching capacitors. Because the cable braids current caused the difficulty to achieve good quadrature operation, baluns were used to suppress the cable braid current. The proposed multinuclear RF volume coil has two resonance frequencies at the same time (¹H&¹³C or ¹H&²³Na). Adjustable capacitors at the low frequency rungs of the coil were tuned to switch between different working modes, as shown in Fig. 1(a). The photo and electrical diagram of the prototype coil were shown in Fig. 1(b) and (c) respectively. In Fig. 1(c), the L_ring and L_rung denoted the inductances of the portions of the rings and the rungs respectively. C_lt and C_ht denoted the tuning capacitors of the ¹³C/²³Na and ¹H channels respectively. C_lm and C_hm denoted matching capacitors. In this coil design, C_ht, C_lm and C_hm were implemented by using trimmer capacitors (NMAP19, Voltronics, Denville, NJ) while C_lt was a variable capacitor (with a measured capacitance range of 1-20pF) connected in parallel with a 38.2-pF fixed capacitor. C_high denoted the fixed capacitors connected between copper tubes and end-rings. The capacitance of C_high was 10 pF in the prototype coil. Resonant modes for each nucleus were measured with an E5070B Network Analyzer (Agilent, Santa Clara, CA) equipped with N4431-60003 electronic calibration module.

Figure 1.

The multinuclear volume coil with quadrature-drive capability for 7T applications (a) Sketch map. (b) Prototype coil. The coil measured 10.16 cm in diameter and 12.7 cm in length and had no RF shielding utilized. (c) Electrical circuit diagram of the prototype coil.

2. Numerical modeling and FDTD simulation

FDTD was normally used to calculate RF fields generated by different RF coils including double-tuned birdcage coils[37,38]. In order to evaluate the efficiency of traditional multiple-tuned volume coil and proposed multinuclear volume coil with two resonance frequencies at the same time, both coils were modeled and simulated by using FDTD method. For comparison, the traditional triple-tuned volume coil had 24 rungs (8 for each frequency) with the same coil dimensions (Diameter: 10.16 cm, Length: 12.7 cm) as the proposed multinuclear volume coil. Different capacitors were placed at the center of each rung. The resonance frequencies of the coils were found by using a Gaussian excitation and a Fourier transform of the time domain response. Tuning was performed by changing the capacitance. All RF magnetic fields (B₁) generated by both RF coils were calculated by using the commercially available software package XFDTD (Remcom, Inc., State College, PA). The size of Yee cells, which are the basic elements of 3D meshes in the FDTD method, was 3 mm in each dimension. The simulation was run with 100,000 time-steps to ensure that the steady state was reached.

In order to confirm the simulation result of the B₁ field distribution, a bench test based on S21 measurement was implemented. The proposed coil was driven by a network analyzer and a magnetic field probe (sniffer) was employed to measure the B₁ field directly. The diameter of the sniffer’s tip was kept as small as 6.35 mm to ensure accuracy and resolution of the measurement. The B₁ field in the center slice (with 74 mm diameter) of the coil was measured with 2 mm × 2 mm resolution. A 2-axis mechanical moving table was used for accurate positioning control in two directions.

3. 7T MR experiments

A cylindrical-shaped corn oil phantom with 6.35 cm diameter and 15.24 cm length was employed for MR experiments. The gap between phantom and coil was approximately 0.5 cm on each side. This gap was needed for accessories, e.g. animal holder, animal physiological monitoring system and anesthesia system when the coil is used for in-vivo imaging. The proton MR imaging and ¹³C spectroscopy experiments with the proposed multinuclear volume coil were performed on a GE 7T/90cm MRI system (GE Healthcare, Waukesha, WI). Proton image was acquired using a gradient echo (GRE) sequence with TE = 7.3 ms, TR = 500 ms, Flip angle = 30°, FOV = 10 cm x 10 cm, slice thickness=3 mm, matrix size = 256 × 256, number of excitation (NEX) =1, and bandwidth=15.63 kHz. ¹³C spectrum of corn oil was acquired with hard pulse by single shot acquisition and TR = 2 sec.

RESULTS AND DISCUSSION

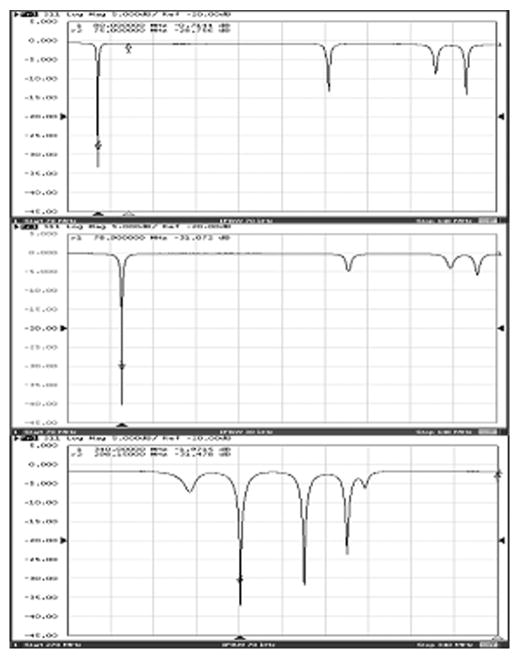
The measured frequency responses (reflection coefficient S11) of the multinuclear volume resonator were illustrated in Fig. 2 by using network analyzer E5070B (Agilent, Santa Clara, CA). All driving ports have been in good matching condition (S11 < −29 dB). The unloaded Q is ~273 for ¹³C and ~198 for ¹H by S11 measurement. Because the loading is corn oil, the loaded Q almost the same as the unloaded Q. The imaging mode (mode 1) for ¹³C MRS and ¹H MRI were tuned to 75MHz and 298MHz respectively. Well-defined resonant mode peaks for ¹³C MRS and ¹H MRI were easily identified in Fig. 2. For ¹³C channel, the frequency gap between mode 1 and mode 2 is larger than 35MHz, and for ¹H channel, the frequency gap between mode 1 and mode 2 is above 10MHz. The isolation between ¹³C channel and ¹H channel is better than −17 dB. Because the resonant frequency of ²³Na is 78.9MHz at 7T, only 3.9MHz different from the resonant frequency of ¹³C at 7T (75 MHz), it is expected to tune the ¹³C channel to resonant frequency of ²³Na easily. Because the current at lower frequency is also flowed on the rungs of higher frequency, the tuning of lower frequency will affect the higher resonant frequency too. In our case, the higher resonant frequency (for proton) increases from 298 MHz to 298.5 MHz if the lower frequency was tuned from 75MHz (¹³C) to 78.9MHz (²³Na). This kind of small resonant frequency shift can be tuned back by changing the tuning capacitor at the driving ports. The frequency response of ²³Na channel was shown in Fig. 2 (middle) and the unloaded Q is ~267. On the other hand, because high resonant frequency rungs and low resonant frequency rungs were placed alternatively, this type of symmetric design can generate relatively identical B₁ field distribution for both high resonant frequency and low resonant frequency. This kind of characteristics can gain benefit from shimming procedure for both nuclei.

Figure 2.

Measured frequency response of the multinuclear volume coil for ¹³C (top), ²³Na(middle) and ¹H (bottom) with the frequency span of 70MHz. Well-defined resonance modes for each nucleus demonstrate proposing behavior of the proposed multinuclear volume coil.

The B₁ fields of the traditional triple-tuned coil and the proposed multinuclear coil for proton frequency have been calculated by using XFDTD. All the B₁ fields were normalized to unit input power. The results (Fig. 3) shown that the proposed multinuclear RF coil, which has two resonant frequencies at the same time, has 8.8% higher efficiency than the traditional triple-tuned RF Coil.

Figure 3.

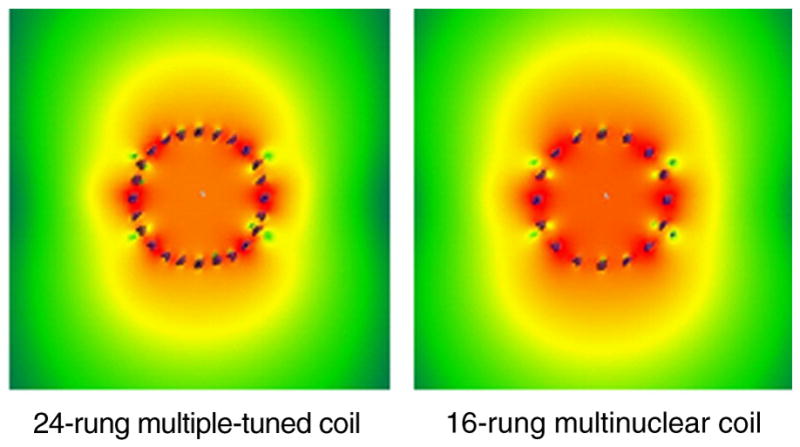
B₁ field distributions at proton frequency for traditional 24-rung multiple-tuned volume coil (left) and proposed 16-rung multinuclear volume coil with two resonance frequencies at the same time (right). Both cases were normalized to 1W net input power. The B₁ field at the center of coil increased from 6.57 μT (24-rung coil) to 7.15μT (16-rung coil).

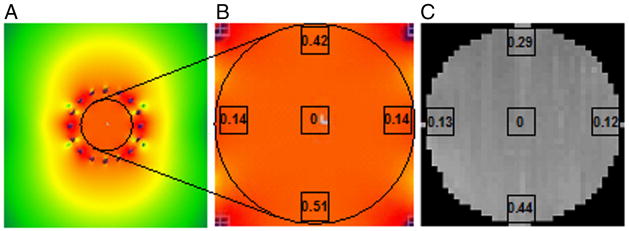
The direct measurement of B₁ field distribution was shown and compared with simulation result in Fig. 4. Fig. 4(a) and (b) showed the simulation result and zoom-in result within 74 mm diameter circle respectively. Fig. 4(c) showed the actual measurement result. Both the B₁ simulation and measurement results were normalized to 0 dB at the center of the coil. The comparison indicated good consistency between the simulation and measurement results, which confirmed the B₁ field distribution.

Figure 4.

Simulation and actual measurement results comparison. (a) simulation result; (b) zoom-in simulation result with 74 mm diameter (unit in dB); (c) actual measurement result (unit in dB). The B₁ field distribution was normalized by the B₁ at the center of the coil, which was indicated as 0 dB.

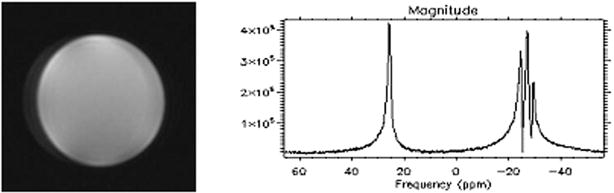
Axial GRE proton image of the cylindrical corn oil phantom acquired using the prototype multinuclear volume coil was shown in Fig. 5 (left). Because the loading of corn oil phantom was very light and the relative permittivity of corn oil was less than 3, the phantom image reflected the intrinsic B₁ field pattern generated by the prototype coil. The relatively homogeneous axial GRE phantom image in Fig. 5 (left) demonstrated the relatively homogeneous intrinsic B₁ field pattern generated by the prototype coil. Fig. 5 (right) illustrated high SNR for ¹³C spectroscopy with single shot acquisition. Based on the bench test and MRI/S experiment, the multinuclear RF volume coil was used successfully at 7T.

Figure 5.

Proton image (left) and ¹³C spectra (right) of a cylindrical corn oil phantom acquired using the multinuclear volume coil on a GE whole body 7T MR scanner. Artifacts in the proton image (left) were caused by chemical shift.

CONCLUSIONS

A practical and easy-implementing multinuclear volume coil for 7T small animal ¹H/¹³C/²³Na MR studies was designed and validated. The multi-tuned design strategy provided a simple and efficient way to overcoming the technical challenges, reducing interference between nuclear channels encountered in multinuclear volume coil designs for ultrahigh field MR. The method is also advantageous to volume coil designs for nuclei of which Larmor frequencies are not separate enough to establish a clear multi-modal performance. Although the design method proposed in this work was demonstrated for ¹H/¹³C/²³Na applications, it can also be adopted for MR applications with other nucleus combinations, e.g. ¹H/¹⁹F.

Appendix

This appendix shows a brief mathematical physics theory of the proposed coil. Based on the Kirchoff’s law, the mesh currents in the coil, as shown in Fig 1(c), satisfy

$$ZI=0$$ [A1]

in which I = [I₁, I₂, …, I₁₆], Z is the impedance matrix following

$$\{\begin{array}{l}\begin{array}{l}{Z}_{ii}=2\omega L_{\mathit{ring}}+2\omega L_{\mathit{rung}}-2\omega M_{\mathit{ring}1,\mathit{ring}2}^{(i,i)}-2\omega M_{\mathit{rung}}^{(i-1,i)}-1/(\omega C_{\mathit{low}})-1/(\omega C_{\mathit{high}})\end{array}\hfill \\ \begin{array}{l}{Z}_{ij}=\omega (M_{\mathit{rung}}^{(i,j)}+M_{\mathit{rung}}^{(i-1,j-1)}-M_{\mathit{rung}}^{(i,j-1)}-M_{\mathit{rung}}^{(i-1,j)}+M_{\mathit{ring}1,\mathit{ring}1}^{(i,j)}+M_{\mathit{ring}2,\mathit{ring}2}^{(i,j)}-M_{\mathit{ring}1,\mathit{ring}2}^{(i,j)}-M_{\mathit{ring}2,\mathit{ring}1}^{(i,j)})\end{array}\hfill \end{array}$$ [A2]

where ω is angular frequency, L_ring and L_rung are the inductances of the portions of the rings and the rungs respectively, $M_{\mathit{rung}}^{(i,j)}$ is the mutual inductance between rung i and rung j, $M_{\mathit{ring}1,\mathit{ring}1}^{(i,j)}$ and $M_{\mathit{ring}2,\mathit{ring}2}^{(i,j)}$ denote the mutual inductances between ith and jth ring segments of the top ring and the bottom ring respectively, $M_{\mathit{ring}1,\mathit{ring}2}^{(i,j)}$ is the mutual inductance between ith ring segment in the top ring and jth ring segment in the bottom ring, and C_low and C_high are the equivalent capacitances of the tuning capacitors for ²³Na/¹³C channel and ¹H channel respectively. In the cases of i = j −1, the terms $\omega M_{\mathit{rung}}^{(i,j-1)}$ in equation A2 should be modified respectively as

$$\begin{array}{l}\omega M_{\mathit{rung}}^{(i,j-1)}=\omega L_{\mathit{rung}}-1/(\omega C_{\mathit{low}}),i=1,3\cdots ,15\\ \omega M_{\mathit{rung}}^{(i,j-1)}=\omega L_{\mathit{rung}}-1/(\omega C_{\mathit{high}}),i=2,4\cdots ,16\end{array}$$ [A3]

In the case of i−1 = j, the terms $\omega M_{\mathit{rung}}^{(i-1,j)}$ in equation A2 should be modified respectively as

$$\begin{array}{l}\omega M_{\mathit{rung}}^{(i-1,j)}=\omega L_{\mathit{rung}}-1/(\omega C_{\mathit{high}}),i=1,3\cdots ,15\\ \omega M_{\mathit{rung}}^{(i-1,j)}=\omega L_{\mathit{rung}}-1/(\omega C_{\mathit{low}}),i=2,4\cdots ,16\end{array}$$ [A4]

Because of the periodicity structure of the proposed coil, the mutual inductance M^{(i, j)} in equation A2 to A4 satisfies

$$M^{(i,j)}=M^{(i+16,j+16)}$$ [A5]

The equation A2 can be modified to

$$\{\begin{array}{l}\begin{array}{l}{Z}_{ii}=2L_{\mathit{ring}}+2L_{\mathit{rung}}-2M_{\mathit{ring}1,\mathit{ring}2}^{(i,i)}-2M_{\mathit{rung}}^{(i-1,i)}-\lambda (1/C_{\mathit{low}}+1/C_{\mathit{high}})\end{array}\hfill \\ \begin{array}{l}{Z}_{ij}=M_{\mathit{rung}}^{(i,j)}+M_{\mathit{rung}}^{(i-1,j-1)}-M_{\mathit{rung}}^{(i,j-1)}-M_{\mathit{rung}}^{(i-1,j)}+M_{\mathit{ring}1,\mathit{ring}1}^{(i,j)}+M_{\mathit{ring}2,\mathit{ring}2}^{(i,j)}-M_{\mathit{ring}1,\mathit{ring}2}^{(i,j)}-M_{\mathit{ring}2,\mathit{ring}1}^{(i,j)}\end{array}\hfill \end{array}$$ [A6]

where λ =1/ω². The equations A3 and A4 are then written respectively as

$$\begin{array}{l}{M}_{\mathit{rung}}^{(i-1,j)}=L_{\mathit{rung}}-\lambda /C_{\mathit{high}},i=1,3\cdots ,15\\ M_{\mathit{rung}}^{(i-1,j)}=L_{\mathit{rung}}-\lambda /C_{\mathit{low}},i=2,4\cdots ,16\end{array}$$ [A7]

$$\begin{array}{l}{M}_{\mathit{rung}}^{(i-1,j)}=L_{\mathit{rung}}-\lambda /C_{\mathit{high}},i=1,3\cdots ,15\\ M_{\mathit{rung}}^{(i-1,j)}=L_{\mathit{rung}}-\lambda /\omega C_{\mathit{low}},i=2,4\cdots ,16\end{array}$$ [A8]

The equation A6 can be viewed as an eigenvalue problem with the variable λ. The eigenvalues λ of this problem represent the resonant frequencies of different modes and the eigenvectors I corresponding to λ represent the mesh currents in each rung. There will be two eigenvectors satisfy A9 respectively

$$\begin{array}{l}{I}_{2n-1}=A_l{e}^{j(t/\sqrt{{\lambda }_l}-2n\pi /8)},n=1,2\cdots ,8\\ I_{2n}^{\prime }=A_h{e}^{j(t/\sqrt{{\lambda }_h}-2n\pi /8)},n=1,2\cdots ,8\end{array}$$ [A9]

in which A_l and A_h are constants. These two eigenvectors I and I′ would provide homogeneity B₁ distribution desired by MRIS and MRI applications. The resonant frequencies for ²³Na/¹³C and ¹H can be calculated by the corresponding eigenvalues λ_l and λ_h.