Functional effect of longitudinal heterogeneity in constricted airways before and after lung expansion

Abstract

Heterogeneity in narrowing among individual airways is an important contributor to airway hyperresponsiveness. This paper investigates the contribution of longitudinal heterogeneity (the variability along the airway in cross-sectional area and shape) to airway resistance (R_aw). We analyzed chest high-resolution computed tomography scans of 8 asthmatic (AS) and 9 nonasthmatic (NA) subjects before and after methacholine (MCh) challenge, and after lung expansion to total lung capacity. In each subject, R_aw was calculated for 35 defined central airways with >2 mm diameter. Ignoring the area variability and noncircular shape results in an underestimation of R_aw (%U_total) that was substantial in some airways (∼50%) but generally small (median <6%). The average contribution of the underestimation of R_aw caused by longitudinal heterogeneity in the area (%U_area) to %U_total was 36%, while the rest was due to the noncircularity of the shape (%U_shape). After MCh challenge, %U_area increased in AS and NA (P < 0.05). A lung volume increase to TLC reduced %U_total and %U_area in both AS and NA (P < 0.0001, except for %U_total in AS with P < 0.01). Only in NA, %U_shape had a significant reduction after increasing lung volume to TLC (P < 0.005). %U_area was highly correlated, but not identical to the mean-normalized longitudinal heterogeneity in the cross-sectional area [CV²(A)] and %U_shape to the average eccentricity of the elliptical shape. This study demonstrates that R_aw calculated assuming a cylindrical shape and derived from an average area along its length may, in some airways, substantially underestimate R_aw. The observed changes in underestimations of R_aw with the increase in lung volume to total lung capacity may be consistent with, and contribute in part to, the differences in effects of deep inhalations in airway function between AS and NA subjects.

heterogeneity in narrowing among airways is an essential feature of asthma and has been the object of many experimental and modeling studies (1, 3, 4, 8–10, 12, 14, 22, 23, 25–27, 30). In most experimental studies, the luminal area was assessed at a single site of the airway (1, 3, 4, 12). However, given the lack of knowledge on the degree of heterogeneity in the cross-sectional area along airways and the extent to which airways narrow heterogeneously along their length, the precision of these measurements is unknown.

This longitudinal heterogeneity in the cross-sectional area when ignored could affect the estimation of airway function such as airway resistance (R_aw). This is due to the nonlinear relationship between the pressure drop and luminal area. For example, R_aw of an airway with a uniform cross-sectional area along its length is always less than that of an airway of equivalent average area but with longitudinal heterogeneity in the cross-sectional area. In addition, the shape of the cross-sectional area may also be important in the estimation of R_aw. For example, R_aw of a tube with a circular cross section is lower than that of a conduit with the same cross-sectional area but elliptical shape. Thus, disregarding these two factors, the noncircularity of the shape of the cross section, and the longitudinal heterogeneity in the cross-sectional area, should result in an underestimation in the calculation of R_aw.

It is also possible that the effect of these two factors on airway resistance calculation may be different between asthmatic (AS) airways and nonasthmatic (NA) ones. If the longitudinal heterogeneity in the cross-sectional area under bronchoconstriction is greater in AS compared with NA airways, we expect that it would accentuate the differences in R_aw between AS and NA airways.

In this study, we evaluated the underestimation of R_aw resulting from ignoring the longitudinal heterogeneity in the cross-sectional area and noncircularity of the shape at baseline, during bronchoconstriction, and after an increase in lung volume to total lung capacity (TLC). Airway dimensions were obtained from the analysis of high-resolution computed tomography (HRCT) images using previously validated three-dimensional (3D) reconstruction algorithms (11, 13, 19, 24).

METHODS

Subjects.

Images obtained from 8 mild-to-moderate asthmatic and 9 nonasthmatic adult volunteers were analyzed. Subject demographics and pulmonary function tests during screening visit, performed while subjects were in an upright position, are shown in Table 1. Subjects with mild-to-moderate asthma were selected according to the National Institutes of Health Global Initiative for Asthma (16a) with forced exhaled volume within 1 s (FEV1), or forced vital capacity (FVC), ≥ 80% predicted, less than daily symptoms, and peak flow or FEV1 variability of ≤30%. Exclusion criteria were the use of tobacco (current smokers and those with >10 pack-years) or oral steroids, symptoms of upper and lower respiratory tract infection or emergency visits or hospitalizations for asthma in the past month, and history of cardiopulmonary disease other than asthma. No systemic or inhaled corticosteroids had been used within 1 wk prior to enrollment. The study protocol was approved by the Massachusetts General Hospital-Institutional Review Board (MGH-IRB). All subjects gave their written informed consent.

Table 1.

Demographics of asthmatic and nonasthmatic subjects and PFT collected from screening in an upright position

Group	Asthmatic Subjects	Nonasthmatic Subjects
Age, yr	30.9 ± 10.9	31.7 ± 10.3
Sex (F/M)	(2/6)	(5/4)
Height, cm	174.5 ± 7.4*	165.7 ± 6.4
Weight, kg	77.8 ± 13.3*	64.9 ± 7.8
FEV1, l (% predicted)	3.50 ± 0.47 (86.7 ± 12.2%)	3.26 ± 0.74 (91.1 ± 10.5%)
FVC, l (% predicted)	4.39 ± 0.56 (93.5 ± 11.2%)	3.79 ± 0.86 (89.8 ± 10.3%)
PC20, mg/ml	1.7 ± 1.3†	>25

Values are means ± SD. Unpaired t-test comparison between asthmatic and nonasthmatic groups:

^*P < 0.05,

^†P < 0.0001.

Prior to the study date, all subjects underwent a methacholine (MCh) challenge to determine their PC20: the concentration of inhaled MCh aerosol that caused a 20% reduction in FEV1. PC20 was determined based on the method published by Crapo et al. (5). The maximum dose given to asthmatics was 8 mg/ml and all nonasthmatic subjects were given 25 mg/ml. Spirometry during the initial screening of subjects was performed while subjects were in an upright position.

Study protocol.

HRCT images were obtained with a Siemens Biograph 64 PET-CT tomograph with the subject in the supine position. In this report, we analyzed HRCT images obtained with the scanner in a helical mode with 64 slices per rotation, 0.6 mm collimation, a pitch of 1,120 kV peak, and 80 mA. The image reconstruction was done using the B31 kernel with a 0.75 mm slice thickness, 0.5 mm slice increment, and 0.25 mm overlap. Images were acquired during a short breath hold (∼20 s) at a lung volume equal to the mean lung volume (MLV) averaged over a 30-s stable breathing period prior to each scan. To guide the breath-hold maneuver, impedance plethysmography (SomnoStarPT, SensorMedics, Yorba Linda, CA) was used and a signal of instantaneous lung volume during breathing, and a reference line corresponding the MLV was presented to the subject via a head mount display. The first and second scans were acquired at baseline and after MCh challenge during a breath hold at MLV. A third scan was then acquired during TLC. We referred to the first, second, and third scan as BM (Baseline-MLV), MM (MCh-MLV) and MT (MCh-TLC) scan, respectively.

Data analysis.

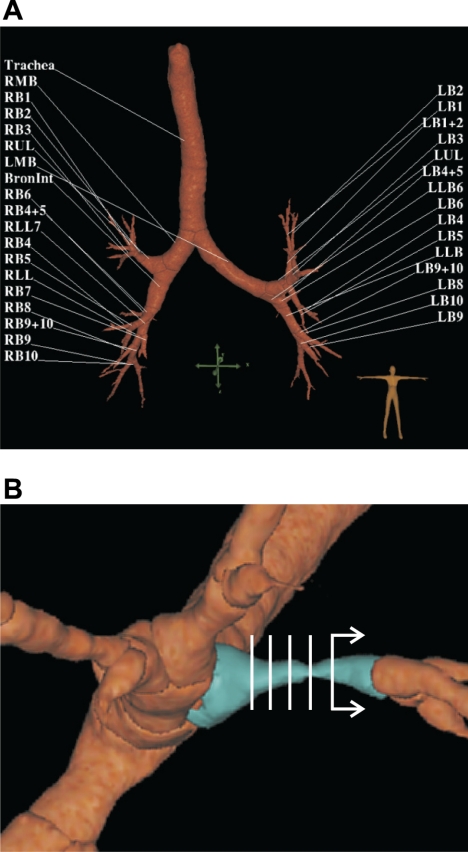
Pulmonary Workstation 2.0 (PW2) software (VIDA Diagnostics, Iowa City, IA) was used to analyze all HRCT scans, obtain 3D-rendered airway trees, and derive airway dimensions. From each scan, we analyzed 35 defined central airways (0–6th generation) with diameter >2 mm (Fig. 1A). Airways with cross sections that were not perpendicular to their centerline due to the segmentation error in PW2 were excluded in our analysis by inspection (on average ∼1–2 airways per subject). We used measurements from the middle half of the airways to minimize potential systematic errors that could have been caused if measurements near bifurcations were used. For each airway, the following parameters were imported into MATLAB (Mathworks, Natick, MA): 1) the major and minor radii (a_i and b_i) for five airway lumen cross sections (i) equally spaced over the middle half of each airway (Fig. 1B), 2) the average luminal area (A_avg), assumed as the average of the five elliptical sections of radii a_i and b_i, and 3) the airway length (L).

Fig. 1.

3D rendered airway tree of an asthmatic subject after MCh challenge imaged at mean lung volume (MLV). A: labels of all 35 defined central airways (0–6th generation) that were included in our analysis. B: a close-up of an airway (in blue) illustrating the presence of large longitudinal heterogeneity in the cross-sectional area. To calculate the resistance of 3 airway models (see Estimation of airway resistance to flow and Fig. 2), dimensions of the 5 luminal cross sections (in white) equally spaced over the middle half of each airway were used. See Data analysis for an explanation.

Estimation of airway resistance to flow.

Using HRCT images, for each airway we computed the resistance of three airway models (Fig. 2) assuming laminar flow as follows.

Fig. 2.

Three models of the airway used for calculating 3 airway resistances [average, total, and airway (R_avg, R_T, and R_A)]. A: model of a cylindrical airway with a constant radius of r_avg and total length of L used for computing R_avg. B: model of an airway with both longitudinal variability in the cross-sectional area and noncircularity of the cross-sectional shape used for computing R_T. The cross section was assumed elliptical with a major and minor radius of a_i and b_i and the segmental length of l_i. C: model of an airway with circular cross sections with the radius r_i and the longitudinal variability in the cross-sectional area used for computing R_A.

1) R_avg:

the resistance of a cylindrical airway with the average radius (r_avg) and L was computed as,

$$R_{\text{avg}}=\frac{8\mu L}{\pi {\text{r}}_{\text{avg}}^4}$$ (1)

where μ is the viscosity of air and,

$${\text{r}}_{\text{avg}}=\sqrt{\frac{{\text{A}}_{\text{avg}}}{\pi }}=\sqrt{\frac{1}{5}{\displaystyle \sum _{i=1}^5{a}_i{b}_i}}$$ (2)

2) R_T:

the total resistance of an airway made of 5 segments (i = 1, 2,…, 5), each of which had the length l_i = L/5 and elliptical cross section with major and minor radii of a_i and b_i. Note that a_i and b_i varied along the airway length. R_T was computed as the sum of resistances of each elliptical segment as follows,

$$R_{\text{T}}={\displaystyle \sum _{i=1}^5\frac{4\mu {\text{l}}_{\text{i}}\left[{\left(a_{\text{i}}/b_{\text{i}}\right)}^2+1\right]}{\pi a_{\text{i}}^{\text{3}}{b}_{\text{i}}}}$$ (3)

3) R_A:

the resistance of an airway made of five segments (i = 1, 2,…, 5), each of which had the length l_i = L/5 and circular cross section with radius of r_i that could vary along the length. To ensure that the circular cross-sectional area was equal to that of the elliptical one, r_i was set to,

$$r_{\text{i}}=\sqrt{a_{\text{i}}{b}_{\text{i}}}$$ (4)

R_A was computed as,

$$R_{\text{A}}={\displaystyle \sum _{\text{i}=1}^5\frac{8\mu l_{\text{i}}}{\pi r_{\text{i}}^{\text{4}}}}$$ (5)

Quantifying the functional effect of longitudinal heterogeneity.

The underestimation of R_aw could result from the longitudinal heterogeneity in the area and the noncircularity of the shape of its cross section. The relative difference between R_T and R_avg was an estimated error when the airway was assumed cylindrical with an average airway luminal area. This relative difference was defined as the total underestimation of R_aw due to longitudinal heterogeneity in area and the noncircular shape termed %U_total.

$$\%{\text{U}}_{\text{total}}=\%\frac{{\text{R}}_{\text{T}}-R_{\text{avg}}}{R_{\text{T}}}$$ (6)

The difference between R_A and R_avg relative to R_T was the underestimation of R_aw of an airway assumed to have a circular cross section when longitudinal heterogeneity in area was ignored (%U_area).

$$\%{\text{U}}_{\text{area}}=\%\frac{R_{\text{A}}-R_{\text{avg}}}{R_{\text{T}}}$$ (7)

The underestimation of R_aw that was caused by the noncircular shape of the cross section (%U_shape) was defined as the difference between %U_total and %U_area.

$$\%{\text{U}}_{\text{shape}}=\%{\text{U}}_{\text{total}}-\%{\text{U}}_{\text{area}}$$ (8)

Factors affecting the underestimation of R_aw.

We evaluated the contribution of two physical factors to the underestimations of R_aw: 1) the longitudinal heterogeneity in the cross-sectional area quantified by the square of the coefficient of variation in cross-sectional area [CV²(A)] and 2) the noncircularity of the cross section, quantified by the average eccentricity (ε) of five elliptical cross sections.

$$\epsilon =\frac{1}{5}{\displaystyle \sum _{\text{i}=1}^5\sqrt{1-{\left(\frac{b_{\text{i}}}{a_{\text{i}}}\right)}^2}}$$ (9)

Because of the quadratic dependence of R_aw on the inverse of the cross-sectional area, if R_aw is estimated assuming a constant average area, it should underestimate the true R_aw in proportion to CV²(A). Because the distributions of %U_area on CV²(A) did not follow normal distributions, we evaluated the dependence between the log-transformed variables as the goodness of fit coefficient (R²) of the a linear regression model:

$$\log \left(\%{\text{U}}_{\text{area}}\right)={\text{k}}_0+{\text{k}}_1·\log \left[{\text{CV}}^2\left(\text{A}\right)\right]$$ (10)

The shear stress resisting fluid flow through a highly noncircular cross section (ε close to 1) is larger than that through a circular cross section of the same cross-sectional area (ε = 0), and thus, the resistance to flow through a tube with noncircular cross section must be higher than that through a tube with circular cross section. Since ε followed the normal distribution while %U_shape did not, we evaluated the dependence of log(%U_shape) on ε in terms of the goodness of fit coefficient of the simple linear regression model to the data:

$$\log \left(\%{\text{U}}_{\text{shape}}\right)={\text{k}}_2+{\text{k}}_3·\epsilon$$ (11)

Statistical analysis.

%U_total, %U_area, and %U_shape from three scans for each subject were reported as the median ± SD (range: minimum–maximum). All statistical analyses were performed using the statistical software package SAS 9.2 (SAS Institute, Cary, NC). Since distributions of %U_total, %U_area, and %U_shape were not normal and better resembled lognormal distributions, we analyzed effects of the group and imaging condition on the log-transformed %U_total, %U_area, and %U_shape using a two-way ANOVA with an interaction term and repeated measurements. Pairwise comparisons between BM, MM, and MT were made within each group based on the two-way ANOVA model for log(%U_total), log(%U_area), and log(%U_shape). The two-way ANOVA model yielded an estimate of the mean of the log(%Ũ_total), log(%Ũ_area), and log(%Ũ_shape) distributions for each scan per group, which were then used to compute Δ_r, the relative change in the log-transformed data, between any two scans. To quantify the change in %Ũ caused by MCh challenge, and by the lung volume increase to TLC, Δ_r was defined as log(%Ũ_MM/%Ũ_BM), and as log%Ũ_MT/%Ũ_MM, respectively. moreover, we investigated whether the effect of lung volume on CV²(A) could be different for airways of different sizes. for each subject, airways were sorted by their average inner cross-sectional area at baseline (A_BM) and divided into four quartiles. using sas, the log of the ratio of CV²(A) before and after TLC in each quartile was compared with 0 to determine if the change in CV²(A) caused by the increase in lung volume to TLC was statistically significant. for all statistical analyses, P < 0.05 was considered significant.

RESULTS

The total underestimation of resistance.

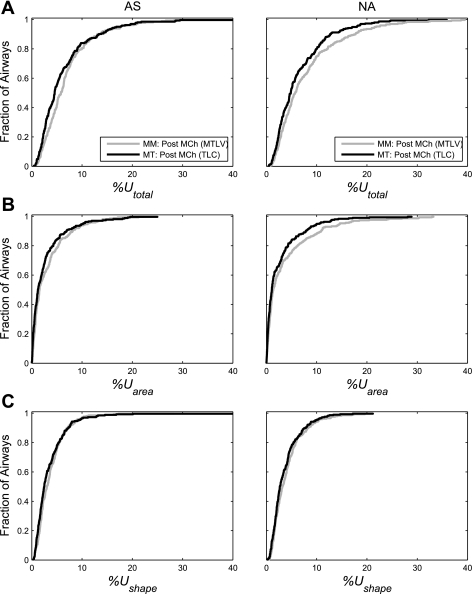
%U_total was highly variable among airways and could be substantial in some (Table 2). The median of %U_total was relatively small (<6%) (Table 2) and not significantly different between the AS and NA group in any of the three conditions imaged (Table 3). However, there was a significant effect of imaging condition on %U_total (P < 0.01; Table 3). This can be illustrated by a left shift of the cumulative distribution function (CDF) of %U_total caused by the increase in lung volume to TLC (Fig. 3A). The left shift observed was more noticeable in the NA than in AS group (Fig. 3A). As a result of the increase in lung volume to TLC, %U_total significantly decreased in both the NA group (P < 0.0001) and the AS group (P < 0.01; Table 4 and Fig. 3A). However, the change in %U_total caused by MCh challenge was not significant in either group (Table 4).

Table 2.

The statistical properties of %U_total, %U_area, and %U_shape in asthmatic and nonasthmatic subjects computed from 3 scans: baseline at MTLV, post- MCh at MTLV, and post-MCh at TLC

	Asthmatic	Nonasthmatic
%Utotal
BM	5.61% ± 5.50 (0.58–32.37%)	5.48% ± 5.79 (0.77–33.06%)
MM	5.81% ± 5.15 (0.67–29.54%)	5.73% ± 6.84 (0.53–39.45%)
MT	4.55% ± 5.90 (0.45–52.99%)	4.87% ± 5.34 (0.44–35.88%)
%Uarea
BM	1.53% ± 4.39 (0.02–28.38%)	1.33% ± 4.43 (0.02–26.36%)
MM	1.55% ± 3.78 (0.22–36%)	1.37% ± 5.67 (0.33–22%)
MT	1.23% ± 3.76 (0.01–24.97%)	1.09% ± 4.02 (0.02–28.86%)
%Ushape
BM	3.17% ± 3.00 (0.31–21.91%)	3.29% ± 3.20 (0.50–21.15%)
MM	3.01% ± 3.08 (0.36–28.22%)	3.20% ± 3.27 (0.49–20.79%)
MT	2.48% ± 4.15 (0.31–51.09%)	2.66% ± 2.99 (0.22–21.18%)

Values shown are medians ± SD with range in parentheses. %U_total, total underestimation of airway resistance due to ignoring the longitudinal heterogeneity; %U_area, the contribution to %U_total caused by longitudinal heterogeneity in the airway cross-sectional area; %U_shape, the contribution to %U_total caused by a noncircular shape; BM, baseline scan at mid-tidal lung volume (MTLV); MM, post-MCh scan at MTLV; MT, post-MCh scan at total lung capacity (TLC).

Table 3.

Two-way ANOVA with repeated measurements of log(%U_total), log(%U_area), and log(%U_shape)

	log(%Utotal)	log(%Uarea)	log(%Ushape)
Effect of group	0.3128	0.7063	0.1226
Effect of imaging condition	0.0052	0.0024	0.0478

P values are shown.

Fig. 3.

Cumulative distribution functions (CDF) of underestimations of airway resistance grouped for all airways of all asthmatic subjects (AS; left) and of nonasthmatic subjects (NA; right) after MCh challenge at mean lung volume (gray) and TLC (black). A: CDF of the total underestimation of airway resistance due to ignoring all longitudinal heterogeneity (%U_total). B: CDF of the contribution to %U_total caused by longitudinal heterogeneity in the airway cross-sectional area (%U_area). C: CDF of the contribution to %U_total caused by a noncircular shape (%U_shape). See The total underestimation of resistance for explanation.

Table 4.

P values and mean estimates of pairwise comparisons of the log-transformed %U_total, %U_area, and %U_shape within asthmatic and nonasthmatic subjects

	Asthmatic		NonAsthmatic
	P value	Δr*	P value	Δr*
log(%Utotal)
BM → MM	0.6085	1.0136	0.2519	1.0206
MM → MT	0.0067	0.8982	<0.0001	0.8835
log(%Uarea)
BM → MM	0.0075	1.8845	0.0059	1.4966
MM → MT	<0.0001	0.2965	<0.0001	0.1511
log(%Ushape)
BM → MM	0.4510	0.9666	0.2825	0.9705
MM → MT	0.0858	0.8877	0.0048	0.8900

^*Value above 1 indicates an increase in the underestimation; value below 1 indicates a decrease in the underestimation. Δ_r, is the ratio of the mean estimate of the log-transformed underestimation computed according to the ANOVA model, i.e., Δ_r = log(Ũ_MM)/log(Ũ_BM) or Δ_r = log(Ũ_MT)/log(Ũ_MM).

Contributions of %U_area and %U_shape to %U_total.

On average, %U_area contributed to 36% of %U_total with the rest contributed by %U_shape. Neither %U_area nor %U_shape was, on average, different between the AS and NA group at any of the three conditions studied (Table 3). Additionally, the two-way ANOVA showed significant effects of the imaging condition on %U_area and %U_shape in both AS and NA groups (Table 3).

The increase in lung volume to TLC resulted in a systematic left shift in the CDF of %U_area in both AS and NA (Fig. 3B). However, there were only minimal changes caused by the increase in lung volume to TLC in the CDF of %U_shape from the AS group (Fig. 3C). The reduction in the mean of the log-transformed %U_shape after the lung volume increase to TLC was significant only in the NA group (P < 0.005 with Δ_r = 0.89) from the two-way ANOVA model (Table 4 and Fig. 4C). No significant effect of MCh on %U_shape in any group was observed.

Fig. 4.

Changes in average underestimations of airway resistance caused by MCh challenge and an increase of lung volume to total lung capacity (TLC). Each point represents the average of all airways for each AS (left) and NA (right) subject. A: average underestimation of resistance caused by ignoring longitudinal heterogeneity in the airway cross-sectional area and the noncircularity of the %U_total. B: average contribution to %U_total caused by longitudinal heterogeneity in the %U_area. C: average contribution to %U_total caused by a %U_shape. BM, image taken at baseline during breath-hold at mean lung volume (MLV); MM, image taken post-MCh challenge at MLV; MT, image taken post-MCh challenge at TLC. Pairwise comparison based on the 2-way ANOVA model with repeated measurements: *P < 0.05, **P < 0.005, and ***P < 0.0001.

A significant reduction in mean log(%U_area) was observed with the increase in lung volume to TLC in both groups (P < 0.0001; Δ_r = 0.2965 for AS and 0.1511 for NA; Table 4 and Fig. 4B). A significant increase in the mean log(%U_area) was observed in both AS (P < 0.01 with Δ_r = 1.8845) and NA group (P < 0.01 with Δ_r = 1.4966; Table 4 and Fig. 4B).

Longitudinal heterogeneity in the cross-sectional area and the noncircular shape.

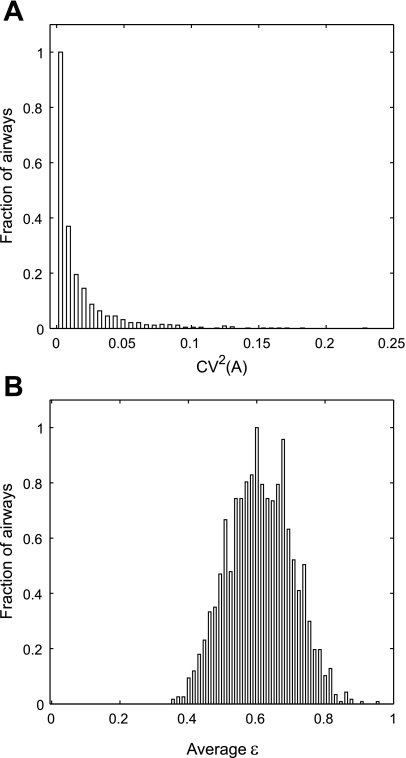
Figure 5, A and B, shows the probability distribution of CV²(A) and that of eccentricity (ε) of all airways in all subjects under three conditions. The first four moments (mean, variance, skewness, and kurtosis) of these distributions are presented in Table 5.

Fig. 5.

The distribution of the longitudinal heterogeneity in the cross-sectional area quantified by CV²(A) and the noncircularity of the shape of the airway cross section (ε). A: the distribution of CV²(A) in all airways of all subjects under 3 conditions. Skewness of this distribution was measured using statistical software, SAS, to be 3.24 with the median of 0.009. B: the distribution of eccentricity of the assumed elliptical cross section (ε). The average ε was 0.611.

Table 5.

The statistical measures of CV²(A) and ε

	Mean	Variance	Skewness	Kurtosis
CV2(A)	0.016	0.001	3.235	14.264
ε	0.611	0.009	0.054	−0.312

CV²(A), longitudinal heterogeneity of the airway cross sectional area; ε, eccentricity of the assumed-elliptical cross section.

Correlations between longitudinal variability in cross-sectional area and shape.

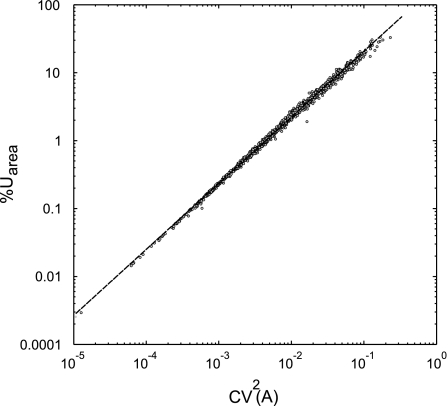
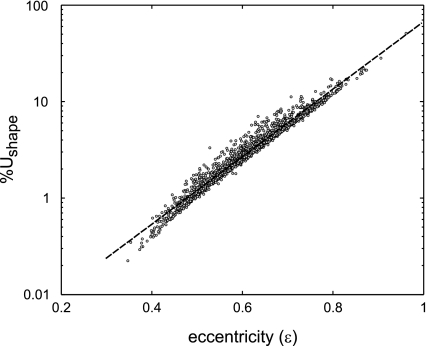
log(%U_area) was linearly related to log[CV²(A)], yielding k₀ = 2.29, and k₁= 0.97 in eq. 10(R² = 0.997; Fig. 6). Similarly, log(%U_shape) was linearly related to ε, yielding k₂ = −1.67 and k₃ = 3.50 for eq. 11 (R² = 0.966; Fig. 7).

Fig. 6.

Correlation between the contribution to %U_total caused by longitudinal heterogeneity in the %U_area and CV²(A) (the square of the coefficient of variation in the cross-sectional area along that airway). Data are taken from all airways in all subjects in all 3 imaging conditions. A least-square linear regression of the log-transformed data shows high correlation between log(%U_area) and log(CV²(A)) had a goodness of fit R² = 0.997.

Fig. 7.

Correlation between the contribution to %U_total caused by the %U_shape and ε (the eccentricity of the assumed-elliptical cross section). Data are taken from all airways in all subjects in all 3 imaging conditions. A least-square linear regression of log(%U_shape) and ε had a goodness of fit R² = 0.966.

Changes in CV²(A) for different sized airways.

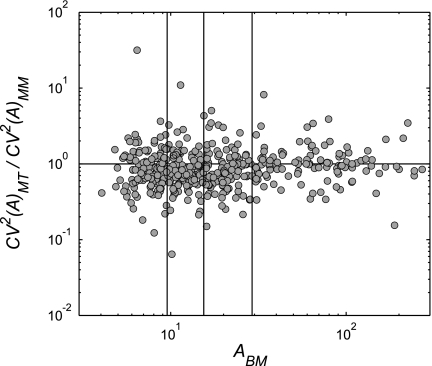
When the lung volume was increased to TLC, the average CV²(A) was significantly reduced in all but the largest airways quartile (airways with average A_BM > 29 mm²; P < 0.05; Fig. 8). However, the reduction in %U_area or in CV²(A) after the lung volume increase to TLC was not correlated with airway size or with the change in airway size (data not shown).

Fig. 8.

Changes in longitudinal heterogeneity in the cross-sectional area caused by the lung volume increase to TLC in airways of various sizes. The average luminal area of an airway at baseline (A_BM) was plotted against the ratio in longitudinal heterogeneity in the CV²(A) at TLC over that at MLV of the same airway. The plot includes all airways of all subjects. A_BM was imaged at MLV. MM, image taken after MCh challenge at MLV; MT, after MCh challenge at TLC. Airways with reduction in CV²(A) after the increase in lung volume to TLC would be below the unity line. Airways were binned into 4 quartiles with an equal number of airways (indicated by vertical lines). Note that in airways with the largest A_BM the increase in lung volume to TLC did not significantly change the longitudinal heterogeneity.

DISCUSSION

This study demonstrated that neglecting the longitudinal variability in the luminal area and the noncircularity of the cross-sectional shape results in an underestimation of R_aw. The effect was highly variable with %U_total being as high as 53% (AS) and 36% (NA) after MCh challenge at TLC. However, the median values were <5% and similar in AS and NA subjects. On average, the longitudinal heterogeneity in the cross-sectional area contributed to ∼1/3 of %U_total, while the noncircular shape contributed to ∼2/3 of %U_total. In both AS and NA subjects, the mean values of %U_total and %U_area increased after MCh challenge and reduced by elevating lung volume to TLC. After lung volume was increased to TLC, %U_shape significantly decreased in nonasthmatic subjects, but not in asthmatic subjects.

It is worth noting that, in contradiction to our initial expectation, longitudinal heterogeneity in these central airways was not higher in AS compared with NA. Indeed, we found no difference between AS and NA in the longitudinal heterogeneity in the area and/or the noncircularity of the shape. Interestingly, the effect of elevating lung volume to TLC was different in both groups in that it made the cross section more circular only in NA.

Methodology limitations.

The methodology used by PW2 was previously validated in Plexiglas phantoms of airways with diameters ranging from 1.98 to 19.25 mm [inner cross-sectional area (A) ranging from 3.08 and 291.04 mm²] (13). In the smallest tube, the contribution of imaging error to CV² in the area estimation was 0.0021. Based on those results, and given that airways analyzed in our study ranged from 4.0 to 271.3 mm², the largest expected contribution of measurement errors to CV²(A) should be <0.0013, which corresponds to 1/13 of the observed average value CV²(A) in the smallest 25% of the airways analyzed and much lower for the larger airways. Given the complexity of the airway tree airway structure in vivo, the contribution of measurement errors to CV²(A) could be somewhat higher. Nonetheless, the error was not of enough magnitude to obscure the small but significant effects of MCh or lung inflation observed.

Model assumptions.

Assumptions for estimating R_aw were steady and fully developed laminar flow, negligible gravity, incompressible fluid, and constant viscosity. In addition, we assumed the airway wall's shape and luminal area were approximately elliptical and changed smoothly along the airway, such that inertial effects or secondary flows due to these effects would be negligible. Depending on the flow rate, the airflow through trachea and large airways could be turbulent (Reynolds number > 2000). Pedley et al. (18) estimated that flow at 100 l/min through airways from the first four generations of a symmetric airway model would be turbulent. Therefore, in high-flow conditions such as during exercise, the pressure drop in large airways would be greater than that calculated assuming laminar flow, thus yielding an even greater underestimation of R_aw. However, during spontaneous breathing in adults, the flow rate is typically ∼12 l/min (20). Hence, a laminar flow assumption would be reasonable. Effects of the unsteady developing flow through the complex tree structure should result in even greater pressure drops and underestimations of R_aw. Nonetheless, independent of the flow conditions, since R_aw is a function of the cross-sectional area elevated to an exponent ≥2, results obtained from this study can be seen as a lower bound of the effect, and the pairwise comparisons should remain qualitatively valid.

Physical parameters determining %U_area and %U_shape.

The longitudinal heterogeneity in the cross-sectional area, taken as its mean-normalized variance [CV²(A)], was highly correlated to the underestimation of resistance attributed to changes in cross-sectional area (%U_area). The two parameters were not identical, or expected to be so a priori, but CV²(A) explained almost all (99.7%) variance in %U_area.

Also, sheer stress is known to be higher in an elliptical cross section compared with a circular one. Therefore, it can be expected that if the noncircularity of the cross section is ignored, R_aw should be underestimated. Our result showed that %U_shape was highly correlated with 10^3.5ε. Given these high levels of correlation, it can be concluded that results and conclusions presented in terms %U_area or %U_shape can also be applicable in terms CV²(A) and ε.

Bronchoconstriction.

MCh challenge caused an increase in the longitudinal heterogeneity in the area and %U_area in both AS and NA subjects with a higher increase in AS. We speculated that such difference in airway response between AS and NA could be due to local differences in airway responsiveness that might be larger in subjects with asthma leading to a higher increase in longitudinal heterogeneity in the cross-sectional area after MCh challenge.

Effects of lung expansion from MLV to TLC.

In both AS and NA groups, an increase in lung volume to TLC during bronchoconstriction reduced %U_total. In NA, the reduction in %U_total at TLC was the result of reductions in %U_area and %U_shape. In contrast, in asthmatics, the reduction in %U_total during the TLC maneuver was only due to the reduced %U_area but not in %U_shape. This suggested that the cross-sectional shape of a nonasthmatic airway once the lung volume increased to TLC became more circular, while that of an asthmatic airway did not. We speculate that parenchymal tethering forces acting on the airway wall could be more heterogeneous along the wall in asthmatic airways compared with nonasthmatics. If parenchymal tethering forces were homogeneous along the perimeter of the airway after lung inflation, one could expect that the airway wall would be distended symmetrically in the radial direction making the cross-section more circular and thus with a lowered eccentricity as we observed in nonasthmatic airways, but not in asthmatic ones. Therefore, our results might suggest that the lack of reduction in %U_shape and ε observed in asthmatic subjects might be due to a less homogeneous lung expansion in asthmatics particularly during bronchoconstriction.

Another explanation for this difference could be related to the reduced elastic recoil in asthmatic patients. Based on results from a computational model of airway narrowing (29) with parameters taken from actual lung tissues from AS and NA patients, Wiggs et al. (28) concluded that airway narrowing was enhanced in the presence of the reduced elastic recoil. Elastic recoil may be reduced in asthmatic patients after asthma attacks (31), during a stable period (16), or even 6 wk following successful treatments of acute attacks (6). This reduction in the elastic recoil in AS could imply a reduced magnitude of the parenchymal stress increase on the increase in lung volume, potentially making the reduction in eccentricity of the cross-sectional shape under ASM tension more difficult in AS compared with NA airways. The reduced sensitivity of the noncircular shape to lung volume increase in AS may also be compatible with differences in the behavior of airway smooth muscle postulated to explain the reduced or absent response of asthmatic lungs to a deep inhalation (7, 15, 21).

Despite observing the trend in the change in underestimations of resistance caused by the increase in lung volume to TLC, individual airways in fact behaved heterogeneously. In a large fraction of the airways (54% of AS airways and 57% of NA airways), the increase in lung volume to TLC caused a decrease in %U_area (>5% decrease), but an increase in %U_area in a substantial number of airways (33% of AS airways and 32% of NA airways with an increase in %U_area of >5%). Originally, we had expected that the high distensibility airway should have a large decrease in longitudinal heterogeneity in cross-sectional area. Surprisingly, we found no correlation between the change in the cross-sectional area caused by the lung volume increase to TLC and the change in %U_area. Therefore, the variability in the change of %U_area was not attributable to differences in airway distensibility per se, and the mechanism responsible for these effects remains elusive. Nevertheless, interesting results emerged as we investigated the reduction in the average longitudinal heterogeneity in the cross-sectional area in airways of different sizes. We found that the average longitudinal heterogeneity in the cross-sectional area was only reduced in the smallest 75% of the airways. It is possible that in the largest 25% of the airways, the relative effect of pleural pressure change or parenchymal tethering could have been small compared with the effects of stiff cartilage plates (17), which are more prevalent in large airways.

In conclusion, we demonstrated that neglecting the longitudinal heterogeneity in airway luminal area and assuming a circular cross section led to underestimations in airway resistance, which could be considerable (∼50%) in some airways, but small on average (median ∼<6%). We estimated the magnitude of the two sources responsible for the underestimation of R_aw: the variability in the cross-sectional area and the noncircularity of its shape. These sources contributed on average to 1/3 and 2/3 of the total underestimation, respectively. We found that an increase in lung volume to TLC during bronchoconstriction reduced on average the longitudinal heterogeneity in the cross-sectional area in both AS and NA airways. However, the magnitude of that effect was variable among airways and the average reduction was smaller and less consistent in AS compared with NA subjects. We speculated that loss of lung recoil, increased wall stiffness, remodeling of the airway wall, or reduced airway-parenchymal interdependence in asthmatic airways might be the cause of the lack of reduction in the noncircularity of the cross section by inhalation to TLC observed in AS subjects.

GRANTS

This research was funded by National Heart, Lung, and Blood Institute Grant HL68011.

DISCLOSURES

No conflicts of interest, financial or otherwise, are declared by the authors.

AUTHOR CONTRIBUTIONS

Author contributions: C.W. and J.G.V. conception and design of research; C.W., R.S.H., M.K., T.W., and J.G.V. performed experiments; C.W. and J.G.V. analyzed data; C.W., R.S.H., H.Z., T.W., and J.G.V. interpreted results of experiments; C.W. prepared figures; C.W. drafted manuscript; C.W., R.S.H., H.Z., M.K., T.W., and J.G.V. edited and revised manuscript; C.W., R.S.H., H.Z., M.K., T.W., and J.G.V. approved final version of manuscript.