The rising cost of warming waters: effects of temperature on the cost of swimming in fishes

Abstract

Understanding the effects of water temperature on the swimming performance of fishes is central in understanding how fish species will respond to global climate change. Metabolic cost of transport (COT)—a measure of the energy required to swim a given distance—is a key performance parameter linked to many aspects of fish life history. We develop a quantitative model to predict the effect of water temperature on COT. The model facilitates comparisons among species that differ in body size by incorporating the body mass-dependence of COT. Data from 22 fish species support the temperature and mass dependencies of COT predicted by our model, and demonstrate that modest differences in water temperature can result in substantial differences in the energetic cost of swimming.

1. Introduction

Mounting evidence suggests that water temperatures have increased in marine and freshwater habitats around the globe and will probably continue to increase in the future [1]. While projections vary, some estimates indicate that sea surface temperatures, for example, may rise by as much as 2–4°C by the end of this century [1]. Water temperature has been linked to many features of fish swimming performance, including respiratory scope and escape swimming speed [2,3]. One feature in particular—the metabolic cost of transport (COT), which describes the amount of energy used to swim a given distance—appears to increase with increasing water temperature in several species [4–6]. However, it is not clear whether the temperature-dependence of COT observed in these species is a general phenomenon that applies across diverse fish species. Moreover, a quantitative model to describe the temperature-dependence of COT based on metabolism and swimming mechanics is still lacking, making it difficult to predict how changes in water temperature might affect fish swimming energetics.

To address this issue, we develop a model to describe the temperature-dependence of COT. The model facilitates comparisons among fishes that differ in body size by incorporating the body mass-dependence of COT. It builds on work addressing the relationship between temperature, body size and maintenance metabolism [7], and on work relating temperature and body size to swimming energetics [2]. We evaluate model predictions using data from swimming experiments on 22 diverse fish species.

2. Model development

The model developed here is based on the hypothesis that COT is governed by three variables: the rate of energy expenditure devoted to swimming (R_swim, W), the rate of energy expenditure devoted to maintenance (R_std, W) and swimming speed (u, m s^–1). This approach has been used to capture the principal features of swimming energetics in previous studies [4–6,8]. The COT (in J m⁻¹) is given by:

2.1

COT is typically a u-shaped function of speed, with a minimum value (COT_min) occurring at speed, u_opt, [6]. We focus on COT_min because, for fishes swimming at steady speeds and water temperatures, it sets a lower bound on the cost of swimming. It thus provides a conservative metric for evaluating effects of changing water temperature on swimming energetics.

From the hypothesis that R_std and R_swim determine COT_min, it follows that differences in COT_min within and among species should be governed by the primary variables that determine R_std and R_swim: temperature and body mass [6,8]. Local adaptations leading to changes in variables such as red versus white muscle fibre recruitment and muscle contractile properties have the potential to influence R_swim [6], but the relative importance of these variables is not well known. We hypothesize that the relationship between COT_min and water temperature is primarily determined by the well-documented relationships between temperature, maintenance metabolism and swimming mechanics. R_std, can be described by the equation, 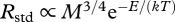, where E is the average activation energy of the respiratory complex (eV), T is body temperature (K, assumed to equal water temperature), k is Boltzmann's constant (eV/K) and M is body mass (kg) [7]. This model posits that R_std changes with temperature owing to the temperature-dependence of core metabolic processes [7]. The mass-dependence of R_std has been hypothesized to result from physical constraints on the delivery networks that regulate energy supply to cells [7], but the origin of this relationship is still debated. In the electronic supplementary material, appendix, we derive an alternative model in which the relation 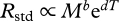is assumed and empirical estimates of b and d are used. Such a model yields similar predictions to the model presented here.

The rate of energy expenditure, R_swim, required to swim at a constant speed is proportional to the product of u and the drag experienced at speed, u [6]. Increasing temperature decreases the viscosity of water [2]. Yet the corresponding reduction in friction drag appears to have a minimal effect on fishes in the size range of most juvenile and adult fishes (i.e. Re > 10³–8.8 × 10³) [2]. We therefore hypothesize that R_swim is independent of temperature. Body mass, by contrast, strongly affects R_swim. R_swim increases with increasing body mass because larger fishes experience greater drag at a given swimming speed. Pressure drag makes the largest contribution to total drag for swimmers in the size range of most juvenile and adult fishes, suggesting that R_swim is roughly proportional to body cross-sectional area, or 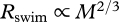, where body cross-sectional area is assumed to be proportional to mass to the two-thirds power [2]. Combining these temperature and mass dependencies with the speed-dependence of R_swim [8] shows that 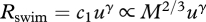, where the speed exponent, γ, describes how R_swim increases with increasing swimming speed and the variable 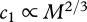is introduced for notational convenience.

The effects of temperature and mass on the variables in equation (2.1) can be used to derive the temperature- and mass-dependence of COT_min. Equation (2.1) can be solved for COT_min by the standard technique of taking the derivative with respect to u, setting the result equal to zero, solving for u_opt and substituting u_opt for u in equation (2.1) ([6,8] and electronic supplementary material, appendix). Expressing R_std and c₁ in the resulting equation as functions of mass and temperature yields:

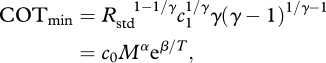2.2

where c₀ is a constant, α = 0.75–0.08γ⁻¹ and β = −(E/k)[1 − 1/γ]. Expressing COT_min as dimensionless COT (J N⁻¹ m⁻¹) does not alter the predicted temperature-dependence (see the electronic supplementary material, appendix).

The constants, E, k and γ can be independently estimated from data or theory to yield quantitative predictions about the values of the α and β parameters. Equation (2.2) thus makes two predictions about the relationship between COT_min, temperature and body mass. First, the temperature exponent, β, is described by the equation β = −(E/k)[1 − 1/γ]. Rigid body hydrodynamic models suggest that γ should be in the range of 2.5–2.8 depending on flow conditions [8]. Boltzmann's constant, k, equals 8.62 × 10⁻⁵ eV K⁻¹ [9] and empirical estimates of E suggest that E ≈ 0.41 eV [7] in fishes. The model therefore predicts that β is between –3.1 × 10³ and –2.9 × 10³. This means that fishes swimming in warmer waters should experience greater COT_min than fishes in colder waters, with the functional form of the temperature-dependence given by equation (2.2). Second, the mass-scaling exponent, #x03B1;, is given by, α = 0.75–0.08γ⁻¹. Our model therefore predicts that α = 0.72. In other words, at a given water temperature, larger fishes should experience greater COT_min than smaller fishes, with COT_min ∝ M^0.72.

3. Material and methods

To test our model, we compiled published studies that reported fish respiration rates over a range of swimming speeds from fishes using body-caudal fin gaits. We used only studies that acclimated fishes to test temperatures and maintained stable O₂ concentrations (see the electronic supplementary material, appendix).

Data collection resulted in a total of over 800 observations from 22 species (electronic supplementary material, table S1 in appendix) ranging in mass from 8.3 × 10⁻³ to 1.2 kg. Swimming respiration rates were measured at water temperatures ranging from 5°C to 30°C. To identify COT_min for each species-by-temperature combination, we converted measurements of total respiration rate into W using standard oxycaloric values (20.1 J mlO₂^–1, 14.1 J mgO₂^–1; [6]) and used nonlinear least squares to estimate COT_min for each species at each temperature (see the electronic supplementary material, appendix).

After calculating COT_min values, we fitted equation (2.2) to COT_min data to estimate α and β parameters. Prior to fitting, we ln-transformed COT_min, and M to linearize the equation (i.e. 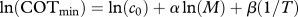). We then fitted the linearized model using generalized least squares to allow for correlation among observations taken from the same species at different temperatures.

4. Results and discussion

The model described by equation (2.2) provided a good fit to data, with mass and temperature explaining 83 per cent of the variation in COT_min (figure 1, best fit model: 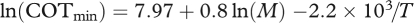). Data supported both quantitative predictions regarding effects of temperature and body mass on COT_min. The 95 per cent confidence interval (CI) for the β parameter (β_est = –2.2 × 10³, 95% CI = (–3.1 × 10³, −1.3 × 10³), p = 1 × 10⁻⁴) overlapped the predicted range (−3.1 × 10³, −2.9 × 10³), confirming that the observed effect of water temperature on COT_min matched that predicted by our model. The observed temperature-dependence is approximately equivalent to a Q₁₀ of 1.3. The 95 per cent CI of the α parameter (α_est = 0.80, 95% CI = (0.69, 0.92), p < 2 × 10⁻¹⁶) overlapped the predicted value of 0.72 (figure 1b), confirming that the observed mass-dependence also matched model predictions. COT_min depends on both temperature and mass; to display data in bivariate plots, COT_min values were normalized based on observed mass-dependence of COT_min (mass-corrected 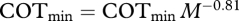; figure 1a) or observed temperature-dependence of COT_min (temperature-corrected 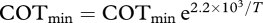; figure 1b).

Figure 1.

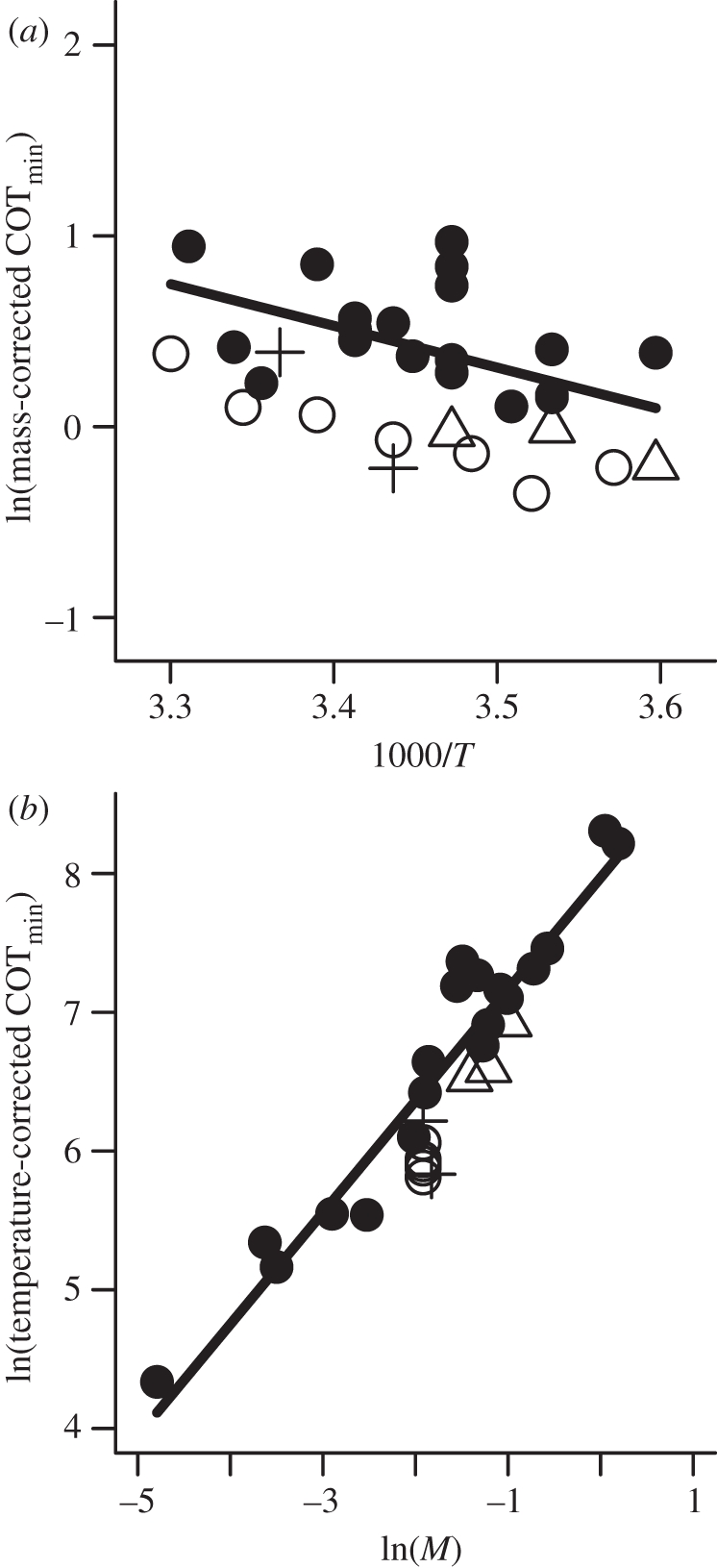
(a) Mass-corrected COT_min (see text) as a function of inverse temperature, 1000/T (K). (b) Temperature-corrected COT_min (see text) as a function of ln(M). Filled circles represent species for which a single measurement was used. Other symbols indicate species for which measurements at multiple temperatures were included (open circles, Dicentrarchus labrax; triangles, Gadus morhua; crosses, Scomber japonicus).

The consistent predictable increase in COT_min with increasing temperature demonstrates that COT_min increases with temperature among species as well as within species [5]. The strong agreement between predicted and observed values of the temperature exponent β, support our model, suggesting that the effect of temperature on COT_min may be primarily mediated by changes in R_std; changes in R_swim are not necessary to produce the observed temperature-dependence. Further support comes from the direct observation that R_swim is independent of temperature in at least one species [5]. This finding contrasts sharply with the strong effect of temperature-mediated changes in viscosity on the swimming mechanics of small larval fishes, where increasing temperature can actually decrease COT [2]. Changes in water temperature thus appear to have opposing effects on the swimming performance of different life stages.

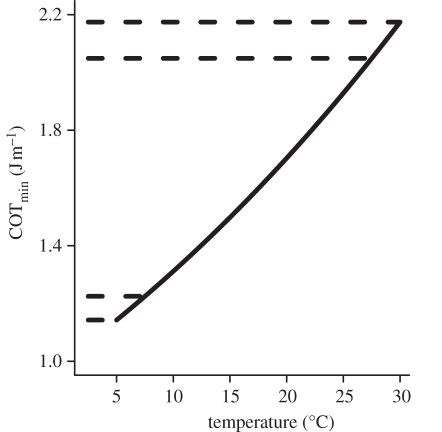
Our results lead to two general insights about the effect of water temperature on the cost of swimming. First, the model and empirical estimates of the α and β parameters can be used to predict the mean change in COT_min under particular climate change scenarios. For example, an increase in water temperature of 2–4°C [1] would lead to a 6–12% increase in the minimum amount of energy required to swim 1 m. Second, the data presented here support our assumption that COT_min changes exponentially with inverse temperature (1/K). If this is generally the case, a given increase in water temperature will elicit a larger absolute change in COT_min in warm water than in cold water. For example, an increase in temperature of 2.5°C, from 27.5°C to 30°C (figure 2, upper dashed lines), causes an increase in COT_min that is 1.5 times greater than that caused by an increase from 5°C to 7.5°C (0.12 J m^–1 versus 0.08 J m^–1; figure 2, lower dashed lines). It is thus plausible that major changes in the cost of swimming will occur in geographical regions where water temperatures are already high.

Figure 2.

COT_min as a function of temperature for a 1 kg fish. Solid line based on equation (2.2) and parameter estimates presented in text. Dashed lines show change in COT_min owing to an increase in temperature from 5°C to 7.5°C (0.08 J m^–1, lower dashed lines) and 27.5°C to 30°C (0.12 J m^–1, upper dashed lines).

Unexplained variation about the fitted lines in figure 1 at least partially reflects variation in the speed exponent γ, which affects COT_min as illustrated by equation (2.2). The mean value γ among the species included in our analysis was 2.4, close to the values predicted by simple hydrodynamic models (γ = 2.5–2.8) [8]. However γ varied around this value (coefficient of variation = 0.44). One promising approach for improving predictions may be using more detailed models of fish swimming mechanics (e.g. [10]) to better understand variation in the γ parameter in the simpler model used here.

Our results suggest that rising water temperatures may increase the energetic cost of routine swimming behaviours such as foraging and migration. The precise impact of climate change will ultimately depend on the degree to which water temperatures rise. However, integrating our results with work on the thermal physiology of larval, juvenile and adult fishes [2,3] will produce a more comprehensive picture of the potential for climate change to affect fishes and the communities they inhabit.