Tracking vesicle fusion from hair cell ribbon synapses using a high frequency, dual sine wave stimulus paradigm

Abstract

Recent experiments describe a technique for tracking membrane capacitance during depolarizations where membrane conductance is varying. This is a major advance over traditional technologies that can only monitor capacitance when conductance is constant because it gives direct information regarding release kinetics from single stimulations. Presented here is additional data supporting the use of this technology with multiple conductances being active including BK-Ca-activated potassium channels, SK Ca-activated potassium conductances and also the rapidly activating sodium conductance. It goes further to illustrate the ability to monitor rapid capacitative changes. And finally, it points out the need to evaluate single step responses because of the use-dependent movement of vesicles.

Ribbon type synapses are commonly found in peripheral sensory cells such as auditory hair cells and photoreceptors, where vesicle fusion is in response to a graded receptor potential.¹ These synapses typically show little fatigue and multiple components of release.² Paired and post synaptic measurements suggest a linear input output function for these synapses, particularly at auditory hair cell afferent fiber synapses.^2-4 Recent evidence suggests that the linearity in release properties arises from the sum of nonlinear processes, the linearity appearing as the processes merge temporally about the hair cell’s resting potential.⁵ In that study, two components of release were identified. The first component varied directly in release rate and magnitude with calcium influx and was saturable with a vesicle population corresponding to those near the synapse. The second ‘superlinear’ component had a constant rate, was indefatigable and required recruitment of vesicles from more distant pools. These new processes were revealed by the use of a dual sine technique which allows for the continuous monitoring of capacitance changes, even in the face of conductance changes.^5,6

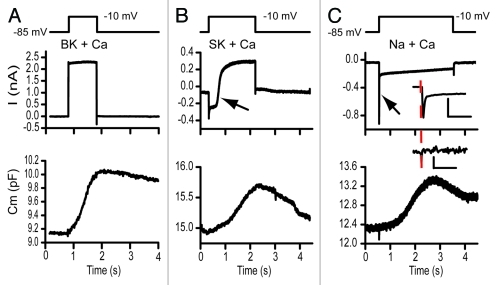
Traditionally, a single sine technique has been used to monitor capacitance.⁷ In this case the imaginary component of the complex current correlates to capacitance, as long as there is no conductance change of either membrane or electrode, otherwise the imaginary component reflects both capacitance and conductance in an inseparable manner.⁷ The two sine method allows for the continual tracking of conductance and capacitance and thus the potential ability to separate each.⁶ The added complexity of monitoring capacitance during conductance change is that the sine wave may activate ion channels, with an attendant intrusive inductance that interferes with measures of capacitive current phase, leading to unacceptable errors in capacitance measures.⁵ Sufficiently higher frequency sine wave stimuli can alleviate this problem because channel kinetics will not be able to respond to the rapid cycle by cycle voltage change. Figure 1 illustrates that the dual sine wave approach can be used to track capacitance in the presence of BK- calcium activated potassium channels, SK-calcium activated potassium channels, as well as rapidly activating and inactivating sodium channels. In each case rapidly activating L-type calcium channels (α 1D) are driving vesicle fusion. The conductance changes do not alter the capacitance measurements.

Figure 1.

The dual sine wave technology allows for monitoring capacitance concomitantly with the occurence of voltage or calcium-dependent currents. In each frame the upper panel depicts the stimulus, the middle panel the extracted currents and the lower panels the capacitance measurements. The sine waves are not shown on the stimulus but had frequencies of 6.3 and 9.4kHz. Stimulus artifacts were digitally removed and data filtered with a fourier filter to remove high frequency components. Both (A and B) were recordings from turtle hair cells while (C) was from an immature rat hair cell. (A) illustrates capacitance changes measured with a potassium based intracellular solution where the BK calcium activated potassium current activates rapidly in response to a depolarization to -10 mV. (B) Similar records with a cesium based intracellular solution without the presence of apamin externally. The large calcium current activated the SK calcium activated current, indicated by the arrow. SK activation had no effect on capacitance measurements (depolarization to -10 mV). (C) presents capacitance measurements from a postnatal day 8 rat inner hair cell that transiently expressed Na currents. This rapidly activating, rapidly inactivating current, indicated by arrow, also had little effect on the measured capacitance response. The inset expands in time each response with the scale bars reflecting 10 ms and 100 fF and 0.5 nA. The dashed line indicates onset time so that stimulus artifact can be separated from Na current. A and B were post filtered, while C was not in order to not lose an fast components.

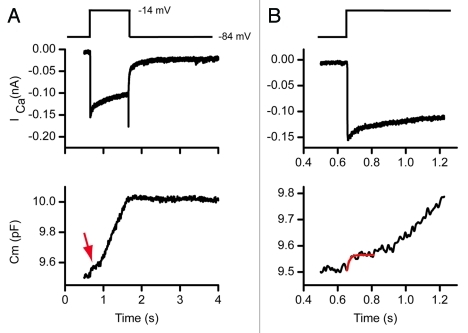
The high frequency sine wave approach samples capacitance at higher rates than the traditional single sine method. These higher sampling rates allow monitoring of vesicle fusion kinetics at least at the macroscopic level. Figure 2 depicts release from an auditory hair cell showing a small rapid saturable pool of release, (indicated by the arrow in A) and a larger less saturable pool of recruitable vesicles. Time expansion of (A) reveals the rapid release rise time; release begins immediately at the stimulus onset and continues until saturation. The solid red line is an exponential fit to this small first component of release.

Figure 2.

The dual sine wave technique allows for rapid kinetic changes to be monitored. (A) shows a calcium current response and corresponding capacitance change. The capacitance response shows multiple release components. (B) is a time expansion of (A) where the rapid release and then plateau of a small pool of vesicles is observed.

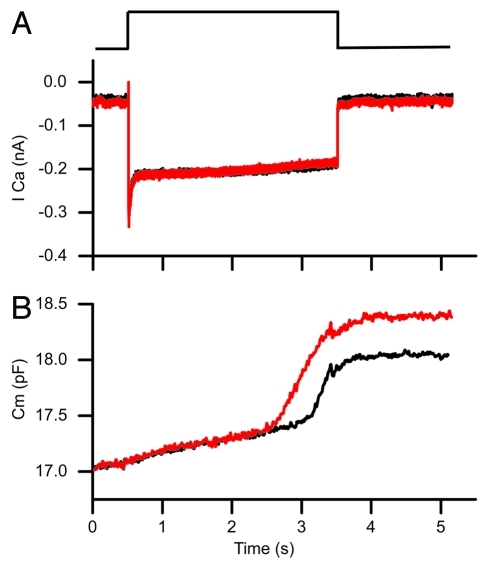
Data using the two sine technology reveals a dynamic equilibrium between vesicles and calcium such that pool sizes are continually in flux between release and recruitment. This dynamic situation makes experiments requiring repetitive stimulation suspect because the baseline vesicle distribution varies with each stimulation; thus, protocols requiring multiple stimulations may not produce the same response as an individual stimuli. Clearly, this is a major limitation of the single sine technique that provides only single time points for each stimulation, and requires substantial stimulus-response averaging both between and within cells to obtain a time course of release. Figure 3 provides an example of this problem uncovered with the dual sine technique. A second repetition of the same stimulation results in faster release with an earlier onset to the second release component. Thus, the apparent calcium-dependence of release varies with each voltage pulse, most likely due to reshuffling of vesicle populations (or some biochemical change to the vesicles that makes them more easily released) as dictated by calcium homeostasis.

Figure 3.

The dual sine technique demonstrates a major flaw with techniques that require repetitive stimulation in that responses are altered by previous stimulations. The black trace was obtained 10 min after breakthrough and is a response to a depolarization to 75% pf the peak calcium current. The red trace was obtained 5 min later and shows an increased release with insignificant change in the calcium current. This likely reflects a redistribution of vesicles following the first stimulation. The red trace was offset in the y-axis in order to clearly overlay traces.

Data obtained with the dual sine technique provide new insight into how ribbon synapses function. These data also challenge the usefulness of single sine techniques for understanding the effects of repetitive stimuli. Finally, the ability to measure capacitance in the face of evoked conductances likely will make the dual sine approach useful for the analysis of a variety of model systems.

Schnee ME, Santos-Sacchi J, Castellano-Muñoz M, Kong JH, Ricci AJ. Calcium-dependent synaptic vesicle trafficking underlies indefatigable release at the hair cell afferent fiber synapse. Neuron. 2011;70:326–38. doi: 10.1016/j.neuron.2011.01.031.