Mathematical modelling of fungal growth and function

Abstract

This contribution is based on the six presentations given at the Special Interest Group meeting on Mathematical modelling of fungal growth and function held during IMC9. The topics covered aspects of fungal growth ranging across several orders of magnitude of spatial and temporal scales from the bio-mechanics of spore ejection, vesicle trafficking and hyphal tip growth to the form and function of mycelial networks. Each contribution demonstrated an interdisciplinary approach to questions at specific scales. Collectively, they represented a significant advance in the multi-scale understanding of fungal biology.

INTRODUCTION

Mathematical modelling continues to play a significant role in the development of our understanding of the growth and function of hyphal networks. One of the main problems that faces modellers is the choice of scale. In the study of fungal mycelia, scale is expressed in an extreme manner: the indeterminate growth habit of fungi ensures that models may have to incorporate processes operating over scales ranging from the (sub) micron to the kilometre. Further complexity is added when modelling physical and nutritional heterogeneity of the host environment and the resultant multifarious sensing and response events that media hyphal growth and thus determine network architecture. Most progress to date has been made by selecting a scale and developing appropriate models to address questions manifest at that chosen scale. However, as is now becoming apparent in other areas of biological research, a meaningful, systems-based understanding requires the transfer information across scales. This session show-cased state-of-the-art mathematical and computational approaches to both scale-specific and most importantly, cross-scale problems in modelling fungal physiology and function.

CONTRIBUTIONS

The session was opened by the meeting organizer Fordyce Davidson, who gave an overview of the main challenges faced in attempting to link the increasing quantity and quality of genetic and sub-cellular experimental data to large-scale morphology and function. He detailed some of his group’s ongoing work, which ranges from the bio-mechanics of hyphal growth and thigmotropism (contact sensing) to qualitative properties of colony growth. He finished by proposing that a multi-scale, quantitative (mathematical modelling) approach is ideal for and perhaps necessary to the efficient translation of molecular genetics to a deeper understanding of the organism as a whole and its role in the host environment (Davidson 2007, 2010, Rosling et al. 2009).

Graeme Boswell then presented new work regarding the modelling of hyphal networks. He presented a discrete-continuous hybrid approach to modelling a fungal mycelium developing in a planar environment. The model comprises a series of geometrically-unconstrained connected line segments used to represent individual hyphal, so that a mycelium is represented by a collection such series (Cohen 1967, Meškauskas et al. 2004). The resultant discrete network develops over a continuous and diffusing distribution of an external substrate, representing a combination of nutrients and minerals, and which is internalized by the network according to Michael-Menten dynamics. Once internalized, substrate is translocated through the network, undergoing both multi-directional diffusion and directed advection toward the unconnected ends of line segments representing hyphal tips (Boswell et al. 2007). New line segments are created through processes analogous to subapical branching and hyphal tip extension. The orientation of new line segments are governed by local concentrations of a self-secreted inhibitor (but additional tropisms, for example in response to toxic metals, are easily included) and it is mathematically represented by a biased circular random walk and the related Focker-Planck equation (Plank & Sleeman 2004). The model was simulated in representations of homogeneous and heterogeneous nutrient configurations and it was shown that the simulated networks display numerous features that are in qualitative and quantitative agreement with experimental data (Fig. 1). Moreover, the model predicts that mycelial cords can develop through an initially stochastic but then self-reinforcing process (cf. Heaton et al. 2010).

Fig. 1.

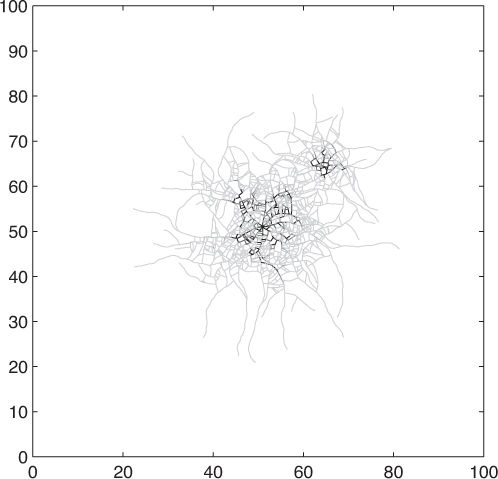
A simulated mycelial network expands from a nutrient source at the centre of the domain and is shown after a representation of four days growth. Following a representation of two days growth, additional external substrate was augmented marginally beyond the biomass edge that promoted further growth not seen when the augmentation was positioned at the biomass edge. Supplied by Graeme Boswell.

Daniel Hofstadler discussed a model for the development of hyphal networks where the key focus was on tip sensing of the environment. The model adopted the same core approach of that discussed by Graeme Boswell, but the main emphasis was on modelling tip movement in more detailed, with network formation based closely on the rule-based techniques of, for example, Meškauskas et al. (2004). A key novel feature of the model was tip-to-tip anastomosis that could be achieved by setting an appropriate tropism mediated by the internal supply of nutrients and spatio-temporal gradients of external autoregulators. This has particular relevance to early network development (Glass et al. 2004). Other more complex tip movements could be obtained by altering rules for tropism.

A complementary study of network development was then presented by Luke Heaton, who discussed growth induced mass flows. He explained that the translocation of resources within fungal networks is ecologically critical, but it is much less well studied than transport in the other major multi-cellular kingdoms of life (Jennings 1987). In particular, he noted that the relative roles of pressure driven mass-flows, diffusion and active transport remain poorly understood. The uptake of water and the maintenance of turgor pressure require an osmotic gradient between the hyphae and their environment, but because aqueous fluids are incompressible, mass flows can only take place when water is able to exit the translocation pathway through either localised exudation (e.g. Serpula lacrymans), evaporation, or by moving into a region of new growth (Cairney 1992, Lew 2005). Growth-induced mass flow can be used to describe the last of these phenomena (Heaton et al. 2010). The key idea here is that as the hyphal tips expand, the incompressibility of aqueous fluids ensures that in the supporting mycelium, there must be a net flow of fluid from the sites of water uptake to the sites of growth. To quantify the scale of growth-induced mass flows and gain insight into the developmental logic of fungal networks, a mathematical model based on circuit theory has been developed, and applied to three empirical networks of Phanerochaete velutina. Given a time series of networks where each edge has a measured volume, the model was used to calculate a current for each edge. These currents reflect the minimal flow of material that is consistent with the measured changes in volume, under the assumptions that: (1) the inoculum is the sole source of water and nutrients; (2) the conductance of cords is proportional to their cross sectional area; and (3) the fluid follows the path of least resistance. Cords that are predicted to carry larger currents were significantly more likely to increase in thickness than the other cords (Fig. 2). Over three replica experiments the Spearman’s rank correlation coefficient between the volumes predicted to have passed through the cords and the cord’s cross-sectional areas was 0.51. In contrast, the correlation coefficient between the age of cords and their cross-sectional areas was only 0.21 (Heaton et al. 2010).

Fig. 2.

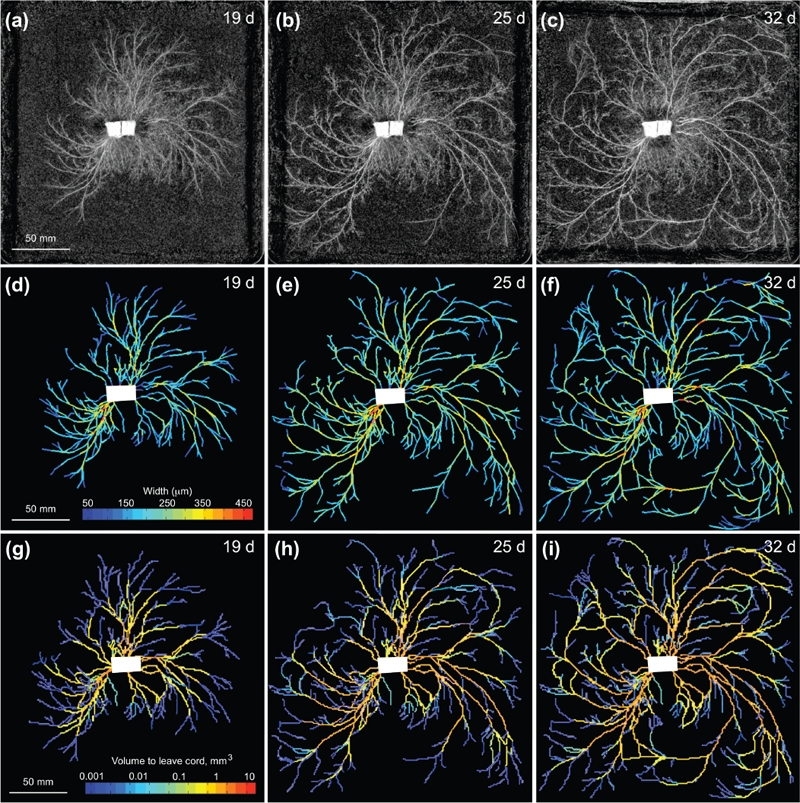
Network development and currents predicted by the mathematical model in Phabaerochaete velutina. (a) – (c) Network development in P. velutina after 19, 25 and 32 d. The image intensity of cords was used to estimate their thickness, enabling the production of the weighted, digitized networks (d) – (f). These are colour-coded to show the estimated thicknesses of all sections of all edges. Images (g) – (i) are colour coded according to the total volume that has passed through each cord, as calculated by our model. Supplied by Luke Heaton.

The focus then shifted to lower spatial scales, but discussion of the processes of internal flow and network development continued. Marcus Roper discussed how fungal thalli grow by extension at hyphal tips, and by the flow of cytoplasm and nuclei into the spaces created at the extending tips (Rasmos-Garcia et al. 2009). For rapidly growing species, like Neurospora crassa, he explained that hyphae grow too fast to be populated by nuclei produced by division at or near the tips. Instead, nuclei are produced by mitosis distributed throughout the entire thallus, and migrate to the growing periphery of the thallus at speeds that may reach 10 μm s⁻¹ or more (Roper et al., unpubl.). By making heterokarya in which nuclei are histone H1 differentially fluorescently tagged, his group has been able to map the dynamics of these nuclear flows during thallus growth. They found complex multidirectional flows several centimeters behind the growing front of the colony, in fact much further from the tips than a simple transport of nuclei to fill spaces created by growth would require. It has long been known that nuclei within a single thallus may be genetically diverse (Caten & Jinks 1966, Maheshwari 2006). The complex and multidirectional paths taken by nuclei suggest that flows may facilitate a mixing of nuclei during their transport to the colony periphery, maximizing genetic diversity at the growing tips, and thus preventing e.g. sectoring. Marcus presented simulations that compared nuclear flows in real thalli with idealized hyphal networks and discussed how this allowed his group to dissect how the network architecture might be designed to maximize mixing and accelerate the dispersal of new nucleotypes that arise e.g. by mutation.

Finally, Mark Fischer discussed the physics of spore ejection. His talk detailed how after high-speed launches, air viscosity causes the rapid deceleration of fungal spores and limits their discharge distance. The fluid dynamics contained within Stokes’ law posits a simple relationship between drag coefficient and velocity (Reynolds number, Re). It provides an excellent fit to experimental measurements of the terminal velocity of free-falling spores and other instances of low Reynolds number motion (Re<1). For movements characterized by higher Re, more complex models have been devised to determine drag but none have been evaluated for modelling the launch of fast moving fungal spores. In Fischer et al. (2010) the first empirical test of Stokes’ Law and a commonly used complex drag model (Clift et al. 2005, White 1974, Vogel 2005) was presented with launch speed data obtained from ultra-high-speed video recordings and experimental measurements of discharge distances (Pringle et al. 2005, Yafetto et al. 2008, Stolze-Rybczynski et al. 2008; Fischer et al. 2010b). They found that discharge distances predicted from launch speeds by the simpler Stokes’ model provide a much better match to measured distances than the more complex drag model. Stokes’ model works better over a wide range spore sizes, launch speeds, and discharge distances, from microscopic mushroom ballistospores discharged at <1 ms⁻¹ over a distance of <0.1 mm (Re=0.3), to macroscopic sporangia of Pilobolus that are launched at >10 ms⁻¹ and travel as far as 2.5 m (Re=167). Even at Reynolds numbers greater than 2000 as observed for Sphaerobolus launches, Stokes’ Law models the relationship between launch speed and discharge distance much better than the more complex drag model (Fig. 3).

Fig. 3.

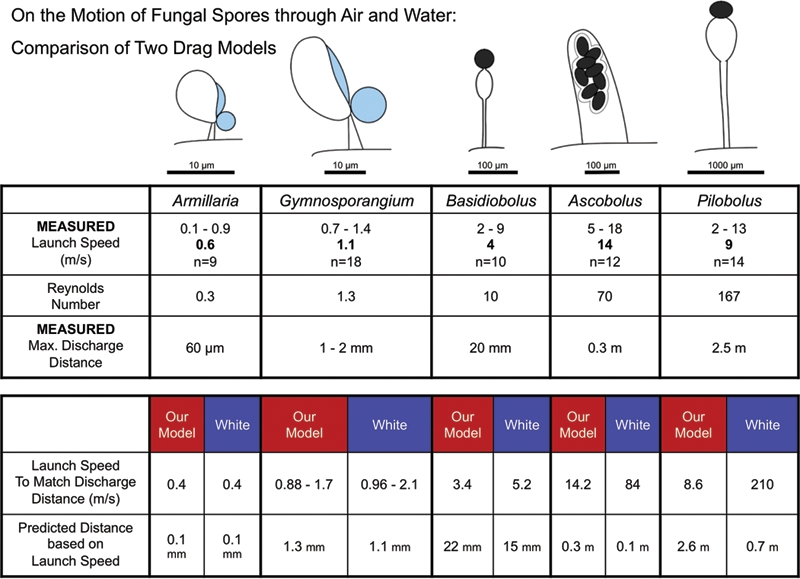
Comparison of two models of viscous drag for projectiles launched from five fungal species whose initial launch speeds are characterized by Reynolds numbers (Re) ranging from 0.3 to 167 (Fischer et al. 2010b). For each species, maximum discharge distances and initial launch speeds were directly measured, the latter by means of ultra high-speed videography. In each case, the launch speed required to obtain the observed discharge distance and the predicted discharge distance based on the observed launch speed were calculated using two drag models: Stokes’ Law (here labelled “Our Model”) and a more complex semi-empirical model (“White”) that is representative of drag models typically used to model viscous drag for Re> 1. For low Re, Armillaria and Gymnosporangium, both models accurately model the drag. For larger Re, Stokes’ Law continues to successfully model the drag. The White model, however, consistently overestimates the drag requiring either extremely large launch speeds (210 ms^–1 for Pilobolus) or severely underestimating the discharge distance (0.7m vs. the observed 2.5 m for Pilobolus). Supplied by Mark Fischer.