Abstract
Tortuous arteries are often associated with aging, hypertension, atherosclerosis, and degenerative vascular diseases, but the mechanisms are poorly understood. Our recent theoretical analysis suggested that mechanical instability (buckling) may lead to tortuous blood vessels. The objectives of this study were to determine the critical pressure of artery buckling and the effects of elastin degradation and surrounding matrix support on the mechanical stability of arteries. The mechanical properties and critical buckling pressures, at which arteries become unstable and deform into tortuous shapes, were determined for a group of five normal arteries using pressurized inflation and buckling tests. Another group of nine porcine arteries were treated with elastase (8 U/ml), and the mechanical stiffness and critical pressure were obtained before and after treatment. The effect of surrounding tissue support was simulated using a gelatin gel. The critical pressures of the five normal arteries were 9.52 kPa (SD 1.53) and 17.10 kPa (SD 5.11) at axial stretch ratios of 1.3 and 1.5, respectively, while model predicted critical pressures were 10.11 kPa (SD 3.12) and 17.86 kPa (SD 5.21), respectively. Elastase treatment significantly reduced the critical buckling pressure (P < 0.01). Arteries with surrounding matrix support buckled into multiple waves at a higher critical pressure. We concluded that artery buckling under luminal pressure can be predicted by a buckling equation. Elastin degradation weakens the arterial wall and reduces the critical pressure, which thus leads to tortuous vessels. These results shed light on the mechanisms of the development of tortuous vessels due to elastin deficiency.
Keywords: tortuosity, buckling, critical pressure, porcine artery
arteries are subjected to significant mechanical loads from blood pressure, surrounding tissue tethering, and body movement. The strength and stability of arteries under these loads are essential to the maintenance of normal arterial function. While the mechanical stress and strength of blood vessels have been well documented, the mechanical stability of arteries is less understood.
Arteries and arterioles often become tortuous, leading to hemodynamic changes and various clinical complications, but the underlying mechanism remains unclear. Tortuous or twisted arteries are commonly seen in the vasculature, from the large aorta (37), to medium-sized carotid arteries and iliac arteries (9, 35, 40, 54), small arterioles (4, 42, 50), and capillaries (34, 42, 51). Tortuous vessels are seen in cerebrals, coronary, retinal, and conjunctival arteries and veins (4, 5, 29, 39, 49, 50, 57). Tortuous veins, such as varicose veins, are also often seen in human legs (3). Severely twisted blood vessels can lead to hemodynamic changes and various clinical complications (10, 40). For example, tortuous internal carotid arteries can lead to stroke, vertigo, syncope, blackout, persistent tinnitus, and other cerebrovascular deficiencies in patients (1, 27, 32, 57). Clinical studies have shown that artery tortuosity is associated with hypertension, atherosclerosis, aging, and other pathological changes (10, 24, 25, 32, 40, 47, 48, 50), suggesting that mechanical factors may play an important role.
Recent theoretical analysis by our group and others suggested that mechanical instability occurs in arteries and subsequently leads to tortuosity (16, 17, 19, 28, 44). Artery instability due to buckling may be a possible mechanism for the development of tortuous arteries. Therefore, it is of clinical importance to understand the buckling behavior of arteries under luminal pressure.
Mechanical stability of arteries depends on their wall stiffness (14, 16, 17). Elastin is an important extracellular matrix component for arterial elasticity and stiffness, and elastin degradation weakens the arterial wall (11). Elastin deficiency has been associated with tortuous arteries in patients with arterial tortuosity syndrome and Loeys-Dietz syndrome, as well as in mice with an elastin gene knockout (7, 36, 49, 52). Elastin degradation has also been associated with tortuous cerebral arteries due to increased blood flow (5, 25). In addition, elastin degradation and arterial tortuosity occur concomitantly in the aged population (13, 46). These lines of evidence suggested that elastin degradation may play a role in the development of tortuous arteries, but the underlying mechanisms are unclear. Therefore, it is important to study the effect of elastin on the mechanical stability of arteries.
Accordingly, the objectives of this study were to determine the critical buckling pressure of porcine carotid arteries, to test the predictive value of the artery buckling model, and to determine the effects of elastin degradation and surrounding matrix support on the mechanical buckling of arteries. We tested the hypothesis that arteries may become mechanically unstable under luminal pressure, and that elastin degradation reduces the critical pressure, thus leading to artery tortuosity.
MATERIALS AND METHODS
Specimen preparation.
Porcine common carotid arteries (the segment distal to the sinus but proximal to the bifurcation of inner and outer carotid arteries) were harvested postmortem from 6- to 7-mo-old farm pigs (100–150 kg) at a local abattoir. After being rinsed with phosphate-buffered saline (PBS) (Dulbecco's PBS, Sigma Chemical, St. Louis, MO), the specimens were placed into PBS solution and transported to our laboratory in an iced cooler. Once in the laboratory, the arteries were cleaned by removing excess connective tissue and were rinsed again with PBS. The in vitro free lengths were measured while the vessels were afloat in PBS solution. The arteries were then mounted onto a luer stopper at one end, attached to a 10-ml plastic syringe at the other end, and were inflated briefly to check for leaks. The inflated diameter and length of the arteries were monitored to ensure the inflation pressure applied was lower than 100 mmHg.
Inflation testing and determination of the stress-strain relationship.
To evaluate the mechanical stiffness of the porcine arteries, we measured their elongation and diameter inflation under internal pressure. Briefly, one end of the arteries was mounted to a cannula in the tissue chamber (Fig. 1, top) used in our laboratory's previous studies (16, 21), while the other end was tied onto a luer stopper and allowed to move freely. The cannula was connected to a pressure meter and a syringe pump filled with PBS. The arteries were preconditioned by gradually inflating them with PBS to a pressure of 200 mmHg and deflating to 0 mmHg for several cycles (8, 33). After that, the arteries were slowly inflated with PBS, at a rate of ∼2 mmHg/s, using the syringe pump and allowed to expand freely in both radial and axial directions. The inflated outer diameter and axial length of the arteries were measured simultaneously as the luminal pressure increased. The inflation process was recorded with a SONY digital camera, and lengths and diameters were measured from the digital photos.
Fig. 1.
Schematic of experimental setup for pressured inflation test (top) and buckling test (bottom). The camera can take views from the top, as shown, or from the side (not shown).
Based on the experimental observation, the arterial walls were assumed as cylindrical thick-walled tubes with an orthotropic nonlinear elastic wall characterized by the Fung strain energy function (15, 26). The pressure and axial force can thus be expressed as functions of the strains (Eqs. A9 and A10 in the appendix). The material constants were determined by fitting these equations with the experimental pressure-diameter-length data using the nonlinear “lsqnonlin” function in Matlab.
For a group of five normal arteries (normal group), the experimental data were fit with the three-dimensional (3D) Fung strain energy function first. Then, for comparison, the data were also fit with the two-dimensional (2D) Fung strain energy function (b3 = b5 = b6 = 0 in Eq. A4) using the thin-walled cylindrical model, as previously described for veins (30).
Buckling testing and measurement of the critical pressure.
To determine the critical pressure, arteries were tied at both ends onto cannulas inside the tissue chamber (Fig. 1, bottom). The arteries were stretched axially to achieve designated axial stretch ratios and then gradually pressurized with PBS using the syringe pump, while being photographed at pressure increments of 5–10 mmHg until a large deflection (lateral displacement) of 5–10 mm was reached at the middle of the vessels. A preliminary reading of the critical pressure was recorded when arteries began to exhibit visually detectable deflection. This process was repeated three times for each artery, and the process was also recorded with a SONY digital camera. The camera was orientated to take photographs from either the top view or the side view, depending on the direction of the buckling. If the artery was buckling in a direction other than the horizontal or vertical planes, we rotated the artery with both cannulas so that the buckling would be perpendicular to the camera lens (there was no contact between the arteries and the chamber walls).
Later, the lateral displacements of the arteries under pressure were measured from the video and photos using ImagePro Plus (Media Cybernetics). First, the positions of the central lines of the arteries at all pressure levels were determined by averaging the coordinates of the two edges of the arteries. Then the deflection was determined as the maximum lateral displacement of the central line (at the middle of the vessel length) from its baseline position at zero pressure. The critical pressure was determined to be the pressure when the deflection became detectable (∼0.5 mm) from the initial baseline.
For the five arteries in the normal group, the buckling tests were done at a series of axial stretch ratios in the range of 1.0–1.7 at a step of 0.1, which covers physiological (1.5 in vivo) and subphysiological ranges (21), to evaluate the effect of axial stretch ratio on the critical pressure.
Model prediction of critical pressure.
The critical buckling pressure (pcr) was determined using the buckling equation
| (1) |
where N is the axial force, and H is the “bending force” given as:
| (2a) |
| (2b) |
wherein
| (3a) |
| (3b) |
| (3c) |
where L is the vessel length; ri and re are the lumen and outer radii, respectively; Eθ, Ez, and Er are the circumferential, axial, and radial components of Green strain, respectively; and b0, b1, . . ., and b6 are material constants. This equation was developed for arteries with two fixed ends as used in the experimental setting following our previous model (17, 18). The detailed derivations of the equation are given in the appendix.
For each artery, the critical pressures at different axial stretch ratios were determined based on its own material constants and initial dimensions determined from the experimental measurements (17, 18). Residual stresses were ignored in the model simulations (an opening angle of 2Θ0 = 360° was assumed, see Fig. A1), since a pilot study using rabbit data demonstrated little effect of the opening angle on the critical pressure of arteries (6, 18) (see discussion).
Elastase treatment.
To explore the possible effect of arterial wall matrix components on arterial critical pressure, a group of nine arteries (elastin group) was treated with elastase, as described previously (11). Briefly, arteries were filled with elastase [8 U/ml, Lyophilized (ESL), Worthington Biochemical, Lakewood, NJ] in the lumen for 60 or 90 min after the baseline inflation and buckling tests were done. Statistical testing showed no significant difference between the elastase treatment groups for 60 or 90 min. After treatment, the arteries were washed with PBS to remove residual elastase, and the pressurized inflation test and buckling test were performed again following the same protocols as described above. The buckling tests for the elastase group were done at only three axial stretch ratios of 1.1, 1.3, and 1.5 to reduce the testing time, since testing of the normal group had depicted the effect of axial stretch ratio on the critical pressure in the full range of 1.0 to 1.7. A subset of arteries in this group was used for opening angle measurement and elastin quantification.
Opening angle measurement.
To determine the effect of elastase treatment on the residual strain in the carotid arteries, we measured the opening angles of a subset of five arteries from the elastase treatment group. Arterial rings (short segments of 2–3 mm in axial length) were cut from the distal and proximal ends of the specimens before elastase treatment and compared with rings cut from the middle after elastase treatment. For the opening angle measurement, arterial ring segments were arranged in PBS (25°C) in a Petri dish and then cut open by radial cuts (20, 22). After the radial cut, the rings popped opened into C-shaped sectors, and the sectors were allowed to stabilize for 30 min to fully release the residual stress (20, 22). The configurations of the sectors were then photographed with a digital camera. The angle between the two lines from the middle of the inner wall to the two tip of the inner wall (20, 22) was measured for each sector using ImagePro Plus. This angle was then convert to the opening angle 2Θ0 based on the relation that this angle plus Θ0 is equal to 180° (6, 18).
Buckling test of arteries within gelatin matrix.
To illustrate the effect of surrounding tissue support, an additional eight arteries (gel group) were placed into gelatin after baseline buckling tests in PBS at a stretch ratio of 1.3. The gelatin had an elastic modulus of 7.4 ± 7 kPa, to mimic the tissue stiffness reported in the literature (58). To prepare the gelatin matrix, gelatin powder (Knox Original Gelatine, Kraft) was added to heated water (37°C) at a concentration of 9 g/200 ml water. The liquid gelatin mixture was then poured into the testing box to submerge the artery and cooled for 3 h until it formed a solid gel. The stiffness of the gel was controlled by the concentration and was premeasured using compression testing. The buckling tests were performed to measure the critical pressure. Then the gel was gently removed and washed. The critical pressure and the buckled mode shape of the arteries were measured again for comparison.
Measurement of arterial initial dimensions.
After inflation and buckling tests, all arteries were removed from the chamber, and short ring segments (∼2 mm in axial length) were cut for cross-sectional dimension measurements. The lumen diameter, outer diameter, and wall thickness were measured from the cross-sectional surfaces under a dissecting microscope and photographed. For each ring segment, the diameters were measured at two radial locations 90° apart, and the wall thickness was measured from four locations 90° apart. The segments were then fixed in 10% formalin for histology.
Histology.
The specimens were fixed overnight in formalin and then processed and embedded in paraffin blocks. Sections (5 μm in thickness) were cut and processed for hematoxylin-eosin staining. For arterial specimens treated with elastase, consecutive sections were processed for hematoxylin-eosin staining, Verhoff staining, and trichrome staining.
Photometric measurement of elastin and collagen contents.
The elastin and collagen contents were measured for arterial cross sections with Verhoff staining and trichrome staining, respectively. Eight to twelve locations from two to four cross sections of each artery, each including the intima, media, and adventitia, were measured. The ratio of the area of the collagen or elastin to the total tissue area was determined using the histogram analysis feature in ImagePro Plus. The percentage of elastin was determined as the ratio of elastin to tissue areas. The same procedure was repeated for collagen.
Measurement of elastin content using Fastin assay.
The change of elastin content due to elastase treatment was further quantified in a subgroup of four arteries in the elastase group using a Fastin assay (31). Briefly, equal segments ∼4 mm in length were cut from arteries before and after elastase treatment. Elastin was extracted by putting each sample into a microcentrifuge tube with 0.25 N oxalic acid solution and immersion inside a 100°C water bath for 1 h. The sample was then centrifuged for 10 min, and the supernatant was removed. This process was repeated 10 times to completely dissolve the tissue sample. A Fastin assay kit (Biocolor Fastin Assay Kit, CLRF2000, Accurate Chemical and Scientific, Westbury, NY) was then used to quantify the elastin content using a microplate reader, according to the manufacturer's specifications. The elastin content is specified as microgram (μg) elastin per milligram (mg) tissue.
Statistical analysis.
All values are presented as means (SD) (standard deviation). Statistical significance between means was determined by having achieved a significance level P value < 0.05. A normalcy test (Instat) was used to find out if the two elastin groups (60 and 90 min) could be combined. A paired Student's t-test was used to determine the significance in the elastin study. The t-test and a power analysis were done using JMP software (SAS Institute).
RESULTS
A total of 22 arteries were examined in three groups. These arteries included either left or right common carotid arteries from 30 animals (the rest were either used for other studies or disposed due to branch or leakage). Five arteries (normal group) were tested to determine the effect of axial stretch ratio and to test the predictive value of the 3D and 2D theoretical models. Nine arteries (elastase group) were examined before and after elastase treatment to detect the effect of elastin degradation, and eight arteries (gel group) were tested in PBS and in a gelatin matrix to illustrate the effect of surrounding tissue matrix support.
Stress-strain relationship of normal arteries (normal group).
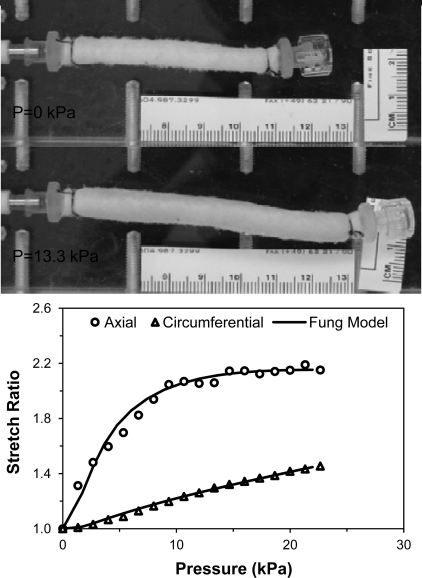
The lengths of the five arteries in the normal group ranged from 47 to 57.2 mm, with an average external radius of 3.0 mm. Dimensions of these vessels are summarized in Table 1. When pressurized with one end free, the artery segments enlarged and elongated simultaneously (Fig. 2). The length (and accordingly axial stretch ratio) increased consistently with increasing pressure, while the radius increase slowed down after 10–15 kPa (Fig. 2). The material constants obtained by fitting the data with Eqs. A9 and A10 in the appendix are summarized in Table 2. The corresponding material constants obtained by fitting the data with the 2D strain energy function using the thin-walled vessel model are listed in Table 3.
Table 1.
Summary of the initial dimensions of the arteries tested (normal group)
| Artery ID | Length | Outer Diameter | Wall Thickness |
|---|---|---|---|
| 5 | 52.00 | 6.99 | 1.86 |
| 6 | 50.04 | 6.19 | 1.75 |
| 7 | 58.70 | 5.44 | 1.40 |
| 8 | 63.00 | 5.81 | 1.27 |
| 9 | 53.50 | 4.44 | 0.87 |
| Mean (SD) | 55.45 (4.74) | 5.77 (0.84) | 1.43 (0.36) |
Values are in mm. ID, artery identification number.
Fig. 2.
Deformation of an artery under pressurized inflation. Photographs: a porcine carotid artery under zero pressure (P = 0 kPa) and internal pressure (P = 13.3 kPa) while its right end was closed and free to move. Graph: the axial stretch ratio (length change ratio) and circumferential stretch ratio (internal radius change ratio) plotted as functions of the luminal pressure. The solid lines are the fitting curves using the Fung three-dimensional (3D) strain energy function.
Table 2.
Material constants for three-dimensional Fung strain energy function (normal group)
| ID | b0 | b1 | b2 | b3 | b4 | b5 | b6 |
|---|---|---|---|---|---|---|---|
| 5 | 55.45 | 0.304 | 0.001 | 0.297 | 0.518 | 0.318 | 0.241 |
| 6 | 43.00 | 0.220 | 0.001 | 0.217 | 0.342 | 0.155 | 0.140 |
| 7 | 31.02 | 0.189 | 0.147 | 0.636 | 0.738 | 0.856 | 0.001 |
| 8 | 70.10 | 0.148 | 0.021 | 0.001 | 0.428 | 0.338 | 0.108 |
| 9 | 330.0 | 0.076 | 0.001 | 0.096 | 0.210 | 0.175 | 0.068 |
b0–b6, Material constants.
Table 3.
Material constants for two-dimensional Fung strain energy function (normal group)
| ID | b0 | b1 | b2 | b4 |
|---|---|---|---|---|
| 5 | 21.67 | 0.7951 | 0.109 | 0.515 |
| 6 | 19.46 | 0.850 | 0.001 | 0.706 |
| 7 | 14.69 | 1.497 | 0.001 | 1.288 |
| 8 | 12.42 | 2.745 | 0.4925 | 0.749 |
| 9 | 34.39 | 1.182 | 0.112 | 0.744 |
Critical pressure of normal arteries.
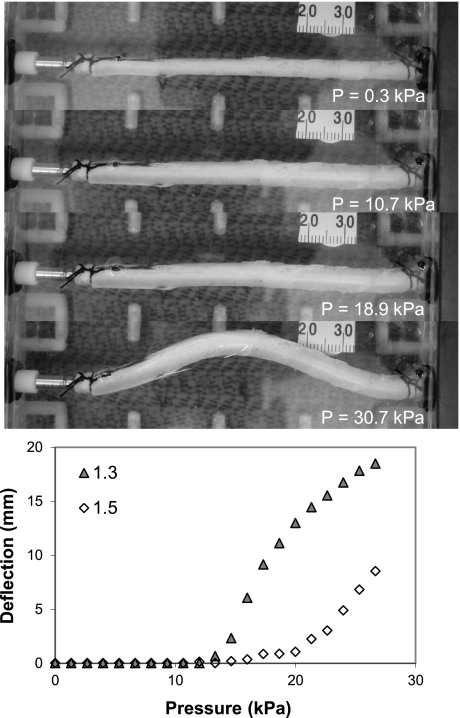
All artery segments buckled when the luminal pressure exceeded critical values. Initially, the arteries inflated, and their diameters increased but did not deflect laterally. When the luminal pressure reached critical pressure levels, arteries deflected from the baseline and became buckled (Fig. 3). The deflection initiated at the critical pressure and increased as the pressure continued to increase, which demonstrated a nonlinear postbuckling behavior (Fig. 3, bottom). One interesting observation was that, once an artery buckled at a specific location and direction under increasing pressure, it would buckle in the same location and direction under repeating pressure loads. The critical pressures were obtained at several levels of axial stretch ratios (1.0–1.7, at a step of 0.1), and the results are summarized in Fig. 4. It is seen that the critical pressure increased as the axial stretch ratio increased.
Fig. 3.
Artery buckling under luminal pressure. A porcine carotid artery under luminal pressure with both ends fixed to cannulas is shown. Photos (from top to bottom) were taken at a pressure of 0.3, 10.7, 18.9, and 30.7 kPa. The axial stretch ratio was 1.5, and the critical buckling pressure was 17.89 kPa. Line graph: the maximum deflection (at the middle point) plotted as functions of the luminal pressure at two axial stretch ratios (1.3 and 1.5). It is seen that the artery started to buckle at a critical pressure that depends on the axial stretch ratio, and the postbuckling deflection increased nonlinearly with luminal pressure.
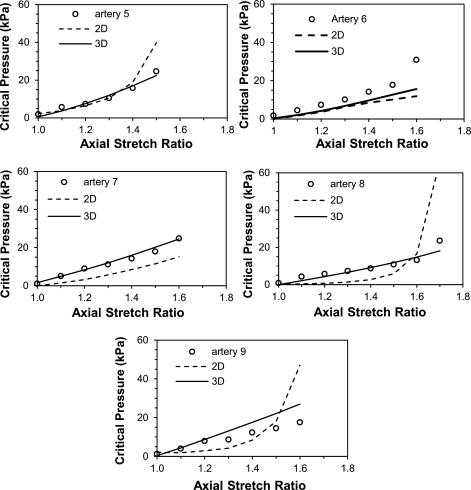
Fig. 4.
Comparisons of critical buckling pressures from experimental measurement (○) and two-dimensional (2D) and 3D model predictions (dahsed and solid lines, respectively) for five arteries in the normal group. The model predictions were obtained for each individual artery by using the material constants given in Tables 2 and 3 and vessel dimensions given in Table 1 as inputs into the buckling equation.
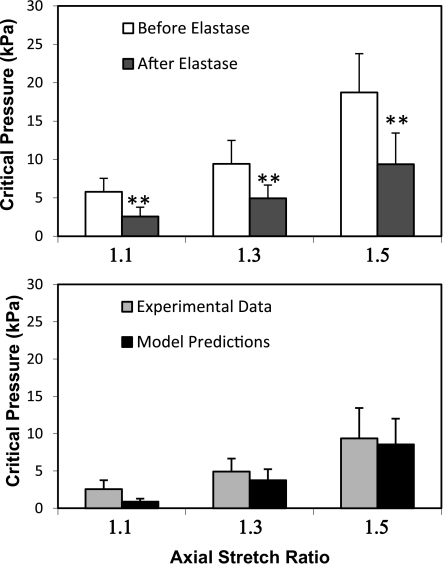
Critical pressures of these arteries were also calculated using buckling equation (Eq. 1) with vessel dimensions and material constants given in Tables 1, 2, and 3 for each individual artery. The results are shown as solid curves in Fig. 4. It is seen that the model-predicted critical pressures matched well with the experimental results, with an average correlation coefficient of r = 0.84. At an axial stretch ratio of 1.3, for example, the critical pressures measured were 9.52 kPa (SD 1.53), while the buckling equation using the 3D model predicted the critical pressures of 10.11 kPa (SD 3.12). Both 2D and 3D buckling models predicted a trend of nonlinear increase of critical pressure as the stretch ratio increased. The 2D model tended to overestimate the critical pressure at the higher stretch ratios, whereas the 3D model was more consistent in its predictions.
Effect of elastase treatment on arterial wall mechanical properties (elastase group).
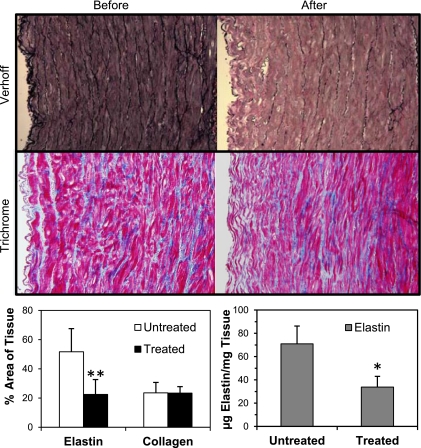
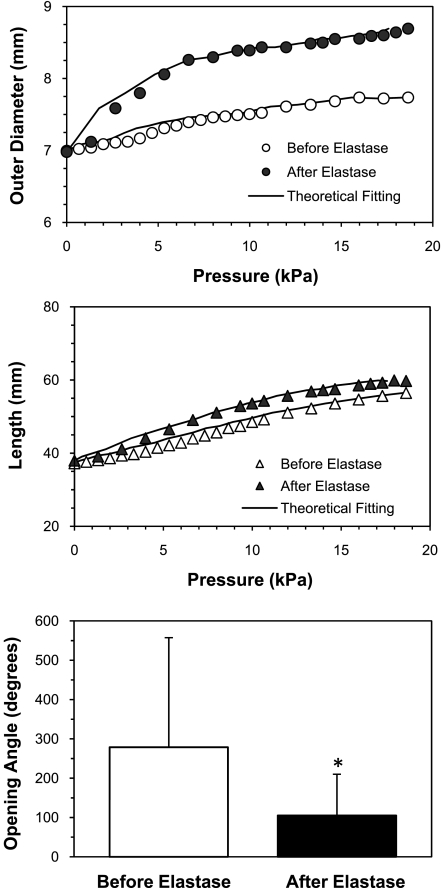
Verhoff staining, which stains elastin in dark purple, clearly showed elastin degradation in elastase treated artery segments compared with untreated segments (Fig. 5). Before elastase treatment, the internal elastic lamina and other elastic fibers were well organized throughout the wall. After elastase treatment the internal elastic lamina was nearly fully removed, and the other elastin fibers were fragmented throughout the wall. Trichrome staining showed no difference in collagen content before and after elastase treatment visually and by photometric measurement (n = 7, P = nonsignificant). Both photometric measurement (n = 7, P < 0.01 at a power of 0.91) and Fastin assay (n = 4, P < 0.05, at a power of 0.98) demonstrated a significant 50–60% reduction in elastin content after elastase treatment (Fig. 5, bottom).
Fig. 5.
Top: histology sections (×10) with Verhoff staining demonstrating the elastin (in black) in an artery before (left) and after (right) elastase treatment. Breakdown of elastin is evident after the treatment. Middle: histology sections with Trichrome staining demonstrating collagen (in blue) before and after elastase treatment. Bottom: quantification of protein concentrations. The left bar graph represents the color metric quantification of elastin and collagen area ratios [mean (SD), n = 7], and the right bar graph shows the elastin concentration [mean (SD), n = 4] measured using Fastin assay. *P < 0.05, **P < 0.01.
Slight changes in the overall vessel dimensions were also observed after elastase treatment. The vessel lengths of the nine arteries were 44.6 ± 8.0 mm before treatment and 43.4 ± 8.0 mm after treatment; outer diameters were 6.5 ± 0.7 mm before treatment and 6.9 ± 0.7 mm after (P < 0.05); and wall thicknesses were 1.19 ± 0.41 mm before treatment and 0.90 ± 0.29 mm after. The differences were statistical insignificant for length and wall thickness.
Pressure inflation tests showed that arteries deformed more under pressure after elastase treatment compared with before treatment, indicating that arterial walls became less stiff post-elastase treatment (Fig. 6). The corresponding material constants of the 3D Fung strain energy changed after the elastase treatment (Table 4). The opening angles Θ0 of arteries were significantly smaller in the elastase-treated arteries (P < 0.05).
Fig. 6.
Effect of elastase treatment on the mechanical properties of arteries (elastin group). Comparison of the outer diameter (circles) and vessel length (triangles) of an artery plotted as functions of luminal pressure before (open) and after (solid) elastase treatment. Solid curves are the fitting results using the Fung 3D strain energy function. Bottom bar graph: comparison of opening angles of arteries [mean (SD), n = 5] before and after elastase treatment. *P < 0.05.
Table 4.
Comparison of material constants of three-dimensional Fung strain energy function of arteries (elastin group) before and after elastase treatment
| ID | b0 | b1 | b2 | b3 | b4 | b5 | b6 |
|---|---|---|---|---|---|---|---|
| Before | |||||||
| E1 | 100 | 0.082 | 0.005 | 0.001 | 0.154 | 0.056 | 0.020 |
| E2 | 28.5 | 0.550 | 0.112 | 0.001 | 1.220 | 0.430 | 0.001 |
| E3 | 17.2 | 0.395 | 0.004 | 0.001 | 0.946 | 0.399 | 0.001 |
| E4 | 71.5 | 1.470 | 0.046 | 0.001 | 0.891 | 0.042 | 0.754 |
| E5 | 217 | 1.070 | 0.045 | 0.001 | 0.641 | 0.077 | 0.690 |
| E6 | 116 | 0.466 | 0.001 | 0.020 | 0.469 | 0.112 | 0.338 |
| E7 | 533 | 0.322 | 0.007 | 0.001 | 0.254 | 0.024 | 0.222 |
| E8 | 641 | 0.302 | 0.001 | 0.001 | 0.162 | 0.001 | 0.205 |
| E9 | 38.5 | 4.500 | 0.213 | 0.001 | 2.670 | 0.281 | 2.600 |
| After | |||||||
| E1 | 216 | 1.950 | 0.050 | 0.001 | 1.080 | 0.070 | 1.130 |
| E2 | 102 | 1.500 | 0.090 | 0.001 | 0.960 | 0.180 | 1.030 |
| E3 | 12.5 | 0.552 | 0.001 | 0.758 | 1.190 | 0.629 | 0.408 |
| E4 | 6.14 | 1.650 | 0.114 | 0.001 | 2.090 | 0.410 | 0.258 |
| E5 | 488 | 0.420 | 0.019 | 0.001 | 0.250 | 0.030 | 0.257 |
| E6 | 5.39 | 0.890 | 0.140 | 0.001 | 1.780 | 0.820 | 0.001 |
| E7 | 46.8 | 0.912 | 0.009 | 0.001 | 0.952 | 0.094 | 0.298 |
| E8 | 10.2 | 76.00 | 0.460 | 25.00 | 3.020 | 0.001 | 0.001 |
| E9 | 0.111 | 39.36 | 0.001 | 37.76 | 1.360 | 0.001 | 10.74 |
E, elastase.
Effects of elastase on the critical buckling pressure (elastase group).
The degradation of elastin reduced the critical buckling pressure. For arteries treated by elastase, the critical buckling pressure significantly decreased posttreatment compared with pretreatment (Fig. 7). At an axial stretch ratio of 1.5, for example, the average critical pressure of arteries was 18.29 kPa (SD 4.58) pretreatment and 9.58 kPa (SD 3.68) posttreatment, respectively (n = 9, P < 0.01). The theoretical model accurately predicted the critical pressure before elastase treatment, but underestimated the critical pressure after elastase treatment.
Fig. 7.
Top: comparison of the critical pressure of arteries [mean (SD), n = 9] measured before and after elastase treatment (elastin group). Bottom: comparisons of experimental measurements with theoretical predictions (Fung 3D) after elastase treatment. ** P < 0.01 vs. before treatment. λ+1.1, λ+1.3, and λ+1.5: axial stretch ratios of 1.1, 1.3, and 1.5, respectively.
The effects of matrix support on the buckling behavior of arteries (gel group).
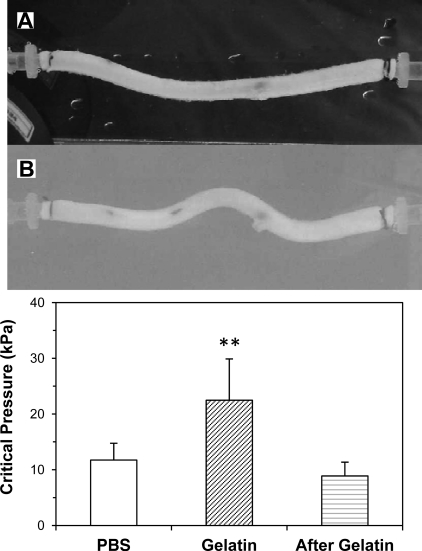
While arteries in PBS (without surrounding matrix support) buckled into one sinusoidal wave, arteries in an elastic matrix buckled into multiple waves (Fig. 8). The buckling pressure was higher when arteries buckled inside an elastic matrix compared with arteries that buckled in PBS. At an axial stretch ratio of 1.3, the buckling pressures of the eight arteries were 11.10 kPa (SD 3.02), 22.50 kPa (SD 7.41), and 8.9 kPa (SD 2.48) at the baseline (in PBS), in gel, and after the removal of the gel (in PBS), respectively. The critical pressure in gel was significantly higher than in PBS (P < 0.01). These findings are consistent with our laboratory's previous theoretical predictions of arteries buckling inside a matrix (17).
Fig. 8.
The effect of surrounding tissue support on artery buckling. Photographs are of an artery buckled under internal pressure in PBS solution (A) and then inside gelatin gel (B). The critical pressures were 13.3 and 30.6 kPa in PBS and in gel, respectively. The axial stretch ratio was 1.3, and the gel modulus was 4.4 kPa. Bar graph: a comparison of critical pressures of arteries [mean (SD), n = 8] at baseline (in PBS), in gel, and after gel removal (in PBS). **P < 0.01.
DISCUSSION
The stability of long arteries under internal pressure has rarely been studied, since arteries are commonly considered stable under luminal pressure and axial tension. Our results clearly demonstrated that arteries buckle and become tortuous under luminal pressure. The critical buckling pressure depended on the axial stretch ratio and wall stiffness. Elastase treatment reduced the stiffness of the arterial wall and its critical pressure, which thus makes arteries vulnerable to instability. In contrast, surrounding tissue matrix support enhanced the artery's stability by increasing the critical pressure. These results are consistent with the conclusions from our previous model analysis, and the buckling equation accurately predicted the critical pressure of the arteries.
One interesting conclusion from these results is that arteries could buckle and become tortuous not only at hypertensive blood pressure but also at physiological pressures due to a weakened wall or reduced axial strain. Arteries are commonly considered stable under their high internal pressure and axial tension. Here we demonstrated that high luminal pressure and/or reduced axial tension can cause instability of arteries. Arteries in vivo are subjected to longitudinal tensions that change significantly due to aging and cardiovascular disease (37). The reduction in axial strain below physiological levels has been shown to induce tortuosity in rabbit carotid arteries in vivo (28). Arterial elongations due to excessive growth in collateral arteries also lead to tortuosity (5, 12). In contrast, significant axial stretch in normal arteries helps maintain the stability of arteries against buckling. Clinical studies have shown that tortuous arteries are often associated with hypertension and reduced axial stretch. Buckling may occur after vascular surgeries due to reduced stretch ratios or reduced surrounding tissue support after surgery (23, 56). Surgical treatment to shorten the redundant arteries in patients usually shows very positive outcomes in symptomatic patients with tortuous arteries (2, 27), indicating that an increasing axial stretch ratio increases arterial stability and prevents buckling, as predicted by our model. Therefore, axial tension is essential in maintaining the stability of arteries and vascular grafts in preventing tortuosity.
Compared with experimental measurement, the 3D thick-wall cylindrical model with the Fung strain energy function accurately predicted the critical pressure for the arteries tested whereas the 2D thin-walled model showed a tendency to overestimate the critical pressure at higher stretch ratios.
It has been shown that elastase treatment reduces wall stiffness (11). Our results confirmed that the arteries were softer post-elastase treatment, although the changes were less significant in the axial direction than in the circumferential direction. A 2D analysis confirmed that the incremental modulus decreased after elastase treatment (data not shown). Our new finding is that weakened arterial walls also compromise the mechanical stability of arteries by reducing the critical pressure and lead to bent buckling that normally will not happen under physiological pressures. Furthermore, our results from the gel group demonstrated that arteries buckle into sinusoidal shapes of multiple waves when matrix support is around the artery. This phenomenon can be explained using our previous model equation of critical pressure (17):
| (4) |
where ks is the modulus of the surrounding matrix, EI is the bending rigidity of the artery, and n is the wave number of the buckling mode shape (17, 19). According to this equation, without surrounding matrix support (ks = 0), pcr is minimal at n = 1; with matrix support (ks > 0), the second term in the equation increases with increasing wave number n, while the third term decreases. So a minimum buckling pressure is reached at a wave number n > 1. Therefore, arteries buckle into multiple wave shapes within the surrounding tissue matrix, and matrix support increases the critical pressure. In fact, strong tissue support can prevent artery buckling (17). In contrast, a straight artery may develop into wavy tortuous shapes when the surrounding tissues are weakened by degenerative diseases. For example, it was reported that tortuous arterioles occurred with the development of small lacuna cavities (status lacunaris) (41).
The model predictions were vessel specific, using the vessel dimensions and material constants as input. Therefore, variations in vessel diameter and length did not affect, but demonstrated, the predictive value of the model. Note that the pressure diameter curve was obtained while the vessels were free to expand longitudinally. Arterial diameter-pressure relations were often obtained at fixed length in the literature (11, 15). Here we used a free-end inflation test to obtain both axial and cross-sectional deformations, since our previous model demonstrated that arterial buckling depends on both axial and cross-sectional stiffness (17, 18).
One limitation of the study was that the pressure was static while arteries in vivo are under pulsatile pressure and flow. Both theoretical modeling and experimental measurements were done under static pressure for simplicity to capture the main feature of artery buckling and for consistency between the model and experiment. Further work needs to be done to study artery buckling behavior under pulsatile pressure, although a theoretical analysis of artery dynamic stability was recently given by Rachev and Gleason (44, 45). Meanwhile, the use of static model provides an effective approach to estimate the buckling pressure. It is similar to the use of mean pressure to estimate the mean stress and strain in arteries, although arteries are subject to pulsatile pressure. Furthermore, in small arteries and arterioles, as well as veins, the pressure can be approximated as a steady pressure as the pulse pressure is dampened down.
The buckling model was developed for the general situation that included the opening angle. However, our previous simulations using rabbit data from Chuong and Fung (6) showed that the opening angle had very little effect on the critical pressure. The difference reported in our laboratory's 2008 paper (18) was due to an error, and corrections were made in an erratum. Thus, in the current simulations, we did not consider the effect of the opening angle.
Another limitation is that we only tested artery buckling under one gel stiffness to illustrate the effect of gel support vs. without gel support (in PBS). According to our theoretical analysis (Eq. 4) (17), gel stiffness affects the critical pressure. Further studies are needed to experimentally determine the relationship between gel stiffness and critical pressure.
The elastase used for the elastase group was not highly purified elastase. Although there may be some collateral damages in addition to elastin breakdown, no change in collagen content was observed in the elastase-treated arteries, while both photometric measurement and Fastin assay confirmed a 50–60% reduction in the elastin content. The results achieved our goal of illustrating that ECM breakdown will reduce the buckling pressure of arteries.
The 3D model simulations were able to accurately predict the buckling of arteries before elastase treatment, but underestimated the critical pressure after elastase treatment. The small changes in wall dimensions due to elastase treatment were already accounted for in the model simulation by using the posttreatment wall dimensions. We speculate that there may be two possible reasons: first, the lumen layer of the arteries became loose and wavy after elastase treatment, which may have led to errors in the lumen diameter measurement posttreatment. Second, we also observed that the lumen layer of the arterial wall was slightly longer than the outer layer when cut into short ring segments after elastase treatment, indicating a difference in axial length across the layers of the vascular wall (31). This nonuniformity of axial length across the lumen may affect the buckling behavior of the arteries, which was not considered in the buckling equations. Further studies are needed to examine this effect.
While our models predicted a lower than physiological critical pressure for porcine arteries, arteries in vivo normally have a higher critical pressure due to higher axial stretch ratios and surrounding tissue support (17, 18). This was illustrated in our results with surrounding matrix support. Arteries with matrix support buckled with a higher pressure of 22.5 kPa, which is above the normal pressure 16 kPa. Thus arteries in vivo do not buckle under normal conditions. Buckling is accelerated if the arteries are under higher pressures, such as in hypertensive patients, lower stretch ratios shown in aged individuals, and decreased elastin, which is shown in diseased arteries, as well as aged arteries (10, 28, 53). The present study suggests that mechanical instability could be an underlying mechanism to trigger the development of tortuous vessels.
The current results broaden our understanding of vascular biomechanics and shed light on the stability of arteries and veins. A high prevalence of tortuosity has been observed in vessels with hypertensive pressure, diminishing axial stretch, and other vascular diseases (10, 24, 37, 40). We demonstrated that arteries buckle under hypertensive pressure or reduced axial stretch ratios. Buckling would change luminal blood flow and wall stress and result in remodeling of the arterial wall (38, 43, 48, 55). Understanding the biomechanics of blood vessel buckling may have wide implications in vascular physiology and pathology, as well as vascular surgery.
In conclusion, arteries buckle due to a decrease in stretch ratio, wall stiffness, and/or an increase in internal pressure, and buckled arteries exhibit tortuous shapes. Mechanical buckling could be a possible mechanism that leads to artery tortuosity, which is observed in many clinical patients.
GRANTS
This work was supported by CAREER award 0644646 from the National Science Foundation and National Institutes of Health (NIH) Grant R01HL095852. A. Y. Lee was also partially supported by a Minority Biomedical Research Support-Research Initiative for Scientific Enhancement predoctoral fellowship under Grant GM60655 from the NIH.
DISCLOSURES
No conflicts of interest, financial or otherwise, are declared by the author(s).
AUTHOR CONTRIBUTIONS
Author contributions: A.Y.L., B.H., S.D.L., C.A.F., and H.-C.H. performed experiments; A.Y.L., B.H., S.D.L., C.A.F., and H.-C.H. analyzed data; A.Y.L., B.H., S.D.L., C.A.F., and H.-C.H. interpreted results of experiments; A.Y.L. and S.D.L. prepared figures; A.Y.L., B.H., and H.-C.H. drafted manuscript; A.Y.L. and H.-C.H. edited and revised manuscript; A.Y.L., B.H., S.D.L., and H.-C.H. approved final version of manuscript; H.-C.H. conception and design of research.
ACKNOWLEDGMENTS
We thank Watark at Poth, TX and Granzins at New Braunfels for generously providing the artery specimen. We also thank Drs. Yangming Xiao, Danika Hayman, and Yong-Ung Lee, and Ricky Martinez for help in this study.
Appendix
Deformation of cylindrical vessel under pressure and axial tension.
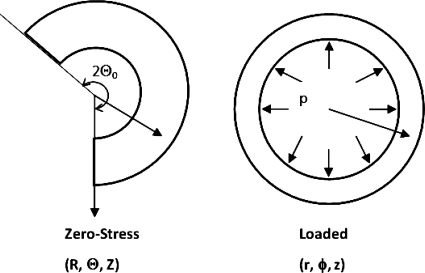
Arteries are modeled as thick-walled, circular cylinders under internal pressure p and axial (longitudinal) tension N with an axial elongation of stretch ratio λz0 (17). We denote the cylindrical coordinates of a material point in the arterial wall to be (R, Θ, Z) in the stress-free state (open sector, with an opening angle of 2Θo), and (r, ϕ, z) in the loaded state (Fig. A1). Thus,
| (A1) |
Fig. A1.
Schematics showing an artery in the zero-stress state with an opening angle of 2Θo and at pressure loaded states. The cylindrical coordinates are defined as (R, Θ, Z) and (r, ϕ, z), respectively.
The stretch ratios in the radial, circumferential, and axial directions are
| (A2) |
The corresponding Green strains (Er, Eθ, Ez) are
| (A3) |
It is seen that the strains are axisymmetric. The circumferential, axial, and radial strains depend on r only, and the axial strain is a constant. The arterial wall was assumed to be incompressible, homogenous, orthotropic, nonlinear elastic and described by the Fung strain energy function (15, 17):
| (A4) |
wherein w0 is the strain energy density, b0 and b1–b6 are material constants, and K is a Lagrangian multiplier.
Accordingly, the stress in the radial, axial, and circumferential directions can be expressed as:
| (A5) |
The equation of equilibrium in the straight axisymmetric vessel become
| (A6) |
With boundary conditions
| (A7) |
where ri and re are lumen and outer radii. Integrating Eq. A6 from re to r and combining with Eqs. A5 and A7 yields
| (A8) |
where ξ is the radial coordinate between re and r.
based on wall incompressibility. Letting r = ri in the first equation of Eq. A8, we have
| (A9) |
The resultant of the stresses on a cross section is only an axial tension N. Integrating σz over the cross-sectional area that yields axial tension N
| (A10) |
Buckling equations for artery segments with fixed ends.
For arteries with fixed ends, our experimental observations and theoretical analysis demonstrated that arteries buckle into a cosine shape (19). The buckling mode shape of the central axis of the vessel is given by:
| (A11) |
where C is a small constant. By assuming that the cross sections of the arteries remain circular when arteries deflect, the radial, circumferential, and axial coordinates of point (r, ϕ, z) after deflection uc are:
| (A12) |
The deformation gradient and strain components can be determined accordingly (17). By neglecting the high-order terms of small deflection uc, the only nonzero incremental strain component due to deflection uc is the axial incremental strain
| (A13) |
This equation indicates that the small bend at buckling leads to non-axisymmetric axial strain, but the circumferential and radial strains remain axisymmetric, and the shear strains remain zero. Accordingly, the change in axial stress can be determined by substituting Ez + ΔEz in the third equation in Eq. A8, and thus the bending moment M(z) can be obtained by integrating the axial stress over the cross-sectional area A
| (A14) |
that yields
| (A15a) |
with
| (A15b) |
wherein
| (A15c) |
On the other hand, the lateral distributed load q generated by the internal pressure due to the deflection uc(z) can be expressed as (18, 19):
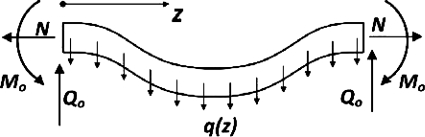
| (A16) |
So the buckled arteries are under this distributed lateral load q(z), axial tension N, reaction force Q0, and reaction moment M0 at the ends (Fig. A2). All of the loads applied to the bent artery are in equilibrium when the artery is buckled. Therefore, the bending moment M(z) can be determined using the equilibrium equations for all the loads. The lateral reaction force Q0 caused by distributed load q(z) equals to
| (A17) |
Fig. A2.
Schematics illustrating an artery buckled under luminal pressure. The reaction forces and moment at the ends as well as the lateral load q(z) generated by the pressure are illustrated. N, axial tension; Q0, reaction force, M0, reaction moment.
Therefore, the bending moment M(z) at axial location z can be determined by
| (A18) |
Taking Eq. A16 into Eq. A18 and integrating yields
| (A19) |
Combining Eqs. A19 and A15a yields:
| (A20) |
Thus, when the pressure reaches the level given in this equation, the artery will achieve equilibrium at the bent shape, and thus buckling occurs. Therefore, this equation (with n = 1) determines the critical buckling pressure of arteries with both ends fixed.
REFERENCES
- 1. Aleksic M, Schutz G, Gerth S, Mulch J. Surgical approach to kinking and coiling of the internal carotid artery. J Card Surg 45: 43–48, 2004 [PubMed] [Google Scholar]
- 2. Ballotta E, Thiene G, Baracchini C, Ermani M, Militello C, Da Giau G, Barbon B, Angelini A. Surgical vs. medical treatment for isolated internal carotid artery elongation with coiling or kinking in symptomatic patients: a prospective randomized clinical study. J Vasc Surg 42: 838–846; discussion 846, 2005 [DOI] [PubMed] [Google Scholar]
- 3. Bergan JJ, Pascarella L, Schmid-Schonbein GW. Pathogenesis of primary chronic venous disease: insights from animal models of venous hypertension. J Vasc Surg 47: 183–192, 2008 [DOI] [PubMed] [Google Scholar]
- 4. Cheung AT, Ramanujam S, Greer DA, Kumagai LF, Aoki TT. Microvascular abnormalities in the bulbar conjunctiva of patients with type 2 diabetes mellitus. Endocr Pract 7: 358–363, 2001 [DOI] [PubMed] [Google Scholar]
- 5. Choy M, Ganesan V, Thomas DL, Thornton JS, Proctor E, King MD, van der Weerd L, Gadian DG, Lythgoe MF. The chronic vascular and haemodynamic response after permanent bilateral common carotid occlusion in newborn and adult rats. J Cereb Blood Flow Metab 26: 1066–1075, 2006 [DOI] [PubMed] [Google Scholar]
- 6. Chuong CJ, Fung YC. On residual stresses in arteries. J Biomech Eng 108: 189–192, 1986 [DOI] [PubMed] [Google Scholar]
- 7. Coucke PJ, Willaert A, Wessels MW, Callewaert B, Zoppi N, De Backer J, Fox JE, Mancini GM, Kambouris M, Gardella R, Facchetti F, Willems PJ, Forsyth R, Dietz HC, Barlati S, Colombi M, Loeys B, De Paepe A. Mutations in the facilitative glucose transporter GLUT10 alter angiogenesis and cause arterial tortuosity syndrome. Nat Genet 38: 452–457, 2006 [DOI] [PubMed] [Google Scholar]
- 8. Davis NP, Han HC, Wayman B, Vito R. Sustained axial loading lengthens arteries in organ culture. Ann Biomed Eng 33: 867–877, 2005 [DOI] [PubMed] [Google Scholar]
- 9. Dawson DL, Hellinger JC, Terramani TT, Najibi S, Martin LG, Lumsden AB. Iliac artery kinking with endovascular therapies: technical considerations. J Vasc Interv Radiol 13: 729–733, 2002 [DOI] [PubMed] [Google Scholar]
- 10. Del Corso L, Moruzzo D, Conte B, Agelli M, Romanelli AM, Pastine F, Protti M, Pentimone F, Baggiani G. Tortuosity, kinking, and coiling of the carotid artery: expression of atherosclerosis or aging? Angiology 49: 361–371, 1998 [DOI] [PubMed] [Google Scholar]
- 11. Dobrin PB, Canfield TR. Elastase, collagenase, and the biaxial elastic properties of dog carotid artery. Am J Physiol Heart Circ Physiol 247: H124–H131, 1984 [DOI] [PubMed] [Google Scholar]
- 12. Eitenmuller I, Volger O, Kluge A, Troidl K, Barancik M, Cai WJ, Heil M, Pipp F, Fischer S, Horrevoets AJ, Schmitz-Rixen T, Schaper W. The range of adaptation by collateral vessels after femoral artery occlusion. Circ Res 99: 656–662, 2006 [DOI] [PubMed] [Google Scholar]
- 13. Fonck E, Feigl GG, Fasel J, Sage D, Unser M, Rufenacht DA, Stergiopulos N. Effect of aging on elastin functionality in human cerebral arteries. Stroke 40: 2552–2556, 2009 [DOI] [PubMed] [Google Scholar]
- 14. Fung YC. Biomechanics: Circulation. New York: Springer, 1997, chapt. 4, p. 206–265 [Google Scholar]
- 15. Fung YC. Biomechanics: Mechanical Properties of Living Tissues. New York: Springer Verlag, 1993 [Google Scholar]
- 16. Han HC. A biomechanical model of artery buckling. J Biomech 40: 3672–3678, 2007 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 17. Han HC. Blood vessel buckling within soft surrounding tissue generates tortuosity. J Biomech 42: 2797–2801, 2009 [DOI] [PubMed] [Google Scholar]
- 18. Han HC. Nonlinear buckling of blood vessels: a theoretical study. J Biomech 41: 2708–2713, 2008 [DOI] [PubMed] [Google Scholar]
- 19. Han HC. The theoretical foundation for artery buckling under internal pressure. J Biomech Eng 131: 124501, 2009 [DOI] [PubMed] [Google Scholar]
- 20. Han HC, Fung YC. Species dependence of the zero-stress state of aorta: pig versus rat. J Biomech Eng 113: 446–451, 1991 [DOI] [PubMed] [Google Scholar]
- 21. Han HC, Ku DN, Vito RP. Arterial wall adaptation under elevated longitudinal stretch in organ culture. Ann Biomed Eng 31: 403–411, 2003 [DOI] [PubMed] [Google Scholar]
- 22. Han HC, Marita S, Ku DN. Changes of opening angle in hypertensive and hypotensive arteries in 3-day organ culture. J Biomech 39: 2410–2418, 2006 [DOI] [PubMed] [Google Scholar]
- 23. Han HC, Zhao L, Huang M, Hou LS, Huang YT, Kuang ZB. Postsurgical changes of the opening angle of canine autogenous vein graft. J Biomech Eng 120: 211–216, 1998 [DOI] [PubMed] [Google Scholar]
- 24. Hiroki M, Miyashita K, Oda M. Tortuosity of the white matter medullary arterioles is related to the severity of hypertension. Cerebrovasc Dis 13: 242–250, 2002 [DOI] [PubMed] [Google Scholar]
- 25. Hoi Y, Gao L, Tremmel M, Paluch RA, Siddiqui AH, Meng H, Mocco J. In vivo assessment of rapid cerebrovascular morphological adaptation following acute blood flow increase. J Neurosurg 109: 1141–1147, 2008 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 26. Humphrey JD. Cardiovascular Solid Mechanics: Cells, Tissues, and Organs. New York: Springer, 2002 [Google Scholar]
- 27. Illuminati G, Ricco JB, Calio FG, D'Urso A, Ceccanei G, Vietri F. Results in a consecutive series of 83 surgical corrections of symptomatic stenotic kinking of the internal carotid artery. Surgery 143: 134–139, 2008 [DOI] [PubMed] [Google Scholar]
- 28. Jackson ZS, Dajnowiec D, Gotlieb AI, Langille BL. Partial off-loading of longitudinal tension induces arterial tortuosity. Arterioscler Thromb Vasc Biol 25: 957–962, 2005 [DOI] [PubMed] [Google Scholar]
- 29. Jakob M, Spasojevic D, Krogmann ON, Wiher H, Hug R, Hess OM. Tortuosity of coronary arteries in chronic pressure and volume overload. Cathet Cardiovasc Diagn 38: 25–31, 1996 [DOI] [PubMed] [Google Scholar]
- 30. Lee AY, Han HC. A thin-walled nonlinear model for vein buckling. Cardiovasc Eng Technol 1: 282–289, 2010 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 31. Lee AY. Determining the critical buckling of blood vessels through modeling and in vitro experiments. In: Biomedical Engineering. San Antonio, TX: University of Texas at San Antonio, 2011 [Google Scholar]
- 32. Leipzig TJ, Dohrmann GJ. The tortuous or kinked carotid artery: pathogenesis and clinical considerations. A historical review. Surg Neurol 25: 478–486, 1986 [DOI] [PubMed] [Google Scholar]
- 33. Martinez R, Fierro CA, Shireman PK, Han HC. Mechanical buckling of veins under internal pressure. Ann Biomed Eng 38: 1345–1353, 2010 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 34. Mathieu-Costello O, Potter RF, Ellis CG, Groom AC. Capillary configuration and fiber shortening in muscles of the rat hindlimb: correlation between corrosion casts and stereological measurements. Microvasc Res 36: 40–55, 1988 [DOI] [PubMed] [Google Scholar]
- 35. Metz H, Murray-Leslie RM, Bannister RG, Bull JW, Marshall J. Kinking of the internal carotid artery. Lancet 1: 424–426, 1961 [DOI] [PubMed] [Google Scholar]
- 36. Nakamura T, Lozano PR, Ikeda Y, Iwanaga Y, Hinek A, Minamisawa S, Cheng CF, Kobuke K, Dalton N, Takada Y, Tashiro K, Ross J, Jr, Honjo T, Chien KR. Fibulin-5/DANCE is essential for elastogenesis in vivo. Nature 415: 171–175, 2002 [DOI] [PubMed] [Google Scholar]
- 37. Nichols WW, O'Rourke MF. McDonald's Blood Flow in Arteries: Theoretical, Experimental, and Clinical Principles (4th Ed.) London: Arnold Publisher, 2005, chapt. 4, p. 67–94 [Google Scholar]
- 38. Northcutt A, Gaddy A, Feng Y, Han HC. A numerical study of blood flow in sinusoidal-shaped arteries. In: Proceedings of the Biomedical Engineering Society (BMES) Annual Fall Meeting. St Louis, MO: BMES, 2008 [Google Scholar]
- 39. Owen CG, Newsom RS, Rudnicka AR, Barman SA, Woodward EG, Ellis TJ. Diabetes and the tortuosity of vessels of the bulbar conjunctiva. Ophthalmology 115: e27–e32, 2008 [DOI] [PubMed] [Google Scholar]
- 40. Pancera P, Ribul M, Presciuttini B, Lechi A. Prevalence of carotid artery kinking in 590 consecutive subjects evaluated by Echocolordoppler. Is there a correlation with arterial hypertension? J Intern Med 248: 7–12, 2000 [DOI] [PubMed] [Google Scholar]
- 41. Poni ES, Liwnicz BH, Ying-Ying Y, North M. Tortuosity of terminal arterioles in the basal ganglia is increased in status lacunaris. Invest Clin 44: 137–145, 2003 [PubMed] [Google Scholar]
- 42. Pries AR, Cornelissen AJ, Sloot AA, Hinkeldey M, Dreher MR, Hopfner M, Dewhirst MW, Secomb TW. Structural adaptation and heterogeneity of normal and tumor microvascular networks. PLoS Comput Biol 5: e1000394, 2009 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 43. Qiao AK, Guo XL, Wu SG, Zeng YJ, Xu XH. Numerical study of nonlinear pulsatile flow in S-shaped curved arteries. Med Eng Phys 26: 545–552, 2004 [DOI] [PubMed] [Google Scholar]
- 44. Rachev A. A theoretical study of mechanical stability of arteries. J Biomech Eng 131: 051006, 2009 [DOI] [PubMed] [Google Scholar]
- 45. Rachev A, Gleason R. Dynamic instability of arteries. Effects of perivascular tissue. In: ASME Summer Bioengineering Conference. Lake Tahoe, CA: ASME, 2009 [Google Scholar]
- 46. Robert L, Robert AM, Fulop T. Rapid increase in human life expectancy: will it soon be limited by the aging of elastin? Biogerontology 9: 119–133, 2008 [DOI] [PubMed] [Google Scholar]
- 47. Sho E, Nanjo H, Sho M, Kobayashi M, Komatsu M, Kawamura K, Xu C, Zarins CK, Masuda H. Arterial enlargement, tortuosity, and intimal thickening in response to sequential exposure to high and low wall shear stress. J Vasc Surg 39: 601–612, 2004 [DOI] [PubMed] [Google Scholar]
- 48. Smedby O, Bergstrand L. Tortuosity and atherosclerosis in the femoral artery: what is cause and what is effect? Ann Biomed Eng 24: 474–480, 1996 [DOI] [PubMed] [Google Scholar]
- 49. Taarnhoj NC, Munch IC, Sander B, Kessel L, Hougaard JL, Kyvik K, Sorensen TI, Larsen M. Straight versus tortuous retinal arteries in relation to blood pressure and genetics. Br J Ophthalmol 92: 1055–1060, 2008 [DOI] [PubMed] [Google Scholar]
- 50. Thore CR, Anstrom JA, Moody DM, Challa VR, Marion MC, Brown WR. Morphometric analysis of arteriolar tortuosity in human cerebral white matter of preterm, young, and aged subjects. J Neuropathol Exp Neurol 66: 337–345, 2007 [DOI] [PubMed] [Google Scholar]
- 51. Vakoc BJ, Lanning RM, Tyrrell JA, Padera TP, Bartlett LA, Stylianopoulos T, Munn LL, Tearney GJ, Fukumura D, Jain RK, Bouma BE. Three-dimensional microscopy of the tumor microenvironment in vivo using optical frequency domain imaging. Nat Med 15: 1219–1223, 2009 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 52. Wagenseil JE, Ciliberto CH, Knutsen RH, Levy MA, Kovacs A, Mecham RP. Reduced vessel elasticity alters cardiovascular structure and function in newborn mice. Circ Res 104: 1217–1224, 2009 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 53. Wagenseil JE, Nerurkar NL, Knutsen RH, Okamoto RJ, Li DY, Mecham RP. Effects of elastin haploinsufficiency on the mechanical behavior of mouse arteries. Am J Physiol Heart Circ Physiol 289: H1209–H1217, 2005 [DOI] [PubMed] [Google Scholar]
- 54. Weibel J, Fields WS. Tortuosity, coiling, and kinking of the internal carotid artery. II. Relationship of morphological variation to cerebrovascular insufficiency. Neurology 15: 462–468, 1965 [DOI] [PubMed] [Google Scholar]
- 55. Wood NB, Zhao SZ, Zambanini A, Jackson M, Gedroyc W, Thom SA, Hughes AD, Xu XY. Curvature and tortuosity of the superficial femoral artery: a possible risk factor for peripheral arterial disease. J Appl Physiol 101: 1412–1418, 2006 [DOI] [PubMed] [Google Scholar]
- 56. Yupu L, Yaotian H, Li Z, Rensheng L, Kaijun S, Ping M, Xiaochao C. Management of major arterial injuries of limbs: a study of 166 cases. Cardiovasc Surg 1: 486–488, 1993 [PubMed] [Google Scholar]
- 57. Zegers ES, Meursing BT, Zegers EB, Oude Ophuis AJ. Coronary tortuosity: a long and winding road. Neth Heart J 15: 191–195, 2007 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 58. Zheng YP, Leung SF, Mak AF. Assessment of neck tissue fibrosis using an ultrasound palpation system: a feasibility study. Med Biol Eng Comput 38: 497–502, 2000 [DOI] [PubMed] [Google Scholar]