Skip to main content
PMC home page
NIHPA Author Manuscripts logoLink to NIHPA Author Manuscripts
. Author manuscript; available in PMC: 2013 Apr 30.
Published in final edited form as: J Biomech. 2012 Mar 3;45(7):1212–1218. doi: 10.1016/j.jbiomech.2012.01.045

A novel in vitro flow system for changing flow direction on endothelial cells

Chong Wang 1,4, Hao Lu 2, Martin Alexander Schwartz 1,3
PMCID: PMC3327813  NIHMSID: NIHMS358235  PMID: 22386042

Abstract

Atherosclerotic plaques localize to regions of flow disturbance, i.e. bifurcations, branch points and regions of high curvature. Shear stress in these regions can be multi-directional due to complex flow patterns such as time-varying vortices. However, commonly used in vitro flow models are incapable of changing flow orientation to any direction other than the reverse. We have developed a novel in vitro flow system to enable changes in flow direction to any angle. When cells were pre-aligned in laminar shear, then rotated 90°, cells re-aligned over 24 hours. Re-alignment involved actin remodeling by gradual rotation of actin stress fibers. This device will enable analysis of how endothelial cells sense changes in flow direction as occur in vivo.

Keywords: Parallel plate device, Shear stress, Endothelial cells, Mechanotransduction, Cell alignment

Introduction

Atherosclerotic plaques can slowly narrow blood vessels to cause ischemia, or vulnerable plaques can rupture and cause myocardial infarction or stroke. Risk factors associated with atherosclerosis include smoking, hypertension, high blood cholesterol, diabetes, etc. However, these factors affect the entire vasculature, whereas atherosclerotic plagues occur mainly near vascular bifurcations, branch points and curves (Asakura,Karino, 1990; DeBakey et al., 1985). Differences in fluid shear stress patterns are proposed to explain the region-specific localization of atherosclerosis (Chatzizisis et al., 2007; Davies, 2008; Malek et al., 1999). Straight segments of arteries experience pulsatile laminar flow with a net forward flow, whereas atherosclerosis-susceptible regions feature flow separation and flow recirculation with complex flow patterns (Ku et al., 1985; Moore et al., 1992; Zhao et al., 2000). For convenience, we group these flow patterns under the term, “disturbed flow”. Many studies have investigated the effects of flow on endothelial cells both in vitro and in vivo (Berk, 2008; Cheng et al., 2006; Chien, 2006, 2008). It has been demonstrated that onset of high unidirectional pulsatile or steady flow causes transient activation of pro-inflammatory and proliferative pathways, however, at longer times, these pathways are downregulated and expression of anti-inflammatory, athero-protective genes are upregulated (Berk, 2008; Chien, 2006). By contrast, disturbed flow patterns cause sustained activation of pro-inflammatory and proliferative pathways (Chien, 2008; Davies, 2008). These results have led to the concept that flow disturbance play a crucial role in development of atherosclerosis.

Three types of in vitro flow systems have been used to study the effects of disturbed flow on endothelial cells (Blackman et al., 2000; Bussolari et al., 1982; Chiu et al., 1998; Frangos et al., 1985; Karino,Goldsmith, 1979; Pritchard et al., 1995). These are: parallel plate flow chambers with uniform spacing in which a syringe pump generates reciprocating shear (Frangos et al., 1985); step flow chambers and tubular sudden expansion flow channels that induce regions of flow separation and reattachment (Chiu et al., 1998; Karino,Goldsmith, 1979; Pritchard et al., 1995); and cone and plate viscometers that simulate complex pulsatile shear stress profile obtained by projecting the instantaneous shear stress vector at individual points on the vessel wall onto its mean flow direction (Blackman et al., 2000; Bussolari et al., 1982; Dai et al., 2004). All of these devices can replicate some features of the local shear stress profiles experienced by endothelial cells in the disturbed flow region. However, none of these systems can change the flow orientation to any direction other than 180°. By contrast, computational analysis based on the actual arterial geometry and flow profiles and direct observations from large transparent artery models and MRI imaging of patients have shown that flow patterns at arterial bifurcations, branch point and regions of curvature are highly complex, with secondary flows including time-varying vortices and helical flows (Frydrychowicz et al., 2008; Frydrychowicz et al., 2009; Ku et al., 1985; Kute,Vorp, 2001; Moore et al., 1992; Stalder et al., 2008; Tsuji et al., 2002; Zhao et al., 2000). As a result, local shear stresses in these regions exhibit directional changes that include a range of angles (Frydrychowicz et al., 2008; Ku et al., 1985). There have been limited attempts to simulate multi-directional flow. One such flow chamber combined rotational flow with forward flow (Lagerquist et al., 2002), however, this flow field was complex and its shear stress profiles have not been characterized. The effects of alternating orthogonal flows on endothelial cells have also been studied, however, this flow chamber could only change flow direction to 90° (Kataoka et al., 1998).

In order to simulate the multi-directional feature of in vivo shear stress profiles, we have developed and validated a novel flow system that can change flow in any direction. As an initial test, responses of shear pre-aligned cells to a single change in flow direction were evaluated for morphology and actin cytoskeleton. This study is the first step toward development of a flow system that can simulate the local shear stress profile from any point on the vessel wall that includes all the directional and magnitude information.

Methods

Description of the flow system

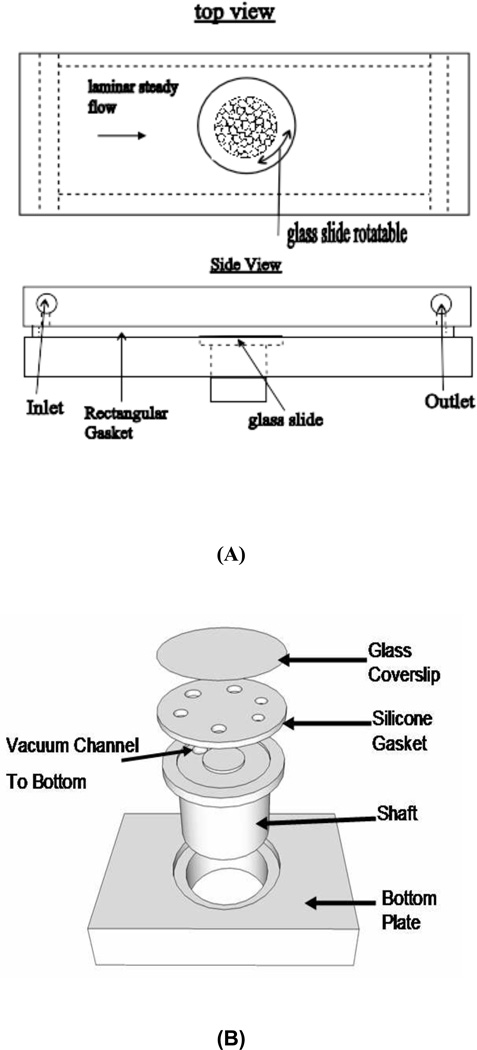
The flow system uses a parallel plate flow chamber with a turntable mechanism built into the bottom plate. A schematic diagram of the device is shown in Fig. 1(A–B). This system consists of a machine-milled polycarbonate top plate, a rectangular silicone gasket, and a polycarbonate bottom plate with a hole and a shaft fitted into the hole. A 40mm diameter glass slide onto which cells are plated is held on top of the shaft by vacuum suction. The top plate, silicone gasket, and bottom plate are held together by screws. The round glass slide is positioned in the same plane as the bottom plate surface to minimize flow disturbances. Cells were seeded only in the central 30 mm diameter area to avoid possible flow disturbances near glass slide edges. Shear direction across the cell monolayer is changed by rotating the shaft. The shaft and the housing hole were made to a tight slip fit such that the shaft can be rotated without leakage of medium. An exploded view of the “turntable” mechanism is shown in Fig. 1B.

Figure 1.

Figure 1

Open in a new tab

Schematic of the flow system. (A): Diagram of the flow system. Rectangular flow channel is formed by a gasket held tight between top and bottom plate. Round glass slides are positioned in line with the bottom plate surface, and can be rotated by rotating the shafts; (B): Rotating mechanism. The vacuum line is connected from the bottom of the shaft to the vacuum channel. The shafts fit into holes very tightly to avoid leakage.

The flow chamber is connected to a peristaltic pump that drives the culture medium through the chamber. Flow pulsation from the pump is eliminated by a pulse dampener (Cole-Parmer, HV-07596-20). Medium returns to the reservoir to make a closed loop. The polycarbonate top plate has two manifolds through which medium enters and exits the channel. The inlet and outlet ports also serve as a bubble traps with a valve opposite the entry port for removal of bubbles. Dimensions of the flow channel are h (channel height) = 0.5mm, b (channel width) = 50mm, l (channel length) = 104mm. The round glass slide is positioned in the middle Shear stress at 12 dynes/cm2 yields Reynolds number of 91 (dynamic fluid viscosity for culture medium DMEM/F12 at 37°C is = 0.78 * 10−3 N.s/m2).

Computational Simulation

The three-dimensional simulations were performed using the OpenFOAM, which is a C++ object oriented library for computational continuum mechanics. The code is capable of solving complex physical models, and has been used in a variety of flows (Jasak, 2009).

Flows in the middle section are laminar, note that the Reynolds number is 18 times smaller than the critical value (1600). However, flows in inflow and outflow sections are considerably dynamic owning to complex geometries and boundary conditions. Thus, we solve large-eddy simulations governed by ∂u¯i∂xi=0,∂u¯i∂t+∂u¯iu¯j∂xj=−∂p¯∂xi−∂τij∂xj+υ∇2u¯i, where p is the effective pressure, υ is the kinematic viscosity and the sub-grid scale (SGS) stress tensor is τij=uiuj¯−u¯iu¯j, which must be closed in terms of the resolved velocity field u̅i. We adopt the widely-used one-equation eddy-viscosity model in this study for two major reasons: (i) this model is developed and carefully tested for achieving isotropic turbulence and anisotropic turbulent cases, such as rotating turbulence and boundary turbulence (Horiuti, 1985; Lu et al., 2008); and (ii) as a one-equation model, the SGS kinetic energy can fade away automatically in the laminar zone.

To determine a satisfactory mesh density for the study, the mesh has been systematically refined throughout the computational domain. The results are found to depend only mildly (not shown here) on the grid resolution under consideration (~175,000 grid points). The time advancement is carried out using a second-order-accurate backward implicit scheme. We set a constant time-step of corresponding to a rather restrictive Courant-Friedrichs-Lewy number of ≈0.1 to suppress the error from the time stepping. Pressure is solved according to pressure-implicit split-operator algorithm (PISO).

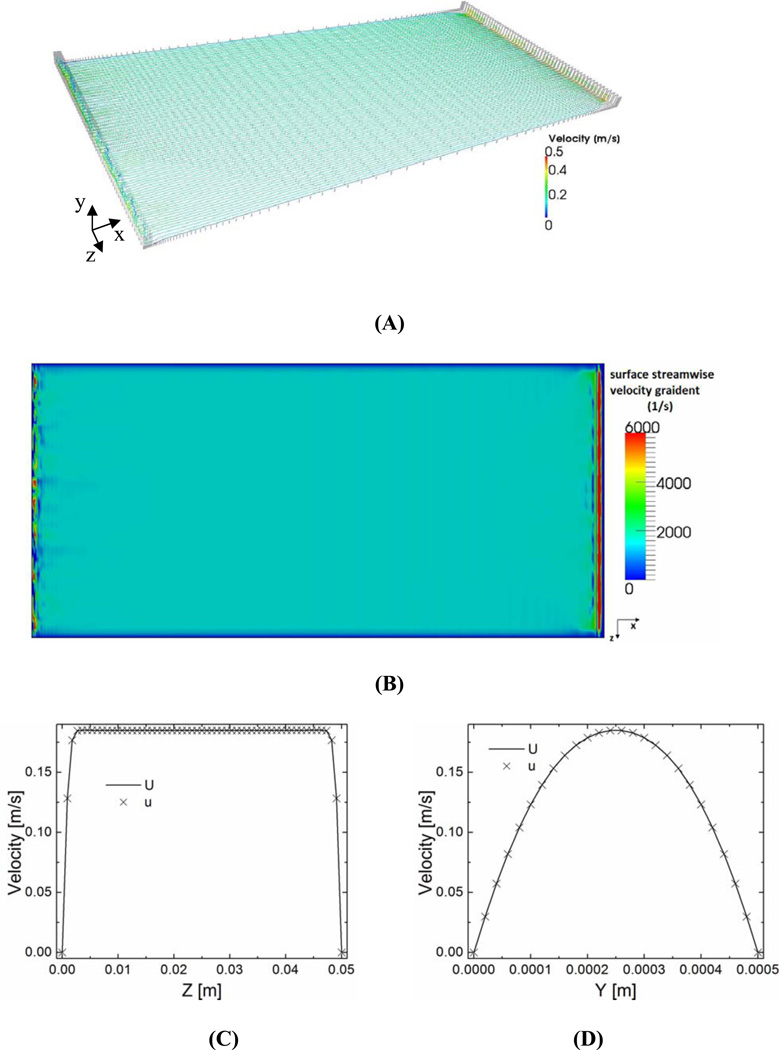
Results from computational simulation are shown in Figure 2. The small gap (0.1mm) between the glass slide edge and the bottom plate surface is ignored because the flow is laminar, and it doesn’t significantly affect the laminar flow as shown in experimental studies (Figure 3).

Figure 2.

Figure 2

Open in a new tab

Computational results. (A): Streamlines (colored with velocity magnitudes) crossing the middle height (y = 0.25 mm). Gray dots represent grid points for computation. Laminar flow is apparent. (B): Filled contour plot of vertical gradient of instantaneous streamwise velocity (proportional to shear stress) on the bottom surface. (C) Instantaneous (×) and time averaged (over 1s, solid line) streamwise velocity profile along the central line of glass slide in the width direction (x = 52mm, y = 0.25mm, z =0–50mm). (D) Instantaneous (×) and time averaged (over 1s, solid line) streamwise velocity profile along the central line of glass slide in the vertical direction (x = 52mm, y = 0–0.5mm, z =25mm).

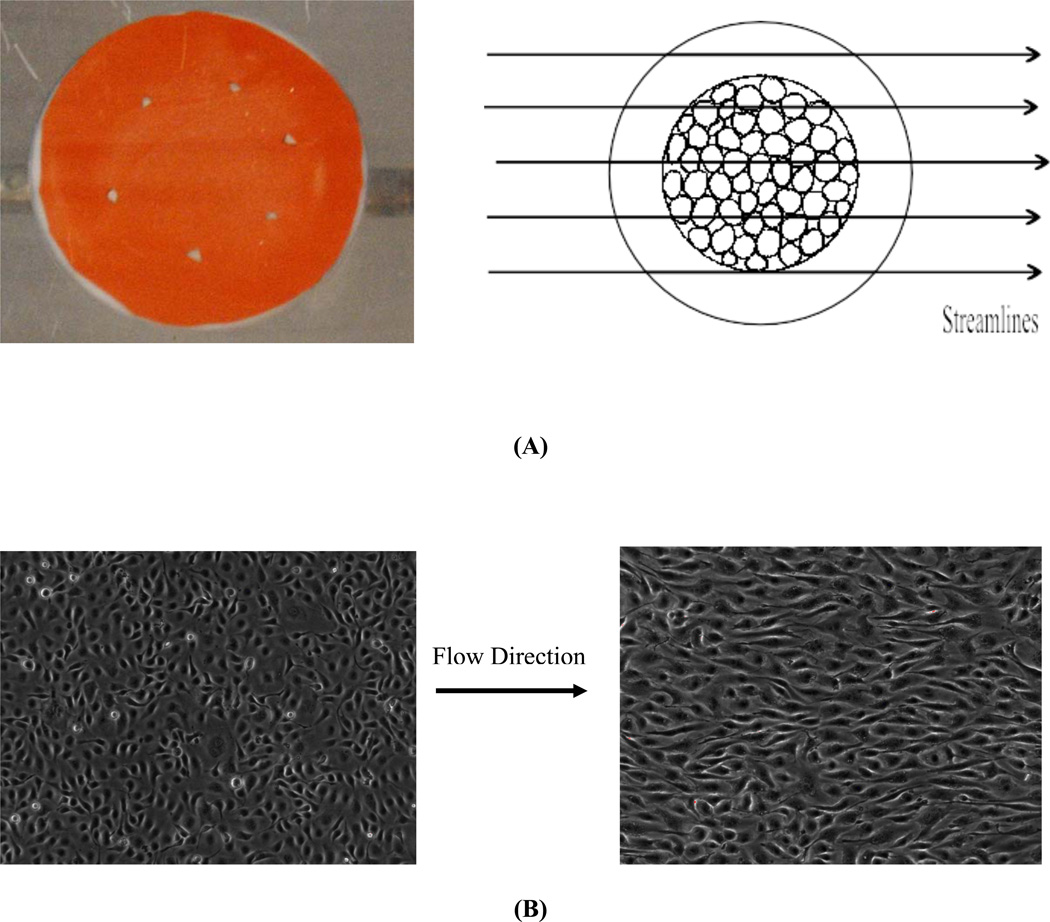
Figure 3.

Figure 3

Open in a new tab

Validation of the flow system. (A, left): Streamlines of flow over the glass slide were visualized using rheoscopic fluid. (A, right) Schematic of the relation between the flow field over the glass slide and the area of seeded cells. (B, left) Bovine aortic endothelial cells in static condition right before the alignment experiment. (B, right) Cells aligned to the flow direction (left to right) after 24h of shear at 12 dynes/cm2.

Experimental Validation of the flow system

To test whether flow over the round glass slide is laminar, we first visualized flow using rheoscopic fluid (kalliroscope.com), which makes streamlines visible with appropriate illumination. Fig. 3A shows laminar streamlines unhindered by the glass slide. Next, endothelial cells in the central regions were sheared for 24h at 12 dynes/cm2 (detailed protocols are provided in the next section). These cells showed obvious alignment in the flow direction (Fig. 3B), a response that is known to require laminar flow (Levesque,Nerem, 1985). Together, these data indicate that the chamber is suitable for studying cell behavior in laminar flow.

Cell culture and application of shear stress

Primary bovine aortic endothelial cells (BAECs) were purchased from VEC Technologies (Rensselaer, NY). BAECs were used from passage 8 to 10. Cells were maintained in DMEM/F12 media (Invitrogen 11320), supplemented with 10% fetal bovine serum (FBS; Atlanta Biologicals), 10 U/ml penicillin and 10 µg/mL streptomycin (Invitrogen). The central 30 mm of 40 mm diameter round glass slides (1.2mm thick, custom-made, Electron Microscopy Sciences, Hatfield, PA) were coated overnight with 20 µg/mL fibronectin using a silicone gasket to block the edges. Cells were seeded in this region and allowed to form a confluent monolayer overnight. The glass disc was then loaded onto the top of the shaft with a silicone gasket (0.5mm, Grace Bio-Labs, Bend, Oregon) underneath it to prevent leaking, vacuum was applied and the two plates and with silicone gasket were held together by screws to form the parallel plate flow channel. Cells were exposed to laminar shear stress at 12 dynes/cm2 for 24 hours to induce alignment. The shaft was then rotated 90°. Re-alignment was examined at subsequent times by phase contrast microscopy of unfixed monolayers. Monolayers were then fixed and stained for F-actin and for nuclei as described below. A maximum of three flow chambers were connected in series in the flow loop to enable multiple time points for each experiment.

Imaging Analysis and Immunofluorescence

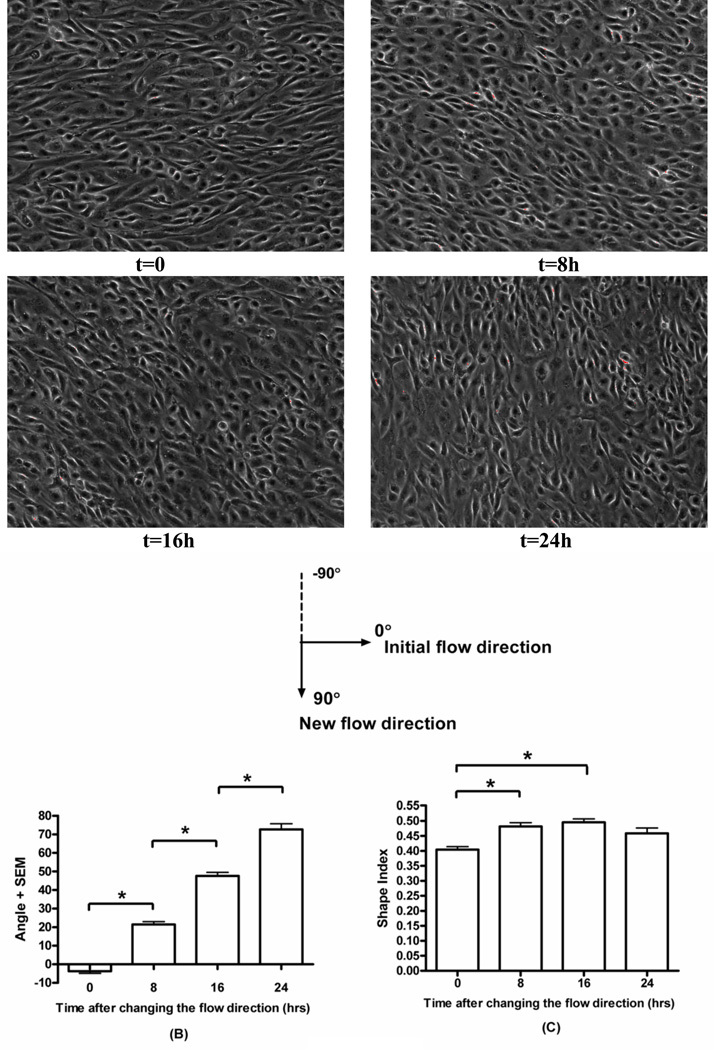
Phase-contrast images taken at 10× were analyzed for geometrical parameters such as shape index and cell orientations. A Matlab program developed by Dr. Brian Helmke’s lab at UVA was used to trace the boundaries of cells from the images and compute shape index and cell orientation from cell borders as previously described (Lin,Helmke, 2008). Shape index is defined as 4πA/P2 (A: cell area, P: cell perimeter), thus, shape index is 1.0 for a circle and 0 for a line. An ellipse was fitted to the individual cell outlines and the angle of cell orientation (−90 degrees to + 90 degrees) was defined as the angle between the primary axis and the initial flow direction. The initial flow direction is 0°, and the new flow direction is 90°, as illustrated in Figure 4.

Figure 4.

Figure 4

Open in a new tab

Re-alignment of cell morphology. BAECs were pre-conditioned by shear (12 dynes/cm2, 24h). Initial flow direction was from left to right, then was changed to vertical (up to down). t indicates time after the direction change. A): Phase-contrast images of shear pre-conditioned endothelial cell monolayers exposed to the vertical flow for indicated times. B) Orientation angle as a function of time of exposure to vertical shear. Values are means ± SEM. (*P<0.001, n=3). C) Shape index as a function of time of exposure to the vertical flow. (*P<0.001, n=3).

For visualizing F-actin, cells were fixed with PBS containing 3.7% formaldehyde for 10 min., permeabilized with 0.2% Triton X-100 for 5 min., incubated with 1:100 Alexa 568-conjugated phalloidin (Molecular Probes) for 1 hour at room temperature, rinsed three times with in PBS, incubated with DAPI (1:1000) for 5 minutes to stain nuclei, and mounted with Fluoromount G. Images were captured using a Zeiss LSM510 scanning confocal microscope with a 40× oil immersion lens.

Statistical analysis

At least three experiments were performed for each condition. Statistical differences among experimental groups were evaluated with analysis of variance (ANOVA) using Tukey post-tests. P-values of 0.05 were considered statistically significant. At least 60 cells from each glass disc were analyzed.

Results

Construction of a novel flow system and validation of the fluid mechanics

We have built and validated a novel in vitro flow system where laminar flow direction can be changed by any angle. The system achieves the flow direction change with a turntable mechanism in the bottom plate of a conventional parallel-plate design (Fig. 1). By rotating the shaft, flow direction is changed relative to the initial orientation.

Computational results are summarized in Figure 2. Figure 2A shows laminar flow in the flow chamber, and shear stress distribution on the bottom plate surface is very uniform especially in the middle section where the round glass slide resides as illustrated in Fig. 2B. Figure 2C further confirms this uniformity by showing the streamwise velocity is the same across the glass slide (40mm in diameter). Parabolic flow profile in Fig. 2D justifies an excellent correlation to a laminar profile (second-order polynomial, coefficient of determination R2=0.99912).

Flow visualization using rheoscopic fluid showed laminar streamlines across the glass disc (Fig 3A). Cells aligned parallel to the flow direction, consistent with laminar shear (Fig 3B). Taken together, these data provide strong evidence for laminar shear in this system.

Morphological changes during the re-alignment process

In vivo, arterial endothelial cells in straight parts of the artery have been shown to re-align after flow direction is changed by 90° (Flaherty et al., 1972). However, systematic in vitro studies of this realignment process have not been reported. We therefore examined how pre-aligned cells re-align following a 90° change in flow direction. Cells responded to the change in flow direction essentially by rotating towards the new direction at a steady angular speed of approximately 25°/8h (Fig. 4A–B). There was a small change in cell shape as cells became slightly more rounded, however, they remained substantially elongated such that orientation direction could easily be determined (Fig. 4C). Elongation recovered to control levels toward the end of re-alignment.

Actin remodeling

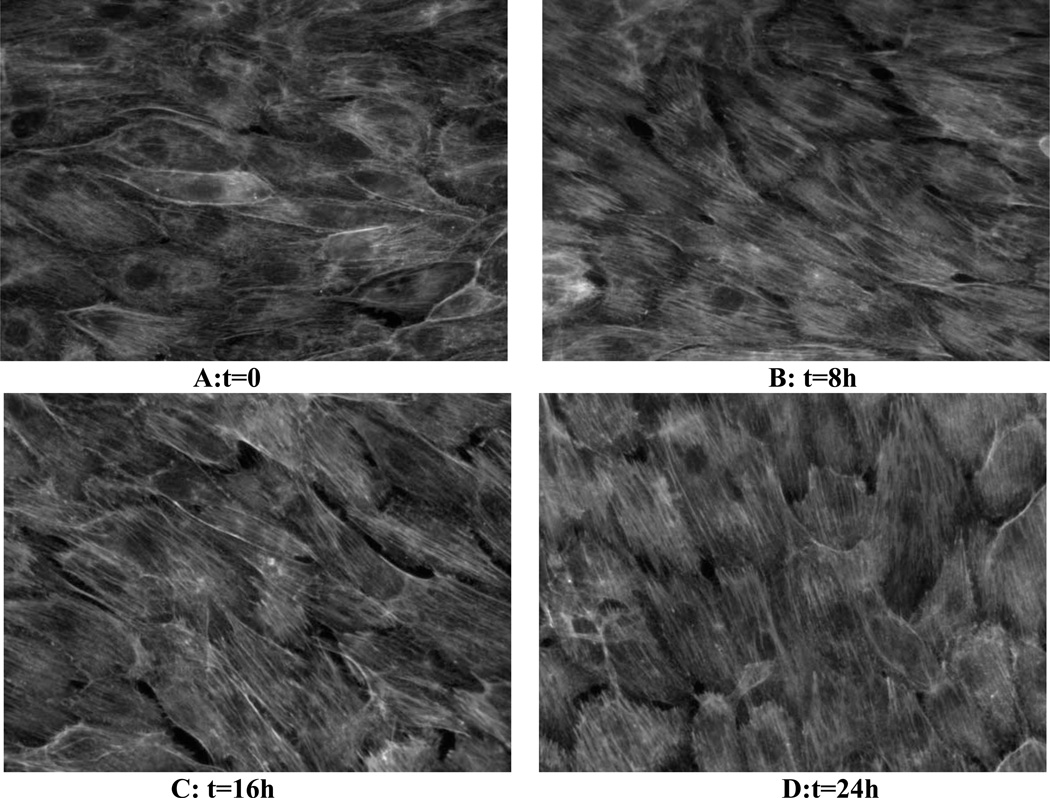
Alignment of F-actin stress fibers is a central component of endothelial alignment in the direction of flow (Noria et al., 2004). We therefore examined F-actin in cells fixed at different times after changing flow direction. Consistent with the changes in cell shape in Fig 4, actin stress fibers responded to a 90° change in flow direction by rotating toward the new axis (Fig. 5).

Figure 5.

Figure 5

Open in a new tab

Re-alignment of the actin cytoskeleton. Endothelial cells were conditioned to flow (horizontal) for 24h (A) then were exposed to the new flow direction (vertical) for the indicated times (B–D). Cells were fixed and stained for F-actin. Results show gradual rotation toward the new flow direction.

Discussion

The effects of disturbed flow on endothelial cells are commonly studied using in vitro flow systems (Blackman et al., 2000; Bussolari et al., 1982; Chiu et al., 1998; Frangos et al., 1985; Karino,Goldsmith, 1979; Pritchard et al., 1995). An important limitation of current in vitro systems is their inability to change the flow to any direction other than 180°. However, in vivo shear stresses at regions of disturbed flow can be multidirectional due to complex flow patterns such as time-varying vortices and helical flows (Frydrychowicz et al., 2008; Frydrychowicz et al., 2009; Ku et al., 1985; Kute,Vorp, 2001; Moore et al., 1992; Stalder et al., 2008; Tsuji et al., 2002; Zhao et al., 2000). In the present study, we designed, built and validated a flow system that enables flow direction to be changed in any direction. Responses of flow-aligned cells to a single change in flow direction were analyzed, and both morphological changes and actin cytoskeleton were evaluated.

To ensure laminar flow, multiple design factors needed to be optimized. First, cell substrates used in the experiments need to have a very flat surface. Thicker glass slides (1.2mm) are chosen over regular cover slips (0.13–0.19mm) because the latter deform under vacuum pressure in our tests. Due to the small channel height (0.5 mm), even slight curvature can negatively affect flow patterns. Second, channel height must be optimized since one that is too small will amply imperfections in the alignment of glass slide with the bottom plate surface, whereas an unnecessary large one requires faster flow rates that might induce non-laminar flows. Third, the round glass slide must be positioned in the same plane with the bottom plate surface. All the parts of the turntable mechanism were made to precise dimensions with highest possible tolerance, and we found in practice that visual inspection was sufficient for detecting misalignment of glass slides.

We also found that cell confluency and shear stress magnitude strongly affected the results. Over-confluent cells aligned but re-aligned slowly (data not shown). Cells were therefore used just as they reached confluence for all experiments. For shear stress, low levels gave poor initial alignment and re-alignment appeared much less homogeneously with large variations in cell orientations and shape index. Shear stress at 12 dynes/cm2 was found to be sufficient.

Re-alignment of pre-aligned endothelial in vivo has been reported (Flaherty et al., 1972), however, to the best of our knowledge, this is the first study to study the re-alignment in detail. Our results revealed that shear pre-conditioned cells re-align by gradually rotating the entire culture towards the new flow direction. The actin stress fibers also rotate in this process. This rotation of the cell and actin cytoskeleton, in which cells are synchronized with each other, is novel and to our knowledge has not been previously reported. It is distinct from the heterogeneous morphodynamics of the alignment of naïve cultures under laminar shear, and points toward a mechanism for sensing flow direction that is at present not understood. Work is currently under way to study this rotation in real time at higher spatial resolution with tracking of both actin and adhesion dynamics. The glass slide used in the current setup is too thick for imaging, so a new vacuum mechanism is being designed to accommodate No. 1.5 coverslip for hi-resolution imaging.

The main goal of this study is to understand how endothelial cells sense flow direction. The current flow system enables us to study how endothelial cells in the athero-resistant parts of arteries, where cells are aligned, sense direction change. And we plan to test the hypothesis that flow-aligned cells desensitize themselves to perpendicular flow in initial signaling responses, therefore providing a physiological benefit. To investigate how cells in athero-prone parts of arteries, where flow is multi-directional and cells are not aligned, sense changing flow direction, an automatically controlled flow chamber capable of continuous changes in flow direction is currently being built. This device will allow us to investigate the biological effects of time varying off-axis flow on endothelial cells phenotype in disturbed flow regions.

Conclusions

We have developed and validated a novel in vitro flow system that can align endothelial cells and then change flow in any direction. When flow-aligned cells were subject to a single change in flow direction, cells re-align to the new direction by gradual rotation. Further studies might provide new insights into cytoskeletal dynamics that can constrain possible mathematical models. Furthermore, this work represents a first step in efforts to replicate physiologically realistic shear stress profiles in vitro with all the directional and magnitude information.

Acknowledgments

The authors thank Dr. Brett Blackman for providing bovine aortic endothelial cells and Dr. Brian Helmke for providing the Matlab routine for image analysis. This study is supported by a post-doctoral fellowship from an American Heart Association fellowship (#10POST4140009) to CW and USPHS grant RO1 HL75092 to MAS. Computing resources for numerical simulations are provided by the Minnesota Supercomputing Institute.

Footnotes

Publisher's Disclaimer: This is a PDF file of an unedited manuscript that has been accepted for publication. As a service to our customers we are providing this early version of the manuscript. The manuscript will undergo copyediting, typesetting, and review of the resulting proof before it is published in its final citable form. Please note that during the production process errors may be discovered which could affect the content, and all legal disclaimers that apply to the journal pertain.

Conflict of interest

None declared.

References

  1. Asakura T, Karino T. Flow patterns and spatial distribution of atherosclerotic lesions in human coronary arteries. Circulation Research. 1990;66:1045–1066. doi: 10.1161/01.res.66.4.1045. [DOI] [PubMed] [Google Scholar]
  2. Berk B. Atheroprotective Signaling Mechanisms Activated by Steady Laminar Flow in Endothelial Cells. Circulation. 2008;117:1082–1089. doi: 10.1161/CIRCULATIONAHA.107.720730. [DOI] [PubMed] [Google Scholar]
  3. Blackman B, Barbee K, Thibault L. In vitro cell shearing device to investigate the dynamic response of cells in a controlled hydrodynamic environment. Annals of Biomedical Engineering. 2000;28:363–372. doi: 10.1114/1.286. [DOI] [PubMed] [Google Scholar]
  4. Bussolari S, Dewey C, Gimbrone M. Apparatus for subjecting living cells to fluid shear stress. Review of Scientific Instruments. 1982;53:1851. doi: 10.1063/1.1136909. [DOI] [PubMed] [Google Scholar]
  5. Chatzizisis Y, Coskun A, Jonas M, Edelman E, Feldman C, Stone P. Role of endothelial shear stress in the natural history of coronary atherosclerosis and vascular remodelingmolecular, cellular, and vascular behavior. Journal of the American College of Cardiology. 2007;49:2379–2393. doi: 10.1016/j.jacc.2007.02.059. [DOI] [PubMed] [Google Scholar]
  6. Cheng C, Tempel D, Van Haperen R, Van Der Baan A, Grosveld F, Daemen M, Krams R, De Crom R. Atherosclerotic lesion size and vulnerability are determined by patterns of fluid shear stress. Circulation. 2006;113:2744–2753. doi: 10.1161/CIRCULATIONAHA.105.590018. [DOI] [PubMed] [Google Scholar]
  7. Chien S. Mechanotransduction and endothelial cell homeostasis: the wisdom of the cell. AJP: Heart and Circulatory Physiology. 2006;292:H1209–H1224. doi: 10.1152/ajpheart.01047.2006. [DOI] [PubMed] [Google Scholar]
  8. Chien S. Effects of Disturbed Flow on Endothelial Cells. Annals of Biomedical Engineering. 2008;36:554–562. doi: 10.1007/s10439-007-9426-3. [DOI] [PMC free article] [PubMed] [Google Scholar]
  9. Chiu J, Wang D, Chien S, Skalak R, Usami S. Effects of Disturbed Flow on Endothelial Cells. Journal of Biomechanical Engineering. 1998;120:2–8. doi: 10.1115/1.2834303. [DOI] [PubMed] [Google Scholar]
  10. Dai G, Kaazempur-Mofrad M, Natarajan S, Zhang Y, Vaughn S, Blackman B, Kamm R, Garcia-Cardena G, Gimbrone M. Distinct endothelial phenotypes evoked by arterial waveforms derived from atherosclerosis-Susceptible and -Resistant regions of human vasculature. Proceedings of the National Academy of Sciences. 2004;101:14871–14876. doi: 10.1073/pnas.0406073101. [DOI] [PMC free article] [PubMed] [Google Scholar]
  11. Davies P. Hemodynamic shear stress and the endothelium in cardiovascular pathophysiology. Nature Clinical Practice Cardiovascular Medicine. 2008;6:16–26. doi: 10.1038/ncpcardio1397. [DOI] [PMC free article] [PubMed] [Google Scholar]
  12. DeBakey M, Lawrie G, Glaeser D. Patterns of Atherosclerosis and their Surgical Significance. Annals of Surgery. 1985;201:115–131. doi: 10.1097/00000658-198502000-00001. [DOI] [PMC free article] [PubMed] [Google Scholar]
  13. Flaherty JT, Ferrans VJ, Tucker WK, Fry DL, Patel DJ, Pierce JE. Endothelial Nuclear Patterns In Canine Arterial Tree With Particular Reference To Hemodynamic Events. Circulation Research. 1972;30 doi: 10.1161/01.res.30.1.23. 23-&. [DOI] [PubMed] [Google Scholar]
  14. Frangos J, Eskin S, Mcintire L, Ives C. Flow effects on prostacyclin production by cultured human endothelial cells. Science. 1985;227:1477–1479. doi: 10.1126/science.3883488. [DOI] [PubMed] [Google Scholar]
  15. Frydrychowicz A, Arnold R, Hirtler D, Schlensak C, Stalder AF, Hennig J, Langer M, Markl M. Multidirectional flow analysis by cardiovascular magnetic resonance in aneurysm development following repair of aortic coarctation. Journal of cardiovascular magnetic resonance: official journal of the Society for Cardiovascular Magnetic Resonance. 2008;10:30. doi: 10.1186/1532-429X-10-30. [DOI] [PMC free article] [PubMed] [Google Scholar]
  16. Frydrychowicz A, Stalder AF, Russe MF, Bock J, Bauer S, Harloff A, Berger A, Langer M, Hennig J, Markl M. Three-dimensional analysis of segmental wall shear stress in the aorta by flow-sensitive four-dimensional-MRI. Journal of magnetic resonance imaging: JMRI. 2009;30:77. doi: 10.1002/jmri.21790. [DOI] [PubMed] [Google Scholar]
  17. Horiuti K. Large eddy simulation of turbulent channel flow by one-equation modeling. J. Phys. Soc. Jpn. 1985;54:2855–2865. [Google Scholar]
  18. Jasak H. Openfoam: Open source cfd in research and industry. Inter J. Nav. Archit. Oc. Engng. 2009:89–94. [Google Scholar]
  19. Karino T, Goldsmith H. Adhesion of human platelets to collagen on the walls distal to a tubular expansion. Microvascular Research. 1979;17:238–262. doi: 10.1016/s0026-2862(79)80002-3. [DOI] [PubMed] [Google Scholar]
  20. Kataoka N, Ujita S, Sato M. Effect of flow direction on the morphological responses of cultured bovine aortic endothelial cells. Medical & Biological Engineering & Computing. 1998;36:122–128. doi: 10.1007/BF02522869. [DOI] [PubMed] [Google Scholar]
  21. Ku D, Giddens D, Zarins C, Glagov S. Pulsatile flow and atherosclerosis in the human carotid bifurcation. Positive correlation between plaque location and low oscillating shear stress. Arteriosclerosis. 1985;5:293–302. doi: 10.1161/01.atv.5.3.293. [DOI] [PubMed] [Google Scholar]
  22. Kute SM, Vorp Da. The Effect of Proximal Artery Flow on the Hemodynamics at the Distal Anastomosis of a Vascular Bypass Graft: Computational Study. Journal of Biomechanical Engineering. 2001;123:277. doi: 10.1115/1.1374203. [DOI] [PubMed] [Google Scholar]
  23. Lagerquist K, Suvatne J, Barakat A. Multi-Directional flow chamber: analysis of endothelial cell morphology dependence on differential shear forces; Proceedings of the Second Joint 24th Annual Conference and the Annual Fall Meeting of the Biomedical Engineering Society] [Engineering in Medicine and Biology. IEEE; 2002. pp. 658–659. [Google Scholar]
  24. Levesque MJ, Nerem RM. The Elongation and Orientation of Cultured Endothelial Cells in Response to Shear Stress. Journal of Biomechanical Engineering. 1985;107 doi: 10.1115/1.3138567. [DOI] [PubMed] [Google Scholar]
  25. Lin X, Helmke B. Micropatterned structural control suppresses mechanotaxis of endothelial cells. Biophys. J. 2008;95:3066–3078. doi: 10.1529/biophysj.107.127761. [DOI] [PMC free article] [PubMed] [Google Scholar]
  26. Lu H, Rutland C, Smith L. A posteriori tests of one-equation LES modeling of rotating turbulence. Int. J. Mod. Phys. C. 2008:1949–1964. [Google Scholar]
  27. Malek A, Alper S, Izumo S. Hemodynamic shear stress and its role in atherosclerosis. JAMA: The Journal of the American Medical Association. 1999;282:2035–2042. doi: 10.1001/jama.282.21.2035. [DOI] [PubMed] [Google Scholar]
  28. Moore J, Ku D, Zarins C, Glagov S. Pulsatile flow visualization in the abdominal aorta under differing physiologic conditions: implications for increased susceptibility to atherosclerosis. Journal of Biomechanical Engineering. 1992;114:391. doi: 10.1115/1.2891400. [DOI] [PubMed] [Google Scholar]
  29. Noria S, Xu F, McCue S, Jones M, Gotlieb AI, Langille BL. Assembly and Reorientation of Stress Fibers Drives Morphological Changes to Endothelial Cells Exposed to Shear Stress. American Journal of Pathology. 2004;164:1211–1223. doi: 10.1016/S0002-9440(10)63209-9. [DOI] [PMC free article] [PubMed] [Google Scholar]
  30. Pritchard W, Davies P, Derafshi Z, Polacek D, Tsao R, Dull R, Jones S, Giddens D. Effects of wall shear stress and fluid recirculation on the localization of circulating monocytes in a three-Dimensional flow model. Journal of Biomechanics. 1995;28:1459–1469. doi: 10.1016/0021-9290(95)00094-1. [DOI] [PubMed] [Google Scholar]
  31. Stalder A, Russe MF, Frydrychowicz A, Bock J, Hennig J, Markl M. Quantitative 2D and 3D phase contrast MRI: optimized analysis of blood flow and vessel wall parameters. Magnetic resonance in medicine: official journal of the Society of Magnetic Resonance in Medicine / Society of Magnetic Resonance in Medicine. 2008;60:1218. doi: 10.1002/mrm.21778. [DOI] [PubMed] [Google Scholar]
  32. Tsuji T, Suzuki J-i, Shimamoto R, Yamazaki T, Nakajima T, Nagai R, Komatsu S, Ohtomo K, Toyo-Oka T, Omata M. Vector analysis of the wall shear rate at the human aortoiliac bifurcation using cine MR velocity mapping. AJR. American journal of roentgenology. 2002;178:995. doi: 10.2214/ajr.178.4.1780995. [DOI] [PubMed] [Google Scholar]
  33. Zhao S, Xu X, Hughes A, Thom S, Stanton A, Ariff B, Long Q. Blood flow and vessel mechanics in a physiologically realistic model of a human carotid arterial bifurcation. Journal of Biomechanics. 2000;33:975–984. doi: 10.1016/s0021-9290(00)00043-9. [DOI] [PubMed] [Google Scholar]

RESOURCES