Greater focus needed on methane leakage from natural gas infrastructure

Abstract

Natural gas is seen by many as the future of American energy: a fuel that can provide energy independence and reduce greenhouse gas emissions in the process. However, there has also been confusion about the climate implications of increased use of natural gas for electric power and transportation. We propose and illustrate the use of technology warming potentials as a robust and transparent way to compare the cumulative radiative forcing created by alternative technologies fueled by natural gas and oil or coal by using the best available estimates of greenhouse gas emissions from each fuel cycle (i.e., production, transportation and use). We find that a shift to compressed natural gas vehicles from gasoline or diesel vehicles leads to greater radiative forcing of the climate for 80 or 280 yr, respectively, before beginning to produce benefits. Compressed natural gas vehicles could produce climate benefits on all time frames if the well-to-wheels CH₄ leakage were capped at a level 45–70% below current estimates. By contrast, using natural gas instead of coal for electric power plants can reduce radiative forcing immediately, and reducing CH₄ losses from the production and transportation of natural gas would produce even greater benefits. There is a need for the natural gas industry and science community to help obtain better emissions data and for increased efforts to reduce methane leakage in order to minimize the climate footprint of natural gas.

With growing pressure to produce more domestic energy and to reduce greenhouse gas (GHG) emissions, natural gas is increasingly seen as the fossil fuel of choice for the United States as it transitions to renewable sources. Recent reports in the scientific literature and popular press have produced confusion about the climate implications of natural gas (1–5). On the one hand, a shift to natural gas is promoted as climate mitigation because it has lower carbon per unit energy than coal or oil (6). On the other hand, methane (CH₄), the prime constituent of natural gas, is itself a more potent GHG than carbon dioxide (CO₂); CH₄ leakage from the production, transportation and use of natural gas can offset benefits from fuel-switching.

The climatic effect of replacing other fossil fuels with natural gas varies widely by sector (e.g., electricity generation or transportation) and by the fuel being replaced (e.g., coal, gasoline, or diesel fuel), distinctions that have been largely lacking in the policy debate. Estimates of the net climate implications of fuel-switching strategies should be based on complete fuel cycles (e.g., “well-to-wheels”) and account for changes in emissions of relevant radiative forcing agents. Unfortunately, such analyses are weakened by the paucity of empirical data addressing CH₄ emissions through the natural gas supply network, hereafter referred to as CH₄ leakage.* The U.S. Environmental Protection Agency (EPA) recently doubled its previous estimate of CH₄ leakage from natural gas systems (6).

In this paper, we illustrate the importance of accounting for fuel-cycle CH₄ leakage when considering the climate impacts of fuel-technology combinations. Using EPA’s estimated CH₄ emissions from the natural gas supply, we evaluated the radiative forcing implications of three U.S.-specific fuel-switching scenarios: from gasoline, diesel fuel, and coal to natural gas.

A shift to natural gas and away from other fossil fuels is increasingly plausible because advances in horizontal drilling and hydraulic fracturing technologies have greatly expanded the country’s extractable natural gas resources particularly by accessing gas stored in shale deep underground (7). Contrary to previous estimates of CH₄ losses from the “upstream” portions of the natural gas fuel cycle (8, 9), a recent paper by Howarth et al. calculated upstream leakage rates for shale gas to be so large as to imply higher lifecycle GHG emissions from natural gas than from coal (1). (SI Text, discusses differences between our paper and Howarth et al.) Howarth et al. estimated CH₄ emissions as a percentage of CH₄ produced over the lifecycle of a well to be 3.6–7.9% for shale gas and 1.7–6.0% for conventional gas. The EPA’s latest estimate of the amount of CH₄ released because of leaks and venting in the natural gas network between production wells and the local distribution network is about 570 billion cubic feet for 2009, which corresponds to 2.4% of gross U.S. natural gas production (1.9–3.1% at a 95% confidence level) (6).^† EPA’s reported uncertainty appears small considering that its current value is double the prior estimate, which was itself twice as high as the previously accepted amount (9).

Comparing the climate implications of CH₄ and CO₂ emissions is complicated because of the much shorter atmospheric lifetime of CH₄ relative to CO₂. On a molar basis, CH₄ produces 37 times more radiative forcing than CO₂.^‡ However, because CH₄ is oxidized to CO₂ with an effective lifetime of 12 yr, the integrated, or cumulative, radiative forcings from equi-molar releases of CO₂ and CH₄ eventually converge toward the same value. Determining whether a unit emission of CH₄ is worse for the climate than a unit of CO₂ depends on the time frame considered. Because accelerated rates of warming mean ecosystems and humans have less time to adapt, increased CH₄ emissions due to substitution of natural gas for coal and oil may produce undesirable climate outcomes in the near-term.

The concept of global warming potential (GWP) is commonly used to compare the radiative forcing of different gases relative to CO₂ and represents the ratio of the cumulative radiative forcing t years after emission of a GHG to the cumulative radiative forcing from emission of an equivalent quantity of CO₂ (10). The Intergovernmental Panel on Climate Change (IPCC) typically uses 100 yr for the calculation of GWP. Howarth et al. (1) emphasized the 20-year GWP, which accentuates the large forcing in early years from CH₄ emissions, whereas Venkatesh et al. (2) adopted a 100-yr GWP and Burnham et al. (4) utilized both 20- and 100-yr GWPs.

GWPs were established to allow for comparisons among GHGs at one point in time after emission but only add confusion when evaluating environmental benefits or policy tradeoffs over time. Policy tradeoffs like the ones examined here often involve two or more GHGs with distinct atmospheric lifetimes. A second limitation of GWP-based comparisons is that they only consider the radiative forcing of single emission pulses, which do not capture the climatic consequences of real-world investment and policy decisions that are better simulated as emission streams.

To avoid confusion and enable straightforward comparisons of fuel-technology options, we suggest that plotting as a function of time the relative radiative forcing of the options being considered would be more useful for policy deliberations than GWPs. These technology warming potentials (TWP) require exactly the same inputs and radiative forcing formulas used for GWP but reveal time-dependent tradeoffs inherent in a choice between alternative technologies. We illustrate the value of our approach by applying it to emissions of CO₂ and CH₄ from vehicles fueled with CNG compared with gasoline or diesel vehicles and from power plants fueled with natural gas instead of coal.

Wigley also analyzed changes in the relative benefits over time of switching from coal to natural gas, but that was done in the context of additional complexities including specific assumptions about the global pace of technological substitution, emissions of sulfur dioxide and black carbon, and a specific model of global warming due to radiative forcing (5). We compare our results with Wigley’s in the next section.

Results and Discussion

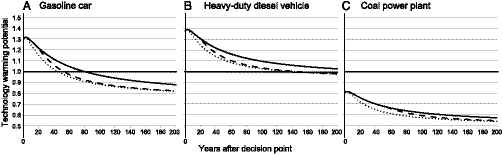
We focus on the TWPs of real-world choices faced by individuals, corporations, and policymakers about fuel-switching in the transport and power sectors. Each of the three curves within the panels of Fig. 1 represents a distinct choice and its associated emission duration: for example, whether to rent a CNG or a gasoline car for a day (Pulse TWP); whether to purchase and operate a CNG or gasoline car for a 15-yr service life (Service-Life TWP); and whether a nation should adopt a policy to convert the gasoline fleet of cars to CNG (Fleet Conversion TWP). In each of these cases, a TWP greater than 1 means that the cumulative radiative forcing from choosing natural gas today is higher than a current fuel option after t yr. Our results for pulse TWP at 20 and 100 yr are identical to fuel-cycle analyses using 20-year or 100-year GWPs for CH₄.

Fig. 1.

Technology warming potential (TWP) for three sets of natural gas fuel-switching scenarios. (A) CNG light-duty cars vs. gasoline cars; (B) CNG heavy-duty vehicles vs. diesel vehicles; and (C) combined-cycle natural gas plants vs. supercritical coal plants using low-CH₄ coal. The three curves within each frame simulate real-world choices, including a single emissions pulse (dotted lines); emissions for the full service life of a vehicle or power plant (15 and 50 years, respectively, dashed lines); and emissions from a converted fleet continuing indefinitely (solid lines). For the pulse and service life analyses, our scenarios assume that the natural gas choice reverts back to the incumbent choice before the switch took place; for the fleet conversion analysis we assume that a natural gas vehicle or power plant is replaced by an identical unit at the end of its service life.

Given EPA’s current estimates of CH₄ leakage from natural gas production and delivery infrastructure, in addition to a modest CH₄ contribution from the vehicle itself (for which few empirical data are available), CNG-fueled vehicles are not a viable mitigation strategy for climate change.^§ Converting a fleet of gasoline cars to CNG increases radiative forcing for 80 yr before any net climate benefits are achieved; the comparable cross-over point for heavy-duty diesel vehicles is nearly 300 yr.

Stated differently, converting a fleet of cars from gasoline to CNG would result in numerous decades of more rapid climate change because of greater radiative forcing in the early years after the conversion. This is eventually offset by a modest benefit. After 150 yr, a CNG fleet would have produced about 10% less cumulative radiative forcing than a gasoline fleet—a benefit equivalent to a fuel economy improvement of 3 mpg in a 30 mpg fleet. CNG vehicles fare even less favorably in comparison to heavy-duty diesel vehicles.

In contrast to the transportation cases, a fleet of new, combined-cycle natural gas power plants reduces radiative forcing on all time frames, relative to new coal plants burning low-CH₄ coal—assuming current estimates of leakage rates (Fig. 1C). The conclusions differ primarily because of coal’s higher carbon content relative to petroleum fuels; however, fuel-cycle CH₄ leakage can also affect results. (As discussed elsewhere in this paper, our analysis considered only the emissions of CH₄ and CO₂. In SI Text, we examine the effect of different CH₄ leak rates in the coal and natural gas fuel cycles for the electric power scenario.)

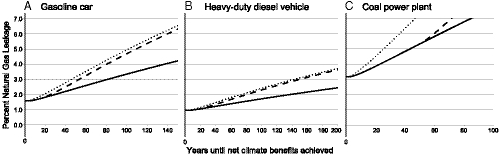
To provide guidance to industry and policymakers, we also determined the maximum well-to-wheels or well-to-burner-tip leakage rate needed to ensure net climate benefits on all time frames after fuel-switching to natural gas (see Fig. 2). For example, if the well-to-wheels leakage was reduced to an effective leak rate of 1.6% of natural gas produced (approximately 45% below our estimate of current leakage of 3.0%), CNG cars would result in climate benefits immediately and improve over time.^¶ For CNG to immediately reduce climate impacts from heavy-duty vehicles, well-to-wheels leakage must be reduced below 1%. Fig. 2C shows that new natural gas power plants produce net climate benefits relative to efficient, new coal plants using low-gassy coal on all time frames as long as leakage in the natural gas system is less than 3.2% from well through delivery at a power plant. Fig. 2 also shows, for a range of leakage rates, the number of years needed to reach the “cross-over point” when net climate benefits begin to occur after a fuel-technology choice is made.

Fig. 2.

Maximum “well-to-wheels” natural gas leak rate as a function of the number of years needed to achieve net climate benefits after choosing a CNG option in lieu of (A) gasoline cars; (B) heavy-duty diesel vehicles; and (C) coal power plants. For A and B, the maximum leakage is the sum of losses from the well through the distribution system plus losses from the CNG vehicle itself (well-to-wheels); for C, the maximum leakage is from the well through the transmission system where most power plants receive their fuel. When leak rates are less than the y-intercept, a fuel switch scenario would result in net climate benefits beginning immediately. The three curves within each frame follow the conventions outlined in Fig. 1 and represent: single emissions pulses (dotted lines); the service life of a vehicle or a power plant, 15 or 50 years, respectively (dashed lines); and a permanent fleet conversion (solid lines).

We emphasize that our calculations assume an average leakage rate for the entire U.S. natural gas supply (as well for coal mining). Much work needs to be done to determine actual emissions with certainty and to accurately characterize the site-to-site variability in emissions. However, given limited current evidence, it is likely that leakage at individual natural gas well sites is high enough, when combined with leakage from downstream operations, to make the total leakage exceed the 3.2% threshold beyond which gas becomes worse for the climate than coal for at least some period of time.^|| Our analysis of reported routine emissions for over 250 well sites with no compressor engines in Barnett Shale gas well sites in Fort Worth, Texas, in 2010 revealed a highly skewed distribution of emissions, with 10% of well sites accounting for nearly 70% of emissions (see SI Text).** Natural gas leak rates calculated based on operator-reported, daily gas production data at these well sites ranged from 0% to 5%, with six sites out of 203 showing leak rates of 2.6% or greater due to routine emissions alone.^††

Our analysis of coal-to-natural gas fuel-switching does not consider potential changes in sulfate aerosols and black carbon, short-lived climate forcers previously shown to affect the climate implications of such fuel-switching scenarios (5, 13). Recently, Wigley concluded that coal-to-gas switching on a global scale would result in increased warming on a global scale in the short term, based on examining a set of scenarios with a climate model that included both the increased warming produced by CH₄ losses from the natural gas fuel cycle and the additional cooling that occurs due to SO₂ emissions and the sulfate aerosols they form as a result of burning coal (5). The applicability of Wigley’s global conclusion to the United States or any other individual country is limited due to the reliance on global emissions scenarios. Analyses such as Wigley’s, which model the climate impacts of all climate forcing emissions, are useful to evaluate specific fuel-switching scenarios; however, their ultimate relevance to policymakers and fleet owners will be determined by the fidelity with which they reflect actual emissions from all phases of each fuel cycle at the relevant geographic scale (e.g., national, continental, or global). The SO₂ emissions that Wigley assumed are much higher than those of the current fleet of coal electrical generation plants in the United States, where SO₂ emissions declined by more than 50% between 2000 and 2010.^‡‡ Moreover, due to state and federal pollution abatement requirements, U.S. SO₂ emissions are projected to continue declining, to roughly 30% of 2000 levels by 2014 (see SI Text). This means that by 2014 the projected sulfur emissions from the U.S. coal electrical generation plant fleet, 3 TgS/GtC, will approach the emission factor that Wigley assumed the global fleet would reach in 2060 (2 TgS/GtC), when he projected the climate benefits of fuel-switching might begin, and significantly lower than Wigley’s estimated 2010 value of 12 TgS/GtC. Accounting for the lower SO₂ from U.S. coal plants in an integrated way will result in greater net climate impacts of using coal than reported by Wigley and in turn the net benefits of fuel-switching will occur much sooner than he projected.

Increasingly, this will also be the case globally. The production of sulfur aerosols as a result of coal combustion causes such negative impacts on human and ecosystem health that it is prudent to assume that policies will continue to be rapidly implemented in many, if not most, countries to reduce such emissions at a much faster pace than assumed by Wigley. Indeed, it has been reported that China has already installed SO₂ scrubbers on power plants accounting for over 70% of the nation's installed coal power capacity (14), such that SO₂ emissions from power plants in 2010 were 58% below 2004 levels (15). The SO₂ emissions factor from Chinese coal plants in 2010 has been estimated to be 204 g/GJ, comparable to the 2010 value of 229 g/GJ (4.7 TgS/GtC) for U.S. coal plants (SI Text).

Little work appears to have been done to evaluate fuel-switching in on-road transportation with methods that consider the implications of all climate forcing emissions, including sulfur aerosols and black carbon, although the effect of short-lived climate forcers on individual transport sectors has been studied (16, 17). One study reports that the influence of negative radiative forcing due to emissions from on-road transport is much lower than for the power generation sector in both the United States and globally (18). This implies that our approach, which considers CO₂ and CH₄ emissions alone, provides a reasonable first-order estimate of changes in radiative forcing from fuel-switching scenarios for the on-road transport sector.

Conclusions

The TWP Approach Proposed Here Offers Policymakers Greater Insights than Conventional GWP Analyses.

GWPs are a valuable tool to compare the radiative forcing of different gases but are not sufficient when thinking about fuel-switching scenarios. TWPs provide a transparent, policy-relevant analytical approach to examine the time-dependent climate influence of different fuel-technology choices.

Improved Science and Data Are Needed.

Despite recent changes to EPA’s methodology for estimating CH₄ leakage from natural gas systems, the actual magnitude remains uncertain and estimates could change as methods are refined. Ensuring a high degree of confidence in the climate benefits of natural gas fuel-switching pathways will require better data than are available today. EPA’s rule requiring natural gas industry disclosure of GHG emissions should begin to produce data in 2012, though it is unlikely that most uncertainties will be resolved and possible systematic biases eliminated. Specific challenges include confirming the primary sources of emissions and determining drivers of variance in leakage rates. Greater direct involvement of the scientific community could help improve estimates of CH₄ leakage and identify approaches that enable independent validation of industry-reported emissions.

Reductions in CH₄ Leakage Are Needed to Maximize the Climate Benefits of Natural Gas.

While CH₄ leakage from natural gas infrastructure and use remains uncertain, it appears that current leakage rates are higher than previously thought. Because CH₄ initially has a much higher effect on radiative forcing than CO₂, maintaining low rates of CH₄ leakage is critical to maximizing the climate benefits of natural gas fuel-technology pathways. Significant progress appears possible given the economic benefits of capturing and selling lost natural gas and the availability of proven technologies. (EPA’s Natural Gas STAR program shows many examples: www.epa.gov/gasstar/tools/recommended.html.)

Methods

Our approach of using TWPs to compare the cumulative radiative forcing of fuel-technology combinations is a straightforward extension of the calculation of GWP, which is given by Eq. 1 over a time horizon, TH, for a pulse emission of 1 kg of a generic GHG producing time-dependent radiative forcing given by RF_GHG(t):

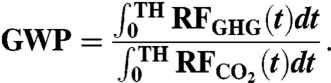[1]

SI Text shows the analytical solution of Eq. 1 (i.e., GWP as a function of time horizon). Plotting the entire curve enables one to see the GWP values for all time horizons.

Our TWP approach extends the standard GWP calculation in two ways: by combining the effects of CH₄ and CO₂ emissions from technology-fuel combinations and by considering streams of emissions in addition to single pulses. Considering streams of emissions is more reflective of real-world scenarios that involve activities that occur over multiyear time frames.

Eq. 2 is our extension of the GWP formula Eq. 1 to calculate TWPs, with the following definitions. We label as Technology-1 the alternative that combusts natural gas and has CO₂ emissions E_1,CO2 and CH₄ emissions from the production, processing, storage, delivery, and use of the fuel: E_1,CH4. If L_REF is the percent of gross natural gas produced that is currently emitted to the atmosphere over the relevant fuel cycle (e.g., electric power or transportation), then Technology-1’s CH₄ emissions at leakage rate L would be: (L/L_REF)E_1,CH4. The calculations of TWP in this paper assume that the leakage rate L is at the national average value L_REF (and thus L/L_REF = 1). The scaling factor L/L_REF is included to allow calculations about changes in the national leakage rate or about individual wells and distribution networks that deviate from the national average. The values we used for L_REF are derived in SI Text using EPA’s estimated emissions with one exception and are equal to 2.1% for a natural gas power plant and 3.0% for CNG vehicles. The exception to the last statement is that we estimated CH₄ from the operation of a CNG automobile to be 20 times that from a gasoline vehicle (11), which is approximately 20% of the well-to-pump CH₄ leakage on a kg/mmBtu basis. This assumption deserves much further scrutiny. Technology-2 combusts gasoline, diesel fuel, or coal and produces CO₂ emissions E_2,CO2 and methane emissions E_2,CH4. Estimates of the Es for each of the technologies considered are reported in Table 1 and are explained in SI Text. The TWPs at each point in time can be obtained by substituting the total radiative forcing values, TRF_CH4(t) and TRF_CO2(t) for CH₄ and CO₂, respectively, and emission factors, E_n,GHG from Table 1 into Eq. 2:

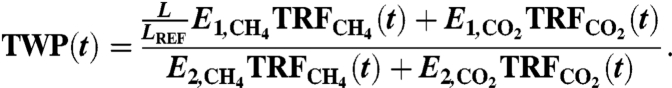[2]

Table 1.

Emission factors used for TWP calculations in this paper

	Power Plants		Vehicles
	Natural gas combined cycle* (kg/MWh)	Supercritical pulverized coal† (kg/MWh)	Light-duty CNG car (kg/mmBtuHHV)‡	Light-duty gasoline car (kg/mmBtuHHV)	Heavy-duty CNG truck (mg/ton-mile)	Heavy-duty diesel truck (mg/ton-mile)
Upstream CH4	3.1	0.65	0.51	0.1	590	100
Upstream CO2	36	7	9.4	15.9	10,000	15,000
In-Use CH4	0	0	0.11	0.0056	15	0
In-Use CO2	361	807	53.1	70.3	80,000	85,000
Fuel cycle CH4	3.1	0.65	0.62	0.11	605	100
Fuel cycle CO2	397	814	62.5	86.2	90,000	100,000

^*Heat rate = 6,798 Btu/kWh.

^†Heat rate = 8,687 Btu/kWh.

^‡1 mmBtu = 10⁶ Btu = 1.055 GJ.

The TRF values needed for Eq. 2 are derived as follows. Let f(t,t_E) be the mass of a gas left in the atmosphere at time t if 1 kg of the gas was emitted at time t_E. The cumulative radiative forcing function, CRF(t) (in units of J m^-2 kg^-1), at a later time t, due to emission of 1 kg of the gas at time t_E, is then:

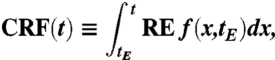[3]

where RE is the radiative efficiency of the gas. The integral in Eq. 3 sums radiative forcing for the t - t_E years from the year in which the gas was emitted, x = t_E, to year x = t. For simplicity, we adopt units which make the RE of CO₂ equal to one, and so the RE of CH₄ is expressed as a multiple of the RE of CO₂. In these units, the RE of CH₄ is determined to be 102, using the values in Table 2 taken from the IPCC (10) and following the IPCC convention that methane’s direct radiative efficiency be enhanced by 25% and 15% to account for indirect forcing due to ozone and stratospheric water, respectively.

Table 2.

Radiative efficiency (RE) values used in this paper

	Direct RE (W m-2 ppb-1)	Relative direct + indirect RE (per ppb or molar basis)	Relative direct + indirect RE (per kg basis)*
CO2	1.4 × 10-5	1	1
CH4	3.7 × 10-4	37	102

^*Obtained by multiplying the molar radiative efficiency by the ratio of molecular weights of CH₄ and CO₂.

Now suppose that instead of a single pulse, the gas is emitted continuously at a rate of 1 kg/yr from t = 0 until some maximum time t_max, as would occur, for example, if emissions were to continue over the service life of a vehicle, power plant, or fleet. For such cases we define the total radiative forcing (TRF) in year t to be:

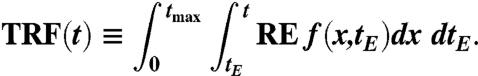[4]

In the special case of a single emission pulse, TRF(t) = CRF(t). Our use of Eq. 4 assumes a constant, unit emission rate; a more general formulation could be employed to reflect potential technology improvements over time.

For CH₄, f(t,t_E) is an exponential decay:

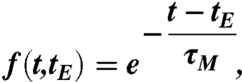[5]

where τ_M is 12 yr. For CO₂, we follow the IPCC and use the Bern carbon cycle model (10):

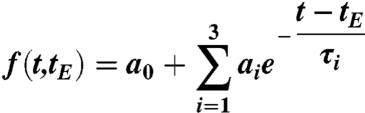[6]

where τ₁ = 172.9, τ₂ = 18.51, τ₃ = 1.186 , a₀ = 0.217, a₁ = 0.259, a₂ = 0.338, and a₃ = 0.186. Our calculations do not consider the CO₂ produced from the oxidation of CH₄, an approximation which introduces a small underestimation of the radiative forcing from a fuel cycle’s CH₄ leakage.

If calculating the TWP for a single pulse of emissions (pulse TWP), then t_E = 0; TRF_CH4(t) is given by Eq. 3 with f(t,t_E) given by Eq. 5; and TRF_CO2(t) is given by Eq. 3 with f(t,t_E) given by Eq. 6. If calculating the TWP for a permanent fuel conversion of a fleet (fleet conversion TWP) then TRF_CH4(t) is given by Eq. 4 with t_max = t and f(t,t_E) given by Eq. 5. Similarly, TRF_CO2(t) is given by Eq. 4 with t_max = t and f(t,t_E) given by Eq. 6. If calculating the TWP for emissions over the service life of a vehicle or power plant (service life TWP) and t ≤ AMAX, where AMAX is the average age at which the asset ceases to emit, then TRF_CH4(t) and TRF_CO2(t) are the same as in the fleet conversion TWP calculations. However, if t > AMAX, then TRF_CH4(t) is given by Eq. 4 with t_max = AMAX and f(t,t_E) given by Eq. 5. Similarly, TRF_CO2(t) is given by Eq. 4 with t_max = AMAX and f(t,t_E) given by Eq. 6. The solutions for all of these cases are in Table 3. We use AMAX = 15 yr for vehicles and 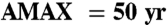for power plants.

Table 3.

Total radiative forcing (TRF) functions for CH₄ and CO₂ used in calculation of TWP in Eq. 2 for three distinct emissions profiles

Case	TRFCH4(t)	TRFCO2(t)
Pulse TWP	RE{τM(1 - e-t/τM)}
Service Life TWP for t≤ AMAX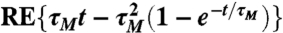
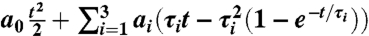Service Life TWP for t > AMAX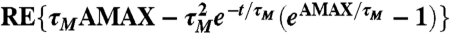
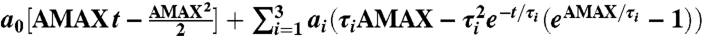Fleet Conversion TWP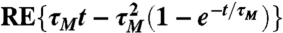

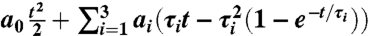RE in these formulas is the radiative efficiency of CH₄ relative to CO₂ and equals 102.

By rearranging terms in Eq. 2 when TWP = 1 to bring L to the left hand side, we obtain an equation for the relationship between the cross-over time (t^∗—the time at which the two technologies have equal cumulative radiative forcing) and the percent leakage that makes this happen (L^∗):

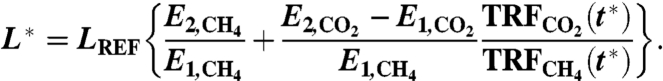[7]

Taking the limit of L^∗ as the cross-over time t^∗ goes to zero, we obtain an expression for the critical leakage rate L₀, which serves as an approximation of the leakage rate below which the natural gas-burning technology causes less radiative forcing on all time frames.

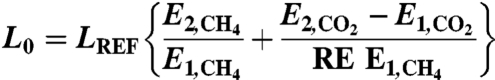[8]

where RE = 102. Eq. 8 must be viewed as an approximation because L^∗ is a nonmonotonic function of t^∗ for small values of t^∗ (see Fig. 2, which plots L^∗ as a function of cross-over time t^∗). The small decrease in L^∗ for small t^∗ is caused by the fact that 18.6% of the emitted CO₂ decays faster than CH₄ in the Bern carbon cycle model (time scales of 1.186 vs. 12 yr). The large increase in L^∗ for t_∗ > 3 years is caused by the rapid decay of CH₄ relative to the remaining 81.4% of the CO₂. The decay curves for CO₂ and CH₄ are shown in SI Text. Calculated values of L_o using Eq. 8 are within 2–3% of the absolute minima for L^∗. Calculations of TWP and L^∗ using Eq. 2 and Eq. 8 were performed with an Excel spreadsheet and are available in Dataset S1.