Adaptive behaviour, tri-trophic food-web stability and damping of chaos

Abstract

We examine the effect of adaptive foraging behaviour within a tri-trophic food web with intra-guild predation. The intra-guild prey is allowed to adjust its foraging effort so as to achieve an optimal per capita growth rate in the face of realized feeding, predation risk and foraging cost. Adaptive fitness-seeking behaviour of the intra-guild prey has a stabilizing effect on the tri-trophic food-web dynamics provided that (i) a finite optimal foraging effort exists and (ii) the trophic transfer efficiency from resource to predator via the intra-guild prey is greater than that from the resource directly. The latter condition is a general criterion for the feasibility of intra-guild predation as a trophic mode. Under these conditions, we demonstrate rigorously that adaptive behaviour will always promote stability of community dynamics in the sense that the region of parameter space in which stability is achieved is larger than for the non-adaptive counterpart of the system.

1. Introduction

The topology of ecological communities is structured through the many and varied interactions active in food webs [1]. Direct trophic interactions, mutualism, competition, commensalism and parasitism are all modes that interconnect various elements of a community and to a certain extent, govern overall ecosystem dynamics [2]. There is also a growing realization that there are subtler, indirect effects in play [3,4] where third-party actors can be subsumed to play the role of a bait or a protector. The interaction of direct and indirect effects is most evident in so-called intra-guild predation, a tri-trophic arrangement where a consumer and its predator share a common resource [5]. While ecological communities are often described by the topology of the connections among species, their dynamics are controlled by the strength of the interactions these connections represent [6]. Further, the strength of these interactions is not static. Whatever the architecture of ecological communities, the interactions that control their function are ultimately enacted at the level of individuals whose behaviour is attuned solely to their evolutionary self-interest [7–10]. In particular, for a given environmental configuration (both its biotic and abiotic components), the behaviour that an individual is conditioned to choose is that which maximizes its fitness. Thus, the dynamics of an ecosystem are governed by the interplay of its structural topology and the fitness-seeking strategies of its individual members.

Adaptive foraging behaviour, in particular, mediates effects that cascade through ecosystems. Trophic interactions are particularly susceptible to changes in search behaviour as increased search effort may well increase encounters with prey, but oftentimes also increases risk through increasing encounters with predators [11]. Furthermore, increased foraging effort also incurs an energetic cost. Within a particular ecosystem configuration, evolutionarily consistent search behaviour is thus one that reflects a trade-off between the benefits, costs and risks such behaviour elicits for an individual. Furthermore, as the prevailing ecosystem configuration changes (e.g. predator and prey abundance), behaviour adapts so as to maintain this trade-off.

Our particular concern here is how adaptive behaviour affects the dynamics of tri-trophic systems that exhibit a degree of intra-guild predation [12]. Omnivory falls into this category, where a predator feeds off both a consumer and the consumer's resource. Seen purely in the light of direct interactions, such trophic arrangements should be quite rare, as the intra-guild prey (i.e. the intermediate consumer) has to endure both direct predation and competition from the top predator [13]. This is borne out in simple dynamic descriptions that suggest that intra-guild predation systems are highly unstable [13] in their restricted admittance of stable coexistence [14,15], large-amplitude limit cycles [14], chaotic dynamics [16,17] and susceptibility to enrichment-induced extinctions [18]. And yet, tri-trophic arrangements including omnivory are ubiquitous features of nearly all ecosystems [5,19–21]. In this context, adaptive foraging behaviour appears to have an effective role in reconciling theory with the observed prevalence of intra-guild predation. For instance, the effect of adaptive behaviour has been shown to stabilize linear food chains [22] and to promote the coexistence of competing predators [23] and prey [24] and have a general stabilizing effect on food webs [25–27]. With regard to intra-guild predation, adaptive, fitness-seeking behaviour of intra-guild predator yields a relatively small effect in facilitating coexistence [28], whereas the effect of adaptive behaviour of the intra-guild prey appears to be more pronounced [29,30].

In this work, we examine the general properties and conditions under which adaptive fitness-seeking behaviour tends to stabilize tri-trophic food webs. Previous works have established that depending on system productivity, stable coexistence depends on two complementary (necessary but not sufficient) conditions: (i) the intermediate consumer is a superior competitor in exploiting the common resource and (ii) the predator gains significantly more from its consumption of the intermediate consumer than the common resource [14]. The focus is on the adaptive behaviour of the intra-guild prey, as its investment in foraging effort is subject to clear trade-offs in terms of the benefits, costs and risks such behaviour imposes on the individual. Further, while previous numerical investigations concentrated on the effect of such adaptive behaviour in promoting system stability [29,30], our aims here are to uncover the generality of these results and the conditions under which stability is promoted.

2. Results and discussion

2.1. A tri-trophic web

The conceptual arena we consider is a simple planktonic ecosystem (figure 1) where the resource is a non-motile phytoplankter (e.g. diatom) the carrying capacity of which is set by some limiting nutrient. Both the consumer (e.g. ciliate) and predator (e.g. copepod) are motile and the trophic interaction between individuals is determined by their encounter rate. We endow the intra-guild prey with adaptive behaviour in that it adjusts its foraging effort (swimming speed) so as to maximize its fitness as expressed in its net per capita growth rate. The underlying trade-off is in terms of benefit (ingestion of prey), cost (hydrodynamics of swimming) and risk (contacts with predators).

Figure 1.

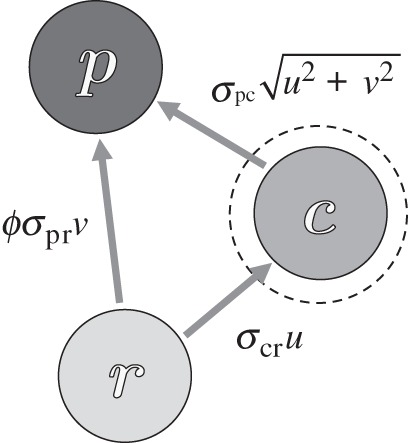
Sketch of a tri-trophic food web with intra-guild predation. The resource r is preyed upon by both an intra-guild predator p and an intra-guild consumer c. The predator p further feeds on the consumer at a rate dependent on its encounter rate and an omnivory parameter ϕ. The consumer is allowed to adapt its foraging effort u so as to optimize its instantaneous per capita growth rate with repercussion for both its encounter rate and thus its interaction strength with both its resource and predator.

For simplicity, we assume that both the consumer and the predator move ballistically in a uniformly random direction in three-dimensional space. The consumer can detect resource organisms at a distance R_cr, while the predator can detect the resource at a distance R_pr and consumer organisms at a distance R_pc. Further we assume that resource, consumer and predator are well mixed (i.e. Poisson-distributed), that contacts lead to a fixed probability of ingestion and that the handling time is much shorter than the mean search interval between encounters. Given these assumptions, the clearance rate of the consumer on resource is 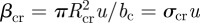while that of predators on resource is 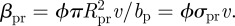Here, ϕ represents the degree of omnivory practiced by the top predator (i.e. selectivity of p on r). That is, at ϕ = 0, the top predator feeds solely on the consumer and the trophic interaction becomes a simple food chain; at ϕ = 1, the predator p ingests all resource r encountered. The clearance rate of predator on consumer is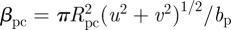 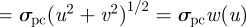. The latter assumes that swimming speeds of both the consumer and the predator follow a Rayleigh probability distribution, with u and v defined as the mean swimming speed of consumer and predator, respectively [31], and is a relatively general formulation for the encounter rate process in plankton. The factors b_c and b_p are the biomass per individual of the consumer and predator, respectively, so that the interaction cross sections (σ) have dimensions L² M⁻¹, while the clearance rates (β) have dimensions L³ T⁻¹M⁻¹.

Provided that all individuals within the resource, consumer and predator populations behave similarly, we can write the population dynamics as a coupled Lotka–Volterra system:

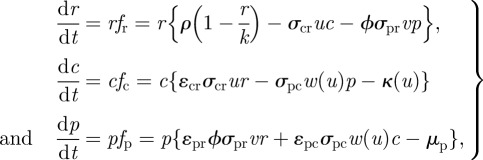2.1

where r, c and p are biomass density (dimensions ML⁻³) for resource, consumer and predator species, respectively.

Here, the carrying capacity of the resource is k, μ_p the mortality rate of the predator, κ(u) the mortality rate of the consumer in the absence of direct predation (an as yet unspecified function of swimming speed u) and ɛ_ij represents the trophic conversion efficiency of consumed biomass of species j into new biomass of species i.

The dynamics of a tri-trophic food web consistent with the system (equation (2.1)) have been previously reported [14]. This system contains six fixed points (i.e. where d(r,c,p)/dt = 0). We focus on one of them, denoted s* = (r*, c*, p*), corresponding to coexistence of all three components. Specifically, the three components can coexist provided the following three conditions hold:

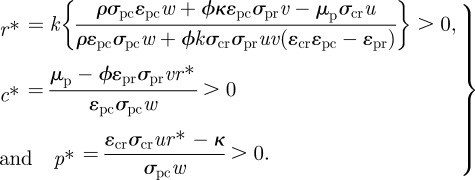2.2

Since all coefficients are positive, the latter two conditions can be rewritten as

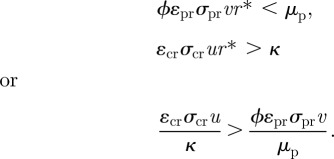This condition can be interpreted as requiring that for a given resource abundance, the consumer must be able to produce more surviving offspring over its expected lifetime (κ⁻¹) in the absence of predation than the predator can for the same resource abundance over its expected lifetime (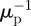). This is generally stated as the condition that in order for stable coexistence, the consumer must be able to outcompete the predator for the resource [14,32].

2.2. Adaptive behaviour

We now come to the role of adaptive behaviour. In particular, we assume that the consumer (ciliate) can adjust its swimming speed u in such a way so as to maximize its instantaneous per capita growth rate. That is, maximize the function

2.3

While the optimization of per capita growth rate is not always the best proxy for fitness [33], it has been used extensively in previous works of this kind [23,27,28], particularly since different fitness estimates such as those based on growth rate or reproductive value converge for stable populations. To investigate the behaviour in a mechanistic context, we assume that the consumer ‘mortality rate’ includes a function reflecting the cost of swimming. Specifically, we set κ(u) = μ_c + qu², where μ_c is the actual mortality rate and qu² reflects the diversion of energy (resources) to foraging which would otherwise fuel reproduction. Its u² dependence arises from the power requirements of a small organism propelling itself against hydrodynamic drag in a fluid, while the factor q involves various contributions from hydrodynamics, biochemical power efficiency and energy to assimilated biomass conversion [34].

The optimal swimming speed is thus a solution of

which gives a per capita growth rate for the consumer of

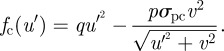The values of the optimal swimming speed and its corresponding per capita growth rate are represented in figure 2 as a function of the resource and predator densities. Optimal swimming speed (figure 2a) is an increasing function of r and a decreasing function of p. While u′ is positive for all values of r and p, the per captia growth rate is not (figure 2b), suggesting that optimality in these cases (i.e. where f_c < 0) should be interpreted as the least detrimental option.

Figure 2.

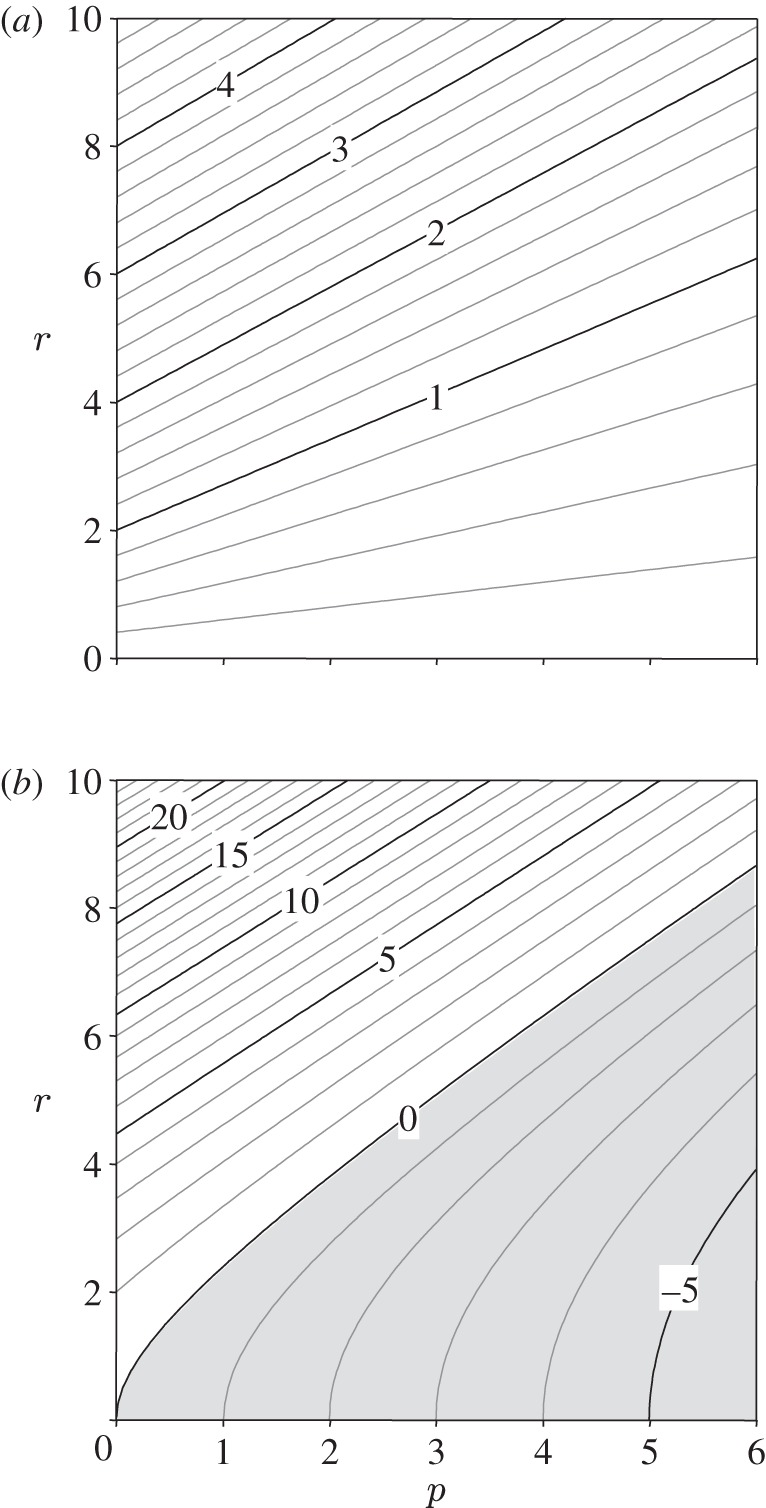
(a) Optimal swimming speed and (b) per capita growth rate of the consumer c as a function of predator p and prey resource concentration within the tri-trophic system. Parameters for this example are v = 1; ɛ_rc = 1; σ_rc = 1; σ_cp = 1; q = 1; μ_c = 0.

2.3. Stability

Our aim is to show that adaptive dynamics has a stabilizing effect on the tri-trophic omnivory system, independent of the interaction details. In order to do that, we study the growth rates in equation (2.3). The optimal swimming speed condition can be written as

2.4

Considering the optimal swimming speed as a function of the predator and resource density, u = u(r,p) and evaluating its derivatives, one can show that assuming

2.5

the optimal swimming speed is an increasing function of resource and a decreasing function of predator as we should expect. The above relations constitute our first set of assumptions. In general equation (2.5) implies that fitness is a dome-shaped function of foraging effort and that a finite optimal value exists. Specifically, both the cost (proportional to κ) and the risk (proportional to w) have a greater than linear dependence on foraging effort (proportional to u).

We proceed by studying the properties of the Jacobian matrix 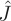of the non-adaptive system and of its adaptive counterpart, 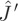(details in appendix A). From the signs of the factors involved, one can immediately show that 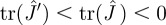, where the Jacobian is evaluated at the fixed point (equation (2.2)). This result already suggests that the stability of the fixed point is enhanced by the effect of adaptation.

A more rigorous proof of stability comes from the analysis of the eigenvalues of the Jacobian matrix, i.e. the roots of the characteristic polynomial

2.6

where (α, β and γ) are coefficients whose detailed expressions are given in appendix A. The fixed point s* is stable when all roots of the characteristic polynomial have negative real parts. As a consequence of the Routh–Hurwitz stability criterion [35], this condition is met when α > 0, β > 0 and αβ > γ > 0. By examining the expression of the coefficients, it can be readily deduced that α and β are always positive. The positivity of γ is ensured when the following condition holds:

2.7

The above equation is our second assumption and has been recognized as a general feature promoting the existence of intra-guild predation as a feasible trophic mode [14,36,37]. Notice how this assumption can be linked to the concept of ‘trophic upgrading’ [38], namely the feasibility of omnivory as a trophic mode is assured provided that the net trophic efficiency to the omnivore via intra-guild predation exceeds that of feeding on the resource directly. Trophic upgrading is relatively common in planktonic food webs [39–41] and arises through two distinct processes, either biochemically where protist consumers synthesize essential fatty acids to be passed to higher trophic levels or through repackaging biomass into larger, more easily captured and assimilated food parcels.

Turning to the final criterion αβ > γ; if it holds, then stability is ensured. This condition does not hold in general and its breakdown is the route to several kinds of unstable behaviours, including oscillations, period doubling and chaos [14–16,42]. While its validity cannot be demonstrated in general, it is possible to show that the presence of adaptation always enhances the stability of the system. Defining α′, β′ and γ′ as the coefficients of the adaptive system and α, β and γ those of their non-adaptive counterpart, one can show that the inequality is more likely to be satisfied for the adaptive system, i.e. one always has α′β′ − γ′ > αβ − γ. Further, the other inequalities are also strengthened by the presence of adaptation, i.e. α′ > α > 0, β′ > β > 0 and γ′ > γ > 0.

If a fixed point with non-negative values for the three species exists, then condition (2.7) is sufficient for its stability [14]. When this assumption is violated, the system can show alternative states of simple food chains (r−p or r−c only), but also stable coexistence [43]. In this case, when the adaptive counterpart is considered, the stability of the system is not constrained anymore since the positiveness of β′ and α′β′ − γ′ is not guaranteed but will depend on the specific parameters used in the model (see appendix A).

2.4. Examples

The stabilizing effect of adaptive behaviour is illustrated in figure 3 for parameter values consistent with one of the classic cases presented by Holt & Polis [14] in their seminal work. Staring with the non-adaptive system, we use condition (2.2) to determine for what region the fixed point s* exists. Next, where s* exists, its linear stability is determined from the eigenvalues of the Jacobian (equation (A 1)). For the adaptive system, we follow the same procedure except that it is embedded in a loop, where an optimal u is successively calculated for which new existence and linear stability conditions on s′* are evaluated. This iterative procedure is terminated when the relative difference in successive evaluations of u is less than 0.01 per cent.

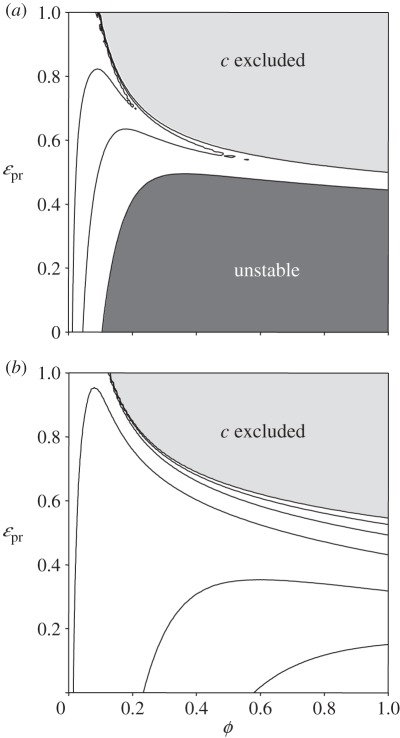
Figure 3.

The coexistence of r–c–p (s*) for system (2.1) as a function of the degree of omnivory ϕ, and ɛ_pr, the trophic conversion efficiency of resource to p. For the fixed case (a) the parameters are: ρ = 1; k = 10; u = 1; v = 1; μ_p = 0.5; ɛ_rc = 1; ɛ_cp = 1; σ_rp = 1; σ_rc = 1; σ_cp = 0.7071; q = 0.05; μ_c = 0.05. The choice of these parameters is consistent with the example given in Holt & Polis [14]. For the adaptive case (b), the consumer swimming speed u is allowed to vary so as to maximize fitness. The light grey shaded area indicates where consumer becomes extinct. Coexistence is possible everywhere else. The dark grey shaded area in (a), i.e. the fixed system, indicates where the fixed point is unstable—describing either limit cycle or chaotic dynamics. For the adaptive case, there is no unstable region and all coexistence points are stable.

For the non-adaptive system (figure 3a), the unstable region of parameter space is characterized by large-amplitude oscillations. When fitness-seeking adaptive behaviour is introduced (figure 3b), this unstable region disappears as the fixed point s′* becomes an attractor for all values of the parameter space. Although not as dramatic, there is also a small decease in the region where the intra-guild prey is excluded. Thus, the general result of the above analysis is borne out in this simple numerical model. A similar stabilizing effect can be shown for other well-documented systems whether cast in terms of the effects of omnivory [14] or enrichment [18].

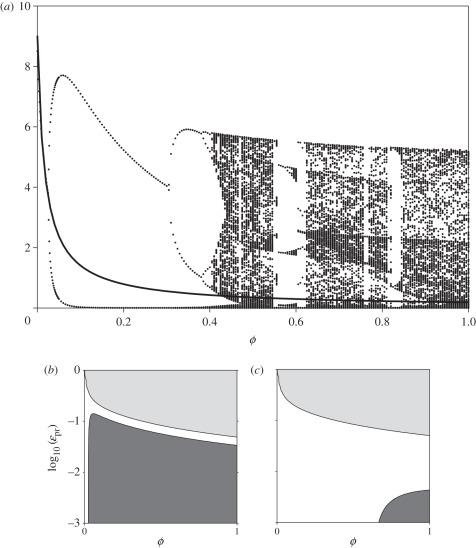
As a final example, we turn to a parameter space which displays all the hallmarks of chaotic instabilities [16,17]. For fixed parameters, system (2.1) exhibits large-amplitude oscillations, period doubling and chaos over a large region of parameter space as can be seen in the bifurcation diagram (figure 4a) over the range of omnivory strength ϕ and for a fixed value of the trophic efficiency ɛ_pr = 10⁻². This is supplemented by the analysis of the corresponding Jacobian matrix (figure 4b) which shows instability over a large region of (ϕ, ɛ_pr) space. When the intra-guild prey is allowed to adapt its foraging effort in order to maximize its per capita growth rate, the region of instability and chaos shrinks dramatically (figure 4c), and evidence of large-amplitude oscillations, period doubling and chaos disappears (figure 4a) as the system relaxes to a stable equilibrium.

Figure 4.

Bifurcation diagram (a) for system (2.1) plotting the value of p when c = c* as a function of the degree of omnivory ϕ. Other parameters are: ρ = 5; k = 12.5; u = 2; v = 2; μ_p = 1.2; ɛ_rc = 1; ɛ_cp = 1; ɛ_pr = 0.01; σ_rp = 10; σ_rc = 0.5; σ_cp = 0.3536; q = 0.175; μ_c = 0.175, and are consistent with the parameter space explored by Tanabe & Namba [16]. The corresponding dynamics of the adaptive system is stable, and its fixed point p* is plotted as a function of ϕ (solid line). The corresponding Jacobian analyses for the system with ɛ_pr = [10⁻³ to 1] (note logarithmic scale) are presented for (b) non-adaptive and (c) adaptive cases. Light grey indicates regions where the inter-guild prey is excluded, and the dark grey regions where the system is linearly unstable at its fixed point s*.

3. Conclusions

The above examples thus serve to illustrate the general stabilizing effects of adaptive fitness-seeking behaviour on tri-trophic food webs. This is not to say that adaptive behaviour always implies stability, rather that for any given parameter space, adaptive behaviour will always ensure that the system is stable over a larger region of parameter space than its non-adaptive counterpart. Further, the stable region of the non-adaptive system is always contained within the stable region of the adaptive system. In other words, the adaptive behaviour of an intra-guild prey will never push a stable system towards instability.

The stability of food webs has been an engaging topic in ecology over the last four decades. Its fascination stems largely from the seeming mismatch between real-world observations and the theory of how we understand food webs to work: the so-called paradox of omnivory [5] and the paradox of enrichment [44] to name but a few of these disparities. Attempts at reconciliation have illuminated a host of mechanisms that govern the behaviour of organisms and the dynamics of the populations and communities within which they live [25,45]. Adaptive fitness-seeking behaviour appears to have enduring repercussions for the stability (in its varied forms of estimation) of communities from simple food chains and webs to complex ecological networks [25,27]. Our contribution here is to highlight the generality of this stabilizing effect in simple tri-tophic food webs. Namely that provided intra-guild predation is a feasible trophic mode and there exists an optimal foraging effort for the intra-guild prey, adaptive behaviour will always drive the system towards stability (although whether stability is actually reached is not assured). It is noteworthy that while the adaptive behaviour of the omnivore exerts some control on the stability of tri-trophic systems [28], our results and others [29,30] suggest that the adaptive behaviour of the seemingly most vulnerable member of the tri-trophic food web exerts a strong control on the dynamics of such systems. The underlying mechanism appears to be the ability of adaptive behaviour to buffer the system from rapid fluctuations, damping out large-amplitude oscillations and bringing a semblance of stability to the system.

Appendix A. stability analysis

Here we provide details of the stability analysis sketched in §2. We start from a more generalized form of equation (2.3):

where s(u) represents the dependence of ingestion rate on foraging effort, a function assumed to be linear in the proceeding development but generalized here with the very reasonable condition that it is an increasing function of effort. The optimal foraging effort u(r, p) is a solution of ∂f_c/∂u = 0. The dependence of u on resource and predator thus follows from

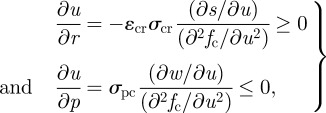A 1

where the sign dependence follows from the condition that f_c(u) is a local maximum, and an optimal foraging effort exists. In particular, plugging assumptions from equation (2.5) into the above expressions, it can be seen that they ensure the proper monotonic behaviour of u. We now study the property of the Jacobian matrix:

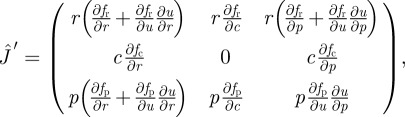A 2

where 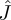can be seen as a special case of 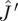by setting ∂u/∂r = ∂u/∂p = 0. Note that we have used the optimality condition (equation (2.4)) to simplify this expression. Linear stability is equivalent to assuming that the characteristic polynomial (equation (2.6)) should be stable, i.e. all its roots must have negative real parts. We write explicitly the coefficients for the non-adaptive system as

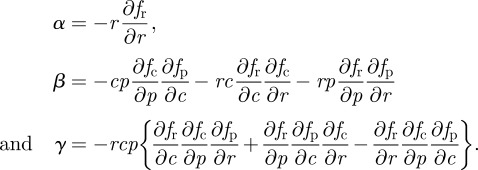The conditions α > 0 and β > 0 follow from the sign of the derivatives of the growth rates with respect to the different arguments. Specifically,

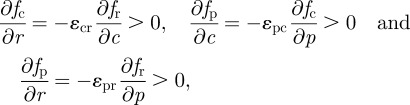which further highlights the symmetries inherent in the trophic interactions. To evaluate γ, we can make use of these symmetries to obtain

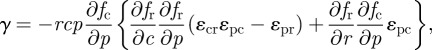A 3

where the condition that γ > 0 follows from our second assumption (equation (2.7)). At this point, stability depends on the condition that αβ − γ> 0, a condition that does not generally hold. To show the stabilizing effect of adaptation, we can examine the difference between the adaptive and non-adaptive systems. That is

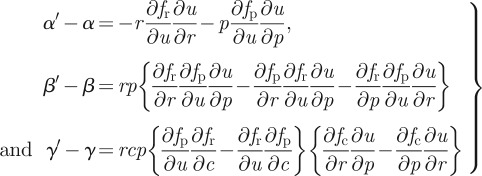A 4

together with

The conditions α′ > α > 0 and γ′ > γ > 0 readily follow from our first set of assumptions (equation (2.5)) via their impact on the foraging effort relationship (equation (A 1)). The condition β′ > β > 0 is further reliant on our second assumption (equation (2.7)) regarding trophic efficiency.

The final condition concerning the sign of α′β′ − γ′ can be examined in relationship to the same function for the non-adaptive system. Namely,

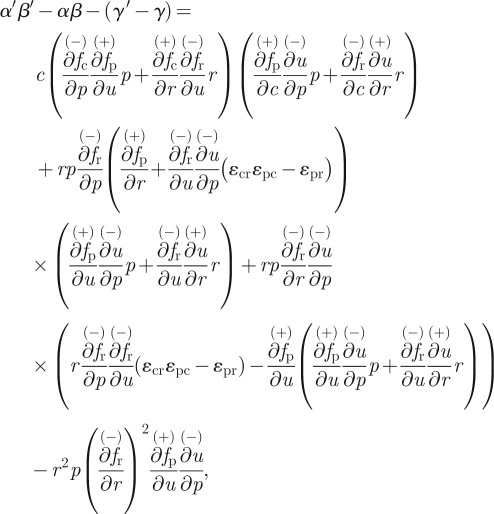where we have explicitly written the sign of each of the components. An examination of the above shows that under both previously encountered assumptions in equations (2.5) and (2.7), we have

That is, while we cannot state with certainty that the adaptive system is universally stable, we can assert that it is stable over a greater range of parameter space than the non-adaptive system.

One additional observation we can make is for the case when assumption (2.7) does not hold and when alternative states may exist [43]. A governing parameter [14] for both the existence and stability of coexistence solutions in the tri-trophic system is

D > 0 is a sufficient condition for coexistence, but more importantly, it is a necessary condition for stability. This can be readily shown from equation (A 3), in that γ > 0 (a necessary condition for stability) when D > 0. Defining D′ as the corresponding parameter for the adaptive system, it can be shown from equation (A 4) that γ′ − γ> 0 when

where w(u) represents the relative speed of the predator and the intermediate consumer. Therefore, the effect on stability of the adaptive behaviour will depend on the actual values of the parameters used in the model. All things being equal, it is more likely for D′ > D when w(u) > w(u′). That is, when going from the non-adaptive system to its adaptive counterpart, the predator biomass at coexistence (p*) decreases or the common resource biomass (r*) increases (figure 2a).