Stripe phases in high-temperature superconductors

Abstract

Stripe phases are predicted and observed to occur in a class of strongly correlated materials describable as doped antiferromagnets, of which the copper-oxide superconductors are the most prominent representatives. The existence of stripe correlations necessitates the development of new principles for describing charge transport and especially superconductivity in these materials.

Thirteen years ago, the discovery (1) of superconductivity in layered copper–oxide compounds came as a great surprise, not only because of the record-high transition temperatures, but also because these materials are relatively poor conductors in the “normal” (that is, nonsuperconducting) state. Indeed, these superconductors are obtained by electronically doping “parent” compounds that are antiferromagnetic Mott insulators—materials in which both the antiferromagnetism and the insulating behavior are the result of strong electron–electron interactions. Because local magnetic correlations survive in the metallic compounds, it is necessary to view these materials as doped antiferromagnets. A number of other related materials, such as the layered nickelates (which remain insulating when doped) and manganites (the “colossal” magnetoresistance materials), are also doped antiferromagnets in this sense.

The conventional quantum theory of the electronic structure of solids (2), which has been outstandingly successful at describing the properties of good electrical conductors (metals such as Cu and Al) and semiconductors (such as Si and Ge), treats the electronic excitations as a weakly interacting gas. This approach, known as the “Fermi liquid theory,” breaks down when applied to doped antiferromagnets. New principles must be developed to deal with these problems, which are at the core of the study of “strongly correlated electronic systems,” one of the central and most intellectually rich branches of contemporary physics. One idea that has evolved over the last decade, and which offers a framework for interpreting a broad range of experimental results on copper-oxide superconductors and related systems, is the concept of a stripe phase. A stripe phase is one in which the doped charges are concentrated along spontaneously generated domain walls between antiferromagnetic insulating regions.

Stripe phases occur as a compromise between the antiferromagnetic interactions among magnetic ions and the Coulomb interactions between charges (both of which favor localized electrons) and the zero-point kinetic energy of the doped holes (which tends to delocalize charge). Experimentally, stripe phases are most clearly detected in insulating materials (where the stripe order is relatively static), but there is increasingly strong evidence of fluctuating stripe correlations in metallic and superconducting compounds. The existence of dynamic stripes, in turn, forces one to consider new mechanisms for charge transport and for superconductivity. More generally, we will show that the concept of electronic stripe phases developed for transition-metal oxides is applicable to a broad range of materials.

Theoretical Background.

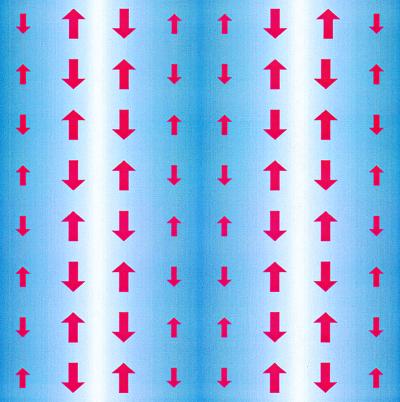
Doped antiferromagnets are a particularly important and well studied class of strongly correlated electronic materials. Here, the parent compound is insulating, even at elevated temperatures, because of the strong short-range repulsion between electrons. At sufficiently low temperatures, antiferromagnetic order develops in which there is a nonzero average magnetic moment on each site pointing in a direction that alternates from site to site (see Fig. 1). Frequently the doping process, “hole doping,” involves chemically modifying the material so that a small fraction of electrons is removed from the insulating antiferromagnet. Whereas the charge distribution in a doped semiconductor is homogeneous, in a doped antiferromagnet the added charge forms clumps—solitons in one dimension, linear “rivers of charge” in two dimensions, and planes of charge in three dimensions, as exemplified by organic conductors, cuprates or nickelates, and manganites, respectively. Typically, these clumps form what are known as “topological defects,” across which there is a change in the phase of the background spins or orbital degrees of freedom. In d dimensions, the defects are (d-1)—dimensional extended objects (3). Stripes in a two-dimensional system are illustrated schematically in Fig. 1.

Figure 1.

Schematic picture of a stripe-ordered phase. The arrows represent the magnetic or spin order, and the blue scale represents the local charge density. Regions of high charge density (stripes) lie between largely undoped regions, where the spin order is much the same as in the undoped antiferromagnet. In the figure, the stripes lie along the direction of the nearest-neighbor bonds, which we refer to as “vertical” stripes; when the stripes lie at 45° to this axis, they are said to be “diagonal.”

Self-organized local inhomogeneities were predicted theoretically (4–7). These inhomogeneities arise because the electrons tend to cluster in regions of suppressed antiferromagnetism (8), which produces a strong short-range tendency to phase separation (9–11) that is frustrated by the long-range Coulomb interaction. The best compromise (7, 12) between these competing imperatives is achieved by allowing the doped holes to be delocalized along linear stripes, while the intervening regions remain more or less in the undoped correlated insulating state.

Experimental Evidence for Stripes.

The most direct evidence for stripe phases in doped antiferromagnets has come from neutron scattering studies. Diffraction of a neutron beam by long-period spin and charge density modulations, extending over a few unit cells as indicated in Fig. 1, yields extra Bragg peaks. The position of such a superstructure peak measures the spatial period and orientation of the corresponding density modulation, whereas the intensity provides a measure of the modulation amplitude. Because neutrons have no charge, they do not scatter directly from the modulated electron density, but instead are scattered by the ionic displacements induced by the charge modulation. The lattice modulation is also measurable with electron and x-ray diffraction.

The antiferromagnetic order found in the parent compounds of the cuprate superconductors is destroyed rapidly as holes are introduced by doping. The first indications of long-period (“incommensurate”) spin-density modulations were provided by inelastic neutron scattering (13) of superconducting La_2−xSr_xCuO₄ and by related measurements on the insulating nickelate analog (14). After the discovery of “incommensurate” charge ordering in the latter system by electron diffraction (15), the proper connection between the magnetic and charge-order peaks was determined in a neutron diffraction study (16) of La₂NiO_4.125. The positions of the observed peaks indicate that the charge stripes run diagonally through the NiO₂ layers (as opposed to the vertical stripes shown in Fig. 1). More recent experiments (17, 18) on La_2−xSr_xNiO₄ have shown that the diagonal stripe ordering occurs for doping levels up to x ≈ 1/2 (corresponding to a hole density of 1 for every two Ni sites), with the maximum ordering temperatures occurring at x = 1/3.

It is significant that the charge ordering is always observed at a higher temperature than the magnetic ordering, which is characteristic (19) of a transition that is driven by the charge. It is also important to note that the period of the charge order is generally temperature dependent, which means that the hole concentration along each stripe also varies with temperature; this is characteristic (20) of structures that arise from competing interactions. These observations are consistent with the idea that the stripes are generated by the competition between the clustering tendency of the holes and the long-range Coulomb interactions. [Weak density–wave order can occur in conventional solids under special conditions (“nested Fermi surfaces”), but the transitions tend to be “spin driven” and occur at a fixed “nesting” wave vector (5)].

Charge order is most easily detected when stripes are static, but perfect static charge order can be shown (21) to be incompatible with the metallic behavior of the cuprates. Nevertheless, to get a better experimental handle on the charge order, one might hope to pin down fluctuating stripes with a suitably anisotropic distortion of the crystal structure. Just such a distortion of the La_2−xSr_xCuO₄ structure is obtained by partial substitution of Nd for La. Neutron diffraction measurements (22) x ≈1/8 a Nd-doped crystal with the special Sr concentration of x ≈1/8 revealed charge and spin order consistent with the vertical stripes of Fig. 1. (An anomalous suppression of superconductivity, associated with the lattice distortion, is maximum for x ≈1/8.) The charge order has since been confirmed by high-energy x-ray diffraction (23). As in the nickelates, the spin ordering occurs at lower temperatures than the charge order, and the hole concentration on a stripe varies as a function of the Sr concentration, x.

Although it has been difficult to observe a direct signature of charge stripes in other cuprate families, the existing neutron scattering studies of magnetic correlations are certainly most easily understood in terms of the stripe-phase concept. The doping dependence of dynamic magnetic correlations (24) in Nd-free La_2-xSr_xCuO₄ is found to be essentially the same as the static correlations in Nd-doped samples (22), and a comprehensive study (25) of a Nd-free sample near “optimum” doping (that is, maximum superconducting transition temperature) indicates that ordering may be prevented by quantum fluctuations. To keep things interesting, static magnetic order has been observed (26) to set in near the superconducting transition temperature in La₂CuO_4+δ. Finally, a beautiful experiment (27) on superconducting YBa₂Cu₃O_6+x has shown that the low-energy magnetic correlations in that system have strong similarities to those in La_2−xSr_xCuO₄.

An example of planar domain walls in a three-dimensional system occurs in nearly cubic La_1−xCa_xMnO₃ with x = 0.5. Charge order has been imaged by transmission electron microscopy (28). The ordering phenomena are somewhat more complex in this case because the occupied Mn 3d orbitals are degenerate. As a consequence, charge, spin, and orbital ordering are all involved, although, again, charge order sets in at a higher temperature than magnetic order.

Electronic Liquid Crystals.

Once the idea of stripe phases of a two-dimensional doped insulator has been established, a major question arises: How can a stripe phase become a high-temperature superconductor, as in the cuprates, rather than an insulator, as in the nickelates? Typically, interactions drive quasi one-dimensional metals to an insulating ordered charge density wave (CDW) state at low temperatures (29) (and quenched disorder only enhances the insulating tendency). However, we have shown (21) that the CDW instability is eliminated and superconductivity is enhanced if the transverse stripe fluctuations have a large enough amplitude. To satisfy this condition, the stripes could oscillate in time or be static and meandering. They are then electronic (and quantum-mechanical) analogues of classical liquid crystals and, as such, they constitute new states of matter, which can be either high-temperature superconductors or two-dimensional anisotropic unconventional metals.

Classical liquid crystals are phases that are intermediate between a liquid and a solid and spontaneously break the symmetries of free space. Electronic liquid crystals are quantum analogues of these phases in which the ground state is intermediate between a liquid, where quantum fluctuations are large, and a crystal, where they are small. Because the electrons exist in a solid, it is the symmetry of the host crystal that is spontaneously broken, rather than the symmetry of free space. An electronic liquid crystal has the following phases: (i) a liquid, which breaks no spatial symmetries and, in the absence of disorder, is a conductor or a superconductor; (ii) a nematic, or anisotropic liquid, which breaks the rotation symmetry of the lattice and has an axis of orientation; (iii) a smectic, which breaks translational symmetry in one direction and otherwise is an electron liquid; (iv) an insulator with the character of an electronic solid or glass. These classifications applied to stripe phases make the stripe notion, which is based on local electronic correlations, macroscopically precise. Neutron and x-ray scattering experiments give direct evidence of electronic liquid crystal phases (conducting stripe ordered phases) in the cuprate superconductors.

Charge Transport.

In the standard theory of solids, the electron’s kinetic energy is treated as the largest energy in the problem, and the effects of electron–electron interactions are introduced as an afterthought. As a consequence, the electronic states in normal solids are highly structured in momentum space (k-space), and therefore, according to the uncertainty principle, they are highly homogeneous in real space. Moreover, as the “normal” (metallic) state is continuously connected to the ground state of the kinetic energy, any phase transition to a low-temperature ordered phase is necessarily (30) driven by the potential energy, inasmuch as it involves a gain in the interaction energy between electrons at a smaller cost of kinetic energy. For transport properties, the central concept of a mean free path l, that is, the distance an electron travels between collisions, is well defined so long as l is much larger than the electron’s de Broglie wavelength, λ_F, at the Fermi energy.

A number of interesting synthetic metals, discovered in the past few decades, seem to violate the conventional theory. They are “bad metals” (31, 32), in the sense that their resistivities, ρ(T), have a metallic temperature dependence [ρ(T) increases with the temperature T] but the mean free path, inferred from the data by a conventional analysis, is shorter than λ_F, so the concept of a state in momentum space would be ill defined. Among the materials in question are the cuprate high-temperature superconductors; other oxides, including the ruthenates, the nickelates, and the “colossal magnetoresistance materials” (manganites), organic conductors, and alkali-doped C₆₀. Most of these materials are doped correlated insulators, in which the short-range repulsive interaction between electrons is the largest energy in the system. However, the ground state of this part of the Hamiltonian is not unique, so the kinetic energy cannot simply be treated as a perturbation; such materials display substantial structure in both real space and momentum space. As a consequence, the conventional theory (2) must be abandoned. Neither the kinetic energy nor the potential energy is totally dominant, and they must be treated on an equal footing.

Superconductivity.

The highly successful theory of superconductivity (33) developed by Bardeen, Cooper, and Schrieffer in the 1950s was designed for good metals, not for doped insulators. A key issue, therefore, is the relation of stripes to the mechanism of high-temperature superconductivity. In fact, there is a strong empirical case for an intimate relation between these phenomena: (i) strongly condensed stripe order can suppress superconductivity (as it does in La_1.6−yNd_ySr_xCuO₄); (ii) weak stripe ordering can, at times, appear at the superconducting transition temperature T_c (as it does in La₂CuO_4+δ; (iii) there is a simple linear relation between the inverse stripe spacing and the superconducting T_c observed in several materials (24, 34) (including La_2−xSr_xCuO₄ and YBa₂Cu₃O_6+x); and (iv) stripe structure and other features of the doped insulator, together with high-temperature superconductivity, disappear as the materials emerge from the doped-insulator regime (“overdoping”). Moreover, there is a clear indication that the optimal situation for high-temperature superconductivity is stripe correlations that are not too static or strongly condensed, but also are not too ethereal or wildly fluctuating. We have argued (21, 35, 36) that the driving force for the physics of the doped insulator is the reduction of the zero-point kinetic energy. This proceeds in three steps: (i) the development of an array of metallic stripes lowers the kinetic energy along a stripe; (ii) hopping of pairs of electrons perpendicular to a stripe in the CuO₂ planes creates spin pairs on and in the immediate neighborhood of a stripe; and (iii) at a lower temperature, pair hopping between stripes creates the phase coherence that is essential for superconductivity. Steps ii and iii lower the kinetic energy of motion perpendicular to a stripe.

Generality of the Stripe Concept.

The physics of charge clustering in doped correlated insulators is general and robust, so one might expect that local stripe structures would appear in other related systems. Indeed, topological doping has long been documented in the case of quasi one-dimensional charge-density-wave systems, such as polyacetylene (29, 37); it is an interesting open question whether it occurs in other higher dimensional systems. One recent fascinating discovery is the observation (38, 39) that, under appropriate circumstances, quantum Hall systems (that is, an ultra-clean two-dimensional electron gas in a high magnetic field) spontaneously develop a large transport anisotropy on cooling below 150 mK. It is likely that this anisotropy is related to stripe formation on short-length scales (40, 41), and it apparently reflects the existence of an electronic nematic phase in this system (42).

Stripe-like structures have also been observed (ref. 43 and references therein) in many other systems with competing interactions, on widely differing length scales. Beyond this generality, the existence of spontaneously generated local structures is clearly important for understanding all of the electronic properties of synthetic metals, including the anomalous charge transport and the mechanism of high-temperature superconductivity. Many of these implications have already been explored in considerable detail, but many remain to be discovered. Here we content ourselves with a few general observations.

The phenomena described above represent a form of “dynamical dimension reduction” whereby, over a substantial range of temperatures and energies, a synthetic metal will behave, electronically, as if it were of lower dimensionality. This observation has profound implications because conventional charge transport occurs in a high-dimensional state, and fluctuation effects are systematically more important in lower dimensions. In particular, in the quasi two-dimensional high-temperature superconductors, stripes provide a mechanism for the appearance of quasi one-dimensional electronic physics, where conventional transport theory fails, and is replaced by such key notions as separation of charge and spin and solitonic quasi-particles (29). At the highest temperatures (up to 1,000 K), in what is often called the “normal state” of the high-temperature superconductors, where coherent stripe-like structures are unlikely to occur, it is still probable that local charge inhomogeneities occur because of the strong tendency of holes in an antiferromagnet to phase separate (44). This behavior can lead to quasi zero-dimensional physics (quantum impurity model physics), which also produces a host of interesting and well documented quantum critical phenomena and may be at the heart of much of the anomalous normal-state behavior of these systems.