Quantum-chemical investigation of the structure and the antioxidant properties of α-lipoic acid and its metabolites

Abstract

Quantum-chemical computations were used to investigate the structure–antioxidant parameter relationships of α-lipoic acid and its natural metabolites bisnorlipoic acid and tetranorlipoic acid in their oxidized and reduced forms. The enantiomers of lipoic and dihydrolipoic acid were optimized using the B3LYP/6-311+G(3df,2p), B3LYP/aug-cc-pVDZ and MP2(full)/6-31+G(d,p) levels of theory as isolated molecules and in the presence of water. The geometries of the metabolites and the values of their antioxidant parameters (proton affinity, bond dissociation enthalpy, adiabatic ionization potential, spin density, and the highest occupied molecular orbital energy) were calculated at the B3LYP/6-311+G(3df,2p) level of theory. The results obtained reveal similarities between these structures: a pentatomic, nonaromatic ring is present in the oxidized forms, while an unbranched aliphatic chain (as found in saturated fatty acids) is present in both the oxidized and the reduced forms. Analysis of the spin density and the highest occupied molecular orbital energy revealed that the SH groups exhibited the greatest electron-donating activities. The values obtained for the proton affinity, bond dissociation enthalpy and adiabatic ionization potential indicate that the preferred antioxidant mechanisms for α-lipoic acid and its metabolites are sequential proton loss electron transfer in polar media and hydrogen atom transfer in vacuum.

Electronic supplementary material

The online version of this article (doi:10.1007/s00894-011-1306-y) contains supplementary material, which is available to authorized users.

Introduction

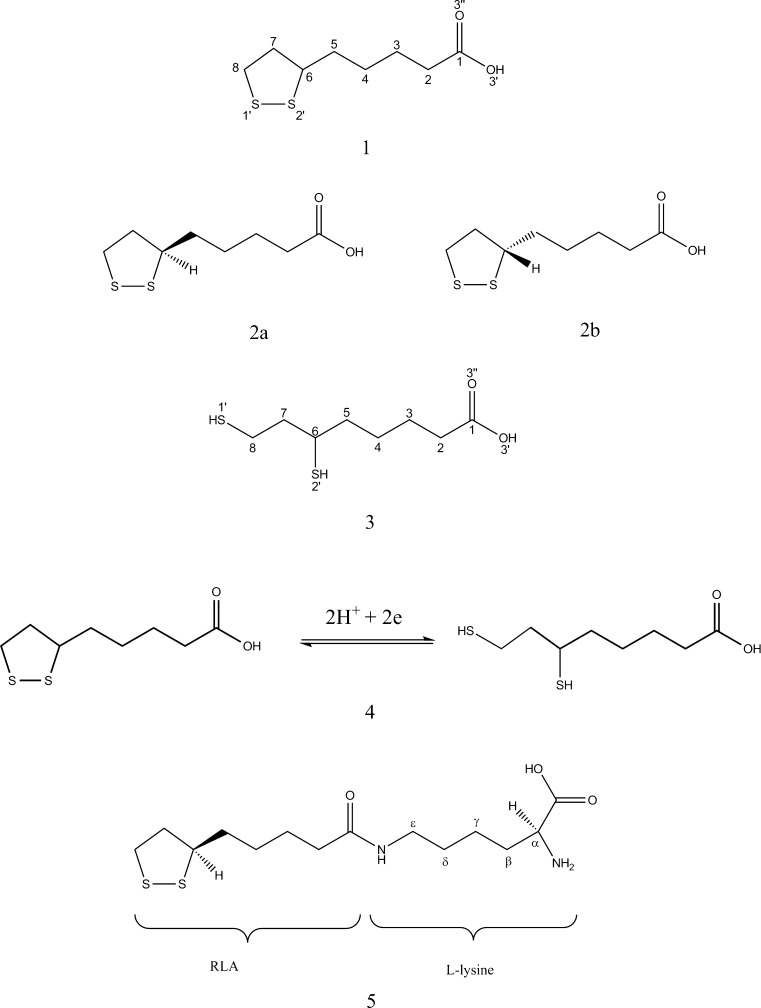
α-Lipoic acid [LA, IUPAC: 5-(1,2-dithiolan-3-yl)-pentanoic acid; 1 in Fig. 1] is an organosulfur compound that is synthesized in small amounts by microorganisms, plants, animals, and humans [1, 2]. It is one of the most important cofactors of the multi-enzyme complexes in mitochondria. In the peptide chain, LA is covalently bound to the nitrogen atom of the lysine, forming lipoyllysine (LA-Lys; 5 in Fig. 1). Physiological demand for LA is met by its synthesis in every organism. While there is currently no record of a disease caused by LA deficiency [3], the usage of LA as a dietary supplement has recently become one of the most popular topics in the scientific and medical worlds. The largest amounts of LA-Lys can be found in the kidneys, liver, heart, and in spinach and broccoli (∼1–3 μg/g) [4].

Fig. 1.

Molecular structures of lipoic acid (1), the enantiomers of lipoic acid: R-(+)-LA (2a) and S-(−)-LA (2b), dihydrolipoic acid (3), the LA/DHLA redox pair (4), and lipoyllysine (5)

α-Lipoic acid was discovered by L.J. Reed during studies aimed at isolating growth-stimulating factor for Lactobacillus casei (1949–1951; note that the prefix α has no chemical meaning, but it is helpful in distinguishing the isolated compound from structurally related forms in biological extracts [5]). The crystallographic structure of LA was determined by Stroud and Carlisle 20 years later [6]. LA is a derivative of octanoic acid with two sulfur atoms attached to carbon atoms C6 and C8. The C6 atom is the chiral center of the molecule, so LA has two optical isomers—the enantiomers R-(+)- and S-(−)- (2a and 2b in Fig. 1, respectively)—and it also exists as a racemic mixture (R/S-LA). However, only the R-(+)-enantiomer is synthesized by organisms and biologically active. In its oxidized form, LA has a characteristic pentatomic ring, as the two sulfur atoms are connected via a disulfide bond (S–S). The oxidized form can be reduced to an open-chain structure with two sulfhydryl groups: dihydrolipoic acid (DHLA; see 3 in Fig. 1). The redox pair LA/DHLA (4 in Fig. 1) interacts with different ROSs (reactive oxygen species), RNSs (reactive nitrogen species), as well as other oxidants and compounds with thiol groups, such as glutathione, cysteine, and N-acetylcysteine [7–9].

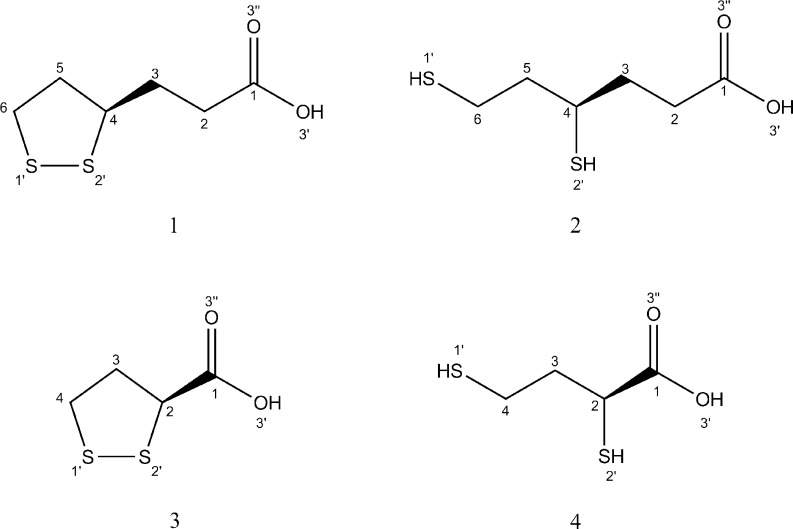
RLA is a multifunctional therapeutic compound which has shown activity in many experimental in vitro and in vivo studies. RLA/RDHLA is one of the strongest natural antioxidants, and is capable of deactivating various free radicals such as the superoxide anion (O₂ ^{• –}), the hydroxyl radical (^•OH), singlet oxygen (¹O₂), peroxynitrite (ONOO^–), and hypochlorous acid (HClO) [10–12]. It is also a very important regenerating factor for other antioxidants: thioredoxin, vitamin E, vitamin C, and glutathione (GSH) [13–16]. It has been proven that α-lipoic acid can induce GSH synthesis [17] and act as a metal-chelating agent [18–22] and intercellular signal-transducing factor [7, 23]. RLA is viewed as a very promising therapeutic and nutritional supplement. On the basis of clinical studies, it was shown that adding RLA to the therapy reduces the symptoms of rheumatoid arthritis [7], cardiovascular diseases [24–31], diabetic polyneuropathy [32], multiple sclerosis [33–36], and Alzheimer’s disease [7]. The biological and therapeutical properties of RLA are dependent on its bioavailability, tissue accumulation, and the process of metabolization [7]. RLA and RDHLA can be β-oxidized and/or S-methylated in cells [37, 38]. The most common RLA/RDHLA metabolites are presented in Fig. 2.

Fig. 2.

Molecular structures of RLA/RDHLA metabolites: bisnorlipoic acid (RBLA, 1), 4,6-bisthiohexanoic acid (RDHBLA, 2), tetranorlipoic acid (STLA, 3), and 2,4-bisthiobutanoic acid (SDHTLA, 4)

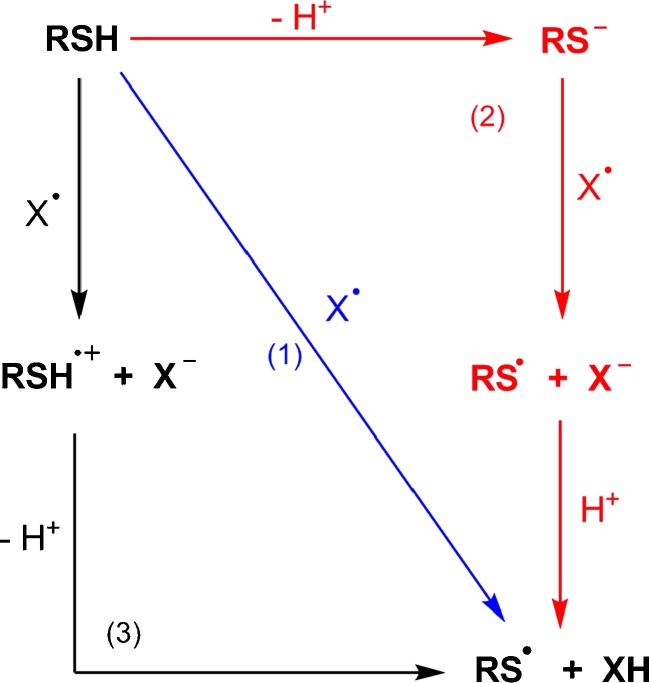
It is well known that free radicals can be deactivated in interactions with phenolic antioxidants via three mechanisms [39]. However, due to the similarity of O–H and S–H bonds, these processes can also be used to describe the antioxidant action of organosulfur compounds such as α-lipoic acid and its metabolites (Fig. 3). We can therefore distinguish the following mechanisms of free-radical scavenging:

1. HAT (hydrogen atom transfer) mechanism:

\documentclass[12pt]{minimal} \usepackage{amsmath} \usepackage{wasysym} \usepackage{amsfonts} \usepackage{amssymb} \usepackage{amsbsy} \usepackage{mathrsfs} \usepackage{upgreek} \setlength{\oddsidemargin}{-69pt} \begin{document}$$ \text{RSH} + {\text{X}^{ \cdot }} \to \text{R}{\text{S}^{ \cdot }} + \text{XH} $$\end{document} 1.1

• 2.SPLET (sequential proton loss electron transfer) mechanism:

\documentclass[12pt]{minimal} \usepackage{amsmath} \usepackage{wasysym} \usepackage{amsfonts} \usepackage{amssymb} \usepackage{amsbsy} \usepackage{mathrsfs} \usepackage{upgreek} \setlength{\oddsidemargin}{-69pt} \begin{document}$$ \text{RSH} \to \text{R}{\text{S}^{ - }} + {\text{H}^{ + }} $$\end{document} 1.2

\documentclass[12pt]{minimal} \usepackage{amsmath} \usepackage{wasysym} \usepackage{amsfonts} \usepackage{amssymb} \usepackage{amsbsy} \usepackage{mathrsfs} \usepackage{upgreek} \setlength{\oddsidemargin}{-69pt} \begin{document}$$ \text{R}{\text{S}^{ - }} + {\text{X}^{ \cdot }} + {\text{H}^{ + }} \to \text{R}{\text{S}^{ \cdot }} + \text{XH} $$\end{document} 1.3

• 3.SET-PT (single electron transfer followed by proton transfer) mechanism:

\documentclass[12pt]{minimal} \usepackage{amsmath} \usepackage{wasysym} \usepackage{amsfonts} \usepackage{amssymb} \usepackage{amsbsy} \usepackage{mathrsfs} \usepackage{upgreek} \setlength{\oddsidemargin}{-69pt} \begin{document}$$ \text{RSH} + {\text{X}^{ \cdot }} \to \text{RS}{\text{H}^{{ \cdot + }}} + {\text{X}^{ - }} $$\end{document} 1.4

\documentclass[12pt]{minimal} \usepackage{amsmath} \usepackage{wasysym} \usepackage{amsfonts} \usepackage{amssymb} \usepackage{amsbsy} \usepackage{mathrsfs} \usepackage{upgreek} \setlength{\oddsidemargin}{-69pt} \begin{document}$$ \text{RS}{\text{H}^{{ \cdot + }}} \to \text{R}{\text{S}^{ \cdot }} + {\text{H}^{ + }}. $$\end{document} 1.5

Fig. 3.

Antioxidant mechanisms of organosulfur compounds: HAT (1), SPLET (2), SET-PT (3). RSH organosulfur antioxidant, X ^• free radical. From [39]

There have been numerous publications describing experimental studies of the antioxidant properties of LA/DHLA, but this compound has not yet been investigated theoretically. In this paper, we report our detailed analysis of several of the molecular properties of LA/DHLA and RLA/RDHLA metabolites (RBLA/RDHBLA and STLA/SDHTLA), such as their molecular geometries and total molecular energies. We also describe the antioxidant properties of the compounds studied, which were derived by calculating physical antioxidant descriptors such as the adiabatic ionization potential (AIP), the bond dissociation enthalpy (BDE), the proton affinity (PA), the highest occupied molecular orbital energy (E _HOMO), and the spin density (SD) [40–44]. Because the free-radical scavenging action occurs in aqueous solution, we account for the solvating effect of water in our quantum-mechanical computations. Moreover, based on the calculations performed, we predict which of the three antioxidant mechanisms specified above is preferred for each of the compounds studied.

Computational methods

The quantum-chemical computations were performed using the Gaussian 03 W software package [45]. Full optimization of the geometries and energies for all of the molecules studied was carried out in the gas phase and in water without symmetry constraints. The geometries of the neutral compounds in their ground states were optimized using DFT with the restricted rB3LYP hybrid functional [46–48] combined with the 6-311 + G(3df,2p) and aug-cc-pVDZ basis sets. The geometry optimization of mono-, di-, and trianions was carried out with the rB3LYP/6-311 + G(df,2p) method. To optimize the radicals and cation radicals, the unrestricted uB3LYP/6-311 + G(3df,2p) level of theory was applied, since it gives the best results for open-shell molecular systems. Additionally, the neutral forms of LA and DHLA enantiomers were optimized using second-order Møller–Plesset perturbation theory [49] combined with the 6-31 + G(d,p) basis set in vacuum and water. rMP2(full)/6-31 + G(d,p) was applied to achieve a better description of electron correlation effects in the compounds studied. Aside from analyzing the geometries, the single point energies for RLA and SLA were calculated using DFT and MP2(full) methods combined with a wide spectrum of basis sets [6-31G(d,p), 6-31+G(d,p), 6-31++G(d,p), 6-311G(d,p), 6-311+G(d,p), 6-311++G(d,p), 6-31G(3df,2p), 6-31+G(3df,2p), 6-31++G(3df,2p), 6-311G(3df,2p), 6-311+G(3df,2p), 6-311++G(3df,2p), cc-pVDZ, cc-pVTZ, cc-pVQZ, aug-cc-pVDZ, and aug-cc-pVTZ] in order to compare the energies of stereoisomers.

To establish the starting geometries of the compounds studied and the locations of the structures with minimum energy, a conformational analysis was performed. Rotational potential energy profiles at rHF/6-31 + G(d,p) for RLA, RDHLA, SLA, and SDHLA were constructed by scanning the internal dihedral angles (α = C₁–C₂–C₃–C₄ and β = C₃–C₄–C₅–C₆ for LA, α = C₁–C₂–C₃–C₄ and γ = C₆–C₇–C₈–S₁′ for DHLA) over the ranges 180° ≤ α ≤ 360°, 180° ≤ β ≤ 360°, 180° ≤ γ ≤ 360°, with no constraint placed on any other geometric parameter (see the “Electronic supplementary material,” ESM). In the next step, the most stable structures obtained from the scan were fully optimized around each potential minimum without symmetry constraints. For all of the optimized structures, harmonic vibrational frequencies were calculated in order to verify the stationary points on the potential energy surfaces (PES).

The antioxidant properties of the molecules studied were described by calculating the numerical parameters (at 298 K) associated with their antioxidant mechanisms (described above), such as their proton affinities (PA; the SPLET mechanism), bond dissociation enthalpies (BDE; the HAT mechanism), and adiabatic ionization potentials (AIP; SET-PT mechanism) [50–55]:

\documentclass[12pt]{minimal} \usepackage{amsmath} \usepackage{wasysym} \usepackage{amsfonts} \usepackage{amssymb} \usepackage{amsbsy} \usepackage{mathrsfs} \usepackage{upgreek} \setlength{\oddsidemargin}{-69pt} \begin{document}$$ \text{PA} = {H_{\text{a}}} + {H_{\text{p}}} - {H_{\text{m}}}, $$\end{document} 1.6.

where H _a is the enthalpy of the anion, H _p is the enthalpy of the proton, and H _m is the enthalpy of the parent molecule;

\documentclass[12pt]{minimal} \usepackage{amsmath} \usepackage{wasysym} \usepackage{amsfonts} \usepackage{amssymb} \usepackage{amsbsy} \usepackage{mathrsfs} \usepackage{upgreek} \setlength{\oddsidemargin}{-69pt} \begin{document}$$ BDE = {H_{\text{r}}} + {H_{\text{H}}} - {H_{\text{m}}}, $$\end{document} 1.7.

where H _r is the enthalpy of the radical, H _H is the enthalpy of the H atom, and H _m is the enthalpy of the parent molecule; and

\documentclass[12pt]{minimal} \usepackage{amsmath} \usepackage{wasysym} \usepackage{amsfonts} \usepackage{amssymb} \usepackage{amsbsy} \usepackage{mathrsfs} \usepackage{upgreek} \setlength{\oddsidemargin}{-69pt} \begin{document}$$ AIP = {E_{\text{cr}}} - {E_{\text{m}}}, $$\end{document} 1.8.

where E _cr is the total energy of the cation radical, and E _m is the total energy of the parent molecule.

In the calculations, the following enthalpy values were used: H(H^•)_vacuum = −0.49764 Ha (i.e. hartrees), [42], Δ_hydr H(H^•) = −0.00152 Ha [56, 57]; H(H⁺)_vacuum = 0.00236 Ha [40]; Δ_hydr H(H⁺) = −0.41516 Ha [58].

Additional parameters describing the electron-donating properties of the compounds studied (the HOMO orbital distribution and the spin density distribution) were calculated at the B3LYP/6-311 + G(3df,2p) level of theory in vacuum and in water.

The optimizations of all forms of the compounds investigated and the calculations of the antioxidant parameters in water were performed using the C-PCM solvation model (conductor-like polarizable continuum model), [59]. In the calculations, a dielectric constant of 78.39 was used for water. This approach was employed and described in our previous works on the antioxidant properties of phenolic compounds (trans-resveratrol and its derivatives) [41, 60–62].

Results and discussion

The optimized geometries of LA and DHLA enantiomers

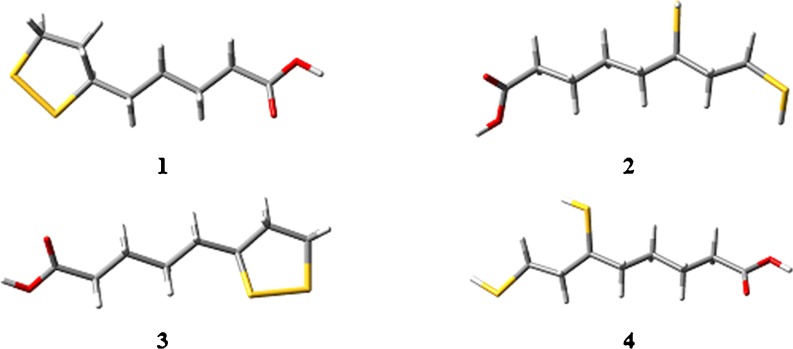
Optimizing all of the compounds’ geometries at the rB3LYP/6-311 + G(3df,2p), rB3LYP/aug-cc-pVDZ, and rMP2(full)/6-31 + (d,p) levels of theory leads to the absolute energy minima on the potential energy surface, which is confirmed by the absence of imaginary frequencies. The bond lengths (in Å) and the angles (in degrees) of the equilibrium optimized neutral structure of RLA in comparison and the corresponding experimental crystallographic data are summarized in Table 1 and Table S1 of the ESM. It should be pointed out, that regardless of the method and basis set used, the equilibrium values of geometrical parameters are almost identical and very similar to the results obtained by Stroud and Carlisle (1972) for the crystallographic structure of LA [6]. Additionally, the results presented in Tables 2 and 3 and Tables S2 and S3 of the ESM reveal that there are no geometric differences between enantiomers (RLA and SLA; RDHLA and SDHLA, see Fig. 4), which is in good agreement with the statement that optical isomers are like mirror images of each other. The analysis of ΔE _total for RLA and SLA, as calculated using DFT and MP2(full) employing a wide range of basis sets (Table 4 and Table S4 of the ESM), confirms that the enantiomers are isoenergetic structures.

Table 1.

The bond lengths (in Å) and angles (in degrees) of RLA [obtained using rMP2(full)/6-31 + (d,p) and optimized in vacuum and water] along with the experimental values from the crystallographic structure of RLA [6]

Bond length or angle	rMP2(full)/6-31 + G(d,p)		Experiment
	Vacuum	Water
C1–C2	1.50	1.50	1.50
C2–C3	1.52	1.52	1.55
C3–C4	1.52	1.52	1.53
C4–C5	1.53	1.53	1.51
C5–C6	1.52	1.52	1.53
C6–C7	1.54	1.54	1.55
C7–C8	1.54	1.54	1.51
S1′–C8	1.82	1.82	1.79
S2′–C6	1.82	1.83	1.83
S1′–S2′	2.07	2.08	2.05
O3′–C1	1.36	1.36	1.31
O3″–C1	1.22	1.22	1.20
C1–C2–C3	113.02	113.46	112.99
C2–C3–C4	111.92	111.70	110.39
C3–C4–C5	112.17	111.93	109.28
C4–C5–C6	112.46	112.18	115.38
C5–C6–C7	113.48	113.56	112.89
C6–C7–C8	111.86	112.29	112.61
C7–C8–S1′	109.26	109.36	112.61
C8–S1′–S2′	90.22	109.36	95.56
C6–S2′–S1′	90.63	90.97	92.84
C5–C6–S2′	110.46	110.27	111.37
C7–C6–S2′	108.17	108.28	106.76
O3′–C1–C2	111.24	111.50	113.39
O3″–C1–C2	126.16	126.09	124.89
O3′–C1–O3″	122.60	122.41	121.89

Table 2.

The bond lengths (in Å) and angles (in degrees) of RLA and SLA [obtained using rMP2(full)/6-31 + (d,p) and optimized in vacuum and water]

Bond length or angle	rMP2(full)/6-31 + G(d,p)
	Vacuum		Water
	RLA	SLA	RLA	SLA
C6–C7	1.54	1.54	1.54	1.54
C7–C8	1.54	1.54	1.54	1.54
S1′–C8	1.82	1.82	1.82	1.82
S2′–C6	1.82	1.83	1.83	1.83
S1′–S2′	2.07	2.07	2.08	2.08
O3′–C1	1.36	1.36	1.36	1.36
O3″–C1	1.22	1.22	1.22	1.22
C6–C7–C8	111.86	111.95	112.29	112.38
C7–C8–S1′	109.26	109.41	109.36	109.72
C8–S1′–S2′	90.22	90.75	90.60	91.65
S1′–S2′–C6	90.63	90.25	90.97	90.29
S2′–C6–C7	108.17	107.91	108.28	107.59
C1–C2–C3–C4	179.47	179.99	179.97	179.77
C3–C4–C5–C6	177.65	172.96	177.37	172.53
C8–S1′–S2′–C6	46.99	46.76	46.02	45.35

Table 3.

The bond lengths (in Å) and angles (in degrees) of RDHLA and SDHLA [obtained using rMP2(full)/6-31 + (d,p) and optimized in vacuum and water]

Bond length or angle	rMP2(full)/6-31 + G(d,p)
	Vacuum		Water
	RDHLA	SDHLA	RDHLA	SDHLA
C6–C7	1.53	1.53	1.53	1.53
C7–C8	1.52	1.52	1.52	1.52
S1′–C8	1.83	1.82	1.83	1.82
S1′–H1′	1.33	1.33	1.33	1.33
S2′–C6	1.83	1.83	1.83	1.83
S2′–H2′	1.33	1.33	1.33	1.33
O3′–C1	1.36	1.36	1.36	1.36
O3″–C1	1.22	1.22	1.22	1.22
C7–C8–S1′	110.06	108.79	110.37	108.93
C7–C6–S2′	112.73	112.94	112.74	112.78
C6–C7–C8	116.05	114.00	116.34	113.88
C1–C2–C3–C4	179.97	179.86	179.90	179.71
C5–C6–C7–C8	176.62	175.94	177.79	174.01

Fig. 4.

The geometries of RLA (1), RDHLA (2), SLA (3), and SDHLA (4) [obtained using rMP2(full)/6-31 + (d,p) and optimized in water]

Table 4.

The total energy values [in Ha] of RLA and SLA [obtained using rMP2(full) and optimized in vacuum]. ΔE _total (in kcal mol⁻¹) of RLA and SLA

Basis set	MP2(full)
	RLA	SLA	ΔE total
6-31G(3df,2p)	−1258.253	−1258.253	0.305
6-31 + G(3df,2p)	−1258.275	−1258.275	0.226
6-31++G(3df,2p)	−1258.276	−1258.277	0.282
6-311++G(3df,2p)	−1258.810	−1258.811	0.526
cc-pVDZ	−1257.728	−1257.728	0.447
cc-pVTZ	−1258.507	−1258.507	0.498
cc-pVQZ	−1258.877	−1258.878	0.543
aug-cc-pVDZ	−1257.861	−1257.861	0.369
aug-cc-pVTZ	−1258.597	−1258.597	0.474

The optimized geometries of RLA/RDHLA metabolites

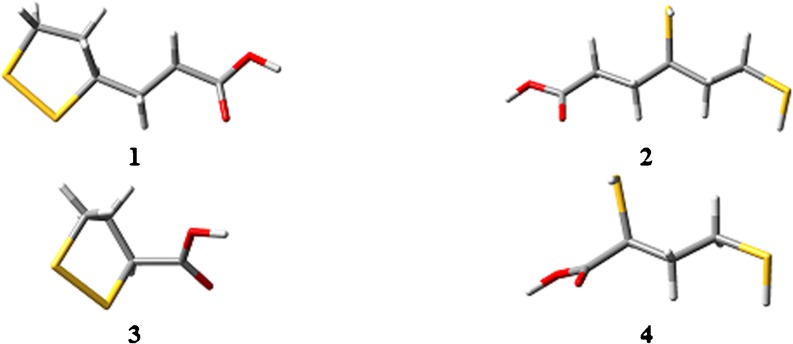
Optimization of the geometries of the metabolites was performed at the rB3LYP/6-311 + G(3df,2p) level of theory in vacuum and water. The equilibrium geometries (Fig. 5) are at the absolute energy minima on the potential energy surface, as confirmed by the absence of imaginary frequencies. A comparative analysis of the geometrical parameters in vacuum (Table S5 of the ESM) and in water (Table S6 of the ESM) reveals that there is good agreement between the molecular structures of RLA, RDHLA, and their metabolites. All of the compounds studied demonstrate similarities in the geometry of the aliphatic chain. The values of the dihedral angle C_*–S₁′–S₂′–C_* in the cyclic forms (where C_* has a different number in each cyclic form) indicate that the pentatomic ring is not a planar system but has the conformation of an envelope. The 1′-S–H and 2′-S–H bond lengths of the reduced forms are practically identical in both vacuum and water, which indicates a lack of influence of solvation effects.

Fig. 5.

The geometries of RLA/RDHLA metabolites [obtained using rB3LYP/6-311 + G(3df,2p) and optimized in water]: RBLA (1), RDHBLA (2), STLA (3), and SDHTLA (4)

AIP, BDE, PA, spin density, and HOMO orbital distribution

AIP is a very important physical property that describes the process of single electron donation by the antioxidant. The smaller the AIP value, the greater the chance that ionization will occur. Molecules with low AIP values are considered very good antioxidants. Calculations (Table 5) indicate that the lowest values of AIP in both vacuum and water media are associated with oxidized forms: RLA, RBLA, STLA (in order of increasing AIP) in vacuum and STLA, RLA, RBLA (in order of increasing AIP) in water. In vacuum, the same order can be ascribed to the reduced forms: RDHLA, RDHBLA, SDHTLA, but in water, we observe the following growing order: RDHBLA, RDHLA, SDHTLA. The AIP values in water are lower than in vacuum for all of the compounds studied. This is connected to the strong electrostatic interaction of the polar medium with cation radical forms. Because AIP represents the SET-PT mechanism, it is possible that this mechanism is more preferable in water than in vacuum.

Table 5.

The AIP (in kcal mol⁻¹) and ΔAIP (in kcal mol⁻¹) values [calculated at the B3LYP/6-311 + G(3df,2p) level in vacuum and water] of LA/DHLA enantiomers and RLA/RDHLA metabolites

Compound	Vacuum		Water
	AIP (kcal/mol)	ΔAIP (kcal/mol)	AIP (kcal/mol)	ΔAIP (kcal/mol)
RLA	171	0	131	0
SLA	171	0	131	0
RDHLA	193	22	159	28
SDHLA	191	21	157	26
RBLA	173	2	132	1
RDHBLA	195	24	158	27
STLA	193	22	128	−3
SDHTLA	199	28	162	31

The stability of the S–H bond can be evaluated using a thermodynamic parameter, the homolytic BDE. Hydrogen atom transfer from antioxidant to radical is a possible antioxidant mechanism. Because the reduced forms of the compounds studied have two S–H bonds, we have taken into consideration all possible reactions. The calculated values of the BDE are presented in Table 6. Based on the results obtained, it is clear that RDHLA and its metabolites have similar S–H bond stabilities. Just as for the AIP results (Table 5), there are no differences between the DHLA stereoisomers in vacuum and in water. In water, the S–H bond is less prone to homolytic fission than in vacuum, because of higher BDE values. This means that the HAT mechanism is not preferable in a polar medium for the compounds studied. Hydrogen atom transfer is hindered because a polar medium increases the stability of S–H bonds.

Table 6.

The BDE (in kcal mol⁻¹) and ΔBDE (in kcal mol⁻¹) values [calculated at the B3LYP/6-311 + G(3df,2p) level in vacuum and water] of RDHLA, SDHLA, RDHBLA, and SDHTLA

Bond	Vacuum		Water
	BDE (in kcal/mol)	ΔBDE (in kcal/mol)	BDE (in kcal/mol)	ΔBDE (in kcal/mol)
1′-SH–RDHBLA	86	1	96	1
1′-SH–RDHLA	85	0	96	0
1′-SH–SDHLA	85	0	95	0
1′-SH–SDHTLA	85	1	96	1
2′-SH–RDHBLA	85	1	97	1
2′-SH–RDHLA	86	1	96	1
2′-SH–SDHLA	86	2	97	2
2′-SH–SDHTLA	87	2	97	2

The PA values were calculated for the neutral forms of RDHLA, RDHBLA, and SDHTLA, as well as for their 3′-O-monoanions, 1′-S,3′-O-dianions, and 2′-S,3′-O-dianions. The results obtained are presented in Table 7. For all of the compounds studied, the PA values in water are much smaller than those in vacuum, which means that in a polar medium the SPLET mechanism is greatly preferred. Turning our attention to the ΔPA values, we notice that S–H bonds in the monoanions and dianions are more prone to heterolytic fission than those in neutral forms. The higher PA values observed for the 2′-S–H bond than for the 1′-S–H bond suggest that the former break more easily.

Table 7.

The PA (in kcal mol⁻¹) and ΔPA (in kcal mol⁻¹) values [calculated at the rB3LYP/6-311 + G(3df,2p) level in vacuum and water] of the neutral, mono-, and dianionic forms of RDHLA and its metabolites

Compound	1′-S–H				2′-S–H
	Vacuum		Water		Vacuum		Water
	PA	ΔPA	PA	ΔPA	PA	ΔPA	PA	ΔPA
RDHLA	344	0	34	0	342	0	44	0
RDHBLA	350	6	23	−11	341	−1	32	−11
SDHTLA	349	4	33	0	339	−3	29	−15
3′-O-mRDHLA	384	40	34	0	388	46	34	−10
3′-O-mRDHBLA	398	53	25	−8	408	66	26	−17
3′-O-mSDHTLA	414	70	27	−7	434	92	28	−16
2′-S,3′-O-dRDHLA	458	113	36	2	−	−	−	−
1′-S,3′-O-dRDHLA	−	−	−	−	461	120	37	−7
2′-S,3′-O-dRDHBLA	466	122	37	3	−	−	−	−
1′-S,3′-O-dRDHBLA	−	−	−	−	476	135	38	−6
2′-S,3′-O-dSDHTLA	481	137	37	3	−	−	−	−
1′-S,3′-O-dSDHTLA	−	−	−	−	501	160	38	−6

Upon comparing the AIP, PA, and BDE values for all of the compounds studied, it is clear that the preferred antioxidant pathway in a polar medium for lipoic acid and its metabolites is the SPLET mechanism. The HAT mechanism is preferred in nonpolar media.

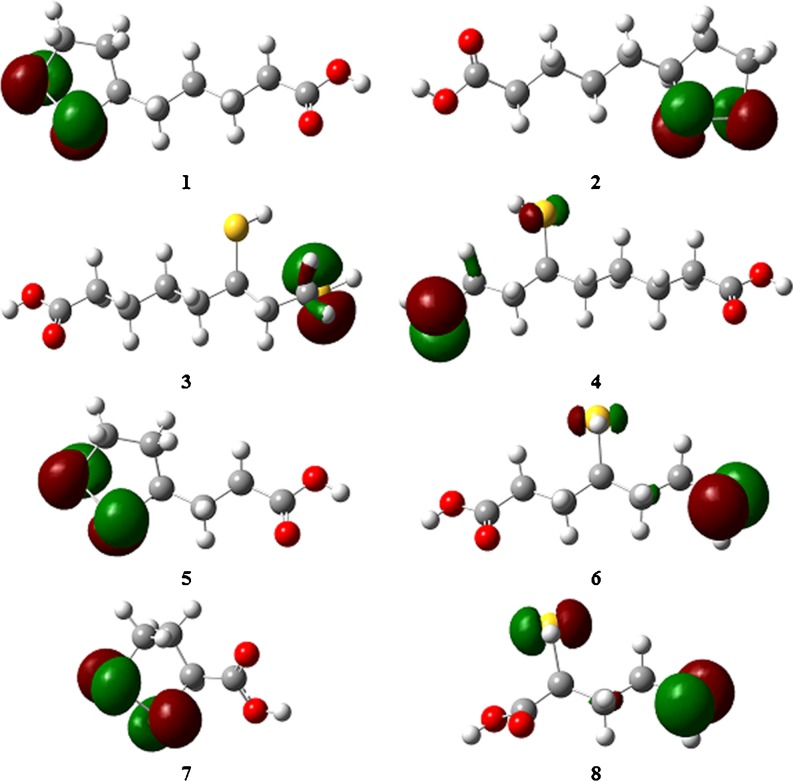
When describing the free-radical scavenging activities of molecules, parameters such as the spin density (SD) distribution in free radicals and the energy of the HOMO orbital are also useful. The SD has been calculated for the 1′-S- and 2′-S-radicals of RDHLA, SDHLA, RDHBLA, and SDHTLA. According to these calculations (Table 8), the highest concentration of SD is observed for the S₁′ atom in 1′-S-radicals and for S₂′ in 2′-S-radicals. In comparison, the SD values for the O₃′ and O₃″ atoms in these radicals are very small, which proves the absence of electron delocalization through the whole molecule. The SD values in water are slightly higher than those in vacuum for all of the radicals studied (Table 8). We can therefore conclude that the antioxidant mechanisms involving radical action are not preferred in polar media. The energy of the HOMO orbital is another important molecular parameter associated with the scavenging of free radicals. Molecules with higher E _HOMO values have stronger electron-donating abilities. All of the compounds studied have similar values of E _HOMO (Table 9). The electronic density distribution in these orbitals is concentrated on the sulfur atoms (Fig. 6). Similar to the SD distribution, there is no delocalization of HOMO orbitals. It can be predicted that S–S and S–H bonds are the most probable sites of free-radical attack in all of the molecules studied.

Table 8.

The spin density (SD) values [calculated at the uB3LYP/6-311 + G(3df,2p) level in vacuum and water] of RDHLA, SDHLA, RDHBLA, and SDHTLA radicals

Radical	Vacuum				Water
	S1′	S2′	O3′	O3″	S1′	S2′	O3′	O3″
1′-S-RDHBLA	1.0662	0.0137	−0.0002	0.0000	1.0700	0.0149	−0.0002	0.0000
1′-S-RDHLA	1.0487	0.0033	0.0000	0.0000	1.0642	0.0045	0.0000	0.0000
1′-S-SDHLA	1.0796	0.0084	0.0000	0.0000	1.0830	0.0080	0.0000	0.0000
1′-S-SDHTLA	1.0522	0.0116	−0.0001	0.0001	1.0569	0.0110	−0.0002	0.0000
2′-S-RDHBLA	−0.0042	1.0496	0.0002	0.0000	−0.0064	1.0540	0.0002	−0.0001
2′-S-RDHLA	0.0041	1.0611	0.0000	0.0001	0.0032	1.0693	0.0001	0.0001
2′-S-SDHLA	−0.0088	1.0560	0.0000	0.0001	0.0163	1.0397	0.0001	0.0003
2′-S-SDHTLA	0.0011	1.0313	0.0031	0.0147	0.0005	1.0349	0.0024	0.0130

Table 9.

The HOMO orbital distributions (E _HOMO and ΔE _HOMO) of LA/DHLA enantiomers and RLA/RDHLA metabolites [obtained using rB3LYP/6-311 + G(3df,2p) and optimized in vacuum and water]

Compounds	Vacuum			Water
	E HOMO (Ha)	E HOMO (eV)	ΔE HOMO (eV)	E HOMO (Ha)	E HOMO (eV)	ΔE HOMO (eV)
RLA	−0.215	−5.9	0.0	−0.211	−5.7	0.0
SLA	−0.214	−5.8	0.0	−0.212	−5.8	0.0
RDHLA	−0.245	−6.7	0.8	−0.240	−6.5	0.8
SDHLA	−0.244	−6.6	0.8	−0.241	−6.5	0.8
RBLA	−0.218	−5.9	0.1	−0.212	−5.8	0.0
RDHBLA	−0.246	−6.7	0.8	−0.247	−6.7	1.0
STLA	−0.220	−6.0	0.1	−0.220	−6.0	0.3
SDHTLA	−0.249	−6.8	0.9	−0.246	−6.7	0.9

Fig. 6.

The HOMO orbital distributions in RLA (1), SLA (2), RDHLA (3), SDHLA (4), RBLA (5), RDHBLA (6), STLA (7), and SDHTLA (8). The calculations were accomplished in vacuum with the isovalue 0.07

Conclusions

Structure–antioxidant parameter relationships of α-lipoic acid and its natural metabolites bisnorlipoic acid and tetranorlipoic acid in their oxidized and reduced forms have been investigated using quantum-chemical methods: DFT/B3LYP and MP2(full). On the basis of the results obtained, we can confirm that the SH groups of the reduced forms are responsible for their antioxidant properties. More importantly, we propose that the most preferable antioxidant mechanism in polar media is SPLET. However, in nonpolar media, the HAT mechanism is preferred, as shown by calculations performed for isolated molecules. α-Lipoic acid and its natural metabolites are not the only available organosulfur acids that act as antioxidants; LA/DHLA derivatives should also be included in quantum-chemical investigations.

Electronic supplementary material

Below is the link to the electronic supplementary material.