Thermal modulation of birefringence observed in a crystalline molecular gyrotop

Abstract

Recently, functional organic materials have been put into practical use. The application of molecular motions has the potential to create new molecule-based materials. For this reason, considerable attention has been focused on the chemistry and properties of molecular machines in which mechanical motions of parts of the molecules are observed. In particular, phenylene rotation in the crystalline state has been investigated using framed molecular gyrotops having a phenylene rotor encased in three long alkyl spokes. In this study, we show thermal modulation of birefringence in a crystal due to the states of dynamic equilibrium of a novel molecular gyrotop. A macrocage molecule having a bridged phenylene rotor was synthesized as a novel molecular gyrotop. Rapid rotation of the phenylene rotor of the molecular gyrotop was confirmed by solid-state ²H NMR spectroscopy that showed changes in the optical properties of a single crystal, i.e., the thermal modulation of birefringence. These results are the first application of the dynamic states in a crystal causing an optical change. These phenomena were also confirmed by control experiments using a molecular gyrotop with a nonrotating xylene rotor. We anticipate our finding to be a starting point for the creation of a new field of material chemistry that will make use of the dynamic states of molecules.

Organic materials, such as liquid crystals and organic light-emitting devices, have been put into practical use, and they have improved the lifestyles of people. These functional organic molecules usually contain moieties of planar π-electron systems such as benzene and naphthalene (1). Benzene is one of the simplest aromatic molecules, and due to its planar structure, a strong anisotropy exists in its physical properties between the in-plane and out-of-plane directions. Therefore, an aggregate of oriented π-electron systems such as crystals and liquid crystals shows anisotropy in its physical properties. When the orientation of molecules inside an ordered aggregation can be switched by partial molecular motion, the physical properties may switch between being anisotropic and isotropic, or they may change the direction of anisotropy as observed in the study of functional liquid crystals and so on. In other words, the application of molecular motions inside an ordered aggregate has the potential to create new molecule-based materials. For this reason, considerable attention has been focused on the chemistry of molecular machines (2–9) in which mechanical motions of parts of the molecules are observed. In particular, the chemistry of partial molecular rotations in the crystalline state has been investigated using molecules that consist of rotors and stators (2, 8–22). Macrocyclic molecules having a rotor encased in a three-spoke stator are expected to have the functions of gyroscopes and compasses in the crystalline state; such molecules were reported as molecular gyroscopes due to structural similarity (10–21). The kinetics of the dipolar rotor have been investigated by spectroscopies (2, 13, 14). However, their functions based on their states of dynamic equilibrium have not been investigated well. We report herein the synthesis of a framed molecular gyrotop 1 and an application that makes use of an optical property change, i.e., the thermal modulation of birefringence a single crystal, due to the structural change resulting from the rapid rotation of the phenylene rotor.

Results and Discussion

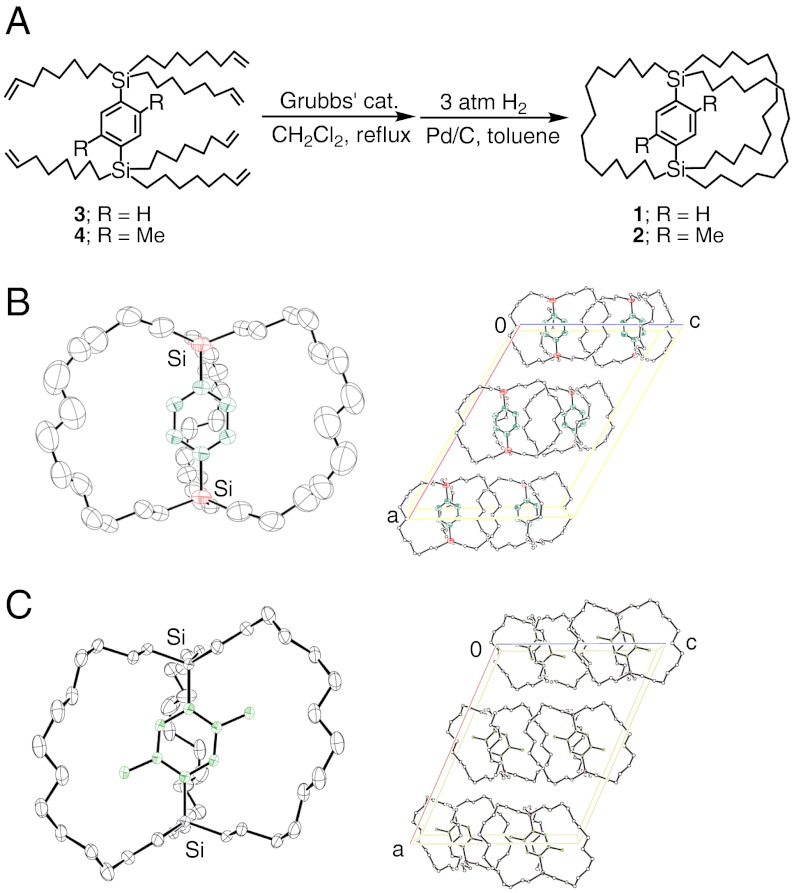
A molecular gyrotop or molecular gyroscope, as first proposed by Garcia-Garibay and coworkers (12), consists of a frame cage and a rotor. We have designed a molecular gyrotop 1 that has a phenylene rotor encased in three long alkyl spokes. The tetradecyl spokes are used as a cage that is suitable for the phenylene rotor because similar phenylene-bridged molecular gyroscopes that have 3, 6, 8, 11-tetrasila-7-oxa-tridecyl spokes have been reported by us previously (18, 19). The scheme for the synthesis of the molecular gyrotops is shown in Fig. 1A. For a control experiment, a second compound, 2, that has a rotor that is bulkier than that of compound 1 was also synthesized. The molecular gyrotops, i.e., compounds 1 and 2 were synthesized by the ring-closing metathesis of p-bis(tri-7-octenyl)silylbenzenes, 3 and 4, that were synthesized by a reaction of dilithiobenzenes with tri-7-octenylchlorosilanes, and subsequent hydrogenation at 3 atm hydrogen pressure with Pd/C as a catalyst (20). The isolation of the cage compounds from the reaction mixture was carried out by preparative gel permeation chromatography. Recrystallization of the purified compounds from a tetrahydrofuran-methanol solution afforded single crystals.

Fig. 1.

Synthesis and structure of a molecular gyrotop 1. (A) Synthesis of a molecular gyrotops 1 and 2. (B) Molecular structure of 1 in the crystalline form (30% thermal probability ellipsoids), and its crystal-packing diagram. (C) Molecular structure of 2 in the crystalline form (30% thermal probability ellipsoids) and its crystal-packing diagram.

The structures of 1 and 2 were confirmed by ¹H, ¹³C, ²⁹Si NMR spectroscopy and X-ray crystallography. Fig. 1 B and C show the molecular structures of a single crystal of 1 and 2, respectively. The crystal structures of 1 and 2 are nearly identical, and they have the same crystal system and the same space group. In addition, both, 1 and 2, have C₂ symmetry in their molecules, and a C₂ axis perpendicular to the rotation axis passes through two silicon atoms. The molecules are packed in crystals arranged by their rotation axes.

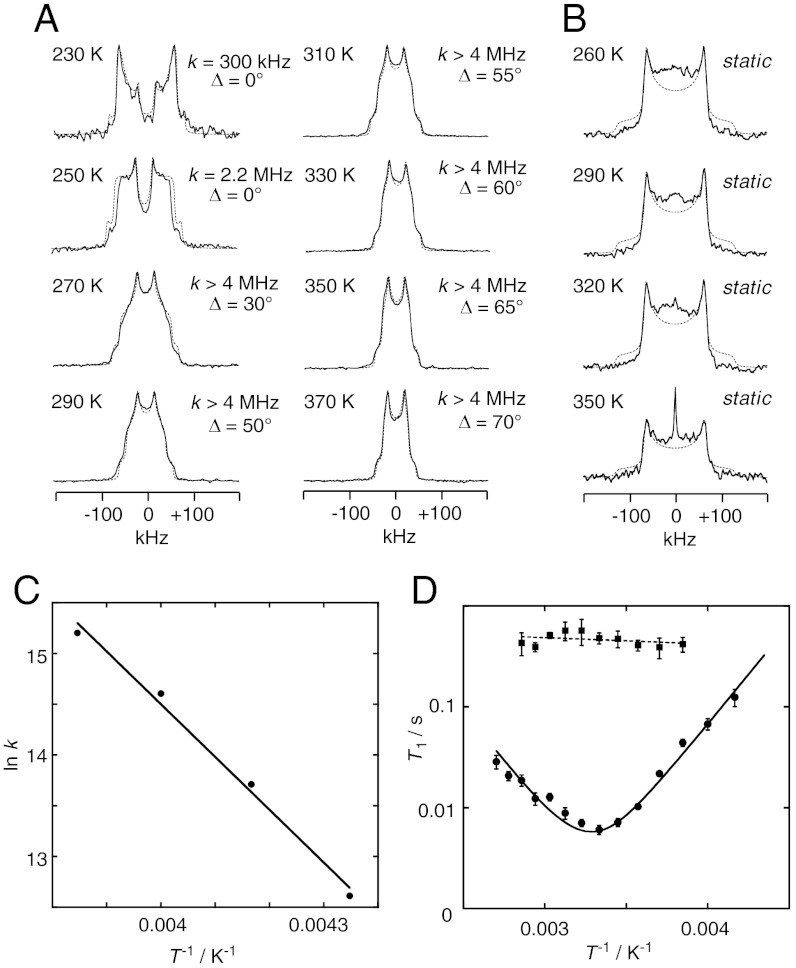
Solid-state ²H NMR spectroscopy was used to estimate the rate and angular range of the phenylene flip in the crystals. Fig. 2A shows the temperature-dependent quadrupolar echo ²H NMR spectra of 1-d₄ in which the phenylene moiety was labeled with four deuterium atoms. At 230 K, the phenylene ring slowly undergoes a 180° flip between the two equilibrium sites that were determined by X-ray structural analysis. The rates of flipping in the temperature range from 230 to 260 K were estimated by simulations (23) of the spectral line shapes (Pake patterns) (Fig. 2A). The activation energy, E_a, for the phenylene flip was estimated by the Arrhenius plot of the rates of flipping (Fig. 2C), and E_a was estimated to be 10.3 kcal/mol.

Fig. 2.

Dynamics and properties of the crystalline molecular gyrotop 1. (A) Temperature dependence of the solid-state ²H NMR spectra of 1-d₄ (solid line: observed spectra; dotted line: spectra simulated with the designated exchange rate constants k and angular displacement Δ). (B) Temperature dependence of the solid-state ²H NMR spectra of 2-d₂ [solid line: observed spectra; dotted line: simulated spectra]. (C) Arrhenius plots of the flipping rates of the molecular gyrotop 1. From the plots, the following parameters were estimated: activation energy, E_a = 10.3 kcal/mol, preexponential factor, A = 2.15 × 10¹⁵ s^-1. (C) Temperature dependence of the ²H spin-lattice relaxation times (T₁) in the molecular gyrotops 1 (●) and 2 (▪). The solid lines represent the predicted values based on the isotropic rotational diffusion model (Eq. 1) whose parameters are as follows: q_cc = 140 kHz, E_a = 9.0 kcal/mol, ω₀/2π = 76.7 MHz, and τ_∞ = 4.65 × 10^-16 s.

Upon heating above 270 K, the line widths of the observed spectra became narrower, indicating a continuous rotation of the phenylene moiety in 1-d₄ with an exchange frequency over the fast limit (> 4 MHz). The spectra were simulated by assuming a 180° flip in the fast exchange limit with an angular displacement (Δ), which corresponds to the 2σ-range of the oscillation angle of the phenylene moiety around the equilibrium position with a Gaussian distribution (cf. Fig. 3A). The simulated spectra perfectly reproduced the observed spectra. In order to confirm the acceleration of the rate of the phenylene dynamics of 1-d₄ at temperatures above 270 K, the temperature dependence of the spin -lattice relaxation time (T₁) was investigated (24). The relaxation time, T₁, for the molecular gyrotops was measured using the inversion-recovery quadrupole echo pulse sequence. Fig. 2D compares the experimental results of the ²H NMR spin-lattice relaxation measurements of the molecular gyrotop 1-d₄ with the calculated spin-lattice relaxation times. The T₁ measurements of 1-d₄ in the temperature range from 240 to 370 K show that T₁ is strongly dependent on temperature and exhibits typical T₁ behavior, with a sharp minimum close to 300 K. At the minimum, the T₁ relaxation time is found to be 0.0060 s. To interpret the temperature dependence of T₁, the isotropic rotational diffusion model (25) (Eq. 1) was used.

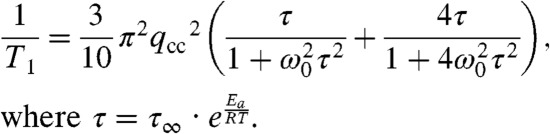[1]

Here, ω₀/2π is the Larmor frequency of deuterium, q_cc is the electric quadrupole coupling constant, and τ is a single correlation time that exhibits Arrhenius behavior. According to the model, the observed values of T₁ agreed with the calculated values of T₁. The fitted parameters calculated by the isotropic rotational diffusion model (Eq. 1) are given in the caption of Fig. 2D. The activation energy and quadrupole coupling constants are consistent with those derived from the analysis of the Pake pattern of the ²H NMR spectra. These results indicate that the phenylene flip accelerated with an increased in the temperature and that the rates completely obeyed the Arrhenius rule in the temperature range from 230 to 370 K at least.

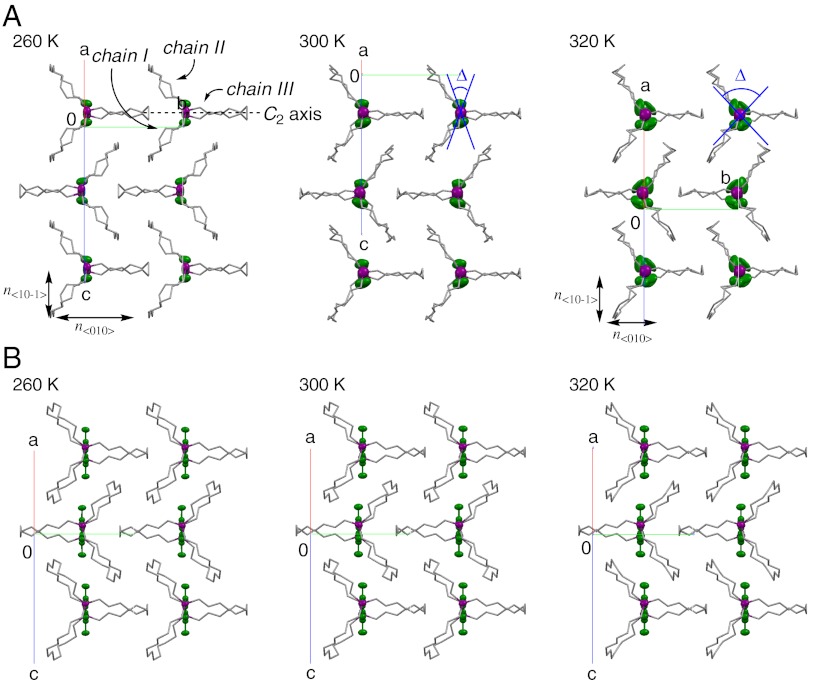
Fig. 3.

Temperature dependence of the crystal structure of molecular gyrotops (A) 1 and (B) 2. Single crystal X-ray diffraction studies [projection on the {100} face with the disorder of the phenylene moiety and the angular displacement Δ]. Silicon atoms and the rotors are indicated with 50% probability ellipsoids. The fast (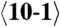) and slow (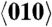) optical axes are indicated and the observed birefringence (Δn) is the difference between these two refractive indices.

In contrast to the dynamic behavior of the phenylene rotor of 1 observed in the crystalline state, the xylene moiety of 2 is static inside the crystal. Fig. 2B shows the temperature-dependent quadrupolar echo ²H NMR spectra of 2-d₂ in which the phenylene moiety was labeled with two deuterium atoms. The observed spectra at temperatures below 350 K were simulated by assuming the xylene moiety is static and do not undergo flipping. That there was no flipping of the xylene moiety of 2 was also confirmed by the spin-lattice relaxation time (T₁) using ²H NMR spectroscopy. Fig. 2D shows the experimental results of the ²H NMR spin-lattice relaxation measurements of the molecular gyrotop 2-d₂. An almost constant T₁ relaxation time of 0.5 ± 0.1 s is observed in the temperature range from 260 to 350 K, indicating that spin-lattice relaxation is not induced by the flipping of the xylene moiety.

One of the most important characteristics of the phenylene moiety of 1 is that the orientation of phenylene in the crystal is ordered at temperatures below 270 K, and it becomes disordered at temperatures above 270 K. X-ray crystallographic analyses of 1 were performed at 260, 300, and 320 K to elucidate the disorder of phenylene in the crystal. Fig. 3A shows the projection on the {100} face of a single crystal of 1 at various temperatures. At 260 K, the phenylene moiety of each molecule is arranged perpendicular to the b axis. The reason for this orientation of the phenylene moiety in the crystal is as follows: Because the cage deforms to recognize the planar phenylene during its slow flip, the molecule adopts a C₂ symmetric structure, with chains I and II identical to each other but different from chain III. Close packing of the molecules with C₂ symmetry in the crystal yields a crystal structure in which all the molecules are oriented in the same direction. At 300 K, the anisotropic temperature factors of phenylene become large, and at 320 K, structurally disordered phenylene was observed in three positions around the rotation axis. This is in close agreement with the results of the rapid rotation of phenylene with angular displacement observed in the ²H NMR study. In contrast, almost identical crystal structures were observed by the X-ray crystallographic analyses of 2 at 260, 300, and 320 K, as shown in Fig. 3B. Because the xylene moiety of the crystals did not show flipping and rotation inside the crystal in the solid-state NMR analysis, it is understood that the position of the xylene moiety is not changed by varying the temperature.

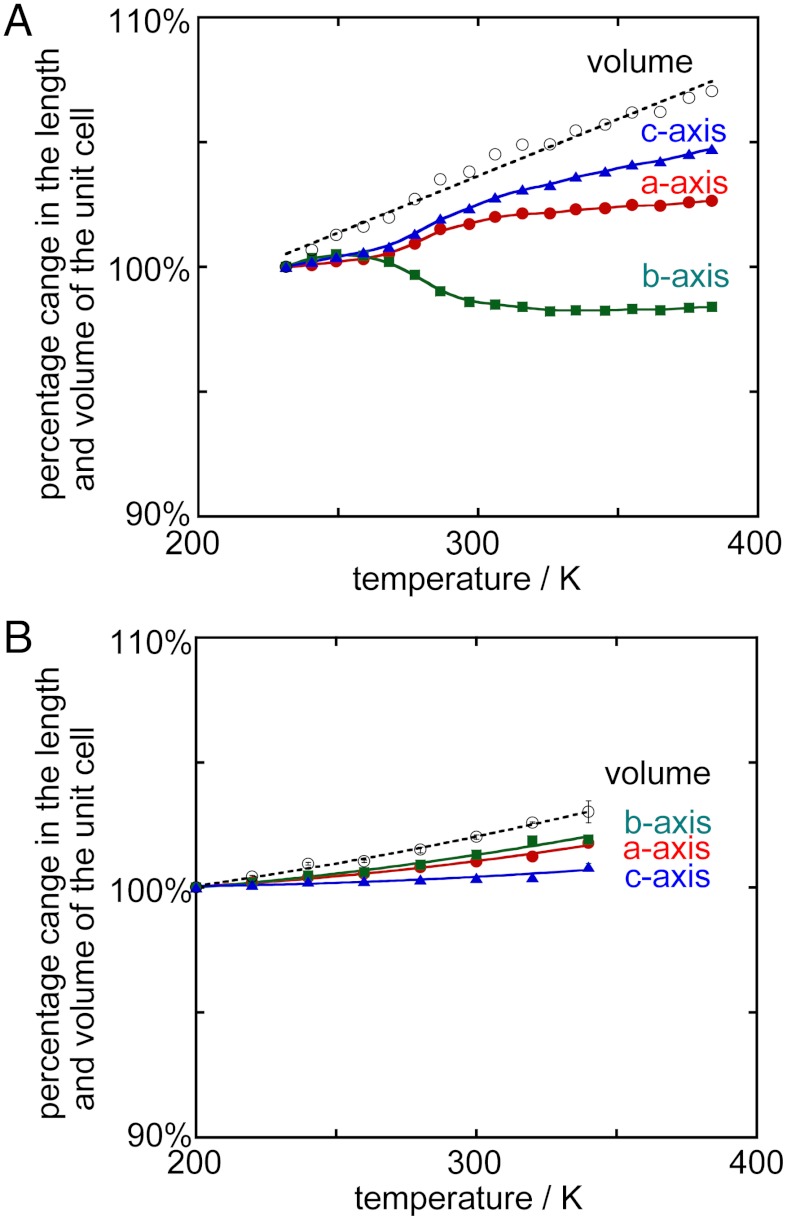
The thermodynamic behavior of phenylene also causes a slight deformation of the crystal lattice. Fig. 4A shows the temperature dependence of the crystal parameters of the molecular gyrotop 1 as determined by an X-ray powder diffraction study. At high temperatures, the crystal lattice varied continuously in a manner consistent with the degree of angular displacement of the phenylene moiety observed by the ²H NMR analysis and single crystal X-ray diffraction. As the temperature increased, the length of the b axis decreased slightly, and due to thermal expansion, the lengths of the other axes and the cell volume increased. On the other hand, anisotropic deformation of the crystal was not observed in 2. Fig. 4B shows the temperature dependence of the crystal parameters of the molecular gyrotop 2 as determined by a single crystal X-ray diffraction study. With an increase in the temperature, all the cell axes became slightly longer and the cell volume became larger due to thermal expansion. Therefore, the rotational motion is essential for the anisotropic deformation of the crystal.

Fig. 4.

Plots of the percentage changes in the lengths and volume of the unit cell percentage of lengths and volume of the unit cell determined by the X-ray diffraction study vs. temperature (A) 1 [the values are normalized to the data at 231 K (100%)] and (B) 2 [the values are normalized to the data at 200 K (100%)].

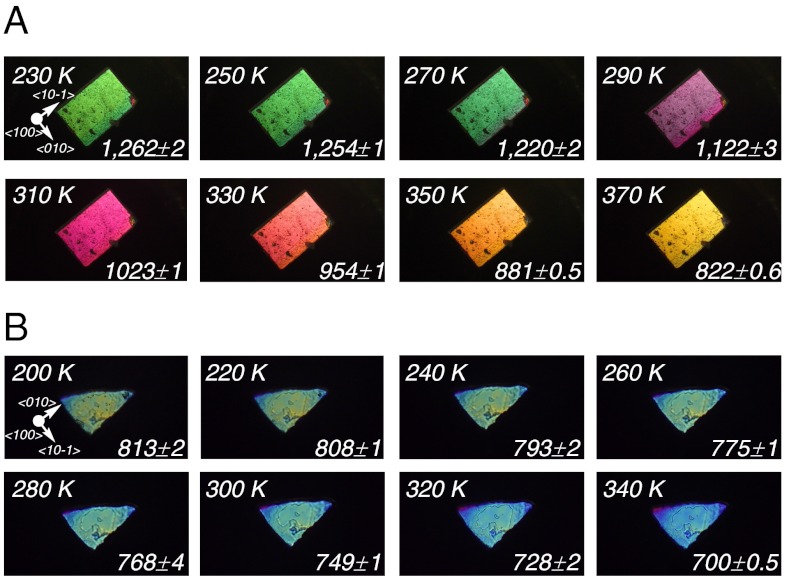
We have used these temperature-dependent structural features of the crystal that are dependent on the dynamics of 1, such as the disorder orientation of the phenylene moiety and deformation of the crystal lattice, to control the birefringence (Δn) of the crystal by changing the temperature (26). A photograph of a single crystal of 1 is shown in Fig. 5A. The widest face of the crystal corresponds to the {100} face, as determined by X-ray diffraction. On viewing this crystal face, we can observe the molecular aggregation along the rotational axis shown in Fig. 3. Fig. 5A shows photographs of the {100} face of a single crystal of 1 that has been irradiated with polarized white light at different temperatures. Interference colors due to retardation were observed. At temperatures above 260 K, the colors changed due to the heating, whereas they did not change at temperatures below 260 K. Fig. 5B shows photographs of the {100} face of a single crystal of 2 that has been irradiated with polarized white light at different temperatures. In the case of the single crystal of 2, a continuous change in the interference colors due to the thermal expansion resulting from the heating was observed.

Fig. 5.

Photographs of the single crystals on the {100} face irradiated with polarized white light [retardations (nm) are shown]: (A) 1, (B) 2.

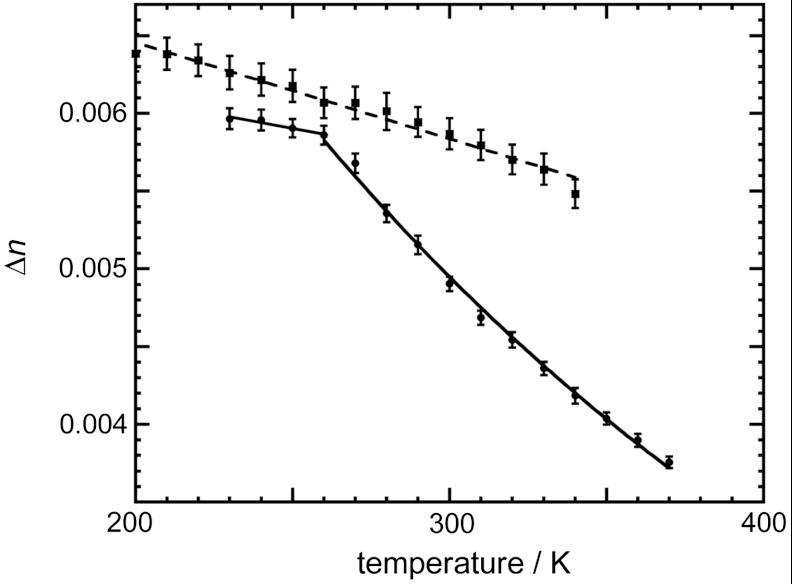
The birefringence (Δn) can be analyzed by measuring the changes in the polarization of light passing through the crystal, i.e., by measuring the retardation. Fig. 6 shows a plot of Δn versus temperature. The Δn of 1 is almost constant for temperatures below 260 K, because the phenylene moiety of 1 undergoes a complete 180° flip. On the other hand, the Δn of 1 decreases markedly as the temperature rises above 260 K, because the phenylene moiety exhibits a 180° flip with angular displacement and deformation of the cage. On the {100} face of the crystal of 1, the fast optical axis is observed to be perpendicular to the slow axis, which is parallel to the 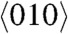, whose direction is identical to the b axis of the cell (Fig. 3). Due to the unidirectional orientation of the phenylene moiety in the crystal, the birefringence of the {100} face, which is the difference between the two refraction indices of the slow and fast optical axes, is relatively large at temperatures below 260 K. At temperatures above 260 K, the orientation of the phenylene moiety is disordered in the crystal. The polarization of light is affected by the randomly oriented benzene molecules that correspond to snapshot of the benzene rotation inside the crystal during passing through the crystal, because the number of gyrotop layers in a crystal are about 500,000 per 100 μm thickness of the crystal. The Δn of the {100} face becomes small at temperatures above 260 K.

Fig. 6.

Plot of Δn versus temperature of the crystal of 1 (●) and 2 (▪) on the {100} face.

These dynamic and optical properties are completely reversible in the temperature range from 230 to 370 K at least. These results are an application of the states of dynamic equilibrium of molecular motions in a crystal causing a change in the birefringence because of a structural change.

Materials and Methods

Synthesis of the Molecular Gyrotops 1 and 2.

To a solution of dichloromethane (500 mL) in the presence of Grubbs’ catalyst, first generation (0.05 g, 0.06 mmol), a dichloromethane solution (200 mL) of 3 (1.42 g, 1.78 mmol) was added dropwise with stirring over 12 h. The mixture was further stirred for another 12 h. The volatile materials were removed in vacuo, and the benzene soluble fraction was treated with flash column chromatography (silica gel, benzene) to remove the metal catalysts. Then, 3 atm of hydrogen gas was introduced into a toluene (5 mL) solution of the reaction mixture in the presence of 10% Pd/C (0.03 g) in an autoclave, and the mixture was allowed to stand for 72 h at 60 °C. After the excess H₂ gas had been released, the mixture was filtered to remove the Pd/C. The volatile materials were removed in vacuo. Using gel permeation chromatography with chloroform as the solvent, fractions containing 1 were collected. Pure compound 1 (310 mg, 0.43 mmol, 24% yield) was obtained by recrystallization of a tetrahydrofuran/methanol (4∶1) solution. Compound 1: colorless crystals, mp 254–256 °C; ¹H NMR (CDCl₃, 400 MHz): δ 0.71–0.78 (multiplet, 12H, Si-CH₂-), 1.18–1.40 (multiplet, 72H), 7.50 (singlet, 4H, aromatic CH); ¹³C NMR (CDCl₃, 100 MHz): δ 13.05, 23.07, 27.72, 27.80, 28.45, 28.85, 32.56, and 133.54 (aromatic CH), 137.77 (SiC); ²⁹Si NMR (CDCl₃, 79.5 MHz): δ -2.40; UV-visible (hexane solution): λ_max/nm(ε) 231 (14,900), 270 (570), 265 (570); Anal. Calcd. for C₄₈H₈₈Si₂: C, 79.92; H, 12.31. Found: C, 79.69; H, 11.97. The Xylene-bridged compound 2 was synthesized from 4 (1.0 g, 1.21 mmol) by the same procedure as that used for the synthesis of 1 and pure 2 (80 mg, 0.11 mmol, 9.7% yield), was obtained by recrystallization. Colorless crystals; mp 129.0–129.6 °C; ¹H NMR (CDCl₃, 400 MHz): δ 0.75–0.79 (multiplet, 12H, Si-CH₂-), 1.10–1.32 (multiplet, 72H), 2.35 (singlet, 6H, Me), 7.12 (singlet, 2H, C₆H₂); ¹³C NMR (CDCl₃, 100 MHz): δ 13.45, 22.51 (Me), 22.97, 27.58, 27.68, 28.39, 28.85, 32.19, 135.51 (CMe), 136.74 (aromatic CH), 139.11 (SiC); ²⁹Si NMR (CDCl₃, 79.5 MHz): δ -0.78; Anal. Calcd. for C₅₀H₉₂Si₂: C, 80.13; H, 12.37. Found: C, 79.86; H, 12.72.

X-ray Crystallographic Analysis of the Molecular Gyrotops 1 and 2.

The diffraction data of 1 and 2 were collected on a Bruker APEX-II CCD system using graphite-monochromatized Mo K-α radiation (λ = 0.71069 Å). Crystallographic data for 1 (260 K): monoclinic, C2/c, a = 25.432(3) Å, b = 11.885(1) Å, c = 18.809(2) Å, β = 120.222(1)°, V = 4912(1) Å³, R1 = 0.0682 (I > 2σI), wR2 = 0.2455 (all data). Crystallographic data for 2 (150 K): monoclinic, C2/c, a = 24.099(3) Å, b = 11.823(1) Å, c = 18.678(2) Å, β = 113.717 (1)°, V = 4872.3(9) Å³, R1 = 0.0605 (I > 2σI), wR2 = 0.1905 (all data). The crystallographic data for 1 and 2 were deposited in the Cambridge Crystallographic Database Centre (CCDC-830990 for 1, CCDC-868972 for 2). The synchrotron X-ray powder diffraction studies of the molecular gyrotop 1 were performed at the beam-line BL19B2 in SPring-8 with the approval of the Japan Synchrotron Radiation Research Institute (Proposal No. 2010A1771). Further details are given in SI Appendix.

Solid-State ²H NMR Analysis of the Molecular Gyrotop 1 and 2.

The temperature-dependent solid-state ²H NMR spectra were recorded on Varian Unity 500 spectrometers using a quadrupolar echo pulse sequence (d1-90° pulse-τ₁-90° pulse-τ₂ free induction decay; 90 pulse = 4.2 μs, τ₁ = 30 μs, τ₂ = 20 μs, d1 = 20 s). Simulations of the ²H NMR spectra were carried out using NMR-WEBLAB (23). The following parameters were used for the simulations: quadrupolar coupling constant q_cc = 130 kHz, asymmetry parameter η = 0, line broadening = 3 kHz. The temperature dependence of the spin-lattice relaxation time (T₁) in the ²H NMR spectra was recorded using an inversion-recovery quadrupolar echo pulse sequence (d1-180° pulse-d2-90° pulse-τ₁-90° pulse-τ₂ free induction decay; 90-pulse = 4.2 μs, τ₁ = 30 μs, τ₂, d1 = 5–3 s, d2 were varied) and standard T₁ analysis software.

Optical Properties of the Molecular Gyrotops 1 and 2.

Retardations were observed using a polarized light microscope (Olympus BX51) equipped with a Berek compensator and monochromatic light at 546 nm generated by a color filter. The birefringence Δn) was calculated from the retardation/thickness. Further details are given in SI Appendix.