Speed, pacing strategy and aerodynamic drafting in Thoroughbred horse racing

Abstract

Choice of pacing strategy and the benefit of aerodynamic drafting are thought to be key determinants of racing performance. These effects have largely been analysed without reference to final outcome, in small datasets with low temporal resolution, and a focus on human swimming, cycling and running. Here, we determined the position and speed of 44 803 racehorses, once per second, in 3 357 races ranging in length from 1006 to 4225 m (50.9–292.9 seconds duration) using a validated radio tracking system. We find that aerodynamic drafting has a marked effect on horse performance, and hence racing outcome. Furthermore, we demonstrate that race length-dependent pacing strategies are correlated with the fastest racing times, with some horses reaching a maximum speed in excess of 19 m s⁻¹. The higher speeds seen with certain pacing strategies may arise due to the nature of pack racing itself, or may be a reflection of individual capabilities, that is, corresponding to horses that perform well in roles suited to their ‘front-running’ or ‘chaser’ personality traits.

1. Introduction

How should an athlete regulate their speed over the course of a race to maximize their performance? The distribution of work, or energy expenditure, over the course of an athletic event is termed a ‘pacing strategy,’ and is understood to be a key determinant of overall performance [1]. In short events (up to 30 s duration), fast start, ‘all out’ strategies are thought to be optimal; in middle distance events (greater than 2 minutes), constant pace may be optimal; finally, in endurance racing, even pace or perhaps a gradual decline in speed throughout the race is thought to be the best strategy [1], although in human racing a spurt of speed at the end of races is often observed [2,3]. The rate and capacity limits of various physiological systems are thought to contribute to these differences, in conjunction with central and peripheral control of exercise intensity that is thought to maintain homeostasis [4]. Little is known, however, as to the specific physiological, cognitive and environmental factors that limit maximal athletic performance [5]. Although horse racing is not a ‘natural’ behaviour, it provides a context for the collective motion of quadrupeds in which motivation for maximum performance is relatively well controlled for; it thus represents an opportunity to uncover the mechanisms that lead to variation in individual or collective performance, and to seek general principles of performance through comparison with other species.

Two environmental factors that are critical to performance are the actions of other competitors [6] and the effects of resistive forces (air resistance or drag in water) [7]. In head-to-head racing, athletes need only perform marginally better than their competitors. Additionally, athletes may become aware that they are falling behind the leader. Both of these influences may modulate the choice of pacing strategy and the perceived exertion required; either may influence the onset of fatigue.

Drafting can mitigate the second environmental factor, the need to overcome resistive forces owing to moving through air or water. Drafting refers to moving close behind another competitor to reduce the work required to overcome drag (pushing fluid—either air or water, out of the way). The benefit of drafting has been estimated to be as high as 35 per cent in cycling [6] and competitively significant in running [8] and open water swimming [7].

2. Material and methods

The raw data consisted of the two-dimensional position and speed of each horse sampled at 0.8 Hz. These data were obtained from a radio-frequency tracking system deployed by TurfTrax Limited (Salisbury, UK), during the UK Thoroughbred racing seasons of 2005–2007. Data were from 10 race courses: Doncaster, Goodwood, Kempton Park, Lingfield Park, Newbury, Newmarket, Sandown Park, Southwell, Wolverhampton and York. The tracking system works by placing tags in the number cloth of each horse that emit radio-frequency chirps that are picked up by base station antennae surrounding the racecourse. Information about received chirps is relayed to a computer, where a real-time processing engine produces a live feed of position and speed of each horse. We validated the accuracy of this system [9], finding it to be accurate to within ± 0.38 m (interquartile range; IQR) in position and to within ± 0.15 m s⁻¹ in speed.

We computed a pack break point, the point at which competitor speeds diverge above a fixed threshold, in the following manner. For each horse that finished, the speed relative to the pack average was computed as a function of distance remaining to finish, at 15 m intervals. The standard deviation across horses of these relative speeds, as a function of distance remaining, was then computed for each race. We defined the break point for each race as the distance remaining at which, starting from the end of the race and moving backwards in time (increasing distance remaining), the slope of the standard deviation of horse relative speeds rose above −0.003 s⁻¹ (figure 1b). This corresponds to the standard deviation of relative speed becoming relatively flat. Races in which the slope of the standard deviation began above the threshold of −0.003 s⁻¹ (650 of 4799 races, or 14%) were excluded.

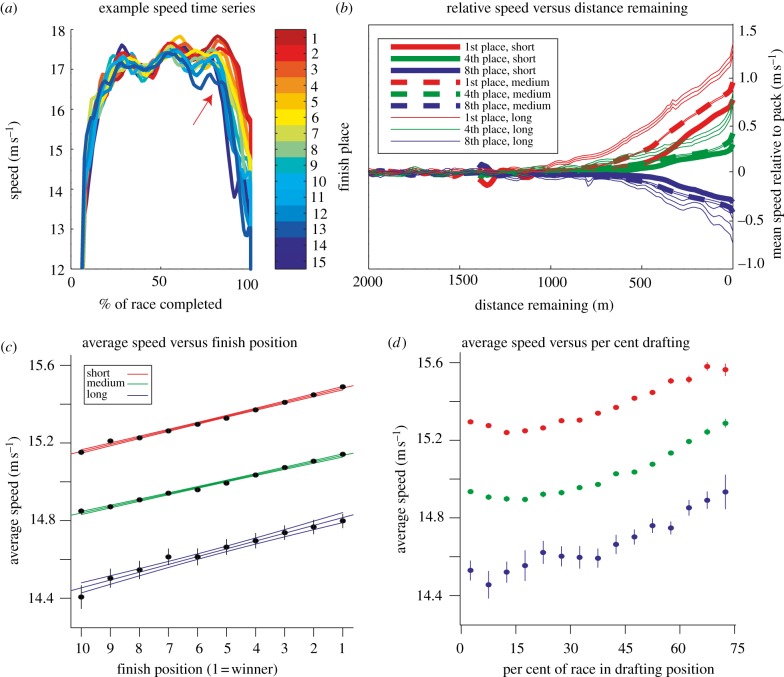
Figure 1.

On average, horse races are decided in the last 500 m of the race, at which point the speed of competitors diverges (red arrow in (a), showing data from a single race, and (b): mean ± s.e.m. of speed of horses relative to pack average, across races by finish place and race length). These were computed for each combination of three finish places (1st, 4th and 8th) and three race distances (short: distance less than 1445 m or seven furlongs and 40 yards; medium: race distance greater than 1445 m and less than 2890 m, or 1 mile six furlongs and 80 yards; long: race distance greater than 2890 m). The n value for these bins varied between a minimum of 119 individual horse starts to 2342 horse starts, with fewer starts at large distances remaining in the long races. Small margins in average speed (0.13 m s⁻¹ or 0.9% between 1st and 5th place, (c) make the difference in winning, and horses that spend time drafting behind other competitors go significantly faster (d).

We estimated the mechanical power required to overcome drag as follows. The Reynolds number for the horse and jockey, taking the density and dynamic viscosity of air to be ρ = 1.29 kg m⁻³ and μ = 17.4 × 10⁻⁶ Pascal-seconds, respectively, a characteristic length of L = 2 m, and a velocity of v = 18 m s⁻¹, is:

This number is much greater than 1000, and thus the quadratic drag equation provides an appropriate estimate of the drag force. The drag force magnitude is thus estimated to be:

Considering the horse and jockey to be a bluff body (and in comparison with a bicyclist with known C_d of 0.9), we choose a drag coefficient C_d of 1.0, and frontal area A equal to 1 m². The power required to overcome this force is therefore:

At 18 m s⁻¹ (cf. figure 1a), this results in a drag force of 209 N, requiring a mechanical power of 3.8 kW. The total mechanical power of horse galloping has been estimated as 22.5 kW [10], and therefore a rough estimate based on these figures is that overcoming drag accounts for 17 per cent of total mechanical power.

To determine the significance of drafting in horse racing, we examined how average speed depends on the percentage of the race that a horse spends ‘covered up,’ or directly behind another horse (figure 1d and electronic supplementary material, movie S1). We defined being in a drafting position as when a competitor's position (i) falls within 10°  to either side of the forward velocity vector of a horse, and (ii) is within 5 m distance of that horse. This cone of acceptance is shown as V-shaped indicators in the electronic supplementary material, movie S1. As the TurfTrax tags were placed in the saddle cloth roughly at the fore/aft midline of the horse, this corresponds to a nose to tail distance of 2.5 m or less, which is approximately one horse length. By comparison, cyclists gain a reduction in cost of about 25 per cent at 2 m wheel spacing, which is slightly more than one bicycle length (1.78 m). Pack dynamics and the computation of drafting position are illustrated in the electronic supplementary material, movie S1.

Linear mixed-effects models (LMMs) were fit to the quantities computed above using the nlme package in R (tables 1 and 2). Distinct race classes (referring to the standard of the horses) were coded as integer values ranging from 1 to 10 (1 = highest: ‘class 1 group 1’, 10 = lowest: ‘class 7’; British Horseracing Authority, www.britishhorseracing.com), and entered as a random effect, as was race course. Data are available upon request.

Table 1.

A linear mixed-effects model analysis of correlation between the average speed achieved during the race and the slope of speed in the middle and at the end of race, controlling for race distance, race course and race class. (The table shows parameter estimates (estimate), standard error (s.e.) and associated test statistic (F-statistic) for fixed effects, and the standard deviation of estimated random effects for random terms (estimate). Average speed during a race versus slope of speed in the middle and at the end of the race (n = 48 426). ***p < 0.001.)

model term	estimate (s.e.)	d.f.	F-statistic
race distance	−4.27 × 10−4 (3.1 × 10−6)	1	18795.78***
middle slope	−1.508 (0.101)	1	2872.62***
end slope × middle slope	−17.941 (0.902)	1	395.634***
end slope	0.922 (0.036)	1	184.54***
course (random term)	0.294
class (random term)	0.273
constant	16.026 (0.096)

Table 2.

A linear mixed-effects model analysis of correlation between the average speed achieved during the race and the per cent of the race spent in a drafting position, controlling for race distance, race course and race class. (The table shows parameter estimates (estimate), standard error (s.e.) and associated test statistic (F-statistic) for fixed effects, and the standard deviation of estimated random effects for random terms (estimate). Average speed versus per cent of race spent in a drafting position (n = 48 426). **p = 0.001. ***p < 0.001.)

model term	estimate (s.e.)	d.f.	F-statistic
race distance	−4.17 × 10−4 (3.0 × 10−6)	1	20252.07***
per cent drafting	0.158 (0.008)	1	10.78**
course (random term)	0.335
class (random term)	0.267
constant	16.026 (0.096)

3. Results

There was a characteristic break point for the field (figure 1a,b; 478 ± 220 m distance remaining, mean ± s.d., n = 3357 races). The break distance varied slightly with race length, happening earlier in longer races (figure 1b; cf short, medium and long races). Ninety two per cent of horses slowed after the break point (44 803 individual horse starts; linear regression, p < 0.05), and the median speed decline (−0.080 ± 0.062 m s⁻², ± IQR) was 8.6 times greater than during the middle of the race (from 20 s after start until 20 s before finishing; −0.0093 ± 0.040 m s⁻²,± IQR). The break point did not coincide with the position of the final bend on the course (ρ_s = −0.09, p = 0.43, n = 77 groups of unique race course/distance combinations).

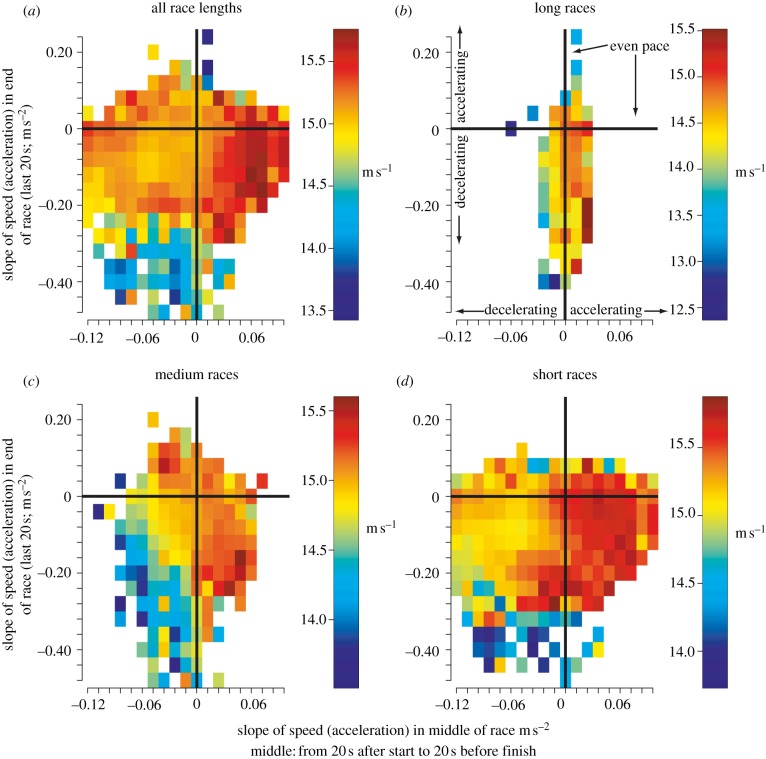
Across all races, the fastest average speeds were achieved by increasing speed during the middle and decreasing speed at the end of the race (figure 2a and table 1; LMM: middle slope × end slope effect size (s.e.) = −17.94 (0.90), p < 0.001, n = 48 426). However, in middle distance races, high average speeds were also obtained with level or slowing speeds in the middle of the race, following by increasing speed at the end of the race (figure 2c). Across all distances, the 98th percentile of maximum speed was 19.05 m s⁻¹.

Figure 2.

The average speed over the entire race was computed for each individual horse start in the dataset. This figure displays the median of these average speeds (colour) in bins determined by the slope of speed in the middle of the race (x-axis, positive = acceleration; from 20 s after start until 20 s before the end of the race; m s⁻²) and slope of speed at the end of the race (y-axis; last 20 s of the race; m s⁻²). Similar plots are shown for (a) all race distances, (b) for long races only, (c) medium races only and (d) short races only (distances as defined in figure 1). Generally, the fastest speeds are correlated with even or increasing speed during the middle of the race (x-axis greater than zero), and decreasing speed at the end of the race (y-axis less than zero). An alternate strategy of even or increasing speed at the end of race (‘chaser’ horses; y-axis greater than zero) can also produce high average speeds; with speeds closest to the decreasing speed strategy seen in medium distance races (c, middle slope approx. −0.04, end slope approx. 0.07 m s⁻²).

Average speed exhibited J-shaped dependence on per cent race spent in a drafting position (figure 1d). After controlling for race distance, course and class, average speed was found to increase with percentage of race spent in a drafting position (table 2: LMM; coefficient = 0.158 ± 0.008, p < 0.001, n = 48 426).

4. Discussion

The divergence of competitor speed at the end of a race emphasizes the head-to-head rather than time trial nature of horse racing. The horse that slows down the least, wins. In an attempt to keep abreast of faster competitors, less capable individuals reach a point of extreme fatigue before the end of a race, and show a dramatic fall off in speed (figure 1a).

Faster competitors exhibited race distance-dependent tactics (figure 2). This mix of strategies could be linked to individual horse personalities; horses are colloquially referred to as performing best in ‘front-runner’, ‘mid-pack’ or ‘chaser’ roles. These roles may be akin to variation in the shy/bold continuum of animal personality studied by behavioural ecologists [11]. Alternatively, pack-blocking effects may be significant: front-runners are less constrained by the pack; chaser horses, with the reserves to move up through the pack, may more easily do so at the end of the race when the pack has dispersed.

Racing competitors gain an advantage from drafting. The metabolic power required to overcome aerodynamic drag is significant (17% of total mechanical power), and the difference in average speed between first place and fifth place (no prize money) is about 2 per cent (figure 1c). This could be accounted for by a 13 per cent reduction in aerodynamic drag. For a horse that drafts for 75 per cent of a race, this effect is worth three to four finish places (figure 1c).