Multilevel complexity of calcium signaling: Modeling angiogenesis

Abstract

Intracellular calcium signaling is a universal, evolutionary conserved and versatile regulator of cell biochemistry. The complexity of calcium signaling and related cell machinery can be investigated by the use of experimental strategies, as well as by computational approaches. Vascular endothelium is a fascinating model to study the specific properties and roles of calcium signals at multiple biological levels. During the past 20 years, live cell imaging, patch clamp and other techniques have allowed us to detect and interfere with calcium signaling in endothelial cells (ECs), providing a huge amount of information on the regulation of vascularization (angiogenesis) in normal and tumoral tissues. These data range from the spatiotemporal dynamics of calcium within different cell microcompartments to those in entire multicellular and organized EC networks. Beside experimental strategies, in silico endothelial models, specifically designed for simulating calcium signaling, are contributing to our knowledge of vascular physiology and pathology. They help to investigate and predict the quantitative features of proangiogenic events moving through subcellular, cellular and supracellular levels. This review focuses on some recent developments of computational approaches for proangiogenic endothelial calcium signaling. In particular, we discuss the creation of hybrid simulation environments, which combine and integrate discrete Cellular Potts Models. They are able to capture the phenomenological mechanisms of cell morphological reorganization, migration, and intercellular adhesion, with single-cell spatiotemporal models, based on reaction-diffusion equations that describe the agonist-induced intracellular calcium events.

INTRODUCTION

The term complexity is currently used in biology and spans different levels, from molecular to multicellular. It refers to some intrinsic peculiar properties of a system, that include the involvement of many components, their nonlinear interactions and physicochemical spatial compartmentalization[1]. Intracellular pathways underlying the cell response to external stimuli are a paradigm of complexity. Calcium signaling, in particular, is a universal and evolutionary conserved regulator of such a network of biochemical reactions. Its complex features are discussed in detail in several reviews[2-4].

Among the biological processes regulated by calcium, tissue vascularization (broadly speaking angiogenesis) is a morphogenetic event occurring under physiological conditions as well as, in an altered way, in cancer and other diseases[5]. The role of calcium in angiogenic progression can be investigated at different levels, thus providing an excellent tool to test the heuristic power of experimental and computational approaches to a complex system.

ENDOTHELIAL CALCIUM SIGNALING AT SUBCELLULAR AND CELLULAR LEVELS

Several extracellular agonists, including growth factors and vasoactive compounds, trigger free intracellular calcium concentration (Ca_i) increases in endothelial cells (ECs), with variable amplitude and time course. Ca_i signals can be “spike-like” (duration of several seconds), slow and long-lasting (duration up to several minutes), oscillating, or a combination of these. The relationship between the intensity of the stimulus and the amplitude of Ca_i signaling can be linear or not, depending on the relevance of positive feedbacks underlying the genesis of the process. The spatiotemporal signature of Ca_i waves conveys information through the regulation of specific downstream targets[6-8].

Ca_i increase is due to the opening of variably selective calcium channels located in the plasma membrane and in the membranes of cell organelles (calcium stores). They are formed by large superfamilies of proteins, often compartmentalized in caveolae, lipid rafts and vesicles[9]. As a consequence, endothelial Ca_i signals are organized in spatiotemporal patterns much more complex than those described in the recent past from the analysis of the bulk single cell level. A lot of evidence has been accumulated about the existence of “calcium microdomains”[3,10]. They are classified on the basis of their different spatial restriction, time course and amplitude, thus contributing to the specificity of cell response[3,10]. Several reports on different cell types have provided evidence that Ca_i spikes differentially activate downstream targets depending on the spatial location of the underlying Ca²⁺ channels[6-8,11-13]. Selective location of calcium sensors (mainly calmodulin and related kinases) near different sources of Ca²⁺ provides an effective mechanism for generating highly specific responses. Specific recruitment of key signaling enzymes [e.g., endothelial NO synthase (eNOS)] and transcription factors (e.g., c-AMP response element binding, and nuclear factor of activated T cells) has been reported following calcium signals of given spatiotemporal profile. In migrating bovine aortic ECs, calcium signals activated by ATP originate from polarized caveolae[14-16]. Moreover it has been reported that eNOS and protein kinase C are sensitive to subcortical waves, spatially distinct from classical endothelial cytosolic Ca_i waves, whereas phospholipase A2 is unaffected[17].

The crucial role of Ca_i signaling in the control of EC functions and angiogenesis under physiological and pathological conditions is now well accepted[18]. Our group and others have reported some biophysical properties of calcium currents activated by proangiogenic factors in normal as well as in tumor-derived ECs[19-26]. Proangiogenic calcium channels are often nonselective, being permeable also to Na⁺ and K⁺ ions. They are modulated by the interplay of intracellular messengers, including arachidonic acid (AA), NO and H₂S[22-24,26,27]. Members of the transient receptor potential protein family (including TRPC1, TRC6 and TRPV4) and Orai1 are some of the channels reported to mediate calcium entry[18,20,23,25,27-31].

Stimulation of bovine aortic ECs with promigratory/tubulogenic concentrations of AA or NO triggers peripheral and localized Ca_i signals[11]. They decay in space and usually fail to propagate into the perinuclear and nuclear regions. Accordingly to the aforementioned literature, we suggest that the peculiar spatiotemporal dynamics of endothelial Ca_i signals could recruit differential patterns of calcium-dependent proteins and genes during the multistepped pathophysiological process of angiogenesis.

ENDOTHELIAL CALCIUM SIGNALING AT THE MULTICELLULAR LEVEL: THE (LARGELY INCOMPLETE) LINK WITH ANGIOGENESIS

ECs organize in tubules when cultured in a 3D Matrigel. This configuration is often used for in vitro tubulogenesis assays. Although very far from the high complexity of vasculature in vivo, this simple condition is useful for the analysis of EC migration, organization and maturation during vessel formation. In a previous study, we showed that AA is able to enhance tumor-derived ECs (e.g., breast carcinoma ECs, BTECs) migration and tubulogenesis in vitro[21]. Notably, AA-induced Ca_i signals, sensitive to the antiangiogenic and antitumor compound carboxyamidotriazole, are specifically detected in the early stages of tubule organization and are subsequently downregulated at more mature stages. This is a fascinating feature that deserves consideration from both the experimental and conceptual points of view. At the cellular level, Ca_i signaling evoked by AA promotes cell migration: this event concurs with the formation of endothelial tubules; a higher multicellular level of organization (bottom-up effect). Once the mature tubule network is stabilized, the ability of the single cells to respond to AA decreases in a sort of a top-down feedback from the higher to the lower level (Figure 1). On the other hand, the response to another vasoactive compound, ATP, is unaffected, suggesting a high specificity of the negative feedback[21].

Figure 1.

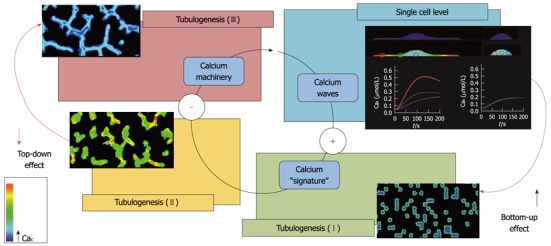
Scheme showing the relationship between calcium signaling and endothelial cell migration and organization in tubules in vitro (tubulogenesis). Proangiogenic calcium signature triggers calcium-dependent machinery in single sparse endothelial cells (ECs). Calcium signature depends on the structural and functional state of any single cell (for the effect of cell shape, see 40: this event promotes migration during the early tubulogenic phases (tubulogenesis I and II; bottom-up effect). In mature tubules (tubulogenesis III) proangiogenic calcium machinery in ECs is inhibited (top-down effect).

Interestingly, the effects of AA on BTECs seem to be different from those observed in normal human microvascular ECs, in which the fatty acid fails to promote migration and evokes smaller calcium signals[24,26]. The functional differences between normal and tumor-derived ECs, in terms of cell signaling and in particular of calcium signature, are intriguing and deserve more in-depth investigation.

COMPUTATIONAL MODELING OF ANGIOGENESIS AND RELATED CALCIUM SIGNALS: BEYOND VALIDATION OF EXPERIMENTAL RESULTS

Modeling proangiogenic calcium signaling at the single-cell level

As previously mentioned, at the single-cell level, the generation of calcium microdomains and global calcium waves can be ascribed to the interplay of several factors: the highly variable cell morphology and the specific distribution of pumps, channels, and buffers (e.g., proteins, mitochondria), with the typical compartmentalization in lipidic rafts, caveolae and supermolecular complexes (signalplexes or signalosomes)[11,15,16,32,33]. However, the most common laboratory-based techniques used to investigate calcium events, despite providing an impressive amount of data, are affected by many drawbacks and alter the physiology of the cell. Calcium imaging is indeed obtained by the use of fluorescent probes that act as calcium buffers and therefore perturb the physiological intracellular homeostasis of the ion. Also, all the typical limitations intrinsic to fluorescence-based techniques, sensitivity to other ions and pH, and bleaching, must be considered. Moreover, protein-based calcium measures require the cell to be transfected. The patch clamp technique is powerful for investigating biophysical quantitative properties of calcium currents and channels, but intracellular solutions are significantly altered, mostly in whole cell configuration. In addition, single-cell electrophysiological measurements are performed in a limited number of cells[34]. All these limitations can be overcome by computational (in silico) approaches, which can be also used, in a predictive manner, to determine the pitfalls of experimental manipulations, thus providing a useful guide for new in vitro realizations.

In the past 20 years, an increasing number of mathematical models have been used for the study of calcium signaling in different cell types, including ECs[35-37]. They are based on continuous reaction-diffusion (RD) systems, in which the kinetics of channels and pumps (located in the plasma membrane and in specific intracellular compartments such as the endoplasmic reticulum) are typically described by saturating Michaelis-Menten functions, and the propagation of the ion is mainly determined by its diffusion coefficient.

We have recently proposed a similar continuous model, based on RD equations and implemented on Virtual Cell software (already successfully applied by other authors for other cell types[38-40]), for the specific analysis of proangiogenic Ca_i signals in a single EC, stimulated by micromolar concentrations of vascular endothelial growth factor (VEGF) and regulated by the interplay between AA and NO[41]. Beside reproducing Ca_i events in close comparison with the results previously obtained with fluorescent probes[11,27], the mathematical approach has been able to focus on the influence of the spatial localization of the complex and variable cell morphology, highly diverging from a regular spherical shape. We have designed realistic 3D reconstructions of the EC starting from the relative confocal images, that is, with and without filopodium-like processes, and have shown how the maximal accumulation of the ion is restricted in the most peripheral and flat regions of the cell. Moreover, our approach has provided additional insights into the role played in calcium machinery of factors that are difficult or even impossible to control in vitro, such as the concentration and distribution of channels or intracellular buffers. In summary, the use of the proposed computational method has been able to analyze relevant biochemical mechanisms and parameters underlying proangiogenic calcium signature. However, further experimental data are now required to upgrade and improve the model, to reduce the number of assumptions and increase the quantitative constraints. Indeed, this continuous feedback and feedforward between in vitro and in silico approaches is the core principle of a systems biology approach, applied with an increasing frequency and success in biology.

Towards an inclusive modeling approach for validating the role of calcium signaling in angiogenesis progression

The intrinsic multilevel nature of angiogenic progression and the related calcium signaling requires us to take into account factors characteristic of different spatiotemporal levels of organization, which range from selected biophysical properties of the entire EC population to specific intracellular biochemical dynamics. An accurate study of this complex flow of information makes evident the need for multilevel research and brings many challenging questions, which can be more efficiently addressed by collaboration with applied mathematics.

The literature on mathematical models of vascular progression is mainly focused on the reproduction and analysis of tubulogenic assays and is partitioned into two main streams, according to the type of approach used, that is, continuous or discrete. From a macroscopic point of view, continuous techniques represent the EC population as a density, which satisfies a set of balance laws and/or diffusion equations. In particular, these methods typically assume that the key mechanisms of tubule formation are: (1) a chemotactic force generated by extracellular gradients of specific growth factors (e.g., VEGF, fibroblast growth factor); and (2) interaction with the matrix substrate, considered as a continuum body whose stretch influences cell movement and stabilization[42-44]. These approaches reproduce in detail the early phases of the vasculogenic process (i.e., before tubule maturation), predicting a characteristic length of the capillary-like network, which is dictated by the diffusion coefficient of the exogenous angiogenic factor, in good agreement with the relative experimental observation. However, such continuous techniques overlook the behavior of single cells and fail to describe their mutual and local interactions. They may therefore be unsatisfactory because, to deal with angiogenic progression, what occurs at the level of the single cell is crucial. Moreover, continuous models are usually unable to describe intracellular biochemical dynamics, including calcium signaling.

The second type of models reproducing vessel formation are the discrete techniques, widely known as individual cell-based models (IBMs) or cellular automata. They approach the problem with a mesoscopic-phenomenological point of view, preserving the identity of individual ECs. ECs are represented as one or a set of spatially-extended units (defined according to some underlying discretization of the simulation domain, which can be either regular, such as square or cubic grids, or irregular, as in the case of Voronoi tessellations), with rules that describe their movements and interactions. Compared to continuous methods, IBMs can more naturally capture detailed biophysical properties of single vascular cells, such as morphological changes or intrinsic motility, and are also able to handle local adhesive interactions. Among discrete models, one of the most used is the Cellular Potts Model (CPM), a grid-based Monte Carlo technique that follows an energy minimization philosophy[45-47]. Published CPMs have shown that relatively simple cell-level mechanisms, such as adhesion[48], elongation[49], and contact-inhibited chemotaxis[50], are sufficient to obtain the organization of a dispersed population of vascular cells into a 2D network. However, those do not include molecular-level processes, and thus neglect the importance of calcium signaling that underlies the phenomenology of ECs.

The limitations of both purely continuous and discrete models can be overcome by creating hybrid computational frameworks, which are able to span the entire spatiotemporal level of the process with a sufficient degree of accuracy, offering the advantages brought by the different methods. We have recently constructed such a multilevel approach by nesting an adapted version of the above-mentioned single-cell model in a CPM[51,52]. As a distinct feature of the mathematical environment, the subcellular biochemistry, described by the continuous subcellular model, has been coordinated to deliver realistically cell biophysical properties (i.e., motility, chemical sensitivity and compressibility) and behavior (i.e., shape reorganization, chemotactic and persistent migration, and intercellular adhesion), described by the discrete CPM, ultimately mediating the overall formation of the capillary-like structure (Figure 2).

Figure 2.

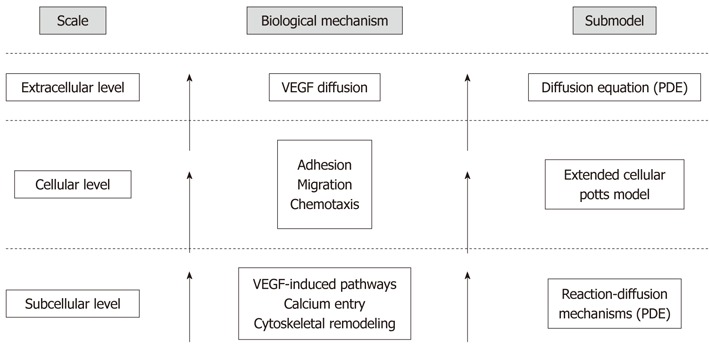
Hierarchy of scales and environments, with the corresponding biological mechanisms and modeling approaches, taken into account in the proposed model of tubulogenesis. Our theoretical method spans the multiple levels involved in the process: it incorporates a continuous model of the specific agonist-induced, calcium-mediated intracellular cascades in a discrete cellular potts model, which represents the phenomenological evolution of the endothelial cell (EC) population. In particular, the microscopic dynamics alter the mesoscopic biophysical properties of the ECs, such as motility, compressibility, and chemotactic strength, affecting their behavior and, ultimately, the overall tubule formation. The different spatiotemporal levels, integrated in a hybrid framework, therefore directly affect each other and feedback over the whole simulation. VEGF: Vascular endothelial growth factor.

The resulting hybrid model has reproduced with remarkable accuracy the kinetics and temporal dynamics of the pattern organization, as well as its final configuration characterized by well-defined topological features (i.e., intercapillary distances, branch length and width). Furthermore, the computational analysis has followed the proangiogenic calcium events during the entire process: evoked in the early phases, when the cell population is still not connected in a mature network, they are significantly downregulated in the later phases. Indeed, such a peculiar temporal evolution of responses may play a specific role in transducing information during the different stages of the process, being likely associated with differential membrane channel functionalities, at either the gene or the protein level. Finally, the computational model has allowed us to test specific antiangiogenic therapies. In particular, the efficacy of strategies that are currently in use (such as interference with VEGF machinery) or in trials (blocking of calcium entry) has been confirmed. At the same time, the model has been able to predict the potential success of biomedical interventions inhibiting the mechanisms of cell cytoskeletal remodeling, chemical sensitivity, and adhesive capability. It is useful to emphasize that the large combinatorial space of possible therapies would have been unfeasible to search using only laboratory-based methods, but it has been efficiently analyzed by a mathematical system, confirming the usefulness of close collaboration between experimental and theoretical researchers.

CONCLUSION

Vascular endothelium is a suitable model to investigate the specific properties and functions of Ca_i signals at different biological levels. The combination of experimental strategies and specific in silico models can improve our knowledge of the role of calcium signaling in vascular physiology and pathology. Mathematical models provide a quantitative validation of experimental data, giving the opportunity to predict experimental results in a faster and less expensive way. Furthermore, and more generally, computational modeling represents a useful tool to unveil the complex crosstalk between subcellular, cellular and supracellular levels in vascular biology.

ACKNOWLEDGMENTS

We would like to thank Dr. Daniele Avanzato for graphical assistance.