Abdicating power for control: a precision timing strategy to modulate function of flight power muscles

Abstract

Muscles driving rhythmic locomotion typically show strong dependence of power on the timing or phase of activation. This is particularly true in insects' main flight muscles, canonical examples of muscles thought to have a dedicated power function. However, in the moth (Manduca sexta), these muscles normally activate at a phase where the instantaneous slope of the power–phase curve is steep and well below maximum power. We provide four lines of evidence demonstrating that, contrary to the current paradigm, the moth's nervous system establishes significant control authority in these muscles through precise timing modulation: (i) left–right pairs of flight muscles normally fire precisely, within 0.5–0.6 ms of each other; (ii) during a yawing optomotor response, left—right muscle timing differences shift throughout a wider 8 ms timing window, enabling at least a 50 per cent left–right power differential; (iii) timing differences correlate with turning torque; and (iv) the downstroke power muscles alone causally account for 47 per cent of turning torque. To establish (iv), we altered muscle activation during intact behaviour by stimulating individual muscle potentials to impose left—right timing differences. Because many organisms also have muscles operating with high power–phase gains (Δ_power/Δ_phase), this motor control strategy may be ubiquitous in locomotor systems.

1. Introduction

Rhythmic locomotor behaviours arise from the periodic production of mechanical power by appendage or body muscles. In such behaviours, control arises from neural feedback acting through the physiology and biomechanics of those locomotor structures subject to clock-like neuromuscular activation. Muscle power production depends on both the frequency and phase of muscle activation (timing of activation relative to length change) as well as the temporal history of muscle strain [1]. In vitro studies across a wide variety of animals have shown that power output strongly depends on phase [2–6], providing the nervous system one opportunity to exert influence on motor control. Here, we ask whether the subtle changes in the timing of muscle activation in conjunction with a strong dependence of power on phase may enable a control mechanism that has been under appreciated.

While we know muscles can adopt a variety of functions, from brakes and motors to struts and springs, these functions are usually considered in the context of steady-state behaviour [7]. By contrast, unsteady tasks that require control, such as stabilization and manoeuvring, may pose greater performance challenges than nominal steady motions, requiring transient changes in the regular pattern of force production and power output. One way in which the nervous system may exert control is via phase modulation of the rhythmic motor commands it sends to muscles during manoeuvres [1,7–9]. Exquisite sensitivity to neural control of phase may be particularly important in muscles that both contribute significant power and have a steep slope in power output around their normal, unperturbed phase of activation.

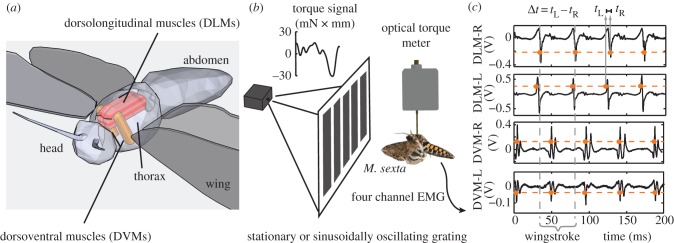
A longstanding, canonical example of dedicated muscle function comes from the large antagonistic pairs of indirect flight muscles that power the majority of insect fliers. These dorsolongitudinal muscles (DLMs, alternatively dl₁ or DLM₁) and dorsoventral muscles (DVMs) deform the thorax to generate downstrokes and upstrokes, respectively (figure 2a) [10,11]. In some insects, including moths and butterflies (Lepidoptera), these muscles are periodically activated by few, often only one, actively propagating muscle potentials (‘spikes’) during each wingstroke (termed synchronous muscle) [11]. In other insect taxa, notably the Diptera, activation of such muscles yields contractions at frequencies well in excess of the frequency of muscle spikes provided by the nervous system (asynchronous muscle) [12–14]. These activation properties have led to the reasonable, if perhaps premature assumption that these muscles perform a dedicated power function responsible for establishing the gross wingstroke trajectory. Under this hypothesis, control functions are attributed to a suite of smaller steering muscles that trim, tension or otherwise modulate the regular, clock-like output of power musculature [11,13,15]. Further indirect evidence for a constant power-like function of the DLMs has emerged in recent work showing that the downstroke muscle's subunits fire in synchrony and that the muscle is capable of producing only the positive power output required for flight in a narrow band of stimulus and strain conditions [6,16].

Figure 2.

Left–right timing differences in pairs of power muscles. (a) Dorsolongitudinal muscles (downstroke: DLMs) and dorsoventral muscles (upstroke: DVMs) power moth flight indirectly via deformation of the thoracic exoskeleton. (b) With the moth tethered to a torque meter, we recorded from both left–right pairs of muscles as the moth turned in the yaw (left–right) plane. (c) Electromyograms showed active muscle potentials (‘spikes’) from each muscle that were easily discriminable (orange dots) using threshold crossing (orange dashed line). During each wingstroke (grey dashed lines; defined by the right DLM spiking), we measured the time difference between the left and right discriminated spike in each muscle pair.

Despite these indicators of a dedicated power function for the main flight muscles, recent findings raise the possibility that they also serve a control function, particularly in synchronous fliers. Tu & Daniel [6] have shown that in the hawkmoth, Manduca sexta, the DLMs operate at a phase that yields only approximately 50 per cent of maximum power, but do so where the instantaneous slope of the power–phase relationship is high (figure 1). From a control perspective, this high physiological gain (Δ_power/Δ_phase) suggests that animals could take advantage of precise timing changes to produce large modulations in muscle power output. For Manduca, the range from zero to maximum positive power output on the power–phase curve is subsumed in a ±4 ms window around steady-state conditions (figure 1). These observations suggest the alternative hypothesis, that power muscles may indeed contribute to control with neural feedback modulating the timing rather than the magnitude of muscle activation. While the timing of activation and temporal details of the strain cycle in the DLMs are interdependent, a steep power–phase relationship remains even under moderate changes in strain [6,16]. This steep slope suggests that there may be a general consequence on power of very subtle timing differences in these rhythmically activated muscles.

Figure 1.

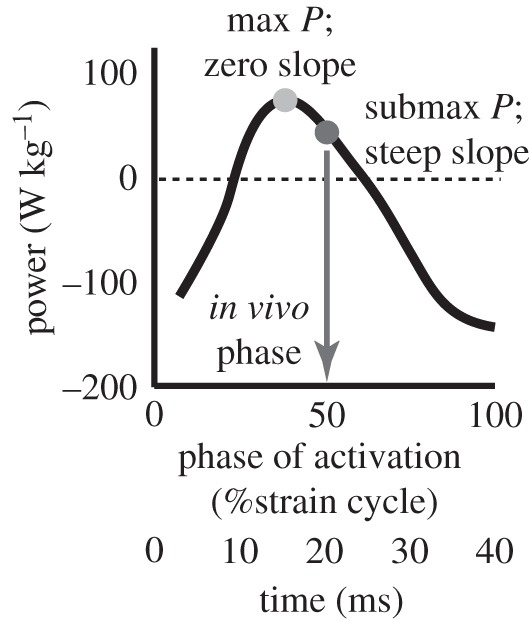
Submaximal phase of muscle activation. Power output from Manduca sexta downstroke muscles depends on the phase of neural activation with respect to muscle length change. The steady-state in vivo phase (dark grey circle and arrow) is submaximal for power production and has a steep slope compared with the phase that maximizes power, but where the slope is zero (light grey circle). A phase of zero marks the onset of muscle lengthening. Figure based upon data in Tu & Daniel [6].

To determine whether an animal takes advantage of timing modulation to accomplish significant control with its power muscles, four lines of evidence are required. (i) The nervous system must time muscle spikes with a high degree of precision, because a large variance in timing would mean that the animal would be unable to reliably specify a operating point on the steep power–phase curve. (ii) Neural feedback from some sensory modality must change muscle timing over a biologically relevant range, and in a manner correlated to the sensory stimulus. (iii) Changes in timing must correlate with significant changes in body dynamics. (iv) Because many muscles could be modulated in response to any given stimulus, timing differences in the power muscles must be causally implicated in the motor response.

To address these four criteria and establish the control authority of flight power muscles, we elicit left—right turning (yaw) behaviours in hawkmoths tethered to a torque metre (figure 2b) and experiencing a visual yaw stimulus. Yaw turns require significant torque production that may, in turn, require significant power differentials between the left and the right side of the animal. This manoeuvre is therefore a likely candidate for revealing timing modulation. We measure electromyograms from the left—right pairs of DLMs and DVMs (figure 2c). We consider how timing differences in each left–right pair contribute to torque production during visually evoked yawing responses. Timing differences in the DLM will generally translate into left–right phase differences, because both muscles insert directly onto the same anterior portion of the thoracic cuticle and should undergo comparable, though not identical, length changes. Under these conditions, the power–phase curve maintains a steep slope [6,10]. However, because these effects operate indirectly, and small strain changes acting at the wing hinge can translate to a variety of wing kinematic changes, it is still important to establish a causal role of left–right timing modulation in producing turning torque. To do this, we use the approach developed in Sponberg et al. [9] of altering the timing of individual muscle potentials in the power muscles alone without concomitant changes in other muscles. We thereby isolate one of the animal's ‘control knobs’, namely left–right timing differences, and explore its role in torque production.

2. Methods

(a). Animals

Moths (Manduca sexta) were housed communally (University of Washington, Department of Biology colony) under continuous light conditions to reduce flight and potential wing damage prior to experimentation. Both naive males and females were used, all between 3 and 5 days post eclosion.

(b). Bilateral recordings of upstroke and downstroke muscles

In these first experiments, both pairs of DLMs and DVMs (figure 2a) were recorded simultaneously in five animals using bipolar tungsten electrodes. Moths were first allowed to dark adapt and performed warm-up shivering. We then collected at least five, 8 s long trials while the moths flew with a stationary visual grating of black and white bars that was projected onto a vellum screen using a small projector (figure 3; ‘stat’ trials). The grating was then sinusoidally oscillated, and we collected at least five 8 s long trials under these conditions (‘sin’ trials). To control for fatigue or other trial-to-trial effects, we then repeated the stationary grating conditions (‘post’ trials). Further recording details are in the electronic supplementary material, methods S1a.

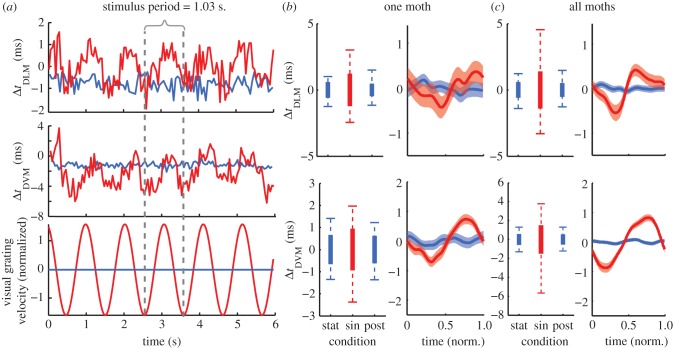
Figure 3.

Precision and timing differences in power muscles during the optomotor response. (a) The DLM and DVM timing differences were measured during optomotor responses with a stationary (blue) and sinusoidally oscillating (red) grating. Mean timing differences (evident in (a)) varied across animals and muscle pairs owing, in part, to spike detection catching different parts of the left and right muscle spikes (see the electronic supplementary material, methods S1a). In order to focus on variation in timing differences, we subtracted the mean timing differences in each bout to give estimates of jitter and variation in each animal ((b) and electronic supplementary material, figure S2) and (c) enable comparison across all animals. Box plots represent the jitter (standard deviation of the timing difference; thick bar) and the 98% timing window (whiskers). The mean response plots (right column in (b) and (c)) are constructed by smoothing the timing differences over successive periods of the visual stimulus using a sliding Gaussian window with an s.d. of 0.05 s and calculated every 0.5 ms. Mean responses illustrate the correlation with the visual stimulus, but underestimate the full magnitude of timing variation because of smoothing (refer to the box plots instead). Normalized time is relative to the period of the visual stimulus (1.03 s.) scaled from 0 to 1. In (b), n = 389, 471 and 484 wingstrokes for stat, sin and post conditions, respectively. In (c), n = 5 animals with 1882, 2660 and 1838 wingstrokes.

Muscle potential (‘spike’) times were detected using threshold crossing (figure 2c). To quantify precision in the upstroke and downstroke muscles, we measured the jitter in timing from one muscle to the other in each left and right pair (Δt_DLM and Δt_DVM; figures 2c and 3a). We define jitter as the standard deviation of the left–right timing differences in a muscle pair (figure 3b,c thicker boxes). This parallels the use of jitter in reporting the precision of sensory spike times to a repeated stimulus [17]. Jitter during trials with a stationary grating gives an estimate of the resolution available for timing control.

We also considered the 98 per cent timing window for the data, excluding the 1 per cent extremes on each side (figure 3b,c whiskers). The 98 per cent timing window during the sinusoidal grating trials indicates the range of timing differences available for control. Statistical tests were implemented in Matlab (Mathworks, Natick, MA, USA) and JMP (SAS Institute, Cary, NC, USA) programs (see the electronic supplementary material, methods S1b).

(c). Torque recordings during manoeuvres and stimulus-induced changes in muscle timing

In the second set of experiments, we included torque measurements and muscle stimulation, but only the DLMs were considered. This was because moths with fewer electrodes typically sustained wing flapping for more experimental flight bouts. The stimulation experiments required many repetitions to generate sufficient data because we stimulated only for a single wingbeat per flight bout, whereas in the earlier experiments, we could record many wingstrokes per bout. Furthermore, stimulation of the DVMs was not possible as we could not target those muscles with bipolar stimulation in our current preparation and hence could not ensure that the entire muscle was selectively stimulated. Power–phase curves were also available only for DLMs in the literature [6].

Seven moths were used, but recording and stimulation conditions were included for each individual. Prior to these experiments, we attached each moth to a custom optical torque meter (see the electronic supplementary material, figure S1). Following this, the stationary and sinusoidal conditions were repeated as in the previous experiments. Given that no differences were found between stationary and ‘post’ trials in the first experiments, the ‘post’ condition was eliminated during these experiments to maximize the number of subsequent stimulation trials. Following the optomotor trials, we altered muscle activity in real time during the flight behaviour using phase-locked muscle stimulation of individual muscle potentials [9] (see the electronic supplementary material, methods S1c). This stimulation method provides selective stimulation that demonstrates threshold recruitment of the muscle, mimics stimulation used during Manduca work loop preparations [6,16], and, in other insect muscles, has been shown to provide equivalent power production as neural stimulation under identical strain conditions [18]. We used a stationary visual stimulus to encourage straight flight, but then imposed timing differences between the left and right DLM to test whether these muscles alone could induce yaw torque production.

3. Results

(a). Precision of left–right pairs of power muscles during steady-state flapping

The relative timing of power muscle activation during the stationary grating conditions revealed the precision with which left and right pairs of muscles fire together (figure 3, blue traces). The jitter between the left and right muscle was 0.58 ms in the DLMs and 0.55 ms in the DVMs (figures 2c and 3a). The 98 per cent timing windows spanned −1.5 to 1.3 ms for the downstroke muscles and ±1.4 ms for the upstroke muscles (figure 3b,c, whiskers). While there were statistically significant differences in variance between moths (Bartlett's multiple group variance test, p < 0.00001; electronic supplementary material, figure S2) and individual flight bouts (p < 0.00001), all jitter measurements were within ±0.3 ms of each other. There was no difference in the pairs of upstroke and downstroke muscles in terms of their left–right precision as measured by the variation in the timing between the two sides (equivariance F-test, p = 0.57). These jitter values probably underestimate the true precision with which the moth can simultaneously activate the left and right muscles, because we were unlikely to have eliminated all active neural modulation even with a fixed visual target, i.e. we cannot ensure a perfect steady-state behaviour. The most precise individual had jitters of 0.28 and 0.38 ms for the downstroke and upstroke muscle pairs, respectively.

(b). Optomotor feedback produces left–right timing modulation

The left–right pairs of upstroke and downstroke muscles both showed phase modulation during the optomotor response to the oscillating grating (figure 3a and electronic supplementary material, video S1). As the moth attempted to turn to the right (clockwise), the right muscle advanced in time with respect to the left muscle. Left–right timing differences varied across a range many times greater than the jitter observed during stationary bouts, spanning −3.3 to +4.6 ms for the downstroke muscles and −5.7 to +3.8 ms for the upstroke pair (figure 3b,c; equivariance F-test, p < 0.00001). These ranges did vary between individuals (electronic supplementary material, figure S2; Bartlett's test, p < 0.00001). These values probably underestimate the full range of timing variation in the muscle pairs, because it is difficult to elicit maximal performance across many animals and trials. By taking the maximum variation in a single flight bout (typically 100–200 wingstrokes) as an upper bound on the variation, we find timing differences that span 11.2 and 12.9 ms for the downstroke and upstroke muscles, respectively.

The ‘post’ stationary conditions produced jitters that were indistinguishable from the first set of trials (downstroke muscle, p = 0.2) or slightly more precise (upstroke muscle, 0.06 ms decrease, p < 0.004), indicating no evidence for increasing timing variation owing to fatigue or other mechanisms.

Changes in the timing differences of both pairs of muscles were correlated with the movement of the visual grating (p < 0.00001; figure 3b,c). The responses lagged the visual stimulus' velocity by about a quarter of a period (approx. 250 ms) owing to a combination of delay and sensorimotor filtering [19]. Timing differences in the DLMs and DVMs were also correlated to one another (p < 0.00001; figure 3).

(c). Yaw torque production correlates to timing differences in power muscles

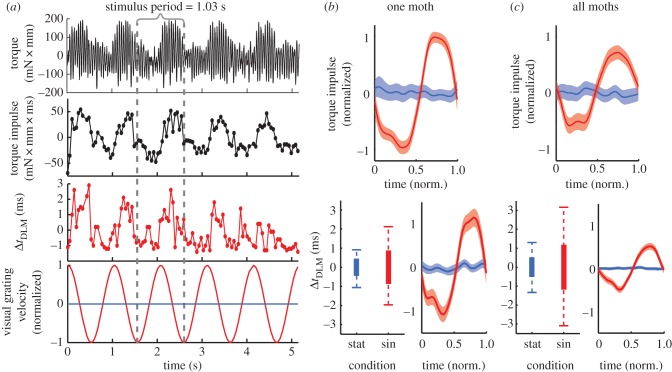
With the moth mounted to the torque meter, wingstroke-to-wingstroke torque was modulated during the optomotor response, allowing us to quantify the moth's turning effort (figure 4a). We integrated torque across each wingstroke defined by the period between subsequent right DLM muscle firings to give the net turning impulse (mN × mm × s; figure 4a and table 1). Turning impulse would correspond to the net change in the moth's angular momentum absent other forces, although actual rotation of the moth is counteracted by the tether and the exact forces and moments experienced can differ during free flight conditions.

Figure 4.

(a) Dorsolongitudinal muscle (DLM) timing differences are correlated with torque modulation. The raw torque signal and integrated torque impulse (dots represent each wingstroke) correlated with changes in DLM timing. Mean response plots and whisker plots were constructed as in figure 3b,c. The torque plots were normalized to the mean torque produced during the maximum optomotor response (red denotes oscillating grating; blue denotes stationary grating). n = 358 and 590 wingstrokes for stat and sin in the example animal and n = 7 animals and 3973 and 4229 wingstrokes altogether.

Table 1.

Summary statistics for changes in left–right DLM timing and torque produced during the optomotor task.^a

animal	J	K	L	M	N	P	Q
jitter (ms)	0.39	0.78	0.58	0.39	0.3	0.34	0.42
98% timing window (ms)	5.7	8.8	6.2	3.8	7.2	3.0	4.2
torque impulse (mN × mm × s)	1.55	0.05	0.42	0.64	0.34	0.25	0.18
gain (ΔTor ms−1 change in Δt)	0.78	n.a.	0.21	0.16	−0.05	0.31	0.10
norm. gain (% turn Tor ms−1)	50	n.a.	50	26	−17	134	57
r-value	0.68	n.a.	0.53	0.23	0.15	0.4	0.21
p-value	<0.0001	0.6	<0.0001	<0.0001	0.0001	0.0001	<0.0001

^aAnimal K had very low torque modulation that was not significantly correlated with changes in DLM timing. Correlation stats were not calculated in this case.

As shown earlier, all seven animals demonstrated timing shifts between their DLMs during the optomotor response in a manner correlated with the stimulus (figure 4; p < 0.0001 across all animals, and for each individual except p < 0.05 for moth K). The timing window over which the muscles shifted varied from 3.0 to 8.8 ms (figure 4b,c box plots), and tracked the torque impulse (figure 4). Changes in torque impulse were correlated with timing differences in the DLMs in six of the seven animals considered (table 1; electronic supplementary material, figure S3; p < 0.0001) although one produced weak correlations in the opposite direction of the others (moth N). Both the mean torque impulse and its standard deviation varied from animal to animal (table 1; electronic supplementary material, figure S3; ANOVA, p < 0.0001; Bartlett's variance test p < 0.0001). To compare across animals, we normalized torque impulse across the turns and found a typical gain (slope) of 50 ± 20 per cent of the turning response per millisecond change in left–right timing.

(d). Inducing a temporal separation in power muscles alone accounts for a large degree of torque production

To establish the causal role of power muscles in generating turning torque, we stimulated the DLMs to mimic their optomotor timing change (figure 5a), but without concomitant changes in other muscles involved in turning, most notably the third axillary [20,21]. Imposing a timing difference in just the downstroke muscles was sufficient to produce significant yaw torque during flight, even with a stationary visual stimulus (figure 5b and electronic supplementary material, figure S4). Across all animals, the change in torque impulse compared with the unstimulated wingstroke was correlated with the induced timing change (regression; p < 0.0001, r² = 0.69; figure 5c and electronic supplementary material, table S1). Consistent with the earlier-mentioned correlation experiments, advancing the left muscle's activation produced a turn to the left and vice versa. In animal Q, we also stimulated the left muscle without trying to induce a timing change (Δt = 0). This control demonstrated no effect on torque production from the stimulation methodology alone (Δt = −0.1 ± 0.3 ms s.e.m.; normalized Δ torque impulse = 3 ± 37% of turning torque; t-test compared with zero, p > 0.1; electronic supplementary material, table S1). The change in torque predicted for a muscle timing difference of 0 ms (the y-intercept of figure 5c) was also not significantly different from zero (intercept F-test, p > 0.1), further supporting the control predictions.

Figure 5.

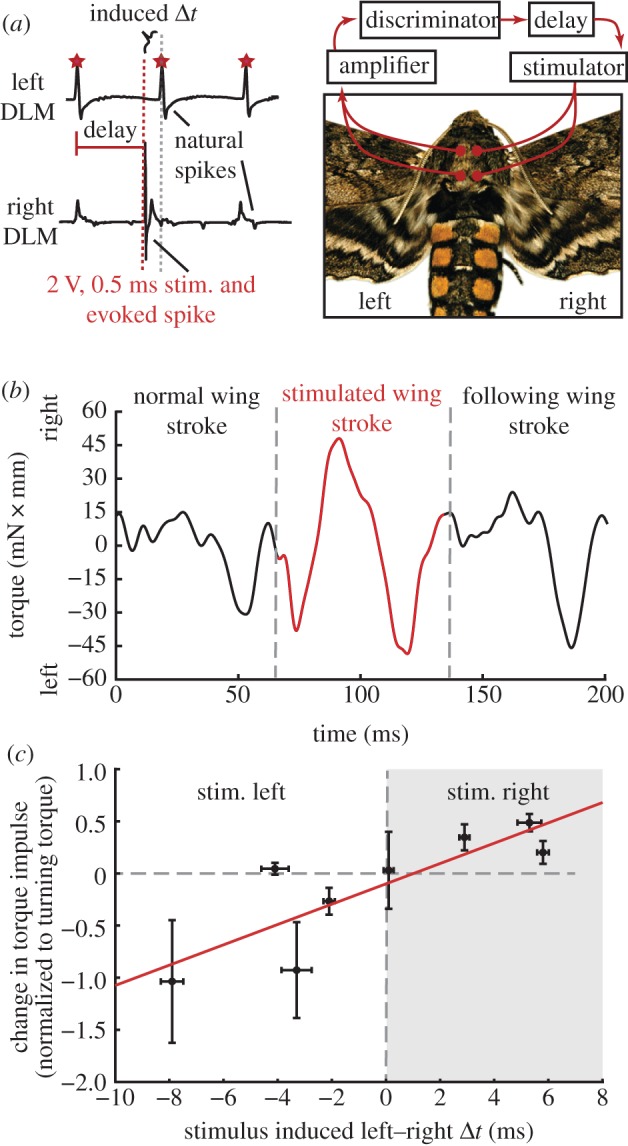
Torque production in response to imposed timing shifts. (a) Using phase-locked stimuli, we induced timing shifts between the left and right dorsolongitudinal muscle (DLMs). Imposing a timing difference in the DLMs alone significantly modified torque production during the stimulated wingstroke ((b), also see the electronic supplementary material, figure S4 for histogram of one animal's response and the electronic supplementary material, table S1 for all animals). (c) Across all animals, the change in torque impulse (stimulated minus preceding wingstroke, normalized as in figure 4) was significantly correlated with the timing difference induced between the left and right DLMs. Error bars (s.e.m.) along the abscissa reflect variation in the timing difference imposed by the stimulus. The point near the origin is the control data from animal Q (see also the electronic supplementary material, table S1).

All animals, except one, showed significant torque modulation in response to the imposed timing difference (see the electronic supplementary material, figure S3). The magnitude of the induced torque varied from 20 per cent to 104 per cent of the torque produced during the optomotor responses, with an average of 37 ± 6 per cent across all trials or 47 ± 14 per cent if averaged across animal means (see the electronic supplementary material, table S1). While there was substantial variation in the response within each individual (see the electronic supplementary material, figure S4), the typical torque production accounted for a significant portion of the net optomotor response.

4. Discussion

Muscles operating submaximally on the steep slope of their power–phase curve raise the possibility of playing a dual role in power production and control through neural feedback modulating muscle timing from wingstroke to wingstroke. Insect flight muscle satisfies the four criteria necessary to demonstrate this control relationship: precision, modulation, correlation and causation. We first showed that pairs of power muscles are normally activated with precise contralateral timing, indicating symmetric, reliable power output (criterion 1: precision). Second, we discovered an optomotor feedback response that breaks this normally precise co-activation and modulates left–right timing variation over an 8 ms window for downstroke muscles (5 ms for upstroke) with a resolution (± the jitter or 2 s.d.) of 0.56 ms (0.76 ms for upstroke) taken from the most precise jitters measured during the stationary trials (criterion 2: modulation). In the downstroke (DLM) pair of muscles alone, this timing modulation is sufficient to span the entire range of positive power production (figure 6a). Third, we showed that small changes in timing correlate to the moth's torque production during an optomotor response (criterion 3: correlation). Finally, we demonstrated a causal link between induced timing changes and torque (criterion 4: causation). Changing the timing of the DLM spike in a single wingstroke can account for 47 per cent or more of the yawing torque. While significant torque was produced with imposed timing differences of even a few milliseconds, these changes did not account for all the torque produced during response to visual stimuli (electronic supplementary material, table S1). This is not surprising because we modulated the timing only in the DLMs without the potential contributions from the DVMs, steering muscles or abdominal reflexes.

Figure 6.

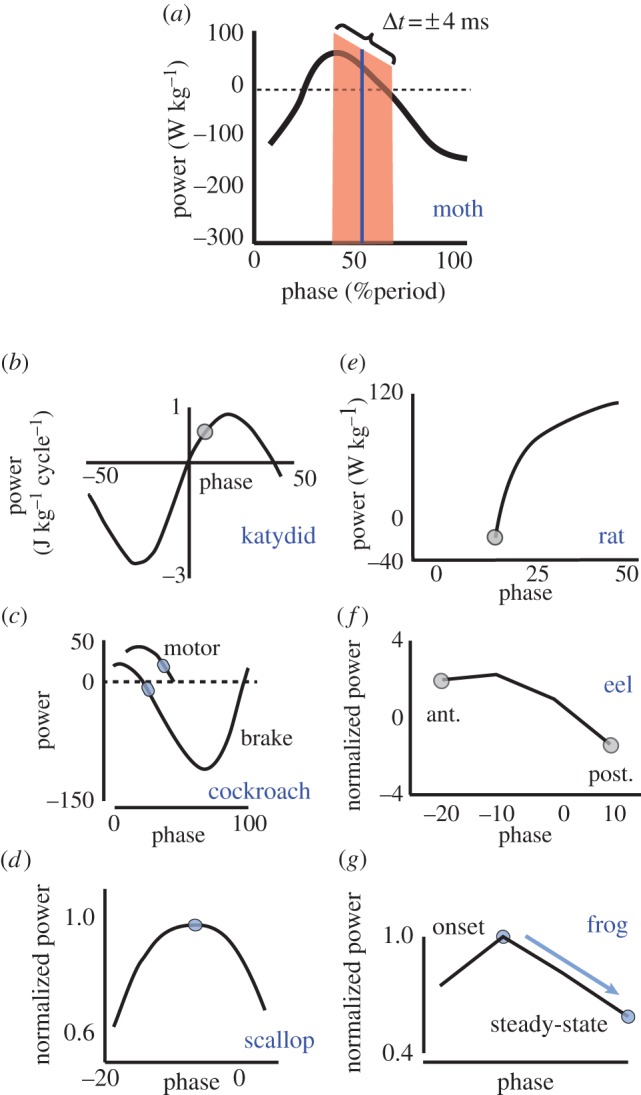
Muscles in a variety of invertebrates and vertebrates show a high power–phase gain (Δ_power/Δ_phase). Shaded blue dots represent the in vivo phase of activation. The shaded red region in (a) represents the range over which Manduca can modulate the timing difference between its left and right power muscles. Zero phase is not the same across all figures, but is consistent with conventions for each organism. (a) Manduca sexta DLM flight power muscle: this study and [6]. (b) Neoconocephalus triops targocoxal flight muscle [2]. (c) Blaberus discoidalis power (muscle 177c) and control (muscle 179) femoral extensors [5]. (d) Argopectin irradians swimming adductor [3]. (e) Rattus norvegicus gastrocnemius (compiled from figure 10 and table 1 of Ettema [22]). (f) Anguilla anguilla posterior (post.) and anterior (ant.) body undulation muscles, red fibres [4]. (g) Hyla versicolor and Hyla chrysoscelis external oblique vocalization muscle [23].

(a). A steep power–phase relationship and precise neural timing enables control

The motor control strategy we found in Manduca flight muscles relies on timing modulation and requires two additional features: a high power–phase gain and sufficient precision to allow reliable specification of muscle activation at the millisecond timescale (figure 3). The remarkable precision (low jitter) in the simultaneous firing of these left–right muscle pairs is less than the jitter noted for most sensory neurons responding to repeated stimuli (typically several milliseconds or more [17,24]). It is comparable to the very low jitter values found in Dipteran haltere mechanoreceptors [25]. However, jitter in motor output is a measure of the simultaneity of the left and right spikes, and so constitutes a somewhat different measure than the sensory responses to repeated presentations of the same stimuli.

The muscles of many other organisms meet power demands with control strategies that use submaximal activation levels at steady-state. They increase the number of spikes or recruit addition motor units to increase power output and meet behavioural demands. Such strategies underlie the power modulation in some birds when varying flight speeds [26]. However, insect flight muscle generally is composed of few motor units, all of which are simultaneously activated in Manduca [27], and whose number of muscle spikes is generally small, constrained by the 40–50 ms wingstroke [11,28]. Therefore, the recruitment strategy for the main flight muscles in vertebrates is not feasible here, and the moth must rely instead on timing control to alter these muscles' power output.

We can estimate the impact of the subtle timing changes on power output by plotting the range of activation times (figure 3b,c, whisker plots) on the DLM power–phase curve (figure 1). Superimposing the timing differences as a range around the steady-state activation point (figure 6a) shows that they span the full range of possible positive power output (from zero to maximal power). It is possible that instead of one muscle shifting while the other remains at the steady-state phase, both muscles shift so that one is earlier and one is later. However, a 4 ms timing difference between the two downstroke muscles translates to a power differential of approximately 50 per cent of maximum power regardless of where it is contained in this range. Furthermore, small left–right differences in the strain cycle experienced by each muscle may cause the left and right DLMs to have somewhat different power–phase curves when timing is altered. However, the power differential remains because Tu & Daniel [6] and George et al. [16] showed that phase, and therefore timing, dependence remains even if strain changes. If anything, increases in strain tend to amplify the power–phase relationship even more, suggesting a potential enhancement effect within the muscle's stress–strain coupling.

The power output from the main flight muscles acts indirectly, deforming the thorax to drive the wings through a complex wing hinge [11]. Changes in the main flight muscles interact with the action of steering muscles also under visual or other sensory control [20,21]. These steering muscles are generally small and directly stiffen, flex or engage small cuticular sclerites at the wing hinge [13,15,21]. They play an important role in turning by mechanically conditioning the wing hinge to affect changes in the wingstroke with relatively subtle changes in the muscle strains [11,21,29]. Ultimately, we know from our stimulation experiments (figure 5) that changes in timing between the main flight muscles do indeed cause yawing torques independent of steering muscles. However, we do not yet know the complete mechanism by which changes in timing and changes in muscle power are translated through a complex wing hinge, a deformable wing and aerodynamics to ultimately produce yaw torque. Further investigation of these mechanisms awaits technology for in vivo imaging of thoracic muscle strain and wing hinge kinematics during flight. Moreover, while we have shown similar precision and modulation in the DVMs as in the DLMs, the efficacy of timing control in the upstroke muscles cannot be fully assessed without their power–phase relationships. However, the even greater magnitude of the 98 per cent timing windows observed in the DVM pair (9.5 versus 7.9 ms for the DLMs) suggests that modulating activation timing may play at least as great a role in the control of power output from these muscles.

(b). An optomotor feedback circuit to moth flight power muscle

Visual modulation of the DLMs and DVMs constitutes a new control pathway for insect flight, targeting the main power muscles in the organism. Our current understanding of flight control has long assumed these were dedicated motors. Revision of this control framework is therefore necessary. Visual feedback to flight muscles must be integrated with the broader multiple input/multiple output control strategy that governs flight. It is already established that multiple sensory inputs can impinge upon one another to modulate their effects (e.g. Drosophila vision and halteres [30]) and alter multiple motor systems (e.g. Manduca visual feedback to wings and abdomen [31]).

In the case of the yawing optomotor response considered here, control is also acting in a multiple output manner. We observe correlated responses across the suite of downstroke and upstroke flight muscles (figure 2 and the electronic supplementary material, S1). However, the resolution of this control is limited, even given the high left–right precision. This is because there is only a narrow timing window over which power output varies from zero to maximum, even if the overall strain is allowed to vary [6,16]. Our results suggest that the moth may use high-gain control of power muscles to modulate the overall power balance during locomotion in combination with the action of more finely tuned, but smaller steering muscles to transfer, redirect or redistribute the larger shifts in power. Recent experiments are showing that timing control may not be limited to the optomotor context we consider here, but may also underlie free flight turning [32].

(c). The ubiquity of submaximal, high-gain-power–phase relationships in muscle

Thus far, we have explored the role that high muscle gain and neural timing control can play in the flight behaviour of moths, but how ubiquitous is this control strategy in other systems? It is well known that phase of activation can change in a variety of muscles. However, the motor control strategy here requires two other properties: high muscle gain and sufficient precision in neural feedback to resolve power differences. Evidence for these two properties comes from in vitro studies that indicate where on the power–phase curve the muscle is operating and in vivo studies demonstrating how phase is modulated. In this study, we have demonstrated these properties in synchronous flight muscle (figure 6a), previously considered one of the least likely muscles to have a significant role in control. While we do not yet have similar studies investigating how small changes in timing affect both muscle physiology and in vivo behaviour in other systems, we can examine whether a steep instantaneous slope in the power–phase relationship exists in other systems. If it is indeed common for other muscles to operate at the steep portion of their power–phase curves, then there exists a potential for precise timing of neural commands to have significant control authority.

Looking across invertebrates, other orders of flying and running insects have submaximal steady-state phases of activation and high power–phase gains in their locomotor muscles (katydid flight muscles, figure 6b [2]; cockroach leg power and control muscles, figure 6c; [5]). Unlike these examples, scallop adductor muscle does use a phase that maximizes power output. This exception to the other examples may arise from different performance demands. This muscle is primarily used for escape swimming and so may require high power rather than large control authority (figure 6d; [3]).

Vertebrates also demonstrate patterns consistent with precision timing strategies for control. The phase of rat gastrocnemius muscle activation during walking is on the rising power–phase slope, but is submaximal for both power and efficiency (figure 6e; [22]). Eel posterior, but not anterior, power muscles have a submaximal, higher-gain phase of activation with significant potential for timing control (figure 6f; [4]). This difference arises from the anterior-to-posterior gradients in the phase of muscle activation during undulatory swimming [4,33]. Finally, frog vocalization muscle in the Hyla genus is maximal at the onset of singing, but falls off to a submaximal phase where the power–phase slope is steeper during steady-state (figure 6g; [23]).

Taken together, our results in Manduca and the earlier-mentioned examples (see the electronic supplementary material, S1 discussion for more detail on each) suggest that the coupling of precise neural feedback control of timing and high power–phase gain can be a general strategy for dealing with control demands. We emphasize that other, non-mutually exclusive control strategies may exist for modulating muscle function, particularly in some of the earlier-mentioned examples where there are sometimes many recruitable motor units and more flexible patterns of activation. Teasing apart the effects of timing change will be challenging when muscle length change has stronger effects on the shape of the power–phase curve than in Manduca. However, regardless of such factors, the point remains that the high sensitivity of muscle power around the steady-state phase of activation in a diversity of organisms indicates that the role of small timing changes in control may be underappreciated. Combining advantageous muscle physiological properties, such as the high gain in power, with precise timing on a wingstroke-to-wingstroke basis enables control in muscles that were long assumed only to produce steady power output.