Abrupt climate change and thermohaline circulation: Mechanisms and predictability

Abstract

The ocean's thermohaline circulation has long been recognized as potentially unstable and has consequently been invoked as a potential cause of abrupt climate change on all timescales of decades and longer. However, fundamental aspects of thermohaline circulation changes remain poorly understood.

The global thermohaline circulation (THC) consists of cooling-induced deep convection and sinking at high latitudes, upwelling at lower latitudes, and the horizontal currents feeding the vertical flows. Contrary to widespread perception, convection and sinking are neither the same nor co-located (1) because when rotational effects are strong, flow tends to be around a patch of maximum surface density (characterizing convection), rather than into it. In the North Atlantic, where most of the deep sinking occurs (2), the THC is responsible for the unusually strong northward heat transport. Together with the equatorward (up-gradient) heat transport in the South Atlantic (3), this results in the relative mildness of western European climate. Here I discuss our conceptual understanding of the THC and fundamental issues concerning its predictability. The THC's role in abrupt climate change is not comfortably established—on the contrary, it poses major scientific challenges and thus provides a powerful focus for climate research.

The most conspicuous feature of the observed global THC is its geographic asymmetry: While water sinks in the North Atlantic at a rate of 15–20 Sverdrups (1 Sverdrup ≡ 10⁶ m³⋅s⁻¹), to depths between 1 and 4 km, no such deep sinking occurs in the North Pacific (4). The reason for this difference lies in the different surface salt concentrations; the North Pacific is so low in surface salinity that not even water cooled to the freezing point becomes dense enough for deep sinking. However, the difference in surface salinity is in part a consequence of the presence and absence, respectively, of a vigorous THC in the North Atlantic and the North Pacific. Thus, high surface salinities at high latitudes can maintain themselves through the THC they help to induce. The observed difference in Atlantic and Pacific THC has been reproduced qualitatively in an idealized global model that had no built-in bias toward Atlantic deepwater formation at all, neither through geometry nor through atmospheric forcing (5).

On the other hand, it is also plausible that a temporary anomalous influx of freshwater might lead to a permanent reduction in the North Atlantic surface salinity and THC. A wealth of model studies, ranging in complexity from the simplest imaginable to comprehensive climate models, have confirmed that such a THC collapse is in principle consistent with the physical laws governing the ocean (6). The associated reduction in the North Atlantic heat transport would lead to a cooling of western European climate, which might have triggered the onset of ice ages and temporary cold spells such as the Younger Dryas (YD).

Basic Concepts.

The THC is maintained by density contrasts in the ocean, which themselves are created by atmospheric forcing (heat and water exchanges) and modified by the THC. Typically, the ocean is heated (thus made less dense) where pure freshwater is evaporated (water thus made saltier and denser), and vice versa, and the central question concerns which of the opposing latitudinal temperature or salinity contrasts dominates the density difference. A crucial, although not widely appreciated, additional question concerns which contrast one should consider—the one between the equator and the poles, or the one between (say) North and South Atlantic, or perhaps even between the North Atlantic and the North Pacific. This choice matters in assessing what order of magnitude changes in surface density it might take to reverse the THC: “Pole-to-pole” density differences are about one order of magnitude smaller than “pole-to-equator” ones and hence much more easily reversed. For many years, the textbook example for explaining multiple states of the THC has been an idealized model confined to a single hemisphere (7), but our conceptual understanding probably should be shaped by this model's two-hemisphere cousin (ref. 8; see Appendix).

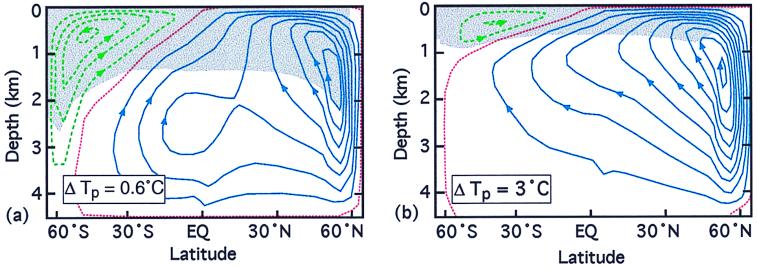
More complex (albeit still idealized) models (9, 10) suggest that very different dynamics apply to the global integral of deep sinking and the portion of it that crosses the equator. The global integral is rate-limited by the average pole–equator density difference and the ocean's ability to warm the upwelling deepwater [but not by the efficiency of high-latitude convective mixing (1)]. In contrast, small pole-to-pole density differences can induce a strong interhemispheric THC (Fig. 1) because they typically induce deep pole-to-pole density differences covering a large depth range, leading to significant deep pole-to-pole pressure differences (10). Hence, if the gross density structure of the ocean and thus the globally integrated deep sinking rate do not change, a reduction in Northern Hemisphere (NH) THC would be associated with an increase in Southern Hemisphere (SH) THC. The SH THC increase could occur through a shallow Atlantic THC or through a Pacific THC. Either way, a decrease in cross-equatorial THC in the Atlantic would tend to cool the NH compared with the SH (11) whereas the associated strengthened SH THC would reduce the SH equator–pole temperature contrast (12), causing high-latitude warming. However, this warming might be lesser in amplitude because the Antarctic Circumpolar Current is an impediment to strong ocean heat transport into high southern latitudes.

Figure 1.

THC streamlines (each contour denoting 2 Sverdrups) from an idealized three-dimensional model spanning both hemispheres of a single ocean basin. The blue solid lines mark the “northern” THC cell (sinking in NH) while the green, dashed lines mark the “southern” THC cell (sinking in SH). The red dotted lines demark the boundary between the cells. Shading represents temperatures higher than the minimum in the SH. The pole-to-pole imposed surface temperature difference is denoted by ΔT_p. (a) Small asymmetries in surface forcing cause strongly asymmetric flows. (b) As the northern cell increases, the southern cell weakens by almost the same amount, leaving their sum nearly constant (adapted from ref. 9).

The scenario of increased SH deepwater formation accompanying decreased NH THC is consistent with the observation that, during the YD, believed to be characterized by a much weaker NH THC, atmospheric radiocarbon only increased over a period much shorter than the YD (13). As the THC removes radiocarbon from the atmosphere, this indicates that global deepwater formation returned to its “normal” rate within 200 years of YD onset (14), implying increased deepwater formation outside the North Atlantic. If this “seesaw” (14) is in operation, NH and SH temperatures would be exactly out of phase; that is, changes would be simultaneous but of opposite sign. There is a debate about whether the Dansgaard-Oeschger oscillations in Antarctic and Greenland ice cores indicate lagged temperature changes of equal sign (15) or simultaneous changes of opposite sign (ref. 16, supporting the seesaw picture). Records such as those shown in ref. 15 pose a powerful constraint on climate models, which have not yet been able to reproduce even crudely these changes in past temperatures.

Further challenges to our understanding abound. Two independent coupled modeling studies simulating YD-like events (17, 18) both reported a transient decrease in high-latitude SH sea surface temperature during the period of greatly reduced North Atlantic THC and hence also lower NH temperatures. The South Pacific THC weakened, rather than strengthened, during the YD (18), just the opposite of the seesaw prediction. In the long run, the THC is unlikely to be weaker in both North Atlantic and South Pacific, owing to the constraint on the globally integrated deep sinking rate. But one might speculate that the global-scale, North Atlantic-to-North Pacific deep density difference drives the deep inflow into the Pacific; this density drop likely was reduced temporarily during the YD. Notice that the observed current rate of SH deepwater formation is poorly determined (19); clearly, the dynamical interaction between Atlantic and Pacific (and Indian Ocean), via the Southern Ocean, requires increased research effort. Concerning the speed of possible transitions, our understanding is very rudimentary as well. The circulation and heat transports can respond to changes in external forcing much faster than on the millennial deep thermodynamic equilibration timescale, but it is not clear which of the various plausible, identifiable timescales, ranging from months to decades, is most relevant (10).

Whether THC changes lead to temperature changes in the NH and SH that are equal in sign and lagged or opposite in sign and synchronous is crucial in deciding whether changes in the THC can initiate ice ages. The major glaciations are global phenomena whereas the immediate consequence of (say) reduced Atlantic THC is likely to be cooling the NH and warming the SH (11). This difficulty is shared with the standard explanation of global ice-age cooling by reduced NH summer insolation, which is concomitant with increased SH insolation (16); possible mechanisms were reviewed recently in ref. 20. The direct effect of the THC is redistribution of energy, but it does not change the global-mean sea surface temperature. Only indirectly, through the nonlinear global-mean radiation balance, can the THC play a significant role in global-mean sea surface temperature changes. These might well occur through sea ice, clouds (both changing the mean reflectivity), CO₂, or water vapor (both changing the atmosphere's opacity to longwave radiation), but even our qualitative understanding of the mechanisms is rudimentary or absent.

One argument says that weaker Atlantic THC increases sea ice cover and hence reflectivity, lowering the temperature. The magnitude of this effect is unclear—of the coupled models that simulate the last glacial maximum, one reports no effect of reduced THC on global mean temperature (21), consistent with ref. 11. The other study obtains a 30% amplification of global cooling and argues (22) that the three-dimensional model analyzed in ref. 11 might have too weak an influence of the THC on the sea ice edge. The jury is still out, as it is on the effect of THC changes on land ice: On the one hand, it can be argued that increased THC makes the high northern latitudes warmer and moister, leading to enhanced snowfall and glacier buildup; on the other hand, glaciers do preferentially grow in colder climates. The very warm Eocene is believed to have been ice-free, for reasons that are likewise unclear.

Dramatic Changes Afoot?

Abrupt climate change may not have been merely a feature of the past but may be induced by the buildup of CO₂ in the atmosphere. Coupled model studies (23) have shown that global warming can lead to a collapse of the North Atlantic THC: Higher atmospheric temperatures lead to a generally wetter atmosphere and hence increased moisture transport from low to high latitudes. The increased precipitation in the North Atlantic leads to reduced surface salinity and density, interrupting deep convection and bringing the Atlantic THC to a halt. As a consequence, northern Europe might cool even under global warming and, more alarming, this cooling might occur much more rapidly than the gradual global warming, thus making adaptation far more difficult. The critical question is, How close to a transition is the real climate system?

Before addressing this directly, let us consider another fundamental point. The projected future weakening of the THC under global warming appears reminiscent of the supposed weaker THC during the last glacial maximum or the YD cooling. But why should both global warming and global cooling cause a reduction in THC? An answer is suggested by an idealized coupled global model that simulated both the transient and the equilibrium responses of the Atlantic THC to global increases in atmospheric moisture transport (12). In the transient case, a doubling in moisture flux over 1,000 years led to the collapse of the THC after a few centuries. In the equilibrium case, however, the doubled freshwater flux led to strongly increased THC (also see Appendix). If the equilibrium response can be interpreted as reflecting the THC's response to very slowly varying atmospheric moisture flux, as might have happened during the glaciations, the glacial weakening of the THC could be explained.

Would We Know Were It Happening Today?

As well as predicting an impending THC collapse, a further problem is detecting such a change. Estimating the strength of the THC is difficult and expensive; the most reliable estimates derive from ship-based transoceanic sections, coast-to-coast and top-to-bottom, with time between repeat sections typically 10 years and more, and a cost of up to half a million dollars per section. The mid-latitude Atlantic THC appears to be quite steady in time (3), but the uncertainty in the estimates is around 25%, resulting largely from the unknown temporal variability. A monitoring strategy for the THC is required that is more cost-effective than ship-borne measurements. It has been suggested (24, 25) to continuously monitor the evolution of density in patches near the “endpoints” of traditional east-west sections; simple and usually well founded ocean dynamics (the thermal wind relationship) suggest that the density difference between the endpoints should be a good indicator of THC strength (26). Whether this is indeed a feasible monitoring strategy remains an outstanding question, which deserves concerted efforts by the theoretical, modeling, and observational communities alike.

A Different Brand of Predictability.

The potential predictability and prediction of the THC raises the thorny issue of assessing the quality of numerical simulations of future climate evolution. The fundamental problem is best understood when juxtaposed with daily weather forecasting. Through thousands of forecasts based on model simulations, it has been established that indeed the predictions have “skill”; that is, they are better than informed guesses. But the time needed to test a weather forecast typically is a day—we'll know tomorrow night whether our picnic gets rained out. An analogous accumulation of evidence for forecast skill is impossible when the prediction lead time is decades and more. How, then, can we state with well defined confidence what is likely in store?

One response (though not a solution) could be that “good decisions, not simply good predictions” matter for policy (ref. 27, p. 309). Still, an abrupt THC change is likely to have massive enough consequences that its probability should be estimated, if only crudely. In lieu of establishing true forecast skill, the models used for climate scenarios should pass all of the tests posed by available data. For example, one would reasonably have more confidence in climate models of future evolution that were able also to simulate the complicated climate history, including the rapid changes, of the last 100,000 years (28). However, it is difficult to establish the “transferability” of putative modeling success of the past; being able to simulate the last ice age is neither strictly necessary nor sufficient for being able to predict the THC's evolution under global warming. Another strategy would be to simulate an ensemble of possible future climates and ascribe probabilities to them. Still, the problem remains of assessing whether these probabilities have the right order of magnitude. A further limit to predictability might arise from chaotic dynamics. In the global model of ref. 12, the timing (and presumably critical threshold) of a THC collapse was fundamentally unpredictable to within a factor of 2. Different realizations of statistically identical random perturbations in the wind field, mimicking different (unpredictable) weather histories, led to collapse times that varied between 250 and 500 years (Fig. 2).

Figure 2.

Time series of the North Atlantic THC strength of an idealized global three-dimensional ocean model. Three sets of random but statistically equivalent wind fluctuations are imposed to mimic different weather histories. In all experiments, the strength of the atmospheric water cycle increases linearly by 10% per century (adapted from ref. 12).

Recommendations.

Abrupt climate change, which could be induced by a THC collapse, poses fundamental unanswered questions of ocean and climate dynamics and predictability. New research avenues should be devoted to detailed comparisons of paleosimulations and paleodata, monitoring the present THC, and THC dynamics in high-resolution ocean models. It remains to be established that the THC has multiple equilibria even in these models. Centuries-long high-resolution coupled simulations appear to be out of reach of current computers, but only barely so, and should be feasible quite soon.

Abbreviations

THC

thermohaline circulation

NH

Northern Hemisphere

SH

Southern Hemisphere

Younger Dryas

YD

A Tale of Two Models.

Fig. 3 shows the conceptual THC model of Stommel (7) in a later, simplified version (29). The model consists of two well mixed boxes (1: high-latitude; 2: low-latitude) of equal volume. The flow strength, q, is related to the density difference by a linear law,

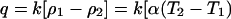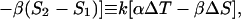1

where k is a hydraulic constant; α and β are the thermal and halince expansion coefficients, respectively. If q > 0, there is poleward surface flow because high-latitude density is greater than low-latitude density, and vice versa. In other words, when q > 0, the temperature difference dominates the density difference and drives the circulation whereas the salinity difference brakes it, and vice versa. In a plausible limiting case, the box temperatures are assumed to be imposed by the atmosphere, as is the surface freshwater exchange, expressed through an equivalent surface salinity flux, H. The conservation equations governing the system then are only those for salinity,

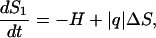2

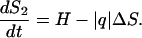3

Subtracting Eq. 2 from Eq. 3 and assuming steady state (marked by an overbar) leads to

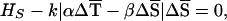4

with solutions

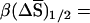5

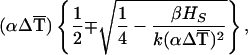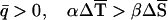(thermally dominated, poleward near-surface flow) and

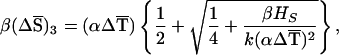6

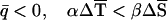(salinity dominated, equatorward near-surface flow; the other root is discarded). It is readily shown that solution 2 in Eq. 5 is unstable. If the radicand in Eq. 5 is positive, this simplest possible model of the THC has two stable equilibria, with sinking either at high or at low latitudes. A closer inspection shows that it is the combination of nonlinearity and different types of forcing for temperature and salinity that creates the multiple equilibria.

Figure 3.

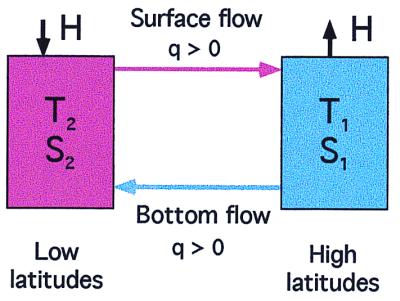
Stommel's conceptual model of the THC.

This model was first formulated in 1961 (7) but went virtually unnoticed for 25 years (30). Meanwhile, in 1982, another box model was independently proposed (ref. 8; Fig. 4) that explained how a two-hemispheric THC symmetric about the equator might become unstable. This result inspired what is arguably the most influential study of the THC (6), but faded out of the public eye owing to the “rediscovery” of ref. 7. It took more than 10 years before the model in Fig. 4 was extensively applied to the steady-state pole-to-pole circulation (31, 32)—which took up only one half-sentence in ref. 8. The model is equivalent to Stommel's except that the flow is assumed to be driven by the pole-to-pole density difference, the equivalent surface salinity fluxes in the two hemispheres differ, and temperature is symmetric about the equator. Assuming flow directions as in Fig. 4, the equations are

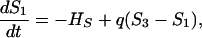7

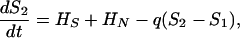8

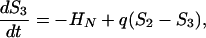9

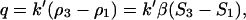10

where k′ is a different hydraulic constant. Insertion of Eq. 10 into Eq. 7 shows that the steady state flow strength is

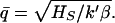11

This result has several remarkable properties. First, the Atlantic THC only depends on SH atmospheric moisture flux (31). Second, the THC increases with increased freshwater flux forcing, in stark contrast with the single-hemispheric box model but consistent with three-dimensional model results, as long as one compares Eq. 11 against the Atlantic component of a global model (12). Third, although H_N does not influence the equilibrium strength of the THC, it can be shown that the q̄ > 0 steady state is unstable if H_N/H_S > 4 (32). If relatively too much freshwater is dumped into the North Atlantic, the “northern sinking” THC cannot be sustained. Finally, if in a three-dimensional model the freshwater forcing keeps increasing (symmetrically about the equator), the increase in Atlantic THC that follows from Eq. 11 eventually should be in conflict with the requirement that all upwelling water must be heated by mixing, and the Atlantic THC might collapse.

Figure 4.

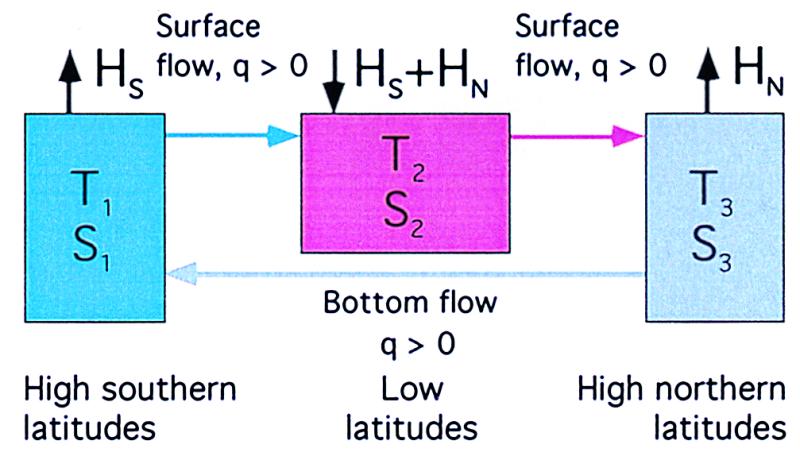
Rooth's interhemispheric conceptual model of the THC.