Skip to main content
PMC home page
NIHPA Author Manuscripts logoLink to NIHPA Author Manuscripts
. Author manuscript; available in PMC: 2013 Mar 1.
Published in final edited form as: Behav Processes. 2011 Oct 14;89(3):197–202. doi: 10.1016/j.beproc.2011.09.010

A fruit in hand is worth many more in the bush: Steep spatial discounting by free-ranging rhesus macaques (Macaca mulatta)

Jerald D Kralik 1,*, William WL Sampson 1
PMCID: PMC3437986  NIHMSID: NIHMS330006  PMID: 22001731

Abstract

Decision making is one of the principal cognitive processes underlying goal-directed behaviour and thus there is justifiably strong interest in modeling it. However, many of these models have yet to be tested outside of the laboratory. At the same time, field work would benefit from the use of experimental methods developed in the laboratory to determine the causal relationships between environmental variables and behaviour. We therefore adapted a laboratory-derived experimental paradigm to test decision making in the wild. The experiment used an indifference-point procedure to determine the influence of both the amount and distance of food on choice behaviour. Free-ranging rhesus monkeys were given the choice between a smaller amount of food at a closer distance and a larger amount farther away. In four conditions, we held the closer amount constant across trials and varied the farther amount to determine the point at which the monkeys were indifferent to the choice alternatives. For example, in condition one, we used one piece of food at the closer location, and determined how many pieces would be equivalent in the farther location. Four different closer amounts were tested to obtain an indifference point curve, with the indifference amounts at the farther location plotted against the closer amounts. The slope of the obtained linear indifference curve was surprisingly high, suggesting that rhesus monkeys significantly discount food that is farther away. Possible reasons for this steep spatial discounting are discussed.

Keywords: Choice, Decision making, Spatial discounting, Reward, Rhesus monkeys

1. Introduction

Decision making is a key process governing goal-directed behaviour, and models of decision making attempt to characterize how cost and benefit parameters influence choice behaviour. For example, with respect to the amount of a positive reward such as food and the delay to receiving it, animals will discount the overall value of the reward if it is not immediately available (Fantino, 1969; Rachlin and Green, 1972; Ainslie, 1974; Mazur and Logue, 1978; Fantino and Davison, 1983; Mazur, 1987; Green and Myerson, 1996). Thus, when choosing between a smaller and larger amount of food, the smaller amount may be preferred if the larger would be received after a significant delay.

To quantify the effects of amount and delay on choice behaviour, evidence has been found for the following relationship:

V=A1+KD (1)

where V is the subjective value of a given option, A is the reward amount, D is the delay to receiving the reward, and K is a free parameter that determines how steeply subjective value changes with delay (e.g. Mazur, 1987, 2000, 2007; Tobin and Logue, 1994). Thus, there is a positive linear relationship between the subjective value of a choice option and the reward amount, and a hyperbolic relationship between subjective value and delay to reward. The hyperbolic relationship captures the fact that the value of a choice option decreases as delay increases, with the discounting becoming less severe with longer delays.

For animals foraging in the wild, delay is often associated with travel distance—individuals normally must travel to obtain food, and traveling takes time and energy (Stephens and Krebs, 1986). Moreover, many choices are based on food sources at known locations and thus the evaluation of a given food source may depend explicitly on the travel distance to the source. One might therefore hypothesize that the relationship of distance to value may also be represented in Eq. (1), with distance replacing delay. That is, one would expect value to decrease with increasing distance, and the severity of this discounting might diminish with increasing distances. We will consider this formulation of the influence of distance on choice – where delay is replaced by distance in Eq. (1) – as Model 1 (see Bateson and Kacelnik, 1996; Janson, 2007).

Other studies have found evidence for the addition of a parameter to Eq. (1):

V=A1+KDB (2)

where B is a scaling factor or represents other factors such as effort or risk (Mazur and Kralik, 1990; McKerchar et al., 2009; Rachlin, 2006). In this second model, we will again consider D to be distance to the food source.

We conducted the current study to determine whether the value of food at a distance is better represented by Model 1 or 2, in cases where animals are making discrete choices before traveling. The experiment was also conducted to determine if models of decision making developed in the laboratory apply to animal behaviour in more natural environments. This ecological validation is critical because laboratory research has uncovered important findings that have yet to be verified in the field. Furthermore, much of the research in the development of these models was conducted on pigeons and rats, and thus we also conducted the experiment to determine if such models also applied to an Old World monkey, the rhesus macaque (Macaca mulatta) (also see Hayden and Platt, 2007; Janson, 2007; Kim et al., 2008; Stevens et al., 2005a,b; Szalda-Petree et al., 2004; Tobin et al., 1996). Finally, the current experiment was conducted to test whether a specific experimental procedure used in the laboratory to quantify the relationship of economic variables to choice behaviour could also be used in the field: Mazur's (1987) indifference point procedure, described in Section 2.2. If successful, it would provide another experimental paradigm with quantitative rigor to study decision making in the wild (also see Janson, 2007).

2. Methods

2.1. Subjects

We tested adult and subadult male rhesus macaques on Cayo Santiago in Puerto Rico. Subadult males were males estimated to be between three and five years of age, whose body size was approximately as large as those of adult males, but whose testes had not completely descended.

2.2. Indifference point procedure

To test between the two models, we utilized an indifference point procedure (Mazur, 1987). The procedure is a popular means for testing decision-making models because it takes advantage of the fact that when one is indifferent between two choice alternatives, the equations for each alternative are set equal to each other, which cleverly removes value, V, a variable that is difficult to measure objectively, and leaves variables such as amount and distance that can be objectively measured. Additionally, it leads to specific indifference point function predictions that can be tested experimentally. The procedure works as follows: when an individual is indifferent between two choice options 1 and 2, the value, V1, of option 1 is equal to the value, V2, of option 2. Using Eq. (1), in the case in which V1 = V2, we have

A11+KD1=A21+KD2 (3)

If we then solve for A2 as a function of A1, we obtain

A2=(1+KD21+KD1)A1 (4)

Thus, the first model, Eq. (1), predicts that the indifference points A2 are a linear function of the corresponding values of A1, with a slope of (1 + KD2) / (1 + KD1), and a y-intercept of zero. Following the same procedure, the second model, Eq. (2), also predicts a linear indifference point relationship, however, the predicted slope is (1+KD2B)∕(1+KD1B).

Evidence for species-typical discounting that likely evolved due to specific ecological or social conditions suggests that it is meaningful to obtain model parameters that capture the general discounting rate across individuals within a species (Green et al., 2004; Mazur, 2000, 2007; Rosati et al., 2007; Stevens et al., 2005a). Clear, systematic results from data collected across subjects would also support the concept of an overall species-typical effect. We therefore tested this possibility by conducting the experiment across multiple subjects. Nonetheless, to minimize individual differences, we tested peripheral males who in general share a comparable position in the social structure of the Cayo Santiago monkeys.

2.3. Test conditions

The experiment consisted of four conditions. In each condition, the amount of food at the closer location remained constant, and the amount at the farther location was increased or decreased across trials to determine the indifference point between the two alternatives – that is, to determine the amount at the farther location that would result in the monkeys, on average, choosing either location 50% of the time. Macintosh apples were used and cut into 1/16 slices. For Conditions 1–4, the amounts at the closer location were 1, 2, 3 and 4 slices, respectively. We used predetermined amounts for the farther location (see below), with ten trials per amount. It is important to note that we did not compile the results until after we conducted all trials of the experiment, thus we were essentially blind to the experimental outcome while conducting the experiment. Finally, to minimize repeated trials with specific monkeys, we tested different monkeys in every block of ten trials, and tested different individuals on any given day (systematically testing monkeys on different parts of the island throughout the day). Thus, no individual monkey should have been tested on more than six trials in the experiment.

2.4. Testing procedure

When we spotted a lone individual, we approached to a distance of 3.1 m (10 ft) and 4.6 m (15 ft) from the monkey, yielding a distance ratio of 1:1.5, and we positioned ourselves so that we formed a 90° angle with the monkey as the vertex (Fig. 1). The two distances were switched at regular intervals across trials from left to right of the monkey, such that each was to the left and right five times within every 10 trials. Once in position, we simultaneously knelt on our right knees, and placed a tray on the ground (white, 30.5 cm length × 20.3 width × 2.5 thickness, rectangular Styrofoam boards attached to a wicker place mat underneath to keep the trays from blowing away) (Fig. 1).

Fig. 1.

Fig. 1

Open in a new tab

Images showing the general test procedure. (A) Experimenters at different distances from the monkey displayed different amounts of apple slices. (B) Once the monkey saw both options, the experimenters placed the food items on the trays then turned and walked away to allow the monkey to choose. (C) A clearer view of the different distances of the experimenters from the monkey (10 and 15 ft) as well as the angle between the experimenters (90°). (D) Illustration of the testing paradigm, with A1 the closer, smaller amount and A2 the farther, larger amount.

We then removed the apple slices from our backpacks and presented them simultaneously to the monkey. After the monkey had clearly looked at both options, we simultaneously placed the food items down on the trays, stood up, turned around and walked away from the trays. The monkeys typically took a straight line to one of the two trays immediately after we stepped away from them. If a monkey did not touch at least one of the apple slices of a choice alternative, either due to approaches by conspecifics or other intervening factors (e.g. a loud noise), the trial was considered a “no response” and aborted; 11% of trials were aborted. The entire procedure took approximately 10–15 s. When the number of slices was 12 or more we presented them to the monkeys in a clear zip lock bag and then laid them out on the trays after removing them from the bag. The monkeys’ behaviour did not appear to be affected by the clear bags themselves, and the systematic results support this lack of effect.

The testing procedure was developed during initial pilot testing. Since the monkeys on Cayo Santiago are generally not afraid of the experimenters, we chose distances at which the monkeys typically refrained from approaching until after the experimenters stepped back. In addition, we chose an angle between the two alternatives in which the monkeys appeared to be choosing one alternative or the other – they could not easily move from one to the other without going out of their way. Although one might be concerned that the choice posed to the monkeys was artificial, given that they might typically be able to go to both food sources, and thus they may not be deciding between the two, several factors argue against this possibility. Most notably, in their semi-wild social environment, food sources are volatile, especially due to conspecific competition, and one is never guaranteed that the second source will remain. In addition, the monkeys rarely attempted to approach the second tray after making their choice. They typically approached the tray and left it quickly, after grabbing the apple slices, or else they sat and ate at the chosen tray when there were multiple slices. We will return to the issue of binary choices versus path planning in Section 4.

3. Results

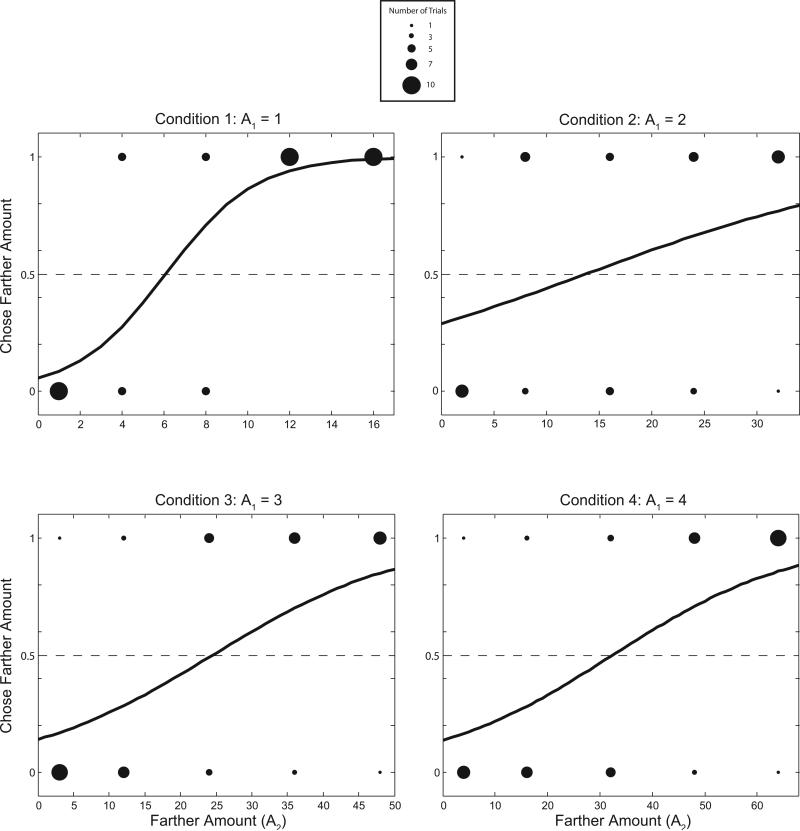
The results are shown in Table 1. Overall, for every condition the monkeys’ behaviour reflected the titration procedure: with the smallest amounts of apple slices on the farther tray, the monkeys chose the closer location most of the time; as the amount on the farther tray increased, the monkeys chose the farther location more frequently. The results for all four conditions are plotted in Fig. 2. Each graph plots every trial of the condition, with the farther amount along the x-axis and each dot representing the number of times the farther amount was or was not chosen (1 = Yes, 0 = No). The curve in each graph is the logistic regression fit to the data points (Condition 1: χ2(1) = 32.16, P < 0.001; Condition 2: χ2(1) = 5.73, P = 0.017; Condition 3: χ2(1) = 13.84, P < 0.001; Condition 4: χ2(1) = 14.29, P < 0.001). Again, for every condition one can see that the monkeys’ behaviour reflected the titration procedure (selecting the closer tray with smaller amounts on the farther tray, and switching progressively to the farther tray with larger amounts).

Table 1.

Results for all four test conditions. A1 represents the amount of apple slices on the closer tray for each condition.

Condition Amount on farther tray (A2) Closer chosen (number of trials) Farther chosen (number of trials) % Farther chosen
A1 = 1 1 10 0 0
4 5 5 50
8 5 5 50
12 0 10 100
16 0 10 100
A1 = 2 2 8 2 20
8 4 6 60
16 5 5 50
24 4 6 60
32 2 8 80
A1 = 3 3 9 1 10
12 7 3 30
24 4 6 60
36 3 7 70
48 2 8 80
A1 = 4 4 8 2 20
16 7 3 30
32 6 4 40
48 3 7 70
64 1 9 90
Open in a new tab

Fig. 2.

Fig. 2

Open in a new tab

The results for each experimental condition, in which the closer tray amount (A1) was 1–4. On each graph, the data points represents the number of trials in which the farther alternative was chosen (1 = Yes, 0 = No) for the different number of apple slices at the farther alternative. The curves are the logistic regression fits to the data. The intersections between the dashed line at .5 and the curves are the points at which the monkeys would be expected to choose the closer and farther trays equally often (i.e. the indifference points). The legend shows examples of dot sizes corresponding to number of trials (exact numbers in Table 1).

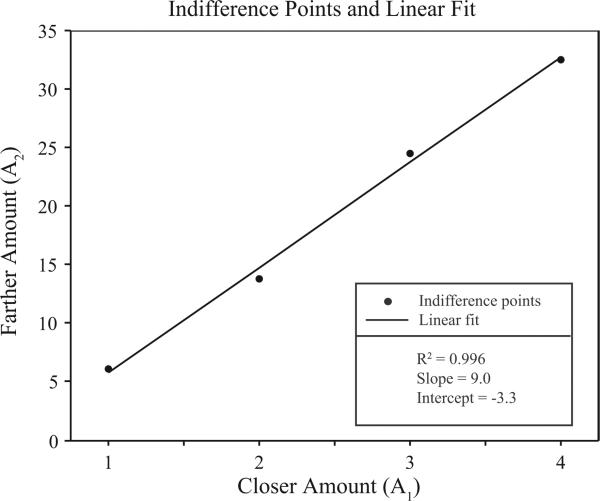
The intersections between the dashed line at 0.5 and the logistic regression curves are the points at which the monkeys would be expected to choose the closer and farther trays equally often (i.e. the indifference points). The resulting A2 indifference points for each A1 condition (1–4) were 6.1, 13.7, 24.5, and 32.5 (Fig. 2). The indifference points (A2) were then plotted against the corresponding closer tray amounts (A1) to obtain the indifference point curve in Fig. 3 (solid line). A general linear fit to these data points accounted for 99.6% of the variance (linear regression, P = 0.002). The slope of this linear fit was 9 and the intercept was –3.3, meaning that each apple slice on the closer tray was equivalent to 5.7 slices on the farther tray.

Fig. 3.

Fig. 3

Open in a new tab

Indifference point results. Indifference points for the farther tray reward amounts (A2) plotted against the corresponding amounts on the closer tray (A1) and the linear regression fit (line).

We next compared the predictions of Models 1 and 2 by determining how well the indifference point curves derived from these models fit the obtained results. Model 1 was unable to account for such a high slope of the line. That is, the slope of the predicted indifference point line for Model 1 is equal to (1 + 15K)/(1 + 10K), which at best can reach 1.5 (the ratio of the two distances used in the experiment, 15 and 10 ft). Thus, Model 1 is unable to account for an indifference curve with a slope greater than 1.5.

For Model 2, the fit of the indifference point curve accounted for 97.8% of the variance. For the best linear fit, the B parameter in the model was equal to 5.1 (and K = 10). It should be noted that although Model 2 appears to have two free parameters, B and K, B was the parameter that allowed the model to fit the high slope of the indifference point data. That is, without the exponent, Model 2 reduces to Model 1, and would suffer the same inability to fit a slope greater than 1.5. Put differently, the indifference point curve derived from Model 2 was able to account for 97.8% of the variance regardless of the value of K (for K ≥ .1).

4. Discussion

We found a linear indifference point curve with a slope (9) significantly higher than the ratio of the two distances (1.5). This degree of devaluation appeared steep, given that the monkeys were only required to travel an additional five feet to obtain the larger amount of food, and yet more than 32.7 apple slices on the farther tray was necessary for them to prefer the farther tray with 4 apple slices on the closer one. Model 2 was able to account for this result, Model 1 could not, which provides further evidence for Eq. (2) as a model of decision making. The model's predictive power has also been extended to a more natural setting and to an Old World primate, the rhesus macaque, providing a quantitative description of how amount and distance influence decision making under naturalistic conditions.

More generally, the current results show that any model of rhesus monkey choice behaviour over short distances with the food items in view must account for the linear indifference point curve we obtained. Other models that fall into this class include related formulations such as V = A/(1 + KD)B (Green et al., 1994; Green and Myerson, 2004; Haynes and Fotheringham, 1984; Janson, 2007; Myerson and Green, 1995).

The high indifference point slope we obtained was unexpected. Even though we turned and walked away, it is possible that the monkeys tended to select the closer option to avoid the experimenters. We believe this is unlikely, however, given that the monkeys on Cayo Santiago are acclimated to people, and researchers tend to have the opposite problem with them: keeping them (and other bystander monkeys) from approaching prematurely. We also do not believe the finding was due to a lack of visibility of all apple slices. To minimize this possibility, we conducted numerous practice trials and made sure to spread out the apple slices during presentation and on the tray.

We have assumed that the rhesus monkeys were making a binary choice between the closer and farther food trays, however, it remains possible that their actual choice was between (a) selecting only the farther tray and (b) first making a detour to the closer tray then proceeding to the farther one (Janson, 2007; Janson and Byrne, 2007). In Section 2.4 we discussed why the monkeys did not appear to be attempting to obtain the food from both trays (such as typically approaching one option directly, grabbing the food, and immediately exiting or else sitting at the chosen site). Nevertheless, it is conceivable that they were. However, models of route planning, such as those that would compare the values of (a) the farther option (e.g. A2/D2) versus (b) the closer option plus traveling from the closer option to the farther one (e.g.(A1+A2)∕(D1+D12+D22)), also do not appear to account for the indifference point slope obtained here. Nonetheless, our results are generally similar to those obtained with capuchin monkeys (Cebus apella), in which they also appeared to select the closer feeding site first, even with up to 12 times more food at the farther site (Janson, 2007). Further work will need to characterize the similarities and differences between the studies. For example, route planning may be more likely with larger distances between sites and lower levels of immediate competition for the food.

Three other factors could potentially underlie the steep spatial discounting that we found: delay, effort, or risk. However, there are reasons to doubt that delay would account for the entire effect. Although response times were not recorded in the current study, they were measured in a pilot version of the experiment. For distances of 1.8 m (6 ft) and 2.7 m (9 ft), the average delay values were 4.1 and 5.9 s, respectively. Assuming a linear relationship between distance and delay, the delays for 3.1 m (10 ft) and 4.6 m (15 ft) in the current study would be 6.5 and 9.5 s, respectively. A difference on the order of 3 s between the two alternatives is unlikely to cause the steep discounting of the farther distance (Evans and Beran, 2007; Szalda-Petree et al., 2004; Tobin et al., 1996).

The second factor potentially underlying the steep spatial discounting is the effort required to travel to the food source (see Janson, 2007; Mazur and Kralik, 1990). Although effort undoubtedly plays a major role in foraging decisions over large distances (Janson, 2007; Janson and Byrne, 2007), it seems less likely to account for the current results, especially given (a) the relatively short distances that we tested, (b) the fact that the indifference point slope was much higher than the ratio of the two distances, and (c) the attention the monkeys appeared to give to nearby conspecifics. The latter observation relates to the third possibility that uncertainty and risk may underlie the steep spatial discounting: the farther a food source, the less likely it will remain before one arrives (e.g. Caraco et al., 1980; Staddon, 1980; Stephens and Krebs, 1986; Rachlin, 1989; Bateson and Kacelnik, 1995; Kacelnik and Bateson, 1997; Krebs and Davies, 1997). Risk may be especially heightened given the social conditions of the monkeys. In general, rhesus monkeys live in large social groups with relatively high competition and potential conflict (Thierry et al., 2004). Such conditions could have selected for decision-making processes that attempt to reduce risk and obtain the most food as quickly as possible (see Cunningham and Janson, 2007). Further work will need to tease apart and directly test these possible factors underlying the distance effect found here.

Although we obtained highly consistent results, we do suspect that significant individual differences exist, especially across different ages, sexes and dominance ranks, and these potential differences should also be examined in the future. Indeed, the indifference point procedure could prove even more powerful if conducted as a within-subjects experiment, in which indifference points are obtained for each monkey. Field logistics, however, sometimes preclude such within-subjects designs, and it is encouraging to find that consistency can be obtained using an across-subjects design. The consistent findings thus support the concepts of species-typical decision making and species-level discounting factors, notwithstanding significant individual differences that certainly exist.

The current experiment showed that the value of food rewards is highly discounted over distance for free-ranging rhesus monkeys. Although this behaviour might appear relatively impulsive, it might also reflect prudence in the wild and in a social setting. Whether this behaviour reflects an underlying evolutionary adaptation, or learning, or some combination of both remains unknown. Whichever way, our findings add to the growing literature demonstrating important contextual and species-typical effects on decision making (Cunningham and Janson, 2007; Green et al., 2004; Hayden et al., 2008; Hayden and Platt, 2007; Janson, 2007; Janson and Byrne, 2007; Mazur, 2000, 2007; Rosati et al., 2007; Stevens et al., 2005a).

The experiment also provided evidence for Model 2 (Eq. (2)) as a description of the value of a choice option based on the amount and distance of a food source. Thus, the explanatory power of Eq. (2) generalizes from choice behaviour in the laboratory to rhesus monkeys in more natural conditions. The successful application of the indifference point procedure provides another example of what experimental manipulations are possible in the wild, and what level of quantification can be achieved (Janson, 2007; Janson and Byrne, 2007). Such experimental paradigms can help provide a more detailed understanding of the economic and social pressures facing decision makers in naturalistic conditions.

Acknowledgements

The project was supported by Grant Number CM-5 P40 RR003640-20 from the National Center for Research Resources (NCRR), a component of the National Institutes of Health (NIH), and the contents are solely the responsibility of the authors and do not necessarily represent the official views of NCRR or NIH. The experiment complied with all current laws and regulations of Puerto Rico and USA.

References

  1. Ainslie GW. Impulse control in pigeons. Journal of the Experimental Analysis of Behavior. 1974;21(3):485–489. doi: 10.1901/jeab.1974.21-485. [DOI] [PMC free article] [PubMed] [Google Scholar]
  2. Bateson M, Kacelnik A. Preferences for fixed and variable food sources: Variability in amount and delay. Journal of the Experimental Analysis of Behavior. 1995;63(3):313–329. doi: 10.1901/jeab.1995.63-313. [DOI] [PMC free article] [PubMed] [Google Scholar]
  3. Bateson M, Kacelnik A. Rate currencies and the foraging starling: the fallacy of the averages revisited. Behavioral Ecology. 1996;7(3):341–352. [Google Scholar]
  4. Caraco T, Martindale S, Whittam TS. An empirical demonstration of risk-sensitive foraging preferences. Animal Behaviour. 1980;28(AUG):820–830. [Google Scholar]
  5. Cunningham E, Janson C. A socioecological perspective on primate cognition, past and present. Animal Cognition. 2007;10(3):273–281. doi: 10.1007/s10071-007-0078-3. [DOI] [PubMed] [Google Scholar]
  6. Evans TA, Beran MJ. Delay gratification and delay maintenance by rhesus macaques (Macaca mulatta). Journal of General Psychology. 2007;134(3):331–332. doi: 10.3200/GENP.134.2.199-216. [DOI] [PubMed] [Google Scholar]
  7. Fantino E. Choice and rate of reinforcement. Journal of the Experimental Analysis of Behaviour. 1969;12:723–730. doi: 10.1901/jeab.1969.12-723. [DOI] [PMC free article] [PubMed] [Google Scholar]
  8. Fantino E, Davison M. Choice: Some quantitative relations. Journal of the Experimental Analysis of Behavior. 1983;40(1):1–13. doi: 10.1901/jeab.1983.40-1. [DOI] [PMC free article] [PubMed] [Google Scholar]
  9. Green L, Fry AF, Myerson J. Discounting of delayed rewards: a life-span comparison. Psychological Science. 1994;5(1):33–36. [Google Scholar]
  10. Green L, Myerson J. Exponential versus hyperbolic discounting of delayed outcomes: Risk and waiting time. American Zoologist. 1996;36(4):496–505. [Google Scholar]
  11. Green L, Myerson J. A discounting framework for choice with delayed and probabilistic rewards. Psychological Bulletin. 2004;130(5):769–792. doi: 10.1037/0033-2909.130.5.769. [DOI] [PMC free article] [PubMed] [Google Scholar]
  12. Green L, Myerson J, Holt DD, Slevin JR, Estle SJ. Discounting of delayed food rewards in pigeons and rats: is there a magnitude effect? Journal of the Experimental Analysis of Behavior. 2004;81(1):39–50. doi: 10.1901/jeab.2004.81-39. [DOI] [PMC free article] [PubMed] [Google Scholar]
  13. Hayden BY, Heilbronner SR, Nair AC, Platt ML. Cognitive influences on risk-seeking by rhesus macaques. Judgment and Decision Making Journal. 2008;3(5):389–395. [PMC free article] [PubMed] [Google Scholar]
  14. Hayden BY, Platt ML. Temporal discounting predicts risk sensitivity in rhesus Macaques. Current Biology. 2007;17(1):49–53. doi: 10.1016/j.cub.2006.10.055. [DOI] [PMC free article] [PubMed] [Google Scholar]
  15. Haynes KE, Fotheringham AS. Gravity and Spatial Interaction Models. Sage Publication; Newbury Park: 1984. [Google Scholar]
  16. Janson CH. Experimental evidence for route integration and strategic planning in wild capuchin monkeys. Animal Cognition. 2007;10(3):341–356. doi: 10.1007/s10071-007-0079-2. [DOI] [PubMed] [Google Scholar]
  17. Janson CH, Byrne R. What wild primates know about resources: opening up the black box. Animal Cognition. 2007;10(3):357–367. doi: 10.1007/s10071-007-0080-9. [DOI] [PubMed] [Google Scholar]
  18. Kacelnik A, Bateson M. Risk-sensitivity: Crossroads for theories of decision-making. Trends in Cognitive Sciences. 1997;1:304–309. doi: 10.1016/S1364-6613(97)01093-0. [DOI] [PubMed] [Google Scholar]
  19. Kim S, Hwang J, Lee D. Prefrontal coding of temporally discounted values during intertemporal choice. Neuron. 2008;59(1):161–172. doi: 10.1016/j.neuron.2008.05.010. [DOI] [PMC free article] [PubMed] [Google Scholar]
  20. Krebs JR, Davies NB. Behavioural ecology. Blackwell Science; Oxford: 1997. [Google Scholar]
  21. Mazur JE. An adjusting procedure for studying delayed reinforcement. In: Rachlin H, editor. Quantitative Analyses of Behaviour: The Effect of Delay and of Intervening Events on Reinforcement Value. Lawrence Erlbaum Associates; Hillsdale, NJ: 1987. pp. 55–73. [Google Scholar]
  22. Mazur JE. Tradeoffs among delay, rate, and amount of reinforcement. Behavioural Processes. 2000;49(1):1–10. doi: 10.1016/s0376-6357(00)00070-x. [DOI] [PubMed] [Google Scholar]
  23. Mazur JE. Species differences between rats and pigeons in choices with probabilistic and delayed reinforcers. Behavioural Processes. 2007;75(2):220–224. doi: 10.1016/j.beproc.2007.02.004. [DOI] [PubMed] [Google Scholar]
  24. Mazur JE, Kralik JD. Choice between delayed reinforcers and fixed-ratio schedules requiring forceful responding. Journal of the Experimental Analysis of Behavior. 1990;53(1):175–187. doi: 10.1901/jeab.1990.53-175. [DOI] [PMC free article] [PubMed] [Google Scholar]
  25. Mazur JE, Logue AW. Choice in a self-control paradigm: Effects of a fading procedure. Journal of the Experimental Analysis of Behavior. 1978;30(1):11–17. doi: 10.1901/jeab.1978.30-11. [DOI] [PMC free article] [PubMed] [Google Scholar]
  26. McKerchar TL, Green L, Myerson J, Pickford TS, Hill JC, Stout SC. A comparison of four models of delay discounting in humans. Behavioural Processes. 2009;81(2):256–259. doi: 10.1016/j.beproc.2008.12.017. [DOI] [PMC free article] [PubMed] [Google Scholar]
  27. Myerson J, Green L. Discounting of delayed rewards: Models of individual choice. Journal of the Experimental Analysis of Behavior. 1995;64(3):263–276. doi: 10.1901/jeab.1995.64-263. [DOI] [PMC free article] [PubMed] [Google Scholar]
  28. Rachlin H. Judgment, Decision, and Choice. W. H. Freeman; New York: 1989. [Google Scholar]
  29. Rachlin H. Notes on discounting. Journal of the Experimental Analysis of Behavior. 2006;85(3):425–435. doi: 10.1901/jeab.2006.85-05. [DOI] [PMC free article] [PubMed] [Google Scholar]
  30. Rachlin H, Green L. Commitment, choice and self-control. Journal of the Experimental Analysis of Behavior. 1972;17(1):15–20. doi: 10.1901/jeab.1972.17-15. [DOI] [PMC free article] [PubMed] [Google Scholar]
  31. Rosati AG, Stevens JR, Hare B, Hauser MD. The evolutionary origins of human patience: temporal preferences in chimpanzees, bonobos, and human adults. Current Biology. 2007;17(19):1663–1668. doi: 10.1016/j.cub.2007.08.033. [DOI] [PubMed] [Google Scholar]
  32. Staddon JER. Limits to action: The allocation of individual behaviour. Academic Press; New York: 1980. [Google Scholar]
  33. Stephens DW, Krebs JR. Foraging Theory. Princeton: Princeton University Press. 1986 [Google Scholar]
  34. Stevens JR, Hallinan EV, Hauser MD. The ecology and evolution of patience in two New World monkeys. Biology Letters. 2005a;1(2):223–226. doi: 10.1098/rsbl.2004.0285. [DOI] [PMC free article] [PubMed] [Google Scholar]
  35. Stevens JR, Rosati AG, Ross KR, Hauser MD. Will travel for food: spatial discounting in two new world monkeys. Current Biology. 2005b;15(20):1855–1860. doi: 10.1016/j.cub.2005.09.016. [DOI] [PMC free article] [PubMed] [Google Scholar]
  36. Szalda-Petree AD, Craft BB, Martin LM, Deditius-Island HK. Self-control in rhesus macaques (Macaca mulatta): controlling for differential stimulus exposure. Perceptual and Motor Skills. 2004;98(1):141–146. doi: 10.2466/pms.98.1.141-146. [DOI] [PubMed] [Google Scholar]
  37. Thierry B, Singh M, Kaumanns W, editors. Macaque Societies: A Model for the Study of Social Organization. Cambridge University Press; Cambridge, UK: 2004. [Google Scholar]
  38. Tobin H, Logue AW. Self-control across species (Columba livia, Homo sapiens, and Rattus norvegicus). Journal of Comparative Psychology. 1994;108(2):126–133. doi: 10.1037/0735-7036.108.2.126. [DOI] [PubMed] [Google Scholar]
  39. Tobin H, Logue AW, Chelonis JJ, Ackerman KT, May JG. Self-control in the monkey Macaca fascicularis. Animal Learning & Behavior. 1996;24(2):168–174. [Google Scholar]

RESOURCES