Molecular structures from femtosecond x-ray pulses

In this issue of PNAS Miao, Hodgson, and Sayre (1) validate a method of three-dimensional structure determination for individual biological macromolecules. Their proposal, based on suggestions by Hajdu and colleagues (2), is supported by careful computer simulation using plausible assumptions, and rests on recent advances in a number of related fields. In brief, they adopt the idea that femtosecond (fsec) x-ray pulses generated by free electron, laser-based x-ray sources will allow the two-dimensional (2D) diffraction patterns (DPs) of individual molecules to be recorded (2). Appropriate registration and averaging of these patterns will allow the full three-dimensional DP of the molecule to be assembled into an effectively continuous function. Previous work by Sayre and colleagues (3, 4) and others (5) has shown that, for objects that are everywhere positive and that are confined to an envelope of known size, the phases of the continuous diffraction pattern can be recovered. Thus, an image of the molecule can be formed by Fourier transformation of the phased diffraction pattern. The quality of the images produced in the simulation is quite high, and generated a burst of pleasure and optimism in this commentator.

Miao, Hodgson, and Sayre weave together strands spun out from the rapid progress in a variety of fields. Of these the newest is the conceptual design of a family of fourth generation synchrotrons that are based on the principles of the free electron laser, and that produce 100-fsec x-ray pulses in the 10 kev energy range (ref. 6 and the Linear Coherent Light Source web site, http://www-ssrl.slac.stanford.edu/lcls/). These pulses, termed x-ray free electron laser (XFEL) radiation, will have a peak brilliance ≈10⁸ times that of current third generation synchrotrons, such as the Advanced Photon Source. XFEL radiation also has substantial coherence. However, this is not a key ingredient of the Miao, Hodgson, and Sayre proposal. Miao, Hodgson, and Sayre visualize that a single XFEL pulse irradiating an individual macromolecule could give rise to an observable 2D DP before radiation damage manifests itself. The dominant mechanism of damage on the fsec timescale involves Coulombic repulsion between positively charged atoms that have lost electrons through interaction with XFEL photons. Calculations show that the accelerations associated with these forces are not great enough to seriously perturb the molecular structure during the time interval of the pulse. However to maintain consistency with other work, Miao, Hodgson, and Sayre assume a 10-fsec pulse in their work, somewhat beyond the current state-of-the art.

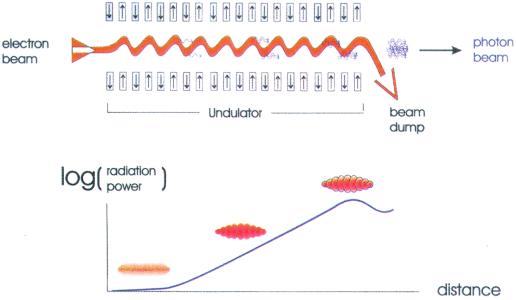
Fig. 1, taken from the technical design report of the TESLA XFEL facility proposed for Deutsches Elektronen Synchrotron (DESY; ref. 6), illustrate how XFEL radiation is generated.

Figure 1.

Schematic diagram of a single-pass free electron laser operating in the Self-Amplified-Spontaneous Emission (SASE) mode. In an XFEL a short, high-energy electron pulse emerging from a linear accelerator is injected into the undulator (Upper). The alternating pattern of N and S magnetic poles in the undulator causes the beam to execute a small amplitude harmonic motion in and out of the plane of the page. This motion represents acceleration of the electrons that causes them to radiate. As the bunch passes down the undulator the interaction of the radiated field with the bunch causes microbunching of the electron pulse (Lower). The electrons in the developing micro bunches eventually radiate coherently, and the number of emitted photons grows exponentially. This is the SASE mode, and it depends critically on the very high instantaneous currents present in the pulses. Note that in reality the number of slices is much larger than shown. [Reproduced with permission from ref. 6 (Copyright 2001, Deutsche Elektronen-Synchrotron).]

The DP recorded from a single molecule represents a section of the full 3D DP, sampled on a spherical shell (the Ewald sphere). To assemble a full 3D DP, 2D DPs of individual molecules in all possible orientations must be recorded, and the DPs properly aligned. The alignment issue is a thorny one. Miao, Hodgson, and Sayre present two scenarios for this, one in which molecules are exposed at random, with the orientations recovered later. The other, more speculative approach is to drive each molecule to a known orientation by using an elliptically polarized and nonresonant laser field. The first scenario has precedents in the alignment of multiple particle images in electron microscopy, and plausible arguments are made that the methods can be extended to their case.

The observed 3D DP is the squared modulus of the Fourier transform of the electron density function of an individual molecule. To produce an image, the phases associated with the underlying complex DP must be known. This is the famous phase problem of x-ray crystallography, reduced to a single molecule level. Fortunately, the problem of finding the phase for a continuous (or finely sampled) DP is more tractable than that for the more coarsely sampled DPs from crystals. Sayre has been involved with this problem for many years. In 1952 he showed that in the special case of centrosymmetric crystals, the phases, which are restricted to be 0° or 180°, could be determined by a simple algorithm (7). This requires that the DP of the crystal be known both at the normal Bragg peaks and the unobservable points halfway between. More recently this work has been extended to noncentrosymmetric structures, such as proteins. Measuring the continuous DP of one molecule gives access to the points between the Bragg peaks, which are hidden in crystal diffraction.

The physical basis of the phase recovery algorithm is that the electron density in the region surrounding the molecular envelope is known to have the constant value zero, and that the electron density within the envelope is nonnegative. This allows an iterative process for phase determination, starting with a randomly chosen set of phases: (i) Make a map by using the observed DP modulus and current phases. (ii) Modify the map by zeroing it outside the envelope, and making it nonnegative within. (iii) Calculate the DP from this modified map. If not converged, use phases from this step and go back to step i. Simulations carried out during the last decade show that the phase recovery procedure is robust even in the face of reasonable errors in the magnitude of the DP.

In the absence of existing hardware, a proposal like that of Miao, Hodgson, and Sayre has many imponderables, and could fail in detail at a number of points. Hitherto, however, conversations about FLEX radiation have centered on the issue of how such a brilliant source could ever be useful in biology, and on how to protect specimens from its awesome power. Here at last is a proactive and exciting idea, well supported by realistic simulations, that harnesses and even requires the FLEX characteristics. It will surely transform the debate on the utility of FLEX in molecular structural studies.