Skip to main content
PMC home page
Scientific Reports logoLink to Scientific Reports
. 2012 Sep 25;2:690. doi: 10.1038/srep00690

N-type graphene induced by dissociative H2 adsorption at room temperature

Byung Hoon Kim 1,6, Sung Ju Hong 2,6, Seung Jae Baek 2, Hu Young Jeong 3, Noejung Park 1, Muyoung Lee 1, Sang Wook Lee 4, Min Park 2, Seung Wan Chu 2, Hyeon Suk Shin 1, Jeongmin Lim 1, Jeong Chul Lee 5, Yongseok Jun 1,a, Yung Woo Park 2,b
PMCID: PMC3457033  PMID: 23012645

Abstract

Studies of the interaction between hydrogen and graphene have been increasingly required due to the indispensable modulation of the electronic structure of graphene for device applications and the possibility of using graphene as a hydrogen storage material. Here, we report on the behaviour of molecular hydrogen on graphene using the gate voltage-dependent resistance of single-, bi-, and multi-layer graphene sheets as a function of H2 gas pressure up to 24 bar from 300 K to 345 K. Upon H2 exposure, the charge neutrality point shifts toward the negative gate voltage region, indicating n-type doping, and distinct Raman signature changes, increases in the interlayer distance of multi-layer graphene, and a decrease in the d-spacing occur, as determined by TEM. These results demonstrate the occurrence of dissociative H2 adsorption due to the existence of vacancy defects on graphene.

Graphene has been regarded as the successor of silicon in the electronics industry due to its remarkably high carrier mobility, transparency, anomalous quantum Hall effect1,2,3,4, and exceptionally high Young's modulus5. However, the electronic structure of graphene, which features gap opening and n- and p-type properties, must be controlled to achieve this demand. One of the methods used to control the properties of graphene is hydrogenation. Hydrogenated graphene, known as graphane, is obtained from hydrogen plasma and shows a hole-doping effect, a reversible insulating state, and the disappearance of the quantum Hall effect6,7,8,9,10. Along with the hydrogenation of graphene, considerable attention has been paid to the adsorption behaviour of molecular hydrogen on graphitic materials, as well as the interaction between atomic hydrogen and graphene, because multilayered graphene has been proposed as a good candidate for hydrogen storage11,12,13,14,15. For physisorption, the attraction between H2 and graphene occurs via overlapping of the σ molecular orbital of H2 with the electrons in the π state of graphene, with no charge transfer16. However, the dissociative adsorption of hydrogen molecules on a graphite surface with vacancies17 and at the armchair edge of graphite18 has been theoretically suggested. Herein, we report on the dissociative adsorption of hydrogen with gate-voltage (VG)-dependent current variations of single-, bi-, and multi-layer graphene (SLG, BLG, and MLG, respectively) as a function of H2 gas pressure from vacuum (approximately 10−6 Torr) to 24 bar at temperatures T ≥ 300 K. As the H2 gas pressure increases, the charge neutrality point (CNP) shifts toward the negative VG region (n-doping). This charge neutrality point does not return to the origin, even after degassing is performed (vacuum and heating at 393 K). Long exposure times and high temperatures in a H2 atmosphere enhance the n-doping effect. H2 exposure leads to the development of defect-related D (approximately 1350 cm−1) and D' (approximately 1620 cm−1) peaks and a peak corresponding to the C-H bond (approximately 2932 cm−1), a blueshift in the G peak, and a change in stacking order deleted by the variation in the 2D peak, as observed in Raman spectra. An increase in the interlayer distance of MLG was observed by atomic force microscopy (AFM) and X-ray diffraction (XRD). Transmission electron microscopy (TEM) showed in-plane compression after H2 exposure. We also found that the extent of CNP variation is related to the number of graphene layers present. These results show that dissociative H2 adsorption is mainly caused by the vacancy defects of graphene, thus providing a better understanding of the interaction between the H2 molecule and graphene and offering evidence of the possibility of accessing more potential degrees of freedom for electronic applications using graphene.

Results

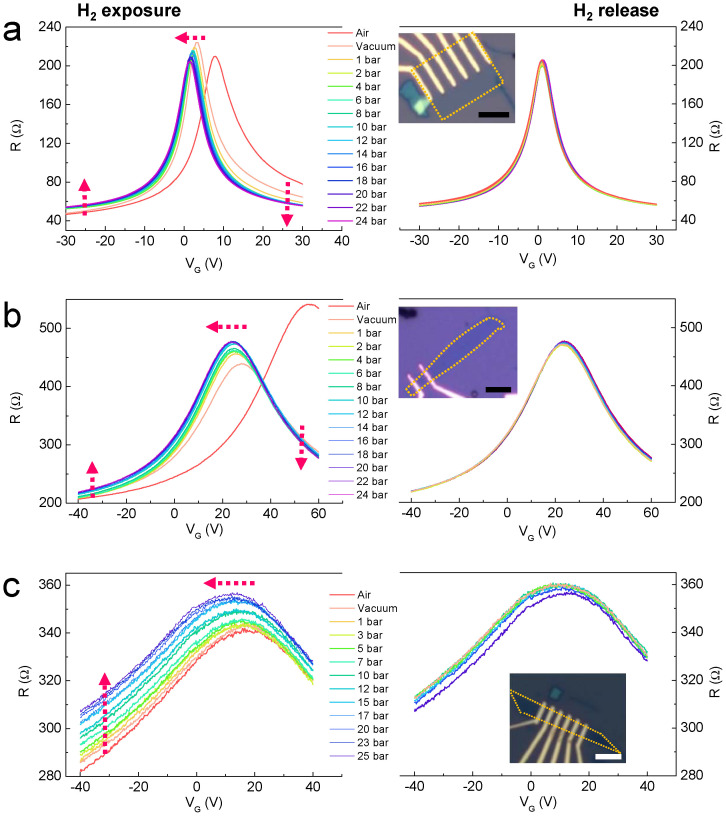
The VG-dependent resistance (R) of SLG (Fig. 1a), BLG (Fig. 1b), and MLG (Fig. 1c) as a function of H2 pressure up to 24 bar was measured. We observed a shift in the CNP toward the negative VG region in a H2 atmosphere. This shift indicates n-type behaviour, which becomes prominent as the layer number increases. Upon exposure to H2 (1 bar), the graphene sheets seemed to be doped by electrons. By further exposing the graphene sheets to H2 molecules, a high H2 pressure enhances this effect. This effect was also observed in the VG-dependent current-voltage (I-V) characteristics (Supplementary Fig. S2). Upon H2 exposure, (1) the maximum resistance (RM) increases in BLG and MLG but the RM of SLG decreases, and (2) far from VG at the CNP (VCNP), R in the VG < VCNP region increases while R in the VG > VCNP region decreases. The main carriers are holes and electrons at VG < VCNP and VG > VCNP, respectively. Hence, the n-doping effect induced by H2 exposure produces result (2) mentioned above. For result (1), the increase in RM in BLG and MLG can be understood with respect to the increase in the impurity carrier density19 but not for the reduction of RM in SLG. Hence, we tentatively suggest that the reduction in RM results from competition between the residual carrier density and the impurity carrier density, i.e., H2 exposure contributes more to the increase in the residual carrier density than the impurity density in SLG.

Figure 1. VG-dependent R as a function of H2 pressure for (a) SLG, (b) BLG, and (c) MLG.

Figure 1

Open in a new tab

At H2 pressure = 0 (vacuum), all graphene samples show the standard ambipolar field effect and the CNP broadens as the layer number increases. Upon H2 exposure (left panels), the CNPs of all graphene samples shift toward the negative VG region. The shift becomes larger with increasing layer number. In SLG, the maximum resistance, RM, decreases, but it increases in BLG and MLG. The decrease in RM may be a characteristic fingerprint of SLG. An increase and decrease in R for VG ≪ VCNP and for VG ≫ VCNP are observed in SLG and BLG. In the case of MLG, this behaviour may be observed over a wide-range VG sweep. During the release process (right panel), the same propensity is shown but is very small. The insets show optical microscopy images for each graphene sample. The scale bar is 5 μm.

Desorption occurred as the H2 pressure was reduced, as shown in the right panels of Figure 1, and only a slight shift in VCNP to the negative VG region was detected, which will be discussed later. More interestingly, even after applying a high vacuum (approximately 10−6 Torr) at 393 K, the CNP did not return to the original value obtained before H2 exposure. This result suggests dissociative H2 adsorption of graphene. The variation in CNP as the H2 pressure was increased and decreased is clearly shown in Supplementary Fig. S3.

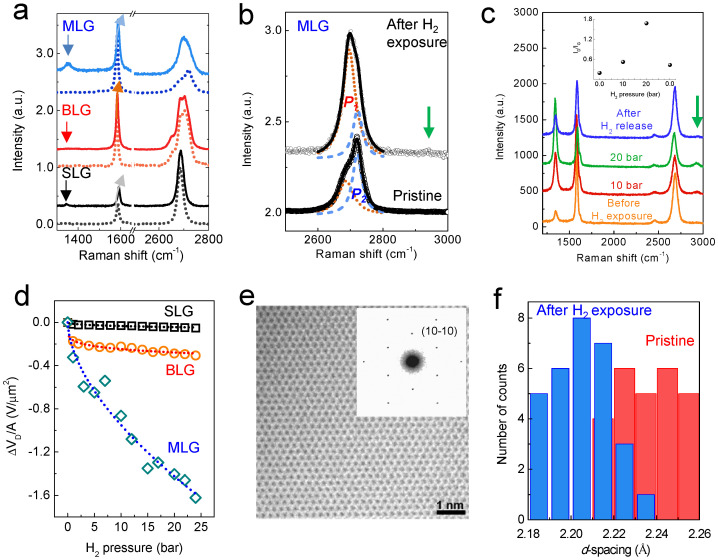
With regard to graphene, the attached hydrogen atoms can be considered impurities that exert the n-doping effect on graphene. Raman spectroscopy can provide information regarding this type of defect. The D peak near 1350 cm−1 is a breathing mode of A1g symmetry, which is ascribed to the presence of disorder. The G peak near 1580 cm−1 is the in-plane stretching mode of sp2 carbon20. The G band is blueshifted by the variation in charge density21. Development of the D peak and a blueshift in the G peak were observed after H2 exposure (Fig. 2a). Before H2 exposure, the G bands were located at 1586 cm−1, 1582 cm−1, and 1583 cm−1 for SLG, BLG, and MLG, respectively. After exposing the graphene to a H2 pressure of 24 bar, these peaks were shifted to 1591 cm−1, 1584 cm−1, and 1588 cm−1, respectively, and a D peak developed near 1350 cm−1 (for BLG, the D peak can be more clearly observed in Supplementary Fig. S4). The 2D band (approximately 2700 cm−1) for SLG and BLG showed little change, but this was not the case for MLG. In the Raman spectra of graphene, the 2D band was very sensitive to the stacking order of the graphene layers. As a result, turbostratic graphite, which lacks a stacking order, can be considered 2-dimensional (2-D) graphite22. The 2D band of MLG consisted of two sub-bands at 2686.52 ± 0.60 cm−1 and 2720.91 ± 0.16 cm−1 (Fig. 2b). These peaks are ascribed to contributions from 2-D graphite and the highly oriented 3-D structure of graphite, respectively23. Before H2 exposure, the peak (P1) corresponding to 2-D graphite was smaller than the peak (P2) originating from the 3-D graphitic structure. After H2 exposure, P1 increases, but P2 decreases. This is also observed in graphene grown by CVD without electrodes (see Supplementary Fig. S4). Note that the development of the peak at approximately 2930 cm−1 was observed in both MLG obtained by mechanical exfoliation and that produced by CVD growth (green arrows in Figs. 2b and 2c). This peak corresponds to the sp3 C-H stretching mode24. The C-H bending mode, which occurs at approximately 1330 cm−1, overlapped with the D peak24,25. To confirm the development of these peaks, we measured the H2 pressure-dependent Raman spectra of CVD graphene (Fig. 2c). Upon reaching a H2 pressure of 10 bar, the D and D' (approximately 1620 cm−1) peaks increased and the peak for the C-H stretching mode at 2930 cm−1 developed. As the H2 pressure was increased to 20 bar, the D and C-H peaks were enhanced. After evacuation at 393 K for 3 hr, the intensities of the two peaks were weakened, but the peaks were not identical to those obtained before H2 exposure. This indicates that a small degree of C-H bonding remains even after evacuation, which is similar to the result observed for the VG-dependent R after the release process. The inset of Figure 2c shows the change in the ID/IG ratio as a function of the H2 pressure. ID/IG increased with the increase in H2 pressure and then decreased after evacuation. The results obtained from Raman study reveals that the attached H atoms dissociated by graphene produce an incommensurate structure such as turbostratic graphite.

Figure 2. Dissociative H2 adsorption.

Figure 2

Open in a new tab

(a) Raman spectra for SLG, BLG, and MLG before (dotted line) and after H2 exposure. Broadening and a blueshift of the G band (sp2 carbon) occur, and the D band near 1350 cm-1 (sp3 carbon) develops due to H2 exposure. (b) A close view of the variation in the 2D band of MLG. The small peak at approximately 2930 cm−1 corresponds to the C-H bond (green arrow). (c) H2 pressure-dependent Raman spectroscopy of the CVD graphene. The defect evolution (ID/IG ratio) is depicted in the inset. Two zero values of H2 pressure are observed, one before H2 exposure (first one) and one after evacuation (left one). The peak for the C-H bond is clearly shown in the CVD graphene. (d) The variation in VCNP increases as the number of graphene layers increases. We chose VCNP at the highest pressure of 24 bar in this study with the assumption that hydrogen is almost saturated on and within the graphene at this pressure. (e) TEM image and ED patterns for graphene after H2 exposure. The carbon honeycomb lattice is clearly shown. (f) A histogram shows the d-spacings (10-10) for thirty different regions of graphene before (red) and after (blue) H2 exposure. The averaged d-spacings for pristine and hydrogen-chemisorbed graphenes are 2.22 ± 0.016 Å and 2.20 ± 0.014 Å, respectively.

By AFM, we observed an increase in the thickness of MLG from 3.2 ± 0.52 nm to 7.2 ± 0.82 nm after H2 exposure (Supplementary Fig. S5). An increase in the interlayer distance was also observed in the graphene prepared by CVD methods. Before H2 exposure, very small XRD peaks near 2θ = 15.8° and 26.5° (3.36 Å) were observed. The small peaks are attributed to the few-layer graphene grown by CVD. Upon H2 exposure, even at 1 bar of H2 pressure, the peak near 2θ = 15.8° (5.61 Å) became larger and shifted to a lower angle: 5.99 Å at 10 bar and 6.16 Å at 20 bar, indicating that the interlayer distance of few-layer graphene increased (Supplementary Fig. S4).

The impurity carrier density is proportional to the ΔVCNP induced by H2 exposure26. To investigate the effect of the impurity carrier density for these three types of graphene, we obtained values for ΔVCNP/A (Fig. 2d). A is the area of the three types of graphene between two electrodes. Increasing the number of graphene layers leads to an increase in ΔVCNP. At 24 bar, ΔVCNP/A = -0.05189 V/μm2 for SLG, -0.3065 V/μm2 for BLG, and −1.6216 V/μm2 for MLG. If we assume that the same number of dissociated hydrogen atoms is attached to each graphene layer, the specific ΔVCNP/A relationship for each of the three graphenes can be determined. Considering the ΔVCNP/A of SLG (−0.05189 μm−2), the ΔVG/A due to the interspace of BLG becomes −0.2546 V/μm2, which shows that hydrogen atoms are attached to not only the edge but also the central region of the graphene. The MLG used in the measurement has seven layers, indicating that it has six BLG interspaces and one layer of SLG (AFM images in Supplementary Fig. S5). Therefore, a variation of −1.5796 V/μm2 can be estimated by the variation in VCNP for SLG and the interspace of BLG. This value is similar to that obtained for the variation in the CNP in MLG (1.6216 V/μm2, the small discrepancy between the two values may be due to the error in the height obtained by AFM). This similarity indicates that the shift in CNP toward the n-type carrier regime is due to the graphene itself and not other effects such as dielectric screening caused by hydrogen molecules between the graphene and the SiO2 substrate27. An increase in interlayer distance at high H2 pressure13 is theoretically expected, and an enhancement in the peak near 2θ = 15° in the XRD patterns of CVD graphene (see Supplementary Fig. S4) shows that the structure of hydrogen molecule-intercalated MLG can be interpreted as second-stage structures such as graphite bromine lamellar compounds28. However, because electrical charge cannot be transferred from hydrogen molecules to graphene16, the physisorption of H2 molecules can be attributed to the increase in the interlayer distance, but this is not the reason for the n-type doping of graphene.

Strong evidence for chemisorption was obtained from TEM images of single-layer graphene grown by CVD methods (Figs. 2e and 2f). Structural modulation was indicated by the electron-diffraction (ED) patterns for 30 different regions of a graphene sample obtained before and after H2 exposure. The d-spacings of pristine graphene were distributed over distances ranging from 2.197 to 2.256 Å. In the case of H2-exposed graphene, the d-spacing was reduced (2.181–2.232 Å), indicating that in-plane compression occurs due to the chemical bonding of hydrogen6.

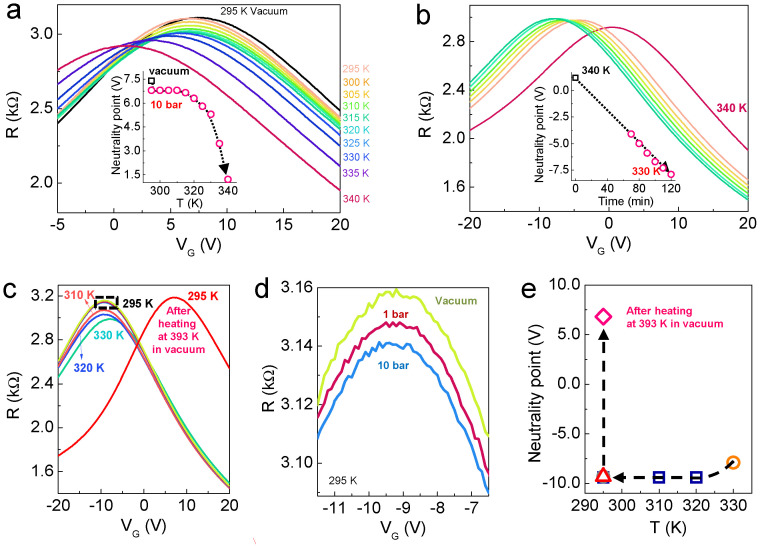
Thus far, we have investigated the properties of graphene upon H2 exposure at 300 K. The results indicate that the dissociation barrier of H2 is reduced on graphene. Accordingly, the VG-dependent R of MLG at constant H2 pressure (10 bar) was measured with increasing temperature up to 340 K (Fig. 3). Upon exposing the graphene to 10 bar of H2 pressure, the CNP shifted from 7.40 V to 6.80 V and then remained constant up to T = 310 K. Beyond T = 315 K, the CNP started to shift again (6.65 V at 315 K and 6.30 V at 320 K) and was slightly enhanced at 325 K (5.80 V at 325 K). At T > 330 K, the CNP changes significantly from 5.30 V to 1.20 V at 340 K (Fig. 3a). The dissociation of H2 was much more favourable at high temperature. This finding indicates that the probability of overcoming the dissociation barrier increases, even for a small thermal energy. During cooling, we observed an interesting feature. Figure 3b shows the variation in CNP as a function of time at 330 K. Although T decreased from 340 K to 330 K, the CNP continuously shifted to −4.1 V (70 min elapsed after the measurement at 340 K). With increasing time, the CNP decreased to −7.9 V at a constant temperature T = 330 K. This shift is natural because the thermal energy at a temperature of 330 K exceeds the dissociation barrier, as shown in Figure 3a. Further shifts in VG during the release process (Fig. 1 and Supplementary Fig. S3) can be understood in the same way. The CNP continued to shift up to 320 K, but it remained constant beyond T = 310 K (Fig. 3c). The CNPs at 295 K also remained unchanged during evacuation (dotted box in Figs. 3c and 3d). However, heat treatment at 393 K in vacuum for 3 hr caused the CNP to return to approximately its original value (6.8 V). The variation in CNP with decreasing temperature is summarised in Figure 3e.

Figure 3. VG-dependent R as a function of temperature for MLG at 10 bar of H2 pressure.

Figure 3

Open in a new tab

MLG samples were used to clarify the n-doping effect. (a) Upon exposing the graphene to 10 bar of H2 at 295 K, the CNP shifts toward the negative VG region. As the temperature increases up to 310 K, the CNP remains nearly constant. In fact, it shifts but cannot be defined because of the resolution (a VG sweep interval of 50 mV). Beyond 315 K, the CNP shifts again, and the variation in CNP increases with temperature up to 340 K. (b) During the cooling process, we set the temperature to 330 K. As the exposure time increases, the CNP continuously shifts along the same direction and RM increases slightly. The insets of (a) and (b) clearly show the variation in the CNP. (c) The shift is maintained down to 320 K but not below 310 K. (d) At 295 K, although the H2 pressure is released, no shift in the CNP is observed. (e) The variation in the CNP during the release process is depicted.

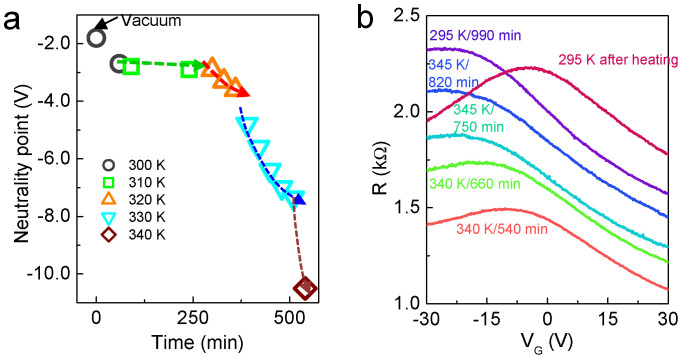
The same experiment with a different MLG sample was performed for a long exposure time (t) and up to a slightly higher temperature (345 K). Before H2 exposure, measurements of the VG-dependent R as a function of T were carried out. The CNP was observed to remain constant up to 345 K (see Supplementary Fig. S6). Upon H2 exposure, an n-doping effect was also observed. However, the shift in CNP and the decrease in hole mobility became significant compared with the results described in Figure 3, as is clearly shown in Figure 4a. Although ΔVCNP/Δt increased as the temperature increased, ΔVCNP/Δt seemed to saturate at each temperature at even longer times, which implies that both sufficient time and high temperature are necessary to enhance the n-doping effect. As a result, the hole mobility strongly decreased at 345 K after 750 min (Fig. 4b). This behaviour was maintained at 295 K. However, although the ambipolarity is recovered, the current level does not return to its original value in air after heating in a vacuum due to the re-adsorption of admolecules such as O2 and H2O in air. If a desorption process (heating in vacuum) is not performed, the CNP moves toward the positive VG region and is then saturated, i.e., the n-type behaviour is maintained even in air (Supplementary Fig. 7).

Figure 4. VG-dependent R as a function of temperature and exposure time for MLG at 10 bar of H2 pressure.

Figure 4

Open in a new tab

(a) The variation in CNP with respect to T and t. Upon exposure to 10 bar of H2 pressure at 300 K, the CNP changes; it then changes slightly at 320 K and t = 300 min after exposure. Beyond 320 K/300 min, the slope of ΔVCNP/Δt increases. (b) Beyond 340 K/540 min, the hole mobility decreases significantly and finally reaches zero. After heating to 393 K for 3 hr, the ambipolarity is restored.

Discussion

The dissociative H2 adsorption barrier on graphite is approximately 3.3 eV29. Although this barrier appears prohibitive, dissociation can occur in particular cases. If a hydrogen molecule approaches a vacancy defect on a graphene surface, the molecule becomes largely polarised and finally dissociates17. At the edge of graphite, the energy barrier is reduced, causing dissociative adsorption18,30. Other possible factors for dissociative H2 adsorption, including electric fields31 and mechanical stress32, have been suggested. In the present study, the C–H bonding of CVD graphene was clearly detected compared with that of mechanically exfoliated graphene. The results show that vacancy defects promote dissociative adsorption because graphene grown by CVD methods has more structural defects than mechanically exfoliated graphene33.

H molecules dissociated on graphene can break C = C double bonds in the structure of graphene. Consequently, two unpaired electrons are produced. An unpaired electron participates in the formation of a C-H bond, and the other is delocalised34,35,36,37. This delocalised electron induces an n-type doping effect on graphene. In addition, we can rule out other possibilities for n-doping on graphene in a H2 atmosphere, including the presence of a Ti adhesion layer (Supplementary Figs. S8 and S9).

Collectively, the exposure of graphene to H2 molecules causes an increase in the interlayer distance of graphene, and molecular hydrogen is partially dissociated due to the existence of vacancy defects on graphene, as theoretically proposed. The dissociated H atoms are expected to be chemisorbed at the edge30 and in the vacancies of the graphene17,38, resulting in the n-type doping of graphene due to delocalised unpaired electrons.

Methods

Graphene samples were extracted from highly oriented pyrolytic graphite (HOPG) by mechanical exfoliation. The graphene samples were deposited on 300 nm SiO2/highly p-doped Si wafers. The samples were selected by the colour contrast observed in optical microscopy images and characterised by micro-Raman spectroscopy (LabRam 300 with a 532 nm laser line of less than 1 mW, JY-Horiba) and AFM (SPA-400, Seiko instrument). A TEM investigation (HRTEM and diffraction pattern) of single-layer graphene was performed using an FEI Titan cube G2 60-300 installed at UNIST, equipped with an image aberration corrector. The microscope was operated at an accelerating voltage of 80 kV to reduce beam damage. Electrodes were fabricated by standard electron beam lithography (VEGA MM5150 with 30 keV filament, Tescan), the evaporation of Ti/Au (5/50 nm) and Cr/Au (10/40 nm) (ZZS550-2/D, Maestech), and lift-off procedures.

The devices were electrically characterised by a back gate sweep with a drain-source bias of Vds = 1.0 or 2.0 mV using a semiconductor characterisation system (4200-SCS, Keithley) in a high-pressure chamber, which was completely made of stainless steel. The procedure is as follows. First, the devices were loaded into the chamber, which was evacuated at 393 K until the pressure reached approximately 10−6 Torr. Then, the graphene surfaces were exposed to 99.9999 % H2 gas at pressures ranging from 1.0 to 25 bar.

Author Contributions

B.H.K. and S.J.H. designed the study, performed experiments, collected and analysed data, and wrote the paper. S.J.B., M.P., S.W.C., J.L., and J.C.L. performed the experiments. H.Y.J. performed and analysed the TEM study. N.P. and M.L. provided the theoretical analysis. S.W.L. provided bilayer graphene and analysed the data. H.S.S. analysed the data and edited the manuscript. Y.J. and Y.W.P. supervised the study and wrote the paper. All authors discussed the results and commented on the manuscript.

Supplementary Material

Supplementary Information

N-type graphene induced by dissociative H2 adsorption at room temperature

srep00690-s1.pdf (1.9MB, pdf)

Acknowledgments

We thank K. S. Novoselov, P. Kim, and C. N. R. Rao for helpful discussions and comments and H.J. Kim for supporting the manufacture of the high-pressure chamber. N.P. was supported by the Hydrogen Energy R&D Center, a 21st Century Frontier R&D Program funded by the Ministry of Science and Technology of Korea. Y.W.P. acknowledges support from the GRDC (2009-00514) through the Ministry of Education, Science and Technology (MEST), Korea. S.W.L. acknowledges support from the WCU program of the MEST (R31-2008-000-10057-0) and from the NRF (2011-0021207). Y. J. acknowledges support from MEST (20120002424, 2012K001288).

References

  1. Novoselov K. S. et al. Electric field effect in atomically thin carbon films. Science 306, 666–669 (2004). [DOI] [PubMed] [Google Scholar]
  2. Zhang Y., Tan Y.-W., Stormer H. L. & Kim P. Experimental observation of the quantum Hall effect and Berry's phase in graphene. Nature 438, 201–204 (2005). [DOI] [PubMed] [Google Scholar]
  3. Geim A. K. & Novoselov K. S. The rise of graphene. Nature Mater. 6, 183–191 (2007). [DOI] [PubMed] [Google Scholar]
  4. Nomura K. & MacDonald A. H. Quantum transport of massless Dirac fermions. Phys. Rev. Lett. 98, 076602 (2007). [DOI] [PubMed] [Google Scholar]
  5. Lee C., Wei X., Kysar J. W. & Hone J. Measurement of the elastic properties and intrinsic strength of monolayer graphene. Science 321, 385–388 (2008). [DOI] [PubMed] [Google Scholar]
  6. Elias D. C. et al. Control of graphene's properties by reversible hydrogenation: evidence for graphane. Science, 323, 610–613 (2009). [DOI] [PubMed] [Google Scholar]
  7. Balog R. et al. Bandgap opening in graphene induced by patterned hydrogen adsorption. Nature Mater. 9, 315–319 (2010). [DOI] [PubMed] [Google Scholar]
  8. Luo Z. et al. Thickness-dependent reversible hydrogenation of graphene layers. ACS Nano 3, 1781–1788 (2009). [DOI] [PubMed] [Google Scholar]
  9. Jaiswal M. et al. Controlled hydrogenation of graphene sheets and nanoribbons. ACS Nano 5, 888–896 (2011). [DOI] [PubMed] [Google Scholar]
  10. Ryu S. et al. Reversible basal plane hydrogenation of graphene. Nano Lett. 8, 4597–4602 (2008). [DOI] [PubMed] [Google Scholar]
  11. Deng W.-Q., Xu X. & Goddard W. A. New alkali doped pillared carbon materials designed to achieve practical reversible hydrogen storage for transportation. Phys. Rev. Lett. 92, 166103 (2004). [DOI] [PubMed] [Google Scholar]
  12. Patchkovskii S. et al. Graphene nanostructures as tunable storage media for molecular hydrogen. Proc. Natl. Acad. Sci. USA 102, 10439–10444 (2005). [DOI] [PMC free article] [PubMed] [Google Scholar]
  13. Aga R. S., Fu C. L., Krčmar M. & Morris J. R. Theoretical investigation of the effect of graphite interlayer spacing on hydrogen absorption. Phys. Rev. B 76, 165404 (2007). [Google Scholar]
  14. Cabria I., López M. J. & Alonso J. A. Hydrogen storage capacities of nanoporous carbon calculated by density functional and Møller-Plesset methods. Phys. Rev. B 78, 075415 (2008). [Google Scholar]
  15. Subrahmanyam K. S. et al. Chemical storage of hydrogen in few-layer graphene. Proc. Natl, Acad. Soc. USA, 108, 2674–2677 (2011). [DOI] [PMC free article] [PubMed] [Google Scholar]
  16. Henwood D. & Carey J. D. Ab initio investigation of molecular hydrogen physisorption on graphene and carbon nanotubes. Phys. Rev. B 75, 245413 (2007). [Google Scholar]
  17. Allouche A. & Ferro Y. Dissociative adsorption of small molecules at vacancies on the graphite (0001) surface. Carbon 44, 3320–3327 (2006). [Google Scholar]
  18. Diño W. A. et al. H2 dissociative adsorption at the armchair edges of graphite. Solid State Commun. 132, 713–718 (2004). [Google Scholar]
  19. Tan Y.-W. et al. Measurement of scattering rate and minimum conductivity in graphene. Phys. Rev. Lett. 99, 246803 (2007). [DOI] [PubMed] [Google Scholar]
  20. Ferrari A. C. & Robertson J. Interpretation of Raman spectra of disordered and amorphous carbon. Phys. Rev. B 61, 14095–14107 (2000). [Google Scholar]
  21. Yan J., Zhang Y., Kim P. & Pinczuk A. Electric field effect tuning of electron-phonon coupling in graphene. Phys. Rev. Lett. 98, 166802 (2007). [DOI] [PubMed] [Google Scholar]
  22. Pimenta M. A. et al. Studying disorder in graphite-based systems by Raman spectroscopy. Phys. Chem. Chem. Phys. 9, 1276–1291 (2007). [DOI] [PubMed] [Google Scholar]
  23. Barros E. B. et al. Raman spectroscopy of graphitic foams. Phys. Rev. B 71, 165422 (2005). [Google Scholar]
  24. Ferrari A. C. & Robertson J. Resonant Raman spectroscopy of disordered, amorphous, and diamondlike carbon. Phys. Rev. B 64, 075414 (2001). [Google Scholar]
  25. Ristein J., Stief R. T., Ley L. & Beyer W. A comparative analysis of a-C:H by infrared spectroscopy and mass selected thermal effusion. J. Appl. Phys. 84, 3836–3847 (1998). [Google Scholar]
  26. Chen J.-H. et al. Charged-impurity scattering in graphene. Nature Phys. 4, 377–381 (2008). [Google Scholar]
  27. Adam S., Hwang E. H., Galitski V. M. & Das Sarma S. A self-consistent theory for graphene transport. Proc. Natl. Acad. Soc. USA 104, 18392–18397 (2007). [DOI] [PMC free article] [PubMed] [Google Scholar]
  28. Sasa T., Takahashi Y. & Mukaibo T. Crystal structure of graphite bromine lamellar compounds. Carbon 9, 407–416 (1971). [Google Scholar]
  29. Miura Y., Kasai H., Diño W., Nakanishi H. & Sugimoto T. First principles studies for the dissociative adsorption of H2 on graphene. J. Appl. Phys. 93, 3395–3400 (2003). [Google Scholar]
  30. Sha X. & Jackson B. The location of adsorbed hydrogen in graphite nanostructures. J. Am. Chem. Soc. 126, 13095–13099 (2004). [DOI] [PubMed] [Google Scholar]
  31. Ao Z. M. & Peeters F. M. Electric field activated hydrogen dissociative adsorption to nitrogen-doped graphene. J. Phys. Chem. C 114, 14503–14509 (2010). [Google Scholar]
  32. McKay H., Wales D. J., Jenkins S. J., Verges J. A. & de Andres P. L. Hydrogen on graphene under stress: molecular dissociation and gap opening. Phys. Rev. B 81, 075425 (2010). [Google Scholar]
  33. Banhart F., Kotakoski J. & Krasheninnikov A. V. Structural defects in graphene. ACS Nano 5, 26–41 (2011). [DOI] [PubMed] [Google Scholar]
  34. Boukhvalov D. W., Katsnelson M. I. & Lichtenstein A. I. Hydrogen on graphene: electronic structure, total energy, structural distortions and magnetism from first-principle calculations. Phys. Rev. B 77, 035427 (2008). [Google Scholar]
  35. Boukhvalov D. W. & Katsnelson M. I. Chemical functionalization of graphene with defects. Nano Lett. 8, 4373–4379 (2008). [DOI] [PubMed] [Google Scholar]
  36. Vergés J. A. & de Andres P. L. Trapping of electrons near chemisorbed hydrogen on graphene. Phys. Rev. B 81, 075423 (2010). [Google Scholar]
  37. Brenner K., Yang Y. & Murali R. Edge doping of graphene sheets. Carbon 50, 637–645 (2012). [Google Scholar]
  38. Bae G., Cha J., Lee H., Park W. & Park N. Effects of defects and non-coordinating molecular overlayers on the work function of graphene and energy-level alignment with organic molecules. Carbon 50, 851–856 (2012). [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information

N-type graphene induced by dissociative H2 adsorption at room temperature

srep00690-s1.pdf (1.9MB, pdf)

Articles from Scientific Reports are provided here courtesy of Nature Publishing Group

RESOURCES