Abstract
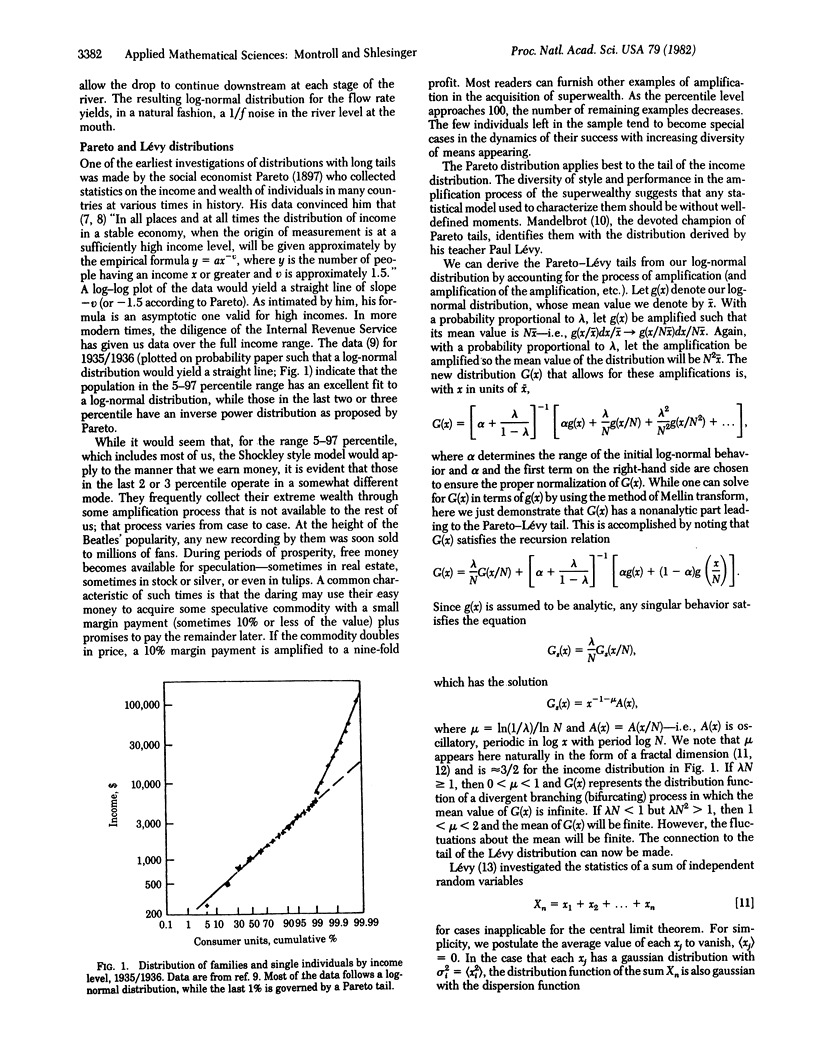
It is shown, following Shockley [Shockley, W. (1957) Proc. IRE 45, 279-290], that, when a population is engaged in tasks whose completion requires the successful conclusion of many independent subtasks, the distribution function for successes in the primary task is log normal. It is also shown that, when the dispersion of the log-normal distribution is large, the distribution is mimicked by a 1/x distribution over a wide range of x. This argument provides a generic set of processes that yields the much observed 1/x distribution, and will also lead to a 1/f noise spectrum. It is commonly found that distributions that seem to be log normal over a broad range (say to the 95th percentile of a population) change to an inverse fractional power (Pareto) distribution for the last few percentile. Annual income distributions are examples with this structure. The very wealthy generally achieve their superwealth through amplification processes that are not available to most. We have introduced a simple amplification model to characterize the transition from a log-normal distribution to an inverse-power Pareto tail.
Keywords: log-normal distribution, Lévy distribution, Pareto distribution, scale-invariant process
Full text
PDF


Selected References
These references are in PubMed. This may not be the complete list of references from this article.
- Hughes B. D., Shlesinger M. F., Montroll E. W. Random walks with self-similar clusters. Proc Natl Acad Sci U S A. 1981 Jun;78(6):3287–3291. doi: 10.1073/pnas.78.6.3287. [DOI] [PMC free article] [PubMed] [Google Scholar]


