Abstract
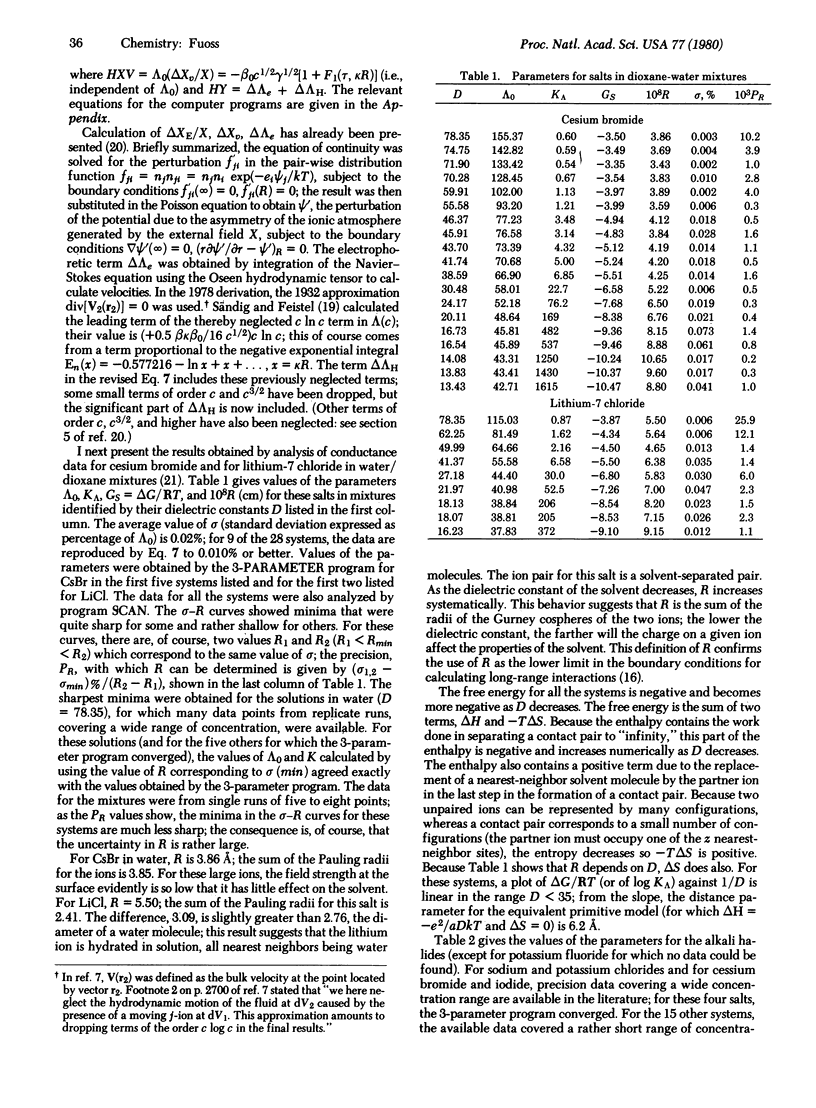
Earlier theories of electrolytic conductance are reviewed; all of these, with the exception of the Arrhenius-Ostwald theory, are based on physical models. Their theory failed to describe the conductance of strong electrolytes because it did not include the effects (then unsuspected) of long-range forces on mobility. Thermodynamic derivations are independent of model; applied to the postulated equilibrium A+ + B- ⇄ A+B- between free ions and nonconducting paired ions, the thermodynamic pairing constant Ka equals ap/(a±)2, and ΔG, the difference in free energy between paired ions (activity = ap) and free ions (activity = a±), equals (-RT ln Ka). Converting to the molarity scale, Ka = (1000 ρ/M)[1 - γ)/cy2(y±)2]. Here ρ is the density of the solvent of molecular weight M, c is stoichiometric concentration of electrolyte (mol/liter), γ is the fraction of solute present as unpaired ions, and y± is their activity coefficient. The corresponding conductance function Λ = Λ(c;Λ0,R,△G)involves three parameters: Λ0, the limiting equivalent conductance; R, the sum of the radii of the cospheres of the ions; and ΔG. Conductance data for cesium bromide and for lithium chloride in water/dioxane mixtures and for the alkali halides in water are analyzed to determine these parameters. Correlations between the values found for R and ΔG and properties characteristic of salt and solvent are then discussed.
Keywords: ion pairs, association constants, conductance theory
Full text
PDF



Selected References
These references are in PubMed. This may not be the complete list of references from this article.
- Fuoss R. M., Hsia K. L. Association of 1-1 salts in water. Proc Natl Acad Sci U S A. 1967 Jun;57(6):1550–1557. doi: 10.1073/pnas.57.6.1550. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Fuoss R. M., Onsager L. CONDUCTANCE OF STRONG ELECTROLYTES AT FINITE DILUTIONS. Proc Natl Acad Sci U S A. 1955 May 15;41(5):274–283. doi: 10.1073/pnas.41.5.274. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Fuoss R. M. Paired ions: Dipolar pairs as subset of diffusion pairs. Proc Natl Acad Sci U S A. 1978 Jan;75(1):16–20. doi: 10.1073/pnas.75.1.16. [DOI] [PMC free article] [PubMed] [Google Scholar]


