Abstract
Nilanjana Dasgupta’s (this issue) stereotype inoculation model (SIM) helps explain why what feels like a free choice to pursue one life path over another “is often constrained by subtle cues in achievement environments that signal who naturally belongs there and is most likely to succeed and who else is a dubious fit” (p. 231). She posits that seeing others like themselves in successful roles inoculates women against negative stereotypes that impede their success and persistence in specific achievement contexts.
As is true of classic theoretical positions (see Nagel, 1961), Dasgupta presents postulates from which she deduces a specific set of hypotheses, and she reviews the relevant empirical/observational data in support of them. It is precisely what this area of research has long needed—moving beyond demonstrations of identity threats to a theory about their underlying causes, conditions, and interventions. This proposal leads her to four broad predictions, the first of which is the primary focus of our comment.
Exposure to Successful Ingroup Peers/Experts
A central tenet of Dasgupta’s theory is that ingroup members who are peers or experts in a high-achievement context can have notable effects on an individual’s career choices and subsequent success. This claim leads her to a set of five hypotheses. The first is that exposure to ingroup experts and peers who are successful in a high-achievement context act as “social vaccines” for those aspiring to pursue a career in that same context. These experts and peers enhance the positive attitudes, perceived self-efficacy, and motivation of aspiring individuals, thus providing a sense of belongingness. The second hypothesis is that social vaccines are particularly influential for members of an ingroup that is associated with negative stereotypes regarding their ability to succeed in a particular high-achievement context (e.g., women in mathematics). Third, ingroup experts will have the greatest effect on aspiring ingroup members who develop a “subjective sense of connection or identification” (p. 233) with the expert. Dasgupta’s fourth hypothesis is that the effects of stereotypes (as well as intervention effects of ingroup experts and peers) on aspiring individuals are often unconscious. She posits four psychological mechanisms that, together, serve to “inoculate the self-concept when individuals encounter ingroup experts and peers” (p. 234). These mechanisms entail an enhanced sense of belongingness, enhanced self-efficacy, a feeling of being challenged when faced with difficulty, and feeling less threatened. Her articulation of SIM theory accords with a great deal of recent research on stereotype and identity threat, but it leaves unanswered certain peculiarities as well as boundary conditions that we describe next.
There is evidence that managing anxiety about poor fit can have deleterious consequences for self-regulatory resources (see Johnson, Richeson, & Finkel, 2011). This is because coping with anxiety usurps working memory resources (Ceci, Williams, & Barnett, 2009). In Dasgupta’s view, contact with successful ingroup peers in high-stakes achievement contexts inoculates women against self-doubt, especially in early years of academic development when individuals’ self-efficacy is in flux. This leads her to anticipate that such contact enhances beginners’ positive attitudes toward the achievement domain in question, strengthens their identification with it, enhances self-efficacy, and increases motivation to pursue career goals. There is a ring of intuitiveness in these expectations, and yet there is a nagging sense of incompletion, too. We illustrate this in the case of women in the domain of mathematics.
In the domain of mathematics, although females are members of a negatively stereotyped group (e.g., Correll, 2004; C. M. Steele, Spencer, & Aronson, 2002; J. Steele, James, & Barnett, 2002), most of the early successes actually go to them. There are no systematic sex differences in mathematics in the primary grades, although starting around fourth grade, boys begin to outperform girls at the right tail of the math achievement distribution on standardized achievement tests (Ceci et al., 2009; Ceci & Williams, 2010a, 2010b). However, there are no differences in mean math scores and, it is important to note, girls outperform boys in math grades throughout elementary and high school and even in college math classes. Thus, from an early age females are exposed to ingroup experts (teachers) and peers who get the highest grades in math, and their achievement continues unabated throughout their schooling. Because of this, unsurprisingly, females compose roughly half of graduating mathematics majors in U.S. colleges and universities and nearly one third of graduate students in mathematics programs (Ceci & Williams, 2010a). Yet girls and women continue to experience negative stereotypes regarding their mathematical ability. If girls are continuously in an environment in which they observe ingroup members (other girls) excelling in mathematics, then why hasn’t this led to greater stereotype inoculation aspirations and increased in their motivation to pursue math-intensive careers? Despite their representation in doctoral programs in mathematics, engineering, physics, economics, computer science, and so on, they do not segue into careers in these math-intensive fields at the same rate as their male counterparts. Is their attrition, as SIM suggests, a constrained choice that results from unconscious cues intimating who belongs in mathematics? And if so, when do these subtle cues take their toll on career aspirations, and what are the boundary conditions (see next)? Dasgupta may be correct, but if so she needs more evidence. Here’s why.
Women are relatively more likely than men to be interviewed and hired for tenure track, math-intensive positions in academia. In a 2009 National Research Council (NRC) analysis, the mean percentage of females interviewed for tenure-track and tenured positions exceeded the mean percentage of female applicants for these positions in all six fields studied. Table 6.2 of the 2009 NRC report shows that although in mathematics only 20% of applicants for tenure-track posts were women, fully 32% of those who were hired were women. Comparable figures showing higher rates of hiring female applicants were reported for physics, civil engineering, and electrical engineering (NRC, 2009).
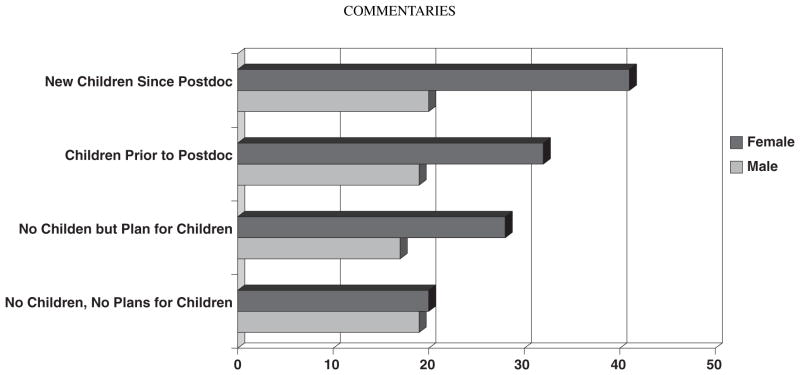
Lest one infer that this is the result of stereotypes cuing women that they do not belong in these male-dominated fields, as can be seen in Figure 1, the biggest reason women opt out of applying for these positions is either the presence of a child during graduate school/postdoc training or the plan to have children later. Women with children during their training years are far more likely than men to conclude that family–work balance is untenable in a tenure-track career, hence they opt not to apply for such positions at nearly twice the rate of men. Among women with no children or plans to have children later, similar percentages of women apply for these positions as do men, and they go on to similar career trajectories as men (remuneration and tenure rates).
Figure 1.
Percentage of University of California postdocs who switched from aspiring to a research professor career. Note. Based on data reported by Goulden, Frasch, and Mason (2009).
Thus, it would be incorrect to surmise that women avoid mathematical careers out of a sense of not belonging that is engendered by cues about their ability. Women are not applying at the same rate as men because some of them view academic careers as incompatible with their family formation plans, despite having excelled at mathematics throughout their entire education.1 Having made this point, however, it is nevertheless worth noting that women still only compose 20 to 30% of tenure-track positions in the most math-intensive fields. Even though this is due to their being less likely to apply for these positions upon completion of their doctoral/postdoctoral training as a result of their family aspirations, rather than the result of identity threat or sense of not belonging, it still underscores the dearth of tenure-track female scientists at research universities and the need to make it easier for those with family aspirations to balance family and work in R1 (research-intensive) universities.
Boundary Conditions
What are the boundaries of SIM? It is not enough to know that there are ingroup experts and peers in one’s field, because we all know of some women in science somewhere, but female scientists are still adversely affected by stereotypes. And schoolchildren observe girls outperforming boys on math grades throughout schooling years. Is it necessary for one to know these ingroup experts/peers personally? Dasgupta (this issue) cites work showing that one can consider someone to be a role model without having known them (Lock-wood & Kunda, 1997), but this raises the question as to whether more interaction with ingroup role models would make the inoculation stronger, or does the amount of exposure not matter after a certain threshold is met? Fleshing out such boundary conditions will be important in future research.
This gets at an issue that Correll (2001, 2004) and others have discussed, which will also benefit from future research, namely, that performance and self-efficacy don’t always coincide. According to Correll (2001), this is at least in part because “cultural beliefs about gender and task competence provide a framing context that biases other information individuals use in assessing their own competence” (p. 1700). In support of this, she found that men tend to rate their math competence higher than women even when performance is comparable and that self-rated math competence was more predictive than performance when it came to looking at career and college major decisions. To date, however, the mechanism underlying this observation has not been elucidated, rendering it circular. How do cultural beliefs regarding gender and math trump actual math achievement? Why is it that in many domains women have ratcheted up their career representation over a very brief period (e.g., veterinary medicine went from being heavily male dominated to heavily female dominated within a short period)? Medicine, law, biology, and other fields also experienced drastic shifts in their numbers of women in short periods. To assert that cultural beliefs about gender are responsible for the dearth of women in some fields but not others requires greater specification of the mechanisms at work. Otherwise, we are left with the reality of more women in some fields than others and the claim that cultural beliefs provide a framing context in some (e.g., engineering) but not other domains (e.g., veterinary medicine).
Being part of a numerical minority can, for some people, be something they are proud of that buffers them from stereotypes; Crisp, Bache, and Maitner (2009) found that female engineering college students, who are coping in a male-dominated field, do not demonstrate the classic stereotype threat effects when reminded of their gender during a difficult math test (unlike their peers majoring in psychology, who performed worse after thinking about their gender). Dasgupta mentions female engineers, and how being a numerical minority can have deleterious effects on performance, but some seem to find strength in it. Again, it will be important for future research on SIM to provide greater clarity of the boundary conditions.
Discussions of numeric minorities have primarily been focused on stereotypes that relate to individuals’ visible identities (e.g., race, gender). However, what about first-generation college students, people from low socioeconomic status backgrounds, or gays and lesbians, all of whom contend with an identity that is stigmatized but concealable (Johnson et al., 2011)? How can they identify ingroup experts and peers in their environment when that is not possible just by looking at the crowd? SIM would seem to require that everyone make their stigmatized identities known to those around them, a choice that some individuals would rather not make. It is important to understand the effects of racial and gender stereotypes and how the SIM contributes to that understanding, and future work could consider the ways in which other kinds of stereotypes, such as those surrounding concealable stigmatized identities, might relate to this model.
One practical consequence that needs to be implemented cautiously is Dasgupta’s recommendation to highlight the presence of ingroup experts and peers, something that must be done without making these experts/peers feel like tokens themselves. For example, a frequent complaint of female faculty in science, technology, engineering, and math fields is that they are asked to be on so many committees (to enhance the diversity of a given committee) that these commitments, an additional burden not shared by most of their male colleagues, end up interfering with their actual work. Emphasizing the importance of female representation in various committees is one way to highlight the presence of ingroup experts and peers, but care should be taken to make sure that these experts and peers are not being disadvantaged by the need to represent their ingroup.
In closing, we want to reiterate that SIM is an ambitious and much needed endeavor, one that will provide greater explanatory clout than the collection of demonstrations has heretofore accomplished. We offer our concerns about boundary conditions not as a criticism of SIM but as a call for more research to actualize what we see as its potential.
Footnotes
Some have argued that “chilly climate” can explain the un-derrepresentation of women among tenure-track professors. This ignores the fact that the single largest loss of women from the scientific pipeline occurs while they are PhD students and postdocs; this is when they decide not to apply for tenure-track positions, and they do so primarily because they find the rigors of earning tenure difficult to reconcile with their family aspirations. Numerous surveys document this massive leakage (e.g., Ceci & Williams, 2011). The deleterious effect of chilly climate accrues over time as women feel isolated and disrespected by male colleagues. In contrast, graduate students and postdocs are hardly aware of chilly climate; thus although it is possible that chilly climate may cause leakage in the posthiring stage, it is highly unlikely that it does so before one even applies for a job, and yet this is the time when women opt out of professoriate in greatest numbers.
References
- Ceci SJ, Williams WM. The mathematics of sex: How biology and society conspire to limit talented women and girls. New York, NY: Oxford University Press; 2010a. [Google Scholar]
- Ceci SJ, Williams WM. Sex differences in math-intensive fields. Current Directions in Psychological Science. 2010b;19:275–279. doi: 10.1177/0963721410383241. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Ceci SJ, Williams WM. Understanding current causes of women’s underrepresentation in science. Proceedings of the National Academy of Sciences. 2011;108:3157–3162. doi: 10.1073/pnas.1014871108. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Ceci SJ, Williams WM, Barnett SM. Women’s un-derrepresentation in science: Sociocultural and biological considerations. Psychological Bulletin. 2009;135:218–261. doi: 10.1037/a0014412. [DOI] [PubMed] [Google Scholar]
- Correll SJ. Constraints into preferences: Gender, status, and emerging career aspirations. American Sociological Review. 2004;69:93–114. [Google Scholar]
- Correll SJ. Gender and the career choice process: The role of biased self-assessments. American Journal of Sociology. 2001;106:1691–1730. [Google Scholar]
- Crisp R, Bache L, Maitner AT. Dynamics of social comparison in counter-stereotypic domains: Stereotype boost, not stereotype threat, for women engineering majors. Social Influence. 2009;4:171–184. [Google Scholar]
- Goulden M, Frasch K, Mason MA. Staying competitive: Patching American’s leaky pipeline in the sciences. Berkeley, CA: Center for American Progress, Berkeley Center on Health, Economic & Family Security, University of California, Berkeley; 2009. [Google Scholar]
- Johnson S, Richeson J, Finkel E. Middle class and marginal? Socioeconomic status, stigma, and self-regulation at an elite university. Journal of Personality and Social Psychology. 2011;100:838–852. doi: 10.1037/a0021956. [DOI] [PubMed] [Google Scholar]
- Lockwood P, Kunda Z. Superstars and me: Predicting the impact of role models on the self. Journal of Personality and Social Psychology. 1997;73:91–103. [Google Scholar]
- Nagel E. The structure of science: Problems in the logic of scientific explanation. New York, NY: Harcourt, Brace & World; 1961. [Google Scholar]
- National Research Council. Gender differences at critical transitions in the careers of science, engineering and mathematics faculty. Washington, DC: National Academy Press; 2009. [Google Scholar]
- Steele CM, Spencer SJ, Aronson J. Contending with group image: The psychology of stereotype and social identity threat. In: Zanna MP, editor. Advances in experimental social psychology. Vol. 34. San Diego, CA: Academic Press; 2002. pp. 379–440. [Google Scholar]
- Steele J, James JB, Barnett RC. Learning in a man’s world: Examining the perceptions of undergraduate women in male-dominated academic areas. Psychology of Women Quarterly. 2002;26:46–50. [Google Scholar]