Emergent states in heavy-electron materials

Abstract

We obtain the conditions necessary for the emergence of various low-temperature ordered states (local-moment antiferromagnetism, unconventional superconductivity, quantum criticality, and Landau Fermi liquid behavior) in Kondo lattice materials by extending the two-fluid phenomenological theory of heavy-electron behavior to incorporate the concept of hybridization effectiveness. We use this expanded framework to present a new phase diagram and consistent physical explanation and quantitative description of measured emergent behaviors such as the pressure variation of the onset of local-moment antiferromagnetic ordering at T_N, the magnitude of the ordered moment, the growth of superconductivity within that ordered state, the location of a quantum critical point, and of a delocalization line in the pressure/temperature phase diagram at which local moments have disappeared and the heavy-electron Fermi surface has grown to its maximum size. We apply our model to CeRhIn₅ and a number of other heavy-electron materials and find good agreement with experiment.

Heavy-electron materials provide a unique f-electron laboratory for the study of the interplay between localized and itinerant behavior. At comparatively high temperatures, itinerancy emerges as the localized f-electrons collectively reduce their entropy by hybridizing with the conduction electrons to form a new state of matter, an itinerant heavy-electron Kondo liquid that displays scaling (non-Landau Fermi liquid) behavior (1–8). The emergent Kondo liquid coexists with the hybridized spin liquid that describes the lattice of local moments whose magnitude has been reduced by hybridization until one reaches the comparatively low temperatures at which unconventional superconductivity and hybridized local-moment antiferromagnetism compete to determine the ground state of the system over a wide regime of pressures. In practice, the phases often coexist, as experiments on the 115 [CeRhIn₅ (9–11) and CeCoIn₅ (12)] and 127 [CePt₂In₇ (13)] Kondo lattice materials demonstrate. A major challenge for the heavy-electron community has been finding a consistent framework and simple phenomenological description of their measured emergent behaviors that include the pressure variation of the onset of antiferromagnetic ordering at T_N, the growth of superconductivity within that ordered state, the onset of quantum critical behavior, and the growth of the heavy-electron Fermi surface.

Although progress has been made on developing a phenomenological theory of Kondo lattice materials (6), we do not yet possess a microscopic theory of the emergence of the Kondo liquid as a new quantum state of matter that displays universal behavior below a characteristic temperature (1–3, 5), T^∗, or a satisfactory general framework for characterizing the conditions necessary for the emergence of the competing low-temperature ordered states, because experiments (4) have shown that the seminal phase diagram proposed by Doniach (14) does not apply to most materials. In this paper we introduce the concept of “hybridization effectiveness” as the organizing principle responsible for the emergence of low-temperature order and show it leads to a new phase diagram that provides a realistic explanation for the emergence of different low-temperature phases for Kondo lattice materials. The role of hybridization effectiveness in reducing the entropy of the lattice of local moments present at high temperatures can be described quantitatively through the pressure dependence of a simple parameter present in the phenomenological two-fluid description (1–8) of heavy-electron materials whose magnitude can be determined from fits to experimental data. We show that our approach makes possible a consistent physical explanation and quantitative description of the low-temperature behaviors of a number of heavy-electron materials and predicts the presence of a delocalization line in the pressure/temperature phase diagram. It offers promise of serving as a standard model of heavy-electron emergent behaviors and providing the basis for the development of a microscopic theory that explains these.

Model

Hybridization Effectiveness.

In the two-fluid description, the heavy-electron Kondo liquid emerges below a characteristic temperature, T^∗, as a collective hybridization-induced instability of the spin liquid that describes the lattice of local moments coupled to background conduction electrons. T^∗ is determined by the effective Ruderman–Kittel–Kasuya–Yosida (RKKY) interaction between the nearest-neighbor local moments (4); the emergent scaling behavior of the Kondo liquid is characterized by the heavy-electron order parameter (3),

[1]

which extends from T^∗ to a comparatively low temperature, T₀, at which ordering begins to set in (7). Over this temperature range it coexists with the “hybridized” quantum spin liquid, whose individual components (that still interact through the intersite RKKY coupling) possess a spectral weight that diminishes with lowering temperature as f_l(T) = 1 - f_h(T).

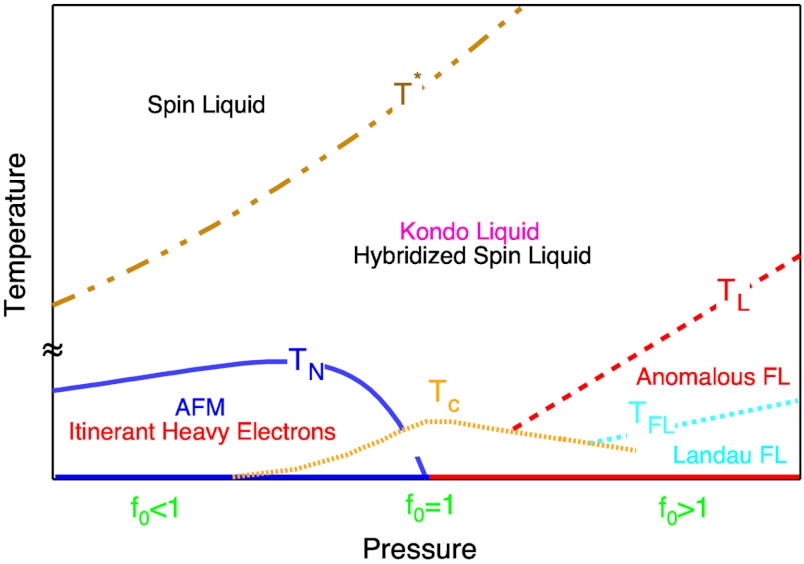
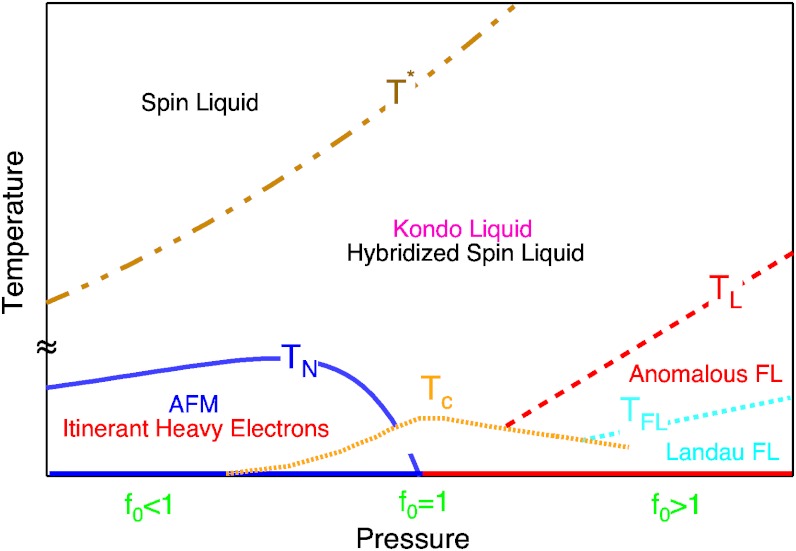
The pressure-dependent parameter, f₀, in Eq. 1 provides a direct measure of the ultimate effectiveness of the underlying collective hybridization in reducing the magnitude of strength of each localized f-moment. Importantly, as may be seen in the schematic summary of our findings in Fig. 1, it constrains the nature of the ground state. As long as f₀ < 1, hybridization is always incomplete, so antiferromagnetic local-moment order becomes possible at a temperature reduced by hybridization: It takes place in the presence of itinerant heavy electrons that can become superconducting, so that over a wide range of pressures local-moment order coexists with superconductivity of magnetic origin. At f₀ = 1, the collective hybridization of local moments becomes complete at T = 0; hence, if superconductivity is suppressed by application of a strong enough magnetic field, one expects to find a quantum critical point, denoting a T = 0 transition between f-electron local order and a fully itinerant heavy-electron state. For f₀ > 1, the hybridization of local moments is complete and the Fermi surface will have grown to its maximum size at some finite temperature, T_L; Kondo liquid scaling behavior is expected between T^∗ and T_L, whereas T_L is fixed by f₀ and T^∗. From Eq. 1, we have:

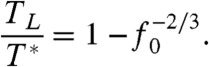[2]

For f₀ close to 1, because of the proximity of a quantum critical point, immediately below T_L one does not expect to find Landau Fermi liquid (FL) behavior; instead, the properties of the heavy electrons will be determined by their coupling to quantum critical fluctuations; anomalous behavior, including further mass enhancement, continues until one reaches the still lower characteristic temperature, T_FL, shown in Fig. 1, at which transport and other properties are no longer dominated by the coupling of quasiparticles to quantum critical fluctuations, and one finds Landau Fermi liquid behavior.

Fig. 1.

A proposed phase diagram in which a quantum critical point at f₀ = 1 separates weak and strong hybridizing materials: If f₀ < 1, antiferromagnetic local-moment order in the presence of heavy electrons sets in below T_N; for f₀ > 1, one has complete delocalization of f-electrons along a line T_L, with the heavy electrons forming a Landau Fermi liquid below T_FL. Around the quantum critical point at f₀ = 1, the heavy electrons may condense into superconductivity below T_c because of magnetic quantum fluctuations. T^∗ marks the onset of Kondo liquid emergence produced by collective hybridization.

The Hybridized Quantum Spin Liquid.

The magnetic properties of the hybridized spin liquid can be studied by assuming its dynamic spin susceptibility takes a mean-field form,

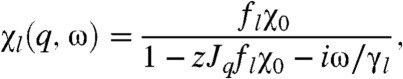[3]

where χ₀ is the local susceptibility of an individual f-moment, z is the coordination number, γ_l is the local relaxation rate, and J_q is the RKKY exchange coupling. For simplicity, the magnetic moment of the f-spins and the Boltzmann constant are set to unity. The hybridized spin liquid will begin to order at a Néel temperature that is, according to Eq. 3, determined by:

[4]

All local physics can be included in the local-moment static susceptibility χ₀. For example, crystal field effects become important when the crystal field splitting from the ground-state doublet is smaller than or comparable to T^∗; if the crystal field configuration is known and plays a role, it can be easily taken into account in χ₀. In this paper, we take for simplicity χ₀ = C/T, where C is the Curie constant. For T > T^∗, Eq. 3 reduces to the Curie–Weiss form of the static susceptibility, χ_l(0,0) = C/(T + θ), where θ = CzJ_q=0. We have then

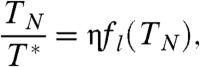[5]

where the parameter η = CzJ_Q/T^∗ reflects the effect of the crystal lattice and magnetic frustration on T_N, whereas f_l(T_N) reflects the role played by collective hybridization in reducing the Néel temperature. Because of the RKKY nature of both T^∗ and J_Q (4), η is pressure-independent.

The magnitude of the ordered moment μ² is determined by the local-moment order parameter:

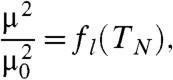[6]

where μ₀ is the local-moment strength above T^∗. Where accurate measurements of the strength of the ordered moment in the antiferromagnetic state exist, a good test of our model is to combine Eqs. 5 and 6 to obtain an expression that relates three experimentally measurable quantities at a fixed value of η,

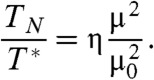[7]

Because T^∗ increases with increasing pressure, whereas μ decreases, it follows that in general the T_N versus pressure curve should exhibit the maximum shown in Fig. 1.

In applying Eqs. 5 and 6 to experiment, one needs to take relocalization effects into account. As discussed in apRoberts-Warren et al. (7) and Shirer et al. (8), Knight shift experiments demonstrate that Kondo liquid scaling ends at a temperature T₀, below which local-moment ordering begins to win out over collective hybridization in determining the temperature evolution of f_l(T). As the temperature is lowered, the rate of collective hybridization is first reduced and then reversed—i.e., the spectral weight of f_l(T) begins to increase as the temperature is further lowered. For the two materials for which this relocalization has been explored in detail, it turns out that f_l(T_N) is approximately f_l(T₀) and T₀ is approximately 2T_N.

Determining f₀.

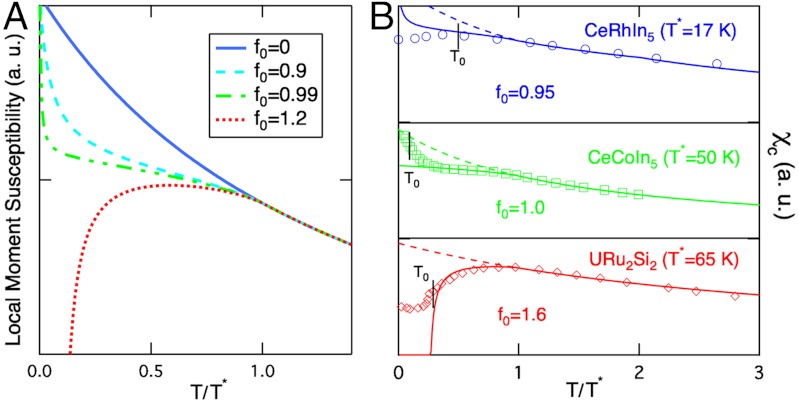
We can use Eq. 3 to determine f₀ from measurements of the static spin susceptibility if we make the physically reasonable assumption that the hybridized spin liquid contribution is dominant for a broad range of temperatures below T^∗. Such approximation is reasonable because of the large magnetic response of the local moments seen at temperatures above T^∗. Fig. 2 shows the evolution of χ_l(0,0) with f₀ and the fit to experimental data in several compounds—from the antiferromagnet CeRhIn₅ (15) to the superconductor CeCoIn₅ (16) to the 5f compound URu₂Si₂ that exhibits hidden order (17). We find f₀ = 0.95 for CeRhIn₅, 1.0 for CeCoIn₅, and 1.6 for URu₂Si₂. With increasing f₀, we find a crossover from peak to plateau to peak structure in the uniform susceptibility of exactly the kind that has been observed in many heavy-electron materials. However, the origin of the peak seen in materials with f₀ < 1, such as CeRhIn₅, and those in which f₀ > 1, such as URu₂Si₂, is different. For f₀ > 1, the peak comes from rapid delocalization of the f-moments at approximately T^∗; for f₀ < 1, it is related to the onset of relocalization of the Kondo liquid as a precursor of the antiferromagnetic ordering at T₀ ≪ T^∗ (7, 8).

Fig. 2.

The role played by hybridization effectiveness in determining the static magnetic susceptibility. (A) Schematic illustration of the influence of f₀ on χ_l(0,0) for a fixed value of θ/T^∗ = 1.4. (B) A comparison of our calculated and experimental values of the c-axis susceptibility χ_c for three materials: CeRhIn₅ (15), CeCoIn₅ (16), and URu₂Si₂ (17). The solid lines are theoretical fits with θ/T^∗ = 1.4, 1.35, and 3.5, respectively; also shown is the temperature, T₀, at which scaling behavior is altered by the onset of low-temperature order.

The Kondo Liquid.

The Kondo liquid contributes to the magnetic entropy and other material properties that display anomalous behavior, as well as physical quantities, such as transport and superconductivity, that originate uniquely in the itinerant f-electrons. The Kondo liquid entropy S_h can be obtained by integrating over the universal scaling expression for its specific heat C/T ∝ [1 + ln(T^∗/T)] (3) and recognizing that continuity at T^∗ requires that S_h(T^∗) = S_l(T^∗) = R ln 2 (R is the gas constant). When the scaling behavior of the Kondo liquid ends at a temperature T_x (= T_L if f₀ > 1), the two-fluid model predicts that the heavy-electron entropy will be given by (6):

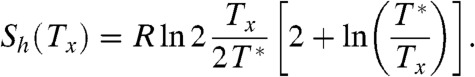[8]

Below T_L, no local moments are present; only heavy electrons are responsible for the measured system behavior and a one-fluid picture is recovered, as may be verified in Knight shift measurement. The Fermi liquid-specific heat coefficient, γ_h, at lower temperatures can then be estimated:

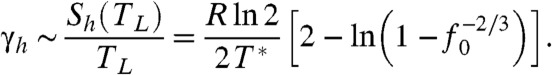[9]

In general, even when f₀ < 1 or T > T_L, the specific heat coefficient can still be calculated using Eq. 11.

Results

Application to CeRhIn₅.

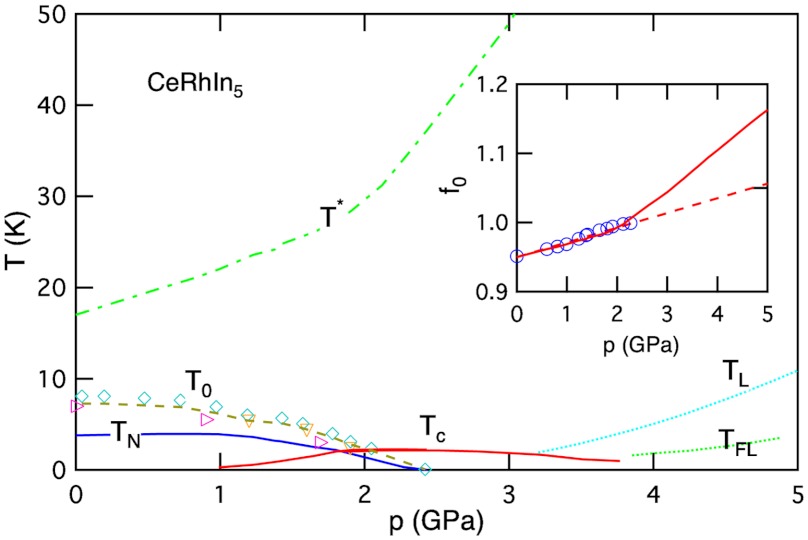
CeRhIn₅ provides an excellent proving ground for the approach we have described. Our proposed phase diagram for CeRhIn₅ is given in Fig. 3, and resembles closely the experimental pressure/temperature phase diagram derived from the resistivity exponent (18). The Knight shift experiments of Shirer et al. (8) show that f_l(T_N) ≈ f_l(2T_N) at ambient pressure; we assume this to be true in the entire pressure range in the following analysis, with f_l(2T_N) given by the scaling formula in Eq. 1.

Fig. 3.

The proposed phase diagram of CeRhIn₅. The pressure dependence of T_N and T_c are taken from refs. 18 and 19, whereas the coherence temperature T^∗ is that determined by Yang et al. (3, 4, 6). The experimental points determining T₀, the cutoff temperature for Kondo liquid scaling, reflect its signature in several different experimental probes: a pseudo–gap-like feature in the spin-lattice relaxation rate (20), a change in the anomalous Hall effect (21) and inelastic neutron-scattering spectrum (22), and a peak in the measured “anomalous” Kondo liquid susceptibility (15). T_L is the delocalization temperature expected if f₀(p) is approximately Jρ, where J is the local Kondo coupling and ρ is the density of states of the conduction electrons. (Inset) The hybridization parameter f₀(p) as a function of pressure: The points are derived from T_N(p); the solid line is its behavior at higher pressures if f₀ scales with the Kondo coupling, Jρ, in the vicinity of the quantum critical point; and the dashed line is a simple linear extrapolation.

Antiferromagnetism.

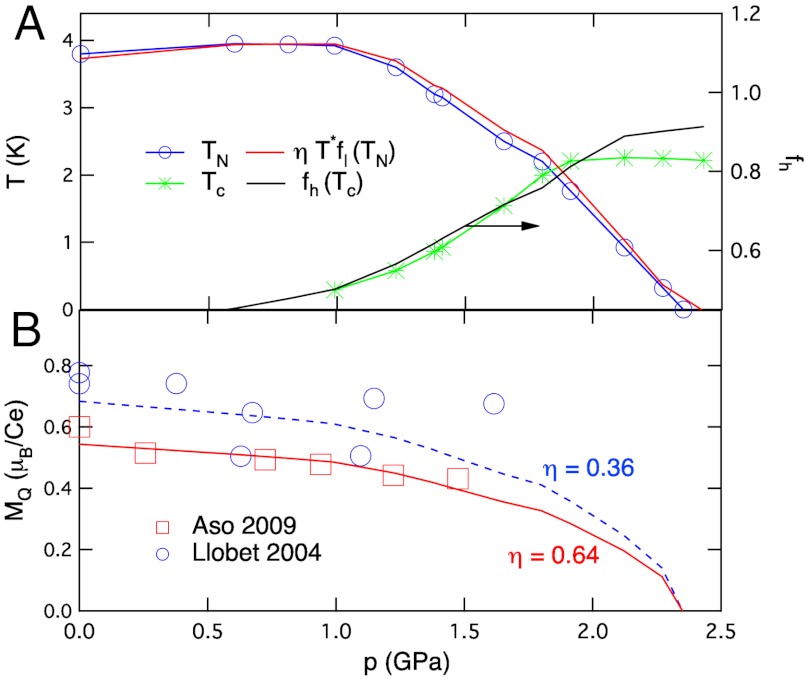
On applying Eqs. 5, 6, and 7 to CeRhIn₅, we obtain the results for the variation of the Néel temperature with pressure, and of the magnitude of the ordered moment found in the Néel state that is given in Fig. 4. Despite its simplicity, our model yields results in good agreement with experiment (19–24). Fig. 4A compares the experimental data of T_N and ηT^∗f_l(2T_N). The best fit to the Néel temperature yields η = 0.36 for f₀ = 0.95. On taking the strength of the local moments above T^∗ to be 0.92 μ_B (25), η = 0.36 gives the right magnitude of the ordered moment when Eq. 7 is used together with the pressure variation of T_N. A corresponding best fit to the results of Aso et al. (23) and Llobet et al. (24) requires that η = 0.64 and 0.32, respectively. Our results are seen to be more consistent with those of Llobet et al. (24).

Fig. 4.

The Néel temperature, superconducting transition temperature, and ordered moments as a function of pressure in CeRhIn₅. (A) Comparison between experimental T_N(p) (18, 19) and ηT^∗f_l(T_N), and between T_c(p) and f_h(T_c) derived from f₀(p) in Fig. 3, Inset, for CeRhIn₅. With f₀ = 0.95, the best fit requires η = 0.36 in the presence of relocalization. (B) The pressure evolution of the Ce-staggered moment at Q = (π,π). Open squares are data reported by Aso et al. (23) and open circles are reproduced from Llobet et al. (24). The dashed line is our theoretical result with η = 0.36 and the solid line is the fit for Aso’s data with η = 0.64. For Llobet’s data, the best fit requires η = 0.32.

In our mean-field approach, the influence of the crystalline lattice and magnetic frustration are incorporated in the coordination number z and η = CzJ_Q/T^∗. To illustrate a reasonable range for these parameters, we consider a 2D square lattice for which the RKKY coupling has the usual form J_q = J_n[cos(q_x) + cos(q_y)] + 2J_nn cos(q_x) cos(q_y), where J_n and J_nn are the nearest- and next-nearest neighbor couplings, respectively. We have θ = 2Cz|J_n + J_nn| and ηT^∗ = 2Cz|J_n - J_nn| for Q = (π,π). If we take the values η = 0.36 and θ/T^∗ = 1.4, obtained in the susceptibility fit in Fig. 2B, we find a frustration ratio J_nn/J_n ≈ 0.6. This indicates T^∗/2Cz|J_n| of approximately 1.1, consistent with its origin in nearest-neighbor RKKY coupling (4).

Itinerant Electrons and Superconductivity Within the Néel State.

Our model provides a natural explanation for the appearance of superconductivity within the Néel state and the pressure dependence of its transition temperature, T_c. Quite generally, local-moment ordering takes place before the relocalization process is complete. Below T_N, a substantial number of heavy electrons coexist with the ordered local moments in the antiferromagnetic phase, a fraction that increases as the pressure increases. For example, in CeRhIn₅, despite relocalization, f_h(T_N) is approximately 0.37 at ambient pressure. How do these heavy electrons lose their entropy within the Néel state? There are two possibilities: (i) The relocalization process continues within the Néel state; or (ii) the heavy-electrons order, most likely in an unconventional superconducting state because there are ample magnetic fluctuations present that can bring this about (26). Both likely occur in practice.

Where superconductivity emerges, it is appealing to argue that, to a first approximation, T_c scales with the density of heavy electrons present, an argument familiar from the well-known Uemura plot for the cuprate superconductors (27). We test this hypothesis by comparing the growth in T_c with pressure with the corresponding growth in the density of heavy electrons. As may be seen in Fig. 4A, the increase in T_c seen from 1 GPa to 1.75 GPa mirrors the increase in the density of heavy electrons able to participate in superconductivity, f_h(T_c) = f_h(2T_N) assuming no further relocalization below T_N. Further confirmation of this view of Kondo liquid behavior comes from the scaling of the Knight shift anomaly above and below T_c seen in CeCoIn₅ (5), and the observation that T_c is maximum in the vicinity of the critical pressure at which some part of the heavy-electron Fermi surface can begin to localize.

Fermi Liquid Regime.

At pressures p > 2.35 GPa, the hybridization parameter is f₀ > 1, so the ground state of CeRhIn₅ should be a Fermi liquid if one applies a magnetic field strong enough to suppress superconductivity. At 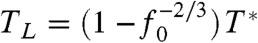, when hybridization is complete, our model predicts a heavy electron-specific heat that depends only on T^∗ and f₀ and is given by Eq. 9. T_L can be determined from a measurement of the Fermi surface, which should reach its maximum size there. Our analysis at low pressures suggests that f₀ may scale with the Kondo coupling, J, in the vicinity of the quantum critical point. If this scaling extends to higher pressures, it leads to the results shown for T_L in Fig. 3. Below T_L, the f-electrons form a Fermi liquid, but its quasiparticles, being scattered by quantum critical spin fluctuations, do not exhibit the classic properties proposed by Landau until one reaches a lower-temperature T_FL.

Spin-Lattice Relaxation Rate.

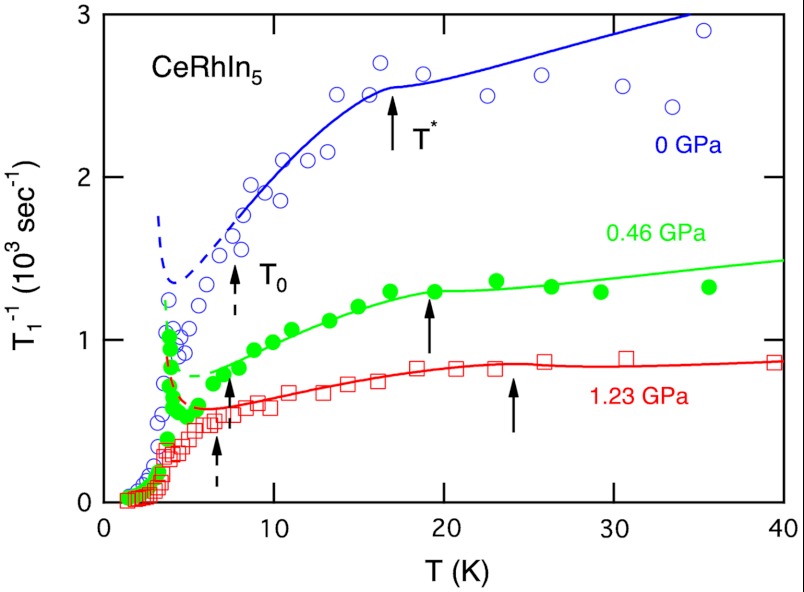
Nuclear magnetic/quadrupole resonance (NMR/NQR) experiments on the pressure dependence of the spin-lattice relaxation rate provide a further confirmation of the approach developed here. We assume that the spin-lattice relaxation rate between T^∗ and T₀ is dominated by local-moment behavior and calculate it using the Moriya formula (28),

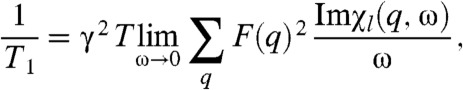[10]

where γ is the gyromagnetic ratio and F(q)² is the form factor. Following ref. 29, we use 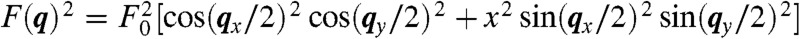, where x, the only free parameter, defines the anisotropy of the hyperfine coupling. On using the values of f₀(p) given in Fig. 3 (Inset) and assuming that J_q is close to its value for a 2D square lattice, we obtain the fit to the NQR data in CeRhIn₅ shown in Fig. 5. We see there the expected change in slope caused by hybridization for 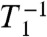and that our model provides a fit to experiment above T₀, with T^∗ = 17 K at 0 GPa, 20 K at 0.46 GPa, and 25 K at 1.23 GPa, respectively, and an anisotropy parameter x at ambient pressure of 0.08 that increases to 0.12 at 0.46 GPa and 0.2 at 1.23 GPa, in agreement with earlier estimates (29). The behavior found above T^∗ reflects the comparatively weak local-moment interaction; the temperature dependence seen between T₀ and T_N originates from the precursor antiferromagnetic fluctuations (22) and Kondo liquid relocalization (7), and cannot be calculated using the present mean-field approximation. The local relaxation rate γ_l has been assumed to be a constant in the fit; a temperature-dependent γ_l may yield improved agreement between theory and experiment.

Fig. 5.

Comparison of measured and calculated NQR spin-lattice relaxation rate at ambient pressure, 0.46 GPa and 1.23 GPa, in CeRhIn₅ (20). The solid lines are our theoretical fit with T^∗ = 17 K, 19 K, and 24 K, and x = 0.08, 0.12, and 0.2, respectively.

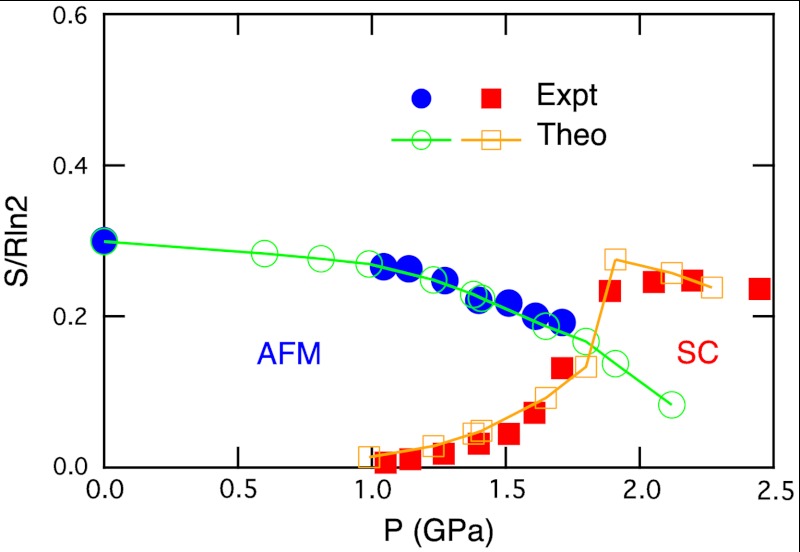
Magnetic Entropy.

The magnetic entropy is another important quantity of experimental interest. In many heavy-electron antiferromagnets, one finds a small entropy release at T_N despite the presence of large ordered moment. As will be shown below, our expanded two-fluid model explains these apparently contradictory results as a direct consequence of hybridization and yields agreement with experiment for the magnetic entropy of a number of heavy-electron materials.

Above T^∗, the magnetic entropy is that of localized f-electron moments in a ground-state doublet that is assumed to have the full magnetic entropy R ln 2. At lower temperatures, both the local moments and the Kondo liquid contribute and the total magnetic entropy is given by ref. 6 as:

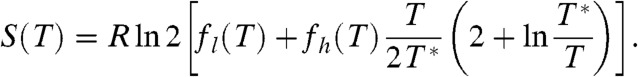[11]

We expect Eq. 11 to be valid down to a temperature T₀, at which low-temperature order begins to compete with hybridization in reducing the local-moment entropy. Above T₀ and for arbitrary f₀, where Eq. 9 does not apply, the specific heat coefficient can still be estimated from the temperature derivative of Eq. 11. For CeRhIn₅, as shown in Fig. 3, a number of different experimental probes suggest that T₀ is of order of 2T_N; importantly, the measurements by Shirer et al. of the Knight shift anomaly show that Kondo liquid behavior is strongly suppressed below T₀, indicating a rapid relocalization of itinerant heavy electrons (7, 8).

The physics below T₀ require that Eq. 11 be modified. In addition to a deviation from scaling in the order parameters f_h and f_l, the contribution of each component to the magnetic entropy is expected to be reduced from its value at T₀ by the approach of antiferromagnetic order. On comparing the magnetic entropy calculated from Eq. 11 with experiment for CeRhIn₅ (19), we find that a factor of r_N of approximately 0.35 has to be introduced at ambient pressure in order to obtain agreement with experiment; a reduction of this size is justified by the similar ratio S(T_N)/S(T₀) of approximately 0.4 found in experiment (19). As may be seen in Fig. 6, with this factor one obtains good agreement with experiment for pressures up to 1.75 GPa. In contrast, at higher pressures, where superconductivity takes over and the Kondo liquid scaling persists down to T_c, no corrections are needed to Eq. 11, which yields good agreement with experiment for the entropy at T_c.

Fig. 6.

Comparison of measured (19) and calculated magnetic entropies at the Néel temperature, T_N, and superconducting transition temperature, T_c, in CeRhIn₅ as a function of pressure (15, 19). Theoretical predictions are obtained by using f₀(p) in Fig. 3, Inset.

In calculating the entropy at the superconducting transition deep within the antiferromagnetic phase (1.0 < p < 1.75 GPa) shown in Fig. 6, we have excluded the contribution from the ordered moments, whose fraction, a(T_c) = (1 - T_c/T_N)^0.5, has been measured (24), and have assumed the Kondo liquid-specific heat does not change between T_N and T_c. The entropy release of the coexisting state is then given by

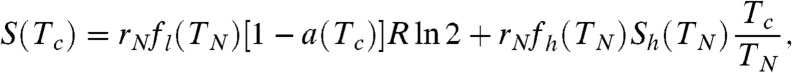[12]

and agrees well with experiment.

At first sight, given the comparatively large magnitude of the ordered moment in CeRhIn₅ (0.6–0.8 μ_B), the entropy released by the antiferromagnetic ordering of the local moments appears unexpectedly small, being only approximately 30% R ln 2 (15). A little thought shows that the reduction comes in three sequential stages, corresponding to the entropy release at T_N, T₀, and T^∗, respectively. When antiferromagnetism is destroyed at T_N = 3.8 K, only a fraction (approximately 63%) of the spectral weight of the strongly hybridized f-moments is available to become “free”; the rest (approximately 37%) of that spectral weight has already gone to the Kondo liquid, whose entropy is already much reduced because of strong entanglement between conduction and f-electrons. It follows that above T_N, the entropy of the freed moments is then only released at a higher temperature T₀ of approximately 8 K because of strong magnetic correlations close to the phase transition (8), whereas the total spin entropy will not be fully recovered until all the correlated Kondo liquid has been transformed into uncorrelated f-moments above T^∗ = 17 K. The continuous and slight change of the magnetic entropy at the ordering temperature as one moves from antiferromagnetism to superconductivity with increasing pressure range requires a gradual change of character of f-moments and argues against a dramatic change of hybridization across the quantum critical region.

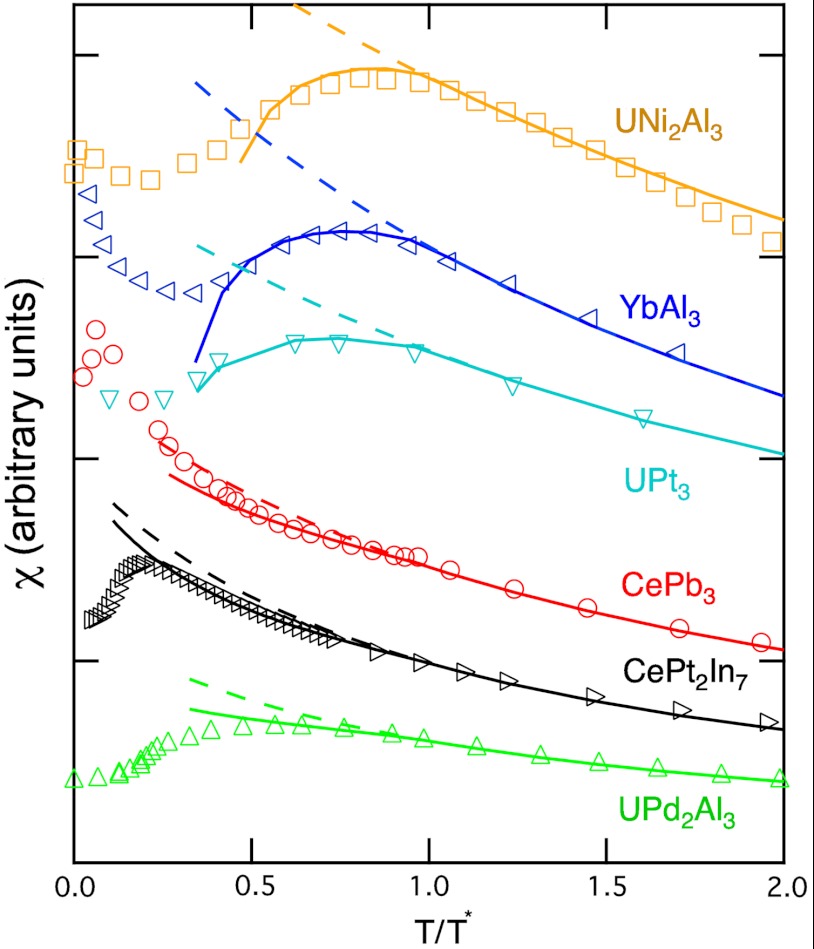
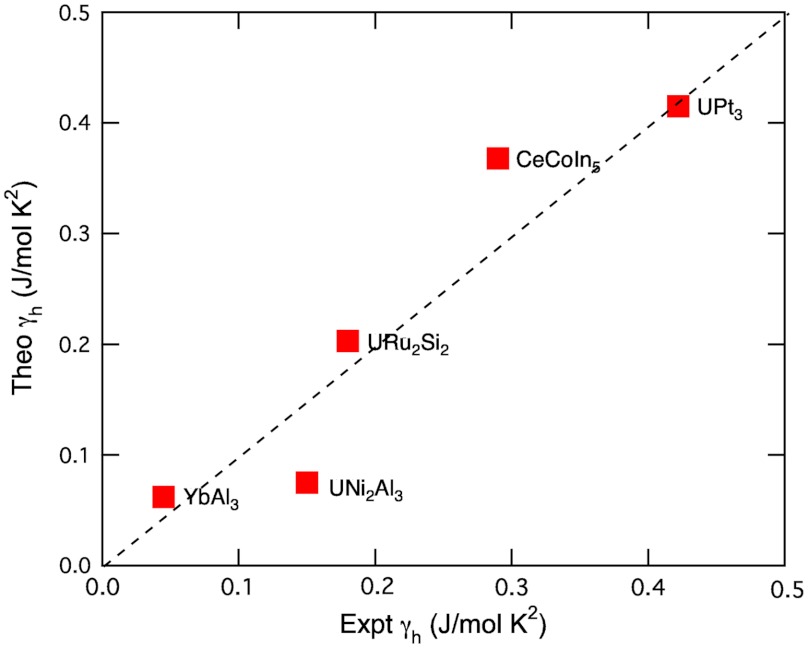
Application to Other Materials.

In applying our model to other prototypical heavy-electron materials, we focus on the relation between f₀ and the magnetic/nonmagnetic ground state, and the entropy release of the ordered state (when f₀ < 1) or the residual specific heat coefficient of the Fermi liquid (if f₀ > 1), because these only require experimental input at ambient pressure. Our results are summarized in Fig. 7 and Table 1, and discussed in the subsections below. Because they are strong hybridizers (f₀ > 1), we conclude that UNi₂Al₃ must be a spin-density wave antiferromagnet in which antiferromagnetic order originates from Fermi surface nesting of the heavy-electron Kondo liquid (30), whereas the hidden-order transition in URu₂Si₂ must be caused by a Kondo liquid instability (31). As may be seen in Fig. 8, our model [Eq. 9] also yields results for the low-temperature Fermi liquid-specific heat of a number of materials that are surprisingly close to their experimental values (17, 32–35), which demonstrates that, for these materials, the average heavy-electron effective mass is determined by the onset of collective hybridization at T^∗ and its effectiveness, f₀.

Fig. 7.

Magnetic susceptibility of several prototypical heavy-electron materials. The solid lines are our theoretical fit using the mean-field formula and the dashed lines are the Curie–Weiss susceptibility. The fitting parameters are shown in the text.

Table 1.

Hybridization effectiveness and low-temperature order

Materials	T∗ (K)	TN/L/c (K)	f0	Order
Local-moment order
CePt2In7	41	5.6	0.4	AFM
CePb3	16	1.1	0.5	AFM
UPd2Al3	60	14.3	0.8	AFM
CeRhIn5	17	3.8	0.95	AFM
Kondo liquid order
CeCoIn5	50	2.3	1.0	SC
UPt3	25	5	1.4	SC
YbAl3	160	38	1.5	FL
URu2Si2	65	17.5	1.6	HO
UNi2Al3	120	39	1.8	SDW

AFM, antiferromagnetism; SC, superconductivity; FL, Fermi liquid; HO, hidden order; SDW, spin-density wave antiferromagnetism.

Fig. 8.

A comparison between the values of the specific heat coefficient predicted by our model and experiment for materials with f₀≥1 (17, 32–35).

CeCoIn₅.

For this material, the Kondo liquid temperature T^∗ = 50 K has been determined using a number of experimental probes (3, 4). On using Eq. 3, we show in Fig. 2B that f₀ of approximately 1 provides a good fit to the susceptibility data (16, 35), a conclusion that is consistent with the expectation that CeCoIn₅ is close to a magnetic quantum critical point (36). We find S(T_c) = 0.18 R ln 2, close to the experimental value of 0.2 R ln 2 at T_c = 2.3 K (35). Taking T_c as the cutoff temperature, the predicted specific heat coefficient at T_c and zero field using Eq. 11 is approximately 368 mJ/mol K², comparable to the experimental value of approximately 290 mJ/mol K² (17).

URu₂Si₂.

As may be seen in Fig. 2B, our best fit to the c-axis susceptibility of URu₂Si₂ gives the hybridization parameter f₀ = 1.6, and T_L of approximately T_HO. Below T_L, all f-electrons become itinerant so that a single-component picture is recovered and the Knight shift becomes once again proportional to the susceptibility. This allows us to subtract the spin susceptibility of the two components without uncertainty, and the detailed analysis given in ref. 8 yields similar results for f₀ and T_L. These suggest that most local moments have disappeared by the time one reaches T_HO, so that the hidden-order transition must have an itinerant and strongly correlated nature, consistent with experimental observation of gap opening (37, 38) and Fermi surface reconstruction at T_HO (39). Our predicted entropy release at T_HO is 0.44 R ln 2, somewhat larger than the experimental value of 0.3 mJ/mol K² (17). The predicted specific heat coefficient without hidden order is approximately 203 mJ/mol K², close to the experimental extrapolated value of 180 mJ/mol K².

CePt₂In₇.

Using θ = T^∗ = 41 K, we find f₀ = 0.4 from a fit to the susceptibility, in which only the local-moment contribution is included. In an earlier analysis that included the Kondo liquid contribution and assumed a Wilson ratio R_W = 2 (7), f₀ was found to be approximately 1, a result in obvious contradiction with the magnetic ground state. The contradiction leads us to conclude that one should not in general assume that R_W = 2, but, rather, determine it on a case-by-case basis. For this material, NMR measurements point to a relocalization temperature T₀ of approximately 14 K, below which the magnetic entropy is strongly suppressed. Our scaling result yields S(T_N) = 0.76 R ln 2, which is very much larger than the experimental value of approximately 0.2 R ln 2 (12): Just as was the case with CeRhIn₅, relocalization effect is large between T₀ = 14 K and T_N = 5.6 K, a result that is consistent with the NMR experimental results (7).

CePb₃.

The susceptibility of CePb₃ has a plateau below T^∗ = 16 K, followed by a peak before the magnetic transition at T_N = 1.1 K (40). Our best fit gives f₀ = 0.5 and θ = 25 K, and yields an entropy release of approximately 0.62 R ln 2 at T_N, which is large compared to the experimental value of 0.32 R ln 2, a discrepancy that is likely caused by the rapid suppression of the magnetic entropy between approximately 3 K and T_N (see figure 8 in ref. 40).

UPt₃.

Our fit to the susceptibility of UPt₃ gives f₀ = 1.4, θ = 71 K, and T^∗ = 25 K. We find T_L of approximately 5 K, consistent with the deviation from scaling seen in the Knight shift anomaly (3), and γ_h = 415 mJ/mol K², in good agreement with the experimental value of 422 mJ/mol K² (33).

UM₂Al₃.

UPd₂Al₃ and UNi₂Al₃ are both antiferromagnetic at low temperatures but, as our fit to the susceptibility shows, their physical origins are different. Because of crystal field effects, for UPd₂Al₃ (4, 41) we can only give a rough estimate of θ = T^∗ = 60 K and f₀ = 0.8, whereas for UNi₂Al₃ (34) we find T^∗ = 120 K, θ = 420 K, and f₀ = 1.8. These quite different values of f₀ strongly suggest that the magnetic ground state of UPd₂Al₃ originates in ordering of its local moments, whereas that of UNi₂Al₃ must reflect spin-density wave ordering that originates in the Fermi surface nesting of the itinerant Kondo liquid.

Because for both materials the specific heat is enhanced by antiferromagnetic fluctuations only in a narrow range above T_N, we can safely neglect the relocalization correction; on doing so we obtain S(T_N) of approximately 0.69 R ln 2 for UPd₂Al₃ and 0.05 R ln 2 for UNi₂Al₃, in good agreement with the experimental values of 0.67 R ln 2 for UPd₂Al₃ and 0.12 R ln 2 for UNi₂Al₃ (30). For UNi₂Al₃, we find T_L = 39 K and γ_h = 75 mJ/mol K², somewhat smaller than the experimental value of 150 mJ/mol K² (16).

YbAl₃.

Our fit to the susceptibility of YbAl₃ (32) gives f₀ = 1.5, θ = 340 K, and T^∗ = 160 K. This is consistent with the nonmagnetic ground state of this compound, for which we find T_L = 38 K. The specific heat coefficient is estimated to be γ_h = 62 mJ/mol K², consistent with the experimental value of 45 mJ/mol K².

For materials for which f₀ cannot be easily obtained, one can still estimate the entropy release by making the approximation f₀ = 1 in Eq. 11. For antiferromagnetic compounds with f₀ < 1, a larger f₀ results in a smaller S(T_N), which may cancel the effect of relocalization. Taking CePd₂Si₂ as an example, we predict S(T_N) = 0.62 R ln 2 using T_N = 9.9 K and T^∗ = 40 K (4), quite close to the experimental value of S(T_N) = 0.68 R ln 2 (42, 43). This suggests a rough relation between S(T_o) and T_o/T^∗ (T_o = T_c,T_N, T_L, etc.) that can be easily applied to other materials in the future.

Conclusions

In summary, our introduction of hybridization effectiveness into the two-fluid description yields a viable alternative to the Doniach phase diagram (14) for heavy electrons, and its determination from experiment leads to a unified phenomenological explanation of heavy-electron magnetic properties. It will be important to extend the present analysis to a number of other heavy-electron materials and to determine the expected magnetic-field dependence of the hybridization-effectiveness parameter, f₀. An analysis of future experiments should yield a hybridization-based 3D pressure/magnetic-field phase diagram, tell us whether it is possible to place all heavy-electron materials on it, and establish the localization line for those materials with f₀ > 1. Experiments may also provide further information on the physical origin of f₀—e.g., does it scale with Jρ and so mirror the Kondo coupling? At this stage, the improved two-fluid description presented here shows considerable promise as a candidate phenomenological standard model of heavy-electron emergent behaviors and should provide the basis for the development of a microscopic theory that explains these behaviors as a natural consequence of the coupling of the local-moment spin liquid to the conduction electron sea in which it is immersed.