Skip to main content
PMC home page
Scientific Reports logoLink to Scientific Reports
. 2012 Nov 12;2:796. doi: 10.1038/srep00796

Vortex Domain Structure in Ferroelectric Nanoplatelets and Control of its Transformation by Mechanical Load

W J Chen 1,2, Yue Zheng 1,2,a, Biao Wang 1,2,b
PMCID: PMC3495285  PMID: 23150769

Abstract

Vortex domain patterns in low-dimensional ferroelectrics and multiferroics have been extensively studied with the aim of developing nanoscale functional devices. However, control of the vortex domain structure has not been investigated systematically. Taking into account effects of inhomogeneous electromechanical fields, ambient temperature, surface and size, we demonstrate significant influence of mechanical load on the vortex domain structure in ferroelectric nanoplatelets. Our analysis shows that the size and number of dipole vortices can be controlled by mechanical load, and yields rich temperature-stress (T-S) phase diagrams. Simulations also reveal that transformations between “vortex states” induced by the mechanical load are possible, which is totally different from the conventional way controlled on the vortex domain by the electric field. These results are relevant to application of vortex domain structures in ferroelectric nanodevices, and suggest a novel route to applications including memories, mechanical sensors and transducers.

Nanoscale ferroelectrics and multiferroics have been actively investigated for many years. Nanoscale ferroelectrics and multiferroics often retain bulk properties, and even exhibit enhanced or novel properties including electronic, optoelectronic, electrochemical, electromechanical and magnetoelectric, properties, due to effects of finite size, surfaces and interfaces. Therefore, nanoscale ferroelectrics and multiferroics play important roles in the fabrication and miniaturization of nanoscale functional devices1,2,3,4,5,6,7,8,9. More recently, exotic ferroelectric domain structures described as topological defects in the polarization field have attracted an enormous amount of attention due to their fundamental scientific interest and potential applications in domain engineering10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,28. In particular, toroidal polarization patterns consisting of flux-closure domains (so-called vortex domain structures) are found in low-dimensional ferroelectrics with extreme geometric confinement. As a new kind of polarization ordering, vortex domain structures are expected to exhibit distinctive characteristics and novel coupling with external fields, which could open exciting opportunities in designing novel nano-memories, sensors and transducers, and other devices. Nevertheless, experimental and theoretical studies for the vortex domain structures in low-dimensional ferroelectrics have proved to be difficult, and properties determined by the vortex domain structures in low-dimensional ferroelectric materials are presently poorly understood.

Existence of vortex domain structures has been predicted in some low-dimensional ferroelectric nanostructures based on theoretical simulations10,11,12,21. In addition, some experimental studies have made great steps towards unambiguous observation of nanoscale polarization vortices14,18,25,26. While it is of fundamental and technological importance to characterize vortex domain structures in low-dimensional ferroelectrics, it is even more important to develop methods to control the vortex domain structures in low-dimensional ferroelectrics. Several theoretical investigations have focused on control of the vortex domain structures in low-dimensional ferroelectrics through external electromechanical fields29,30,31,32,33,34,35,36. Using the effective Hamiltonian method, Ponomareva et al.29 investigated effects of the electric boundary condition and epitaxial strain on the dipole pattern of Pb(Zr0.4Ti0.6)O3 nanodots and wires. Combining simulations and an analytical model, Prosandeev and Bellaiche30 studied the characteristics and signatures of dipole vortices in ferroelectric nanodots. Mechanisms of vortex switching, vortex rotation and vortex-to-polarization transformation induced by various kinds of electric fields in ferroelectric nanostructures were also investigated by effective Hamiltonian simulations31,32,33,34,35. Using phase-field simulation, vortex switching under curled electric field in ferroelectric nanostructures has also been investigated36. These works provide useful information on how to detect and control vortex domain structures in ferroelectric nanostructures.

The effect of mechanical strains and stresses on polarization and polarization-related properties in ferroelectrics and multiferroics is well known4,5,6,7,8,9,10. In contrast, investigations of the mechanical load effect on the vortex domain structures in low-dimensional ferroelectrics and multiferroics are scarce10,29,37. In general, it is expected that the size, number and orientation of dipole vortices should be affected by mechanical load. In order to exploit the coupling between the vortex domain structure and mechanical loads in applications, a number of questions must be answered, including how mechanical load affects the formation and stability of vortex domain structures, and whether mechanical-load-controlled transformations between different “vortex states” are possible.

In this work, in order to understand the effect of the mechanical load on stability and structure of the vortex domain in nanoscale ferroelectrics, we present a theoretical investigation of the vortex domain structure in three-dimensional ferroelectric nanoplatelets (FNPL) with careful consideration of the effects of inhomogeneous electromechanical fields, ambient temperature, surfaces, size, and other factors. Systematic phase-field simulations are conducted to explore effects of an external mechanical load on the evolution and equilibrium of vortex domain structures in nanoplatelets. We calculate temperature-stress (T-S) phase diagrams, and depict vortex domain structure as a function of mechanical load and ambient temperature. By considering different initial polarization distributions in nanoplatelets, transformations between different “vortex states” induced by mechanical load are demonstrated, and dynamic features during the transformations are also revealed.

Results

Vortex domain structure in free-standing FNPL

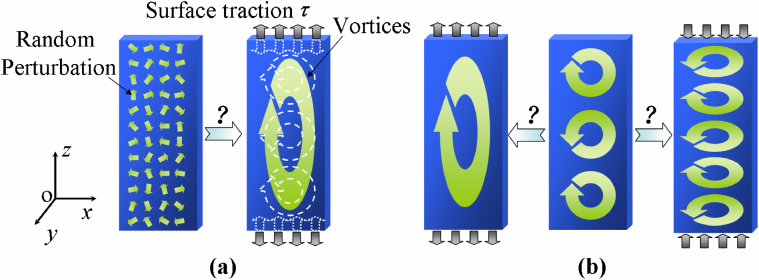
We consider prolate PbTiO3 nanoplatelet under open-circuit electric condition in our simulations. The initial polarization distribution of the nanoplatelet is either a random polarization perturbation or a vortex domain structure as shown in Fig. 1. By controlling the surface traction τ at the top and bottom surfaces, evolution of the spontaneous polarization P in FNPL under tensile or compressive mechanical load along the prolate direction (i.e., z-direction) can be simulated based on the phase-field method. The toroidal moment, i.e., 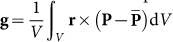Inline graphic, is adopted to characterize the vortex domain structure. Here V is the volume of the system, r is the position vector, and 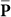Inline graphic is the mean spontaneous polarization of the system13. To confine the toroidal moment along y-direction, a three-dimensional 10Δx×3Δy×30Δz meshing at a scale of Δx = Δy = Δz = 1 nm is employed. Results obtained in our simulations are not only confined to this simple perovskite. Similar effects should be found in other ferroelectric materials such as BaTiO3, BaxSr1-xTiO3 and Pb(ZrxTi1−x)O3, etc., which are also predicted to adopt vortex domain structures in low-dimensional nanostructures.

Figure 1. Schematics of the simulated nanosystems.

Figure 1

Open in a new tab

A ferroelectric nanoplatelet under different surface traction along its prolate direction with (a) initial random polarization perturbation or (b) initial vortex domain structure.

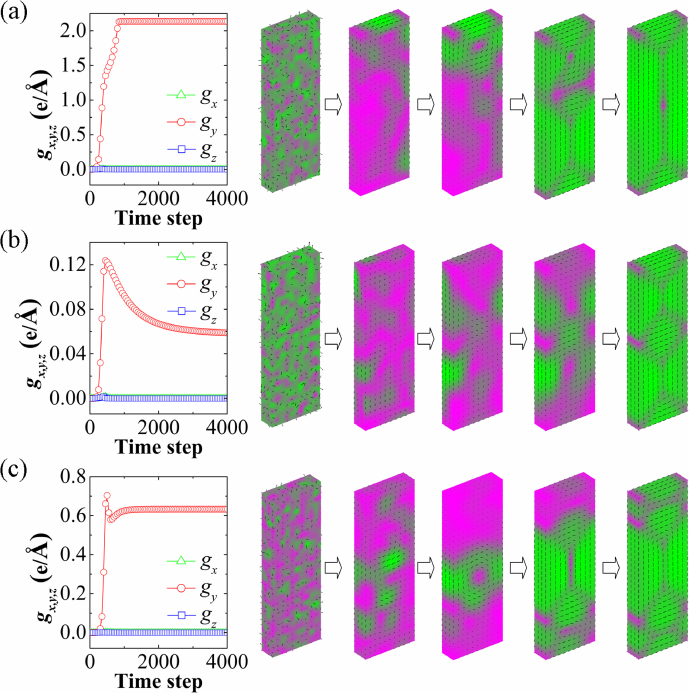
We start with an investigation on the vortex domain structure of a free-standing nanoplatelet. Simulations of polarization evolution at various temperatures from up to 102 sets of initial random perturbations are conducted to find the possible vortex domain structures. The random perturbations obey the normal distribution with zero mean polarization and standard deviation being 10–4Cm–2. Simulated results indicate that multiple “vortex states” (i.e., vortex domain structures with different size and number of vortices, or toroidal direction) are stable in the free-standing nanoplatelet, especially at low temperature. As shown in Fig. 2, “vortex states” with up to three dipole vortices are generally observed at T = 0K. It can be seen that the vortices lie in the x-z plane due to the strongest confinement in y-dimension, and manifest with near-null x- and z-components of the toroidal moment. Tracing the evolution of toroidal moment and domain morphologies as shown in Fig. 2, we classify three typical behaviors during the formation of vortex domain structure, i.e., nucleation, growth and adjustment. At the beginning, dipole vortices nucleate to decrease the high energy density caused by strong polarization inhomogeneity. After that, the vortices grow rapidly and generally lead to a sudden increase of toroidal moment. During the evolution, the vortices keep adjusting their sizes to balance each other, and some of them may be extruded out or merge into a bigger one (e.g., see Fig. 2a).

Figure 2. Evolution of the toroidal moment and domain morphology in a free-standing nanoplatelet.

Figure 2

Open in a new tab

Toroidal moment evolution and snapshots of domain evolution from different initial random perturbations towards equilibrium (a) 1-vortex, (b) 2-vortices and (c) 3-vortices states.

Mechanical-load-controlling vortex domain structure of FNPL

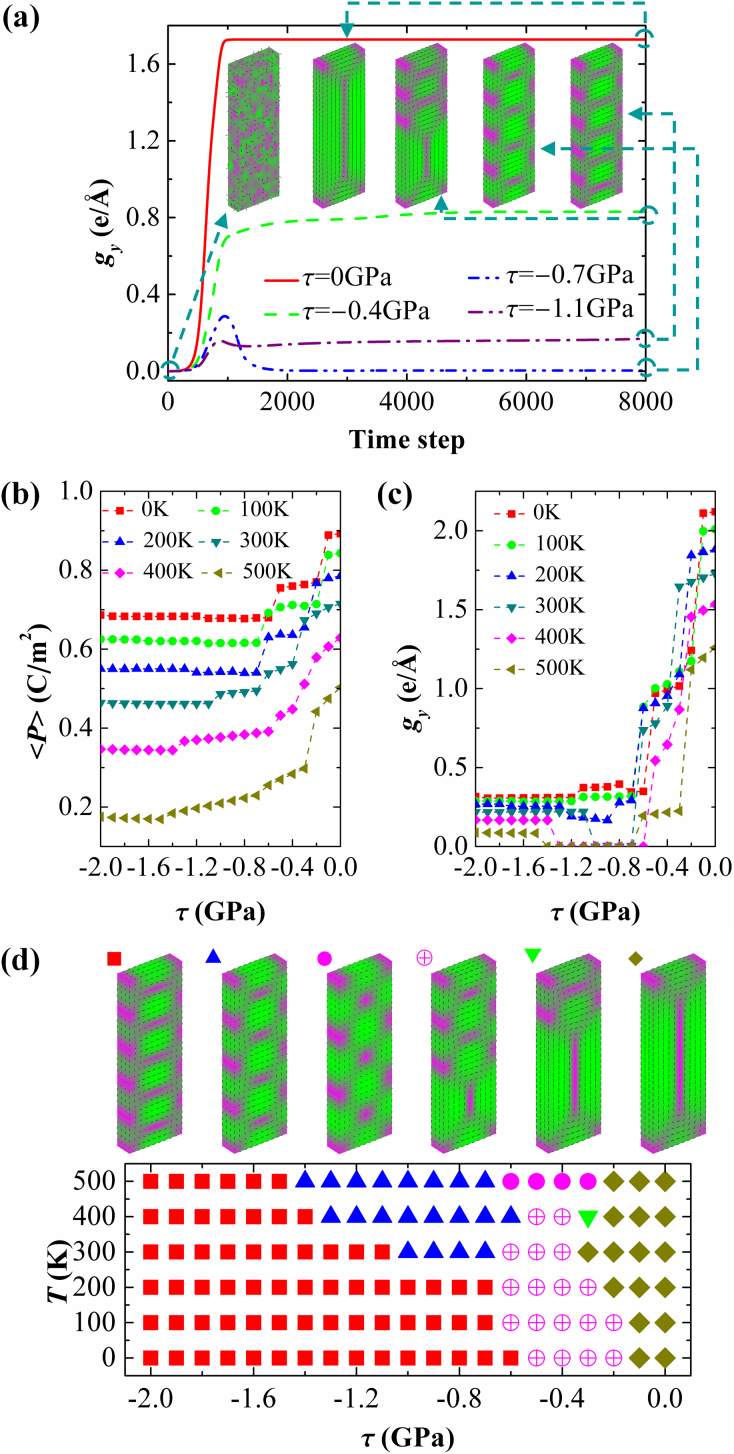
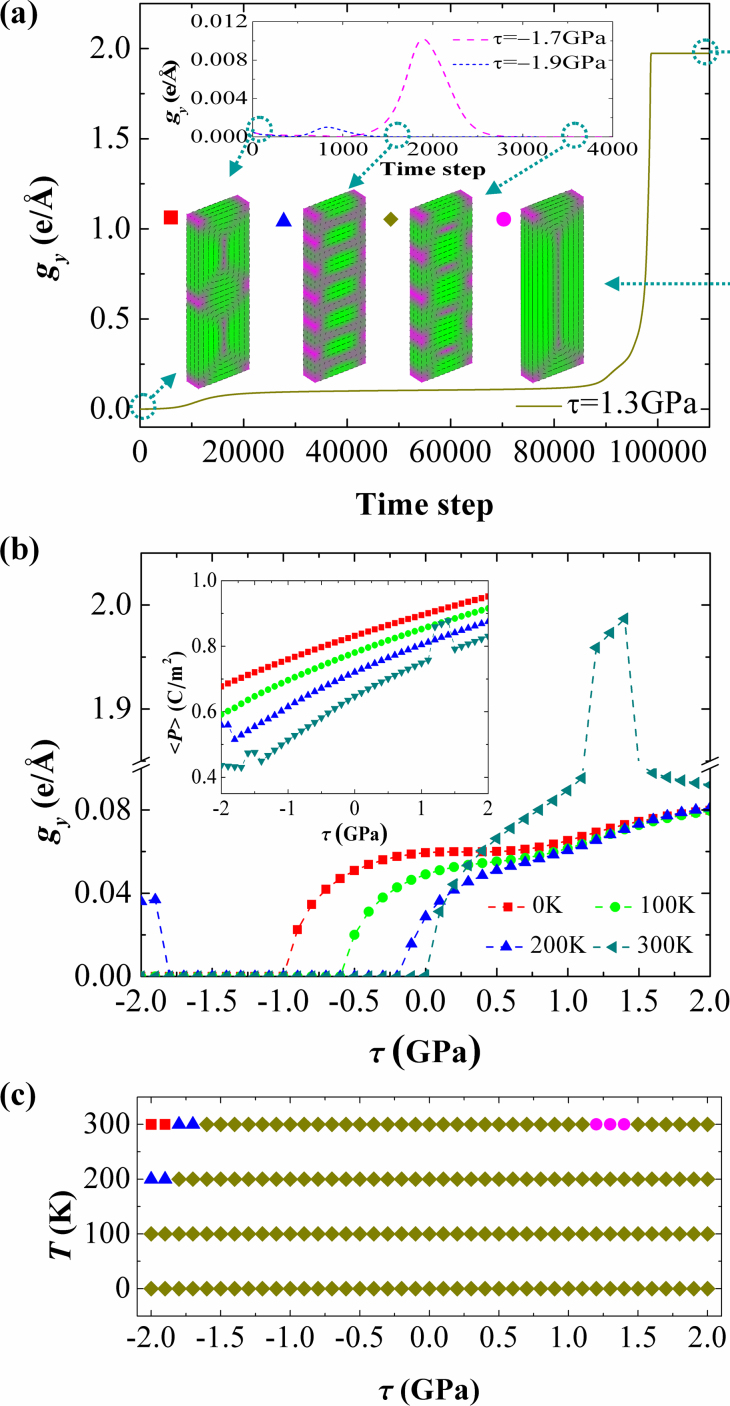
The multiplicity of stable vortex domain structures in low-dimensional ferroelectric nanostructures indicates an uncertainty of achieving specific “vortex state”, which also shows some potential applications, such as developing multiple-bits memory unit. The key that brings such multiplicity to application is an efficient control of vortex domain structure through external loads. Considering the interaction between polarization and mechanical stress field, regular controllability of the vortex domain structure by mechanical load is also possible. In order to summarize this behavior, we further simulate the vortex domain structure of a nanoplatelet under different surface traction with the initial random perturbation as shown in Fig. 2a. For a nanoplatelet under different surface traction τ, evolution of the y-component of toroidal moment gy is shown in Fig. 3a at room temperature (T = 300K). The initial and equilibrium domain morphologies are also depicted in the inserts. It can be seen that the equilibrium vortex domain structure is obviously dependent on the surface traction. As τ takes values from –2.0 GPa to 0 GPa by a step of 0.1 GPa, simulations show that the equilibrium vortex domain structures have five vortices at τ ∈ [−2.0 GPa, −1.1 GPa], four vortices at τ ∈ [−1.0 GPa, −0.7 GPa], three vortices at τ ∈ [−0.6 GPa, −0.4 GPa], and one vortex at τ ∈ [−0.3 GPa, 0 GPa], respectively. Meticulously tracing the domain morphologies evolutions, we can find that mechanical load significantly affects the nucleation and balance of vortices and thus results in various equilibrium vortex domain structures (see Supplementary Fig. S1 on line).

Figure 3. Mechanical load effect on a nanoplatelet with initial random perturbation.

Figure 3

Open in a new tab

A nanoplatelet is under various mechanical loads but with a given initial random perturbation as that shown in Fig. 2a. (a) The evolution of the toroidal moment component gy under different surface traction at T = 300 K. The inserts depict the initial and equilibrium domain morphologies. (b) and (c) depict the mean polarization magnitude and the toroidal moment component of equilibrium vortex domain structure as functions of surface traction under given temperatures. (d) Phase diagram of the equilibrium vortex domain structure as a function of surface traction and temperature.

As ambient temperature is able to affect the weights of free energy components and the stability of vortex domain structure (see Supplementary Fig. S2 on line), the mechanical load effect on the vortex domain structure in nanoplatelet is expected to be dependent on temperature. In Fig. 3b and 3c, we depict respectively the simulated mean magnitude of polarization over all sites, i.e., 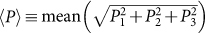Inline graphic, and the y-component of toroidal moment gy of equilibrium domain structure as functions of surface traction τ at different temperatures. For each given temperature, it can be seen in Fig. 3b and 3c that the mean magnitude of polarization and the toroidal moment have strong dependence on surface traction, where abrupt change at some values indicates different vortex domain structures. At the same time, effect of temperature is also particularly important to the mean magnitude of polarization and the toroidal moment. For a given vortex domain structure, it can be found that the increase of temperature tends to decrease the mean magnitude of polarization and toroidal moment. Besides, the abrupt positions are also changed along the axis of surface traction with variation of temperature as shown in Fig. 3b and 3c, which indicates that the existing ranges of specific vortex domain structures are significantly dependent on temperature.

More importantly, in order to clearly investigate the common influence of mechanical load and temperature on the vortex domain structure in FNPL, we depict the temperature-stress (T-S) phase diagram (as shown in Fig. 3d) of equilibrium vortex domain structure according to the results shown in Fig. 3b and 3c. As shown in Fig. 3d, we obtain six different equilibrium vortex domain structures in a nanoplatelet under the combining effects of mechanical load and temperature. In general, the nanoplatelet tends to adopt vortex domain structures with more/fewer vortices at more compressive/tensile surface traction. Note that the nanoplatelet with large compressive surface traction adopts vortex domain structures with four or five vortices. However, these domain structures are difficult to obtain from random perturbations in a free-standing nanoplatelet. After removing the surface traction, vortex domain structures with more vortices may remain stable or destabilize into those with fewer vortices, which is also strongly dependent on the temperature (see Supplementary Fig. S3a on line). Therefore, combining effects of temperature and mechanical load, we can find the rich vortex domain structures in a ferroelectric nanoplatelet.

Mechanical-load-controlling transformation of vortex domain structure in FNPL

While the above results demonstrate strong effect of mechanical load on formation of vortex domain structure, it is of particular significance to investigate the response of existing vortex domain structures to mechanical load, and to confirm that mechanical-load-controlling transformations between “vortex states” are possible. To the best knowledge of us, such kind of vortex transformation has not yet been predicted for three-dimensional ferroelectric nanostructures. In order to find the relationship between mechanical load and transformation of vortex domain structure, simulations of a nanoplatelet under different surface traction are conducted in following investigation.

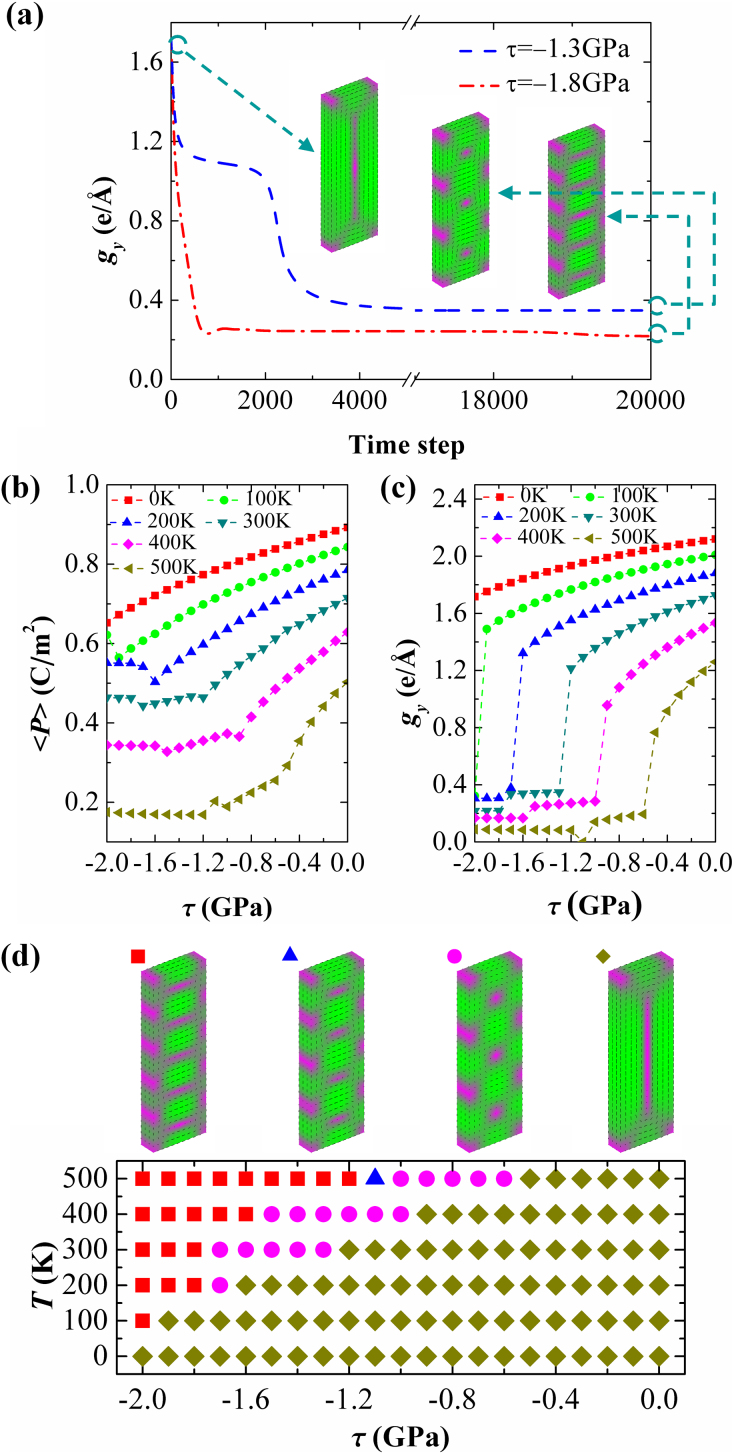
The simulated results of a nanoplatelet with initial 1-vortex state are shown in Fig. 4. According to the evolution of toroidal moment together with the inserted initial and equilibrium domain morphologies (Fig. 4a), it can be seen that the mechanical load indeed can induce transformations between “vortex states”. In this case, the initial 1-vortex state is compressed into 3-vortices state at –1.3 Gpa and into 5-vortices state at –1.8 Gpa at room temperature, manifested with a significant decrease of the equilibrium toroidal moment. As what we will discuss later in this section, the above transformations are accomplished by nucleation of new vortices at the core of initial vortices or at the surfaces of nanoplatelet.

Figure 4. Mechanical load effect on a nanoplatelet with initial 1-vortex state.

Figure 4

Open in a new tab

A nanoplatelet is under various mechanical loads but with an initial 1-vortex state obtained at free-standing condition. (a) The evolution of the toroidal moment component gy under different surface traction T = 300 K. The inserts depict the initial and equilibrium domain morphologies. (b) and (c) depict the mean polarization magnitude <P> and the toroidal moment component gy of equilibrium vortex domain structure as functions of surface traction under given temperatures. (d) Phase diagram of the equilibrium vortex domain structure as a function of surface traction and temperature.

To clearly study the effect of mechanical load on vortex domain structure in FNPL, the equilibrium mean magnitude of polarization and the toroidal moment are simulated as functions of surface traction at different temperatures (Fig. 4b and 4c). At T = 0K, the mean magnitude of polarization and the toroidal moment decrease smoothly with increasing magnitude of surface traction from 0 to 2.0 GPa. At higher temperature, abrupt changes of the two quantities as function of surface traction are observed, indicating that transformations of the initial 1-vortex state into other “vortex states”. Similar to the result of a nanoplatelet with initial random perturbation (see Fig. 3), the abrupt positions have strong dependences on the temperature. From the calculated T-S phase diagram as shown in Fig. 4d, it can be seen that these abrupt changes correspond to transformations of the initial 1-vortex state into “vortex states” with three, four and five vortices, respectively. Increasing temperature tends to decrease magnitude of the minimum transforming surface traction. For example, for transformation from 1-vortex state to 3-vortices state, the minimum compression surface traction changes from −1.7 GPa to −0.6 GPa with the increase of temperature from 100 K to 500 K. This indicates that ambient temperature can significantly affect the transforming barrier of the “vortex states”. Moreover, the domain structures with more vortices may remain stable or destabilize into other ones with fewer vortices, and the transformations are also dependent on temperature (see Supplementary Fig. S3b on line).

For a nanoplatelet with initial 2-vortices state (note, the vortices are in similar size as that shown in Fig. 2b), the effect of mechanical load is further investigated. Fig. 5a depicts the evolution of toroidal moment at room temperature, where the initial 2-vortices state is compressed into domain structures with four and six vortices at τ = −1.7 GPa and −1.9 GPa, respectively, and it can be also stretched into 1-vortex state when τ reaches 1.3 GPa. Combining effects of mechanical load and temperature, we also calculate the mean magnitude of polarization, the toroidal moment, and the corresponding T-S phase diagram. The results are shown in Fig. 5b and 5c. Note that the investigated temperature is no higher than 300 K, as the 2-vortices state for a FNPL with free-standing condition is unstable when temperature is higher than about 330 K. Compared with the case of initial 1-vortex state, transformations of 2-vortices state into “vortex states” with more vortices seem more difficult, since nucleation of new vortices at the core of smaller vortex or at the surfaces is more difficult. In the investigated range of surface traction, no transformation is observed at T = 0 K and T = 100 K. Nevertheless, transformation from 2-vortices state to 4-vortices state is observed at T = 200 K, and that from 2-vortices state to 6-vortices state is also found possible at T = 300 K. Furthermore, transformation of 2-vortices state to 1-vortex state can be induced by a tensile surface traction at room temperature, yet appears in a not very regular way as shown in Fig. 5c. Our simulations show that this transformation happens only with the surface traction τ between 1.3 GPa and 1.5 GPa. According to the vortex domain structure in Fig. 5c, we attribute this stable 2-vortices state to the similar size of the two vortices, which easily leads to a balance of the two vortices under range of our analyzed stress load. For the initial 2-vortices state with vortices in distinct size, or for the initial 3-vortices state, their transformations into 1-vortex state are much easier to be induced by tensile surface traction (see Supplementary Fig. S4 on line).

Figure 5. Mechanical load effect on a nanoplatelet with initial 2-vortices state.

Figure 5

Open in a new tab

A nanoplatelet is under various mechanical loads but with an initial 2-vortices state obtained at free-standing condition. (a) The evolution of the toroidal moment component gy under different surface traction T = 300 K. The inserts depict the initial and equilibrium domain morphologies. (b) and (c) depict the mean polarization magnitude and the toroidal moment component gy of equilibrium vortex domain structure as functions of surface traction under given temperatures. (d) Phase diagram of the equilibrium vortex domain structure as a function of surface traction and temperature.

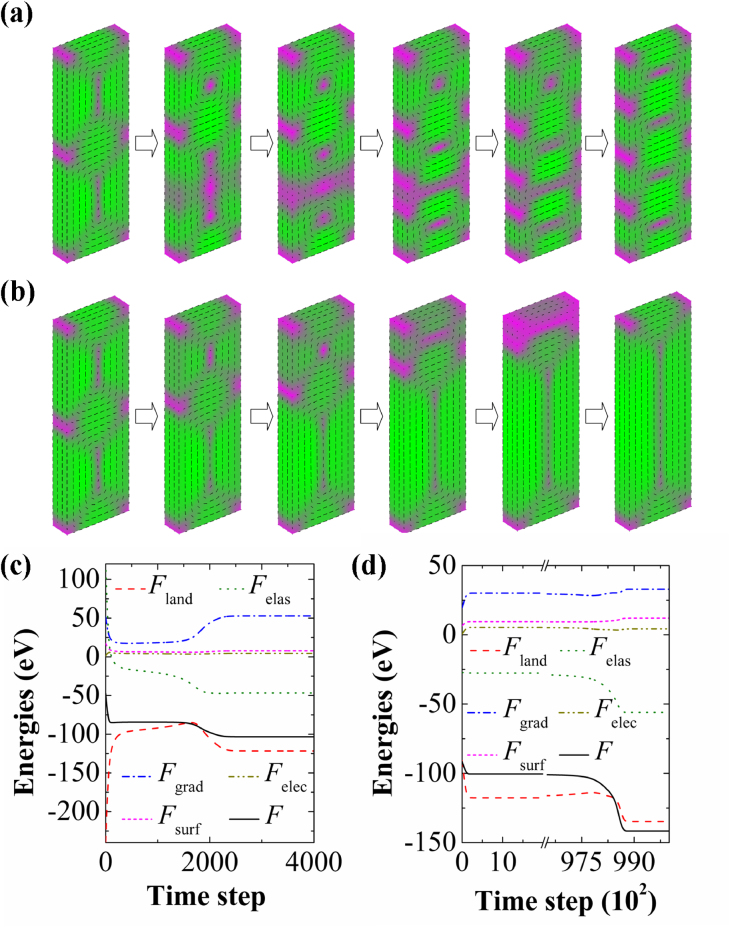
In view of the above results, we would like to investigate the evolutions of mechanical-load-controlling transformations between “vortex states”. At room temperature, Fig. 6a and 6b depict evolutions of a nanoplatelet with initial 2-vortices state transforming into 4-vortices state at τ = −1.7 GPa and 1-votex state at τ = 1.4 GPa, respectively. Results of other different kinds of transformations can be found in Supplementary Fig. S5 on line. For the 2-vortices to 4-vortices transformation as shown in Fig. 6a, it can be seen that new vortices nucleate at the core of the initial vortex. In general, through this way, the initial big vortex evolves into (2n+1) small vortices, with n being the integer. Transformations such as 2-vortices state to 6-vortices state, 1-vortex state to 3-vortices state and 1-vortex state to 5-vortices state are all through this mechanism. It should be noted that vortex nucleation at the surface is also possible (e.g., transformation from 1-vortex state to 4-vortices state as shown in Supplementary Fig. S5 on line). As depicted by Fig. 6b, under influence of tensile traction, the transformation from 2-vortices state to 1-vortex state is fulfilled by the squeezing out of one vortex. This squeezing out mechanism is more likely to happen when the initial vortices have different size. Otherwise, the vortices may fall into a balance that is difficult to break.

Figure 6. Evolution of the domain morphology and free energies during transformations of vortex states.

Figure 6

Open in a new tab

Snapshots of the domain evolution during (a) the transformation from 2-vortices state to 4-vortices state under compressive loading τ = −1.7 GPa and (b) the transformation from 2-vortices state to 1-vortex state under tensile loading τ = 1.3 GPa at T = 300 K. (c) and (d) depict the corresponding evolutions of the free energy and its components during the two transformations, respectively.

Tracing the evolution of the free energies as shown in Fig. 6c and 6d, it can be seen that the transformations are driven by decreasing of the total free energy of the system. The elastic energy, Landau energy and gradient energy contribute most of the total free energy, and they change significantly during transformations of the vortex domain structures. Meanwhile, the electric energy and surface energy remain small levels but also change a little bit. For the transformation from 2-vortices state to 4-vortices state shown in Fig. 6a, as compressive mechanical load acts on the nanoplatelet, the elastic energy contributed by the coupling of compressive stress and z-directed polarization is relatively large, and causes a depressing of z-polarization domains. This is accompanied by increase of the Landau energy and decrease of the gradient free energy. Vortex nucleation takes place as the decrease of elastic and gradient energy overwhelms the increase of Landau energy. At this point, local depolarization field should play an important role in vortex nucleation. After that, the Landau energy decreases and the gradient free energy increases significantly due to the growth of new vortices. For transformation from 2-vortices state to 1-vortex state, the evolutions of the free energies indicate different features. In this case (see Fig. 6d), the decrease of total free energy is more significant, which is mainly driven by the decrease of elastic energy and Landau energy. The squeezing out of one vortex induced by the tensile loading increases the magnitude of polarization quite an amount, and results in large decrease of elastic energy and Landau energy, but also an increase of gradient energy. Nevertheless, despite the large thermodynamic driving force, the transformation from 2-vortices state to 1-vortex state takes a much longer simulating time than that from 2-vortices state to 4-vortices state, which indicates a larger kinetic barrier.

Discussion

Our simulations demonstrate significant effects of mechanical load on the vortex domain structure and its transformation in ferroelectric nanoplatelet. Results show that the size and number of dipole vortices in the domain structure of ferroelectric nanoplatelet can be regularly controlled by adjusting the mechanical load, and yields rich temperature-stress (T-S) phase diagrams of vortex domain structure. Vortex domain structures with more/fewer vortices can be generally obtained by applying compressive/tensile mechanical load to the ferroelectric nanoplatelet. Consequently, the toroidal moment of the nanoplatelet and the polarization magnitude of the dipoles exhibit remarkable controllability. As an important finding, our calculations demonstrated that the mechanical-load-controlling transformations between different “vortex states” are possible, which are totally different from those conventionally controlled on the vortex domain by external electric fields. The regular controllability of vortex domain structure and control of its transformation by mechanical load are believed common in nanoscale ferroelectrics adopting vortex domain structures. At the same time, results in present work should be relevant for applications exploiting the vortex domain structure of nanoscale ferroelectrics.

Nevertheless, we only have considered nanoplatelets with a special size and shape under open-circuit condition in present work. According to configuration of the ferroelectric nanoplatelets, the direction of the toroidal moment is strongly fixed along the y-axis, thus mechanical load mostly affect the magnitude of toroidal moment by changing the size and number of vortices and the polarization magnitude of dipoles. Actually, for other ferroelectric nanostructures, such as nanodots and nanorods, not only the magnitude but also the orientation of toroidal moment should be strongly dependent on the mechanical load. Moreover, ambient temperature, boundary conditions and size of the system can strongly affect the relative weights of the components of free energy, thus the combination of these factors and mechanical load should bring more complicated vortex domain patters. These issues need further investigations in the future.

Methods

Phase field simulation on ferroelectric nanoplatelet

In the phase field simulation, the domain structure of a ferroelectric is described by the order parameter, i.e., the spontaneous polarization P = (P1, P2, P3). The electric displacement field is expressed in terms of electric field, linear induced polarization and nonlinear spontaneous polarization as D = ε0E + χbE + P = εbE + P, where E = −∇ϕ is the electric field, ϕ is the electric potential, χb is the background susceptibility tensor, ε0 is the vacuum permittivity, and εb = ε0 + χb is the dielectric constant tensor of background material38,39,40. Since the background material is in cubic paraelectric phase, the background dielectric constants along the three axis directions are the same, i.e., εb = ε11b = ε22b = ε33b.

The evolution of polarization field toward its equilibrium distribution is driven by the decrease of total free energy of the system, which is phenomenologically described by the time-dependent Ginzburg–Landau (TDGL) equations, i.e.,

graphic file with name srep00796-m1.jpg

where F is the total free energy, M the kinetic coefficient related to the domain wall mobility and t the time.

Based on the phenomenological theory, the total free energy of the system is expressed as a functional of order parameter field and applied fields. For the FNPL considered here, taking into account effects of mechanical stress, electric field, surface and spatial polarization variation, the total Gibbs free energy could be written as sum of the Landau free energy FLand, elastic energy Felas, gradient energy Fgrad, electrostatic energy Felec and surface energy Fsurf, that is,

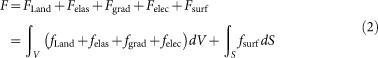graphic file with name srep00796-m2.jpg

where fLand, felas fgrad, felec and fsurf are the corresponding free energy densities, V and S the volume and surface of the FNPL.

For perovskite ferroelectrics, the Landau free energy density fLand can be generally expressed up to eight-order polynomial expansion for a zero stress as41,42,43,44,

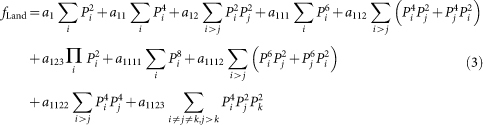graphic file with name srep00796-m3.jpg

where ai, aij, aijk and aijkl are dielectric stiffness and higher order coefficients fitted to bulk properties.

Under the condition of applied stress, the mechanical stress field (applied and internal) and its coupling with polarization contribute to the elastic energy density, which is described by,

graphic file with name srep00796-m4.jpg

where sijkl and Qijkl are the fourth-rank elastic compliance and electrostrictive coefficients, respectively. σij are stress field components caused by the incompatibility of eigenstrains and external mechanical load. In the absence of body forces, σij are determined by the mechanical equilibrium equations σij,j|V = 0 and corresponding mechanical boundary condition τk|S = niσik|S, where the comma in the subscript denotes spatial differentiation, τk are the surface tractions and ni the ith component of the unit vector normal to the surface.

The spatial polarization variation contributes a gradient energy to the total free energy. To the lowest order of Taylor expansion, the gradient energy density takes the form as 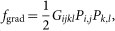Inline graphic, with Gijkl being the fourth-rank gradient energy coefficients. Due to truncation at the surface of nanoplatelet, the spontaneous polarization is inhomogeneous across the out-of-plane direction. Thus an additional surface energy is necessary to describe this intrinsic effect. Using the so-called extrapolation length45, the surface energy density of the ferroelectric nanoplatelet can be approximately given by 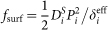Inline graphic, where 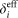Inline graphic are extrapolation length and 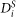Inline graphic the material coefficients related to the gradient energy coefficients and the surface orientation. According to the previous works38,39,46, the electric energy density of a given polarization distribution is written as 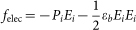Inline graphic. In the absence of external electric field, the total electric field is equal to depolarization field induced by spatial polarization variation and incomplete screening of the polarization charges at truncated surfaces. Under the open-circuit condition, for a free-charge-absent body the depolarization field can be calculated by the electrostatic equilibrium equation Di,i|V = 0 and the corresponding boundary condition Dini|S = 0.

Ferroelectric phase transition involves structural changes and results spontaneous strains. At stress-free state, these strains are called eigenstrains, which are related to the polarization as 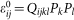Inline graphic. Considering the eigenstrains, the stress fields are given by

graphic file with name srep00796-m5.jpg

where ekl are the elastic strains and εkl the total strains, which must be compatible and are related to the displacement as 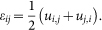Inline graphic.

Substitute Eq. (5) into the mechanical equilibrium equations and its boundary condition, we have

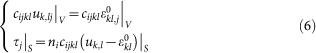graphic file with name srep00796-m6.jpg

Similarly, we have the electrostatic equilibrium equation and its boundary condition in term of the potential as

graphic file with name srep00796-m7.jpg

Phase-field simulations are conducted by numerically solving the TDGL Eq. (1) together with the mechanical and electrostatic equilibrium Eqs. (6) and (7). The TDGL equations are solved using the finite difference method, whereas the mechanical and electrostatic equilibrium equations are solved by the finite element method. The time step is chosen to be Δt = 0.01a0M, with a0 = |a1|T = 300K. Values of the expansion coefficients of the Landau-potential, electrostrictive coefficients, elastic properties in calculations are listed Table 1. For PbTiO3, a commonly used six-order Landau-potential is adopted in this study.

Table 1. Values of parameter used in the phase-field simulations (SI units and T in K).

Parameter Value Unit
a1 3.85(T−752)×105 C−2m2N
a11 −7.3×107 C−4m6N
a12 7.5×108 C−4m6N
a111 2.6×108 C−6m10N
a112 6.1×108 C−6m10N
a123 −3.7×109 C−6m10N
s11 8.0×10−12 m2N−1
s12 −2.5×10−12 m2N−1
s44 9.0×10−12 m2N−1
Q11 0.089 C−2m4
Q12 −0.026 C−2m4
Q44 0.0675 C−2m4
G11 3.46×10−10 m4NC−2
G12 0 m4NC−2
G44 1.73×10−10 m4NC−2
G′44 1.73×10−10 m4NC−2
DSi 3.46×10−10 m4NC−2
δieff 5×10−9 m
εb 4.425×10−10 Fm−1
Open in a new tab

Values of the expansion coefficients of the Landau potential, elastic compliance and electrostrictive coefficients are from ref. 42, the isotropic gradient coefficients are from ref. 47, the extrapolation length is from ref. 48, and the background dielectric constant is from ref.38 and 39.

Finite element method to solve mechanical and electrostatic model

In our phase field simulation, the inhomogeneous mechanical stress and electric fields are solved using the finite element method. According to the variation relationship, the mechanical and electrostatic equilibrium equations, i.e., Eqs. (6) and (7), can be solved by finding the extreme value of following functionals, i.e.,

graphic file with name srep00796-m9.jpg
graphic file with name srep00796-m8.jpg

where the freedoms of the two functionals are the displacement ui and the electric potential ϕ, respectively.

In the following, it is convenient to rewrite Eqs. (8) into matrix form, i.e.,

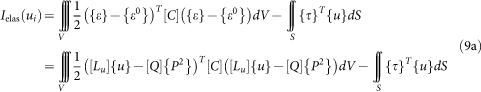graphic file with name srep00796-m11.jpg
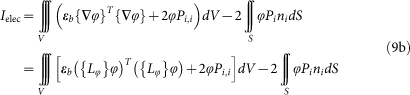graphic file with name srep00796-m10.jpg

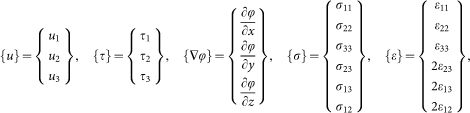
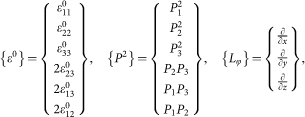
with some vectors and matrices defined as follows,

graphic file with name srep00796-m38.jpg
graphic file with name srep00796-m39.jpg
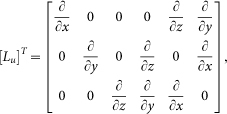graphic file with name srep00796-m40.jpg
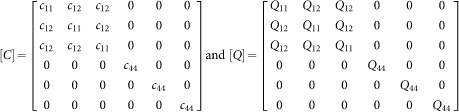graphic file with name srep00796-m12.jpg

In the finite element method of this study, the simulated ferroelectric is meshed into eight-node cubic elements. The displacement vector and the electric potential at the ith nodes of an element are denoted as 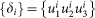Inline graphic and ϕi, with i = 1, 2, 3, …, 8. The displacement vector and the electric potential of the eight nodes in each element is then expressed by column vectors as

graphic file with name srep00796-m14.jpg
graphic file with name srep00796-m13.jpg

The displacement and the electric potential in the element are expressed in terms of those of the nodes, i.e.,

graphic file with name srep00796-m15.jpg

in which

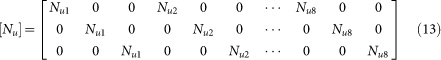graphic file with name srep00796-m16.jpg

and

graphic file with name srep00796-m17.jpg

where Nui and Nϕi are the interpolation functions.

Substitute Eqs. (12) into Eqs. (9), we have,

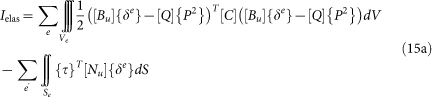graphic file with name srep00796-m19.jpg
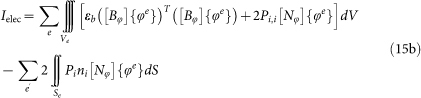graphic file with name srep00796-m18.jpg

where e and e' label the elements in the volume and those at the surface, respectively, matrices [Bu]≡[Lu][Nu] and [Bϕ]≡[Lϕ][Nϕ].

Variation of the two functionals in Eqs. (15) with respect to the displacement {δe} and the electric potential {ϕe} at all nodes should be zero, which results

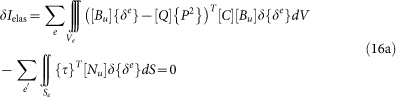graphic file with name srep00796-m21.jpg
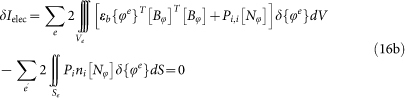graphic file with name srep00796-m20.jpg

Based on the variation principle, the following element equations can be obtained from Eqs. (16), i.e.,

graphic file with name srep00796-m22.jpg

where

graphic file with name srep00796-m26.jpg
graphic file with name srep00796-m25.jpg
graphic file with name srep00796-m24.jpg
graphic file with name srep00796-m23.jpg

are the stiffness matrices and node force vectors of element e. Assembling the stiffness matrices, displacement and electric potential vector, and the force vectors of all the elements together yields the global equations:

graphic file with name srep00796-m27.jpg

where [Ku] and [Kϕ] are global stiffness matrices, {Fu} and {Fϕ} are global force vectors, and {U} and {Φ} are the vectors containing all the node displacement and electric potential, respectively. The node displacement and electric potential can be conveniently determined by solving Eqs. (19) using the Gauss-Seidel iteration method.

Author Contributions

Y. Z. initiated and performed this work and manuscript. W. J. C. performed the simulations. B. W. suggested the principle idea. Y. Z. and W. J. C. analyzed the numerical results. W. J. C., Y. Z. and B. W. wrote the manuscript. All authors contributed to discussion and reviewed the manuscript.

Supplementary Material

Supplementary Information

Supplementary Information

srep00796-s1.pdf (5.2MB, pdf)

Acknowledgments

Helpful discussions with Dr. D. C. Ma and Dr. S. P. Lin are gratefully acknowledged. The authors also acknowledge the financial support of the National Natural Science Foundation of China (NSFC) (Nos. 10902128, 11232015, 50802026, 10972239). Y. Z. also thanks support by the Fundamental Research Funds for the Central Universities to Micro&Nano Physics and Mechanics Research Laboratory, and New Century Excellent Talents in University, Research Fund for the Doctoral Program of Higher Education, and Fok Ying Tung Foundation.

References

  1. Scott J. F. Nanoferroelectrics: statics and dynamics. J. Phys.: Condens. Matter 18, R361 (2006). [DOI] [PubMed] [Google Scholar]
  2. Rørvik P. M., Grande T. & Einarsrud M.-A. One-dimensional nanostructures of ferroelectric perovskites. Adv. Mater. 23, 4007–4034 (2011). [DOI] [PubMed] [Google Scholar]
  3. Zhuravlev M. Y., Sabirianov R. F., Jaswal S. S. & Tsymbal E. Y. Giant electroresistance in ferroelectric tunnel junctions. Phys. Rev. Lett. 94, 246802 (2005). [Google Scholar]
  4. Zheng Y., Zheng Y., Woo C. H. & Wang B. Pulse-loaded ferroelectric nanowire as an alternating current source. Nano Lett. 8, 3131–3136 (2008). [DOI] [PubMed] [Google Scholar]
  5. Xu S., Hansen B. J. & Wang Z. L. Piezoelectric-nanowire-enabled power source for driving wireless microelectronics. Nat. Commun. 1, 93 (2010). [DOI] [PubMed] [Google Scholar]
  6. Yang S. Y. et al. Above-bandgap voltages from ferroelectric photovoltaic devices. Nat. Nanotech. 5, 143–147 (2010). [DOI] [PubMed] [Google Scholar]
  7. Zheng Y. & Woo C. H. Giant piezoelectric resistance in ferroelectric tunnel junctions. Nanotechnology 20, 075401 (2009). [DOI] [PubMed] [Google Scholar]
  8. Luo X., Wang B. & Zheng Y. Tunable tunneling electroresistance in ferroelectric tunnel junctions by mechanical loads. ACS Nano 5, 1649–1656 (2011). [DOI] [PubMed] [Google Scholar]
  9. Cao S. et al. Extreme chemical sensitivity of nonlinear conductivity in charge-ordered LuFe2O4. Scientific Report 2, 330 (2012). [DOI] [PMC free article] [PubMed] [Google Scholar]
  10. Fu H. & Bellaiche L. Ferroelectricity in barium titanate quantum dots and wires. Phys. Rev. Lett. 91, 257601 (2003). [DOI] [PubMed] [Google Scholar]
  11. Naumov I. I., Bellaiche L. & Fu H. Unusual phase transitions in ferroelectric nanodisks and nanorods. Nature 432, 737–740 (2004). [DOI] [PubMed] [Google Scholar]
  12. Naumov I. & Bratkovsky A. M. Unusual polarization patterns in flat epitaxial ferroelectric nanoparticles. Phys. Rev. Lett. 101, 107601 (2008). [DOI] [PubMed] [Google Scholar]
  13. Prosandeev S., Ponomareva I., Naumov I., Kornev I. & Bellaiche L. Original properties of dipole vortices in zero-dimensional ferroelectrics. J. Phys.: Condens. Matter 20, 193201 (2008). [Google Scholar]
  14. Gruverman A. et al. Vortex ferroelectric domains. J. Phys.: Condens. Matter 20, 342201 (2008). [Google Scholar]
  15. Prosandeev S. & Bellaiche L. Hypertoroidal moment in complex dipolar structures. J. Mater. Sci. 44, 5235–5248 (2009). [Google Scholar]
  16. Hong J. et al. Topology of the polarization field in ferroelectric nanowires from first principles. Phys. Rev. B 81, 172101 (2010). [Google Scholar]
  17. Schilling A. et al. Domains in ferroelectric nanodots. Nano Lett. 9, 3359-3364 (2009). [DOI] [PubMed] [Google Scholar]
  18. Rodriguez B. J. et al. Vortex polarization states in nanoscale ferroelectric arrays. Nano Lett. 9, 1127–1131 (2009). [DOI] [PubMed] [Google Scholar]
  19. Ivry Y., Chu D. P., Scott J. F. & Durkan C. Flux closure vortexlike domain structures in ferroelectric thin films. Phys. Rev. Lett. 104, 207602 (2010). [DOI] [PubMed] [Google Scholar]
  20. McGilly L. J., Schilling A. & Gregg J. M. Domain bundle boundaries in single crystal BaTiO3 lamellae: searching for naturally forming dipole flux-closure/ quadrupole chains. Nano Lett. 10, 4200–4205 (2010). [DOI] [PubMed] [Google Scholar]
  21. Stachiotti M. G. & Sepliarsky M. Toroidal ferroelectricity in PbTiO3 nanoparticles. Phys. Rev. Lett. 106, 137601 (2011). [DOI] [PubMed] [Google Scholar]
  22. Nelson C. T. et al. Spontaneous vortex nanodomain arrays at ferroelectric heterointerfaces. Nano Lett. 11, 828–834 (2011). [DOI] [PubMed] [Google Scholar]
  23. McGilly L. J. & Gregg J. M. Polarization closure in PbZr(0.42)Ti(0.58)O3 nanodots. Nano Lett. 11, 4490–4495 (2011). [DOI] [PubMed] [Google Scholar]
  24. McQuaid R. G. P. et al. Mesoscale flux-closure domain formation in single-crystal BaTiO3. Nat. Commun. 2, 404 (2011). [DOI] [PMC free article] [PubMed] [Google Scholar]
  25. Balke N. et al. Enhanced electric conductivity at ferroelectric vortex cores in BiFeO3. Nature Phys. 8, 81–88 (2011). [Google Scholar]
  26. Jia C. L., Urban K. W., Alexe M., Hesse D. & Vrejoiu I. Direct observation of continuous electric dipole rotation in flux-closure domains in ferroelectric Pb(Zr,Ti)O3. Science 331, 1420–1423 (2011). [DOI] [PubMed] [Google Scholar]
  27. Schilling A. et al. Shape-induced phase transition of domain patterns in ferroelectric platelets. Phys. Rev. B 84, 064110 (2011). [Google Scholar]
  28. Catalan G., Seidel J., Ramesh R. & Scott J. F. Domain wall nanoelectronics. Rev. Mod. Phys. 84, 119–156 (2012). [Google Scholar]
  29. Ponomareva I., Naumov I. & Bellaiche L. Low-dimensional ferroelectrics under different electrical and mechanical boundary conditions: Atomistic simulations. Phys. Rev. B 72, 214118 (2005). [Google Scholar]
  30. Prosandeev S. & Bellaiche L. Characteristics and signatures of dipole vortices in ferroelectric nanodots: first-principles-based simulations and analytical expressions. Phys. Rev. B 75, 094102 (2007). [Google Scholar]
  31. Prosandeev S., Ponomareva I., Kornev I., Naumov I. & Bellaiche L. Phys. Rev. Lett. Controlling toroidal moment by means of an inhomogeneous static field: an ab initio study. 96, 237601 (2006). [DOI] [PubMed] [Google Scholar]
  32. Naumov I. & Fu H. Vortex-to-polarization phase transformation path in ferroelectric Pb(ZrTi)O3 nanoparticles. Phys. Rev. Lett. 98, 077603 (2007). [DOI] [PubMed] [Google Scholar]
  33. Prosandeev S., Ponomareva I., Kornev I. & Bellaiche L. Control of vortices by homogeneous fields in asymmetric ferroelectric and ferromagnetic rings. Phys. Rev. Lett. 100, 047201 (2008). [DOI] [PubMed] [Google Scholar]
  34. Prosandeev S. & Bellaiche L. Controlling double vortex states in low-dimensional dipolar systems. Phys. Rev. Lett. 101, 097203 (2008). [DOI] [PubMed] [Google Scholar]
  35. Naumov I. & Fu H. Cooperative response of Pb(ZrTi)O3 nanoparticles to curled electric fields. Phys. Rev. Lett. 101, 197601 (2008). [DOI] [PubMed] [Google Scholar]
  36. Wang J. Switching mechanism of polarization vortex in single-crystal ferroelectric nanodots. Appl. Phys. Lett. 97, 192901 (2010). [Google Scholar]
  37. Chen W. J., Zheng Y. & Wang B. Phase field simulations of stress controlling the vortex domain structures in ferroelectric nanosheets. Appl. Phys. Lett. 100, 062901 (2012). [Google Scholar]
  38. Zheng Y. & Woo C. H. Thermodynamic modeling of critical properties of ferroelectric superlattices in nano-scale. Appl. Phys. A: Mater. Sci. Process. 97, 617–626 (2009). [Google Scholar]
  39. Woo C. H. & Zheng Y. Depolarization in modeling nano-scale ferroelectrics using the Landau free energy functional. Appl. Phys. A: Mater. Sci. Process. 91, 59–63 (2008). [Google Scholar]
  40. Tagantsev A. K. Landau Expansion for Ferroelectrics: Which Variable to Use? Ferroelectrics 375, 19–27 (2008). [Google Scholar]
  41. Haun M. J., Furman E., Jang S. J., McKinstry H. A. & Cross L. E. Thermodynamic theory of PbTiO3. J. Appl. Phys. 62, 3331 (1987). [Google Scholar]
  42. Pertsev N. A., Zembilgotov A. G. & Tagantsev A. K. Effect of Mechanical Boundary Conditions on Phase Diagrams of Epitaxial Ferroelectric Thin Films. Phys. Rev. Lett. 80, 1988 (1998). [Google Scholar]
  43. Li Y. L., Cross L. E. & Chen L. Q. A phenomenological thermodynamic potential for BaTiO3 single crystals. J. Appl. Phys. 98, 064101 (2005). [Google Scholar]
  44. Wang Y. L. et al. Anharmonicity of BaTiO3 single crystals. Phys. Rev. B 73, 132103 (2006). [Google Scholar]
  45. Kretschmer R. & Binder K. Surface effects on phase transitions in ferroelectrics and dipolar magnets. Phys. Rev. B 20, 1065 (1979). [Google Scholar]
  46. Landau L. D., Lifshitz E. M. & Pitaevskii L. P. Electrodynamics of continuous media, Oxford University Press: New York, (1984). [Google Scholar]
  47. Li Y. L. et al. Effect of electrical boundary conditions on ferroelectric domain structures in thin films. Appl. Phys. Lett. 81, 427 (2002). [Google Scholar]
  48. Ishikawa K. & Uemori T. Surface relaxation in ferroelectric perovskites. Phys. Rev. B 60, 11841 (1999). [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information

Supplementary Information

srep00796-s1.pdf (5.2MB, pdf)

Articles from Scientific Reports are provided here courtesy of Nature Publishing Group

RESOURCES