Abstract
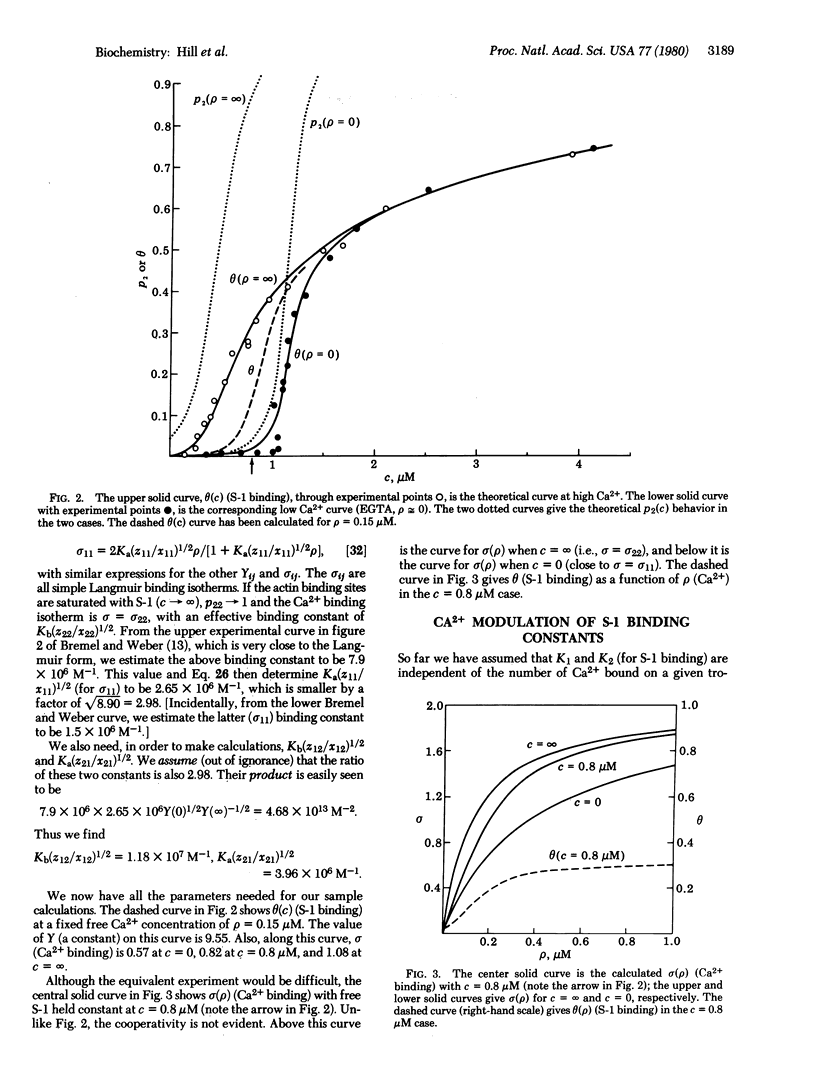
Recent experimental data on the equilibrium binding of myosin subfragment 1 (S-1) to regulated actin filaments in the presence and in the absence of Ca(2+) are analyzed by using a linear Ising model. In the model, each tropomyosin-troponin unit (including seven sites on the actin filament) can be in one of two possible states, which have different intrinsic free energies and different binding constants for S-1. Bound S-1 molecules do not interact with each other. There are nearest-neighbor (pair) interactions between these units that depend on the state of each member of the pair and on the number of Ca(2+) bound to one member of the pair. There are two sources of positive cooperativity in this system: the fact that seven actin sites change state together as part of a single unit; and the existence of attractive nearest-neighbor interactions between units. Parameters in the model are evaluated by fitting the data, both in the presence and in the absence of Ca(2+). Several extensions of this model are discussed.
Full text
PDF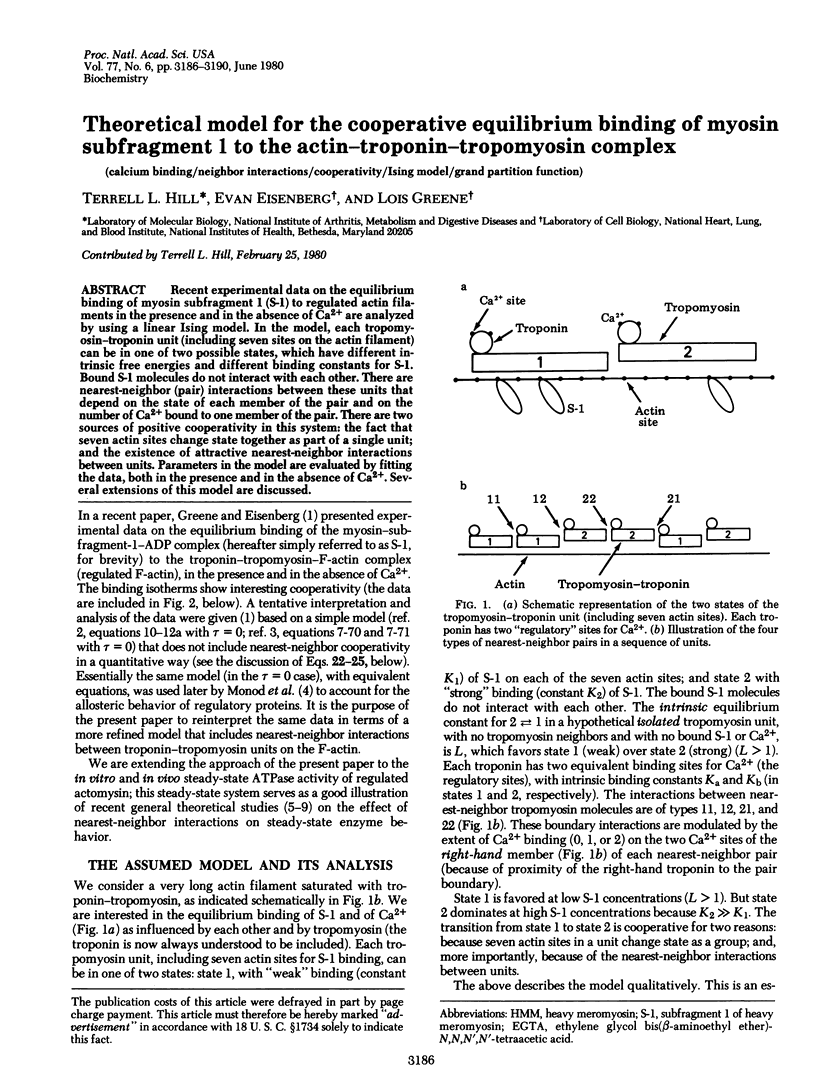



Selected References
These references are in PubMed. This may not be the complete list of references from this article.
- Greene L. E., Eisenberg E. Cooperative binding of myosin subfragment-1 to the actin-troponin-tropomyosin complex. Proc Natl Acad Sci U S A. 1980 May;77(5):2616–2620. doi: 10.1073/pnas.77.5.2616. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hill T. L. Binding of monovalent and divalent myosin fragments onto sites on actin. Nature. 1978 Aug 24;274(5673):825–826. doi: 10.1038/274825a0. [DOI] [PubMed] [Google Scholar]
- Hill T. L. Further study of the effect of enzyme-enzyme interactions on steady-state enzyme kinetics. Proc Natl Acad Sci U S A. 1977 Oct;74(10):4111–4115. doi: 10.1073/pnas.74.10.4111. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hill T. L. Steady-state phase or cooperative transitions between biochemical cycles. Proc Natl Acad Sci U S A. 1979 Feb;76(2):714–716. doi: 10.1073/pnas.76.2.714. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hill T. L., Stein L. Properties of some three-state, steady-state Ising systems, according to the Bragg-Williams approximation. Proc Natl Acad Sci U S A. 1980 Feb;77(2):693–697. doi: 10.1073/pnas.77.2.693. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hill T. L. Theoretical study of the effect of enzyme-enzyme interactions on steady-state enzyme kinetics. Proc Natl Acad Sci U S A. 1977 Sep;74(9):3632–3636. doi: 10.1073/pnas.74.9.3632. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hill T. L. Unsymmetrical and concerted examples of the effect of enzyme--enzyme interactions on steady-state enzyme kinetics. Proc Natl Acad Sci U S A. 1978 Mar;75(3):1101–1105. doi: 10.1073/pnas.75.3.1101. [DOI] [PMC free article] [PubMed] [Google Scholar]
- MONOD J., WYMAN J., CHANGEUX J. P. ON THE NATURE OF ALLOSTERIC TRANSITIONS: A PLAUSIBLE MODEL. J Mol Biol. 1965 May;12:88–118. doi: 10.1016/s0022-2836(65)80285-6. [DOI] [PubMed] [Google Scholar]


