Abstract
The temperature-size rule (TSR) is an intraspecific phenomenon describing the phenotypic plastic response of an organism size to the temperature: individuals reared at cooler temperatures mature to be larger adults than those reared at warmer temperatures. The TSR is ubiquitous, affecting >80% species including uni- and multicellular groups. How the TSR is established has received attention in multicellular organisms, but not in unicells. Further, conceptual models suggest the mechanism of size change to be different in these two groups. Here, we test these theories using the protist Cyclidium glaucoma. We measure cell sizes, along with population growth during temperature acclimation, to determine how and when the temperature-size changes are achieved. We show that mother and daughter sizes become temporarily decoupled from the ratio 2:1 during acclimation, but these return to their coupled state (where daughter cells are half the size of the mother cell) once acclimated. Thermal acclimation is rapid, being completed within approximately a single generation. Further, we examine the impact of increased temperatures on carrying capacity and total biomass, to investigate potential adaptive strategies of size change. We demonstrate no temperature effect on carrying capacity, but maximum supported biomass to decrease with increasing temperature.
Keywords: Cyclidium glaucoma, development, growth, temperature-size rule
Introduction
The temperature-size rule (TSR) is a ubiquitous intraspecific phenomenon affecting most (>80%) ectotherms: individuals reared at cooler temperatures mature at a larger size than those reared at warmer temperatures (Atkinson, 1994). The rule is common both in multicellular (Atkinson 1994; Forster et al., 2011a, 2011b) and in unicellular organisms, having been found in bacteria and many protists (Montagnes and Franklin, 2001; Atkinson et al., 2003). Recently, reduced body sizes at both the species and community level have been identified as a universal ecological response to global warming (Daufresne et al., 2009; Gardner et al., 2011; Sheridan and Bickford, 2011). Therefore, we need to understand how the TSR is brought about, and how it impacts on the ecology of ectothermic species.
Despite the ubiquity of the TSR, the effect of temperature on organism body size remains poorly understood. There has recently been a significant amount of work on how the TSR is established in multicellular organisms, with studies investigating how and when size changes occur during the life cycle (Karan et al., 1998; Petersen et al., 2000; Forster and Hirst, 2011; Potter et al., 2011). Further, differences in the thermal sensitivities of growth and development rates during ontogeny have been shown to drive and maintain the TSR in multicellular organisms (Forster et al., 2011a, 2011b). Currently, such empirical study of how the TSR is established in unicellular organisms does not exist.
A simple conceptual model has demonstrated that the mechanism underpinning the TSR must be different in unicellular and multicellular organisms (Forster et al., 2011a). This difference is highlighted by the equation that links size and rates:
 |
where g is the mass-specific growth rate of the individual (day−1), D is the development rate (day−1, that is, 1/doubling time), MA is the mass of the adult and MP is the mass of a single progeny. We use the term ‘adult' here to refer to the mass of a mother cell at the point of division in unicells. Similarly, ‘progeny' (referring to eggs in multicellular organisms) refers to a single daughter cell just after division of the mother cell. We use the exponential form of the model in Equation 1 (Forster et al., 2011a), as this most accurately represents individual growth of unicells (Krasnow, 1978; Olson et al., 1986). In multicellular organisms, changes in size have been shown to differ in adults and progeny. Size changes in acclimated adults are ∼2.5% °C−1 but <1% °C−1 in progeny. This cannot be the case in unicells: dividing in half requires the TSR to equally impact mother and daughter size in unicells at acclimation. This in turn means the rates driving the TSR, growth and development, can only become temporarily decoupled during acclimation in unicells (Figure 1). This temporary decoupling suggests a fundamentally different mechanism of the TSR in unicellular compared with multicellular organisms, where rates remain decoupled (Forster et al., 2011a), despite both groups obeying the TSR. Currently, this disparity between uni- and multicellular organisms remains theoretical: we still require testing of changes in mother and daughter size during the acclimation phase in unicellular organisms. Studies of unicellular organisms typically allow species to acclimate to new temperatures before carrying out size measurements (for example, five generations; Montagnes and Franklin, 2001). However, we need to understand when, and for how long, mother and daughter sizes become decoupled. Such research will show whether fundamental life-history rates relevant to all living organisms, growth and development (Equation 1), respond differently to temperature in different groups. We carry out this research here by measuring cell size changes in the ciliated protozoan Cyclidium glaucoma during thermal acclimation. Further, including parameters for temperature, time and population abundance within a general linear model (GLM), we can ascertain and account for the impact of population abundance on cell size during the acclimation and thus singularly determine the importance of temperature in determining cell size.
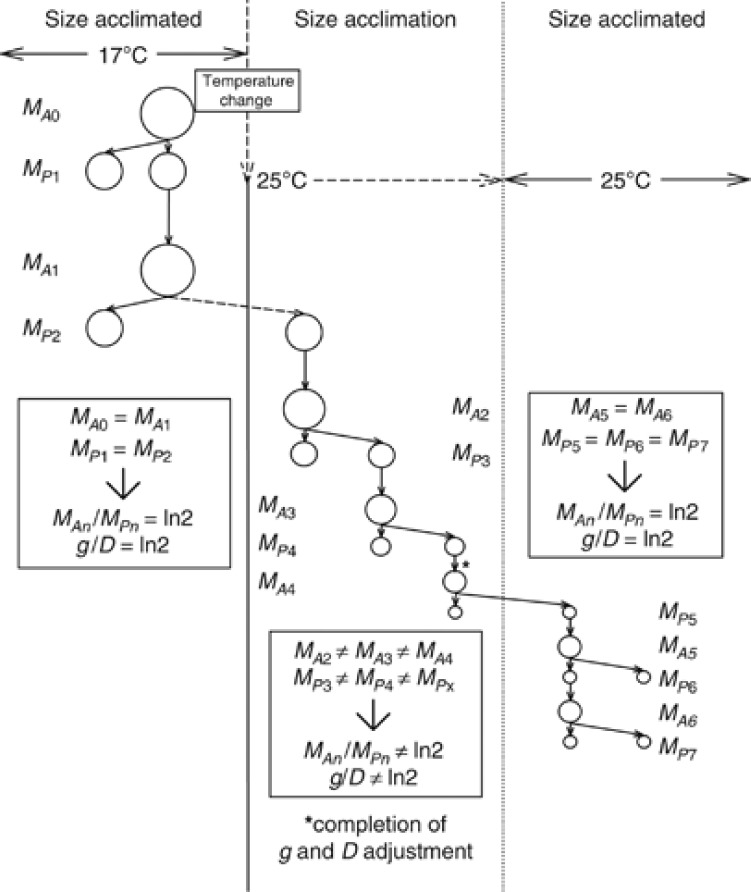
Figure 1.
A hypothetical example of the effect of temperature change on a unicellular organism which adheres to the TSR, where MA=adult mass (mother cell), MP=progeny mass (daughter cell) and subscript numbers represent generation number. The organism starts at 17 °C, MA/MP is a fixed ratio and thus g/D is fixed too. The organism is then displaced into an environment at 25 °C (indicated by the vertical arrow), as cell size must change, the g/D ratio must become temporarily decoupled. Finally, g/D returns to a fixed state of ln2 (0.69, in this example at the fourth generation, between MP4 and MA4) and adult and progeny size attain an acclimated size (MPn to MAn).
Along with understanding how size changes are brought about in unicells at an individual level, we need to understand the potential impact of the TSR on carrying capacity and biomass. There have been few studies examining the impact of temperature on these traits in unicellular organisms: previous ecological theory predicts carrying capacity (defined here as the number of organisms supported in a given volume) to decrease with increasing temperature, following an Arrhenius function (Savage et al., 2004), thus we may expect maximum number of individuals in a culture to decrease with increasing temperature. Further, mesocosm experiments investigating the impact of temperature on freshwater phytoplankton found higher temperatures to be associated with a reduced total biomass (Yvon-Durocher et al., 2011). However, this was not conducted at an individual species level, thus we do not know whether changes in biomass are related to shifts towards smaller species or intraspecific size changes. Here, we compare carrying capacity and biomass (the product of carrying capacity and mean cell volume (MCV)) in C. glaucoma across a range of temperatures to see whether these traits do indeed scale negatively with temperature.
Using the ciliate species C. glaucoma, we shall therefore address the following questions: How do mother and daughter cell sizes change when acclimating to a novel thermal environment? Does carrying capacity scale negatively with temperature in C. glaucoma? Finally, how does temperature impact on the maximum biomass of C. glaucoma populations?
Materials and methods
The protist species C. glaucoma was chosen due to its short generation times; having a standard geometric shape, such that cell volumes could be accurately determined from length and width measurements; and individuals undergoing fixation in formalin maintaining an excellent cell structure. Ten sterile culture flasks (30 ml flasks, Corning Incorporated, Corning, NY, USA) were prepared for C. glaucoma, using 30 ml of sterilised 30% filtered sea water, passed through a 0.20-μm Minisart filter (Sartorius Stedim Biotech, Goettingen, Germany). To each flask, one wheat grain, cut into two, was added. Wheat grains were boiled vigorously for 3 min before their addition to sterilise them. In all, 500 μl inoculum was then added to each of the 10 sterile culture flasks. Batch cultures of C. glaucoma used to inoculate these flasks were originally isolated, cloned and established in culture for a previous investigation (Finlay et al., 2006). These batch cultures were maintained at 17 °C for 2 weeks before experimentation. To allow the initiation of growth of the cultures, inoculated C. glaucoma flasks were maintained at 17 °C for a further 10 days, in accordance with pilot experiments, which indicated this was the minimum lag phase associated with this species. After this time, cultures were placed at a range of temperatures (8, 13, 17, 21 and 25 °C), with two flasks as replicates at each temperature. In all, 500 μl subsamples were taken from each culture over the course of 2 weeks. During the first 3 days, samples were taken every 4 h to obtain high temporal resolution results, and during this period we assumed acclimation would be most likely. Frequency of subsampling was then gradually reduced over the remainder of the 2-week period, such that the final subsamples were taken 3 days apart. C. glaucoma samples were preserved with 50 μl formalin for later counting.
Size, temperature and population abundance
Subsamples were analysed to calculate population abundances and cell volumes. Each was placed on a Sedgewick Rafter cell, which divides each sample into squares with 1 μl volumes. For each subsample, the numbers of individuals were counted in 50 randomly selected squares, and the mean number of cells calculated per 1 μl. Thirty individual cells were randomly selected, photographed under a × 100 magnification optical microscope and then measurements of length and width (μm) made from these photographs using QCapture Pro (QImaging Software, Surrey, British Columbia, Canada). Cell volume was calculated for each individual, assuming a standard geometric shape (prolate spheroid). Having estimated cell volumes for each individual, MCVs were calculated for the 30 cells per sample. MCVs and population abundances were subsequently plotted against time for each temperature and replicate. Doubling rates were calculated during the log period of growth, assuming mortality to be negligible during this phase. Doubling rates were calculated for each replicate as the slopes of natural logged population abundance vs time divided by ln(2). Further, to determine the relationship between MCV and population abundance, temperature and time, we constructed a GLM:
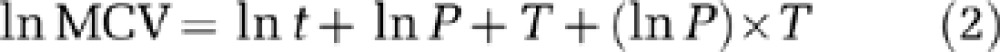 |
where MCV=mean cell volume (μm3), t=time (hours), P=population abundance (cells μl−1) and T=temperature (°C). MCVs, time and population abundance were natural logged to maintain the homoscedasticity of residuals. Further, these equation forms for each parameter were shown to fit the data well (Figure 2) and thus deemed appropriate models. Using these parameters, we determined whether each parameter had a significant effect on MCVs. When the interaction term (ln P) × T was not significant (P<0.05), this was removed from the GLM.
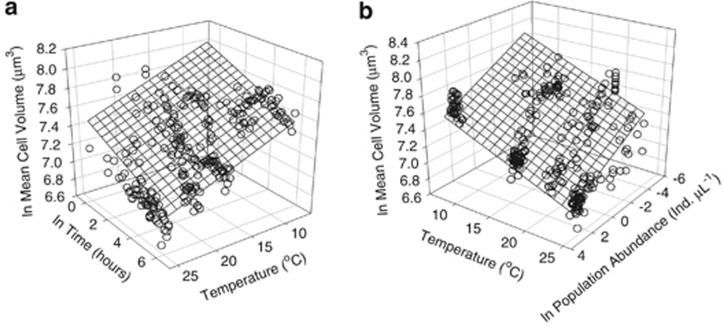
Figure 2.
Cyclidium glaucoma 3D scatter plots of ln MCV vs (a) ln time (t, hours) and temperature (T, °C); (b) temperature (T, °C) and ln population abundance (P, individuals μl−3). Meshes indicate best fit models using equation forms applied to the data in the GLM, that is, ln MCVs vs ln t, ln P and T. Meshes provide good fits to the data (P<0.0001 for all parameters).
Mother cell sizes were calculated from the mean size of the 6 largest cells per 30 cell sample. Similarly, daughter cell sizes were calculated from the mean size of the smallest 6 cells. As data were normally distributed in each sample of 30 cells at a particular time interval, this approximately estimates size data >1s.d. away from the mean value (∼20% of data in each tail). Moreover, the estimates for daughter cell sizes were similar to those for cells on the verge of division, photographed and calculated in pilot studies. Using these measurements for mother and daughter volumes, we similarly applied the GLM (Equation 2) to the data. We found a clear impact of population abundance upon the cell sizes of the organisms in the cultures. During the temperature acclimation period, population size was changing (log phase of population growth). We wished to remove the impact of this from the cell size change, such that the thermal acclimation could be calculated singularly. We corrected data to the population abundance, ensuring we were correcting only for population size and not temperature by using the slope of population abundance for the GLM applied to 17 °C only, as this was the culture in which temperature was in effect unchanged. Using the slope constant for population abundance (−0.233), cell volumes were all corrected to the temperature-independent carrying capacity (13.4 cells μl−1). Corrected cell volumes were subsequently plotted against temperature (across all treatments) at each observation time point, and the linear regression for each used to calculate percentage volume changes from that at 15 °C (following Atkinson et al., 2003). These percentage changes in volume were then plotted against time. Acclimated data were determined, working from the last observation point to that at which 95% confidence intervals (CIs) for a prior observation no longer overlapped. Using this point of acclimation, we calculated acclimated population abundance-corrected MCVs for each replicate. The time to cell size acclimation for each temperature and replicate was determined as being the first observation point where MCV was not significantly different from the acclimated MCV.
The TSR, carrying capacity and maximum biomass
To compare our temperature-size results with those for the many protists described in the meta-analysis conducted by Atkinson et al. (2003), we calculated acclimated MCVs at carrying capacity, and calculated the linear regression line between these values and temperature. We calculated the carrying capacity for each replicate, defining this as the point at which the slope of ln population abundance vs time was not significantly different from zero (one-way analysis of variance). We compared these carrying capacities across the different experimental temperatures. We also calculated the product of carrying capacity (cells μl−3) and MCV at this peak to determine the maximum biomass, and examined whether a decrease in cell size was associated with a concomitant decrease in maximum biomass.
Results
Size, temperature and population abundance
MCV increased with time when subjected to temperatures less than that at which they had previously been acclimated (that is, 17 °C), while MCV decreased with time at temperatures greater than this, thereby following the TSR. Changes in MCV were also associated with changes in population abundance. During the exponential growth phase, these changes in population abundance were accompanied by decreased MCV, suggesting a negative relationship between the two (see example in Figure 3). Application of the GLMs to ln MCVs revealed that all three factors (Equation 2) had a significant effect on MCV (P<0.001 in all cases). Population abundance had a negative effect on size, though the interaction term between population abundance and temperature was not significant (P=0.73). This suggests that the effects of population abundance and temperature on MCVs are additive and therefore independent of one another.
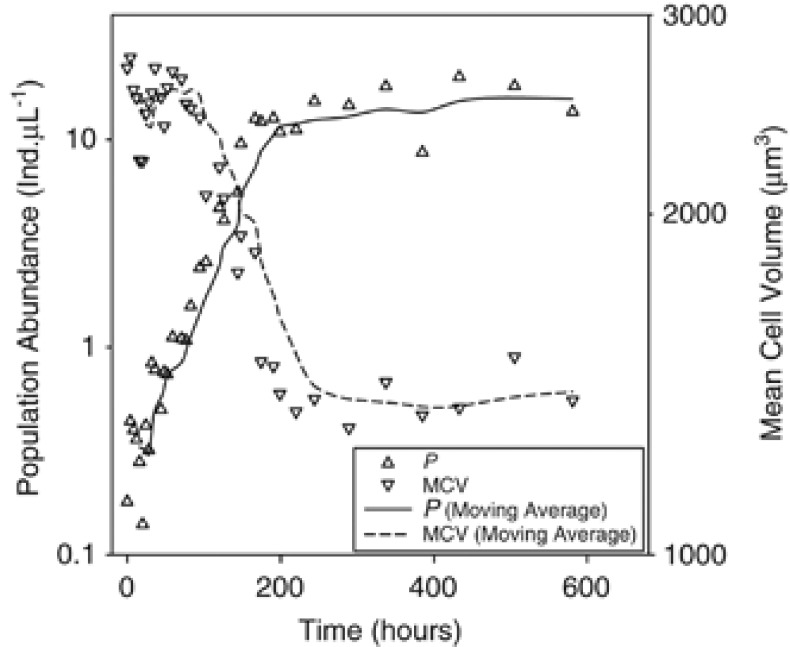
Figure 3.
Cyclidium glaucoma population abundance (LHS) and MCV (RHS) vs time (hours). Population abundance (P) and MCVs are plotted on log10 scales. Solid lines represent moving averages for population abundances and dashed lines represent moving averages for MCVs. Moving averages were calculated from the average of the previous five data points. Data presented are for a single replicate at 17 °C.
Mother and daughter size
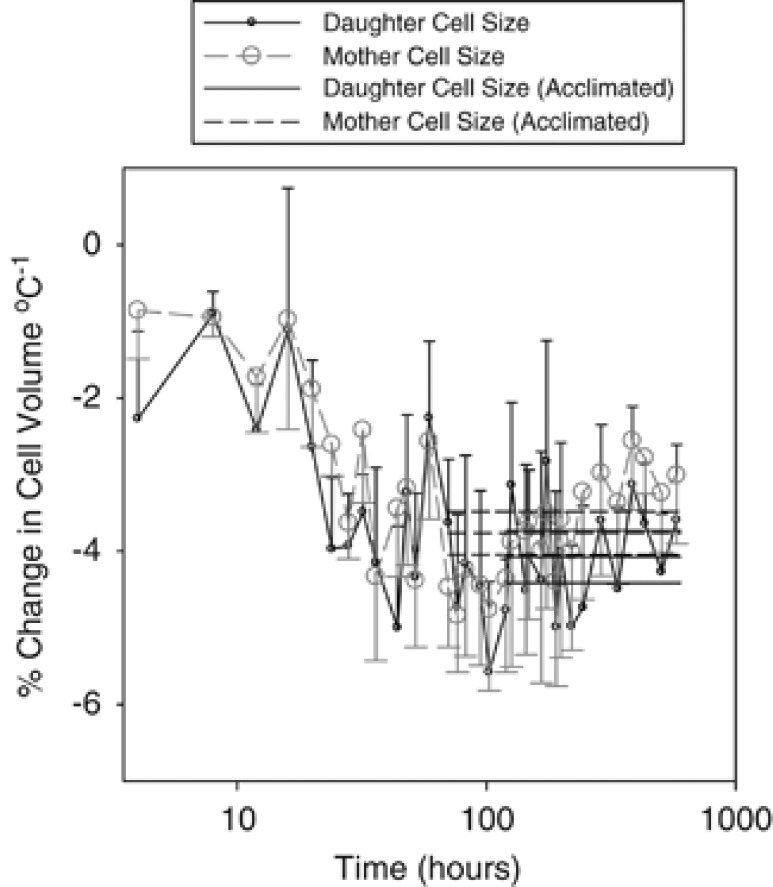
Having applied corrected mother and daughter size data (to remove the effect of population abundance on cell sizes), the percentage change in volume (from that at 15 °C) showed temperature acclimation in both mother and daughter size. The temperature dependence of mother and daughter size was determined as being acclimated at ∼70.5 and ∼120 h, respectively (Figure 4). Once daughters became acclimated, there was no discernible difference between the percentage change in volume in mothers or daughters (Figure 4).
Figure 4.
Cyclidium glaucoma slopes of mother and daughter cell volumes vs temperature plotted against time. Mother cell volumes were calculated from the mean of the largest 20% of cells in a sample (n=2 × 3), daughter volumes were calculated from the smallest 20% of cells in a sample (n=2 × 3). Slopes were calculated as % changes in size per °C from that at 15 °C, using linear regression of cell volumes vs temperature at each time interval. Thick horizontal lines are mean % size changes for time periods where confidence intervals overlap for daughter (solid line) and mother (dashed line) data. Thinner lines either side =95% confidence intervals.
The TSR, carrying capacity and maximum biomass
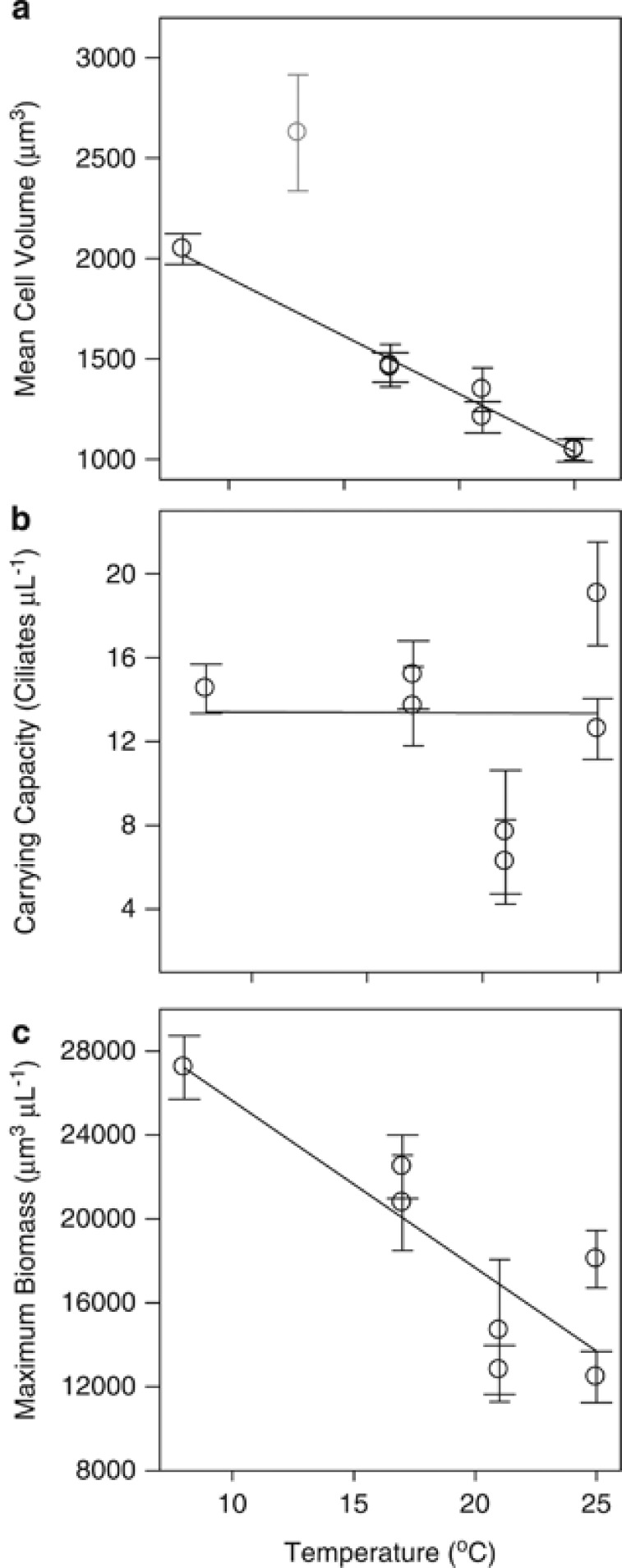
C. glaucoma followed the TSR, with a linear MCV change of −3.6% (CIs±0.45%) per °C (from the size at 15 °C, Figure 5a). The time at which MCV became acclimated corresponded to a point at or near the carrying capacity, except at 13 °C, where individuals exhibited poor population growth in both replicates. These data were confounded by low population abundances and were therefore excluded from the TSR calculation. This linear decline in size pattern was present across the entire thermal range, from 8 to 25 °C (Figure 5a). Carrying capacities were reached at 8, 17, 21 and 25 °C. There was no significant regression slope between population abundance and temperature (Figure 5b). The peak biomass was similarly plotted against temperature (Figure 5c); the highest biomass was associated with the lowest temperature, showing a linear decrease with increasing temperature (Figure 5c).
Figure 5.
(a) Temperature-size changes in the protist Cyclidium glaucoma. MCVs were calculated for acclimated samples at carrying capacity (‘acclimated' being defined as the point at which ln MCV across each time interval no longer had a slope significantly different from zero). Error bars represent 95% confidence intervals for each mean. The MCV for sample 13 °C A in C. glaucoma (given in grey) was not included within the linear regression, as the culture had not reached carrying capacity. (b) C. glaucoma carrying capacity (P). Mean values at each temperature were calculated over the range at which the regression of ln population abundance vs time had a slope which was not significantly different from zero (P>0.05). (c) Maximum biomass, calculated from the product of carry capacity and MCV. Error bars are 95% confidence intervals.
Discussion
The unicellular organism C. glaucoma followed the TSR, with acclimated cells showing a negative linear decline in the volume (from the size at 15 °C) of 3.6% (±0.45% CIs) per 1 °C increase in temperature (Figure 5a). This change in cell volume is not significantly different to the meta-analysis of protist size responses made by Atkinson et al. (2003), which showed cell size to change linearly by approximately −2.5% °C−1 (±0.78% CIs) from the volume at 15 °C. Further, there was no significant difference between the TSR in autotrophic and heterotrophic species in their study. C. glaucoma therefore appears to be a reasonable unicellular organism in which to study the establishment of the TSR, on the basis that the degree to which it changes size with temperature is fairly typical.
Data for mother and daughter size revealed thermal acclimation to occur rapidly, this being reached within ∼70.5 and 120 h, respectively (assessed as the point when the temperature-size response was not significantly different from acclimated response). These acclimated percentage mass changes required the slope of temperature vs cell size to reach a constant. Given the doubling time at 8 °C was 165 h, this lowest temperature treatment likely limited how long the TSR took to acclimate. Even so, it appears that size acclimation was intra-generational. Size changes were subsequently maintained beyond the point of acclimation. Although size changes are seen in both mother and daughter, we found that the daughters took longer to reach an acclimated state than did mothers (Figure 4). This is likely to be due to size changes in daughters being dependent on maternal cell size change, with a lag before the population of daughter cells became acclimated. This is the first study, of which we are aware, where the period over which thermal acclimation of size in a unicellular organism has been determined, and one of few studies which has focused on acclimatory changes associated with novel environments (salinity acclimation: Finlay et al., 2006). Our results have important implications with regard to Equation 1: we confirm that individual growth and development rates (doubling rates) do indeed become only temporarily decoupled, while the ratio of mother to daughter cell size becomes re-coupled after ∼120 h (Figure 4).
The significant decoupling of growth and development during thermal acclimation (during 0–120 h, Figure 4) has not been recorded previously in unicells. The changes in cell size we have shown during the log phase of population increase of this protist may have important implications upon other studies relying upon data from this same phase. For example, growth rates of many protozoa are commonly calculated from measurements of increase in cell numbers, as doubling rates during the log growth phase (for example, Eppley, 1972; Rose and Caron, 2007). The implicit assumption is that a doubling of numbers is associated with a doubling of biomass (with constant cell size). This may not be a complete description of growth, as it does not consider the MCVs, which change considerably with conditions (temperature and population abundance here). Moreover, classic studies used to estimate field growth rates use dilution experiments and subsequent doubling rates (Landry and Hassett, 1982). Again, there may be problems here if cell size is changing and increase in numbers is no longer coupled to an increase in total biomass. Furthermore, the fact MCVs change with temperature and population abundance will cause discrepancies between growth rates estimated from fluorescence (which will presumably be impacted by cell size change) vs those estimated from cell numbers. This highlights the importance of identifying the decoupling of growth and doubling rate during the acclimation, as found in this study.
Our results regarding size and rate acclimation to temperature reveal both similarities and differences between uni- and multicellular organisms. Both groups display rapid thermal acclimation: C. glaucoma appears thermally acclimated in size within one generation. Similarly, multicellular organisms appear to acclimate their size to their thermal environment within a single generation (Karan et al., 1998; Forster and Hirst, 2011). Further, these size changes are subsequently maintained in C. glaucoma across multiple generations, which have also been found in multicellular organisms (Karan et al., 1998; Forster and Hirst, 2011; Potter et al., 2011). However, relative size changes differ between these two groups. C. glaucoma shows acclimated size changes in daughters and mothers which are not significantly different from one another −4.07% °C−1 (CIs±0.34%) and −3.76% °C−1 (CIs±0.28%), respectively, Figure 4) whereas acclimated progeny size in multicellular organisms are commonly less temperature dependent than adult sizes (Forster et al., 2011a), resulting in acclimated development and growth rates having different temperature dependence (Forster et al., 2011a, b).
The TSR in unicellular organisms
What does this mean for our understanding of the TSR? Proximate mechanisms previously suggested to explain the TSR have been driven by either decoupling of growth and development rates (Sibly and Atkinson, 1994; van der Have and de Jong, 1996; Walters and Hassall, 2006), or different thermal sensitivities of anabolism and catabolism (Strong and Daborn, 1980; Perrin, 1995; Woods, 1999; Karl and Fischer, 2008). As the ratio MA/MP changes with temperature in multicellular organisms, growth and development (and possibly anabolism vs catabolism) have to be decoupled and have different temperature dependence across multiple (acclimated) generations (Forster et al., 2011a). However, this cannot be the case in protists, as sizes return to an acclimated state, and MA/MP becomes a constant (Figure 4). This requires g/D to also be a constant across temperatures when acclimated (Equation 1). Our results provide empirical evidence to support the conceptual scheme of Forster et al. (2011b), thus unicellular species achieve size changes associated with temperature through different mechanisms than those used by multicellular species. Across multicellular organisms, size changes have been shown to be brought about through changes in cell size (van Voorhies, 1996; Stelzer, 2002), changes in cell number (Noach et al., 1997; Arendt, 2007) or both (French et al., 1998; Blanckenhorn and Llaurens, 2005). Even within an organism, specific size changes have been shown to occur in some cell types but not others (Atkinson et al., 2006). These differences suggest there is no single universal proximate mechanism to explain the TSR in uni- and multicellular organisms, thus we are led to suggest that the TSR is an adaptive response, that is, that smaller size at warmer temperatures confers a fitness advantage to the majority of the species. The next important step is to determine why being smaller at warmer temperatures is advantageous.
Size, temperature and population abundance
Along with temperature-driven size changes in C. glaucoma, we found population abundance to significantly affect cell sizes. The negative correlation between cell size and population abundance was present across the entire exponential growth phase at higher temperatures (for example, Figure 3). If increased population abundances are associated with decreased MCVs in C. glaucoma, then how do we know there is a true temperature-size effect and that size changes are not simply driven by increasing population abundances? We find from the GLM that temperature has a significant effect on size, independent of population abundance, as there was no interaction between these two parameters. Therefore, these factors independently drive size changes. Indeed, along with C. glaucoma, decreasing MCV with increasing population abundance has previously observed in the dinoflagellates Alexandrium ostenfeldii (Jensen and Moestrup, 1997) and A. tamarense, and four diatom species (Prakash et al., 1973), suggesting this may be common in protists.
Why do MCVs change with population abundance in C. glaucoma? One factor potentially driving size changes is food concentration: as population abundances increase, food concentrations decrease and MCVs are reduced. Many studies have been conducted observing predator–prey interactions using protists as model organisms. In these predator–prey interactions, we find a cyclical population response, where protist populations increase to a peak and then crash as food becomes scarce (Sharon and Morin, 1993). If decreased food concentrations were driving the changes in size associated with the exponential growth phase seen in C. glaucoma, then we would expect to see a crash in population abundance following a peak. However, when we observe replicates 17 °C B and 25 °C A, where population abundances peak within the first 100 h of the experiment, we see no such crash in population abundance over the subsequent 500 h, suggesting food concentrations can support these population abundances and thus size changes are not driven by food shortages. Also, once cultures reached carrying capacity, MCVs are maintained (for example, from 200 h onwards, in Figure 3), whereas one would predict continued cell size reduction if food became more limiting. Further, a previous study investigating temperature-food interactions on cell volumes of Oxyrrhis marina (Kimmance et al., 2006) found an interactive effect between food and temperature, yet our GLM found no significant interaction between population abundance and temperature, again suggesting changes in size related to population abundance were not food driven.
The fact that cell sizes do change with population abundance in C. glaucoma, and have been shown to change during the exponential growth phase in a number of other protist species (Prakash et al., 1973; Jensen and Moestrup, 1997), has important implications for our understanding of the TSR. First, it shows a highly plastic response in cell volume in protist species; cell size can respond rapidly to changes in environmental conditions and population abundances. Second, it shows that measuring protist species' size during the exponential growth phase is likely to be associated with high variability in MCVs, driven by the additive effects of changes in population abundance and temperature. As Jensen and Moestrup (1997) pointed out, this exponential growth phase is not indicative of ‘balanced growth in a constant environment'. A previous meta-analysis of protist data attempted to resolve this problem by only recording cell size data taken during the log phase of population growth (Atkinson et al., 2003), yet we suggest this phase to be associated with high variability of MCV, driven by population abundance.
The TSR, carrying capacity and maximum biomass
The carrying capacity data for C. glaucoma revealed no clear pattern relating this to temperature (Figure 5b). Although the temperature dependence of intraspecific carrying capacity has received little attention, previous theoretical work focused on carrying capacity (number of cells per volume) predicts this to scale negatively with temperature (Savage et al., 2004), in line with the relationship found at an interspecific level. Similarly, interspecific data have been used to predict a universal decline in carrying capacity with increased body mass (Belgrano et al., 2002), in line with the metabolic theory of ecology (Brown et al., 2004). Such predictions for the impact of temperature and body size on intraspecific carrying capacity are not supported in this study on C. glaucoma. We found carrying capacity to be temperature and size independent: we therefore suggest extrapolating this to the intraspecific relationships should be conducted with caution.
Although carrying capacity does not change with temperature, this is not the case for biomass. As biomass is a product of size (Figure 5a) and population abundance (Figure 5b), the lowest temperature was associated with the highest biomass values, with a linear decrease in maximum supported biomass as temperatures increased, due to the temperature-size response of MCV (Figure 5a). This provides potential clues as to the adaptive nature of the TSR in unicellular organisms. If the number of individuals which can be supported is temperature invariant, then maximising reproductive rate at the cost of individual cell size with increased temperature will maximise fitness, as faster-reproducing individuals will make up a greater proportion of the final population (the law of ‘compound interest', Atkinson et al., 2003).
This study demonstrates rapid, intra-generational responses in a unicellular species' size to changing temperature. This shows fundamental differences between uni- and multicellular organisms. Further, population abundance is found not to be temperature dependent but maximum biomass decreases with temperature, driven by individual cell size change in line with the TSR. Data focusing on the interaction between cell size, population abundance and temperature during acclimation next require an extension beyond heterotrophic Protista, both at the intraspecific and at the food web level to determine the potential impacts of warming on size, population abundance and biomass.
Acknowledgments
J Forster was supported by a Natural Environment Research Council studentship (NE/G523655/1). We thank Tom Fenchel and anonymous reviewers, whose comments greatly improved the manuscript.
References
- Arendt J. Ecological correlates of body size in relation to cell size and cell number: patterns in flies, fish, fruits and foliage. Biol Rev. 2007;82:241–256. doi: 10.1111/j.1469-185X.2007.00013.x. [DOI] [PubMed] [Google Scholar]
- Atkinson D. Temperature and organism size - a biological law for ectotherms. Adv Ecol Res. 1994;25:1–58. [Google Scholar]
- Atkinson D, Ciotti BJ, Montagnes DJS. Protists decrease in size linearly with temperature: ca. 2.5% degrees C−1. Proc Biol Sci. 2003;270:2605–2611. doi: 10.1098/rspb.2003.2538. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Atkinson D, Morley SA, Hughes RN. From cells to colonies: at what levels of body organization does the ‘temperature-size rule' apply. Evol Dev. 2006;8:202–214. doi: 10.1111/j.1525-142X.2006.00090.x. [DOI] [PubMed] [Google Scholar]
- Belgrano A, Allen AP, Enquist BJ, Gillooly JF. Allometric scaling of maximum population density: a common rule for marine phytoplankton and terrestrial plants. Ecol Lett. 2002;5:611–613. [Google Scholar]
- Blanckenhorn WU, Llaurens V. Effects of temperature on cell size and number in the yellow dung fly Scathophaga stercoraria. J Therm Biol. 2005;30:213–219. [Google Scholar]
- Brown JH, Gillooly JF, Allen AP, Savage VM, West GB. Toward a metabolic theory of ecology. Ecology. 2004;85:1771–1789. [Google Scholar]
- Daufresne M, Lengfellner K, Sommer U. Global warming benefits the small in aquatic ecosystems. Proc Natl Acad Sci USA. 2009;106:12788–12793. doi: 10.1073/pnas.0902080106. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Eppley RW. Temperature and phytoplankton growth in the sea. Fishery Bull. 1972;70:1063–1085. [Google Scholar]
- Finlay BJ, Esteban GF, Brown S, Fenchel T, Hoef-Emden K. Multiple cosmopolitan ecotypes within a microbial eukaryote morphospecies. Protist. 2006;157:377–390. doi: 10.1016/j.protis.2006.05.012. [DOI] [PubMed] [Google Scholar]
- Forster J, Hirst AG. The temperature-size rule emerges from ontogenetic differences between growth and development rates. Funct Ecol. 2011;26:483–492. [Google Scholar]
- Forster J, Hirst AG, Atkinson D. How do organisms change size with changing temperature? The importance of reproductive method and ontogenetic timing. Funct Ecol. 2011a;25:1024–1031. [Google Scholar]
- Forster J, Hirst AG, Woodward G. Growth and development rates have different thermal responses. Am Nat. 2011b;178:668–678. doi: 10.1086/662174. [DOI] [PubMed] [Google Scholar]
- French V, Feast M, Partridge L. Body size and cell size in Drosophila: the developmental response to temperature. J Insect Physiol. 1998;44:1081–1089. doi: 10.1016/s0022-1910(98)00061-4. [DOI] [PubMed] [Google Scholar]
- Gardner JL, Peters A, Kearney MR, Joseph L, Heinsohn R. Declining body size: a third universal response to warming. Trends Ecol Evol. 2011;26:285–291. doi: 10.1016/j.tree.2011.03.005. [DOI] [PubMed] [Google Scholar]
- Jensen MO, Moestrup O. Autecology of the toxic dinoflagellate Alexandrium ostenfeldii: life history and growth at different temperatures and salinities. Eur J Phycol. 1997;32:9–18. [Google Scholar]
- Karan D, Morin JP, Moreteau B, David JR. Body size and developmental temperature in Drosophila melanogaster: analysis of body weight reaction norm. J Therm Biol. 1998;23:301–309. [Google Scholar]
- Karl I, Fischer K. Why get big in the cold? Towards a solution to a life-history puzzle. Oecologia. 2008;155:215–225. doi: 10.1007/s00442-007-0902-0. [DOI] [PubMed] [Google Scholar]
- Kimmance SA, Atkinson D, Montagnes DJS. Do temperature-food interactions matter? Responses of production and its components in the model heterotrophic flagellate Oxyrrhis marina. Aquat Microb Ecol. 2006;42:63–73. [Google Scholar]
- Krasnow RA. Mass, length and growth rate in single cells. J Theoret Biol. 1978;72:659–699. doi: 10.1016/0022-5193(78)90278-3. [DOI] [PubMed] [Google Scholar]
- Landry MR, Hassett RP. Estimating the grazing impact of marine micro-zooplankton. Marine Biol. 1982;67:283–288. [Google Scholar]
- Montagnes DJS, Franklin DJ. Effect of temperature on diatom volume, growth rate, and carbon and nitrogen content: reconsidering some paradigms. Limnol Oceanogr. 2001;46:2008–2018. [Google Scholar]
- Noach EJK, De Jong G, Scharloo W. Phenotypic plasticity of wings in selection lines of Drosophila melanogaster. Heredity. 1997;79:1–9. doi: 10.1038/hdy.1997.153. [DOI] [PubMed] [Google Scholar]
- Olson RJ, Watras CJ, Chisholm SW. Patterns of individual cell growth in marine centric diatoms. J Gen Microbiol. 1986;132:1197–1204. [Google Scholar]
- Perrin N. About Berrigan and Charnov life-history puzzle. Oikos. 1995;73:137–139. [Google Scholar]
- Petersen C, Woods HA, Kingsolver JG. Stage-specific effects of temperature and dietary protein on growth and survival of Manduca sexta caterpillars. Physiol Entomol. 2000;25:35–40. [Google Scholar]
- Potter KA, Davidowitz G, Woods HA. Cross-stage consequences of egg temperature in the insect Manduca sexta. Funct Ecol. 2011;25:548–556. [Google Scholar]
- Prakash A, Skoglund L, Rystad B, Jensen A. Growth and cell size distribution of marine planktonic algae in batch and dialysis cultures. J Fisheries Res Board Canada. 1973;30:143–155. [Google Scholar]
- Rose JM, Caron DA. Does low temperature constrain the growth rates of heterotrophic protists? Evidence and implications for algal blooms in cold waters. Limnol Oceanogr. 2007;52:886–895. [Google Scholar]
- Savage VM, Gillooly JF, Brown JH, West GB, Charnov EL. Effects of body size and temperature on population growth. Am Nat. 2004;163:429–441. doi: 10.1086/381872. [DOI] [PubMed] [Google Scholar]
- Sharon PL, Morin PJ. Food web architecture and population dynamics in laboratory microcosms of protists. Am Nat. 1993;141:675–686. doi: 10.1086/285499. [DOI] [PubMed] [Google Scholar]
- Sheridan JA, Bickford D. Shrinking body size as an ecological response to climate change. Nat Clim Change. 2011;1:401–406. [Google Scholar]
- Sibly RM, Atkinson D. How rearing temperature affects optimal adult size in ectotherms. Funct Ecol. 1994;8:486–493. [Google Scholar]
- Stelzer CP. Phenotypic plasticity of body size at different temperatures in a planktonic rotifer: mechanisms and adaptive significance. Funct Ecol. 2002;16:835–841. [Google Scholar]
- Strong KW, Daborn GR. The influence of temperature on energy budget variables, body size, and seasonal occurrence of the isopod Idotea baltica (Pallas) Can J Zool. 1980;58:1992–1996. [Google Scholar]
- van der Have TM, de Jong G. Adult size in ectotherms: temperature effects on growth and differentiation. J Theoret Biol. 1996;183:329–340. [Google Scholar]
- van Voorhies WA. Bergmann size clines: a simple explanation for their occurrence in ectotherms. Evolution. 1996;50:1259–1264. doi: 10.1111/j.1558-5646.1996.tb02366.x. [DOI] [PubMed] [Google Scholar]
- Walters RJ, Hassall M. The temperature-size rule in ectotherms: may a general explanation exist after all. Am Nat. 2006;167:510–523. doi: 10.1086/501029. [DOI] [PubMed] [Google Scholar]
- Woods HA. Egg-mass size and cell size: effects of temperature on oxygen distribution. Am Zool. 1999;39:244–252. [Google Scholar]
- Yvon-Durocher G, Montoya JM, Trimmer M, Woodward G. Warming alters the size spectrum and shifts the distribution of biomass in freshwater ecosystems. Global Change Biol. 2011;17:1681–1694. [Google Scholar]