Unifying model of shoot gravitropism reveals proprioception as a central feature of posture control in plants

Abstract

Gravitropism, the slow reorientation of plant growth in response to gravity, is a key determinant of the form and posture of land plants. Shoot gravitropism is triggered when statocysts sense the local angle of the growing organ relative to the gravitational field. Lateral transport of the hormone auxin to the lower side is then enhanced, resulting in differential gene expression and cell elongation causing the organ to bend. However, little is known about the dynamics, regulation, and diversity of the entire bending and straightening process. Here, we modeled the bending and straightening of a rod-like organ and compared it with the gravitropism kinematics of different organs from 11 angiosperms. We show that gravitropic straightening shares common traits across species, organs, and orders of magnitude. The minimal dynamic model accounting for these traits is not the widely cited gravisensing law but one that also takes into account the sensing of local curvature, what we describe here as a graviproprioceptive law. In our model, the entire dynamics of the bending/straightening response is described by a single dimensionless “bending number” B that reflects the ratio between graviceptive and proprioceptive sensitivities. The parameter B defines both the final shape of the organ at equilibrium and the timing of curving and straightening. B can be estimated from simple experiments, and the model can then explain most of the diversity observed in experiments. Proprioceptive sensing is thus as important as gravisensing in gravitropic control, and the B ratio can be measured as phenotype in genetic studies.

Plant gravitropism is the growth movement of organs in response to gravity that ensures that most shoots grow up and most roots grow down (1–6). As for all tropisms, a directional stimulus is sensed (gravity in this case), and the curvature of the organ changes over time until a set-angle and a steady-state shape are reached (2, 7, 8). The change in shape is achieved by differential elongation for organs undergoing primary growth (e.g., coleoptiles) or by differential differentiation and shrinkage of reaction wood for organs undergoing secondary growth (e.g., tree trunks) (9). Tropisms are complex responses, as unlike other plant movements (e.g., fast movements) (5, 10) the motor activity generated is under continuous biological control (e.g., refs. 3, 11, 12).

The biomechanics of plant elongation growth has been analyzed in some detail (5, 13, 14), but less is known about the biological control of tropic movements and differential growth (3, 6). Many molecular and genetic processes that occur inside sensing and motor cells have been described (2, 15). For example, statocysts are cells that sense gravity through the complex motion of small intercellular bodies called statoliths (16). However, a huge number of sensing and motor cells act together to produce the growth movements of a multicellular organ. How are the movements of an organ controlled and coordinated biologically? This is a key question, as establishing the correct posture of aerial organs with respect to the rest of the plant has important physiological and ecological consequences (e.g., access to light or long-term mechanical stability) (4).

The gravitropic responses of some plants and even fungi have similar features (8). In essence, this has been described as a biphasic pattern of general curving followed by basipetal straightening (GC/BS) (4, 17). First, the organ curves up gravitropically, then a phase of decurving starts at the tip and propagates downward, so that the curvature finally becomes concentrated at the base of the growth zone and steady (7–9, 18–20). This decurving, which has also been described as autotropic (i.e., the tendency of plants to recover straightness in the absence of any external stimulus) (7, 21), may start before the tip reaches the vertical (4). It is striking that organs differing in size by up to four orders of magnitude (e.g., from an hypocotyl to the trunk of an adult tree) display similar traits, despite great differences in the timing of the tropic movement and the motor processes involved (3). However, there are also differences in the gravitropic responses. Depending on the species and the growth conditions, plants may or may not oscillate transiently about the stimulus axis or reach a proper alignment with the direction of the stimulus (e.g., ref. 8).

Currently, the phenotypic variability of the GC/BS biphasic pattern over a broad sample of species is, however, hard to estimate quantitatively, as most studies of gravitropism have only focused on measuring the tip angle (3). As we shall demonstrate, it is necessary to specify the local curvature C (or equivalently, the inclination angle A) over the entire growth zone (Fig. 1) and how it changes over time. If this is done, it is possible to build up a minimal dynamic model for tropic movements in space. This can be combined with dimensional analysis (as is used in fluid mechanics, for example) to characterize the size and time dependencies and set up dimensionless control parameters. This then makes it possible to compare experiments with predictions from the model quantitatively over a broad taxonomical sample of species with very different sizes and growth velocities and to reveal universal behaviors and controlling mechanisms.

Fig. 1.

Successive shapes formed by plant organs undergoing gravitropism and a geometrical description of these shapes. (A) Time-lapse photographs of the gravitropic response of a wheat coleoptile placed horizontally (Movie S1). (B) Time-lapse photographs of the gravitropic response of an Arabidopsis inflorescence placed horizontally (Movie S2). White bars, 1 cm. (C) Geometric description of organ shape. The median line of an organ of total length L is in a plane defined by coordinates x, y. The arc length s is defined along the median line, with s = 0 referring to the base and s = L referring to the apex. In an elongating organ, only the part inside the growth zone of length L_gz from the apex is able to curve (with 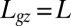at early stages and 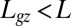later on), whereas the whole length is able to curve in organs undergoing secondary growth (i.e., 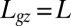). 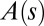is the local orientation of the organ with respect to the vertical and 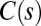the local curvature. The two curves shown have the same apical angle 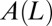but different shapes, so to specify the shape we need the form of 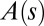or 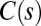along the entire median. Due to the symmetry of the system around the vertical axis, the angle A is a zenith angle—that is, it is zero when the organ is vertical and upright. Thus, an orthotropic organ has a gravitropic set point angle of 0. For simplicity, clockwise angles are considered as positive.

The gravitropic responses of 12 genotypes from 11 plant species were studied, representing a broad taxonomical range of land angiosperms (SI Appendix, Fig. S4), major growth habits (herbs, shrubs, and trees), as well as different uses (agriculture, horticulture, and forestry but also major laboratory model plants for genetics and physiology). Different types of organs were studied: coleoptile, hypocotyl, epicotyl, herbaceous and woody vegetative stems, and inflorescence stems, representing the two types of tropic motors (differential elongation growth, reaction woods) and varying by two orders of magnitude in organ size and in the timing of the tropic movements. Organs were tilted horizontally and the gravitropic growth was recorded through time-lapse photography.

All of the plant organs studied first curved upwards before eventually reaching a near vertical steady-state form where the apical part was straight, as shown for two examples in Fig. 1 and in Movies S1 and S2. The images were used to generate color maps of the curvature of the organ in space (along the organ) and time, as shown for three examples in Fig. 2. Shortly after plants were placed horizontally, the dominant movement observed was a rapid up-curving (negatively gravitropic) along the entire organ. However, the apex soon started to straighten and the straightening gradually moved downward along the organ. Finally, the curvature tended to concentrate at the base of the growth zone, becoming fixed there. Such a typical GC/BS behavior was observed in all 12 cases studied, despite differences of around two orders of magnitude in organ sizes and convergence time, the time 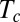taken for the organ to return to a steady state, ranging from several hours to several months.

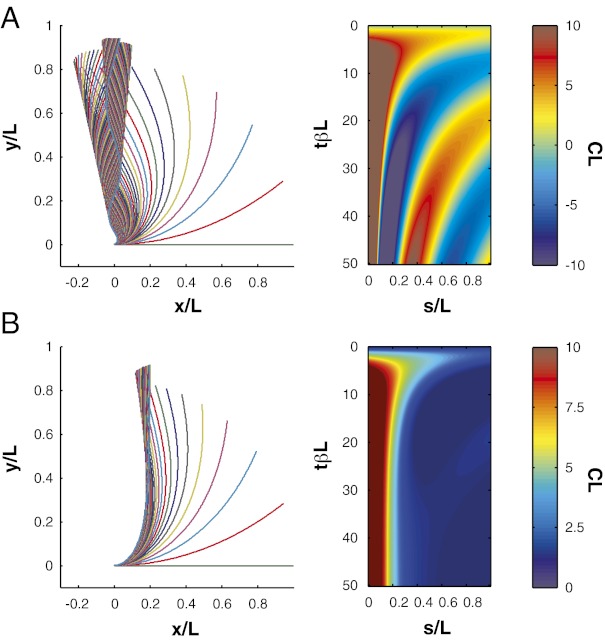
Fig. 2.

Kinematics of the entire tropic movement of tilted plant organs shown as color maps plotting the curvature with respect to time t and curvilinear abscissa s (the arc length along the median measured from the base to apex of the organ; Fig. 1). (A) Wheat coleoptile (Triticum aestivum cv. Recital). The yellow bar is 1 cm long. (B) Arabidopsis inflorescence (A. thaliana ecotype Col0). The yellow bar is 1 cm long. (C) Poplar trunk (Hybrid Populus deltoides x nigra cv I4551), reprocessed data from ref. 9.

Despite the common properties of the response, time lapse photography showed that plant organs acted differently when approaching the vertical. The apices of some plant organs never overshot the vertical (Fig. 1A), whereas others did so several times, exhibiting transient oscillations with the formation of C- or even S-shapes (Fig. 1B). Thus, a minimal dynamic model of gravitropism has to explain both the common biphasic GC/BS pattern and the diversity in transient oscillation and convergence time.

According to the literature, the current qualitative model of gravitropism in aerial shoots is based on the following hypotheses:

• H1: Gravisensing is exclusively local; each element along the length of the organ is able to respond to its current state (22), since statocysts are found all along the growth zone (16). Gravisensing by the apex does not have a special influence (e.g., the final shapes of organs after decapitation are similar to intact controls) (1, 23).

• H2: The local inclination angle A (Fig. 1) is sensed. This sensing follows a sine law (3, 6) (see below).

• H3: In our reference frame, the so-called gravitropic set angle (GSA) (24) is equal to 0 (Fig. 1) so the motion tends to bring the organ upward toward the vertical (this corresponds to the botanical term “negative ortho-gravitropism,” a most common feature in shoots).

• H4: The action of the tropic motor is fully driven by the perception–regulation process and results in a change in the local curvature through differential growth and/or tissue differentiation. This response can only be expressed where differential growth and differentiation occurs, namely in the “growth zone” of length 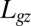(3).

To form a mathematical model, we shall describe the shape of the organ in terms of its median—that is, its central axis (Fig. 1). We parameterize the median by the arc length s going from the base 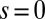to the apex 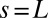, and the angle 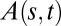describes the local orientation of the median with respect to the vertical at time t. The corresponding local curvature is the spatial rate of change of A along s and from differential geometry we know that:

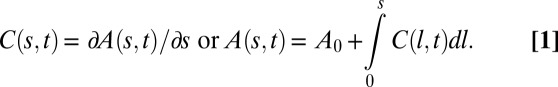The so-called “sine law” was first defined by Sachs in the 19th century and has been widely used since (see ref. 3 for a review). It can be expressed as a relationship between the change in the local curvature and the local angle as in:

where β is the apparent gravisensitivity. Note that Eq. 2 is unchanged when A changes to 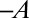and C changes to 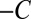, as would be expected. This model is only valid in the growth zone, 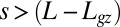, where L is the total organ length and 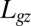is the length of the effective zone where active curving can be achieved. Outside this region, the curvature does not change with time.

In this model, changes in the overall length of the organ are not taken into account. This is quite reasonable in the case of woody organs, as they undergo curving through relatively small maturation strains in reaction woods, but it is less applicable to organs curving through differential elongation (3, 14). In expanding organs, each segment of the organ in the growth zone “flows” along the organ being pushed by the expansion growth of distal elements (3, 14) so Eq. 2 would remain valid only in a “comoving” context. To fully specify the changes in curvature, we would thus have to introduce local growth velocities into the model, replacing the derivative in Eq. 2 with the comoving derivative 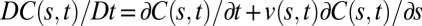, where is the local growth velocity. However, in tropic movement, the growth velocities are generally small compared with tropic bending velocities (and the length of the organ that has left the growth zone during the straightening movement is also small) (14), so 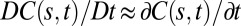. The limits of this approximation will be discussed.

To obtain a more tractable model, which we shall solve analytically, we can use the approximation and approximate Eq. 2 by:

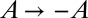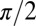where we note that the symmetry is retained. Because in our experiments did not exceed and because we are primarily interested in values near zero, this is a reasonable approximation (3). It should be noted that 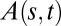and are not independent, as any further variation in curvature modifies the apical orientation through the “lever-arm effect” expressed in Eq. 1. In other words, the effect of changes in curvature on downstream orientation angles is amplified by the distance along the organ (3).

The solution of Eq. 3, which we shall call the “A model,” is:

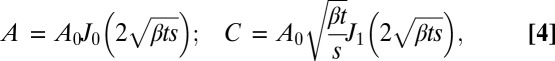where are Bessel functions of the first kind of order n. It has interesting properties. Firstly, the angle A does not depend on space s and time t individually, but only on the combination of 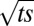and 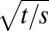and is thus an oscillatory function of 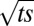. However, the dynamics of the A model demonstrates that such a system cannot reach a vertical steady state when tilted and clamped at its base (Fig. 3A and Movie S3). Indeed, the only steady state in Eq. 3 is 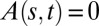, but this is forbidden by the basal clamping of the organ fixing 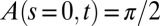for all t. Oscillations therefore go on indefinitely, whereas their wavelengths decrease with time. Numerical simulations of Eqs. 3 or 2 displayed the same behavior (SI Appendix Fig. S2). This does not agree with any of the experimental results. The A model based on the sine law is therefore not a suitable dynamic model of the gravitropic straightening movement and has to be rejected. To account for the steady state attained after tilting, another hypothesis needs to be introduced:

• H5: Each constituent element of the organ perceives its local deformation, the curvature, and responds in order to restore local straightness (7, 19). In animal physiology, this type of sensing is generally called “proprioception,” a self-sensing of posture or orientiation of body parts relative to the rest of the organism (25). This is not an unreasonable assumption as it is known experimentally that (i) plants can sense imposed bending (26, 27) and (ii) the curvature of the organ and subsequent mechanical loads have a direct effect on the orientation of microtubules that may then modify the rate of differential growth (28, 29).

Fig. 3.

Solutions of the dimensionless A and AC models. (Left) Time-lapse shapes along the movement. (Right) Color-coded space–time maps of curvature 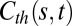. (A) Graviceptive A model where the response only depends on the local angle. As the organ approaches the vertical, the basal part continues to curve. The organ overshoots the vertical, and the number of oscillations increases with time (Movie S3). (B) Graviproprioceptive AC model where the response also depends on the local angle and the local curvature. Here the curvature decreases before reaching the vertical. It does exhibit an S shape, but oscillations are dampened, and the organ converges to a solution where the curvature is focused near the base (Movies S4 and S5).

This hypothesis yields a model called the “graviproprioceptive” model, or the “AC model”:

in the growth zone (i.e., for ), and 0 elsewhere. Here the change in curvature is directly related to the local curvature itself via the parameter γ, the proprioceptive sensitivity. A more systematic derivation of the A and AC models from symmetry arguments and rod kinematics is given in SI Appendix. The solution of the AC model has the form:

where it is seen that the dependence on 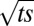and 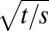is retained, but there is now an infinite sequence of Bessel functions. The first of the two expressions is appropriate for short times. The latter is appropriate for long times and shows that the oscillations are now dampened toward a final steady state, whose form is:

The dynamics of the AC model (Fig. 3B and Movies S4 and S5) is now qualitatively consistent with the experiments: the oscillations are dampened, and the organ converges to a steady state where the curvature is focused near the base through a typical GC/BS biphasic pattern.

The convergence length 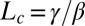is given by the decay length of the exponential toward the vertical, and it results from the balance between graviception and proprioception. The AC model thus gives a direct explanation of the common BS (autotropic) phase, where curvature starts to decrease before reaching the vertical (7, 20). For purely geometrical reasons (lever-arm effect, Eq. 1), the apical angles decrease faster than the basal angles. Thus, curvature sensing first takes over gravisensing at the tip and decurving starts there. It then moves downward together with the decrease of A without any need for a systemic basipetal propagative signal. Another important scale is 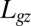, the effective length of the growth zone where active curving can be achieved. The ratio 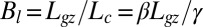is a dimensionless number that controls important aspects of the dynamics.

To assess whether the organ has time to converge to a steady state before the apex crosses the vertical, thereby avoiding overshooting, the time of convergence 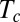can be compared with the time required for the apex to first reach the vertical, . Using Eq. 5, 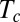can be approximated from the proprioceptive term that dominates when approaching convergence as 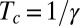and 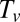can be approximated as from the graviceptive term dominating initial dynamics. This gives a “temporal” dimensionless number 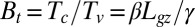, which is actually identical to . The fact that 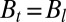gives a direct link between convergence timing, transient modes, and steady-state form (i.e., a kind of form-movement equivalence). We call this number the “bending number” denoted by B.

To compare theory and experiments, B, 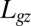, and 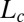were measured morphometrically from initial and steady-state images as shown for Arabidopsis inflorescence in Fig. 4. Because 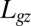is the length of the organ that has curved during the experiment, it can be directly estimated by comparing the two images. By definition, 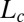can be measured directly on the image of the final shape as the characteristic length of the curved part (Fig. 4). The bending number B ranged from around 0.9–9.3 displaying broad intraspecific and interspecific variability over the experiments. Therefore, the AC model can be assessed from them.

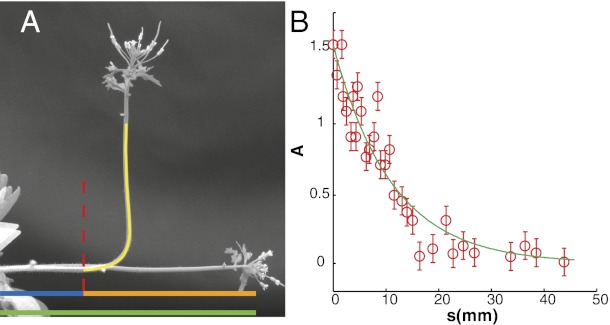
Fig. 4.

Morphometric measurement of the bending number B from steady-state configurations of Arabidopsis inflorescences. (A) Estimation of the effective length by superimposing the first and last kinematics images. The red dotted lines indicate the zone where the organ started to curve. The effective length of the organ can then be defined as the distance from this point to the apex of the initial plant on the first image. (B) Estimation of the convergence length 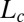by plotting the local inclination angle 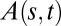along the organ beginning from the curved zone. To extract the convergence length 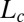, the angle 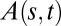is fitted with the exponential 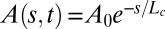, n = 28, R² = 0.99.

The kinematic data from wheat, Arabidopsis, and poplar was analyzed in more detail to track the tropic movement after tilting (Fig. 1). The analytical solution 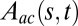for the AC model (Eq. 6) was compared with the experimental angle space-time maps, given the bending number value. Angles were chosen instead of curvature here, as otherwise the determination of curvature would involve a derivative, producing more noise. The initial value of B for parameter estimation was estimated morphometrically. As the AC model does not account for elongation growth, we trimmed the data for wheat and Arabidopsis to the length of the growth zone at the beginning of the experiment, as shown in Fig. 5. Typical results from Arabidopsis infloresences are shown in Fig. 5, and additional results from Arabidopsis, wheat, and poplar are provided in SI Appendix, Figs. S6, S7, and S8, respectively. The AC model was found to capture the common features of the angle space-time maps over the entire GC/BS process (compare Fig. 5 A and B). The (dimensionless) mean slope of comparison of the model vs. data (for the three species together) was 1.00 ± 0.15, the intercept was 0.07 ± 0.20, and the coefficient of determination was 0.92 ± 0.05, so the AC model captured around 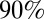of the total experimental variance in 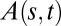and displayed no mean quantitative bias.

Fig. 5.

Quantitative comparison between experimental (exp) and predicted (th) angle space–time maps of 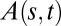for an Arabidopsis inflorescence for the whole gravitropic response. (A) Experimental angle space–time map of trimmed for 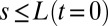, as the AC model does not consider changes in length. (B) Angle space–time map predicted by the AC model . (C) Quantitative validation plot of experimental 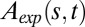vs. theoretical . Orthogonal linear fit slope, 1.13; intercept, 0.17; R² = 0.94.

The form–movement equivalence predicted by the AC model was then directly assessed through a simple morphometric analysis of the tilting experiments on the 12 angiosperm genotypes. More precisely, we assessed whether the AC model predicted the discrete transitions between transient oscillatory modes around the vertical (e.g., Fig. 1 and SI Appendix, Fig. S5) with increasing values of the bending number B. At a given time t, the current mode is defined as the number of places below the apex where the tangent to the central line of the organ is vertical (SI Appendix, Fig. S5). If there is one vertical tangent more basal than the apex, then the organ overshoots the vertical once. This is mode 1, when a C shape is formed. If an S shape develops, then the transient mode will be mode 2, and a shape is mode 3, and so on. The mode number M of the whole movement is then given by the maximal mode of all of the transitory shapes (e.g., in SI Appendix, Fig. S5, the mode of the movement of the inflorescence is 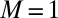as a transient C shape is seen but not an S shape). In Fig. 6, the modes of 12 plant organ responses were plotted against the respective estimated bending numbers and compared with the predictions of the AC model.

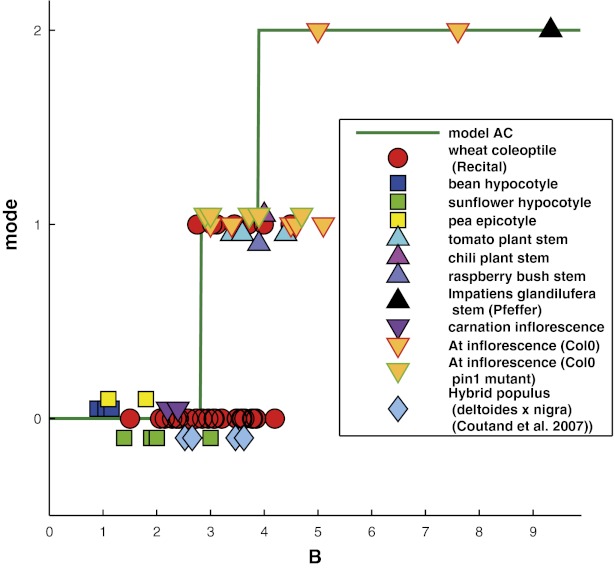
Fig. 6.

Mode number M plotted against bending number 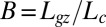for individual plants (N = 67). The green line shows the same plot for the AC model.

The prediction displays stepwise increases in modes at bending numbers corresponding to 2.8 for the transition from mode 0 to mode 1 and 3.9 for the transition from mode 1 to mode 2. No plant in the experiments displayed mode transitions for smaller bending numbers than was predicted by the AC model. Many individual plant responses were found near the transition from mode 0 to mode 1—that is, between the mode in which they cannot reach the vertical and the mode where they overshoot the vertical and oscillate. The transition from mode 2 to mode 3 only occurs for very large bending numbers (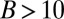) and was never seen in any of the experiments. In two-thirds of the plants, the prediction of the oscillations by the AC model was correct. However, about one-third of the plants oscillated less than predicted. To some extent, this may be due to inaccuracies in the estimation of bending numbers, but second-order mechanisms (possibly related to elongation growth) are likely to be involved, ones that add to the common graviproprioceptive core described by the AC model.

Nevertheless, the fact that the AC model accounts for the common GC/BS pattern with no quantitative bias and captures the transitions between three different modes over one order of magnitude of bending numbers and a broad taxonomical range is an indication of its robustness. All this strongly suggests that hypothesis 5 and its mathematical description by the AC model captures the universal core of the control over gravitropic dynamics. The longstanding sine law for gravitropism (3) should thus be replaced by the graviproprioceptive dynamic AC model, which highlights the equal importance of curvature- and gravisensing. Doing so has already yielded three major insights.

• i) The AC model can achieve distinct steady-state tip angles for the same vertical GSA. In particular, plants with 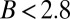cannot reach their GSA (as specified in the gravitropic term of the AC model) even in the absence of biomechanical and physiological limits in their motor bending capacity (3, 10, 12). Therefore, the GSA cannot be measured directly from experiments and can only be assessed by AC model–assisted phenotyping.

• ii) The fact that most plants display very few oscillations before converging to the steady state despite destabilization through lever-arm effects does not actually require the propagation of long-distance biological signals and complex regulation. The value of the dimensionless bending number simply has to be selected in the proper range—that is, graviceptive and proprioceptive sensitivities have to be tuned together as a function of organ size possibly pointing to molecular mechanisms yet to be discovered.

• iii) The AC model can account for the behavior of actively elongating organs despite neglecting the effects of mean elongation growth. Subapical elongation growth may have destabilizing effects by spreading curvature, convecting, and fixing it outside the growth zone in mature tissues (14). Our result means that the values for the time of convergence to the steady-state 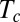were small enough compared with the characteristic times for elongation growth in all of the species studied. As 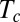depends mostly on the proprioceptive sensitivity, possibly there is natural selection for this trait as a function of the relative elongation rate (and organ slenderness) and for fine physiological tuning.

Proprioceptive sensing is thus as important as gravisensing for gravitropism. The study of molecular sensing mechanisms (2, 15) can thus now be extended to the cross-talk between gravi- and propriosensing as a function of organ size. Candidate mechanisms for the proprioception of the curvature may involve mechanical strain- or stress-sensing (27, 30) triggering microtubules reorientation (28, 29). Ethylene seems to be involved (17) but not the lateral transport of auxin (21). Whatever the detailed mechanisms involved, putative models of molecular networks controlling graviproprioceptive sensing (31) should be consistent with the AC model and with the existence of a dimensionless control parameter, the bending number. Moreover, the bending number B is a real quantitative genetic trait (32, 33). It controls the whole dynamics of tropic movement and encapsulates both the geometry and the perception–regulation functions involved (34). The simple measurement of B is now possible and this may be used for the high-throughput phenotyping of mutants or variants in many species. From a more general perspective, it would now be interesting to explore how plants manage to control gravitropism despite the destabilizing effects of elongation growth. Areas to investigate are whether there is physiological tuning of B during growth and whether there is natural selection for proprioceptive sensitivity as a function of the relative elongation rate and organ slenderness. For this, it will be necessary to combine noninvasive kinematics methods to monitor elongation growth at the same time as curvature (e.g., refs. 32, 33) with a more general model that explicitly includes the expansion and convection of cells during growth (3, 14). Finally, this approach can also be used to study the gravitropism of other plant organs and other growth movements like phototropism or nutation, which will show whether this theory of active movement is universal.

Materials and Methods

Experiments were conducted in growth cabinets for etiolated wheat coleoptiles (Triticum aestivum cv. Recital) or controlled temperature greenhouses for the nine other types of plant organs—bean hypocotyl (Phaseolus vulgaris), sunflower hypocotyl (Helianthus annuus), pea epicotyl (Pisum sativum), tomato stem (Solanum lycopersicum), chili stem (Capsicum annuum), raspberry cane (Rubus ideaus), carnation inflorescence (Dianthus caryophyllus), and Arabidopsis thaliana inflorescences from a wild-type (ecotype Col0) and its pin1 mutant [a mutant of the PIN1 auxin efflux carier displaying reduced auxin longitudinal transport (11) (see SI Appendix, sections S2.1 and S2.4 for more details)]. Plants were grown until a given developmental stage of the organ of interest (e.g., until the beginning of inflorescence flowering for Arabidopsis in Fig. 4). They were then tilted and clamped horizontally A(s = 0, t) = ϕ/2 for all t under constant environmental conditions in the dark (to avoid interactions with phototropism). Number of replicates were 30 for wheat, 15 for Arabidopsis, and 5 for all the other species. Published data were also reprocessed from similar experiments on Impatiens glandilufera stems by Pfeffer (35) and on poplar trunks (Populus deltoides x nigra cv I4551) by Coutand et al. (9). More precisely, two types of experiments were conducted, as explained in SI Appendix, section S2.2 and S2.5: (i) detailed kinematics experiments on two model species (Arabidopsis and wheat), based on time-lapse photography and quantitative analysis of curving-decurving kinematics (SI Appendix, sections S2.2 to S2.4) and (ii) simplified morphometric experiments on all the genotypes, to estimate the bending number (through B_l = L_gz/L_c) and the (transient) global mode M, defined as the maximum number of places below the apex where the tangent to the central line of the organ is vertical (SI Appendix, Fig. S5 and section S2.5). Quantitative assessment of the AC model was conducted by fitting Eq. 6 to the datasets from the detailed kinematics experiments (including also poplar; see SI Appendix, section S2.6), whereas a qualitative assessment on mode transitions and space-time equivalence was conducted on the dataset from the morphometric experiment (including also Impatiens; see SI Appendix, section S2.5).