Skip to main content
PMC home page
Medical Physics logoLink to Medical Physics
. 2013 Feb 12;40(3):031706. doi: 10.1118/1.4791671

The effect of flattening filter free delivery on endothelial dose enhancement with gold nanoparticles

Alexandre Detappe 1, Panagiotis Tsiamas 2, Wilfred Ngwa 3, Piotr Zygmanski 3, Mike Makrigiorgos 3, Ross Berbeco 3,a)
PMCID: PMC3585757  PMID: 23464301

Abstract

Purpose: The aim of this study is to quantify and to compare the dose enhancement factor from gold nanoparticles (AuNP) to tumor endothelial cells for different concentrations of AuNP, and clinical MV beam configurations.

Methods: Tumor endothelial cells are modeled as slabs measuring 10 × 10 × 2 μm. A spherical AuNP is simulated on the surface of the endothelial cell, within the blood vessel. 6 MV photon beams with and without the flattening filter are investigated for different field sizes, depths in material and beam modulation. The incident photon energy spectra for each configuration is generated using EGSnrc. The dose enhancement in the tumor endothelial cell is found using an analytical calculation. The endothelial dose enhancement factor is defined to be the ratio of the dose deposited with and without AuNPs.

Results: It is found that clinical beam parameters may be chosen to maximize the effect of gold nanoparticles during radiotherapy. This effect is further amplified ∼20% by the removal of the flattening filter. Modulation of the clinical beam with the multileaf collimator tends to decrease the proportion of low energy photons, therefore providing less enhancement than the corresponding open field.

Conclusions: The results of this work predict a dose enhancement to tumor blood vessel endothelial cells using conventional therapeutic (MV) x-rays and quantify the relative change in enhancement with treatment depth and field size.

Keywords: gold nanoparticle, vascular-disrupting agent, flattening filter free, dose enhancement, Monte-Carlo

INTRODUCTION

There has been an increasing interest in nanoparticles for cancer treatment.1, 2, 3, 4 Recent work has showed their potential to improve drug delivery,5 imaging contrast,6 and radiation therapy.2, 7 The use of gold nanoparticles (AuNP) as a radio-enhancer is especially compelling because of their biocompatibility,8 ease of surface functionalization, and high atomic number (Z = 79). This last property is important in the generation of short range photoelectrons or Auger electrons when irradiated with low energy photons which can enhance the dose locally. Theoretical results have been buttressed by preclinical studies showing the potential for significant therapeutic gain when AuNP are administered prior to radiation therapy.2

Previous Monte Carlo studies have prematurely dismissed high energy photons as a clinically enhancing source.9, 10, 11 This is mainly due to the common assumption of a homogeneous distribution of AuNP within the tumor. While most of these studies calculate dose to the whole tumor, some authors have acknowledged that a substantial local enhancement is possible.11, 12 Coupled with experimental studies of AuNP accumulation in tumor vasculature,13, 14 these results suggest the potential use of gold nanoparticles as vascular disrupting agents (VDA) during clinical (MV) radiotherapy.

Analytical calculations of brachytherapy15 and external beam radiotherapy12 have shown the potential benefit of targeted gold nanoparticles as VDA. The goal of VDA therapies is to collapse a solid tumor with a vascular structure by depriving it of nutrients and oxygen.16 We have hypothesized that AuNPs can be used in combination with standard radiation therapy for this same purpose. In our concept, the therapy target can be covered by conventional radiation doses, while the local dose enhancement is delivered in close proximity to the AuNPs.

In this work, we extend the previous analytic calculations by including photon energy spectra generated with Monte Carlo under various clinical conditions. The underlying hypothesis is that increasing proportions of low energy photons will lead to larger endothelial dose enhancement factors (EDEF). The goal of this paper is to determine the EDEF under these various clinical beam configurations simulated for a 6 MV linac. The results will provide a basis for further translational research.

MATERIALS AND METHODS

Photon energy spectra

The photon energy spectra used in this study are generated using the EGSnrc Monte Carlo code for a clinical linac-generated 6 MV photon beam. Standard (STD) delivery and delivery without the flattening filter (FFF) are investigated for different field sizes, depths in material and beam modulation. The investigated beam conditions are shown in Table 1.

Table 1.

Clinical configurations simulated for STD and FFF at the central axis.

  Depth in water
Field size (cm2) 2 cm 10 cm 20 cm
3 × 3 + + +
5 × 5 + + +
10 × 10 + + +
10 × 10 (SW) + + +
14 × 28 + + +
Open in a new tab

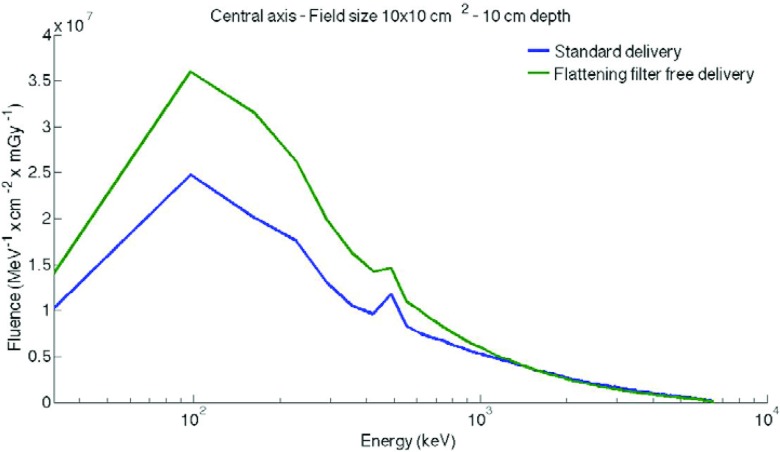
All beams represent a specific configuration of the linear accelerator which has been experimentally validated in our clinic.17 In this study, all calculations are performed at the central axis. Intensity-modulated radiation therapy (IMRT) is simulated by a 1 cm sweeping gap (SW). The fluence in MeV−1 ⋅ cm−2 is obtained from these simulations for each 65 keV energy bin between 0 and 6.5 MeV. The dose is calculated with the DOSXYZnrc code. The phantom size simulated is 100 × 100 × 40 cm and placed at 100 cm from the source (SSD 100 cm). The calculation is based on a uniform voxel measuring 1.0 × 1.0 × 0.5 cm. Thus, the fluence is divided by the dose calculated in mGy, for each depth in water (2, 10, and 20 cm). The fluence in MeV−1 ⋅ cm−2 ⋅ mGy−1 is used for the rest of the study. Examples of the photon energy spectrum are shown in Fig. 1 for STD and FFF deliveries, at 10 cm depth for a 10 × 10 cm2 field.

Figure 1.

Figure 1

Open in a new tab

Representation of the fluence in MeV−1⋅cm−2⋅mGy−1 at the central axis for a 10 × 10 cm2 field size with a semilog scale.

The analytical calculation

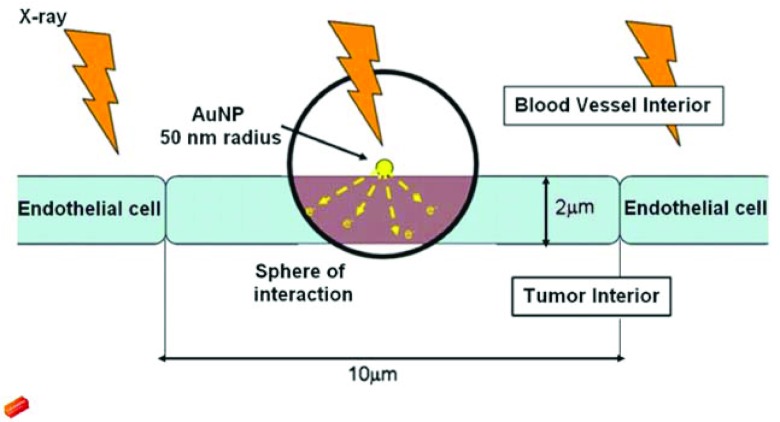
The analytical calculation we used is presented in a previous paper.12 Each AuNP is simulated as a sphere on the exterior surface of an endothelial cell (Fig. 2). This is a conservative location for the AuNP as endocytosis is likely to occur. The size of the AuNP is arbitrary in this calculation as it will cancel out when the enhancement relative to AuNP concentration is calculated. Self-absorption of photoelectrons within the AuNP is not considered, as it is negligible for MV beams.18 The endothelial cell is simulated as a thin slab measuring 2 μm (thickness) × 10 μm (length) × 10 μm (width). The positioning of the nanoparticles along the exterior of the endothelial cell is not an important consideration because lateral equilibrium is assumed.

Figure 2.

Figure 2

Open in a new tab

The sphere of interaction represents the range of photoelectrons generated by AuNPs [Berbeco et al. (Ref. 22)].

RESULTS

The energy bin with the largest contribution has a mean value of 97.5 keV. For this energy bin, the probability to create a photoelectron is equal to 6.9 × 10−4 per photon incident upon a gold nanoparticle with a 100 nm diameter. The size is arbitrarily chosen for ease of comparison with the earlier work. As in that study, the nanoparticle size ends up canceling out when the overall local concentration is investigated. The additional dose deposited inside the adjacent endothelial cell by one AuNP-photon interaction at this energy is 3.7 × 10−3 Gy.

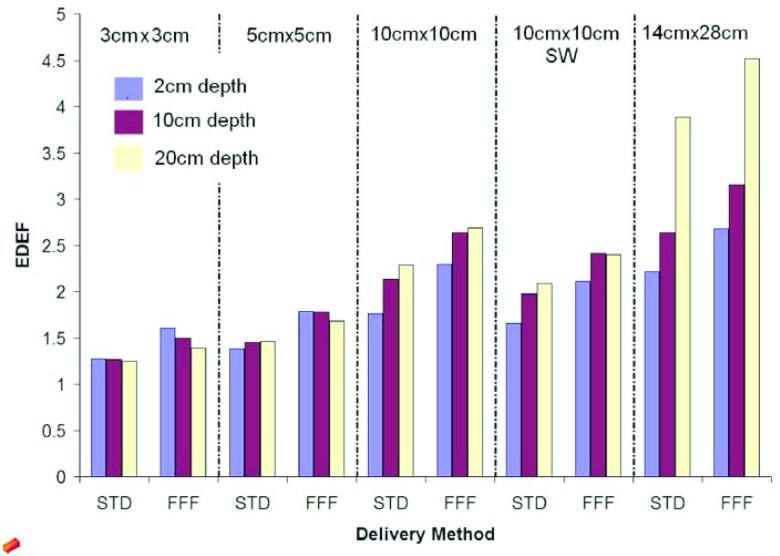
With a local concentration of AuNPs equal to 30 mg/ml, a STD delivery method, at 2 cm depth, for a field size 10 × 10 cm2, the EDEF is equal to 1.64 for the 97.5 keV energy bin. This result is similar to our previous study using a different source for the photon energy spectra.12 Summing all of the energy bins for this set-up, the total EDEF is 2.1. The results for EDEF as a function of depth are shown in Fig. 3 for a concentration of AuNPs equal to 30 mg/ml of tissue.

Figure 3.

Figure 3

Open in a new tab

The total EDEF with 30 mg/ml of gold nanoparticles for 3 different depths: 2, 10, and 20 cm for 5 field sizes: 3 × 3, 5 × 5, 10 × 10, 10 × 1 0 (SW), and 14 × 28 cm2.

In general, EDEF increases with field size, going from 1.2 (3 × 3 cm2, STD, 20 cm depth) to 3.9 (14 × 28 cm2, STD, 20 cm depth). The total range in EDEF calculated is from 1.2 (3 × 3 cm2, STD, 20 cm depth) to 4.5 (14 × 28 cm2, FFF, 20 cm depth). The differences in EDEF for each configuration can be explained by the effects of field size, depth, and delivery mode, on the spectral content of the photon beam at the point of interaction.

For field sizes greater than 5 × 5 cm2, EDEF increases with increasing depth for both STD and FFF deliveries. For 5 × 5 cm2 and below, EDEF is roughly constant with depth for STD delivery and decreases slightly with depth for FFF delivery.

EDEF increases linearly as a function of the local AuNP concentration, regardless of depth and delivery type. This is due to the linear component of concentration in the calculation. For each field size and depth, the EDEF is highest for FFF, then FFF SW, then STD, and the least for STD SW.

The EDEF for FFF deliveries are always higher than for the corresponding STD delivery, due to the inclusion of more low energy photons in the primary beam. The percentage difference between FFF and STD is greatest for the smallest field sizes and shallowest depths (Fig. 3). For the same method of delivery, a higher value of the EDEF is obtained for the open beams than the respective modulated one.

DISCUSSION

Hainfeld et al.2 demonstrated experimentally that it is possible to enhance the effects of radiation therapy by prior administration of AuNPs in a murine tumor model. This study and the theoretical ones that followed have focused mainly on irradiation with kV x-rays, due to the clear advantage, in terms of photoelectric interaction efficiency as well as Auger electron production. However, the use of kV x-rays in humans is severely limited due to either gross under-coverage of the tumor, very poor skin-sparing in the patient or necessitating the implantation of radioisotopes, an invasive procedure which is only appropriate for a small subset of patients. By focusing our attention on MV photon beams produced by clinical linear accelerators, we prepare for a broader clinical application and potentially clearer path to clinical trials.

In this study, we have calculated the dose enhancement due to photoelectric interactions only, excluding the contributions of Auger electrons as well as Compton interactions. Auger production was not included because it has been shown that the impact of Auger electrons is most substantial below 20 keV incident photons.19 In addition, the very short range of Auger electrons necessitates close proximity of the source with the target DNA, something which is not included in our conservative model. The cross-section for Compton interactions has little dependence on the atomic number (Z) of the material and, therefore, is not expected to contribute substantially to the EDEF. It should be noted, however, that if our assumptions are incorrect and Auger and Compton interactions were left out in error, this would only lead to larger dose enhancement than is reported in this study. Therefore, our results can be taken as a conservative estimate on these grounds.

Published experimental results in cell culture indicate similar findings albeit with less energy dependence than expected. Using fitted LQ parameters, Chithrani et al.20 showed dose enhancement factors of 1.66 (105 kVp), 1.43 (220 kVp), 1.18 (137Cs), and 1.17 (6 MVp). Jain et al.21 found factors of 1.41 (160 kVp), 1.29 (6 MV), and 1.16 (15 MV) for MDA-MB-231 cells and no significant effect for L132 or DU145 cells. Similar effects were seen by Liu et al.,7 but the published results are not easily comparable. While some of these differences may be due to nanoparticle formulation, cell line, experimental setup, dose calculation, and other experimental procedures, it is still possible that some other factor is limiting the efficacy of the kV beams. Speculation on this is beyond the scope of the current work, but it is something that should be investigated.

In this paper, we have calculated the dose enhancement to tumor endothelial cells, anticipating that targeted gold nanoparticles may be used as vascular disrupting agents, when irradiated with clinical photon beams. The results of this study indicate that clinical beam parameters may be chosen to maximize the proportion of low energy photons incident on the nanoparticles, and thereby maximizing the therapeutic effect. When more scattering material (e.g., larger field sizes, depths in tissue) precedes the AuNP, the beam will be relatively “softer” than the primary, alone. An exception occurs for very small field sizes (3 × 3 cm2). In this case, the enhancement decreases slightly as a function of depth, as the primary beam is the dominant contributor.

The enhancement effect for every field size and depth investigated is amplified by the removal of the flattening filter; a finding that has been experimentally confirmed in an in vitro study by Berbeco et al.22 These findings are also consistent with the known increase in the contribution of low energy photons in a FFF delivery. The advantage of the inherently softer FFF primary beam is lessened at increasing treatment depths for small field sizes. Modulation of the external clinical beam with the multileaf collimator tends to decrease the proportion of low energy photons, therefore providing less enhancement than the corresponding open field for both STD and FFF delivery techniques.

Due to the complexity of biological systems, the unknown influence of the tumor microenvironment as well as the response of the rest of the tumor system to local vascular damage, the values given in the results may not translate to clinical outcomes in a linear fashion. However, the relative damage enhancements expected due to differing depth, field size, and delivery mode should translate readily to the human system.

In a future clinical application, AuNP could be administered prior to radiation therapy to enhance tumor damage. The amount, concentration, frequency, and timing of injection are all factors that have not yet been determined. Numerous safety studies are necessary before clinical implementation of this technology. Given the known biocompatibility of gold and the precision with which modern radiation therapy can be delivered, we expect less toxicity less than for comparable chemoradiotherapeutics.

CONCLUSIONS

The results of this work predict a dose enhancement to tumor blood vessel endothelial cells using conventional therapeutic (MV) x-rays and quantify the relative change in enhancement with treatment depth and field size. The radiation dose delivered to tumor endothelial cells during AuNP-aided radiation therapy will depend on the location in the body, treatment beam parameters as well as the local concentration of AuNP. Experiments at the preclinical level are planned to further corroborate these results.

ACKNOWLEDGMENTS

The project described was supported, in part, by a Brigham and Women's Hospital Biomedical Research Institute Seed Grant and by Award No. R03CA164645 from the National Cancer Institute. The content is solely the responsibility of the authors and does not necessarily represent the official views of the National Cancer Institute or the National Institutes of Health.

References

  1. Hainfeld J. F., Dilmanian F. A., Slatkin D. N., and Smilowitz H. M., “Radiotherapy enhancement with gold nanoparticles,” J. Pharm. Pharmacol. 60(8), 977–985 (2008). 10.1211/jpp.60.8.0005 [DOI] [PubMed] [Google Scholar]
  2. Hainfeld J. F., Slatkin D. N., and Smilowitz H. M., “The use of gold nanoparticles to enhance radiotherapy in mice,” Phys. Med. Biol. 49(18), N309–N315 (2004). 10.1088/0031-9155/49/18/N03 [DOI] [PubMed] [Google Scholar]
  3. Jain S., Hirst D. G., and O’Sullivan J. M., “Gold nanoparticles as novel agents for cancer therapy,” Br. J. Radiol. 85(1010), 101–113 (2012). 10.1259/bjr/59448833 [DOI] [PMC free article] [PubMed] [Google Scholar]
  4. Moghimi S. M., Hunter A. C., and Murray J. C., “Long-circulating and target-specific nanoparticles: Theory to practice,” Pharmacol. Rev. 53(2), 283–318 (2001). [PubMed] [Google Scholar]
  5. Siemann D. W., Chaplin D. J., and Horsman M. R., “Vascular-targeting therapies for treatment of malignant disease,” Cancer 100(12), 2491–2499 (2004). 10.1002/cncr.20299 [DOI] [PubMed] [Google Scholar]
  6. Popovtzer R. et al. , “Targeted gold nanoparticles enable molecular CT imaging of cancer,” Nano Lett. 8(12), 4593–4596 (2008). 10.1021/nl8029114 [DOI] [PMC free article] [PubMed] [Google Scholar]
  7. Liu C. J. et al. , “Enhancement of cell radiation sensitivity by pegylated gold nanoparticles,” Phys. Med. Biol. 55(4), 931–945 (2010). 10.1088/0031-9155/55/4/002 [DOI] [PubMed] [Google Scholar]
  8. Connor E. E., Mwamuka J., Gole A., Murphy C. J., and Wyatt M. D., “Gold nanoparticles are taken up by human cells but do not cause acute cytotoxicity,” Small 1(3), 325–327 (2005). 10.1002/smll.200400093 [DOI] [PubMed] [Google Scholar]
  9. Cho S. H., “Estimation of tumour dose enhancement due to gold nanoparticles during typical radiation treatments: A preliminary Monte Carlo study,” Phys. Med. Biol. 50(15), N163–N173 (2005). 10.1088/0031-9155/50/15/N01 [DOI] [PubMed] [Google Scholar]
  10. Cho S. H., Jones B. L., and Krishnan S., “The dosimetric feasibility of gold nanoparticle-aided radiation therapy (GNRT) via brachytherapy using low-energy gamma-/x-ray sources,” Phys. Med. Biol. 54(16), 4889–4905 (2009). 10.1088/0031-9155/54/16/004 [DOI] [PMC free article] [PubMed] [Google Scholar]
  11. Jones B. L., Krishnan S., and Cho S. H., “Estimation of microscopic dose enhancement factor around gold nanoparticles by Monte Carlo calculations,” Med. Phys. 37(7), 3809–3816 (2010). 10.1118/1.3455703 [DOI] [PubMed] [Google Scholar]
  12. Berbeco R. I., Ngwa W., and Makrigiorgos G. M., “Localized dose enhancement to tumor blood vessel endothelial cells via megavoltage x-rays and targeted gold nanoparticles: New potential for external beam radiotherapy,” Int. J. Radiat. Oncol., Biol., Phys. 81(1), 270–276 (2011). 10.1016/j.ijrobp.2010.10.022 [DOI] [PubMed] [Google Scholar]
  13. Murphy E. A. et al. , “Nanoparticle-mediated drug delivery to tumor vasculature suppresses metastasis,” Proc. Natl. Acad. Sci. U.S.A. 105(27), 9343–9348 (2008). 10.1073/pnas.0803728105 [DOI] [PMC free article] [PubMed] [Google Scholar]
  14. Perrault S. D., Walkey C., Jennings T., Fischer H. C., and Chan W. C. W., “Mediating tumor targeting efficiency of nanoparticles through design,” Nano Lett. 9(5), 1909–1915 (2009). 10.1021/nl900031y [DOI] [PubMed] [Google Scholar]
  15. Ngwa W., Makrigiorgos G. M., and Berbeco R. I., “Applying gold nanoparticles as tumor-vascular disrupting agents during brachytherapy: Estimation of endothelial dose enhancement,” Phys. Med. Biol. 55(21), 6533–6348 (2010). 10.1088/0031-9155/55/21/013 [DOI] [PubMed] [Google Scholar]
  16. Lippert J. W., “Vascular disrupting agents,” Bioorg. Med. Chem. 15(2), 605–615 (2007). 10.1016/j.bmc.2006.10.020 [DOI] [PubMed] [Google Scholar]
  17. Tsiamas P. et al. , “A modification of flattening filter free linac for IMRT,” Med. Phys. 38(5), 2342–2352 (2011). 10.1118/1.3571419 [DOI] [PubMed] [Google Scholar]
  18. Leung M. K. K., Chow J. C. L., Chithrani D. B., Lee M. J. G., Oms B., and Jaffray D. A., “Irradiation of gold nanoparticles by x-rays: Monte Carlo simulation of dose enhancements and the spatial properties of the secondary electrons production,” Med. Phys. 38(2), 624–631 (2011). 10.1118/1.3539623 [DOI] [PubMed] [Google Scholar]
  19. Van den Heuvel F., Locquet J. P., and Nuyts S., “Beam energy considerations for gold nano-particle enhanced radiation treatment,” Phys. Med. Biol. 55(16), 4509–4520 (2010). 10.1088/0031-9155/55/16/S06 [DOI] [PubMed] [Google Scholar]
  20. Chithrani D. B. et al. , “Gold nanoparticles as radiation sensitizers in cancer therapy,” Radiat. Res. 173(6), 719–728 (2010). 10.1667/RR1984.1 [DOI] [PubMed] [Google Scholar]
  21. Jain S. et al. , “Cell-specific radiosensitization by gold nanoparticles at megavoltage radiation energies,” Int. J. Radiat. Oncol., Biol., Phys. 79(2), 531–539 (2011). 10.1016/j.ijrobp.2010.08.044 [DOI] [PMC free article] [PubMed] [Google Scholar]
  22. Berbeco R. I. et al. , “DNA damage enhancement from gold nanoparticles for clinical MV photon beams,” Radiat. Res. 178(6), 604–608 (2012). 10.1667/RR3001.1 [DOI] [PMC free article] [PubMed] [Google Scholar]

Articles from Medical Physics are provided here courtesy of American Association of Physicists in Medicine

RESOURCES