Abstract
The aim of this study was to assess the relationship between power variables in the vertical jump and full squat with the sprint performance in soccer players. Fourteen under-21 soccer players were evaluated in two testing sessions separated by 7 days. In the first testing session, vertical jump height in countermovement was assessed, and power output for both loaded countermovement jump (CMJL) and full squat (FS) exercises in two progressive load tests. The second testing session included sprinting at 10, 20, and 30m (T10, T20, T30, T10–20, T10–30, T20–30). Power variables obtained in the loaded vertical jump with 20kg and full squat exercise with 70kg showed significant relationships with all split times (r=−0.56/–0.79; p≤ 0.01/0.01). The results suggest that power produced either with vertical jump or full squat exercises is an important factor to explain short sprint performance in soccer players. These findings might suggest that certain levels of neuromuscular activation are more related with sprint performance reflecting the greater suitability of loads against others for the improvement of short sprint ability in under-21 soccer players.
Keywords: strength, physical capacity, vertical jump, full squat, team sports
Introduction
Soccer is the world’s most popular sports game. Because of its popularity, many studies have been conducted in an attempt to understand the fundamental skills required by the soccer player (Wisloff, 1998; Cometti, 2001; Wisloff, 2004; Chelly, 2009; Meylan and Malatesta, 2009; Mújica, 2009; Requena, 2009). Unfortunately, the scientific understanding of this issue remains to lag behind its practice, with most participants acquiring skills through individual experience rather than research-based instruction.
Many sports depend heavily upon muscular strength and power especially at competition level (Baker, 2001; Haff, 2001), soccer being no exception (Wisloff, 2004). During a soccer match, lower body power is important for executing different activities as stopping and changing running speed as well as direction (Wisloff, 1998; Hoff and Helgerud, 2004; Thorlund, 2009). Although power performance has been studied in soccer (Wisloff, 1998; Wisloff, 2004), the relationships between lower body muscular power and linear sprint in soccer players have not been of primary researchers’ interest. Additionally, mostly isokinetic or isoinertial dynamometry has been used to elucidate the associations between strength/power measures and sprinting performance (Sleivert and Taingahue, 2004). Several studies have examined the possible associations between sprint ability and distinct strength and power measures in isoinertial exercises (Young, 1995; Wisloff, 1998; Baker and Nance, 1999; Henessy and Kilty, 2001; Sleivert and Taingahue, 2004; Cronin and Hansen, 2005; Marques and González-Badillo, 2006; Harris, 2008a; Requena, 2009; Habibi, 2010). In terms of lower body exercises, squat and squat vertical jump movements have been systematically used in order to explain sprint performance (Wisloff, 1998; McBride, 2002; Sleivert and Taingahue, 2004; Wisloff, 2004; Cronin and Hansen, 2005, Harris, 2008a; Harris, 2008b; González-Badillo and Marques, 2010), but determining the exact associations between these exercises and short sprinting ability has proved elusive. Indeed, for many sports activities, such as tennis, squash, and basketball, the athletes never attain maximum velocity during sprinting. Thus, the velocity over the first steps (first-step quickness) and the ability to rapidly increase velocity (acceleration) would be considered of greater importance to successful performance (Sleivert and Taingahue, 2004; Dellal, 2011). During a soccer match, sprints most frequently occur over very short distances while standing, stopping and changing the direction of movement (Di Salvo, 2009; Bradley, 2009; 2010). Thus, the acceleration phase is of major importance to soccer players. Moreover, little attention has been given to develop or refine new methods to improve soccer specific acceleration. It is commonly accepted that squatting and jumping are two important exercises to improve lower body strength, power and speed (Wisloff, 1998; Sleivert and Taingahue, 2004; Harris, 2008a; Requena, 2009). Previous investigations have shown significant associations between these two exercises and short sprint velocity (Sleivert and Taingahue, 2004; Harris, 2008a). However, the results of literature relating isoinertial assessment and sprint performance in team sports have been contradictory. Cronin and Hansen (2005) failed to find any relationship between sprint time and measurements of maximal strength, but reported weak correlations (r=−0.43 to −0.66) between vertical jump performance with 5, 10, and 30-m sprint times in professional rugby players (23.2 ± 3.3 years). In contrast, others showed moderate to strong relationships between squat performance and 10-m sprint times (r= 0.94), 30-m sprint times (r= 0.71), and jumping height (r= 0.78) (Wisloff, 1998). These results illustrate the difficulty in identifying how performances in various field tests can be related to one another.
Exercise intensity is acknowledged as an important variable related to changes in strength (ACSM Position stand, 2009) and has been commonly identified with relative load (% of one maximum repetition: 1RM) or with the maximum number of repetitions that can be performed with a given submaximal weight (XRM). However, to our best knowledge, no study prior to ours has examined the relationships between strength parameters with submaximal and absolute loads, without the evaluation of 1RM or XRM, and short sprint performance in soccer players. The understanding of these relationships may offer greater insight into the underlying determinants of short sprint performance and offers valuable information to improve the training process in soccer players.
Therefore, the purpose of this study was to assess the relationship between power variables in the vertical jump, full squat and sprinting performance in U-21 soccer players. It was hypothesized that the power for both exercises would be significantly related to sprint acceleration, but loaded vertical jump power would show a stronger relationship to sprint performance than traditional full squat power because it was a more sprint specific exercise.
Material and Methods
Study design
The present study used a cross-sectional experimental design to examine the relationship between muscle power and sprinting variables in a group of amateur (U-21) soccer players. Subjects were acquainted with all test procedures four weeks before the measurements were applied. All testing was completed at the end of a resistance-training period, in the middle of the competitive phase. This entailed a resistance-training program twice per week. The program included the full squat, the countermovement jump, and sprinting exercises. All the soccer players were well conditioned and familiar with all the testing exercises, which they had been performing regularly as part of training sessions.
Subjects
Fourteen U21 soccer players (age 20.14 ± 0.4yr, body mass 75.5 ± 7.7 kg, body height 1.79 ± 0.10m) participated in the study. All participants belonged to the same sports club. Before commencing the experiment all subjects underwent a physical examination and were cleared of any medical disorders that might limit full participation in the study. The study was approved by the Research Ethics Committee. The subjects received information about the characteristics and goals of the study, the procedures, free participations, possibility of quitting at any moment, and confidentiality of data. Written informed consent was obtained from the subjects before beginning the study.
Procedures
Participants were familiar with the testing procedures since they performed the exercises as part of their normal training routine (one or two weekly sessions). Following a standardized warm-up the subjects performed countermovement jumps, full squat, and sprinting exercises. Measurements were assessed in two sessions separated by 7 days amongst all participants. The first testing session included only countermovement jumps and full squats, whereas the second session accessed the sprint performance. During the execution of these tests, the players were verbally encouraged to give their maximal effort.
Testing
Power was measured through the following tests: countermovement jump (CMJ), and both CMJ and full squat with external load on the Smith machine (Fitness Line Peroga, Murcia, Spain). The vertical jump height was measured using a trigonometric carpet (Ergo Jump Bosco System, S. Rufina di Cittaducale, RI, Italy). Subjects began from a standing position, performed a crouching action followed immediately by a jump for maximal height. Each subject completed five attempts. Two minutes of rest for complete recovery was given between jumps. The hands were on the hips throughout the entire jump. The best and the worst jumps were eliminated from the data, and the average of the other three jumps was recorded. The CMJ had an intraclass correlation coefficient (ICC) of 0.95 (0.92–0.97), and a coefficient of variation (CV) of 5.8%.
Subsequently, maximal power was assessed for loaded CMJ and full squat exercises. Power measurement for both exercises was accessed in a Smith Machine. The bar of the Smith Machine had a linear transducer attached (Globus Real Power, Italy). The rotary encoder of the linear transducer recorded the position and direction of the bar to within an accuracy of 0.0002 m. in order to calculate different variables for each repetition of the CMJ and full squat. Only the concentric phase of the movement was taken for further analysis. Concentric movement was defined from the moment following the end of the eccentric phase until maximal positive velocity was achieved. The CMJ weighted test began with a load of 20 kg (CMJ20), and the weight was increased with 10 kg increments. The test ended when CMJ height was less than 20 cm. This height was used as reference because a jump lower than 20 cm progressively decreased the reliability of the jump (Vitaasalo, 1985) and it increases the risk of injury. Four minutes of rest were provided between each attempt to minimize the likelihood of fatigue. The best attempt for peak power with each load was recorded for later analysis. The CV of variation for test-retest reliability for CMJ was 4.3% and less. In addition, the CMJ with additional weights showed an ICC of 0.93 or more.
The progressive test of full squats with external loads was performed on the Smith machine. For this test, each soccer player descended until the top of the thigh was below parallel with the floor. The number of full squat repetitions for a particular load was determined according to the velocity of the first repetition. Three repetitions were performed when the subject displaced the bar with an average velocity ≥ 1m·s−1. In contrast, only two repetitions were executed when the average velocity was <1m·s−1. The increase in the load was done in 10 kg increments. Four minutes of recovery were taken between each set. The test ended for each subject when the average velocity of movement was ≤ 0.7 m·s−1 (19). This velocity was chosen as reference because in a pilot study it was observed that maximum average power was attained at higher velocities. The highest peak power and mean power of the repetitions done with external loads and the load achieved by each player were used for later analysis (CV: 2.9–4%; ICC: 0.92–0.94).
Finally, subjects were required to perform three maximum effort sprints of 30 metres. Times at 0–10m (T10), 0–20m (T20), 0–30 (T30), 10–20m (T10–20), 10–30m (T10–30), and 20–30m (T20–30) were recorded using Brower equipment (Wireless Sprint System, USA). Subjects performed trial sprints separated by 3 minutes of rest. Only the best attempt was considered. The sprints reported ICCs of 0.92–0.99 and CVs of 1.2–2.6%.
Statistical analyses
To assess the relationship between sprint performances and jump and full squat performances Pearson correlation and univariate analysis of variance between the different variables were used. Post hoc Bonferroni comparison was used to locate significant differences. Intraclass correlation coefficient (ICC) was used to determine between-subject reliability of jumping tests. Within-subject variation for all tests was determined by calculating the coefficient of variation (CV) as outlined by Hopkins (2000). Statistical significance was accepted at p ≤0.05 for all analysis.
Results
Means values of the countermovement jump and 30m sprint performances are presented in Table 1.
Table 1.
Means ± SD of counter movement jump and 30m sprint performances in all tested subjects.
| Variables | Values |
|---|---|
| Counter movement jump (cm) | 38.34 ± 4.44 |
| Time at 10 m (s) | 1.92 ± 0.06 |
| Time at 20 m (s) | 3.22 ± 0.09 |
| Time at 30 m (s) | 4.43 ± 0.14 |
| Split time between 10 and 20 m (s) | 1.28 ± 0.04 |
| Split time between 10 and 30 m (s) | 2.50 ± 0.05 |
| Split time between 20 and 30 m (s) | 1.20 ± 0.05 |
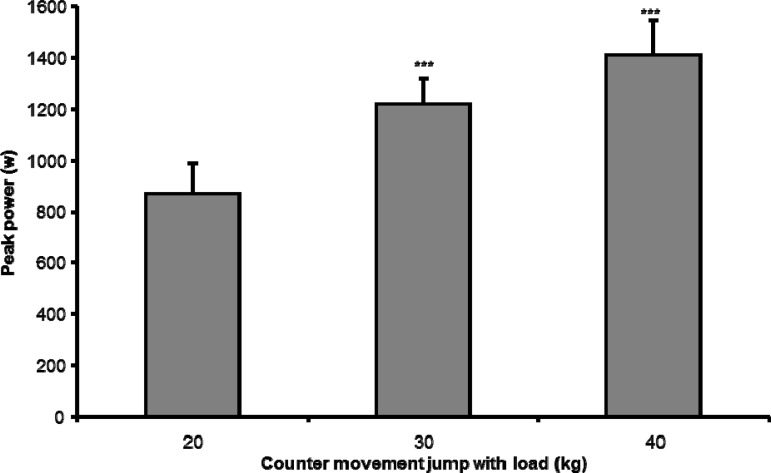
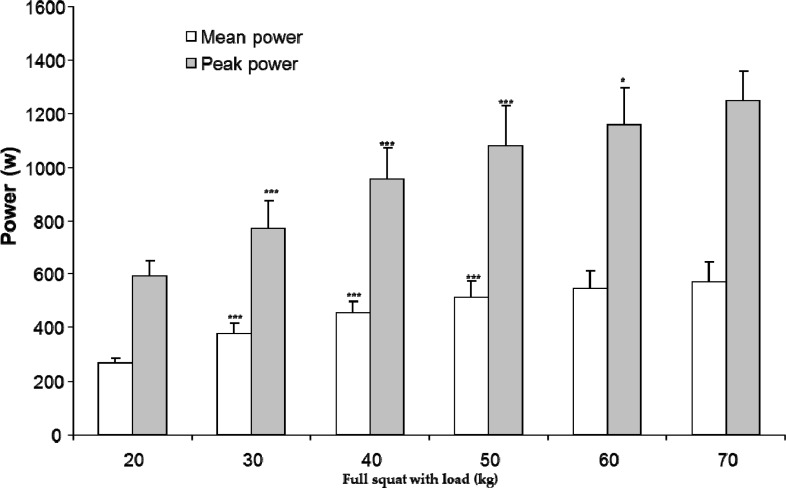
Peak and mean power output showed significant differences with the increasing load in the countermovement jump and full squat (Figure 1 and 2) (i.e. the mean and peak power increased with increasing load).
Figure 1.
Average peak power and SD at the counter movement jumps with different weights (Significant difference in peak power output with previous load= *p ≤0.05; **p ≤0.01; ***p ≤0.001).
Figure 2.
Average mean and peak power and SD at the full squats with different weights. (Significant difference in power output with previous load= *p ≤0.05; ***p ≤0.001).
The present results showed significant correlations between the countermovement jumping height and the sprint times at 20 and 30m. Peak power in CMJ test with an external load of 20kg correlated significantly with sprint times at every distance (Table 2). Finally, the last meters of the sprint (T10–30 and T20–30) were associated with peak power at different weighted CMJ. No correlations were found between the CMJ with 20 and 30kg load and the times at 10 and 20m.
Table 2.
Correlations between height in countermovement jump and peak power output in countermovement jumps with different weights, and the different sprint times.
| CMJ | CMJ20 | CMJ30 | CMJ40 | |
|---|---|---|---|---|
| Height | Peak power | |||
| Time at 10 m | −.46 | −.56* | −.27 | −.44 |
| Time at 20 m | −.54* | −.58* | −.37 | −.46 |
| Time at 30 m | −.55* | −.69* | −.58 | −.61* |
| Split time 10–20 m | −.49 | −.57* | −.62* | −.51 |
| Split time 10–30 m | −.52 | −.75† | −.79† | −.72* |
| Split time 20–30 m | −.48 | −.75† | −.65* | −.72* |
CMJ = countermovement jump; CMJL= counter movement jump with load. Significance:
p≤0.05;
p≤0.01.
Regarding the full squat, maximal average power was obtained with 62 ± 9kg, equivalent to 82% of players body mass. Furthermore, maximal peak power was attained with 65 ±12kg (86% of body mass). Only the peak power output with 20 and 30kg showed significant correlations with different sprint times (Table 3). The mean power in full squat with an external load of 70kg showed significant correlations with the majority of sprint times (Table 3). A significant correlation was also found between the time at 10m and the average power during the full squat with 30 and 40 kg external load. No other significant correlations were observed between sprint times and the power parameters during the full squat with different external loads (Table 3)
Table 3.
Correlations between peak power and mean power of full squats with different weights and the different sprint times
| Full squat20 | Full squat30 | Full squat40 | Full squat50 | Full squat60 | Full squat70 | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| PP | MP | PP | MP | PP | MP | PP | MP | PP | MP | PP | MP | |
| Time at 10 m | −.59 | −.50 | .47 | −.62* | −.30 | −.60* | −.21 | −.44 | −.31 | −.45 | −.21 | −.63* |
| Time at 20 m | −.44 | −.38 | −.40 | −.44 | −.29 | −.50 | −.21 | −.37 | −.37 | −.49 | −.08 | −.73† |
| Time at 30 m | −.58 | −.48 | −.5 | −.50 | −.36 | −.55 | −.28 | −.43 | −.37 | −.51 | −.03 | −.78† |
| Split time 10–20 m | −.44 | −.33 | −.18 | −.18 | −.24 | −.28 | −.08 | −.20 | −.21 | −.42 | −.05 | −.62* |
| Split time 10–30 m | −.63* | −.50 | −.48 | −.42 | −.33 | −.48 | −.28 | −.39 | −.29 | −.50 | −.14 | −.76* |
| Split time 20–30 m | −.64* | −.56* | −.58* | −.53 | −.34 | −.55 | −.35 | −.45 | −.30 | −.49 | −.07 | −.71* |
Full squatL= full squat with load; PP= peak power, MP=mean power Significance:
p≤0.05;
p≤0.01
Discussion
The purpose of this study was to investigate the relationships between short sprint performance and power parameters during vertical jump and full squat exercises in under-21 soccer players. The results showed significant correlations between all sprint times and peak power in loaded countermovement jump with 20kg, and between the loaded countermovement jumps and split times from 10 to 30m sprint. The average power with the full squat with 70kg also showed significant positive correlations with the sprint times.
The CMJ height has been greatly used to access lower body power in soccer players (Wisloff, 1998; Helgerud, 2001; Núñez, 2008; Ronnestad, 2008). Nevertheless, to our knowledge, only two previous studies (Gorostiaga, 2004; López-Segovia, 2010) have used loaded countermovement jump (CMJL) exercise for testing lower limb power in this population. Unfortunately, these authors (Gorostiaga, 2004; López-Segovia, 2010) did not include sprint evaluations in their studies.
Different factors such as lower reliability of testing at very short distances, the static start position in the sprint test and the location of the first photoelectric cells (30 cm behind start in these two studies) could explain the lack relationship reported between CMJ and time at 10m. Although, the relationship obtained between the vertical jump and 30m sprint time (present study: r= −0.55; p<0.05 vs. r= −0.60; p<0.01) was similar to the study of Wisloff (2004), the relationships observed between the vertical jump and last running meters are consistent with the results perceived with loaded jump, given a similarity of muscle action in both types of jumps. Significant association between peak power during loaded CMJ and later stages of the sprint (r=−0.544 to −0.611; p≤0.05) were obtained. The T10–30 and T20–30 were significantly related with peak power observed in the CMJL exercise with 20, 30, and 40kg external load. Cronin and Hansen (2005) observed similar results in professional rugby players between loaded (30kg) vertical jump height and 5m, 10m, and 15m sprint times. The higher relationships (R2= 41–62%) observed in the present study were perceived with the longer distances rather than the initial run. As running velocity approaches maximum, those strength measures that require force to be produced at high velocities have been reported to be significantly related to sprint performance (Wilson, 1995; Young, 1995; Nesser, 1996). Wilson (1995) reported a significant relationship between force at 30 ms in a concentric squat jump and 30m sprint time (r= 0.62). Nesser (1996) claimed significant correlations between 40m sprint time and peak isokinetic torque at a velocity of 7.85 rad/s for the hip and knee extensors and knee flexors (r= 0.54 to 0.61). We agree with the assertion that results show a slight tendency of increased relationships such as velocity and distance increased (Table 2).
Moreover, data showed that power output during the vertical jump with 20kg best explained sprint performance. This parameter was also significantly correlated with all split speed measurements, including the first sprint stages. Although correlations do not signify causation, CMJ training with light loads could be important to improve sprint performance in soccer player’s under-21. McBride (2002) also observed that training with light loads (squat jump at 30% of 1RM vs. 80% of 1RM) was more effective to velocity improvement. Further, this type of strength training can reduced the risk of injuries compared to heavy resistance training regimens. Finally, this training method can be used with athletes of short resistance training experience as has beeen shown favorably in previous studies with soccer players (Gorostiaga 2004; López-Segovia 2010).
Only one previous study has investigated the muscular power output in the squat in soccer players (Requena, 2009). Yet, no studies have analysed the power output in the full squat exercise in this population. Maximal peak power output reported in the present study was 1181 ± 188W, with an external load equivalent to the 86% of body weight. This result was very similar compared to the values (1149W) claimed by Requena (2009) among semi-professional soccer players. However, these researchers used the traditional half squat exercise and not the full squat movement. Although similar peak power outputs (1181 vs. 1149) were reported between theses two studies, ours was equivalent to 86% of players body weight, while the experiment conduced with semi-professional soccer players was obtained with 112.5% of BW. These differences could be explained by a different range of motion of these two exercises (half vs. full squat), by different measuring equipment, and by distinct kinematic parameters. Thereby a load equivalent to 55% of 1RM in the half squat represents in a full squat approximately 30–45% of the subjects maximum capability (Baker 2001). It is also possible that the differences could be linked to the players experience in resistance-training programs. Baker (2001) observed that professional rugby players produced maximal peak power values with a load equivalent to 104% bodyweight, while in a group of college rugby players the percentage obtained was lower (92%), close to our results. Furthermore, both studies (ours and of Baker, 2001) were conduced with participants who had limited resistance-training experience. Nevertheless, these results seem to indicate that power output could be a determinant factor to identity full squat performance at different levels.
Like in loaded countermovement jump, the power output obtained in full squat with lighter loads was more related as running velocity approaches maximum (Table 3). These relationships are in accordance with the findings of Murphy (2003) showing that at initial acceleration, the application of reactive strength is longer and the stretch shortening cycle is slower that in the last meters of the sprint. The major relationships observed in the last sprint stages may also indicate individual ability to produce muscle force at higher velocities, and also a better neuromuscular activation during the stretch shortening cycle.
The current study showed that the T10 correlated significantly with the average power during the full squat with 30 and 40kg (r= −0.591, −0.621, and r=−0.602 respectively; p≤0.05). The relationships found between maximal strength variables (1RM or XRM) and initial run capacity is not well establish. In fact, only Wisloff (2004) reported significant correlations (r= 0.94–0.71, p≤0.01–0.001) between maximal strength and sprint times at 10m, and 30m. Other studies showed small or no significant correlations between lower body maximal strength and sprint performance (Baker and Nance, 1999; Cronin and Hansen, 2005; Harris, 2010). More recently, however, López-Segovia (2010) described significant correlations (r= 0.53–0.64, p≤0.05) between the changes in short sprint performance and the changes in the bar velocity and displacement in the full squat exercise after four months of resistance training combining soccer training and weekly competitions. It shows the importance of combined speed-strength velocity in full squat exercise as specific neural adaptations in order to improve sprint performance (McBride, 2002). In sport science, many experiments, set up to enhance the performance of the athlete, are based on a force-velocity relationship. The relationships between power and velocity and/or force are also a way of examining the force-velocity relationship from another point of view.
Interestingly, the present data also showed significant relationships between average maximal power during the full squat with 70kg and sprint times (r=−0.62 to −0.78; p≤0.05). This load was equivalent to 93% of body mass and very similar to the result reported by Requena (2009) with semi-professional soccer players. These researchers indicated that the load representative to 100% of body mass was significantly related to 15m sprint time (r=−0.62; p≤−0.01). These relationships might suggest that certain levels of neuromuscular activation, assessed by mean power output and produced versus loads very close to body weight and maximal power, are more related with sprint performance reflecting the greater suitability of loads against others for the improvement of this quality. Of great interest was that power output produced with this specific load (FS70) it’s near to maximal average power, and based in theoretical principles training with this range of loads may cause neural and muscle fiber adaptations related to sprint action (Wilson, 1993; Baker, 2001). Although the study’s limitations do not allow to verify the following possibility, the constant relationship found between FS70 and all split of sprint could be due to similar motor-unit recruitment and synchronization to type II muscle fibers, which are important neural factors to explosive movement production (Moritani, 1993).
In summary, power parameters obtained in a loaded jump with 20kg and full squat exercise with 70kg demonstrated significant relationships with short sprint performance in under-21 soccer players. Therefore, the results may suggest that power produced either with vertical jump or full squat exercises is an important parameter to explain short sprint performance in soccer players.
Although these relationships do not imply the causes, the neuromuscular adaptations provoked by light loads in exercises such as the squat and loaded vertical jump might have a significant effect on sprint ability, as previously documented (McBride, 2002). Additionally, using lighter weights diminishes the risk of injury.
Practical applications
Power produced either with vertical jump or full squat exercises is an important parameter to explain short sprint performance in soccer players. This information could be useful for practitioners to monitor their strength training programs in order to improve sprint performance in under-21 soccer players. Future research with resistance training with light loads is required to elucidate the efficiency of this type of training focus on the importance of shortening capacity of the extensor muscles at higher velocities, against the traditional strength training focus on the high magnitude of load lifted.
References
- ACSM. American College of Sports Medicine position stand Progression models in resistance training for healthy adults. Med Sci Sports Exercise. 2009;41:687–708. doi: 10.1249/MSS.0b013e3181915670. [DOI] [PubMed] [Google Scholar]
- Baker D. Comparison of upper-body strength and power between professional and college-aged rugby players. J Strength Cond Res. 2001;15:30–35. [PubMed] [Google Scholar]
- Baker D, Nance S. The relationship between running speed and measures of strength and power in professional rugby league players. J Strength Cond Res. 1999;13:230–235. [Google Scholar]
- Baker D, Nance S, Moore M. The load that maximizes the average mechanical power output during jump squats in power-trained athletes. J Strength Cond Res. 2001;15:92–97. [PubMed] [Google Scholar]
- Bangsbo J. The physiology of soccer: with special reference to intense Intermittent exercise. Acta Physiol Scand. 1994;15(Suppl):1–156. [PubMed] [Google Scholar]
- Bradley PS, Di Mascio M, Peart D, Olsen P, Sheldon B. High-intensity activity profiles of elite soccer players at different performance levels. J Strength Cond Res. 2010;24:2343–2351. doi: 10.1519/JSC.0b013e3181aeb1b3. [DOI] [PubMed] [Google Scholar]
- Bradley PS, Sheldon W, Wooster B, Olsen P, Boanas P, Krustrup P. High-intensity running in English FA Premier League soccer matches. J Strength Cond Res. 2009;15:159–168. doi: 10.1080/02640410802512775. [DOI] [PubMed] [Google Scholar]
- Chelly MS, Fathloun M, Cherif N, Ben Amar M, Tabka Z, Van Praagh E. Effects of a back squat training program on leg power, jump, and sprint performances in junior soccer players. J Strength Cond Res. 2009;23:2241–2249. doi: 10.1519/JSC.0b013e3181b86c40. [DOI] [PubMed] [Google Scholar]
- Cometti G, Maffiuletti NA, Pousson M, Chatard JC, Maffulli N. Isokinetic strength and anaerobic power of elite, subelite and amateur French soccer players. Int J Sports Med. 2001;22:45–51. doi: 10.1055/s-2001-11331. [DOI] [PubMed] [Google Scholar]
- Cronin JB, Hansen KT. Strength and power predictors of sport speed. J Strength Cond Res. 2005;19:349–357. doi: 10.1519/14323.1. [DOI] [PubMed] [Google Scholar]
- Dellal A, Chamari C, Wong DP, Ahmaidi S, Keller D, Barros MLR, Bisciotti GN, Carling C. Comparison of physical and technical performance in European professional soccer match-play: The FA Premier League and La LIGA. Eur J Sport Sci. 2011;11:51–59. [Google Scholar]
- Di Salvo V, Gregson W, Atkinson G, Tordoff P, Drust B. Analysis of high intensity activity in Premier League Soccer. Int J Sports Med. 2009;30:205–212. doi: 10.1055/s-0028-1105950. [DOI] [PubMed] [Google Scholar]
- González-Badillo JJ, Marques MC. Relationship between kinematic factors and countermovement jump height in trained track and field athletes. J Strength Cond Res. 2010;24:3443–3447. doi: 10.1519/JSC.0b013e3181bac37d. [DOI] [PubMed] [Google Scholar]
- Gorostiaga EM, Izquierdo M, Ruesta M, Iribarren J, González-Badillo JJ, Ibáñez J. Strength training effects on physical performance and serum hormones in young soccer players. Eur J Appl Physiol. 2004;91:698–707. doi: 10.1007/s00421-003-1032-y. [DOI] [PubMed] [Google Scholar]
- Habibi W, Shabani M, Rahimi E, Fatemi R, Najafi A, Analoei H, Hosseini M. Relationship between Jump Test Results and Acceleration Phase of Sprint Performance in National and Regional 100 m Sprinters. J Human Kinetics. 2010;23:29–35. [Google Scholar]
- Haff GG, Withley A, Potteiger JA. A brief review: Explosive exercises and sports performance. Strength Cond J. 2001;23:13–20. [Google Scholar]
- Harris NK, Cronin JB, Hopkins WG, Hansen KT. Inter-relationships between machine squat-jump strength, force, power and 10 m sprint times in trained sportsmen. J Sports Med Phys Fitness. 2010;50:37–42. [PubMed] [Google Scholar]
- Harris NK, Cronin JB, Hopkins WG, Hansen KT. Relationship between sprint times and the strength/power outputs of a machine squat jump. J Strength Cond Res. 2008a;22:691–698. doi: 10.1519/JSC.0b013e31816d8d80. [DOI] [PubMed] [Google Scholar]
- Harris NK, Cronin JB, Hopkins WG, Hansen KT. Squat jump training at maximal power loads vs. heavy loads: effect on sprint ability. J Strength Cond Res. 2008b;22:1742–1749. doi: 10.1519/JSC.0b013e318187458a. [DOI] [PubMed] [Google Scholar]
- Helgerud J, Engen LC, Wisloff U, Hoff J. Aerobic endurance training improves soccer performance. Med Sci Sports Exer. 2001;33:1925–1931. doi: 10.1097/00005768-200111000-00019. [DOI] [PubMed] [Google Scholar]
- Henessy L, Kilty J. Relationship of the stretch-shortening cycle to sprint performance in trained female athletes. J Strength Cond Res. 2001;15:326–331. [PubMed] [Google Scholar]
- Hoff J, Helgerud J. Endurance and strength training for soccer players: Physiological considerations. Sports Med. 2004;34:165–180. doi: 10.2165/00007256-200434030-00003. [DOI] [PubMed] [Google Scholar]
- Hopkins WG. Measures of reliability in sports medicine and science. Sports Med. 2000;30:1–15. doi: 10.2165/00007256-200030010-00001. [DOI] [PubMed] [Google Scholar]
- López-Segovia M, Palao JM, González-Badillo JJ. Effect of four months of training on aerobic power, sterngth, and acceleration in two under-19 soccer teams. J Strength Cond Res. 2010;24:2705–2714. doi: 10.1519/JSC.0b013e3181cc237d. [DOI] [PubMed] [Google Scholar]
- Marques MC, González-Badillo JJ. In-season Resistance Training and Detraining in Professional Team Handball Players. J Strength Cond Res. 2006;20:563–571. doi: 10.1519/R-17365.1. [DOI] [PubMed] [Google Scholar]
- McBride JM, Tripplett-McBride T, Davie A, Newton RU. The effect of heavy vs. ligth load jump squats on the development of strength, power, and speed. J Strength Cond Res. 2002;16:75–82. [PubMed] [Google Scholar]
- Meylan C, Malatesta D. Effects of in-season plyometric training within soccer pratice on explosive actions of young players. J Strength Cond Res. 2009;23:2605–2613. doi: 10.1519/JSC.0b013e3181b1f330. [DOI] [PubMed] [Google Scholar]
- Moritani T. Neuromuscular adaptations during the acquisition of muscle strngth, power and motor task. J Biomech. 1993;26:95–107. doi: 10.1016/0021-9290(93)90082-p. [DOI] [PubMed] [Google Scholar]
- Mújica I, Santiesteban J, Castagna C. In-season effect of short-term sprint and power training programs on elite junior soccer players. J Strength Cond Res. 2009;23:2581–2587. doi: 10.1519/JSC.0b013e3181bc1aac. [DOI] [PubMed] [Google Scholar]
- Murphy A, Lockie RG, Coutts AJ. Kinematic determinants of early acceleration in field sports athletes. J Sports Sci Med. 2003;2:144–150. [PMC free article] [PubMed] [Google Scholar]
- Nesser TW, Latin RW, Berg K, Prentice E. Physiological determinants of 40-meter sprint performance in young male athletes. J Strength Cond Res. 1996;10:263–267. [Google Scholar]
- Núñez VM, Da Silva-Grigoletto ME, Castillo EF, Poblador MS, Lancho JL. Effects of training exercises for the development of strength and endurance in soccer. J Strength Cond Res. 2008;22:518–524. doi: 10.1519/JSC.0b013e318163468f. [DOI] [PubMed] [Google Scholar]
- Requena B, González-Badillo JJ, Saez de Villarreal ES, Ereline J, García I, Gapeyeva H, Pääsuke M. Functional performance, maximal strength, and power characteristics in isometric and dynamic actions of lower extremities in soccer players. J Strength Cond Res. 2009;23:1391–1401. doi: 10.1519/JSC.0b013e3181a4e88e. [DOI] [PubMed] [Google Scholar]
- Rienzi E, Drust B, Reilly T, Carter JEL, Martin A. Investigation of anthtopometric and work-rate profiles of elite South American international players. J Sports Med Phys Fitness. 2000;40:162–169. [PubMed] [Google Scholar]
- Ronnestad BR, Kvamme NH, Sunde A, Raastad T. Short-term effects of strength and plyometric training on sprint and jump performance in professional soccer players. J Strength Cond Res. 2008;22:773–780. doi: 10.1519/JSC.0b013e31816a5e86. [DOI] [PubMed] [Google Scholar]
- Sleivert G, Taingahue M. The relationship between maximal kump-squat power and sprint acceleration in athletes. Eur J Appl Physiol. 2005;91:46–53. doi: 10.1007/s00421-003-0941-0. [DOI] [PubMed] [Google Scholar]
- Thorlund JB, Aagaard P, Madsen K. Rapid muscle force capacity changes after soccer match play. Int J Sports Med. 2009;30:273–278. doi: 10.1055/s-0028-1104587. [DOI] [PubMed] [Google Scholar]
- Vitaasalo JP. Measurement of force-velocity characteristics for sportmen in field conditions. In: Winter DA, Norman RW, Wells RP, editors. Biomechanics IX-A. Champaign, IL: Human Kinetics; 1985. pp. 91–95. [Google Scholar]
- Wilson GJ, Lyttle AD, Ostrowski KJ, Murphy AJ. Assessing dynamic performance: a comparison of rate of force development tests. J Strength Cond Res. 1995;9:176–181. [Google Scholar]
- Wilson GR, Newton R, Murphy A, Humphries B. The optimal training load for the development of dynamic athletic performance. Med Sci Sports Exercise. 1993;23:1279–1286. [PubMed] [Google Scholar]
- Wisloff U, Helgerud J, Hoff J. Strength and endurance of elite soccer players. Med Sci Sports Exerc. 1998;30:462–467. doi: 10.1097/00005768-199803000-00019. [DOI] [PubMed] [Google Scholar]
- Wisloff U, Castagna C, Helgerud J, Jones R, Hoff J. Strong correlation of maximal squat strength with sprint performance and vertical jump height in elite soccer players. Br J Sports Med. 2004;38:285–288. doi: 10.1136/bjsm.2002.002071. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Young W, McLean M, Ardagna J. Relationship between strength qualities and sprinting performance. J Sports Med Phys Fitness. 1995;35:13–19. [PubMed] [Google Scholar]