Eye movement sequence generation in humans: Motor or goal updating?

Abstract

Saccadic eye movements are often grouped in pre-programmed sequences. The mechanism underlying the generation of each saccade in a sequence is currently poorly understood. Broadly speaking, two alternative schemes are possible: first, after each saccade the retinotopic location of the next target could be estimated, and an appropriate saccade could be generated. We call this the goal updating hypothesis. Alternatively, multiple motor plans could be pre-computed, and they could then be updated after each movement. We call this the motor updating hypothesis. We used McLaughlin’s intra-saccadic step paradigm to artificially create a condition under which these two hypotheses make discriminable predictions. We found that in human subjects, when sequences of two saccades are planned, the motor updating hypothesis predicts the landing position of the second saccade in two-saccade sequences much better than the goal updating hypothesis. This finding suggests that the human saccadic system is capable of executing sequences of saccades to multiple targets by planning multiple motor commands, which are then updated by serial subtraction of ongoing motor output.

Introduction

Humans are endowed with high acuity vision only over a very restricted part of the retina, the fovea. The ability to quickly and accurately change the direction of gaze is thus instrumental to an effective interaction with the environment. Because of the large inertia of the head and the trunk, this is accomplished mostly through eye movements, particularly saccades.

Saccades are overwhelmingly studied in isolation, i.e., one at a time in response to external cues. Typically, while the subject is looking at a visual stimulus (fixation point), a target appears in the periphery. The subject then makes a saccadic eye movement to direct gaze to the target. Thanks to the countless experiments that have relied on this simple task, we now have a reasonably clear idea about the neural processes at play (e.g., Wurtz & Goldberg, 1989). At the input level, the location of the visual target can be described by a 2-D vector V⃗, which specifies where on the retina the image of the target falls. Since this target is recognized as behaviorally relevant (i.e., salient), its appearance does not go unnoticed, but it is instead internalized by activating a small subset of cells in several retinotopically organized neural maps. The location of the active site in these maps can be described by a 2-D vector G⃗. This vector is not a purely sensory signal, but it is also not a motor signal per se. It is used to keep track of where relevant targets are and to indicate to a motor system the desired sensory consequences of a motor act (e.g., point the fovea at the target). For simplicity, we will refer to it as the movement goal, hence the use of the letter G. Once a command to foveate the target is issued, an eye movement will be produced. This movement can also be described by a 2-D vector M⃗ (in this simplified description, we omit eye torsion).

Ideally, when a target is presented on the retina, G⃗ = V⃗. In reality, the representation of the goal is affected by the visual properties of the target, possibly by the context in which the target is presented, and can deteriorate over time. More generally, we then have that

$$\overrightarrow{G}=f_{\text{G}}(\overrightarrow{V},\mathcal{C})+{\epsilon }_{\text{G}},$$ (1)

where with we indicate all possible contextual effects, and with ε_G we indicate the goal noise. Similarly, when a goal is selected for a saccade, the movement should be appropriate to foveate the target, which would require M⃗ = G⃗. The mapping from an internalized target to a movement vector is, however, also subject to many factors, which we can again lump together in the context , and of course there is going to be some motor noise. Hence

$$\overrightarrow{M}=f_{\text{M}}(\overrightarrow{G},\mathcal{C})+{\epsilon }_{\text{M}}.$$ (2)

This focus on individual saccades generated in response to an external cue is somewhat limiting, because under more natural conditions saccadic eye movements are usually generated in sequences. Obviously, it would not be very efficient to reanalyze the entire visual scene after each eye movement to select a new target. Instead, the aforementioned retinotopic maps can, and do, keep track of multiple targets over time. Evidence suggests that programs for saccades might actually be pre-planned and retrieved from memory in packets of 2 to 4 saccades at a time (Ditterich, Eggert, & Straube, 1998; Zingale & Kowler, 1987). A large body of literature is available about how saccadic sequences are planned, for example, when scanning visual scenes or during reading (e.g., Land, 2009; Rayner, 1998). However, much less effort has been devoted to understanding how individual saccades in a planned sequence are generated. This issue is the focus of our study.

The double-saccade paradigm

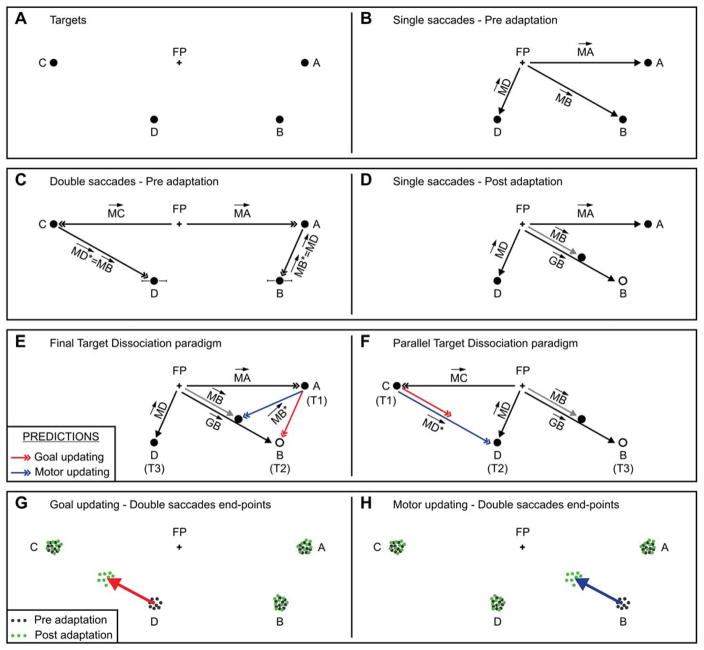
Typically, sequences of saccades are studied using the double-saccade paradigm (Figure 1A). At the beginning of each trial, the subject looks at a fixation point (FP), and two targets (T1 and T2) are presented. His/her task is to direct the eyes first at the location of T1, and then at the location of T2. Importantly, both T1 and T2 are removed before the first saccade is completed (and usually even before the first saccade starts). With this paradigm, three sets of vectors must be considered. Of course, we have the visual vectors for the two targets, which again are only present before the first saccade even starts ( $\overrightarrow{V1}$ and $\overrightarrow{V2}$). Then, we have the two motor vectors, which correspond to the amplitude and direction of the two saccades ( $\overrightarrow{M1}$ and $\overrightarrow{M{2}^{\ast }}$). Finally, we have the goal vectors, which in this case are three: $\overrightarrow{G1},\overrightarrow{G2}$, and $\overrightarrow{G{2}^{\ast }}$. To clarify, we label vectors as follows: the first letter indicates whether the vector refers to the location of a target on the retina (V), to a movement goal (G), or to a saccadic eye movement (M); the second character (either a number or a letter) identifies the target with which the vector is associated; finally, a trailing asterisk indicates that the retinotopic location of a goal was updated following a movement, or that the saccade vector was executed after looking at another target first.

Figure 1.

Experimental paradigms. (A) Traditional double-saccade paradigm. The subject initially looks at the fixation point (FP) and is then required to execute a sequence of two saccades, one to the location of T1 followed by one toward T2. Neither target is visible by the time the first saccade starts. Vectors $\overrightarrow{V1}$ and $\overrightarrow{V2}$ describe the location of the targets on the retina, before the start of the first saccade. Vectors $\overrightarrow{G1}$ and $\overrightarrow{G2}$ describe the internalized goal vectors for the two targets, before any saccade is executed. Vectors $\overrightarrow{M1}$ and $\overrightarrow{M{2}^{\ast }}$ describe the amplitude and direction of the two saccades. Vector $\overrightarrow{G{2}^{\ast }}$ is the updated goal vector $\overrightarrow{G2}$ after the first saccade has been executed. Vector $\overrightarrow{M2}$ represents the amplitude and direction of a hypothetical saccade aimed directly at T2. (B) Double-saccade paradigm modified for testing the goal and motor updating hypotheses. T3 is placed relative to FP as T2 is placed relative to T1. Other symbols as in (A). (C) If the goal and motor vectors of saccades aimed directly at the final target in the sequence are dissociated by adaptation of $\overrightarrow{M2}$ (FTD paradigm), the goal updating hypothesis predicts no change in $\overrightarrow{M{2}^{\ast }}$ (red arrow), whereas the motor updating hypothesis predicts a change (blue arrow). (D) If the vectors associated with saccades aimed at a target placed “in parallel” to the final target in the sequence are dissociated by adaptation of $\overrightarrow{M3}$ (PTD paradigm), the opposite pattern is expected.

Experiments can be designed to shed light on the neural processes that convert the initial goal vectors $\overrightarrow{G1}$ and $\overrightarrow{G2}$ into the movements vectors $\overrightarrow{M1}$ and $\overrightarrow{M{2}^{\ast }}$. The generation of the first movement is actually quite straightforward, since it follows Equation 2; what is considerably more interesting is how the second saccade (i.e., $\overrightarrow{M{2}^{\ast }}$) is generated. There are three possible alternatives, which we now describe.

The ballistic programming hypothesis

One possibility is that the difference between the two initial goal vectors is computed, yielding an estimate of the location of the second goal relative to the first one (in retinal coordinates):

$$\overrightarrow{G{2}^{\ast }}=\overrightarrow{G2}-\overrightarrow{G1}.$$ (3)

Ignoring for the time being the influence of context, once the first saccade $\overrightarrow{M1}=f_{\text{M}}(\overrightarrow{G1})+{\epsilon }_{\text{M}}$ has been executed, a second saccade could then be generated based on $\overrightarrow{G{2}^{\ast }}$:

$$\overrightarrow{M{2}^{\ast }}=f_{\text{M}}(\overrightarrow{G{2}^{\ast }})+{\epsilon }_{\text{M}}=f_{\text{M}}(\overrightarrow{G2}-\overrightarrow{G1})+{\epsilon }_{\text{M}}.$$ (4)

Note that under Equation 4 the second saccade in a sequence would be independent of the first saccadic vector $\overrightarrow{M1}$. We call this the ballistic programming hypothesis, since under it both saccade vectors could be programmed in advance and executed ballistically.

The goal updating hypothesis

Alternatively, after the first saccade has been executed the brain could update, based on the actual movement vector, the second goal vector. Equation 3 would thus be replaced by

$$\overrightarrow{G{2}_{\text{m}}^{\ast }}=\overrightarrow{G2}-\overrightarrow{M1},$$ (5)

where the “m” indicates that this internal estimate is based on motor-related information, not just initial goal information as in Equation 3. In the context of the double-saccade experiment, Equation 5 suggests that the second saccade could be generated based on $\overrightarrow{G{2}_{\text{m}}^{\ast }}$, i.e.,

$$\overrightarrow{M{2}^{\ast }}=f_{\text{M}}(\overrightarrow{G{2}_{\text{m}}^{\ast }})+{\epsilon }_{\text{M}}=f_{\text{M}}(\overrightarrow{G2}-\overrightarrow{M1})+{\epsilon }_{\text{M}}.$$ (6)

We call this the goal updating hypothesis, since under it the second saccade would be based on an estimate of the goal location in retinotopic coordinates, updated following the first movement.

The motor updating hypothesis

Although rarely considered, there is a third alternative. The saccadic vector for the second saccade could be computed by subtracting the motor vector $\overrightarrow{M1}$ from the motor vector $\overrightarrow{M2}$ that would be needed to move the eyes directly from FP to T2 (Figure 1A):

$$\overrightarrow{M{2}^{\ast }}=\overline{M2}-\overrightarrow{M1}.$$ (7)

Equation 7 posits that, when a sequence of saccades is planned, the system internally converts the two goals into the movement vectors appropriate to look directly at each target ( $\overrightarrow{M1}$ for T1 and $\overrightarrow{M2}$ for T2 in our case). Once the first movement is executed, the motor plan for the second one is updated directly, bypassing the update in goal space. We call this the motor updating hypothesis, since under it saccade generation would rely only on the storage and updating of motor information. Of course, this would not imply that an update in goal space could not occur, but simply that it would not be used to compute the saccadic vector $\overrightarrow{M{2}^{\ast }}$.

Note that from a purely theoretical point of view, this last hypothesis should be preferred. The brain supplies innervation signals to the eye muscles, and they in turn generate forces, which are transmitted by the tendons, resulting in torques being applied to the eyeball. Since forces and torques are commutative entities, it follows that to point the eye at a target, say T2, the innervation supplied to the eye muscles must always be the same, regardless of how the eye ended up pointing to T2. Hence, physics dictates that $\overrightarrow{M1}+\overrightarrow{M{2}^{\ast }}$ should always be equal to $\overrightarrow{M2}$.

Testing the hypotheses

Several experiments bearing on the ballistic programming hypothesis have been carried out already (Bock, Goltz, Bélanger, & Steinbach, 1995; Ditterich et al., 1998; Doré-Mazars, Vergilino-Perez, Collins, Bohacova, & Beauvillain, 2006; Joiner, Fitzgibbon, & Wurtz, 2010; Munuera, Morel, Duhamel, & Deneve, 2009; Tanaka, 2003). Taken together, they demonstrated that, both in humans and monkeys, the mechanism that generates the second saccadic vector $\overrightarrow{M{2}^{\ast }}$ uses information about the first saccadic vector $\overrightarrow{M1}$ and does not simply rely on the original goal vectors. Note, however, that this only applies to saccades aimed at individual targets (so-called targeted saccades), which are the focus of our study. In contrast, saccades used to explore a single object appear to be programmed ballistically (Doré-Mazars et al., 2006).

To discriminate between the goal (Equation 6) and motor (Equation 7) updating hypotheses, two tests can be applied. Once again, referring to a vector diagram simplifies the description (Figure 1B). This diagram extends the one in Figure 1A by adding a new target, which we call T3. This target is located relative to the FP as T2 is located relative to T1, i.e., $\overrightarrow{V3}=\overrightarrow{V2}-\overrightarrow{V1}$. Assuming that the retinotopic maps are properly calibrated, and ignoring the noise terms, it follows that $\overrightarrow{G3}=\overrightarrow{G2}-\overrightarrow{G1}$.

The first test (Figure 1C) can be carried out by adaptively changing the metrics of saccades aimed directly at T2 ( $\overrightarrow{M2}$, gray arrow). Saccades aimed directly at T1 ( $\overrightarrow{M1}$) or at T3 ( $\overrightarrow{M3}$) are not altered. According to the goal updating hypothesis (Equation 6), because $\overrightarrow{G2},\overrightarrow{M1}$, and $\overrightarrow{M3}$ have not changed, the second saccade in a double-saccade sequence FP–T1–T2 should also not change (red arrow):

$$\begin{array}{l}\overrightarrow{M{2}^{\ast }}=f_{\text{M}}(\overrightarrow{G{2}_{\text{m}}^{\ast }})=f_{\text{M}}(\overrightarrow{G2}-\overrightarrow{M1})=f_{\text{M}}(\overrightarrow{G2}-\overrightarrow{G1})\\ =f_{\text{M}}(\overrightarrow{G3})=\overrightarrow{M3}.\end{array}$$ (8)

In contrast, according to the motor updating hypothesis (Equation 7), the second saccade in a double-saccade sequence should change (blue arrow), because $\overrightarrow{M2}$ has changed. Thus, with this paradigm a significant change in eye position at the end of the sequence would constitute evidence in favor of the motor updating hypothesis. We call this the final target dissociation (or FTD) paradigm, since it revolves around an adaptive change of saccades aimed directly at the final target in a sequence.

The second test requires altering the metrics of saccades aimed directly at T3 ( $\overrightarrow{M3}$, gray arrow), for example by making them shorter: $\mid \overrightarrow{M3}\mid <\mid \overrightarrow{G3}\mid$ (Figure 1D). In this case, saccades aimed directly at T1 ( $\overrightarrow{M1}$) or at T2 ( $\overrightarrow{M2}$) are not altered. Under these circumstances, in a double-saccade sequence FP–T1–T2, the motor hypothesis (Equation 7) predicts no change in $\overrightarrow{M{2}^{\ast }}$ (blue arrow), since neither $\overrightarrow{M1}$ nor $\overrightarrow{M2}$ have been affected. The goal hypothesis instead predicts a change. Because $\overrightarrow{M1}$ has not been affected by the adaptation, also in this paradigm we have that Equation 8 holds, and $\overrightarrow{M{2}^{\ast }}=\overrightarrow{M3}$. Hence, the second saccade in the sequence (red arrow) will be directly affected by the adaptive change induced on $\overrightarrow{M3}$. Thus, with this paradigm a significant change in eye position at the end of the sequence would constitute evidence in favor of the goal updating hypothesis. We call this the parallel target dissociation (or PTD) paradigm.

In this paper, we describe the results from testing human subjects in a new paradigm that combines the FTD and PTD paradigms. We found that, under our experimental conditions, the motor updating hypothesis predicts human behavior much more closely than does the goal updating hypothesis.

Methods

Subjects

Five subjects gave informed consent and participated in the experiment. Two (subjects S1 and S2) were authors, whereas the others were not aware of the purpose of the experiment. All had normal or corrected-to-normal visual acuity. All had previous experience with wearing search coils. Experimental protocols were approved by the Institutional Review Board concerned with the use of human subjects. Two different experimental setups were used.

Visual apparatus: CRT setup

The subjects sat in a dark room, positioned so that their eyes were located approximately in the center of a cubic box (70-cm side) containing orthogonal magnetic field-generating coils. Their chin and forehead rested on padded supports, and their head was stabilized using a head band. Visual stimuli were presented on a CRT monitor (Sony Trinitron GDM-C520) located straight ahead 562 mm from the corneal vertex. The monitor screen was 400 mm wide (corresponding to 39.2° of visual angle) by 300 mm high (corresponding to 29.9° of visual angle). The monitor resolution was set to 800 by 600 pixels, and the vertical refresh rate to 170 Hz (5.9-ms frame duration). The background luminance was set to 0.006 cd/m² (as reported by a Konica Minolta LS100 luminance meter); this is a very dim background, but the edge of the monitor was visible to the subjects throughout the experiment. The fixation point and the targets had a luminance of 1.23 cd/m². The fixation point was a disk, with a diameter of 1.25 mm (corresponding to 0.13° of visual angle). The targets were also disks but with a larger diameter (2.5 mm, corresponding to 0.25° of visual angle in central gaze). Only the red gun of the monitor was used, since it had the shortest persistence (using a photocell we verified that the transition from target to background luminance occurred in less than 3 ms). Given the refresh rate used, once a target was to be removed, it was never on the screen for more than 3 ms if at the top of the screen, or 9 ms if at the bottom of the screen. Since our targets were never too far vertically from the center of the screen, 7 ms was the upper limit. Conversely, adding a target to the screen was always accomplished within 12 ms (9 ms at the center of the screen).

Visual apparatus: Tangent screen setup

The subjects sat in a completely dark room; the fixed chair was positioned so that their eyes were located approximately in the center of a cubic box (200-cm side) containing orthogonal magnetic field-generating coils. Their chin and forehead rested on padded supports, and their head was stabilized using a head band. Visual stimuli were back-projected on a tangent screen located straight ahead 100 cm from the corneal vertex. The tangent screen was 183 cm wide by 183 cm high (corresponding to 84.9° of visual angle, both horizontally and vertically). Throughout the experimental session, the subjects could perceive nothing but the fixation point and targets, when presented. The fixation point was presented to the subject by switching on a fixed red LED back-projected onto the tangent screen. The targets were presented by back-projecting onto the tangent screen the light emitted by a red laser. The position of the target on the screen was controlled by appropriately tilting two motor-controlled mirrors placed in the path of the laser. Both the fixation point and the targets had a luminance of 2.67 cd/m² and a diameter of 4 mm (corresponding to 0.23° of visual angle). Fixation point and targets were turned on and off instantaneously. However, repositioning the mirrors took up to 6 ms (2-ms delay plus up to 4 ms to rotate by the desired angle and come to a complete stop). Accordingly, whenever a target was moved, the following procedure was used: (1) the laser was turned off; (2) the command to change the orientation of the mirrors was sent; (3) after 7 ms, the laser was turned on. This guaranteed that a motion streak was never presented to the subject.

Eye movement recording

A scleral search coil embedded in a silastin ring (Skalar; Collewijn, van der Mark, & Jansen, 1975) was placed in each subject’s dominant eye following application of topical anesthetic (proparacaine HCl). Wearing time never exceeded 30 min. The horizontal and vertical orientations of the eye was recorded using an electromagnetic induction technique (Robinson, 1963). The AC voltages induced in the coil were processed by phase-locked amplifiers (CNC Engineering), providing separate DC voltages proportional to the horizontal and vertical orientations of the eye. These outputs were calibrated at the beginning of each recording session by having the subject fixate small targets placed at known eccentricities. Peak-to-peak noise levels resulted in an uncertainty in eye position recording of less than 0.03° in either setup. Coil signals were sampled at 1000 Hz.

Experiment control

In the CRT setup, the experiment was controlled by two computers, communicating over TCP/IP. The Real-time EXperimentation software package (Hays, Richmond, & Optican, 1982), running on the master computer under the QNX operating system, was responsible for providing the overall experimental control as well as acquiring, displaying, and storing the eye movement data. Another machine, directly connected to the CRT display, generated the required visual stimuli in response to REX commands. This was accomplished using the Psychophysics Toolbox v. 3.0.8 (Brainard, 1997; Pelli, 1997), an extension of Matlab (Mathworks, MA).

In the tangent screen setup, the experiment was entirely controlled by a computer running REX (Hays et al., 1982). It provided the overall experimental control, acquired, displayed, and stored the eye movement data, and controlled the operation of the LED, laser, and movable mirrors. In addition, it acquired and stored the actual position of the movable mirrors.

Experimental procedures

As we noted in the Introduction section, we devised a paradigm that combines the FTD and PTD paradigms, allowing us to perform, in each session, two independent tests of the goal/motor updating hypotheses (Figure 2).

Figure 2.

Trial types and predictions. (A) Arrangement of fixation point (FP) and targets (A–D) in our experimental paradigm. (B) Before saccade adaptation is induced, single saccades are aimed at targets A, B, and D and are generally quite accurate. (C) Two sequences of double saccades (note double arrowheads) are tested: FP–A–B and FP–C–D. The location of the targets for the second saccade in each sequence is actually jittered horizontally around targets B and D. Targets are arranged so that, on average, the vector for second saccade in the FP–A–B sequence is equal to the vector of saccades aimed directly at target D. Similarly, the vector of the second saccade in the FP–C–D sequence is equal to the vector of saccades aimed directly at target B. (D) After adaptation is induced, vectors $\overrightarrow{GB}$ and $\overrightarrow{MB}$ are dissociated. (E) If saccades involving target C are excluded, the paradigm reduces to the Final Target Dissociation paradigm (Figure 1C). (F) If saccades involving target A are excluded, the paradigm reduces to the Parallel Target Dissociation paradigm (Figure 1D). (G) According to the goal updating hypothesis, the second saccade end points for FP–A–B sequence will not change after adaptation; in contrast, the end points for the FP–C–D sequence will change (red arrow), approximately in the same way as the end points of saccades aimed directly at target B. (H) According to the motor updating hypothesis, the second saccade end points for FP–C–D sequence will not change after adaptation; in contrast, the end points for the FP–A–B sequence will change (blue arrow), following the changes in the end points of saccades aimed directly at target B.

Throughout our experiment, we kept the location of the fixation point (FP) constant and presented targets at four locations (Figure 2A). The target locations were selected according to the following basic set of rules:

1. Target A was between 8° and 12° away from the FP, with amplitude increase (decrease) experiments starting off with smaller (larger) eccentricities.

2. Target C was, relative to the FP, diametrically opposite target A.

3. Target B was approximately as far away from FP as target A was.

4. Target D was placed relative to target C as target B was placed relative to FP (and thus target B was placed relative to target A as target D was placed relative to FP).

5. The $\widehat{\mathit{BFPD}}$ angle was approximately 90°.

6. The pattern was centered on the screen to minimize eye eccentricity and thus coil-related discomfort.

7. Large vertical eccentricities were avoided, since they are more likely to result in coil slippage or discomfort.

In all, we used four sets of target positions, which we felt best met the above-mentioned criteria. The arrangement illustrated in Figure 2A is the basic one; the other three were obtained by flipping that arrangement around the vertical axis, horizontal axis, or both.

In our paradigm, there were five different trial types (conditions), one for each type of movement that the subject was required to perform. The first three conditions involved making visually guided saccades (meaning that the target was visible at saccade onset) to individual targets (A, B, or D, Figure 2B). Each trial started with a blank screen. Once the fixation point appeared, the subject had to fixate it within 1 s, or the trial was aborted. After 200 ms, the target appeared, and 800–1000 ms later, the FP disappeared, signaling the subject to make the required saccade (GO). The eye position was monitored to ensure that the eyes exited a small invisible window around the FP at least 100 ms, but not more than 1 s, after the GO signal; otherwise, the screen was blanked, a buzzer was activated, and the trial was aborted. All aborted trials had to be repeated, within the same block of 60 trials. For saccades toward targets A and B, the target was present when the saccade was completed, and it stayed on for another second, or until the subject looked back at the location of the now invisible FP. For saccades toward target D, the target disappeared as soon as the saccade started (the signal to turn off the target was sent once the eyes turned 1°). In this condition, the subjects had no visual feedback and were thus unaware of their performance.

The other two conditions in our paradigm required the subjects to make sequences of two saccades (Figure 2C). In one sequence, the first saccade was directed to target A and the second to target B; in the other sequence, the first was aimed at target C and the second at target D. Traditionally in the double-saccade task, a brief flash (usually between 80 ms and 120 ms) of the first target is followed by a brief flash of the second target (usually between 20 ms and 80 ms). Once both flashes are over, the subject must make a sequence of two saccades in complete darkness. Unfortunately, it is quite difficult to accurately localize and memorize the location of two small targets presented for such a short time. Not surprisingly, this invariably leads to a large scatter in the end point of the saccades, even for the first of the two. To get around this problem, we modified the task as follows. Once again, the trial started with a blank screen, and then the FP appeared. Once the subject looked at the FP, the second target in the sequence (i.e., B in the first sequence, and D in second one) appeared. After 800–1000 ms, that target disappeared, and the first target in the sequence (i.e., A in one sequence, and C in the other) appeared. After 300 ms, the FP disappeared, signaling the subject to make the required sequence of saccades. Early saccades caused the trial to be automatically aborted. The target for the first saccade in the sequence (A or C) disappeared as soon as the saccade started (the signal to turn off the target was sent once the eyes turned 1°). The subjects thus had no visual feedback about either of their saccades.

This modified double-saccade paradigm has several advantages over the traditional one. First of all, both targets, and especially the target for the second saccade, are present for a relatively long time, leading to a considerably more accurate localization and memorization (which translates into smaller saccade end-point scatter). Second, the first saccade is visually guided, and thus much more accurate. Third, when target B (D) appears the subjects have no way of knowing if they will have to make a saccade directly to it, or if this trial requires a double saccade. Accordingly, the subjects have an incentive to plan a movement to the target. If the FP disappears they will actually execute the plan, whereas if the target disappears they will have to change the plan. Fourth, because the targets were arranged so that the second saccade in a sequence was neither horizontal nor vertical, it was difficult to semantically memorize the target location. Finally, this task is very easy to learn, since it requires following a very simple rule: once the FP goes off, the subject must always make a saccade to the target currently visible. If a target had appeared before, then the subject has to look at its remembered location.

Another issue that plagues most double-saccade experiments is that only a small number of target locations are used throughout a session. This arrangement is forced upon the experimenter by the relatively large scatter associated with memory-guided saccades, which makes averaging a necessity. The downside of this solution is that it makes it impossible to know whether, on a trial-by-trial basis, the subject uses the visual information that was just provided to plan the saccades, or whether long-term memory plays a role. It is well known that subjects can remember the location of a flashed target for a long time, even while making a large number of movements (Becker & Klein, 1973; Hansen & Skavenski, 1977; Skavenski & Steinman, 1970). It is thus possible that in the course of an experiment double saccades would not always be planned in the same way. The second problem with this type of experiment is that it is not possible to ensure that the subject pays attention to where the target appears: cheating, by simply aiming in the general direction of the target, is simple and requires considerably less effort. To get around these problems, instead of always using the same two locations (within one session) for the second target in a sequence (B and D in Figure 2C), we jittered the target location around those positions from trial to trial. After each experiment, we could then correlate the saccade end points with the location of the target. A significant correlation, with a slope not significantly different than one, could only be found if the subjects performed the experiment as instructed. For simplicity, we decided to jitter only the horizontal location of the target. Pilot experiments previously carried out showed that, given the variability observed when the targets are always in the same position, jittering the target location by ±1.0–1.5° would be sufficient to find a significant correlation between saccade end points and target location, if present. Each subject used in our experiment was able to perform, under our experimental conditions, double saccades for which second saccade end points and target position were strongly correlated. Some subjects were capable of this naturally, whereas others required some training sessions. One subject did not manage to achieve this performance even after three training session and was excluded from further testing (this subject was in addition to the five mentioned above, not one of them). This is not particularly surprising, because making saccades in darkness to the remembered location of targets is not a natural activity. In all experiments reported here, the slope of the relationship between the horizontal location of the target and the post-saccadic horizontal eye position was significantly different than zero (p < 0.01) and not significantly different than one (p > 0.05). As expected, the relationship between the horizontal location of the target and the post-saccadic vertical eye position was never significantly different than zero (p > 0.05).

Each experimental session consisted of three phases. In the first phase (pre-adaptation), the baseline behavior was ascertained by having the subject perform 60 trials (10 each of the single saccade conditions, 15 each of the double-saccade conditions), randomly intermixed. We then initiated the adaptation phase, in which the amplitude of single saccades to target B was altered by applying McLaughlin’s intra-saccadic target step paradigm (McLaughlin, 1967). In this paradigm, the subject is asked to make a saccadic eye movement toward a visual target; while the eyes are moving at high speed, the target is displaced. This is repeated over and over. Initially, the subject’s eyes land around the initial location of the target, and the target is eventually acquired by a secondary (corrective) saccade. However, over time the primary saccade is modified, so that after a while the subjects aim their eyes directly at (or close to) the final target location. The relationship between the visual and motor vectors is thus modified. Importantly, several lines of evidence (reviewed in the Discussion section) indicate that the dissociation likely occurs not at the level of the conversion of the visual vector into a goal vector, but rather in the conversion of the goal vector into a movement vector. It is thus f_M() that is modified by this paradigm. The duration of this phase varied from session to session, depending on how quickly saccadic adaptation was induced (i.e., the amplitude of $\overrightarrow{MB}$ changed). For amplitude reductions, we initially stepped back target B by 20% of the distance between B and FP. This percentage was then increased to 30%, and in some subjects up to 35%. Since $\overrightarrow{VA}$ and $\overrightarrow{VB}$ are not very far apart, we typically counteracted the tendency of adaptation to spread to $\overrightarrow{MA}$ (Alahyane, Devauchelle, Salemme, & Pélisson, 2008; Alahyane et al., 2007; Albano, 1996; Collins, Doré-Mazars, & Lappe, 2007; Deubel, 1987; Frens & van Opstal, 1994; Kojima, Iwamoto, & Yoshida, 2005; Miller, Anstis, & Templeton, 1981; Noto, Watanabe, & Fuchs, 1999; Semmlow, Gauthier, & Vercher, 1989; Straube, Fuchs, Usher, & Robinson, 1997; Wallman & Fuchs, 1998) by intra-saccadically stepping target A further away from FP during trials that required single saccades to A. This was sufficient to considerably limit (although not to abolish) changes in $\overrightarrow{MA}$. For amplitude increases, we initially stepped forward target B by 20% of the distance between B and FP. This percentage was then increased to 30%. Since in our setup gain increases were harder to induce than gain decreases (Deubel, 1987; Deubel, Wolf, & Hauske, 1986; Miller et al., 1981), we never found it necessary or useful to push any further. In this case, target A was not intra-saccadically displaced during single saccades to it, since we did not find it to be necessary. During the adaptation phase, single saccades to target B were considerably more frequent (70% for amplitude decreases, 80% for amplitude increases) than the other trial types (which had equal frequency). This phase was terminated once an acceptable level of adaptation was reached. By default, this occurred after 150 adaptation trials, but the experimenter could either shorten this phase (but to no less than 120 adaptation trials) if an asymptote had been reached, or lengthen it (but to no more than 200 adaptation trials), if adaptation was proceeding slowly. The post-adaptation phase was similar to the pre-adaptation phase, with the only differences being that it encompassed twice as many trials (120) and that the intra-saccadic steps applied during the adaptation phase were continued (thus maintaining the adaptation).

Note that if we only consider the trial types that do not involve target C, what we are left with is an FTD paradigm (Figure 2E). Targets A, B, and D correspond to T1, T2, and T3, respectively, from Figure 1C. In contrast, if we only consider the trial types that do not involve target A, what we are left with is a PTD paradigm (Figure 2F). Targets C, D, and B correspond to T1, T2, and T3, respectively, from Figure 1D. From each experimental session, we thus extracted two independent tests of the hypotheses, which could be discriminated simply by examining the differences in the end points of the second saccade in the sequences brought about by the adaptation phase. If the goal updating hypothesis holds (Figure 2G), the end points of the second saccade in the FP–C–D sequences would change, following the changes in the end points of saccades aimed directly at B. In contrast, the end points of the second saccade in the FP–A–B sequences would not shift. Under the motor updating hypothesis (Figure 2H), the opposite pattern is expected: the end points of the second saccade in the FP–C–D sequences would not shift, whereas the end points of the second saccade in the FP–A–B sequences would follow the changes in the end points of saccades aimed directly at B.

Each subject performed multiple sessions in the CRT setup, with different target arrangements. To ensure extinction of leftover effects from previous sessions, successive sessions were separated by at least 10 days (Alahyane & Pélisson, 2005). To verify the potential impact of visual references on the outcome of our experiments, two subjects also performed multiple sessions in the tangent screen setup.

Data analysis

For each saccade being generated in our task, we had to extract the starting and ending eye positions. The former can always be easily determined. We defined as the start position of each saccade the average eye position in a 100-ms window preceding the instant when the eye speed exceeds 10°/s. The eye velocity trace was computed from the eye position trace using a Savitzky–Golay filter (Savitzky & Golay, 1964; 4th order polynomial, kernel size 11). Because saccades are sometimes followed by slow drifts (Bahill, Clark, & Stark, 1975), determining the saccade end position is more problematic. These drifts, called glissades, are equally likely in darkness and when the target is present. If the target is present, they are equally likely to move the eyes toward or away from the target. They are thus not visually guided; they most likely emerge because of a mismatch between the static and dynamic (step and pulse, respectively) components of the innervation signal (Bahill, Clark et al., 1975). Since we were interested in inferring from the data the planned saccadic end point, in our main analysis we considered these drifts as part of the plan and included them. Accordingly, we defined as the end position of each saccade the average eye position in a 100-ms window preceding the instant when the next saccade started (or the end of the trace if no other saccades were generated in the recording window). So-called corrective saccades (Becker & Fuchs, 1969), rarely observed for saccades of the size we used, were not included. All saccades so marked were visually inspected to ensure the validity of the method. In the rare cases in which the procedure failed, the saccade’s start and end were marked by hand. The glissades were always very small, accounting on average for 0.02°(across subjects: Mean = −0.04°–0.03°, STD = 0.1°–0.17°, Absolute Mean = 0.08°–0.15°). We repeated the analysis excluding the glissades, but we found no significant differences. Occasionally, some very small saccades (<3°) were generated, later followed by a larger saccade to the target. Such trials (always less than three in any one session) were excluded from the analysis.

The above-described procedure worked appropriately for saccades to single targets and for the first saccade in a sequence. However, it was unreliable for the second saccade in a sequence, because quite often extra saccades were generated. We found that the prevalence of this behavior, of which the subjects themselves were unaware, is quite variable across subjects, and even across sessions for the same subject. We noticed that with practice subjects improved their ability to perform just two saccades in a sequence. Consequently, we had some subjects perform one or two practice sessions, which included only double-saccade trials. The target configurations used in these sessions were different than those used in our main experiment. Even so, a small fraction (less than 20%) of the double-saccade sequences generated during our experiment included three saccades. For those trials, we marked the end of both the second and third saccades in the sequence. We then performed all our analyses twice, once using the initial end point for the second saccades (i.e., the last end point in two-saccade sequences, and the second end point in three-saccade sequences) and once using the final end point. We never found any significant difference (which is not surprising, since in over 80% of the trials the same data points were used), and thus we only report analyses based on the final end point. Other authors tackled this problem by using the end point that was closest to the target position (Ditterich et al., 1998), but we did not consider that approach viable in the context of our experiments. We discarded the occasional trials in which more than three, or only one, saccades were generated, or the saccades were executed in the wrong order.

Once we had so extracted the saccade end points, we had to compensate for the jitter introduced in the horizontal position of the targets for the second saccades in the sequences. As explained above, in all our sessions there was a strong correlation between the horizontal location of the target and the horizontal component of the end points (slope of the correlation is always significantly different from zero, and not significantly different from one).¹ We thus corrected the second saccade end points for the jitter by simply shifting them in the opposite direction and by the same distance, as the target. For example, if in a given trial the second target for an FP–AB sequence was presented 1° to the left of target B, the end point of the second saccade in that sequence was shifted 1° to the right. No vertical shifts were applied. Note that the only effect of this procedure was to reduce the horizontal scatter of the end points, by compensating for the scatter that could, and should, be attributed to the target jitter. Because the target was equally likely to be shifted to the left or to the right, this procedure minimally affected the location of the center of the distribution of saccade end points (mean absolute shift: 0.14°, maximum absolute shift: 0.29°).

Our analysis boiled down to testing the statistical significance of adaptation-induced changes in the end points of double saccades (Figures 2G and 2H). The most straightforward approach would have been to use either a MANOVA or Hotelling’s two-sample T-square statistic to compare the distribution of end points predicted by the two hypotheses with the end points actually measured. However, multivariate statistical tests have lower power than univariate ones, and this was of particular concern in our case since our experimental conditions limited the number of repetitions. Instead, we opted for a univariate analysis that, in addition to having a much higher statistical power, allowed us to quantify the distance of the measured data from either hypothesis. We illustrate this analysis in detail in the Results section.

All analyses were carried out using the Enthought Python Distribution (Enthought, Austin, TX).

Results

In the set of experiments described here, we used McLaughlin’s (1967) intra-saccadic step paradigm to change the size of saccades aimed directly at target B (Figure 2), thus inducing a dissociation between visual and motor vectors. We then exploited this dissociation to discriminate between two hypotheses regarding the generation of sequences of saccades. Because we found a significant effect only when saccades were shortened, we devote most of this section to those experiments. We address saccade lengthening experiments separately at the end.

Visuomotor dissociation

The size of single saccades aimed directly at target B was reduced by intra-saccadically stepping target B back toward the FP (by 30% to 35% of the target eccentricity). The saccade amplitude reduction ranged, across sessions, between 17% and 33% of the initial saccade size. By the end of the adaptation phase, the amplitude change was stable in all sessions. This change was always maintained in the post-adaptation phase, with only a slight tendency to fade away (causing saccade size to slightly increase).

Final target dissociation paradigm

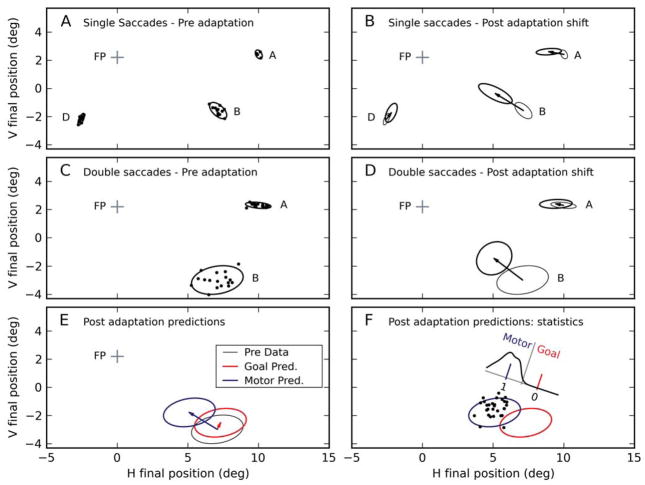
If we restrict our analysis to the trial types that do not involve target C, what we are left with is an FTD paradigm (Figure 2E). In Figure 3, we report the results obtained under this paradigm in one representative session (in subject S1). In the pre-adaptation phase, saccades to a single target are quite accurate and have very limited scatter. In Figure 3A, we plot the end points of such saccades, together with their 95% confidence interval ellipses. After adaptation is induced by back-stepping target B (Figure 3B), the amplitude of saccades aimed at it is considerably reduced (28% in this case). The amplitude of saccades to targets A and D is also somewhat reduced (in this case, 11% for the former and 10% for the latter). Before adaptation, FP–A–B double-saccade sequences are characterized by a small end-point scatter for the first saccade, only slightly larger than that observed for single saccades, and by a much larger end-point scatter for the second saccade (Figure 3C). After adaptation was induced, we found that the end points of the first saccade barely shifted; in contrast, the end points of the second saccade shifted considerably (Figure 3D).

Figure 3.

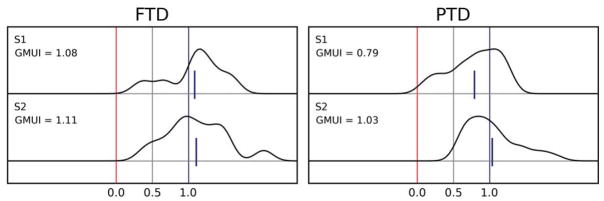
Sample amplitude-shortening session: FTD paradigm. (A) Before adaptation, saccades to a single target are accurate and have a small scatter (95% confidence ellipses are shown, together with actual saccade end points). (B) After adaptation, the amplitude of saccades to target B are considerably reduced (by 28% in this case); saccades aimed at A and D are only mildly affected. Thin ellipses indicate pre-adaptation end points, whereas thick ellipses indicate post-adaptation end points. Arrows indicate mean shift. (C) Before adaptation, the end points of the first saccade in the FP–A–B sequence are accurate; end points of the second saccade are considerably more scattered (note that the horizontal scatter due to the target jitter has already been compensated for). (D) After adaptation, we observed a large change in the end points of the second saccade, but not the first saccade, in the FP–A–B sequence. (E) Based on the pre-adaptation end points of the second saccades, the motor and goal updating hypotheses make clear predictions about the post-adaptation end points. The motor updating hypothesis (blue ellipse and arrow) predicts that they will shift like the end points of single saccades aimed at B, whereas the goal updating hypothesis (red ellipse and arrow) essentially predicts that they will mimic the changes of single saccades aimed at D. (F) In this session, the post-adaptation second saccade end points (black dots) obviously match the motor prediction (blue ellipse) much better than the goal prediction (red ellipse). We tested this statistically by projecting these points onto the axis that connects the centers of the two predictions. We normalize this axis to compute an index (GMUI) such that a value of 0.0 (1.0) corresponds to the center of the goal (motor) prediction. A distribution mean significantly smaller (larger) than 0.5 supports the goal (motor) hypothesis. In this case, the mean is 1.06. Data from subject S1.

We can now go back to Figure 2 to see what the predictions of the two hypotheses would be for this paradigm. According to the motor updating hypothesis (Equation 7), the end points of the second saccade should shift, tracking the changes in the end points of saccades aimed directly at B. Consequently, the motor hypothesis predicts that the pre-adaptation end points (black thin ellipse in Figure 3E) will be shifted (blue arrow and ellipse in Figure 3E) in the same direction and by the same distance as single saccades aimed at B (Figure 3B). According to the goal updating hypothesis, the end points of the second saccade should not shift (Figure 2E). However, that prediction was based on the assumption that single saccades to D, and the first saccade in the sequence, would not change. In reality, some small changes are always observed. Under these circumstances, Equation 8 does not strictly hold, and even the goal updating hypothesis then predicts some shift in the second saccade end points (red arrow and ellipse in Figure 3E). This predicted shift is approximately equal to the shift in the end points of saccades aimed directly at target D. This follows directly from Equation 6 (exact calculations can be found in Appendix A). Intuitively, under the goal updating hypothesis the magnitude of a saccade depends only on the relative location of the two targets and is independent of the initial eye position. A saccade from A to B would then be identical to (and thus in our case track the changes of) a saccade between FP and D.

Obviously, in this session the motor prediction matched the data much more closely than the goal updating prediction (Figure 3F, black dots are post-adaptation end points of second saccades), but a statistical test is nonetheless required. To quantify whether the post-adaptation data matched either prediction, we started from the observation that only variations along the axis that goes through the centers of the two predicted distributions (ellipses in Figure 3F) are useful to discriminate between the two hypotheses. Variations orthogonal to it are, as far as hypothesis testing is concerned, simply noise. Thus, we projected the post-adaptation saccade end points (black dots in Figure 3F) onto this axis. The distribution of these projections (density plot in Figure 3F) captures everything we need to know about the hypotheses and the data. To make it easier to compare across sessions, we can normalize these projections, computing an index such that a point at the center of the goal prediction is mapped to 0.0, while a point at the center of the motor prediction is mapped to 1.0. We call this the Goal/Motor Updating Index (GMUI). Student’s one-sample t-test can then be used to verify whether the distribution of GMUI values is significantly different from 0.5. If it is not, we can only conclude that the data do not favor either hypothesis. If the GMUI is significantly larger than 0.5, we can then use the same statistical test to verify whether it is significantly different than 1.0. A negative result would allow us to conclude that the data are completely consistent with the motor hypothesis. Conversely, if the mean GMUI is not significantly different from 0.0, we would conclude that the data are completely consistent with the goal updating hypothesis. If the mean GMUI is significantly different from all three values, we can then use its value directly to estimate how close to each hypothesis the data fall. For the session shown in Figure 3, the mean GMUI is 1.06, and it is not significantly different from 1.0; in this session, the results from this paradigm thus fully support the motor updating hypothesis.

Parallel target dissociation paradigm

Besides the FTD paradigm, our experiment also embedded the PTD paradigm (Figure 2F). In Figure 4, we report the results obtained under this paradigm in the same session used to describe the FTD paradigm results. Again, in the pre-adaptation phase, saccades to a single target are very consistent (Figure 4A). As seen in Figure 3, after adaptation is induced by back-stepping target B, the amplitude of single saccades aimed at both target B and target D was reduced (Figure 4B). Before adaptation, the first saccades in FP–C–D double-saccade sequences are highly consistent; the end points for the second saccades are, however, much more variable (Figure 4C). After adaptation was induced, the end points of the first saccade did not change, whereas the end points of the second saccade shifted slightly (Figure 4D).

Figure 4.

Sample amplitude-shortening session: PTD paradigm. (A–D) Analogous to Figures 3A–3D, but here the FP–C–D sequence is considered. (E) Based on the pre-adaptation end points of the second saccades, the motor and goal updating hypotheses can be used to predict the post-adaptation end points. The motor updating hypothesis (blue ellipse and arrow) predicts that they will shift like the end points of single saccades aimed at D, whereas the goal updating hypothesis (red ellipse and arrow) essentially predicts that they will mimic the changes of single saccades aimed at B. (F) Once again the post-adaptation second saccade end points (black dots) match the motor prediction (blue ellipse) much better than the goal prediction (red ellipse). In this case, the mean GMUI is 0.97, not significantly different than 1.0. Data from subject S1.

According to Figure 2H, the motor updating hypothesis predicts that in this paradigm the end points of the second saccade should not be affected by the adaptation. However, this prediction was based on the assumption that saccades aimed directly at target D were unaltered. In the more general case, we have that the end point of the second saccade in the sequence would have to track the changes of a direct saccade to the final target (D in this case). Thus, the motor hypothesis predicts (blue ellipse in Figure 4E) that the pre-adaptation end points (black solid ellipse in Figure 4E) will be shifted in the same direction and by the same distance as single saccades aimed at D (Figure 4B). According to Figure 2G, the goal updating hypothesis predicts that the end points of the second saccade should shift, tracking the changes in the end points of saccades aimed directly at B. That prediction was based on the assumption that the first saccade in the sequence would not change. In reality, some small changes are often observed (although not in this particular case), which require a small adjustment (exact calculations in Appendix A) to the predicted shift (red ellipse in Figure 4E).

As done before, we can now use the GMUI to quantify how well the post-adaptation data match the predictions. In this case, the motor prediction obviously matches the data much more closely than the goal prediction (Figure 4F). For the session shown in Figure 4, the mean GMUI is 0.97, and it is not significantly different from 1.0; the results from this paradigm thus also fully support the motor updating hypothesis.

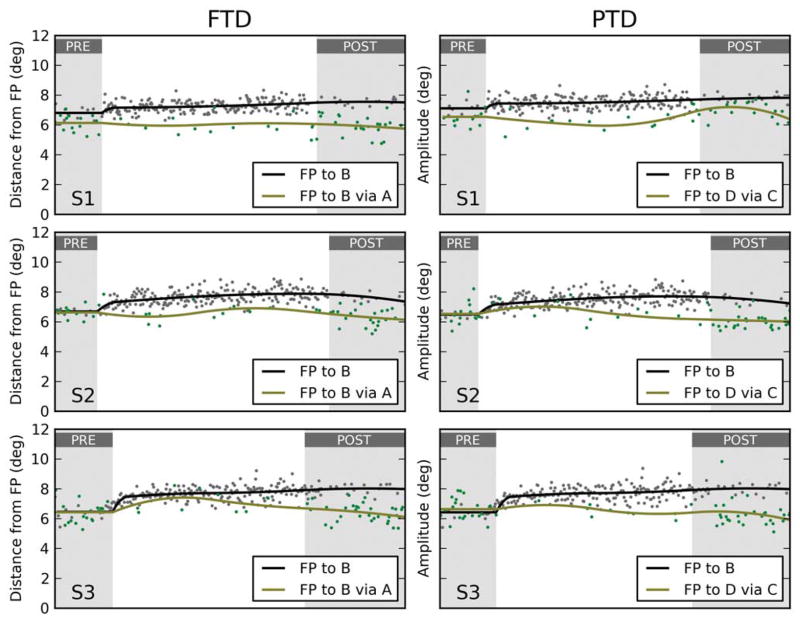
Variability across subjects

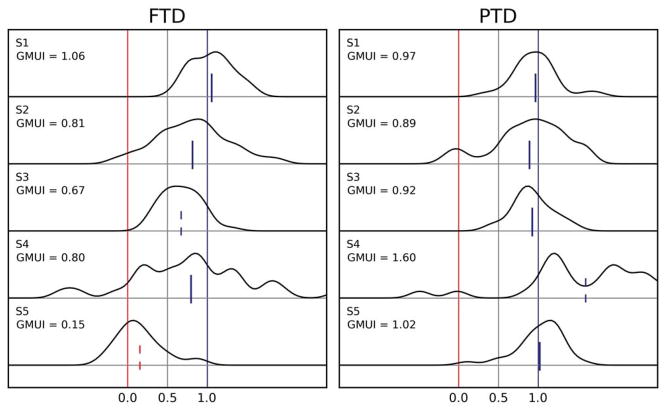
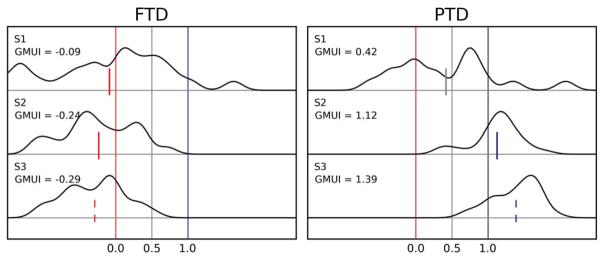
The data from our five subjects are presented in Figure 5. The left column contains the distribution of second saccade end points, projected along the GMUI axis, in the FTD paradigm; data from the PTD paradigm are reported in the right column. Each row corresponds to a different experimental session, each performed by a different subject. The data in the first row are the same as those used in Figures 3 and 4. For the first four subjects, we have that for both paradigms the motor updating hypothesis accounts best for the data. The mean GMUI (indicated by a vertical segment) is not significantly different than 1.0 in six out of eight tests (solid blue). In the other two cases (dashed blue), it is significantly larger than 0.5, but it also is significantly different than 1.0. The data from the last subject do not follow this pattern. In the PTD paradigm, this subject’s responses are compatible with the motor updating hypothesis (GMUI not significantly different than 1.0), but in the FTD paradigm they are instead compatible with the goal updating hypothesis (even though the GMUI is significantly larger than 0.0, dashed red segment). To understand what is happening, it is useful to go back to the diagrams in Figure 2. In the PTD paradigm, the motor updating hypothesis (blue arrow in Figure 2F) predicts that the end points of the second saccades will not be affected by the adaptation. In the FTD paradigm, the goal updating hypothesis (red arrow in Figure 2E) also predicts that the end points of the second saccades will not be affected by the adaptation. In other words, the pattern observed in subject S5 means that, in both paradigms, the end points for the second saccades are largely unaffected by the adaptation.

Figure 5.

Amplitude-shortening sessions from all subjects. The density distributions of the projected end points based on which the Goal/Motor Updating Index (GMUI) is computed are plotted for all subjects, separately for the FTD and PTD paradigms. A mean value around 1.0 (0.0) indicates support for the motor (goal) hypothesis. The vertical segments in each plot mark the mean GMUI, with blue indicating support for the motor updating hypothesis, red for the goal updating hypothesis, and gray for neither. A solid segment indicates that the evidence fully supports a hypothesis (mean not significantly different from 1.0 or 0.0), whereas a dashed segment indicates partial support (GMUI significantly different from 1.0 and 0.0). Under the FTD paradigm (left column), subjects S1–S4 showed strong support for the motor hypothesis, whereas the data from subject S5 better matched the prediction of the goal hypothesis. Under the PTD paradigm (right column), the data from all subjects supported the motor hypothesis.

Time course

So far we have compared the distribution of second saccade end points before and after the adaptation-induced visuomotor dissociation. It would however be interesting to know how these changes evolve over time. Unfortunately, during the adaptation phase we could only introduce a small number of double-saccade trials. In addition, during this phase the system is by definition evolving. Accordingly, it is not possible to apply the analysis used above to see how the GMUI evolves over time. It is however possible to plot the time course of some univariate measures that are a reasonable surrogate for the GMUI. For the FTD paradigm, we could compare the distance from the fixation point of: (1) end points of single saccades to target B, and (2) end points of the second saccades in the FP–A–B sequences. According to the motor updating hypothesis, the latter should track the former, whereas according to the goal updating hypothesis the latter should not change. Similarly, for the PTD paradigm we could compare the amplitude of: (1) single saccades to target B, and (2) second saccades in the FP–C–D sequences. According to the motor updating hypothesis, the latter should not change, whereas according to the goal updating hypothesis the latter should track the former.²

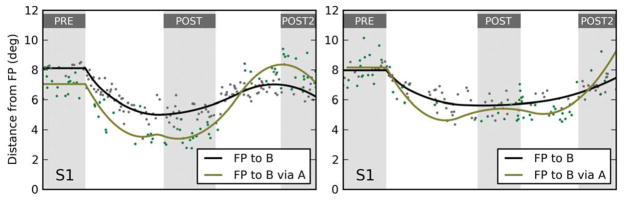
In the top row of Figure 6, we plot these measures for subject S1 (FTD paradigm in the left column, PTD paradigm in the right column). The gray shaded areas mark the pre- and post-adaptation phases. During the adaptation phase, saccades to B are progressively shortened, and thus their end points get progressively closer to the fixation point (black, left panel) and their amplitude decreases (black, right panel). Since fixation was tightly controlled, the black lines in the right and left panels are almost identical. The distance from the fixation point of the end points of the second saccades in the FP–A–B sequences (green, left panel) closely tracks the black line, as predicted by the motor updating hypothesis. In contrast, the time course of the amplitude of the second saccades in the FP–C–D sequences (green, right panel) is stable throughout the experiment. Under the goal updating hypothesis, it should have tracked the black line, but this does not occur, as predicted by the motor updating hypothesis.

Figure 6.

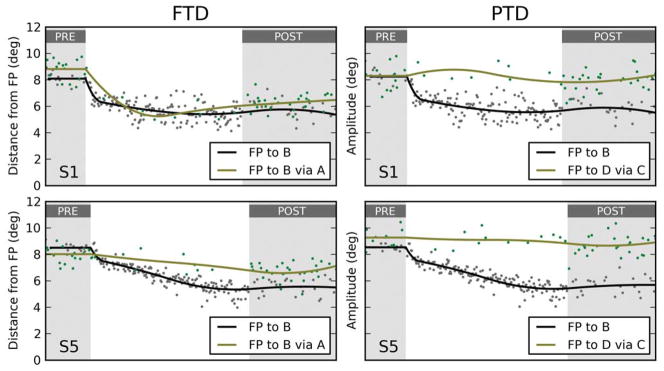
Time course of adaptation-shortening effect in two subjects. The effects of adaptation on two measures are plotted, separately for the FTD and PTD paradigms, for subjects S1 and S5. In subject S1 (top row), under the FTD paradigm (left column) the distance between the FP and the end points of the second saccades in the FP–A–B sequences (green) follows the adaptation-induced changes in the end points of saccades aimed directly at target B (black), as predicted by the motor hypothesis. Under the PTD paradigm (right column), the amplitude of the second saccades in the FP–C–D sequences (green) does not follow the adaptation-induced changes in the amplitude of saccades aimed directly at target B (black). This is again compatible with the motor hypothesis. In subject S5 (bottom row), we find a virtually identical behavior in the PTD paradigm. However, in the FTD paradigm the change in the second saccade end points, while significant, is only a fraction of the change predicted by the motor hypothesis. In all panels, individual data points are indicated by dots, whereas the lines are spline fits to the data.

In the bottom row of Figure 6, we plot the same measures for subject S5. We can immediately see that also in this subject the adaptation has a great impact on single saccades to target B. However, double saccades in the FTD paradigm are only partially affected. The end points of second saccades in the FP–A–B sequence are clearly moving toward the fixation point (green, left panel), but to a much smaller extent than predicted by the motor updating hypothesis. While this does not strongly support the motor hypothesis, the complete lack of any amplitude reduction in the second saccades in the FP–C–D sequences (green, right panel) clearly rules out the goal updating hypothesis. The most straightforward explanation for this behavior is that, in subject S5, the visuomotor dissociation induced by McLaughlin’s paradigm in the context of single saccades transferred only partially (15% according to the GMUI for this experiment) to the double-saccade sequence. We will further address this issue in the Discussion section.

Reproducibility across sessions

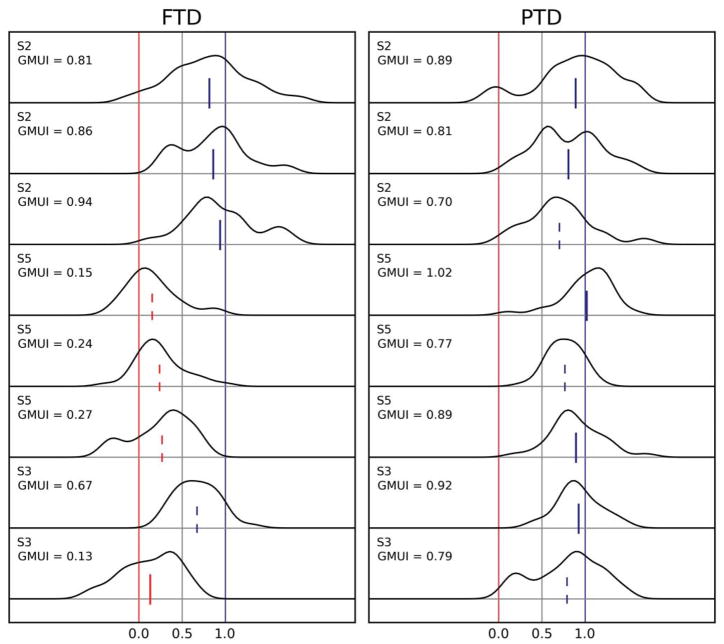
The data shown in Figure 5 were collected during the first session in which each subject participated. To verify that the results obtained were consistently reproducible, we had three subjects (S2, S3, and S5) perform additional sessions, spaced at least 3 weeks apart. The rationale for selecting these subjects was that they were representative of the range of behaviors observed in the first session: S2’s data were wholly compatible with the motor updating hypothesis, S3’s data were mostly supportive (GMUI significantly larger than 0.5 but smaller than 1.0 in the FTD paradigm), and in S5, our dissociation paradigm was only minimally successful. In Figure 7, we plot, using the same format used for Figure 5, the results from three sessions from S2, three sessions from S5, and two sessions from S3 (for each subject, the first session shown is the one already reported in Figure 5). Subjects S2 and S5 were highly consistent, showing virtually identical behaviors across sessions, and thus retaining their differences. The second session from subject S3 was, however, quite surprising: the end point of second saccades in that session was not affected by the adaptation phase. Somehow, the dissociation paradigm, which successfully influenced S3’s double-saccade sequences 6 weeks before, had now become completely ineffective as a probe of our hypotheses.

Figure 7.

Repeatability of adaptation-shortening effects: multiple sessions per subject. Three subjects participated in multiple amplitude decrease sessions. Subject S2 (rows 1–3) exhibited a virtually identical behavior in three sessions, always yielding data that strongly support the motor hypothesis. Subject S5 (rows 4–6) was just as consistent, showing no adaptation-induced changes in the end points of second saccades in the PTD paradigm and only partial (but significant) changes in the FTD paradigm. Subject S3 (last two rows) was instead inconsistent, exhibiting strong support for the motor hypothesis in the first session but showing no adaptation-induced changes in the end points of second saccades under either paradigm in the second session. For each subject, 3 or more weeks separated each session.

Effect of visual references

All the experiments described so far were carried out by presenting the visual stimuli on a CRT screen. As we noted in the Visual apparatus: CRT setup section, the screen background was very dim but bright enough that the subjects could perceive its border at all times. We had elected to make the background visible, because even when we made it as dark as possible (luminance lower than 0.001 cd/m², the threshold for our luminance meter) within 5 min of being in complete darkness the subjects started to perceive it. For obvious reasons, we preferred to avoid such a transition. This, however, raises the possibility that the presence of visual references might somehow contribute to our findings. To investigate this hypothesis, we had two of the subjects repeat the experiment in a different setup. Instead of using a CRT screen, the visual stimuli were LED or laser-generated light spots, back-projected on a tangent screen in an otherwise completely dark room. Under these conditions, no visual references were available during the experiment. In both subjects, McLaughlin’s paradigm reduced the amplitude of single saccades to target B by 25%. In Figure 8, we plot the GMUI density functions for these two experiments. The format is the same used in Figures 5 and 7. In both cases, the GMUI was not significantly different than 1.0 in either paradigm, fully replicating the results obtained in these same subjects in the CRT setup.

Figure 8.

Amplitude shortening in the tangent screen setup. Two subjects performed the amplitude reduction experiment in a setup that guaranteed the absence of any visual references. The behavior was unchanged in both subjects, strongly supporting the motor updating hypothesis (in all cases, GMUI not significantly different from 1.0).

Saccade lengthening

Our efforts to use McLaughlin’s paradigm to increase the amplitude of single saccades aimed at target B were largely unsuccessful. Out of eight sessions (five with the CRT setup, and three with the tangent screen setup), in only three were we able to increase the saccade amplitude by more than 10%, a level under which no significant effect can be reasonably expected. In Figure 9, we plot the GMUI densities for these three experiments (all recorded with the CRT setup). The gain change induced by the adaptation in these three sessions was 10%, 16%, and 24%, respectively. However, in none of these three cases was any strong effect of the adaptation on the distribution of the end points of second saccades observed. The GMUI density distributions are also considerably more scattered than for the amplitude reduction cases, especially in S1. This is due to the much reduced amplitude change for saccades to target B, which causes the predictions made by the motor and goal updating hypotheses to be much closer, making the GMUI more noise-sensitive.

Figure 9.

Saccade-lengthening sessions. All our subjects were tested in the amplitude increase experiment. However, in only three cases the amplitude change induced was larger than 10%. Here we plot the GMUI distribution for those three sessions, separately for the FTD and PTD paradigms. Note that in all cases the distributions are more scattered than those for amplitude reduction. In addition, we never found consistent support over both paradigms for either hypothesis. In essence, all subjects exhibited only very small adaptation-induced changes in the end points of second saccades, under both paradigms.

The absence of any significant change in the end points of second saccades can also be verified by plotting the data from these three experiments in the same format used in Figure 6. Thus, in Figure 10, we plot in the left column the time course of the distance from the fixation point of: (1) the end points of single saccades to target B (black); and (2) the end points of the second saccades in the FP–A–B sequences (green). Similarly, in the right column, we plot the time course of the amplitude of: (1) single saccades to target B (black); and (2) second saccades in the FP–C–D sequences (green). It is clear that, whereas some amplitude increase is achieved in single saccades aimed at B, no consistent change is observed for the saccade sequences.

Figure 10.

Time course of amplitude increase effect. Same sessions shown in Figure 9. In all three subjects, there was a significant, albeit relatively small, increase in the size of saccades aimed directly at target B (black). However, neither the distance from the FP of end points of second saccades in the FP–A–B sequence (green, left column) nor the amplitude of second saccades in the FP–C–D sequence (green, right column) was systematically affected. The changes were small in all cases and occasionally were even in the opposite direction.

Adaptation washout

The asymmetry between the behavior under amplitude reduction and lengthening raised the following question: what happens when the amplitude reduction is reversed? It is currently not clear whether the so-called washout of adaptation should be considered akin to an adaptation-induced amplitude increase, or if it is a distinct process. In principle, the speed of this process should readily answer this question, but in fact the results reported are quite confusing. In one study in human subjects (Deubel et al., 1986), it was reported that amplitude shortening was one order of magnitude faster than amplitude lengthening. When adaptation-induced amplitude shortening was washed out by having the subject make saccades to a target that was not intra-saccadically displaced, the time course of recovery was slow, comparable to amplitude lengthening adaptation. In another study, also in humans, it was instead found that shortening and washout had the same time course (Moidell & Bedell, 1988). Studies in monkeys also reported that the washout is fast, with a time course comparable to that of the amplitude-reducing phase (Kojima, Iwamoto, & Yoshida, 2004; Robinson, Soetedjo, & Noto, 2006; Straube et al., 1997). To clarify this issue, we had one subject (S1) perform two sessions (one in the CRT setup, and one in the tangent screen setup) in which we first induced an amplitude decrease, and then reversed it by not applying the intra-saccadic target step. With our original paradigm, this would have required too many trials (given the eye position recording method used). We thus limited ourselves to those trial types that involved targets A and B. This made it impossible to run our original analysis, and thus to statistically validate either hypothesis. However, it allowed us to investigate the time course of the changes in saccade end points, for both single saccades and sequences. In Figure 11, we report these measures, which are the same as plotted in the left column in Figures 6 and 10. In both cases, the adaptation washout seemed relatively fast (black lines), certainly faster than the adaptation-induced amplitude increase in this subject (compare with first row in Figure 10). The second saccade end points (green lines) followed approximately the same time course, so that both effects were mostly washed-out by the end of the session. Note that in this experiment we also had to reduce the number of trials in the post-adaptation phase (which were now two, one after the adaptation and one after the washout).

Figure 11.

Time course of adaptation-induced amplitude shortening and washout. We tested one subject (S1) in an experiment in which we first reduced the size of saccades aimed at B (by intra-saccadically displacing the target toward the fixation point), and then washed-out this adaptation by stopping the intra-saccadic displacement. A reduced paradigm was used, excluding trial types that involved targets C and D. Here we plot data from two sessions, one in the CRT setup (left) and one in the tangent screen setup (right). Washout was relatively fast and complete and affected the end points of both single (black) and double (green) saccades. In the tangent screen setup, toward the end of the session coil discomfort ensued, resulting in very few valid double-saccade trials. Three blocks of increased double-saccade trials (gray bars) flanked blocks with a preponderance of adaptation trials.

Discussion

The goal of this study was to shed some light on how the saccadic vectors for individual saccades in a sequence could be generated. We outlined what we regard as the most sensible alternatives: the goal updating hypothesis and the motor updating hypothesis. Under the former, an estimate of the location (relative to the fovea) of the goal for the second saccade is updated after the first saccade, and a saccade to this goal is then generated. Under the latter, movement vectors to both targets are preprogrammed, and the second movement vector is updated following the first saccade.

We carried out an experiment that, by combining two paradigms (FTD and PTD), tested the two hypotheses in human subjects at the same time. While the results we obtained are not as clear-cut as we would have hoped, amplitude reduction experiments pointed decisively in the direction of the motor updating hypothesis. Notably, not once did we find positive evidence (i.e., a statistically significant change in the second saccade end points under the PTD paradigm) in favor of the goal updating hypothesis. Furthermore, in four out of five subjects we observed large changes under the FTD paradigm, predicted by the motor, but not the goal, updating hypothesis. This occurred regardless of the presence of potential additional visual references. Our results thus suggest that the human saccadic system is capable of pre-computing two motor commands, and updating the second after the first movement has been executed. This is compatible with, and further extends, previous suggestions that two saccadic eye movements can be planned and (partially) executed in parallel (Becker, 1989). Whether this result generalizes to other sequences of saccades, or is instead limited to our experimental conditions, remains to be seen.

We are quite troubled by our inability to find evidence in support of either hypothesis in the amplitude lengthening experiments. We believe that we were particularly hampered by our need to intermix adaptation trials with other trial types, since this has been shown to considerably slow down the speed of adaptation (Miller et al., 1981; Scudder, Batourina, & Tunder, 1998; Straube & Deubel, 1995). It is certainly possible that, given the small amplitude changes that we induced, noise masked any effect on saccade sequences in the FTD paradigm. Nonetheless, we would have expected to see at least some change in subjects S1 and S2, who exhibited robust changes in the gain reduction experiments. Instead, the small changes observed were no different from those observed when the experiments were run without applying any intra-saccadic step to target B (control experiment on three subjects, data not shown). We do not have any definitive explanation for this behavior. However, there is mounting evidence to suggest that amplitude decreases and increases engage different adaptive mechanisms (Ethier, Zee, & Shadmehr, 2008a; Hernandez, Levitan, Banks, & Schor, 2008; Noto et al., 1999; Panouilleres et al., 2009; Semmlow et al., 1989; Straube & Deubel, 1995; Tian, Ethier, Shadmehr, Fujita, & Zee, 2009; Zimmerman & Lappe, 2010). In particular, there is evidence (Ethier et al., 2008a; Semmlow et al., 1989; Zimmerman & Lappe, 2010), at least in humans, to indicate that while amplitude decreases are accomplished by adaptively altering the motor plan associated with a desired eye displacement (i.e., the mapping of the goal vector into a movement vector), amplitude increases are accomplished by altering the desired eye displacement itself (i.e., the mapping of the retinal vector into a goal vector). Under this hypothesis, a lack of an effect in the amplitude increase experiments would be expected if this proposed remapping of the desired eye displacement does not take place in the context of a planned sequence (there is no transfer of adaptation across the two saccade types). Experiments of longer duration probably necessitating different eye movement recording techniques will be needed to clarify this issue.

We will now discuss the limits of our approach, compare our results with those obtained in previous studies, and discuss possible alternative interpretations of our results. Finally, we will illustrate a prediction of the motor updating hypothesis.

Vector dissociation

Both our experimental tests (FTD and PTD paradigms) relied on a dissociation between motor and sensory vectors. As far as we know, the only non-invasive means to induce such a dissociation is McLaughlin’s intra-saccadic target step paradigm (Hopp & Fuchs, 2004; McLaughlin, 1967; Pelisson, Alahyane, Panouilleres, & Tilikete, 2010). We believe that there is substantial evidence to suggest that, at least under our experimental conditions, this technique affects mostly the transformation of the goal vector into the movement vector, and only mildly (if at all) the transformation of the retinal vector into the goal vector. Because others either disagree or consider the evidence ambiguous (e.g., Awater, Burr, Lappe, Morrone, & Goldberg, 2005; Bruno & Morrone, 2007; Collins et al., 2007; Collins, Rolfs, Deubel, & Cavanagh, 2009; Georg & Lappe, 2009; Zimmermann & Lappe, 2009), we will outline our reasoning.

First, if motor adaptation simply reflected a changed association between the location of the target and its internalized goal location, one would expect the speed of a saccade to depend only on the goal location, and thus on the actual amplitude of the movement. However, in humans amplitude-shortening adaptation affects the kinematics of same-size saccades (Ethier et al., 2008a; Zimmerman & Lappe, 2010). Furthermore, since the various retinotopic goal maps are heavily interconnected, if the internalization of visual targets onto retinotopic maps were altered it might be expected that perception (in the absence of saccades) would be affected by saccadic adaptation. However, it has been shown repeatedly that perception during fixation is not, or only marginally, affected by adaptation (Awater et al., 2005; Bahcall & Kowler, 1999; Collins et al., 2007; Georg & Lappe, 2009; Moidell & Bedell, 1988; Zimmerman & Lappe, 2010). The recent experiment by Zimmerman and Lappe (2010) is particularly relevant for our study. Using similar target eccentricities, intra-saccadic adaptation-inducing steps, and overall experimental conditions, they reported a robust perceptual change following amplitude lengthening, but no change following amplitude shortening. By altering McLaughlin’s adaptation paradigm to impose a constant post-saccadic visual error (instead of using a fixed intra-saccadic step), they further showed that mislocalization during fixation arises whenever a large post-saccadic error is present over the course of many (hundreds) trials. They thus suggested that with the classic McLaughlin’s paradigm mislocalization during fixation is not observed during amplitude-reducing experiments because of the fast time course of adaptation, which prevents the persistence of large post-saccadic errors. In this framework, adaptation and mislocalization during fixation can thus be seen as two separate processes: the former is driven by any post-saccadic error, whereas the latter requires large and persistent post-saccadic errors (which were not present in our experiments).

Similarly, limb movements might also be expected to be affected by adaptation. Here the picture is not as clear. Some studies reported no, or only very small (less than 20% of the change in saccade size), changes in pointing movements (with an unseen hand) after saccade adaptation (de Graaf, Pélisson, Prablanc, & Goffart, 1995; Hernandez et al., 2008; Kröller, De Graaf, Prablanc, & Pélisson, 1999; McLaughlin, Kelly, Anderson, & Wenz, 1968). Another study found significant changes only when the adapted saccades were very large (30°) and aimed at stable targets; for smaller saccades, or when saccades to jumping targets were adapted, no changes in pointing movements were reported (Cotti, Guillaume, Alahyane, Pelisson, & Vercher, 2007). Note that the vast majority of natural human saccades, and all the saccades studied in our experiment, are smaller than 15° (Bahill, Adler, & Stark, 1975). In contrast, three studies have reported a considerable change in pointing behavior (Bekkering, Abrams, & Pratt, 1995; Bruno & Morrone, 2007; Kröller et al., 1999). Importantly, in these three experiments the pointing movement was not executed during fixation; instead, the adapted saccade was executed before, or together with, the pointing movement. This brings us to a series of experiments that have shown that saccade adaptation can affect the localization of a probe, but again only when the probe is presented pre-saccadically, and its location is reported post-saccadically (Awater et al., 2005; Bahcall & Kowler, 1999; Collins et al., 2007, 2009; Georg & Lappe, 2009; Zimmermann & Lappe, 2009). It has long been known that targets flashed right around (±50 ms) saccade onset are mislocalized (e.g., Matin & Pearce, 1965; Ross, Morrone, & Burr, 1997), but these studies revealed that even probes flashed long before (up to 1 s; Georg & Lappe, 2009) a saccade are mislocalized after adaptation (again, provided that the adapted saccade is made before the perceptual report). The most interesting result is arguably the one reported by Collins et al. (2007). Unlike most experiments, in which probes are only placed along the fixation point-target axis, they probed a wide swath of the visual field. They found that the pattern of mislocalization resembled the adaptation field (Albano, 1996; Deubel, 1987; Deubel et al., 1986; Frens & van Opstal, 1994; Miller et al., 1981; Noto et al., 1999; Semmlow et al., 1989), so that the perceived location of the probe, reported after the adapted saccade was generated, was deviated toward the location where a direct saccade to the probe would bring the eyes. This finding has led to the proposal that adaptation might not only affect the conversion of a goal vector into a motor vector, but to a large extent also the conversion of the visual vector into the goal vector, restructuring perceptual space (Collins et al., 2007, 2009; Georg & Lappe, 2009; Zimmermann & Lappe, 2009).

To summarize, the idea that saccadic adaptation acts by changing the transformation of a retinal vector into a goal vector is supported mostly by the observed trans-saccadic perceptual mislocalization. As also argued by others (Awater et al., 2005), this goal remapping hypothesis is, however, not sufficient to account for all the data. First, the perceptual effect is always a fraction of the motor effect, hence at best one could argue for a dual effect of adaptation. Second, the perceptual effects are only seen if the perceptual report follows a saccade (actually the adapted saccade), whereas if goal remapping underlied adaptation they would be expected also during fixation. Finally, this theory makes a clear prediction regarding the kinematics of saccades, which as noted above has been repeatedly shown not to hold.

It is worth pointing out that goal remapping is also not necessary to account for the above-mentioned trans-saccadic mislocalization, since the motor updating hypothesis could account for those results as well. We will now briefly outline an alternative explanation for those findings. We start by assuming that gain-down adaptation affects only the transformation of the goal vector into the movement vector, and not at all the sites activated in goal/perceptual maps. Obviously, it would not be unreasonable for adapted saccades to be kinematically different than same-size pre-adaptation saccades. We now make two additional assumptions regarding experiments in which subjects are asked to report the location of a briefly flashed probe. The first is that subjects always automatically plan a saccade to the probe (in addition to a saccade to the target, when present). The second is that to localize the probe they combine information from two sources: the site activated by the probe in perceptual/goal maps and the motor plan for the saccade to the probe. The information from the two sources could be combined according to their relative reliability (e.g., in a Bayesian manner). When a probe is flashed during fixation, the information from perceptual maps is deemed more reliable, and hence, the report is not, or is only marginally (Moidell & Bedell, 1988), affected by adaptation. After the saccade to the target is made, information in the perceptual maps is updated (i.e., remapped), and so is the motor vector for the implicitly planned saccade to the probe, in accordance with the motor updating hypothesis. It is safe to assume that these updating operations degrade the information, making it less reliable. Accordingly, when the report is made after a saccade both sources of information are used to localize the probe. This theory then predicts that (1) the impact of adaptation on perceptual mislocalization should be only a fraction of the saccadic effect (the more the motor information is weighted, the larger the effect), and (2) the pattern of perceptual localization should mirror the adaptation field. This mechanism could thus account for all the findings mentioned above, without invoking any effect of adaptation on goal/perceptual maps.

It must be pointed out that the majority of the experiments mentioned above studied reactive saccades, i.e., saccades made to suddenly displaced targets. In contrast, all the saccades in our study were voluntary. Reactive and voluntary saccades have a lot in common, but there is considerable evidence (recently reviewed by Pelisson et al., 2010) that they might engage partially distinct neural circuits. However, it should be noted that the minority of probe localization experiments that studied adaptation of voluntary saccades (Bekkering et al., 1995; Collins et al., 2007; Cotti et al., 2007; McLaughlin et al., 1968) reported results that were in line with those obtained with reactive saccades, at least under experimental conditions (amplitude-shortening adaptation, size of saccades, and size of the intra-saccadic target step) similar to ours. Furthermore, as previously mentioned, there is evidence to suggest that mislocalization during fixation arises when a large post-saccadic visual error is present over many trials (Zimmerman & Lappe, 2010), and it is thus tied to the time course of adaptation. Since the time course of amplitude-shortening adaptation is the same for reactive and voluntary saccades (Alahyane et al., 2007), and was certainly fast in our experiments, the conditions that appear to lead to probe mislocalization did not arise during our experiment. Nevertheless, additional work that directly compares perceptual mislocalization patterns following voluntary and reactive saccade adaptation would be desirable.

McLaughlin’s adaptation paradigm

This uncertainty about what is being dissociated is not the only limit of McLaughlin’s intra-saccadic step paradigm. In addition, the results yielded by it are, especially in human subjects, neither particularly robust nor highly repeatable. For example, it is well known that, for a given set of experimental conditions, the speed and extent of adaptation varies widely across subjects, and in the same subject across sessions (Albano & King, 1989; Becker & Fuchs, 1969; Chaturvedi & van Gisbergen, 1997; Fujita, Amagai, Minakawa, & Aoki, 2002). It has also been commonly reported that reducing the amplitude of saccades is easier (i.e., yields faster and larger changes) than increasing it (Deubel, 1987; Deubel et al., 1986; Miller et al., 1981). However, sometimes the rate of adaptation has been reported to be the same in the two conditions, with differences only in the extent of the change (Semmlow et al., 1989); in other studies, no significant differences were observed between the two conditions (Albano, 1996; Bahcall & Kowler, 2000; Ethier, Zee, & Shadmehr, 2008b). Yet, others have reported that it is actually the other way around: it is easier to increase than to decrease the amplitude (Alahyane & Pélisson, 2004; Albano & King, 1989). Similarly, it is usually stated that adaptation is a gradual process. Yet, there are countless examples of virtually instant adaptation: for example, in McLaughlin’s original study complete adaptation was achieved on the third trial (McLaughlin, 1967). In McLaughlin’s next study (McLaughlin et al., 1968), the amplitude was reduced by 40% in less than 5 trials. More recent examples of such super-fast adaptation abound (Erkelens & Hulleman, 1993; Ethier et al., 2008b; Fujita et al., 2002; Moidell & Bedell, 1988; Semmlow et al., 1989). Even more important, with this technique the extent of the adaptation is context-dependent, especially in humans. For example, when the amplitude of visually guided saccades is changed, the amplitude of memory-guided saccades is only partially modified, and vice versa (Deubel, 1995b; Hopp & Fuchs, 2004, 2010; Pelisson et al., 2010). It is thus said that the transfer of adaptation is incomplete. Once again, different studies report quantitatively different results.

The limits of this dissociation technique are clearly reflected in our results: although we managed to consistently reduce the amplitude of saccades, the degree varied considerably across subjects and to a lesser extent across sessions for the same subject. Increasing the amplitude of saccades proved much harder. It must, however, be noted that our paradigm included several different trial types, and not just adaptation trials. Most likely, this negatively affected both the adaptation rate and extent (Miller et al., 1981; Scudder et al., 1998; Straube & Deubel, 1995).

In our experiments, the issue of adaptation transfer was particularly important, since we applied McLaughlin’s paradigm to one type of saccades (usually referred to as visually guided delayed, or overlap, saccades) and tested the effect of this dissociation on the second saccade in a sequence (memory-guided, executed in darkness). We found that in some subjects the effect of adaptation transferred fully from one saccade type to the other under the FTD paradigm (even though the saccade vector was different for the two saccades). However, in one subject (S5) this transfer was quite limited, making the goal/motor dissociation only marginally effective (Figures 5–7). Subject S3 was particularly interesting, since his behavior changed over time: in his first session, he showed a considerable amount of transfer, which disappeared in a later session (Figure 7).

It is often suggested that the limited, and asymmetric, adaptation transfer might be due to a set of partially overlapping neural circuits, which are differently engaged during the generation of different saccade types (Alahyane, Fonteille et al., 2008; Alahyane et al., 2007; Erkelens & Hulleman, 1993; Gancarz & Grossberg, 1999; Hopp & Fuchs, 2004, 2010; Pelisson et al., 2010; Straube, Deubel, Spuler, & Buttner, 1995). While it is certainly true that the neural circuitry involved in the generation of saccades varies across saccade types (Gaymard, Ploner, Rivaud, Vermersch, & Pierrot-Deseilligny, 1998; Moschovakis, Scudder, & Highstein, 1996; Tusa, Zee, & Herdman, 1986), this observation is not sufficient to explain the large variability across subjects, and our finding that the amount of transfer can change across sessions, a phenomenon observed also in monkeys (Fuchs, Reiner, & Pong, 1996). One hypothesis that might account for these findings was put forward by Edelman and Goldberg (2002). They proposed that the amplitude of a saccade is determined by the cerebellum upon recognition of a specific pattern of activity on its inputs. Under this hypothesis, differences in the extra-cerebellar circuits engaged by the various saccade types are relevant only to the extent that they result in patterns of cerebellar afferent activations that are recognized as being different. The advantage of this theory is that it readily accounts for the selective ability to use external cues to induce context-specific adaptation (Aboukhalil, Shelhamer, & Clendaniel, 2004; Alahyane & Pélisson, 2004; Bahcall & Kowler, 2000; Chaturvedi & van Gisbergen, 1997; Deubel, 1995a, 1995b; Fuchs et al., 1996; Herman, Harwood, & Wallman, 2009; McLaughlin, 1967; Melis & van Gisbergen, 1995; Shelhamer & Clendaniel, 2002a, 2002b): only cue-related information that reaches the cerebellar afferents can be effective, and only if it is recognized as task-relevant. The higher contextual sensitivity of saccadic adaptation in humans than in monkeys (Fuchs et al., 1996; Hopp & Fuchs, 2004; Pelisson et al., 2010) could then be due to the vast expansion of the cerebellum itself, and of its interconnectivity with the rest of the brain, in the evolution of humans (Macleod, Zilles, Schleicher, Rilling, & Gibson, 2003; Whiting & Barton, 2003). The time-varying results in S3 might then simply reflect a change in the subject’s internal context (e.g., how he thought about the experiment); while highly speculative, this seems more likely than the engagement of different neural circuits across sessions.

Comparison with previous studies

As far as we know, only two papers have directly addressed the impact of saccadic adaptation on the second saccade in a sequence. The first study, by Frens and van Opstal (1994), represents one of the most extensive investigations of the properties of saccade adaptation in humans. Besides many other experiments, they also used two paradigms that are quite similar to ours. One paradigm (which they call configuration A) is analogous to our FTD paradigm (Figure 2E), but they reported only a very small adaptation-induced change in the end points of the second saccades (i.e., evidence for the goal updating hypothesis). There are two issues that might account for our conflicting results. First, as reported in the Results section, we also had subjects (S5 and later on S3) that behaved similarly, and we explained already how this finding cannot be construed as conclusive evidence in favor of the goal updating hypothesis. Furthermore, in one of their three subjects, they actually observed a sizable change in end points (corresponding to a GMUI value of approximately 0.65, which should be classified as evidence for the motor hypothesis). Second, there are some differences in the experimental setup that might also play a role. For example, in their experiments targets were placed at a considerably larger eccentricity (21°) than ours (around 10°), and were flashed for a very brief time (80 ms). This most likely made an accurate localization difficult to achieve, and the subjects might well have relied on their long-term memory of target location. This is all the more likely since during the preceding adaptation phase saccades were directed to the same location in space as the second target in the sequences, over and over without any other intermixed trial types. It is thus hard to rule out that (some of) their subjects, instead of pre-programming two saccades, made a first saccade followed by a second independently programmed saccade, directed by their long-term memory of that location in space. As noted by Hopp and Fuchs (2002), “subjects may have been able to predict where the target would appear.” In our experiments, we ruled this out by jittering the location of the target for the second saccade, which allowed us to strictly verify the compliance of the subjects with our instructions.

Frens and van Opstal also used another paradigm (configuration B), which is similar to our PTD paradigm (Figure 2F). They reported that in this case the end point of second saccades changed, evidence in favor of the goal updating hypothesis. However, we think that there are some problems with this interpretation. First of all, the change measured was far short of that predicted by the goal updating hypothesis (corresponding to GMUI values of approximately 0.55 and 0.65 in two subjects; in the third we could not compute an estimate for lack of the needed information). Most importantly, the end points of control saccades aimed directly at the final target (FP–D in Figure 2F) were not measured; if the amplitude of these saccades had been reduced during the adaptation phase, the partial change that they reported might actually match the motor hypothesis prediction. This is compatible with the configuration of their targets and the extent of the so-called adaptation field (Albano, 1996; Deubel, 1987; Deubel et al., 1986; Frens & van Opstal, 1994; Miller et al., 1981; Semmlow et al., 1989), which was actually reported by Frens and van Opstal in their study. In fact, their Figure 5 provides strong support for our speculation that the end points of saccades aimed directly at the second target in the sequence were most likely affected by the adaptation. Note that the approximate GMUI values we reported above underestimate the actual ones, since we estimated them without taking into account the effect of the adaptation field. Finally, the issue of how the saccades were planned and executed could have had a significant impact in this case, too. If the second saccade in a sequence was programmed as a single saccade toward a remembered location in space, support for the goal hypothesis would have been expected. These arguments call into question the conclusion reached by Frens and van Opstal.

In monkeys, Wallman and Fuchs (1998) applied the PTD paradigm (Figure 2F) and reported strong evidence in favor of the goal updating hypothesis. However, the paradigm used by Wallman and Fuchs is fundamentally different from ours. In their experiment, the target for the second saccade was turned on immediately before the first saccade (based on the average latency of each particular monkey) and was extinguished when the eye speed exceeded a threshold. Unlike our subjects, their monkeys were thus not intentionally planning two saccades but were instead coaxed into making two saccades by presenting another target when it was too late for them to cancel the first movement (the monkeys were rewarded for looking at the target, not for making two saccades). This paradigm has been used for a long time to study the ability to cancel one planned action in favor of another one (Becker & Jurgens, 1975, 1979; Findlay & Harris, 1984; Hallett & Lightstone, 1976; Hou & Fender, 1979; Levy-Schoen & Blanc-Garin, 1974; Lisberger, Fuchs, King, & Evinger, 1975; Westheimer, 1954; Wheeless, Boynton, & Cohen, 1966), and it has revealed the capacity of the saccadic system to plan two saccades in parallel (i.e., not strictly sequentially). In our experiments, subjects were instead clearly instructed to plan and execute two distinct saccades, sequentially. Recently, it has been shown that, at least in humans, paradigm differences similar to these are sufficient to elicit distinct behaviors and might engage different neural circuits (Ray, Schall, & Murthy, 2004). This methodological difference could then very well account for the difference in outcome: our two studies might have probed different behaviors. Another possibility is that there is a species difference: saccadic adaptation in humans might be different than in monkeys, as argued by others (Doré-Mazars et al., 2006; Fuchs et al., 1996; Gancarz & Grossberg, 1999; Hopp & Fuchs, 2004; Pelisson et al., 2010). There certainly is evidence to support this possibility. For example, it is generally agreed that: (1) adaptation is much faster in humans (Albano & King, 1989; Deubel, 1987; Tian et al., 2009); (2) the super-fast adaptation (complete adaptation in a handful of trials, see above) observed sometimes in humans is never observed in monkeys; (3) the transfer of adaptation across different saccade types is much more complete and symmetric in monkeys (Fuchs et al., 1996; Hopp & Fuchs, 2004; Pelisson et al., 2010); (4) gain increases are always much harder to induce in monkeys, whereas in humans very different results have been reported (reviewed above).

Two other pertinent studies are those conducted by Tanaka (2003) in monkeys and Doré-Mazars et al. (2006) in humans. Both studies concluded that when the first saccade in a sequence ( $\overrightarrow{M1}$) is adaptively modified, the second saccade ( $\overrightarrow{M{2}^{\ast }}$) partially compensates for the adaptation-induced change. While this ruled out the ballistic programming hypothesis (Equation 4), it also seemed to indicate that only part of the change in the first saccade vector was accounted for when planning the second saccade. In other words, Equation 6 (i.e., the goal updating hypothesis) seems to only partially hold. It should, however, be noted that in neither of these experiments direct saccades to the second targets were tested. Given how the two targets were arranged, it is actually quite likely that the second target fell in the adaptation field. What was reported as a partial compensation might then in fact have been a perfect match for the motor updating hypothesis (Equation 7). Some support for this interpretation comes from the following observation. In Tanaka’s experiments, before adaptation the monkey was required to make a horizontal saccade followed by a vertical one. After adaptation, the second saccade acquired a horizontal component, which partially compensated for the shortening of the first horizontal saccade. Under these conditions, the motor updating hypothesis makes a clear prediction: if different amplitudes of vertical saccades are tested, larger vertical saccades should be associated with larger (post-adaptation) horizontal components for the second saccade (because their end points lie further away from the center of the adaptation field). Interestingly, multiple amplitudes of the vertical saccade were in fact tested in the course of the experiment, and although the results were combined in the final paper, a subsequent analysis revealed the pattern predicted by the motor updating hypothesis (Masaki Tanaka, personal communication). Notably, the monkeys in Tanaka’s experiment were instructed, and rewarded, to make a sequence of two saccades; the task is thus similar to ours, and unlike that used by Wallman and Fuchs.

Finally, in the discussion of an unrelated study, Hopp and Fuchs (2002) mentioned that they attempted to apply the PTD paradigm in humans, but the results were inconsistent and did not reach statistical significance. As noted above, this lack of robustness, largely imputable to the variable nature of the adaptation technique employed, also plagued our experiments. It was only due to a carefully tailored experimental design, in part inspired by Hopp and Fuchs’s observations, that we managed to enforce a strictly controlled behavior, increasing the signal-to-noise ratio to an acceptable level, at least in the amplitude-shortening experiments.

Alternative explanations

We interpreted our results as supportive of the motor updating hypothesis, within the context of pre-planned saccadic sequences. There are, however, two other theories that might, in principle, account for our data.

First, one could hypothesize that saccade adaptation operates in head-centric coordinates (i.e., relative to the head, not to the fovea), so that what is adapted is not a saccadic vector, but rather the association between the location of a target in head coordinates and the muscle innervation required to move the eye to that orbital location. There is, however, overwhelming evidence that saccade adaptation, at least of the kind induced through McLaughlin’s paradigm, acts predominantly in retinocen-tric (i.e., at the vector level), and not head-centric, coordinates (Albano, 1996; Deubel, 1995a; Noto et al., 1999; Shelhamer & Clendaniel, 2002a). Nonetheless, it has been shown that the position of the eye in the orbit can act as a contextual cue, so that the same saccadic vector can be adapted differently depending on orbital position (Aboukhalil et al., 2004; Alahyane & Pélisson, 2004; Shelhamer & Clendaniel, 2002a, 2002b). One might then be tempted to conclude that this could account for our finding that in the PTD paradigm the amplitude of the second saccades in our FP–C–D sequences did not follow the changes of the amplitude of the FP–B saccades: the saccade vectors were the same, but the orbital position was not. However, the above-mentioned studies revealed that, to induce orbital position-specific adaptation, the two saccades must receive independent, and different, visual feedback. We never provided any visual feedback about the accuracy of double saccades. Importantly, we did not even provide any feedback for the saccades aimed directly at target D, thus ruling out the possibility of any eye position-related learning. Because of the above-mentioned sensitivity of saccadic adaptation to the experimental conditions, we were however encouraged by a reviewer to verify the effect of orbital position on adaptation in the context of our experiment. We thus modified our paradigm by replacing saccades aimed directly at target A with saccades aimed directly at target C. In a fraction of these trials, we lit target D as soon as the saccade to C was detected. The subjects were instructed to look as quickly as possible to a target that suddenly appeared. The screen was blanked as soon as the saccade toward target D was detected. We found that, as in our other experiments, C–D saccades that were part of FP–C–D sequences were not significantly affected by the adaptation, whereas A–B saccades within FP–A–B sequences tracked the changes in FP–B (adapted) saccades. Crucially, the amplitude of the newly introduced visually guided C–D saccades also tracked the changes of the FP–B saccades. The magnitude of the changes (which were always highly significant, with no overlap between the pre-adaptation and post-adaptation end-point distributions) averaged 75% of the changes in FP–B saccades. Orbital position dependency thus cannot account for the lack of effect in the PTD paradigm in our experiments.

A less strict version of the head-centric theory, which postulates that a sort of head-centric adaptation could persist shortly after a saccade, could in principle account for the changes we observed in the FTD paradigm. More precisely, the remapping of visual space into retinotopic coordinates that must follow each saccade (Cavanagh, Hunt, Afraz, & Rolfs, 2010; Klier & Angelaki, 2008; Wurtz, 2008) could be sluggish. To test this hypothesis, we performed another control experiment, analogous to the one just mentioned. In this case right after a subject started a single saccade toward target A, we lit target B. As soon as the subject initiated a saccade toward target B, the screen was blanked. Under the “sluggish updating” hypothesis, this saccade might be expected to be similar to the second saccade in the FP–A–B sequences, thus exhibiting adaptation-induced changes (Figure 3D). Two subjects performed this experiment, and in neither was this borne out. The saccades to the flashed target B were equally accurate before and after adaptation, in spite of obvious changes in the second saccade in the FP–A–B sequences. The latencies for these visually guided second saccades were quite long (the median was 367 ms in one subject and 379 ms in the other), which is not unexpected since these trials were quite rare (<5%) and essentially caught the subjects by surprise. However, these latencies were well within the range of the inter-saccadic interval between two saccades in a sequence.

Our results could also be easily explained by assuming that McLaughlin’s paradigm affects the relationship between retinal and goal vectors, changing the perception of where targets are, in retinotopic coordinates. The predictions of such a hypothesis would be, as far as sequence end points are concerned, indistinguishable from those made by the motor updating hypothesis (Figure 2H), and thus would match the outcome of our experiments. As discussed at length above, our reading of the literature does not support this hypothesis. However, as we already mentioned, a large fraction of the data that bears on this issue comes from experiments that relied on adaptation of reactive saccades. The few experiments that used experimental conditions similar to ours generally confirmed those findings, but additional experiments would certainly be welcome.

Prediction

Differences between the predictions made by the goal and motor updating hypothesis are not limited to the paradigms above described. One particularly interesting task does not involve saccadic adaptation at all but relies instead on the observation that when different sequences of saccades share a common final target, the end points of the sequences often do not coincide (Karn, Moller, & Hayhoe, 1997). We think that the well-known tendency of human subjects to make, within the impoverished visual environment typical of an experimental setup, saccades smaller than required (10% saccadic undershoot; Becker, 1989; Henson, 1978, 1979) might account for this finding. More importantly, if this is the case the goal and motor updating hypotheses make different predictions regarding the end points of sequences that have different starting points, and a common final target. Suppose, for example, that three targets are arranged as the vertices of a triangle (Figure 12). If A is used as the fixation point and C is used as the target, because of the aforementioned saccadic undershoot A–C saccades will tend to land between A and C. Similarly, if B is the fixation point and C is the target, B–C saccades will tend to land between B and C (gray dashed arrows). Suppose now that A is the fixation point, and that a sequence of two saccades, first to B and then to C, is executed (Figure 12A). Where will the eyes finally land? According to the goal updating hypothesis (red double arrow), they should land between B and C (because the second saccade in the sequence is B–C). Their end points should thus cluster with those of single saccades starting from B. However, according to the motor updating hypothesis (blue double arrow) they should land between A and C, i.e., where a saccade directly from A to C ends. Their end points should thus cluster with those of single saccades starting from A. An analogous argument can be made about the end points of B–A–C sequences (Figure 12B).

Figure 12.

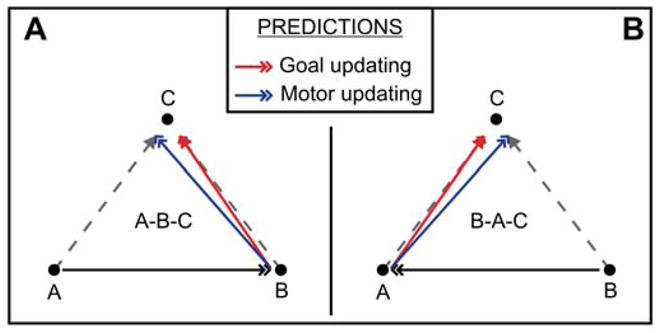
Additional prediction of the motor updating hypothesis. Implications of the systematic saccadic undershoot observed in human subjects when their eye movements are measured in an impoverished visual environment. Saccades aimed at a single target (gray dashed arrows) do not reach the target but fall short, so that the landing position is between the fixation point and the target. Accordingly, both the goal and motor updating hypotheses predict that sequences of saccades starting from different initial positions but sharing the same final target will have different landing positions. (A) Predictions (colored double arrows) of the two hypotheses for A–B–C sequences. (B) Predictions for B–A–C sequences. Note that in both cases the goal updating hypothesis predicts that sequences that start on the left end up on the right of the final target, and vice versa. In contrast, the motor updating hypothesis predicts that saccades that start on the left (right) end up on the left (right).

Future directions

Our results highlight the need for some additional experiments. First, it would be useful to measure the effect of amplitude increasing adaptation on sequences of saccades, with our paradigm. Second, it would be extremely important to know whether our results are limited to our experimental conditions, or can be generalized to sequences of saccades generated under different conditions. In a pilot study, we found the same behavior reported here if the two targets for the saccade sequence are presented simultaneously, and either color or luminance is used to indicate the first target in the sequence. Using a richer display would, however, be more informative. Finally, it is crucial to have human subjects behave in a modified version of our paradigm that mimics the paradigm used by Wallman and Fuchs (1998) in monkeys. This would provide valuable insights into the different way in which planned and corrective saccades are generated, and possibly in the differences between saccades planned sequentially and concurrently. This comparison could then also be extended to the generation of multiple saccades aimed at a single target (Crawford, 1991; van Donkelaar, Saavedra, & Woollacott, 2007). Conversely, it would be extremely useful to know how monkeys behave in our paradigm. Were monkeys to behave in our paradigm as they behave in the paradigm used by Wallman and Fuchs (1998), then it would become of paramount importance to apply to monkeys the perceptual paradigms that in humans have been shown to be affected by adaptation. Since most of our understanding of the neurophysiology of the oculomotor system comes from monkeys, whereas most of the behavioral experiments are carried out in humans, any proven species difference would be extremely important.

Appendix A

Our main analysis compares the adaptation-induced changes in landing position of the second saccade in a sequence with those predicted by two hypotheses. In general, we have that the landing point of the second saccade can be described as

$$\mathbf{L}{\mathbf{P}}_{\mathbf{2}}=\mathbf{FP}+\overrightarrow{M1}+\overrightarrow{M{2}^{\ast }}.$$ (A1)

The effect of adaptation will manifest itself as follows:

$$\mathbf{\Delta }\mathbf{L}{\mathbf{P}}_{\mathbf{2}}=\mathrm{\Delta }\overrightarrow{M1}+\mathrm{\Delta }\overrightarrow{M{2}^{\ast }}.$$ (A2)

According to the motor updating hypothesis (Equation 7),

$$\mathrm{\Delta }\overrightarrow{M{2}^{\ast }}=\mathrm{\Delta }\overrightarrow{M2}-\mathrm{\Delta }\overrightarrow{M1}.$$ (A3)

Thus,

$$\mathbf{\Delta }\mathbf{L}{\mathbf{P}}_{\mathbf{2}}^{\mathbf{m}}=\mathrm{\Delta }\overrightarrow{M2}.$$ (A4)

Under the motor updating hypothesis, the end-point LP₂ changes just like the vector of saccades aimed directly at the second target T2.

According to the goal updating hypothesis (Equation 6),

$$\mathrm{\Delta }\overrightarrow{M{2}^{\ast }}=f_{\text{Mpost}}(\overrightarrow{G2}-\overrightarrow{M{1}_{\text{post}}}-f_{\text{Mpre}}(\overrightarrow{G2}-\overrightarrow{M{1}_{\text{pre}}}).$$ (A5)

Before adaptation (pre), $\overrightarrow{M1}=\overrightarrow{G1}$, and thus

$$f_{\text{Mpre}}(\overrightarrow{G2}-\overrightarrow{M{1}_{\text{pre}}}=f_{\text{Mpre}}(\overrightarrow{G2}-\overrightarrow{G1})=f_{\text{Mpre}}(\overrightarrow{G3})=\overrightarrow{M{3}_{\text{pre}}}.$$ (A6)

If the effect of adaptation is limited to $\overrightarrow{M2}$ and/or $\overrightarrow{M3}$ and does not affect $\overrightarrow{M1}$, we could similarly conclude that

$$f_{\text{Mpost}}(\overrightarrow{G2}-\overrightarrow{M{1}_{\text{post}}})=\overrightarrow{M{3}_{\text{post}}},$$ (A7)

from which, since we have assumed $\mathrm{\Delta }\overrightarrow{M1}=0$, it would follow that

$$\mathbf{\Delta }\mathbf{L}{\mathbf{P}}_{\mathbf{2}}^{\mathbf{g}}=\mathrm{\Delta }\overrightarrow{M3}.$$ (A8)

LP₂ would thus change just like the vector of saccades aimed directly at target T3.

When adaptation-induced changes to $\overrightarrow{M1}$ are not small enough to be ignored, the prediction of the goal updating hypothesis becomes slightly less straightforward. Equation A6 would still hold, but Equation A7 would have to be replaced with

$$\begin{array}{l}{f}_{\text{Mpost}}(\overrightarrow{G2}-\overrightarrow{M{1}_{\text{post}}})=f_{\text{Mpost}}(\overrightarrow{G2}-\overrightarrow{M{1}_{\text{pre}}}-\mathrm{\Delta }\overrightarrow{M1})\\ =f_{\text{Mpost}}(\overrightarrow{G3}=-\mathrm{\Delta }\overrightarrow{M1}).\end{array}$$ (A9)

Because in our experiments we never observed adaptation-induced directional changes in saccades aimed at a single target, within a small range around $\overrightarrow{G3}$, we can consider f_M as a scaling factor k, i.e.,

$$\overrightarrow{M{3}_{\text{post}}}=f_{\mathit{Mpost}}(\overrightarrow{G3})=k\overrightarrow{G3}.$$ (A10)

Since in our experiments $\mid \overrightarrow{G3}\mid >10\mid \mathrm{\Delta }\overrightarrow{M1}\mid$ in all sessions, given the known size and properties of the adaptation field, we can approximate Equation A9 as follows:

$$f_{\text{Mpost}}(\overrightarrow{G3}-\mathrm{\Delta }\overrightarrow{M1})=\overrightarrow{M{3}_{\text{post}}}-k\mathrm{\Delta }\overrightarrow{M1}.$$ (A11)

It follows that

$$\begin{array}{l}\mathbf{\Delta }\mathbf{L}{\mathbf{P}}_{\mathbf{2}}^{\mathbf{g}}=\mathrm{\Delta }\overrightarrow{M1}+\mathrm{\Delta }\overrightarrow{M{2}^{\ast }}=\mathrm{\Delta }\overrightarrow{M1}+\overrightarrow{M{3}_{\text{post}}}-k\mathrm{\Delta }\overrightarrow{M1}-\overrightarrow{M{3}_{\text{pre}}}\\ =\mathrm{\Delta }\overrightarrow{M3}+(1-k)\mathrm{\Delta }\overrightarrow{M1}.\end{array}$$ (A12)

Equation A8 must thus be extended to take into account the correction term $(1-k)\mathrm{\Delta }\overrightarrow{M1}$; in our experiments, the magnitude of this term is always smaller than 0.15°.