New macroecological insights into functional constraints on mammalian geographical range size

Abstract

Understanding the determinants of variation in the extent of species distributions is a fundamental goal of ecology. The diversity of geographical range sizes (GRSs) in mammals spans 12 orders of magnitude. A long-standing macroecological model of this diversity holds that as body size increases, species are increasingly restricted to occupying larger GRS. Here, we show that the body size–GRS relationship is more complex than previously recognized. Our study reveals that the positive relationship between body size and GRS does not hold across the entire size range of mammals. Instead, there is a break point in the relationship around the modal mammal body size. For species smaller than the mode, GRS actually decreases with body size. We discuss mechanisms to account for these observations in the context of the energetics of body size. We also examine the possibility that the patterns are the result of a statistical artefact from combining two random, uni-modal, skewed distributions, but conclude that the patterns we describe are not artefactual. Our results redefine our view of the functional relationship between body size, energetics and GRS in mammals with implications for assessing vulnerability to extinction resulting from range size reductions driven by large-scale environmental change.

1. Introduction

It has long been recognized that the geographical range sizes (GRSs) of even closely related species vary widely [1–6]. In mammals, GRS spans 12 orders of magnitude [7], but the determinants of this variation are poorly resolved [6,8]. Understanding GRS variation is a fundamental goal of ecology: large distributions confer resistance to extinction [9–12] and can increase rates of speciation, population subdivision, colonization and persistence [6,13].

The field of macroecology, which statistically relates ecological variables to species traits over large taxonomic and spatial scales, matured two decades ago partly as an effort to understand the orders of magnitude variation in the extent of species distributions [6,8,14–18]. One of the earliest macroecological relationships examined was between body size and GRS in mammals and birds [14,19,20]. At the scale of global or continental assemblages, the correlation is weakly positive but, moreover, forms a roughly triangular trait space [16,19–21]. The pattern (figure 1a) suggests that small species can attain the full range of observed GRS, but that as body size increases species are increasingly restricted to larger GRS.

Figure 1.

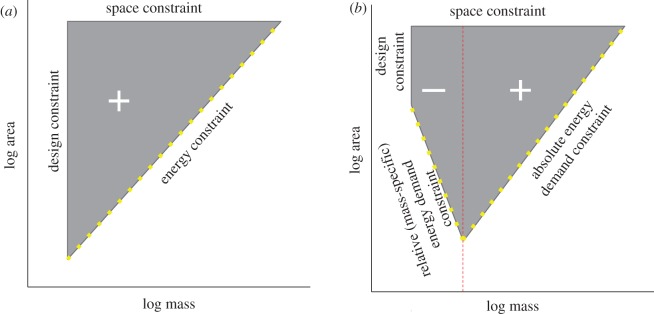
Theoretical constraint space describing the functional relationship between the average body mass of individuals of a species and the extent of its geographical distribution. (a) The original constraint space proposed by Brown & Maurer [20]. (b) The modified constraint space proposed in this study. The red dotted line indicates the modal body size. Solid boundaries to the constraint space indicate absolute constraints; yellow dotted boundaries indicate probabilistic constraints. Positive and negative symbols indicate the direction of the relationship within the constraint space.

As shown in figure 1a, Brown & Maurer [20] postulated three constraints on the body size–GRS relationship that collectively, through differential speciation and extinction, restrict the combination of points to a triangular space: (i) a size-invariant upper bound set by the maximum amount of land area available to potentially occupy (a physical space constraint); (ii) a space-invariant left-hand bound set by the minimum body size attainable by a species in a specific taxon (a design constraint); and (iii) a systematically increasing lower bound on minimum GRS as a function of body size. This third constraint was viewed as probabilistic in that this region of trait space reflects a relatively diffuse boundary, across which there is an ever-decreasing probability of species either arising or persisting through time with these combinations of body size and GRS.

The mechanism proposed by Brown & Maurer [20] to explain the lower bound in figure 1a is that as animals get larger they require more energy [22], larger home ranges [23,24], lower densities [25], and therefore larger distributions to maintain minimum viable populations to avoid extinction. We term this the ‘energetic constraint on minimum range size’ or ECMS hypothesis. The non-existence of mammals with large body size and small GRS (figure 2a,b; see below) presumably reflects a region of trait space with high rates of extinction or low probability of persistence. The implications for conservation are that large-bodied species are inherently more vulnerable to range size reductions (e.g. from habitat loss or climate change) and that regardless of body size, species already close to the hypothetical ECMS lower bound in figure 1a are especially vulnerable to demographic collapse if GRS were reduced [21,26].

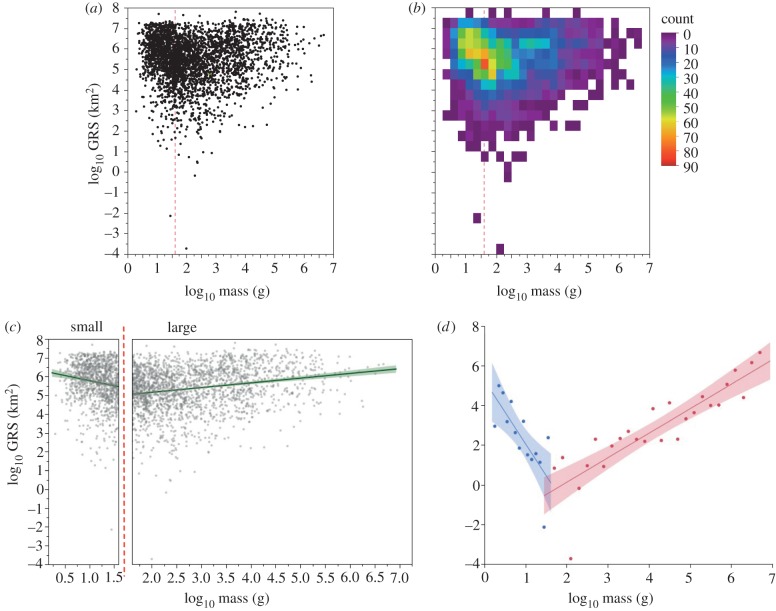
Figure 2.

Empirical relationship between body size and GRS in mammals. (a) Scatterplot of GRS versus mass. (b) Density plot of GRS versus mass. (c) Relationship between GRS and mass on either side of the modal mass. Lines are lines-of-best-fit from ordinary least-squares regression with 95% CIs about the fit. (d) The minimum observed GRS in body size classes on either side of the mode. Small mammals (less than 40 g) are in blue. Large mammals (more than 40 g) are in red. Points along the x-axis are plotted at the midpoint of each respective body size class. Dotted red lines in (a–c) indicate the modal mass for all mammals.

Here, we show that this current interpretation of the body size–GRS relationship is incomplete and we advance a new view of this classic macroecological pattern. Specifically, we find that the relationship between body size and GRS in mammals is nonlinear and that for species smaller than the modal body size, the relationship is actually negative. We discuss mechanisms to account for this new observation in the context of the ECMS hypothesis. We also address the largely ignored possibility that this seminal macroecological pattern is artefactual, instead of a result of functional constraints arising from the energetics of body size.

2. Material and methods

We used all available data on extant or recently extinct terrestrial mammals (including bats) from the PanTHERIA database [7] to re-examine the relationship between body size (adult mass) and GRS in mammals (n = 3268 species, approx. 57% of ca 5700 known species [27] representing 27 Orders and 131 Families). We log₁₀-transformed GRS and mass prior to analysis because the native distributions are strongly right-skewed and vary over several orders of magnitude. A scatterplot (figure 2a) and density plot (figure 2b) were used to illustrate the body size–GRS relationship.

Because the body size–GRS relationship is polygonal, we used a simple procedure [28,29] to characterize quantitatively the lower bounds of the trait space. Specifically, after showing that the relationship reverses sign on either side of the modal body size (see below), we estimated the relationship between body size and the minimum GRS observed in body size classes on either side of the mode. We binned mass into equal size classes, recorded the minimum observed GRS per size class, and fit a regression line through these data. Because the numbers of species and the total body mass range on either side of the mode are so different, we computed different bin sizes for small (bin width = log₁₀ [0.1], n = 14 size classes) and large (log₁₀ [0.2], n = 27) species.

The distributions of log₁₀ GRS and log₁₀ mass are both uni-modal and are oppositely skewed (see the electronic supplementary material, figure S2a). Because the expected pattern when two uni-modal, oppositely skewed distributions are multiplied is a roughly triangular trait space (see the electronic supplementary material, figure S1), similar to the observed relationship between body size and GRS, we evaluated whether the observed body size–GRS relationship is a statistical artefact. To do this, we derived a null model by randomizing the data without replacement and comparing the observed relationship to the randomized relationship (details in the electronic supplementary material). Briefly, we simulated 1000 permutations of the data, computed regression statistics for GRS and minimum GRS as a function of body size as described above, and then compared the empirical parameter values to the distribution of random values.

All correlations were computed as Pearson-product moment correlations. Regressions were computed using model I ordinary least squares with 95% CIs about the fit.

All analyses were conducted using JMP Pro v. 10 (SAS Institute, Cary, NC, USA). Statistical tests were considered significant at p < 0.05.

3. Results

Our analysis revealed that the previously recognized positive correlation between body size and GRS and the lower bound on minimum GRS does not apply to the entire continuum of body sizes, and further, that a converse body size–GRS relationship exists for the smallest mammals. Inspection of the scatter (figure 2a) reveals that the cloud of data (from largest to smaller sizes) tapers to a point somewhere around log₁₀ (2) = 100 g. The density plot (figure 2b) identifies the same pattern: the red pixel in figure 2b indicates the mode of the bivariate relationship, or the combined GRS and mass with the highest number of observations. Again, somewhere between a mass of log₁₀ (2) and the mode on the density plot there is a region in which a positively increasing lower bound originates. The modal body size of mammals in our dataset occurs at log₁₀ (1.6) = 40 g with a median at log₁₀ (1.95) = 90 g. From this point on, we use the mode as an estimate of the position of the break point in the body size–GRS relationship.

When the data are partitioned at the mode into small (less than 40 g) and large mammals (more than 40 g), two body size–GRS relationships emerge. For larger mammals, the relationship is significantly positive (r = 0.22, n = 2144, p < 0.0001), and the pattern indicates that GRS increases with body size, as previously recognized (figure 2c ‘large’). For smaller mammals, the relationship is significantly negative (r =−0.15, n = 1124, p < 0.0001) and indicates that GRS actually decreases with body size (figure 2c ‘small’). This pattern is also evident within Rodentia, an Order that spans the modal mammal body size (figure 3c; see below). Again we find a negative body size–GRS relationship for small species (r =−0.17, n = 342, p = 0.0013) and a positive relationship for large species (r = 0.13, n = 945, p < 0.0001). In comparison, the random null model failed to predict a significant relationship on either side of the mode (see the electronic supplementary material, figure S3), and therefore failed to predict the break point in the body size–GRS relationship at the mode.

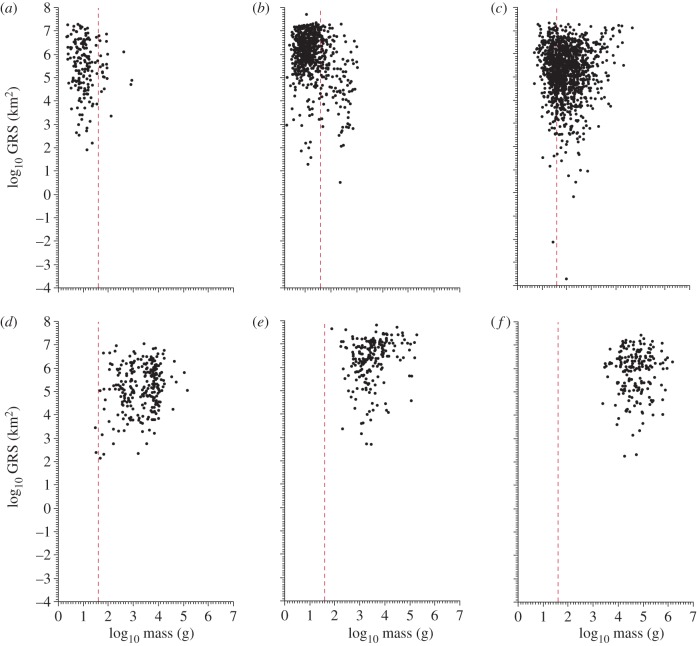
Figure 3.

Relationship between body size and GRS in different mammal Orders. Note the mean clade-specific mass increases from left to right. Dotted red lines indicate the modal mass for all mammals. (a) Soricomorpha, n = 175, r = –0.11; (b) Chiroptera, n = 683, r = –0.33*; (c) Rodentia, n = 1287, r = 0.02; (d) Primates, n = 259, r = 0.22*; (e) Carnivora, n = 210, r = 0.21*; (f) Artiodactyla, n = 196, r = 0.03. Significant correlations are indicated by an asterisk.

In terms of the lower bounds on minimum GRS, figure 2d illustrates the estimated decreasing negative bound for small species (log₁₀ (GRS) = 5.34−3.33 × log₁₀ (mass); r² = 0.59, p = 0.0013) and the increasing positive bound for large species (log₁₀ (GRS) =−2.34+1.24 × log₁₀ (mass); r² = 0.75, p < 0.0001). Although it did not predict the internal structure (see above), the random null model predicted the overall shape of these lower bounds on the body size–GRS relationship (see the electronic supplementary material, figure S4a). However, for large species, the null model significantly underestimated the slope (p = 0.01) and amount of variation (p = 0.02) in minimum GRS explained by body size (i.e. it over predicts the number of large-bodied species with small GRS; electronic supplementary material, figure S4b). The observed lower bound for small species did not deviate significantly from the null model (see the electronic supplementary material, figure S4b).

4. Discussion

(a). The body size–GRS macroecological pattern: fact or artefact?

The triangular shape of the body size–GRS relationship in mammals (and birds) has been recognized for 30+ years and generally has been interpreted as the result of functional constraints arising from the energetics of body size (see below). Although not widely considered in the literature, the possibility exists that some macroecological patterns are actually statistical artefacts [30–32]. In particular, we are unaware of any prior deliberate statistical exercise that has examined the body size–GRS relationship from the perspective of a null expectation. Our simulations (see the electronic supplementary material) show that the expected pattern between body size and GRS produced from a random draw process is superficially similar to the observed pattern (see the electronic supplementary material, figure S2). Nevertheless, we conclude that the observed body size–GRS relationship is not simply an artefact because the null model fails to predict the expected functional structure (see below) within the data scatter on either side of the mode (see the electronic supplementary material, figures S2 and S3). Furthermore, although it predicted the negative lower bound on minimum GRS for small species where the data density is greatest (see the electronic supplementary material, figure S4b), it overpredicts the number of large-bodied species with small ranges (i.e. it predicts a positive lower bound with shallower slope and lower R²; electronic supplementary material, figure S4b). Because the cumulative statistical evidence indicates that the overall structure of the trait space deviates significantly from the null, we explore functional constraints, as have been previously hypothesized, as a better explanation for the data. We also discuss the underappreciated role of phylogenetic information in macroecological analyses.

(b). Energetics of body size and consequences for space use

Brown et al. [33] derived a model of reproductive fitness adduced through the allometric scaling of body size with rates of resource acquisition and conversion of acquired resources into offspring. When parametrized for North American land mammals, the model predicted a body size distribution close to the actual distribution (right-skewed even on a log scale) with an ‘optimal’ size between 80 and 250 g. This value spans the same order of magnitude of body size estimated here to be a break point in the body size–GRS relationship. Furthermore, they predicted that relationships between ecological variables and mass would be reversed on either side of the modal (‘optimal’) body size, because it is at this size that the rate-limiting process shifts from resource acquisition (in small species) to resource conversion (in large species). Here, we find a reversal in the sign of the body size–GRS relationship on either side of the modal mammal body size, which provides strong empirical support for this prediction. A similar pattern has been identified previously in analyses of home range size [23,24], which we discuss below.

The ECMS hypothesis [20] for the previously recognized positive correlation between body size and GRS and the positive lower bound (i.e. for large species to the right of the modal body size) is logically consistent with the observations that energetic demand increases strongly with body size in animals [22], that larger animals have larger individual space needs [23,24] and that larger animals occur at lower population densities [25]. In mammals, adult mass explains approximately 94 per cent of the variation in basal metabolic rate (BMR) [34]. There are many fewer interspecific data available for BMR than for mass. Elsewhere, we plotted GRS versus BMR for 574 species of mammals and compared this with the traditional body size–GRS relationship (S. J. Agosta, J. Bernardo, G. Ceballos, M. A. Steele, 2013 unpublished manuscript). As expected based on the tight allometry between mass and BMR, the relationships are nearly identical confirming that the body size–GRS space is truly a relationship between GRS and baseline levels of energy demand. Alternative mechanisms for positive body size–GRS relationships have been proposed [26,35], but centre on advantages to being large (e.g. broader thermal tolerance and higher mobility), which seems inconsistent with the data because species of all sizes can achieve large GRS.

The mechanism underlying the negative body size–GRS relationship and negative lower bound on minimum GRS for small mammals is less clear. The relationship between body size and home range size in mammals shows a similar break point around 100 g with a positive lower bound to the right and negative lower bound to the left [23,24], although the overall shape of the relationship (log-linear) is very different from the body size–GRS relationship (polygonal). Because BMR scales with body mass with a slope < 1 [34], small mammals have higher mass-specific metabolic capacity/demand than large mammals [22]. Small mammals thus have high capacity to convert resources into offspring but are limited in their ability to acquire resources, whereas large mammals have high capacity to acquire resources but are limited in their ability to convert resources into offspring. According to this model [33], the tradeoff between these two rate-limiting processes (resource acquisition versus conversion into offspring) results in an ‘optimal’ body size for offspring production, which for mammals is predicted to be near the empirical mode (ca 80–250 g). Presumably, this range of body sizes—at which absolute and mass-specific energy demands are jointly minimized compared with larger or smaller body sizes—relaxes constraints on minimum space needs of individuals and therefore species. If true, the break point in body size–GRS space thus may reflect a transition in the energetics of body size (and see Kelt & Van Vuren's [23,24] discussions on the body size-home range size relationship).

Although large mammals can potentially acquire energy at a faster rate than they can use it, they are restricted to large GRS because of the absolute energy demand of being large and the need to forage widely in the face of spatio-temporal variation in resource availability (‘absolute energy demand constraint’ in figure 1b). Conversely, small mammals require less absolute energy, but minimum GRS may be restricted because individuals must forage relatively widely (for their body size) to meet their high mass-specific energy demand, again in the face of spatio-temporal variation in resource availability (‘relative (mass-specific) energy demand constraint’ in figure 1b). The break point in the relationship between home range size and body size around 100 g is consistent with this argument: for species smaller than 100 g there is a lower bound on minimum home range size that increases with decreasing body size [23,24]. Thus, the smallest species tend to have larger individual home ranges than predicted by mass alone, which points to the high mass-specific energy demand of small body size as a constraint on space use. Scaling up to GRS, we would therefore expect the smallest species to have larger GRS than expected for their body size, which is what we observe (figure 2). Additionally, spatio-temporal variation in population densities may scale negatively with body size [36], such that large GRS is necessary to maintain minimum viable meta-populations at the smallest sizes.

(c). The underappreciated role of phylogeny in macroecological analyses

Our modified conception of the body size–GRS relationship (figure 1b) lends new understanding to how the mammal trait space is structured phylogenetically. Figure 3 illustrates that the mammal trait space is a composite of different relationships among individual Orders and that the sign of the apparent constraints on space use that clades experience is a function of clade-specific differences in body size. To the left of the modal body size, Orders of small mammals such as Soricomorpha (figure 3a) and Chiroptera (figure 3b) exhibit negative correlations between body size and GRS, consistent with the negative correlation and lower bound to the left of the mode identified in the analysis of all mammals. To the right of the modal body size, Orders of relatively large mammals such as Primates (figure 3d) and Carnivora (figure 3e) exhibit positive correlations between body size and GRS. For Artiodactyla, there is no apparent relationship between body size and GRS, but a lower bound with positive slope is visually evident in the bivariate space (figure 3f). Rodentia are the modal type of mammal (i.e. most speciose), and they span the modal mammalian body size (figure 3c). As noted above, rodents also mirror the all-mammal trait space in having a negative relationship between body size and GRS to the left of the mode and positive to the right of it.

The key point for macro-scale analyses is that the central tendency of macroecological relationships that emerges from considerations of large datasets may deteriorate when phylogentically structured subsets of the data are considered, meaning that putative functional relationships may not actually apply to all evolutionary groups. On the other hand, the large-scale pattern that emerges can be viewed as a description of the functional constraints that have shaped the overall diversification of the lineages within the larger group. However it is seen, the statistical considerations arising from the shared history of individual species should be considered in future macroecological studies as they are in most other kinds of comparative biology (S. J. Agosta, J. Bernardo, G. Ceballos, M. A. Steele 2013, unpublished manuscript).

(d). Broader implications

Our re-examination of the body size–GRS relationship in mammals modifies our understanding of the structure of the space from the original conception in figure 1a to a new conception in figure 1b. Our results imply that (i) the relationship is not simply the result of multiplying two random distributions and (ii) the mammal assemblage experiences two types of functional constraint on space use, a positive constraint with respect to body size to the right of the modal body size and a negative constraint with respect to body size to the left of the mode (figure 1b). Because the distribution of mammal body sizes has remained relatively constant over much of the Cenozoic Era and across continents, even after accounting for Pleistocene megafaunal extinctions [37], the body size–GRS patterns in this dataset are probably representative of macroecological patterns throughout much of mammalian diversification. Consequently, the trait space and the operant constraints that define it (figure 1b) are inferred to have played a role in historical patterns of species origins, range occupation and extinctions during mammalian diversification.

GRS is a key species attribute that is codified as a predictor of extinction vulnerability [38]. The assumption underlying GRS as a key criterion for vulnerability is that stenotopy (narrow distribution) is inherently risky and congruently, that eurytopy (broad distribution) confers resistance to stochastic extinction, or, more simply, that vulnerability to extinction is linearly related to GRS. This may be a sound assumption all else being equal. However, at least for mammals, this assumption may be incorrect given the apparent functional relationships between GRS and body size (figure 1b), which suggest clade-specific and nonlinear relationships between GRS, body size and extinction risk. As a case in point, species and clades near the modal body size (i.e. 10s to 100s of grams) achieve the full range of observed GRS. This suggests that species in this size range are least vulnerable to reductions in GRS, possibly because this represents some energetically favourable (‘optimal’) body size for mammals [33]. Of course, one way species might respond to reductions in GRS and decreased resource availability is to evolve body size or express plastic adaptive phenotypes that effectively reduce the energy and space needs of individuals.