Desert ants achieve reliable recruitment across noisy interactions

Abstract

We study how desert ants, Cataglyphis niger, a species that lacks pheromone-based recruitment mechanisms, inform each other about the presence of food. Our results are based on automated tracking that allows us to collect a large database of ant trajectories and interactions. We find that interactions affect an ant's speed within the nest. Fast ants tend to slow down, whereas slow ones increase their speed when encountering a faster ant. Faster ants tend to exit the nest more frequently than slower ones. So, if an ant gains enough speed through encounters with others, then she tends to leave the nest and look for food. On the other hand, we find that the probability for her to leave the nest depends only on her speed, but not on whether she had recently interacted with a recruiter that has found the food. This suggests a recruitment system in which ants communicate their state by very simple interactions. Based on this assumption, we estimate the information-theoretical channel capacity of the ants’ pairwise interactions. We find that the response to the speed of an interacting nest-mate is very noisy. The question is then how random interactions with ants within the nest can be distinguished from those interactions with a recruiter who has found food. Our measurements and model suggest that this distinction does not depend on reliable communication but on behavioural differences between ants that have found the food and those that have not. Recruiters retain high speeds throughout the experiment, regardless of the ants they interact with; non-recruiters communicate with a limited number of nest-mates and adjust their speed following these interactions. These simple rules lead to the formation of a bistable switch on the level of the group that allows the distinction between recruitment and random noise in the nest. A consequence of the mechanism we propose is a negative effect of ant density on exit rates and recruitment success. This is, indeed, confirmed by our measurements.

1. Introduction

Animals living in groups need to communicate in order to organize. They achieve this on several scales of organization: on an individual scale, complex communication can stem from the ability of animals to transmit, sense and distinguish a large number of signals [1,2]. On the level of the group, collective ‘rule of thumb’ is repeatedly used and leads to the emergence of complex swarming behaviours [3,4]. Obviously, the nature and efficiency of the signals that can be communicated depends on the transmitting and receiving apparatus [5]. Signals can range from simple mechanical collisions [6–9] to sophisticated, pheromone-based interactions [10]. This poses the puzzle of how to construct efficient codes given the specifics of the relevant apparatus.

We approach this question by following the rudimentary recruitment behaviour of the desert ant, Cataglyphis niger [11]. We show how they produce reliable collective messages, using an extremely limited signal repertoire that is enhanced by the persistence of their action. Deciphering messages within animal groups is difficult because of the large number of processes that concurrently occur over several scales of organization. In general, as long as they are consistent with phenomenology, simple models of communication are preferred to more complex descriptions [12] because they are more elegant and depend on fewer assumptions. Such simple descriptions, often referred to as swarm models, have been highly useful in recounting collective patterns within a wide variety of species, including fishes, birds, locusts and other social insects [4,13–15]. They use mathematical modelling to show how the observed large-scale experimental phenomena emerge from a small set of proposed behavioural rules. However, this methodology suffers from a certain ambiguity, namely several sets of behavioural rules can predict similar collective patterns and distinguishing between them may be difficult. Overcoming this ambiguity has been facilitated by recent technological advances that we specify in the following.

Improvements in camera technology and computing power have opened up the possibility of continuously tracking individuals within a behaving group [16,17]. In the context of animal communication, this technology allows for experimental quantification of the effect that single interactions have on an individual. Examples in this direction describe how individuals on the move respond to their neighbours and provide new perspectives on the dynamics of collective motion [18–21].

Several principles have been put forward to explain the emergence of collective patterns within animal groups and, specifically, social insects [3]. Positive feedback loops are known to promote coordinated actions by enhancing the effects of the individual on the group. Such processes are typically terminated by late, negative feedback loops that also help in maintaining homeostasis. A third principle includes the combination of averaging and response thresholds as a means of correcting errors. For example, harvester ants count the rate at which they encounter midden workers as part of their decision of which task to engage in next [22]. Interaction rates that fall within a certain window induce these ants to turn to midden work themselves. This thresholding process can be viewed as collective error-correcting because it reduces the influence of single ants.

In this work, we study recruitment behaviour in the desert ant, C. niger. The physical strength and navigation capabilities of several desert ant species allow them to forage individually, with no reliance on pheromone trails, as they scavenge for dead arthropods [23]. Nonetheless, a recruitment system to large food items has been previously observed [23] and recently studied [11]. This recruitment is rudimentary in the sense that recruited ants appear to possess no information regarding the location of the food item. Rather, they exit the nest in all directions and rely mostly on chance to locate the food [11].

We study the recruitment process in a controlled laboratory setting by isolating a small number of ants in the entrance chamber of the nest while introducing an immobilized food item outside the nest. The ant that discovers the food recruits nest-mates to exit the nest, similar to the behaviour observed in the field [11]. During this process, we track the whereabouts and interaction history of each individually identified ant and collect a full set of interaction data. We use these data to quantitatively analyse the behavioural changes induced by interactions and estimate the amount of information that they convey. This is conducted by formulating an information-theoretical description of our system. In particular, we view the signal repertoire of the ants as an alphabet, and the number of distinguishable signals as a channel capacity [24]. Using such analogies, we show that the ants use messages of extremely low information content (much less than a single bit per interaction), reminiscent of previously described alerting behaviours [6–9]. The puzzling question is then how such bad signalling suffices at all to transmit a positive message about the discovery of food.

While recruiting interactions are quite non-specific, and nearly indistinguishable from random encounters between nest-mates, they still suffice to transmit a message: this is achieved by two principles that we put forward. The first suggests that individual ants not only control the extent to which they spread a message but also tune their sensitivity to incoming messages. The second involves an early negative feedback loop that serves to restrict the amplification of false-positives which are, in the case we study, large-scale responses to non-recruiting individuals. In the last part of this article, as a further control of single-ant rules, we measure and interpret how recruitment is affected by the density of ants in the nest. In particular, we observe and explain why higher density actually lowers the success rate of recruiting.

2. Results

2.1. Recruitment behaviour

In each experiment, a group of ants was isolated in a chamber of the nest from which there is an exit into an arena. Small groups of two to 13 individuals were used to accentuate single-ant effects. Immediately before the beginning of each experiment, the passage between this entrance chamber and the main chamber was blocked, and ants present in the arena were removed. Then, a food item, an Acheta domestica cricket firmly held in place by tweezers, was placed at the far end of the arena (figure 1a).

Figure 1.

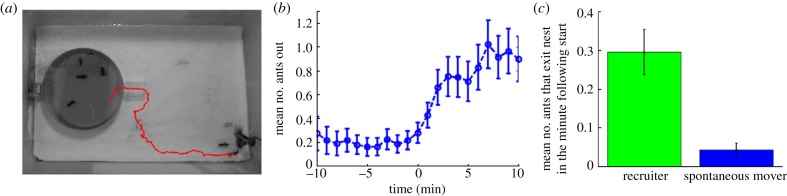
Recruitment behaviour. (a) A snapshot of the experimental arena. The entrance chamber of the nest (d = 9 cm) is at the left and the fixed food item at the bottom right. The red line shows the recent trajectory of one ant. (b) The number of different ants outside the nest during 1 min periods as averaged over (n = 36) experiments. Time is aligned such that t = 0 is the entry of the first recruiter to the nest. The recruiter herself is excluded from the count. The nonlinear rise at t = 0 indicates recruitment. (c) The effect of a recruiter on the number of ants that exit the nest is compared with the effect of a spontaneously (i.e. not immediately following an interaction) moving ant. To isolate the effects of these ants, we take into account events where only all other ants were initially immobile. Results shown are for nests with four to eight ants (n = 229 events). The number of initially immobile ants that left the nest in the minute following recruiter entry is sevenfold higher than the number of ants that exit following a spontaneous movement.

Ants that find the cricket, which we call recruiters, are unable to withdraw it and begin bouts of travelling to the nest and back. When a recruiter moves in the nest, she interacts with other ants and causes some of them to exit into the arena (figure 1b). As some of these ants find the cricket, they, in turn, become recruiters. Any recruiter interacting within the nest causes a larger number of exits than another randomly moving ant (figure 1c, p < 0.01 under the one-tailed Mann–Whitney–Wilcoxon U-test). This means that the ants can differentiate between the signals presented by a recruiter and a nest-mate.

The simplest mechanism that would explain this distinction is a communication system that includes two distinct signals: ‘recruit’ and ‘other’. In §2.2, we show that the information content of the messages communicated between the ants is too low to allow for two signals. In §2.3, we will then derive from the experiment that reliable communication is still possible by using repetition of a simpler message.

2.2. Information content of interactions

We now turn to study the communication mechanism that facilitates reliable recruitment. This is carried out in three steps: first, we show that an ant's speed within the nest can be used to predict her propensity to leave the nest (i.e. to be recruited). Second, we demonstrate that an ant changes her speed by engaging in contact-dependent pairwise interactions with other moving ants. Finally, we quantitatively estimate the information transmitted in interactions by quantifying the change in speed of the message-receiving ant. Specifically, we calculate the information-theoretical channel capacity [24] of the alerting interactions used during this process.

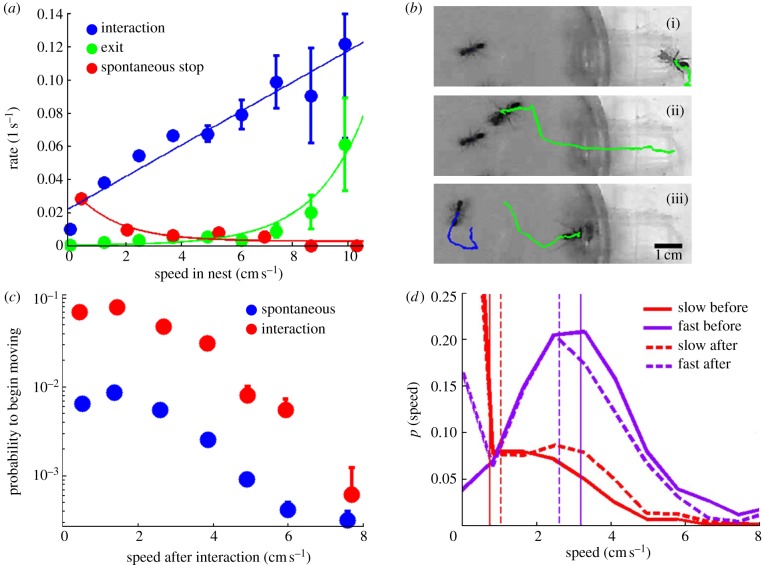
The rate at which ants experience events within the nest depends on their speed (figure 2a). First, the interaction rate is seen to increase linearly with the ant's speed. This is a natural geometrical fact that suggests an unregulated process in which faster running directly translates into proportionally higher encounter rates. Second, the ant's speed tells us something about her internal state, in that the probability for her to stop spontaneously decreases if she is moving fast. Third, and most important, is the ant's propensity to leave the nest. We find that exit rates are directly related to an ant's speed within the nest. This exit rate grows with speed in a nonlinear manner, with a soft threshold at approximately 8 cm s⁻¹. This nonlinear behaviour indicates that the increase in an ant's exit probability is not a simple, geometrical consequence of her high speed. Furthermore, given an ant's speed, her exit rate is independent of whether or not she has, within the last 2 min, interacted with a recruiter (two-tailed χ²-test per bin of figure 2a; average value of p = 0.46, and p > 0.1 for all bins). This last finding supports the hypothesis that an ant's internal state contains no ‘hidden variables’ that are not reflected in her speed but do affect her exit rates.

Figure 2.

Pairwise interactions and speed. (a) Rates of events experienced by an ant in the nest are a function of her speed. (b) An example of a contact-dependent, pairwise interaction: a recruiter entering the nest contacts the abdomen of an immobile ant, causing her to commence movement. Panels (i–iii) in (b) are 8 s apart and are overlaid with the trajectory of each ant in the preceding 8 s. (c) The probability per time unit (of approx. 5 s) that an ant starts moving as a function of the speed at which she will move. Movement is more likely to happen directly following an interaction than not (n = 52 330, 5 s periods). (d) Interactions have an averaging effect on the speeds of non-recruiter ants. Solid lines specify the speed distributions of fast and slow ants just before interaction, whereas dashed lines specify those just after interaction. Vertical lines denote the corresponding distribution's mean. (Distributions were calculated over all interactions in which at least one of the ants changed her speed, n = 1722 interactions.)

Having found that high speeds increase an ant's exit rate, we are interested in the possible mechanisms by which ants gain in speed. We find that immobile ants increase their speed following a pairwise contact-dependent interaction (only 10% of the increases are spontaneous; figure 2b,c). On the other hand, an immobile ant's speed is not affected by near-passages (see §4) of a moving ant even if that ant happens to be a recruiter. Of 152 near-passes by recruiters, only two induced movement in immobile ants—in line with what would be expected of spontaneous acceleration. These facts support contact-dependent rather than long-range, pheromone-dependent recruitment [8,9,25]. Interaction-dependent speed shifts are not a property of immobile ants alone. In fact, an interaction between two non-recruiter ants has an averaging effect on their speeds (figure 2d). Thus, because the exit rate depends on the speed, an ant's exit probability will increase following repeated interactions with faster nest-mates, but decrease after interactions with slower ones.

An ant changes her speed as a result of interactions; we seek to find how this change depends on the state of the ant she interacts with. Figure 3a shows that the change in the speed of an immobile ant after an interaction depends on the speed of the ant she met (Kolmogorov–Smirnov test on the extreme speed bins gives; p < 0.05) but not on whether this ant is a recruiter or not (Kolmogorov–Smirnov, p = 0.50 on average where none of the bins show statistically significant differences, p > 0.1 for all bins). Similarly, recruiters have no special effect on the speed of mobile ants: when an ant interacts with another ant at a given speed, she will gain a negligible 0.17±0.08 cm s⁻¹ (mean±s.e.m.) more speed if the second ant is a non-recruiter (n = 2699) rather than a recruiter (n = 1077).

Figure 3.

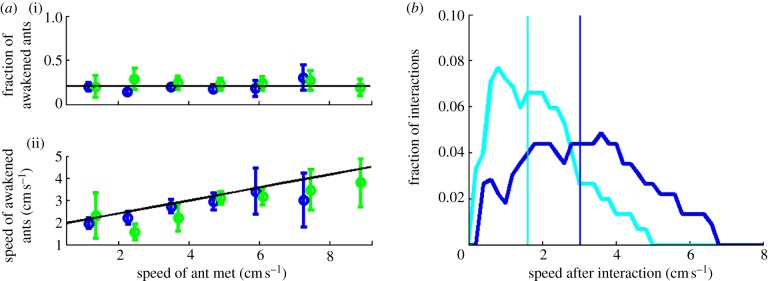
Information content of interactions. (a) Reactions of immobile non-recruiter ants to interactions with another non-recruiter (blue circles) or recruiter (green circles) ant moving at a given speed. (i) Fraction of immobile ants that start moving after the interaction. (ii) The speed of the immobile ants that became mobile after the interaction. The speed change depends on the speed of the ant contacted but not on whether this ant is a recruiter or not (n = 636 interactions). (b) Smoothed histograms of speed responses of immobile ants to an interaction with either a fast (more than 8 cm s⁻¹; blue) or slow (less than 1 cm s⁻¹; cyan) moving ant. Distribution means are specified by vertical lines and correspond to the data given in (ii) of (a). The two distributions are statistically different (Kolmogorov–Smirnov test, p < 0.05). However, the large overlap makes the attribution of a single sample to one histogram or the other ambiguous.

All this provides evidence that any information transferred by an ant is reflected in her speed as she enters an interaction. This precludes the possibility of pheromone signals and other scent cues that are unique to the recruiter. It is also consistent with the fact that interactions include antennations and pushes that are incurred on different parts of the ant's bodies (figure 2b). Our conclusion is consistent with tactual alerting interactions that were previously observed to non-specifically increase activity during recruitment in bumble-bees and other ant species [6,8,9]. We stress that this does not go to say that speed might not be a derivative of some internal state of the ant. Rather, we suggest that speed is a sufficient indicator for this internal state for the purposes of predicting the ant's propensity to be recruited.

We next quantify the efficiency of this non-specific communication in information-theoretical terms by focusing on interactions between a mobile and an immobile ant. We define an information channel [24] whose input is the speed of the mobile ant before the interaction and whose output is the speed of the previously immobile ant afterwards (figure 3a). We calculate the capacity of this channel, an intrinsic property that sets the limits on the amount of information that may be transmitted through it [26]. Because speed carries all information that is relevant to exits, the channel capacity gives an estimate of the efficiency of alerting interactions towards recruitment. One could imagine an efficient information channel that allows an immobile ant to start moving fast, if she interacted with a fast ant, or slow, if she interacted with a slow one. Such a channel has high discriminatory power and a capacity of one bit per interaction. Our measured statistics tell a different story, namely there is a substantial overlap between the output speed distributions corresponding to two very different speed inputs (figure 3b). The channel capacity provides a quantification of this overlap and signifies the degree of ambiguity associated with the information channel in question. We estimate channel capacity at 0.22±0.11 bits (mean±s.e.m., see electronic supplementary material), intuitively, this implies that four to five interactions are required to transmit a single bit. This holds the surprising consequence that, at least during the recruitment process, the ants use an alphabet of just over one symbol (2^0.22 = 1.2 symbols). In particular, this alphabet is smaller than two distinguishable signals (i.e. ‘recruit’ and ‘other’) as one might have expected for this task. The fact that the desert ants have relatively little use for such recruitment behaviour [8,11,27,28] could explain this seemingly degenerate communication mechanism.

To summarize, while speed is an essential indicator of an ant's state, it is so poorly communicated during interactions that we have to discard the two message mechanism introduced at the end of §2.1.

2.3. From a small alphabet to reliable collective messaging

Having concluded that speed tells us all about the state of an ant, we are confronted with a new problem: how does the system distinguish between recruitment messages and the fact that ants may move within the nest for other, unrelated reasons? Two opposing goals must be satisfied: to control noise, reactions to interactions should be kept to a minimum so that random movements within the dense (and dark) nest environment do not elicit large-scale effects. On the other hand, reactions to interactions should be large enough for recruitment to succeed. Achieving this is not trivial, especially in the light of the fact that all interactions appear to be carrying the same effective message.

The answer lies not in the receiver side of the message but on the side of the transmitter. Direct exposure to food has a lasting effect on an ant's reactions to pairwise interactions. Recruiters that have been exposed to information hold on to it, they move fast (figure 4a) and their speed is marginally affected only by interactions (figure 4b). Ants that have not been directly exposed to the food behave differently. They move slower (figure 4a, p < 0.01, one-tailed Mann–Whitney–Wilcoxon U-test) and react to interactions by altering their speed (figures 4b,c and 2b–d). The interesting point is that these effects are mainly independent of the state of the other ant and thus do not require any accurate communication mechanism.

Figure 4.

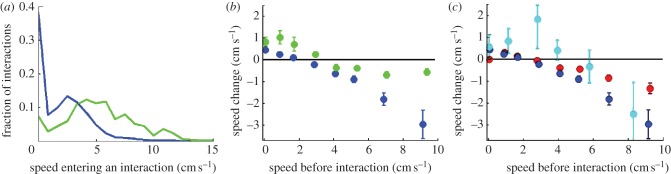
Differentiating recruitment from random nest activity. (a) Speed distributions of recruiters (green) and non-recruiters (blue) as they approach an interaction within the nest. Recruiters tend to be faster than non-recruiters (n = 2693 interactions). (b) The mean linear speed shift owing to interaction (speed after interaction minus the speed before interaction), as a function of the speed before the interaction. While recruiter (green) speed changes only marginally following an interaction, the speed of a non-recruiter (blue) typically decreases (n = 4912 interactions). (c) The mean linear speed shift of ants within the nest following an interaction (blue), an interaction with a relatively fast ant (cyan) (speeds over 11 cm s⁻¹, typical of a fast recruiter), and no interaction at all (red), i.e. spontaneous shift (n = 59 940 events).

These very simple behavioural rules lead to an effective noise-control mechanism at the level of the colony. Figure 4c explains what is happening: a non-recruiter ant increases her speed after interaction if it was slow, but decreases it when it was fast, as we explained earlier, with a crossing at approximately 2 cm s⁻¹. So, in principle, all speeds would eventually move to this crossing point, which is far below the exit threshold. This fixed point is left unchanged, if we include interaction-independent speed changes (figure 4c). Note that when all ants are immobile, there are no interactions and this defines a second fixed point, which persists as long as none of the ants commences spontaneous movement. All this describes a dissipative environment in which ants slow down and reduce their likeliness to leave the nest.

However, looking again at figure 4c, we see that acceleration and exits can occur if fast ants are present within the nest. A recruiter ant maintains high speed and interacts with multiple ants and may therefore raise the speed fixed point towards 6 cm s⁻¹, which is much closer to the exit speed threshold. Note that a fast-moving, non-recruiter will not be able to produce a similar effect as she loses her speed upon interactions.

2.4. Group-level consequences

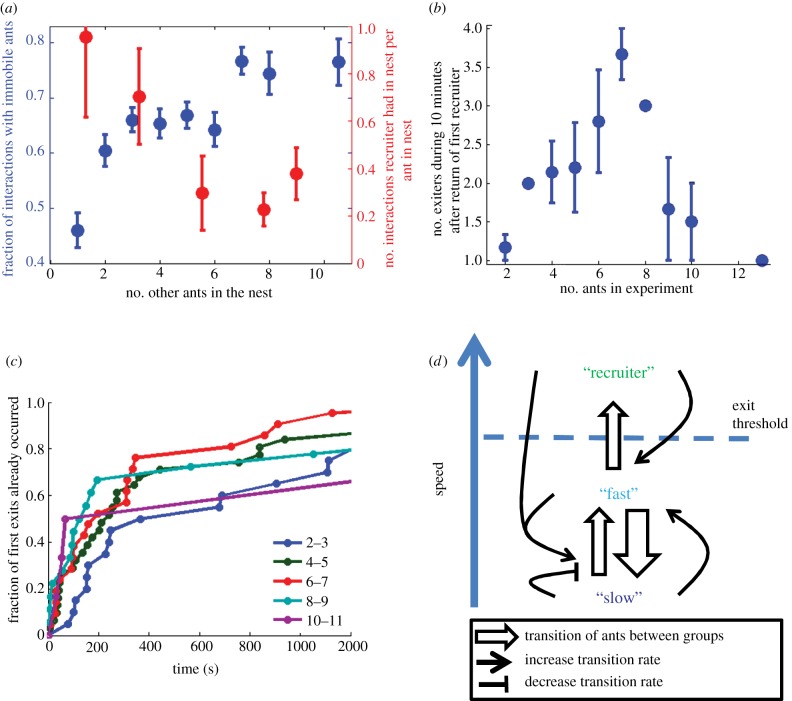
As demonstrated earlier, the ants’ exit rates are decreased by interactions with slow nest-mates. We find that the fraction of interactions with immobile nest-mates increases with the number of ants in the nest (figure 5a). In line with our general outlook, we therefore expect to find stronger dissipation effects when the number of ants increases. We tested this for recruitment and spontaneous exits (those that happen before the food is found). In both cases, we observed response functions that corroborate our picture.

Figure 5.

Group-level phenomena. (a) The fraction of interactions that a moving ant has with immobile ants increases with the number of ants in the nest (blue symbols, n = 2497 interactions). Although the total number of interactions a recruiter has within the nest in a single bout slightly increases with group size, this effect is marginal. In fact, the number of interactions between the recruiter and a given ant decreases as group size increases (red symbols, n = 108 recruiting bouts). (b) The number of different ants that have been outside the nest during the 10 min after the return of the first recruiter given as a function of the total number of ants in the experiment (n = 34 experiments). The three points with 0 s.e.m. signify single measurements. (c) The probability that the first ant's exit from the nest had already occurred as a function of the time from the beginning of the experiment (n = 96 experiments). Each trace signifies a different number of ants in the nest as specified in the legend. (d) A schematic summarizing the feedback loops that regulate collective behaviour and suppress noise as suggested by our model. The diagram reduces the ants’ state from continuous values of speed into three discrete states: ‘slow’, ‘fast’, and ‘recruiter’. A fourth, ‘forager’, state relating to ants that have left the nest but did not yet find the food is ignored for simplicity. Non-filled arrows depict the possible transitions between states, and thin arrows signify how interactions between ants change the rate at which ants transition between these states. For example, a larger number of ants in the slow state induce an increased suppression of the rate at which slow ants transition to the fast state. The feedback loops between the slow and fast states constitute a bistable switch that is initially skewed towards the slow state. It is this dissipation that prevents fast movers from retaining their speed and protects the switch from erroneous activation. The appearance of ‘recruiter’ ants in the system reduces the dissipative skew of the bistable switch and allows for a recruitment process. Finally, recruited ants become recruiters and allow for a positive feedback loop as long as the food item is present.

To quantify the recruitment success, we count the number of different ants that left the nest within 10 min following the return of the first recruiter. As the number of ants in the nest grows, the number of recruited ants initially grows simply owing to the larger workforce available. However, a decrease in the number of recruited ants is visible when initial nest-occupation exceeds seven ants (figure 5b). Apart from speed dissipation, a second mechanism contributes to this non-monotonous behaviour: during a visit to the nest, the number of interactions with the recruiter increases slightly with group size. The number of interactions with a given ant decreases with increasing group size (figure 5a), basically because there is more choice. But this means that a given ant gets excited less often and is less likely to leave the nest.

As a further verification of this phenomenon, figure 5c shows the cumulative distribution function of first exit times for different numbers of ants in the nest. On short timescales, the response scales with group size, and exits happen earlier in the larger groups. This is not surprising as for larger groups, the probability that at least one of the ants becomes spontaneously mobile—a prerequisite for an eventual exit—is also larger. However, on longer timescales, we observe an interesting crossover: exits still happen faster up to a group size of six to seven ants, but then become slower as group size grows. This is so because an ant that starts moving is more likely to exit when there are few ants, while the mutual damping of the motion through interactions with other ants will slow it down too much in a dense environment.

A density threshold value (six to seven ants) for these large-scale dissipative effects is clearly visible in the two different datasets. This strengthens our claim that desert ants do not employ a distinct recruitment signal and that spontaneous exit and recruitment are, in fact, two faces of the same coin. While this is intuitively clear, any modelling to determine the exact threshold density seems too difficult, in view of such parameters as the nest's geometry, the positions of ants within it, and the response heterogeneity between ants.

3. Discussion

We found that C. niger ants demonstrate reliable recruitment to a food source. Based on collective scale observations, we then hypothesized a simple single-bit communication model (‘recruiter’ versus ‘other’) that we tested by first focusing on single interactions. It turns out that even this single-bit model is not consistent with the low information content (0.22 bits) of individual interactions. Furthermore, the error-prone interactions we observe pose a problem to recruitment as single ants are not able to reliably distinguish between recruitment attempts and other interactions. We were thus led to suggest a different, actually even simpler, model that combines positive and negative feedback loops (as summarized in figure 5d). This model is consistent with both macroscopic and microscopic measurements. We then used this revised model to predict and verify the effects of total density on exit times and recruitment success probabilities.

It is interesting to view the noise control suggested by our analysis on two different scales: that of single ants and that of the group. Note how behaviour that is reasonable on the level of the individual leads to robust dynamics on the level of the group.

On the level of single ants, we distinguish between ants that have found the food and those that are moving for other reasons. Recruiters act with conviction; they keep on interacting and communicating their message (this is similar to earlier studies [7–9]). In addition, they ‘tune down’ their sensitivity to the state of other ants and do not alter their speed upon interactions. Contrary to recruiters, ants that have not seen the food are sensitive to interactions that typically slow them down. By doing so, these ants effectively self-restrict their own tendency to engage in further interactions that would excite further nest-mates. These ants are also sensitive to meeting with very fast ants, which ‘convince’ them to speed up. To summarize, individual ants behave reasonably: those that are certain of their message disseminate it and are not affected by other opinions, those that are less sure change their behaviour according to the states of others.

On the level of the group, these simple behaviours lead to a number of feedback loops (as depicted in figure 5d) that suppress the effects of random noise but still permit recruitment when required. Ants either slow down or speed up upon interactions and this forms a double positive–negative feedback system that gives rise to a group-level bistable switch. Indeed, the activity within the nest can switch between the ‘fast’ and ‘slow’ fixed points as shown in figure 4c. Interactions have a higher tendency of slowing ants down and thus the negative feedback dominates and pushes the switch to its slow position. Note that this early negative feedback is very different from the late, homeostatic, feedbacks typically described in the context of social insects [3,12,29,30]. Rather than terminating the response or regulating its amplitude, the rapid negative feedback we observe here (see [31]) serves a completely different goal: limiting possible far-reaching effects of random events.

In the presence of a recruiter, ants will tend to have more interactions with fast nest-mates (in particular, with the recruiter), gain in speed and leave the nest (see [22]). Thus, recruiters push the switch position towards the fast state (figure 5d) from which there are increased exit rates. Finally, ants that leave the nest become themselves recruiters and establish a second positive feedback loop that enables foraging as long as food is present.

The balance between positive and negative feedbacks is not fixed, and depends on the density of ants in the nest. Larger groups have higher dissipation rates that may not be overcome by a recruiter's conviction (figure 5). This serves as an example of how a poor communication system can constrain efficient collective performance to groups of a certain size. It would be interesting to find how our results relate to natural settings. First, field observations [11] reveal rudimentary recruitment behaviour that is similar to what we see in the laboratory. This suggests that no new modes of communication appear in natural field conditions. More quantitative comparisons between our work and natural conditions depend on factors such as the natural structure of the nest, the density of ants and their actual spatial distribution within the entrance chamber: although very simple, our laboratory nests hold some important similarities to natural ones. The latter typically contain an entrance chamber which is somewhat removed from deeper nest chambers; furthermore, this entrance chamber is typically occupied by a small number of workers ([32] and personal observations, 2011). In natural conditions, one may therefore expect to see density effects similar to those we describe here.

Noise control via an activation threshold coupled with a rapid negative feedback is not unique to our system. For example, membrane leakage and spiking threshold are set to make neurons respond only to a critical level of synaptic inputs [33]. Similar mechanisms have been observed at the cellular as well as cellular network scales [34–37]. Our results expose a mechanism by which reliable stimuli may overcome the activation threshold and be amplified even if they are carried by only a single member of the group.

Our observations include some evidence that argues against a more complex communication system: ants react to the speed of other ants and not to whether those ants have found the cricket (figure 3a). Further, we observe some successful recruitment attempts in which the recruiter contacts only the gaster of the recruited ant (figure 2b). Last, fieldwork shows that recruited ants have as much difficulty finding the food item as the earlier explorers [11]. Although our statistical evidence coincides with a simple communication model, our analysis might be blind to more complex interaction schemes. While more complex signalling mechanisms cannot be excluded by our experiment, we do present a simple and elegant mechanism that is sufficient for recruiting.

Our study shows that even a very restricted alphabet, as used by C. niger, is sufficient to transmit a message reliably. The presence of food and the corresponding recruiting are achieved by a repetition of this simple message by the ant that has found the food. This is reminiscent of studies where individuals within groups modulate their interactions as a function of their personal knowledge or state [7,38]. The beauty of the mechanism we observe is that it simultaneously avoids false-positives by a damped reaction of the receiver of the message. This finding also sheds new light on studies of rumour-spread processes [39] or effective leadership within animal groups [40]. These can be interpreted as a combination of a convinced sender and a set of receivers that need to be alerted several times before they perform the desired task.

To summarize, our model suggests that ants achieve their aim not only by extending their alphabet, but also by a persistent interaction. In information-theoretical jargon, one can transmit a message by a short string of a rich alphabet, or by a long string of just 0s and 1s. Both methods work, but, clearly, the second option requires a less sophisticated reading apparatus. This second option seems to be implemented in C. niger ants.

4. Methods

4.1. Colonies used

The study was carried out, using four colonies of the desert ant C. niger, collected in the Rehovot area in Israel on March 2011. Each colony consisted of a few hundred ants. While two of the colonies included male brood, all of them were queenless. This is not expected to be a major limitation as C. niger ants are polydomous and it is most likely that some of their natural nests contain queens while others do not [32]. Furthermore, previous works report no observed effects of the presence of the queen on behaviour outside the nest [41,42]. The experiments were conducted during August–November 2011.

4.2. Experimental protocol

Several weeks before experiments began, each colony was moved into a set-up that contained a two chamber nest and an arena (17 × 26.5 × 7 cm), the sides of which were covered with Fluon AD208E (AGC Chemicals Europe, Ltd) to prevent ants from escaping. Normally, most ants stayed in the larger, inner chamber while some ants were in the smaller entrance chamber and in the arena. Prior to each experiment, the ants were starved for 3–7 days.

Each experiment was prepared in the following way: the door between the entrance chamber and the inner chamber was closed, and ants that were in the arena were removed. Then, a cricket clamped by tweezers was placed in a far corner of the arena, and the experiment began. The only ants participating in the experiment are the ones in the entrance chamber at this point. Thus, when discussing the experiment, we refer to the entrance chamber as the nest.

During the experiment, both visible light and IR light (850 nm) were used to illuminate the arena. Recruitment was filmed at eight frames per second with a webcam whose IR-blocking spectral filter was removed. The entrance chamber was covered by an IR pass filter, so that it is transparent for imaging while appearing dark for the ants, which do not see in the infrared [43].

4.3. Data analysis

The experiments were analysed by offline image analysis to identify the ants in each frame, and then linking ants between consecutive frames, using the Munkres assignment algorithm. Using the resulting trajectory data, the following events were identified for each ant: exiting the nest, entering the nest, entering the cricket area (defined to be slightly more than touching distance from the cricket), leaving the cricket area and interaction with another ant. Errors in the automatic analysis were manually corrected. Interactions were defined as any physical contact between two ants. They were identified as events where two ants are closer than a threshold distance of 4 mm, which is about half the size of the ant. In order to check the presence of long-range, pheromone-dependent interaction, we tested the effect of near-passages on ants. Such passages were defined as a passage of the centre of the two ants within a distance of 1.5–2 cm from each other. This value was chosen as the smallest ant-to-ant distance that assures that they do not touch. The ants’ movements typically consist of short bursts of motion followed by short stops (both lasting approx. 1–2 s). Owing to this spastic behaviour, we define an ant's speed as the 80-percentile value of the enveloping function of her actual speed in consecutive 5 s periods.

In all results presented with error bars, the error bars denote the standard error of the mean.