Monkeys match and tally quantities across senses

Abstract

We report here that monkeys can actively match the number of sounds they hear to the number of shapes they see and present the first evidence that monkeys sum over sounds and sights. In Experiment 1, two monkeys were trained to choose a simultaneous array of 1-9 squares that numerically matched a sample sequence of shapes or sounds. Monkeys numerically matched across (audio-visual) and within (visual-visual) modalities with equal accuracy and transferred to novel numerical values. In Experiment 2, monkeys presented with sample sequences of randomly ordered shapes or tones were able to choose an array of 2-9 squares that was the numerical sum of the shapes and sounds in the sample sequence. In both experiments, accuracy and reaction time depended on the ratio between the correct numerical match and incorrect choice. These findings suggest monkeys and humans share an abstract numerical code that can be divorced from the modality in which stimuli are first experienced.

Introduction

Number representation and calculation are not the unique province of humans. Various non-human species can represent and compare number independently of non-numerical stimulus features such as density or surface area, and their accuracy and reaction time in making these numerical judgments are modulated by the ratio between compared values (e.g., Cantlon & Brannon, 2006). Similarly, when adult humans are prevented from verbally counting, their number judgments are also ratio dependent, and performance is often indistinguishable from that of nonhuman animals tested on the same tasks (e.g., Cantlon & Brannon, 2006, 2007; Cordes, Gelman, & Gallistel, 2001; Pica et al., 2004; Whalen et al., 1999).

A language-independent, analog magnitude system has been proposed to underlie many of these nonverbal numerical abilities (e.g., Dehaene, 1997; Gallistel & Gelman, 1992). This is a separate system from that underlying verbal numerical knowledge. The signature property of the analog magnitude system is that it is ratio dependent and obeys Weber’s Law, which states that ΔI/I = C, where ΔI is the increase or decrease in stimulus intensity that is required to produce a detectable change in a standard stimulus and C is a constant. Therefore, if a student requires a gain or loss of 2 pounds to detect a change in a 10-pound backpack, the same student would need a 20-pound increment or decrement to detect a change in a 100-pound backpack.

Data showing that numerical discriminations adhere to Weber’s Law have been obtained using a wide variety of different species and paradigms (e.g., Beran, 2004; Cantlon & Brannon, 2006; Emmerton & Renner, 2006; Fetterman, 1993; Jordan & Brannon, 2006; Judge et al., 2005; Nieder, Freedman & Miller, 2002; Platt & Johnson, 1971; Roberts, 2005; Smith et al., 2003). These discriminations are not limited to the visual modality, as there is evidence that non-human animals can represent number in the auditory modality and that these representations are also limited by ratio. For instance, Hauser and colleagues (2003) found that cotton-top tamarin monkeys familiarized to sequences with a constant number of sounds orient longer to sequences that contain a novel number of sounds, and that their ability to detect numerically novel sequences is dependent on the ratio between the novel and familiar numerosity. Similarly, Meck and Church (1983) found that rats’ numerical discriminations in an operant task using auditory stimuli conformed to expectations of Weber’s Law. Thus, number discrimination is ratio-dependent in both the visual and auditory domain.

Animals are also capable of matching numerosities within either of these sensory modalities across presentation formats. For example, Hauser and colleagues (2002) found that cotton-top tamarin monkeys spontaneously recognized the numerical equivalence between small numbers of speech syllables and tones. Similarly, in the visual modality, Nieder and colleagues (2006) found that rhesus monkeys could select a visual array that numerically matched a sample of visual elements that was presented sequentially for the small values 1-5.

Number is an amodal property of a set of discrete elements, and adult humans easily count over sounds, sights, touches, smells, or even concepts. While language clearly allows humans to represent the number of events abstractly independent of the sensory modality in which an event is experienced, a recent study indicates that even when adults are representing number without language, their representations traverse sensory modalities. Barth, Kanwisher, and Spelke (2003) presented adults with two sequences of tones or circles too rapid to verbally count and asked subjects to indicate whether there were the same or a different number of events in the sequences. They found that humans show virtually no cost in accuracy for comparing numerosities across the visual and auditory modalities compared to within a single modality, suggesting that they possess nonverbal number representations independent of stimulus modality. If non-human animals and humans share a nonverbal system for representing number as analog magnitudes, is it thus possible that even the number representations held by nonhuman animals are sufficiently abstract to transcend sensory modality?

A recent study found that rhesus monkeys looked longer at a video containing images of the number of monkeys matching the number of monkeys they simultaneously heard vocalizing (Jordan et al., 2005). In that study, monkeys heard choruses of 2 or 3 monkeys vocalizing, and regardless of which number they heard, they looked longer at the numerically matching video. Field studies also suggest that non-human animals predict the number of intruders they expect to see based on the number of vocalizing intruders they hear (e.g., McComb et al., 1994; Kitchen, 2006); the probability that a group of chimpanzees, for example, will approach a speaker emitting vocalizations from an unfamiliar conspecific depends on the number of chimpanzees present in the group (Wilson et al., 2001). An important question that remains unresolved, however, is whether the ability to cross-modally match based on number is context-specific and isolated to social judgments or instead is sufficiently abstract to extend to arbitrarily related, non-ecologically relevant stimuli. A second related question is whether animals can actively match across sensory modalities such that they can choose a numerically matching array that is presented in a different sensory modality. In other words, are the numerical cross-modal matching abilities suggested by social contexts accessible to the monkey, or do they reflect more implicit knowledge that might be used only in specific contexts?

A third vital question is whether non-human animals, like humans, rely on a ratio-dependent, analog magnitude system for representing and comparing a large range of numerosities across senses. Previous studies have used only small numerical values and are therefore unable to determine whether animals’ cross-modal numerical capacities generalize to larger values or show the ratio-dependent hallmark of human nonverbal number judgments. For example, a pair of prior studies that tested the ability of rats to make numerical discriminations with light flashes and tones limited the numerosities tested to a few small values (Church & Meck, 1984; Davis & Albert, 1987). Church and Meck (1984) trained rats to press one lever after hearing 2 tones or seeing 2 lights and a second lever after hearing 4 tones or seeing 4 lights. The rats were then presented with a compound stimulus of 2 tones and 2 lights. Rats reliably chose the lever associated with 4 tones or 4 lights when presented with these compound stimuli, suggesting they had summed across lights and sounds. In contrast, however, Davis and Albert (1987) trained rats to discriminate 3 sequentially presented sounds from 2 or 4 sounds and found no evidence that rats transferred their auditory numerical discrimination to the visual modality when presented with sequences of 2, 3, and 4 lights. The results from Davis and Albert (1987) raise the possibility that the rats in the Church and Meck study (1984) made dichotomous, intensity-based judgments (i.e., they equated the less intense sound with the less intense light), leaving open the question of whether the calculations made by the animals were in fact based on the representation of numerical equivalence.

A final question we seek to answer is whether animals can sum across sensory modalities. Only one prior study has attempted to address whether nonhuman animals can go beyond basic cross-modal numerical comparisons and perform other arithmetic operations, such as summation, across sensory modalities. In this study by Church and Meck (1984) which was described above, rats behaved as if they summed 2 sounds and 2 sights by classifying the compound stimulus as 4. However, it is possible that they merely categorized the 4-compound stimulus as more intense than the alternative stimuli. Furthermore, the representational system that might enable non-linguistic organisms to sum items across sensory modalities has never been investigated. No current data inform whether non-human animals use a ratio-dependent system to nonverbally sum a large range of numerical values across senses. Given that Barth and colleagues (2006) found that adult humans can nonverbally add visual and auditory items and that accuracy is modulated by ratio, it seems likely that if non-human animals and humans share a system for representing number as analog magnitudes, animals can also integrate items across different senses to extract the total numerical value.

Experiment 1 tests whether non-human primates can actively match arbitrarily related stimuli based on numerical correspondences across different sensory modalities. Monkeys were presented with a sample sequence of tones or squares and subsequently with a choice between two simultaneous visual arrays, only one of which contained the number of squares matching the sample. Monkeys were required to ignore nonnumerical variables and numerically match stimuli across presentation formats (sequentially vs. simultaneously presented arrays) within the visual modality or across stimulus formats and modalities (visual vs. auditory). In Experiment 2, each sample sequence was composed of a collection of randomly interspersed sights and sounds, and monkeys were required to choose from two simultaneous visual arrays, only one of which contained the total number of sounds and sights presented in the sample. Thus, the task required monkeys to ignore nonnumerical variables and numerically integrate stimuli across the visual and auditory modalities. Both experiments tested whether accuracy and latency to respond showed the hallmark ratio dependence of the analog magnitude representational system.

Experiment 1

Methods

Subjects

Subjects were 2 female rhesus macaques (Macacca mulatta). Both subjects were approximately eight years old and were housed socially at Duke University in a communal Primate Products cage in a colony room with a 12:12-h light:dark cycle. Monkey chow was available ad libitum along with daily foraging enrichment.

Apparatus

Monkeys were tested in small soundproof rooms constructed by Industrial Acoustics Company, Inc. Each subject had previously been trained to sit in a primate chair and touch a touch-sensitive monitor mounted on the inside wall of the room. A Crist Instrument Company juice system was used to provide reinforcement through a juice spout, which was attached to the chair directly in front of the monkey’s mouth. A Dell OptiPlex GX400 computer executing a software program written in-house in Real Basic was used to create, run, and control the sessions (i.e., to project stimuli onto the touchscreen and play auditory stimuli from a speaker inside the room) and to register responses. Experimenters monitored the subjects remotely via live video.

Stimuli

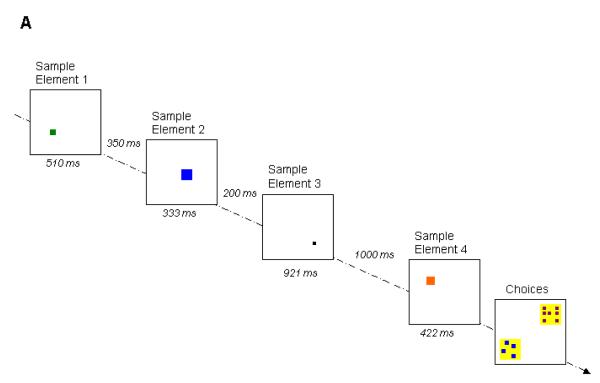
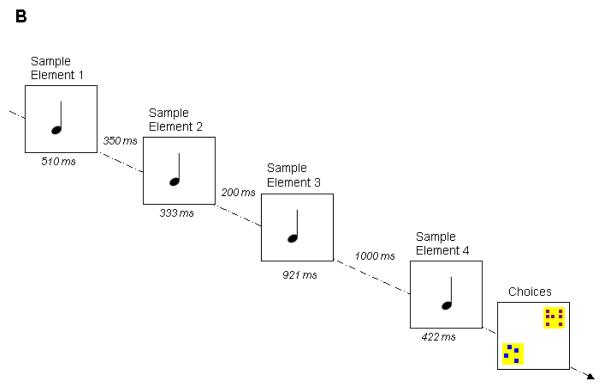
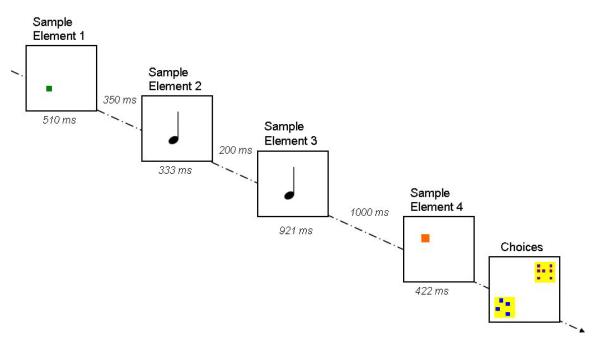
Sample stimuli contained a variable number of elements presented sequentially. Auditory stimuli were presented at a uniform frequency of 440 Hz throughout all phases of training and testing; they were always presented from a centrally located speaker at a uniform amplitude (see Figure 1b). Visual stimuli contained a variable number of square-shaped elements presented sequentially on a white background (see Figure 1a). The color of these elements was randomized within and between trials (green, red, blue, orange, purple, black), as was the diameter of these elements (220-2600 sq pixels). Positions of these elements on the screen varied randomly within a 10×10cm central location within and between trials. Choice stimuli were always two simultaneously presented visual arrays containing distinct numerosities of square-shaped elements. One array numerically matched the sample. These choice arrays were each presented on a yellow background measuring 10×10cm. The elements were a uniform diameter within each choice stimulus within any one trial, but varied randomly in diameter between trials from 220-2600 sq pixels; similarly, the elements were a uniform color within each choice stimulus within any one trial, but varied randomly in color between trials. The choice stimuli could appear in any one of nine positions on the monitor. All stimuli were trial-unique. Additional details about the stimuli in each phase of the experiment are provided below.

Figure 1. Examples of sample stimuli.

(A) Example of a visual sample with 4 elements. Sample elements varied in color, size, duration, and location. (B) Example of an auditory sample with 4 elements. Individual element and inter-element interval duration varied between 200-1000 ms for both tones and squares. On all trials, choices were two simultaneous arrays of squares, only one of which matched the number of elements in the sample. All stimuli were trial-unique.

Procedure

Pretraining

Subjects had already been trained in previous experiments to numerically match a simultaneously presented sample with simultaneously presented choice stimuli for juice reward (Jordan & Brannon, 2006). However, they had never been presented with sequential sets and importantly, had never been tested with auditory stimuli.

Trial and session structure

To begin a trial, monkeys were required to press a white square located in the lower right corner of the screen. For trials that contained an auditory sample, the sample tones were then played sequentially from the speaker; for trials that contained visual sample elements, these elements appeared sequentially in the center of the screen. Immediately after the last sample element, two choice stimuli appeared. If the monkey correctly chose the numerical match, a green border flashed for 700 ms around the match, a positive sound occurred (a high-pitched ding), and a juice reward was delivered. In contrast, if the monkey touched the distracter, the screen turned black, a negative sound was emitted (a low-pitched beep), and a 3-6 second time-out ensued. No correction procedures were employed during training or testing. The inter-trial intervals varied between 1-2 seconds. Each session contained between 100 and 150 trials (approximately 30-90 minutes).

Training

Monkeys were advanced through each of 5 stages of training after reaching a performance criterion of 70% accuracy on two consecutive days. In Training Phases 1-4, samples were audio-visual and no purely audio or purely visual trials were included. In Phase 1 (2 vs 8 DMTS), the numerical values were limited to 2 and 8. The sample elements were 500ms in duration, as were the intervals between the sample elements. Thus, temporal and numerical cues were confounded in Phase 1. Monkeys M and F required 7 and 4 sessions to reach criterion, respectively. In Training Phase 2 (2,3,6,8 DMTS), the numerical values were expanded to include 2, 3, 6, and 8. Monkeys M and F required 9 and 7 sessions to reach criterion in Phase 2, respectively. In Training Phase 3 (Temporal Parameters Variation), individual sample element durations and the intervals between these elements were varied randomly within and between trials from 200-1000ms to discourage monkeys from matching based on temporal parameters such as rate and individual sample element duration. Monkeys M and F required 6 and 7 sessions to reach criterion in Phase 3, respectively. In Training Phase 4 (Surface Area Variation), the surface area of individual sample elements was varied randomly within and between trials to discourage monkeys from attending to this feature as a possible cue to the correct match. Monkeys M and F required 7 and 4 sessions to reach criterion in Phase 4, respectively.

In Training Phase 5 (Audiovisual, Auditory, and Visual Samples) 1/3 of the trials provided an audiovisual sample as in previous phases, 1/3 of trials provided a purely auditory sample, and 1/3 of trials provided a purely visual sample. By the end of this phase and for all of testing, sessions included trials that used only visual elements as samples and trials that used only tones as samples. Samples were initially audiovisual during training and only eventually purely visual or auditory because these monkeys had never before participated in a task requiring them to attend to and base an explicit response on auditory stimuli but had been trained in multiple tasks requiring them to explicitly respond to visual stimuli. Therefore, their initial training was audiovisual and loosely modeled after methodology employed by Murray and Gaffan (1994), who conducted a cross-modal match-to-sample study (unrelated to numerical cognition) in which rhesus monkeys learned to attend to sample auditory stimuli through gradual phasing out of accompanying, synchronous visual sample stimuli. In fact, only one of the two monkeys in the current study needed this gradual phasing out of visual information to learn to attend to purely auditory stimuli. Otherwise, training progressed identically for both monkeys. Monkeys M and F required 103 and 14 sessions to reach criterion in Phase 5, respectively.

A movie of a monkey completing training trials is available at http://www.duke.edu/web/mind/level2/faculty/liz/xmodal.htm.

Testing

After the monkeys learned to match novel exemplars of 2, 3, 6 and 8 across presentation format and sensory modalities on the basis of numerosity, they were tested with the novel values 1, 4, 5, 7, and 9 using the same DMTS paradigm. Here, the sample was any value between 1-9 and the test was a choice between the matching numerosity and any other value 1-9. All possible numerical samples and sample-distracter combinations occurred with equal frequency. The reinforcement was as described for Phases 1-5; thus, correct responses resulted in a squirt of juice, and incorrect responses resulted in a time-out. As in Phases 1-5, individual sample element durations and the intervals between these elements were varied randomly within and between trials from 200-1000ms to discourage monkeys from matching based on temporal parameters. During the test phase, half of the trials contained purely auditory samples and half of the trials contained purely visual samples; there were no audiovisual trials. All test stimuli were trial-unique. Monkeys were tested for 2400 trials across 24 sessions.

Results

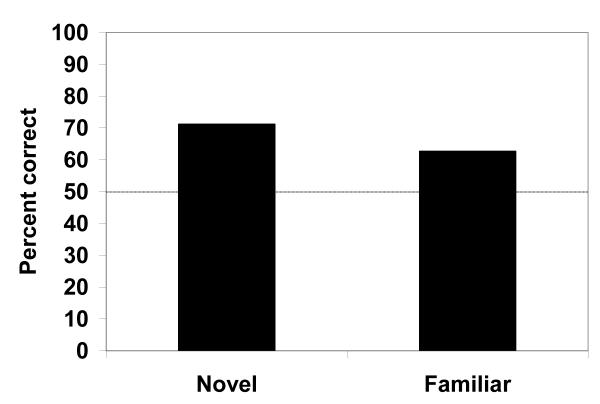
The main finding was that the monkeys matched the number of tones they heard to the number of squares they saw with above chance accuracy in the first test session (Monkey M: 70%: binomial, p<.0034; Monkey F, 64%, binomial, p<.033). Similarly, monkeys matched the number of squares they saw presented sequentially to the number of squares they saw presented simultaneously with above chance accuracy in the first test session (Monkey M: 72%: binomial, p<.001; Monkey F, 72%, binomial, p<.002). There was no significant cost in accuracy for matching between versus within modality in the first test session (Monkey M: t(98)= −.218, p=.828; Monkey F: t(98 )= −.852, p=.396). Performance was statistically equivalent on the novel (1, 4, 5, 7, and 9) (average 71.2%) and familiar (2, 3, 6, and 8) (62.7%) sample numerosities for both auditory and visual samples (Monkey M: auditory: t(48)=.596, p=.554; visual: t(48)=6.20, p=.538; Monkey F: auditory: t(48)=−.501, p=.619; visual: t(48)=−.422, p=.676; Figure 2).

Figure 2. No difference in accuracy for familiar training vs. novel test values in first test session.

Performance was statistically equivalent on the novel (1, 4, 5, 7, and 9) and familiar (2, 3, 6, and 8) sample numerosities for both auditory and visual samples. Dotted line indicates chance performance (50%).

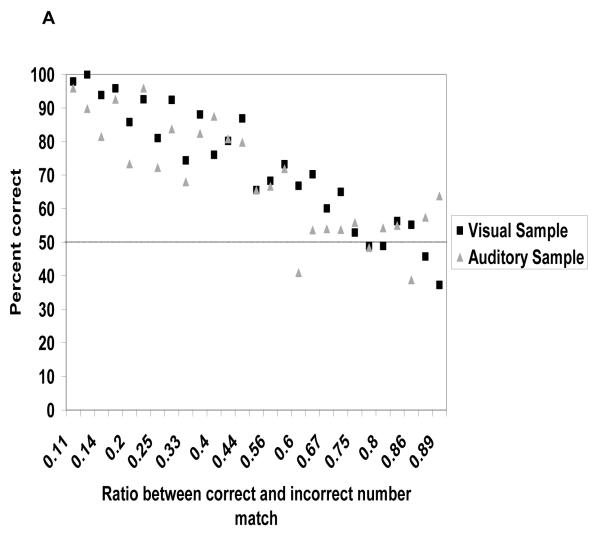
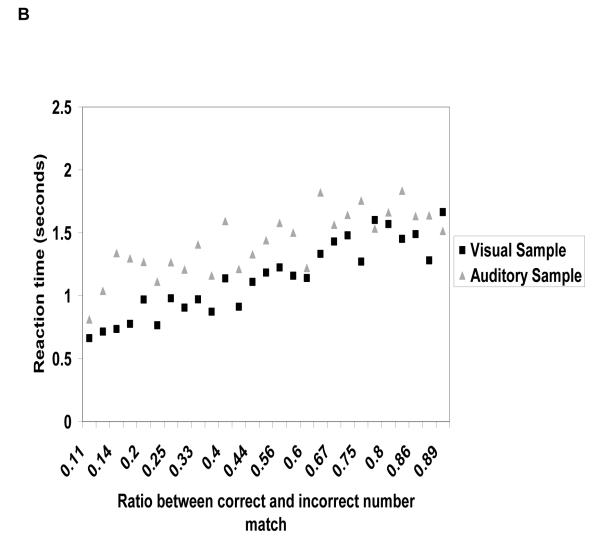
Across the 24 100-trial test sessions, monkeys continued to perform above chance expectations (Monkey F 24/24 sessions above chance accuracy, binomial p<.001; Monkey M 24/24,above chance accuracy, binomial p<.001). Neither monkey showed improvement in accuracy across the 24 sessions (Monkey F: R²=.087, p=.161; Monkey M: R²=.024, p=.473). Across test sessions, there was no difference in average accuracy for trials with visual versus auditory samples (visual average 70.4% vs. auditory average 68.4%; t(23)= −.242, p=.255). There was, however, a significant cost in reaction time for auditory (mean = 1.43 s) versus visual (mean = 1.12 s) samples (t(23)= 8.11, p<.001). Importantly, accuracy for both auditory and visual samples was modulated by ratio (auditory: R²=.69, p<.001; visual: R²=.90, p<.001; Figure 3A). Similarly, reaction time to choose the numerically matching stimulus was modulated by ratio (auditory: R²=.63, p<.001; visual: R²=.87, p<.001; Figure 3B). Thus, as the ratio between the correct numerical match and the incorrect choice approached 1, monkeys were less accurate and slower in selecting their choices.

Figure 3. Cross-modal number matching in rhesus monkeys is modulated by ratio.

(A) Average accuracy across both monkeys during the twenty-four 100-trial test sessions as a function of the ratio between the numerical value of the two choice stimuli. Black squares indicate visual samples and gray triangles indicate auditory samples. Accuracy in both modalities was modulated by ratio. Dotted line indicates chance performance (50%). (B) Average reaction time across both monkeys during the twenty-four test sessions as a function of the ratio between the numerical value of the two choice stimuli.

Experiment 2

Experiment 1 demonstrated that monkeys can actively match the number of sounds they hear to the number of sights they see. Next, we asked whether the same two monkeys could tally the number of sounds they heard and sights they saw when sounds and sights were presented in random succession.

Methods

Subjects and Apparatus

The same two subjects were tested in the same apparatus as Experiment 1.

Stimuli

Sample stimuli were sequences of both visual and auditory elements. Every sample sequence contained at least one visual and one auditory element, and auditory and visual elements occurred in random order (Figure 4). Auditory elements were tones, as described for Experiment 1. Visual elements were square shapes with the same parameters described for Experiment 1. The intervals between elements within each sample sequence also followed the parameters described for Experiment 1. Choice stimuli followed the same parameters as described for Experiment 1. One array numerically matched the sample, and the alternative choice could be any other value between 2 and 9. As in Experiment 1, all stimuli were trial-unique.

Figure 4. Example stimuli from Experiment 2.

A sample stimulus with 2 visual and 2 auditory.elements.

Procedure

Trial and session structure were the same as in Experiment 1. The only difference was that each sample contained between 2 and 9 sequentially presented elements consisting of interspersed squares and tones, and the monkey was then given a choice between an array that contained the sum of the sample elements and a randomly chosen different number of elements between 2 and 9. All possible numerical samples and sample-distractor combinations occurred with equal frequency. Reinforcement was as described for Experiment 1. Each monkey was tested for 3750 trials across 25 sessions.

Results

Monkeys tallied the number of tones they heard and the number of squares they saw with above chance accuracy even in the first 150-trial test session (Monkey F, 68%: binomial, p<.001; Monkey M, 62.6%; binomial, p<.002). Across the 25 test sessions, monkeys continued to perform above chance expectations (Monkey F, 71.6%; 25/25 sessions above chance accuracy, binomial, p<.001; Monkey M, 65.5%; 25/25 sessions above chance accuracy, binomial, p<.001) Neither monkey showed improvement in accuracy across the 25 sessions (Monkey F: R²=.119, p=.090; Monkey M: R²=.0061, p=.71).

To rule out the possibility that monkeys were ignoring stimuli from one modality in order to make the correct choice, an analysis was performed on the subset of trials (514 trials total) in which the number of auditory elements in the sample was equal to the distractor and a second subset of trials (504 trials total) in which the number of visual elements in the sample was equal to the distractor. Performance was statistically above chance on both of these trial types (auditory sample elements = distractor number, 63.8%; binomial, p<.001; visual sample elements = distractor number, 64.8%; binomial; p<.001). Monkeys were similarly accurate when a sample contained a greater number of visual elements (67.3%) and when a sample contained a greater number of auditory elements (66.0%). Performance was also equivalent with the distractor numerosity was smaller (69.1%) or larger (68%) than the correct sum.

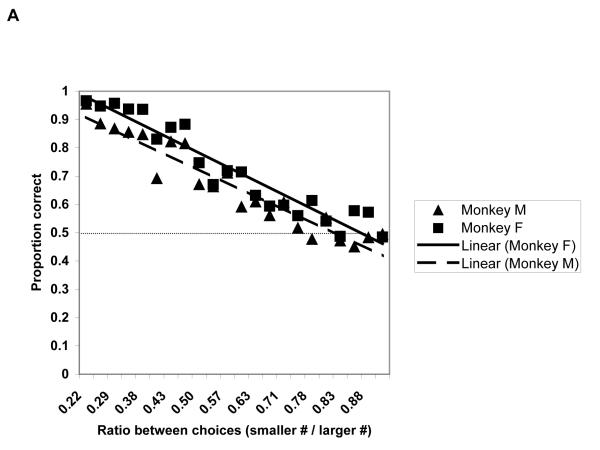
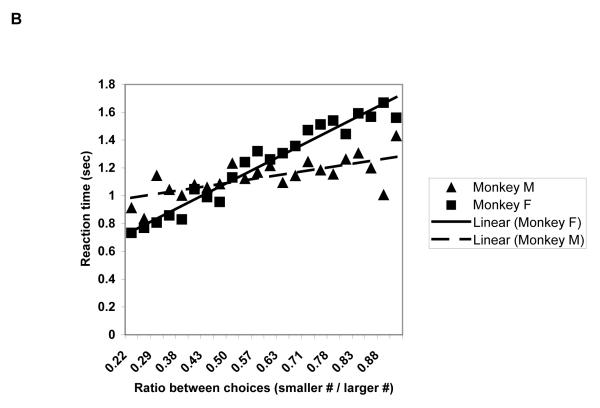
Both accuracy and reaction time to make a choice were modulated by the ratio between the correct and incorrect values. Figure 5a shows accuracy as a function of the ratio between the correct and incorrect choices (Monkey F: R²=.917, p<.0001; Monkey M: R²=.910, p<.0001). Figure 5b shows reaction time as a function of the ratio between the correct and incorrect choices (Monkey F: R²=.949, p<.0001; Monkey M: R²=.483, p=.0003). Thus, as the ratio between the correct numerical sum and the incorrect choice approached 1, monkeys were less accurate and slower in selecting their choices.

Figure 5. Summing events across sensory modalities in rhesus monkeys is modulated by ratio.

(A) Average accuracy during the twenty-five test sessions as a function of the ratio between the numerical value of the two choice stimuli. Dotted line indicates chance performance (50%). (B) Average reaction time during the twenty-five test sessions as a function of the ratio between the numerical value of the two choice stimuli.

General Discussion

Collectively, our results demonstrate that even in the first session of testing, monkeys are able to actively match the number of tones they hear to the number of squares they see; they are also able to tally the number of elements in a sequence without regard to the sensory modality in which stimuli are presented. In both experiments, monkeys matched across a large range of numerical values, and their ability to do so was modulated by ratio.

Although a previous study (Jordan et al., 2005) suggested that monkeys could match the number of voices they heard to the number of faces they saw when tested with small numbers, the design of Experiment 1 allowed us to address three novel and important questions. First, our experiment used arbitrarily related, non-ecologically relevant stimuli. This design allowed us to assess whether monkeys possess a general capacity to equate sets of sights and sounds or instead a more specific capacity that is limited to socioecologically relevant stimuli. Second, we tested monkeys with a large range of numerical values, which allowed us to ask whether monkeys rely on a ratio-dependent, analog magnitude system of representation when matching number across modalities. Finally, the operant paradigm we used allowed us to ask whether monkeys can actively make numerical matches across sensory modalities.

Furthermore, Experiment 2 was designed to address whether monkeys can tally the number of sights and sounds when they are presented in random succession—investigating a topic that only one prior study has ever examined in a non-human animal. Posthoc statistical analyses indicated that monkeys were not tallying events from only one of the two sensory modalities. If monkeys had been relying on purely visual or auditory information to make their choices, they should have reliably chosen the incorrect choice on trials in which the number of visual or auditory elements in the sample was equal to the incorrect choice. However, this was not the case: monkeys in fact performed significantly above chance on these subsets of trials, supporting our conclusion that monkeys were integrating numerical information from the visual and auditory modalities.

Data from both experiments indicate that monkeys rely on a ratio-dependent, analog magnitude system of representation when comparing and summing across modalities. Specifically, the accuracy and reaction time in performing these intersensory computations was modulated by ratio for both monkeys. This discovery of ratio-dependent accuracy in both experiments suggests that monkeys and humans rely on the same analog magnitude representations of number regardless of whether they are comparing or adding values within or between sensory modalities (Barth et al., 2003, 2006).

Interestingly, although there was no cost in accuracy for comparing number across, versus within, sensory modality in Experiment 1, monkeys did show a significant cost in reaction time when matching across versus within sensory modality. The significantly slower decision time to match across modalities may indicate additional steps in processing and should spur further research into psychophysical and perhaps neurobiological similarities and differences between crossmodal nonverbal number representation in non-human animals and adult humans.

Our results also raise questions about whether non-human animals can extract numerical information from sensory modalities other than vision and audition, and whether other arithmetic operations, such as subtraction, can be performed across sensory modalities. It is possible that in Experiment 2, monkeys were performing explicit addition which would entail combining two or more numerical representations to form a new representation, or alternatively they may have been integrating items in an ongoing accumulation process (Cantlon and Brannon, 2007). Finally, it is important to note that our experimental design rewarded monkeys in Experiment 2 for integrating items across modalities. This was necessary given that Experiment 2 was conducted after Experiment 1 in which audiovisual trials were treated as redundant representations of the same value rather than the sum of all the signals. Thus, our design did not allow us to ask whether monkeys would spontaneously integrate across sensory modalities.

Regardless, our findings demonstrate that monkeys can sum the number of sounds they hear and the number of sights they see. They do so over a large range of numerical values. Ratio dependence in accuracy and reaction time suggests that they rely on analog magnitude representations of number when performing numerical computations within or between senses. Thus, when humans nonverbally quantify sights and sounds in the world around them, they are likely tapping an evolutionarily primitive system that monkeys and perhaps many other animal species share.