Sex-specific prediction of neck muscle volumes

Abstract

Biomechanical analyses of the head and neck system require knowledge of neck muscle forces, which are often estimated from neck muscle volumes. Here we use magnetic resonance images (MRIs) of 17 subjects (6 females, 11 males) to develop a method to predict the volumes of 16 neck muscles by first predicting the total neck muscle volume (TMV) from subject sex and anthropometry, and then predicting individual neck muscle volumes using fixed volume proportions for each neck muscle. We hypothesized that the regression equations for total muscle volume as well as individual muscle volume proportions would be sex specific. We found that females have 59% lower TMV compared to males (females: 510±43 cm³, males: 814±64 cm³; p<0.0001) and that TMV (in cm³) was best predicted by a regression equation that included sex (male=0, female=1) and neck circumference (NC, in cm): TMV=269+13.7NC−233 Sex (adjusted R²=0.868; p<0.01). Individual muscle volume proportions were not sex specific for most neck muscles, although small sex differences existed for three neck muscles (obliqus capitis inferior, longus capitis, and sternocleidomastoid). When predicting individual muscle volumes in subjects not used to develop the model, coefficients of concordance ranged from 0.91 to 0.99. This method of predicting individual neck muscle volumes has the advantage of using only one sex-specific regression equation and one set of sex-specific volume proportions. These data can be used in biomechanical models to estimate muscle forces and tissue loads in the cervical spine.

1. Introduction

Neck muscle volume data are needed for most computational neck models because the volume can be used to estimate a muscle’s peak force. Biomechanical models often assume that peak muscle force is proportional to physiologic cross-sectional area (PCSA), and PCSA is estimated from the ratio of muscle volume to optimal muscle fiber length (Spector et al., 1980; Zajac, 1989).

Neck muscle volumes are commonly estimated from cadaveric morphometry studies (Kamibayashi and Richmond, 1998) or from in vivo magnetic resonance imaging (MRI) data by tracing the muscle boundaries (Chancey et al., 2003; Oi et al., 2004; Van Ee et al., 2000). In previous studies, the neck muscle volumes obtained from young healthy volunteers by MRI were larger than those from elderly cadavers, likely due to age related atrophy, peri-mortem atrophy and post-mortem desiccation in the cadavers (Chancey et al., 2003; Delp et al., 2001). A disadvantage of both approaches, however, is that they are expensive and time-consuming.

Our goal was to determine if neck muscle volumes could be estimated accurately from external measurements rather than MRI. Burnett et al. (2007) have related the MRI-based volume of seven neck muscles to external anthropometric measurements using seven regression equations—each with a different set of anthropometric parameters. These regression equations were the same for males and females even though other work has shown that males and females have significantly different head and neck geometry and neck strength (Vasavada et al., 2008a). Sex differences have also been found in the size of the limb muscles (e.g., thigh and elbow) and total skeletal muscle mass (Abe et al., 2003; Akagi et al., 2010; Chen et al., 2011). However, the sex differences in neck muscle size have not been investigated according to our knowledge.

In this study, we propose a simple, inexpensive and noninvasive approach to predict individual neck muscle volumes: first by predicting the total neck muscle volume from subject sex and anthropometry; and then by predicting the individual neck muscle volumes using sex-specific proportions of total muscle volume. We hypothesized that (1) total neck muscle volume can be predicted from sex-specific regression equations based on anthropometric data, and (2) the volume proportion of the individual neck muscles among the total neck muscle volumes is sex specific.

2. Methods

2.1. Subjects

Seventeen subjects (6 females, 11 males) underwent magnetic resonance imaging (MRI) at either Washington State University or the University of British Columbia. Subjects with a history of neck musculoskeletal disorders, metal implants, or pregnancy were excluded. The use of human subjects was approved by the Washington State University Institutional Review Board and the University of British Columbia Clinical Research Ethics Board, and all subjects provided informed consent. Each subject’s height, weight, neck length, neck circumference and head circumference were measured (Table 1). The average age of the subjects was 30 years, and the range was 20–46 years for male subjects and 23–43 years for female subjects.

Table 1.

Anthropometric data, grouped by those subjects with complete muscle data including trapezius (10 of 11 used in model development), and those lacking trapezius data (used for validation). Data shown are the average ± standard deviation, with the range in parenthesis. Neck length was defined as the vertical distance between the C7 spinous process and the tragus, measured using a wall-mounted stadiometer. Neck circumference was the average of the circumferences above and below the thyroid cartilage. Head circumference was the maximum circumference measured with a tape passing over the superciliary ridge and the opisthocranion. Percentile data are provided according to the data of Gordon et al. (1989); however, Gordon’s definition of neck circumference was slightly different: circumference at the infrathyroid landmark. Head circumference data were not available for all subjects in the group without trapezius data.

All muscle data	Female		Male
	n=3	Percentile	n=8	Percentile
Age (years)	29.9±10.8 (23–43)		31.6±8.7 (20–46)
Height (cm)	165.1±5.1 (161.2–170.9)	40%–89%	174.8±7.9 (161.1–184.0)	1%–89%
Weight (kg)	68.0±7.7 (60.7–76.0)	47%–94%	75.2±7.2 (65.3–87.3)	11%–79%
Neck length (cm)	11.3±0.9 (10.6–12.4)	78%–99%	11.8±1.9 (9.3–14.5)	13%–100%
Neck circumference (cm)**	34.5±1.3 (33.3–35.9)	87%–100%	39.1±1.9 (36.9–43.0)	30%–99%
Head circumference (cm)*	56.4±1.0 (55.6–57.6)	76%–97%	58.6±1.4 (56.3–60.0)	88%–100%
No trapezius data	n=3		n=3
Age (years)*	29.7±1.2 (29–31)		25.7±2.1(24–28)
Height (cm)*	166.2±5.3 (160.0–169.5)	33%–85%	178.0±3.5 (174.0–180.0)	41%–75%
Weight (kg)	67.7±5.8 (61.0–71.0)	49%–86%	79.3±7.4 (71.0–85.0)	26%–74%
Neck length (cm)**	11.1±0.6 (10.5–11.7)	75%–96%	13.0±0.3 (12.6–13.2)	97%–100%
Neck circumference (cm)	34.0±1.5 (32.4–35.3)	72%–98%	36.5±2.1 (35.0–38.9)	6%–70%

^*Sex differences noted by 0.01 ≤p<0.05

^**Sex differences noted by p<0.01.

2.2. MRI

Axial proton density-weighted MR images were obtained from the occiput to the T2 or T4 spinal level. Slice thickness/gap ranged from 3.0/0.3 mm to 5.0/1.0 mm among subjects. The image field of view included the trapezius muscle to its lateral border on the acromion process in 11 subjects (8 males and 3 females); in the other 6 subjects (3 males and 3 females), the field of view was smaller, and the lateral part of trapezius was not completely imaged. For the 11 subjects with the larger field of view, a wooden jig (Vasavada et al., 2008b) or foam pads were used to hold the subject’s head and neck in the neutral posture (the Frankfurt plane – the plane including the tragus of the ear and the inferior border of the orbit – was vertical while the subject was lying horizontal). In the other 6 subjects, there was no padding behind the head, resulting in a slightly extended posture.

2.3. Data analysis

2.3.1. Neck muscle volumes and total neck volume calculation

Neck muscle boundaries (Fig. 1) were outlined manually on each MRI slice, and the muscle volumes were estimated by integrating the cross-sectional muscle areas (colored areas in Fig. 1) over slice thickness. The total neck muscle volume was the sum of all individual neck muscle volumes. The individual muscle volume proportion was the ratio of each individual muscle volume to the total neck muscle volume.

Fig. 1.

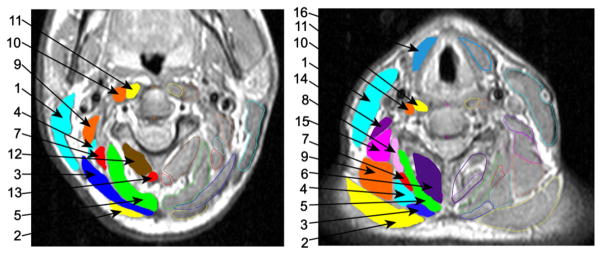
MRI scans with neck muscles outlined. Axial proton density-weighted MRI images at C2 (left) and C5 (right) of a subject in the neutral posture. Neck muscles are outlined and identified by number: 1—Sternocleidomastoid, 2—Trapezius, 3—Splenius Capitis, 4—Splenius Cervicis, 5—Semispinalis Capitis, 6—Semispinalis Cervicis+Multifidus (traced together), 7—Longissimus Capitis, 8—Longissimus Cervicis, 9—Levator Scapulae, 10—Longus Capitis, 11—Longus Colli, 12—Obliqus Capitis Inferior, 13—Rectus Capitis Posterior Major, 14—Scalenus Anterior, 15—Scalenus Posterior, 16—Infrahyoids.

The neck region (Fig. 2) for each subject was defined from the base of the skull to the slice just superior to the second thoracic vertebrae (T2). The total neck volume (from the base of the skull to T2 level) was estimated by multiplying the traced neck length ((total number of traced slices − 1) × (slice thickness+gap)) by the neck circumference around C4 (Table 1). The volume of most neck muscles was calculated from their superior attachment to the last slice above T2. Sternocleidomastoid and infrahyoids, however, were traced down to the level of their sternal attachment.

Fig. 2.

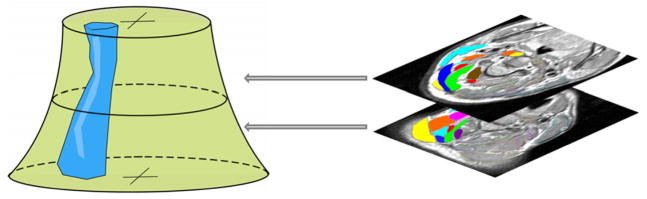
Illustration for the neck region, one neck muscle and cross-sectional views (MRI) of the neck.

2.3.2. Neck muscle volume estimation

The approach to estimate the individual neck muscle volumes involved two steps and used the muscle data from only 10 of the 11 subjects who had complete neck muscle MRI data (Table 1); one male subject was left for validation. First, the total neck muscle volume was predicted from the anthropometric data using the R² selection method (SAS Institute Inc., 2010), which performs all possible subset regressions and displays the models in decreasing order of R² magnitude within each subset size. Individual predictors screened were sex, height, (height)², weight, neck circumference, (neck circumference)², head circumference and neck length. In addition, predictors which were combinations of these factors were also screened: body mass index (BMI: mass/height²), height² × weight, (neck length) × (neck circumference)², and (neck length)/(neck circumference). Second, the individual neck muscle volume was obtained from the predicted total neck muscle volume (based on the best regression) and the individual neck muscle volume proportion.

2.3.3. Statistics for sex differences

T-tests were used to study the sex differences in muscle volume data: the total neck muscle volume, the total neck volume, the ratio of total neck volume to the total neck volume, and the individual muscle volume proportion. Equal or unequal variance t-tests were used based on the results of an F-test for equality of the variances.

2.4. Validation

The estimation procedure for individual muscle volumes was validated in two ways: using one subject with complete neck muscle volume data from MRI, and using six other subjects (Table 1) with all neck muscle volumes except trapezius. The agreement between the regression-predicted muscle volumes and MRI-estimated muscle volumes was assessed using the coefficient of concordance (ρ_c; Lin, 1989):

$${\rho }_{\text{c}}=\frac{2\rho {\sigma }_x{\sigma }_y}{{{\sigma }_x}^2+{{\sigma }_y}^2+{\left({\mu }_x-{\mu }_y\right)}^2}$$ (1)

where μ_x and μ_y are the means for the two variables, σ_x² and σ_y² are the corresponding variances, and ρ is the correlation coefficient between the two variables.

3. Results

3.1. Neck muscle volumes and total neck volume

From 11 subjects with complete muscle data, we found that females have a 59% smaller total neck muscle volume compared to males (p<0.0001), but the total traced neck volume (estimated by multiplying the area around C4 by the total traced neck length) was only 27% smaller in females (p=0.03). The ratio of the total neck muscle volume to the total traced neck volume was 25% lower in females compared to males (p=0.03; Table 2).

Table 2.

Total neck muscle volume and the total neck volume. Data shown are the average±standard deviation.

	Females (n=3)	Males (n=8)	p-value for sex difference
Total neck muscle volume** (cm3)	510.4±43.0	813.9±63.6	<0.0001
Total neck volume* (cm3)	2030.4±233.4	2571.6±326.2	0.03
Ratio of total neck muscle volume to total neck volume*	26%±5%	32%±3%	0.03

Sex differences are indicated by the p-value of a student’s t-test and noted by

^*0.01 ≤p<0.05;

^**P<0.01.

The individual neck muscle volume proportions (the ratio of the individual neck muscle volume to the total neck muscle volume, N=10 subjects) were found to be consistent among female and male subjects (p>0.05) for all muscles, except for longus capitis, obliqus capitis inferior, and sternocleidomastoid (0.01<p<0.05; Fig. 3, Table 3).

Fig. 3.

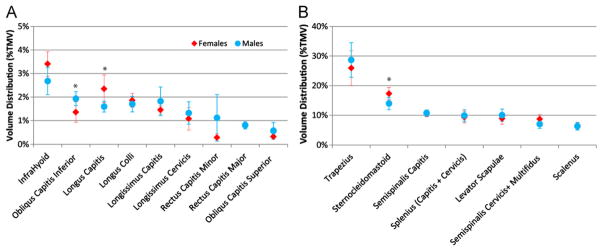
Individual neck muscle volume distribution (females vs. males). (A) Volume distribution for smaller muscles (<5% of total neck muscle volume). (B) Volume distribution for larger muscles (>5% of total neck muscle volume); ’^*’ indicates a significant sex difference in the percentage (p<0.05).

Table 3.

Individual neck muscle volume distribution. Data shown are the average ± standard deviation and p value of the t-test on sex difference

	Females (n=3)	Males (n=7)	Average (n=10)	p value
Trapezius	25.9%±2.1%	28.7%±5.9%	27.9%±5.1%	0.46
Sternocleidomastoid*	17.3%±0.9%	14.0%±2.1%	15.0%±2.4%	0.03
Semispinalis capitis	10.6%±2.0%	10.7%±1.1%	10.7%±1.3%	0.88
Splenius (capitis+cervicis)	9.3%±0.1%	9.9%±2.0%	9.7%±1.6%	0.48
Levator scapulae	8.9%±0.7%	10.0%±2.1%	9.7%±1.8%	0.40
Semispinalis cervicis+ multifidus	8.7%±0.6%	7.0%±1.5%	7.5%±1.5%	0.09
Scalenus	6.4%±1.5%	6.3%±1.2%	6.3%±1.2%	0.90
Infrahyoids	3.4%±0.5%	2.7%±0.6%	2.9%±0.6%	0.10
Obliqus Capitis inferior*	1.4%±0.4%	1.9%±0.3%	1.8%±0.4%	0.04
Longus capitis*	2.4%±0.6%	1.6%±0.2%	1.8%±0.5%	0.01
Longus colli	1.9%±0.3%	1.7%±0.3%	1.7%±0.3%	0.49
Longissimus capitis	1.5%±0.2%	1.8%±0.6%	1.7%±0.5%	0.35
Longissimus cervicis	1.1%±0.5%	1.3%±0.5%	1.2%±0.5%	0.48
Rectus capitis minor	0.3%±0.2%	1.1%±1.0%	0.9%±0.9%	0.20
Rectus capitis major	0.8%±0.1%	0.8%±0.1%	0.8%±0.1%	0.96
Obliqus capitis superior	0.3%±0.0%	0.6%±0.3%	0.5%±0.3%	0.12

^*0.01<p<0.05.

3.2. Neck muscle volume estimation

R² selection regression analysis showed that neck circumference was the best single predictor of total neck muscle volume (adjusted R²=0.636). Prediction of total neck muscle volume was improved when sex was added as a predictor. The adjusted R² was improved to 0.868 for the equation with sex and neck circumference, and 0.867 for sex and (neck circumference)². Adding a third predictor (head circumference or neck length) did not improve the prediction (adjusted R²=0.847, or 0.860). Using sex and one combination predictor, the adjusted R² was slightly larger: 0.879 for sex and BMI, and 0.871 for sex and (neck length) × (neck circumference)².

The results indicate that total neck muscle volume can be predicted by the following equations:

$$\text{Total}\text{Neck}\text{Muscle}\text{Volume}=269+13.7\times \text{Neck}\text{Circumference}-233\times \text{Sex};$$ (2)

$$\text{Total}\text{Neck}\text{Muscle}\text{Volume}=482+13.0\times \text{BMI}-297\times \text{Sex};$$ (3)

where Sex=0 for male subjects and Sex=1 for female subjects (p=0.005; ‘Total Neck Muscle Volume’ is in cm³ and ‘Neck Circumference’ is in cm). For the individual neck volume prediction below, however, we chose to use neck circumference as the predictor more closely related to neck size.

The individual muscle volume was the predicted total neck muscle volume multiplied by the corresponding sex-specific muscle volume proportion (Table 3). Using this method, individual neck muscle volumes could be predicted with good agreement to MRI-estimated volumes. The coefficient of concordance was 0.99 when assessing the agreement between the predicted individual neck muscle volumes and MRI-estimated neck muscle volumes for the male subject with complete neck muscle data who was not used in developing the model (Fig. 4). Furthermore, six subjects without trapezius volume data were also used to validate this two-step muscle volume prediction (data for a female subject with a relatively low concordance coefficient of 0.92 is shown in Fig. 5). The coefficients of concordance between the predicted individual neck muscle volumes and MRI-estimated neck muscle volumes for these six subjects were 0.94±0.02, ranging from 0.91 to 0.96 (Table 4).

Fig. 4.

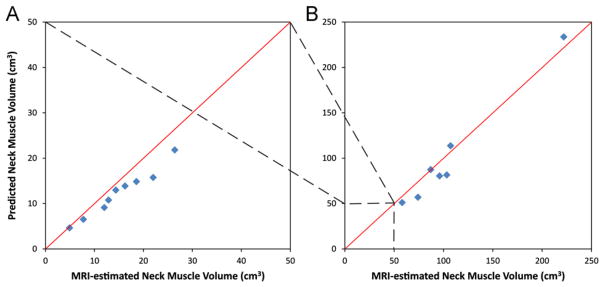
Predicted muscle volumes vs. MRI-estimated muscle volumes. Each symbol represents the muscle volume for a specific muscle (sum of left and right side muscles) relative to the unity line (diagonal line). Data shown are for a male subject (with complete neck muscle data) that was not used to generate the regression equation or muscle volume distribution; coefficient of concordance=0.99. (A) Smaller muscles (same muscles as in Fig. 3A). (B) Larger muscles (same muscles as in Fig. 3B).

Fig. 5.

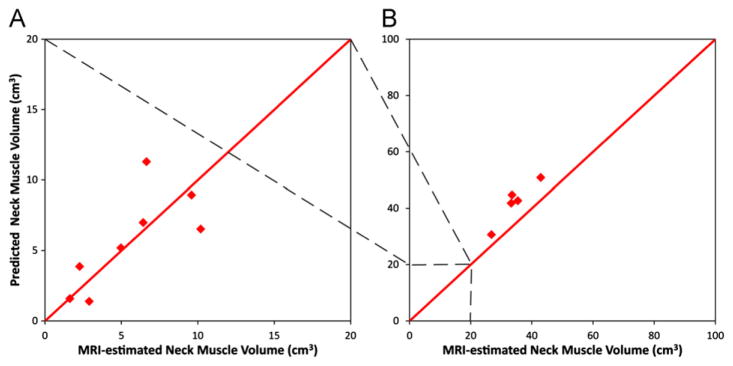
Predicted muscle volumes vs. MRI-estimated muscle volumes. Each symbol represents the muscle volume for a specific muscle (sum of left and right sides) relative to the unity line (diagonal line). Data shown are for a female subject without trapezius volume data that was not used to generate the regression equation or muscle volume distribution; coefficient of concordance=0.92. (A) Smaller muscles (same muscles as in Fig. 3A). (B) Larger muscles (same muscles as in Fig. 3B except for trapezius).

Table 4.

Range of the subject anthropometric data and coefficient of concordance.

	10 subjects (3 F, 7 M) for regression (with complete neck muscles)	One male subject for validation (with complete neck muscles)	6 subjects (3F, 3M) for validation (with all neck muscles except trapezius)
Age
Male range	20–42	41	24–28
Female range	23–43		29–31
Height
Male range	161.1–181.4	186	174.0–180.0
Female range	161.2–170.9		160.0–169.5
Weight
Male range	65.3–87.3	82	71.0–85.0
Female range	60.7–76.0		61.0–71.0
Neck circumference
Male range	36.9–43	38.9	35.0–38.9
Female range	33.3–35.9		32.4–35.3
Neck length
Male range	9.3–14.5	14.0	12.6–13.2
Female range	10.6–12.4		10.5–11.7
Coefficient of concordance		0.99	0.91–0.96

4. Discussion

We have demonstrated that it is possible to predict individual neck muscle volumes accurately using sex, neck circumference, and a set of sex-specific neck muscle proportions. The results support our first hypothesis, that the total neck muscle volume regression equation is sex specific. Because three muscles have sex-specific volume proportions, the data only partially support our second hypothesis, that the proportions of individual neck muscle volumes to total neck muscle volume are sex-specific. Mainly because the volume proportion of sternocleidomastoid (one of the major neck muscles) is sex-specific, we decided to calculate the individual neck muscle volumes by multiplying the sex-specific muscle volume proportion by the total neck muscle volume (predicted by the sex-specific regression). The muscle regression models were validated using independent subjects, whose heights were within or slightly beyond the height range of the subjects used to develop the models (Table 4).

The method developed here uses only sex and a single anthropometric measurement to accurately predict the volume of 16 neck muscles. This method is considerably simpler than the seven regression equations—each relying on a different set of anthropometric measures—developed by Burnett et al. (2007), yet provides good accuracy (adjusted R²=0.87).

This study also confirms previous studies that suggest sex differences in neck muscle size (Vasavada et al., 2008a). The individual neck muscle volumes were found to be significantly larger in males, despite no significant sex difference in the neck length. This result implies that the cross-sectional areas of the neck muscles (and thus their capacity to generate force) are larger in males than females, and is consistent with an ultrasound study in which the cross-sectional areas of the deep posterior neck muscles were found to be significantly larger in male subjects (Rankin et al., 2005). It is also consistent with comparisons of whole-body muscles, where males tend to have larger muscles than females in both absolute and relative terms (Abe et al., 2003; Janssen et al., 2000; Lee et al., 2000).

Several methodological issues and limitations should be recognized for the interpretation of these results and future use of this method. First, the MRI data from two institutions were acquired with different slice thicknesses. To examine the effect of slice thickness on muscle volume calculation, we re-calculated the volume of the 6 subjects who had slices 3.3 mm apart using every alternate slice. The average difference in muscle volume calculation was 6%±11%. However, only the small suboccipital muscles (which comprise only 3.5% of the total neck muscle volume) had differences greater than 10%. For all of the other muscles, the average difference in muscle volume calculation between the 6.6 mm and 3.3 mm slice thicknesses was 2% (±2%).

In MRI data, slight differences in muscle cross-sectional area measurements may occur due to the orientation of the slices. We attempted to control the subject’s posture as close to neutral as possible, and slight postural variations are unlikely to significantly affect muscle volume estimates. Preliminary data examining MRI muscle volume estimate variations with posture revealed that the total neck muscle volume varied 1% in scans with 30° flexion–extension rotation, 2.5% in 30° axial rotation, and 5% in 10° lateral bending compared to the neutral posture. Individual neck muscle volumes varied, on average, by 5% among postures compared to the neutral, and the maximum variation in a single subject was up to 10–12% error compared to the neutral posture. The largest variation with posture in calculated muscle volume is in the small, suboccipital muscles. The postural variation from neutral in this study was much smaller than those mentioned above; therefore we also expect smaller variation in volume due to posture.

Finally, the posterior neck muscles were truncated at the T2 level for consistency. Although some neck muscles continue below T2, they become difficult to distinguish from thoracic muscles on the MRI scans. The entire volume of trapezius could not be determined because it would have required a much longer scan. Therefore, the trapezius volume presented here is the volume from its superior attachment on the skull, to a horizontal plane through T2 because that is the portion of trapezius relevant to neck models.

Although our sample size was low—especially for females–a post-hoc statistical power analysis on t-test analysis of the sex differences in the total neck muscle volume yielded a power of 0.90. Further, even with this limited number of female subjects we were able to predict neck muscle volumes with close agreement in other female subjects. However, the female subjects were close to average or above average height, so the muscle volume prediction should be validated on smaller females in the future.

A caveat of using this method is that the regression equations were developed using data from a group of men and women of a specific size and age (Table 1). The age range of subjects was 20–46 years. Currently there are no data available for neck muscle volumes in an elderly or pediatric population; therefore, we do not recommend extrapolating these results beyond a healthy young adult population. The BMI of our study population ranged from 23 to 29 (normal to overweight); there were no obese or underweight subjects, and it is not known if these results will apply outside this range of BMI.

This method provides a simple and accurate estimation of neck muscle volume for biomechanical modeling. It incorporates the known sex differences in neck muscle size and the relative sizes of muscles that are consistent among subject size. Independent validation showed good correspondence with MRI-predicted neck muscle size over a range of sizes in both males and females.