Chaotic advection in blood flow

Abstract

In this paper we argue that the effects of irregular, chaotic motion of particles transported by blood can play a major role in the development of serious circulatory diseases. Vessel wall irregularities modify the flow field, changing in a nontrivial way the transport and activation of biochemically active particles. We argue that blood particle transport is often chaotic in realistic physiological conditions. We also argue that this chaotic behavior of the flow has crucial consequences for the dynamics of important processes in the blood, such as the activation of platelets which are involved in the thrombus formation.

I. INTRODUCTION

It is well known that streamlines and particle trajectories are different in non-stationary fluid flows. In particular, simple flow fields may give rise to a complex dynamics of the advected particles. Even in very simple flows, such as, for example, laminar time-periodic ones, the particle trajectories are in general very complex, and often display chaotic behavior [1]. This chaotic advection has been shown to have far-reaching consequences for the dynamics of active flows [2] — flows in which some kind of chemical or biological activity takes place. Application of the theory of transient chaos to the dynamics of active processes has shown that advective chaos changes the effective rate equations of the active processes, and in many cases it enhances the total productivity of the reaction (or biological process) [4]. This phenomenon is very general, and has found applications in a number of systems, including atmospheric chemistry [5], plankton population dynamics [3, 6] and even the early evolution of life [7]; for a review see [8].

Blood flows in our veins and arteries follow a pulsating pattern, driven by the heart. This means that we can model blood flow as a time-periodic flow; the above discussion then suggests that the motion of advected particles may be chaotic. It is very important to investigate this possibility, since blood is an active flow. The many kinds of cells and organic molecules making up blood are not just carried passively by the flow around them; they participate in a multitude of chemical reactions and biological interactions. The rates and other properties of these processes will be crucially affected by the dynamics of the flow if it has chaotic advection. Although there are plenty of studies based on numerical simulations of blood flow, to our knowledge there is no study addressing the issue of chaotic advection and its consequences in blood flow.

The purpose of this paper is to use the concepts, tools and techniques of dynamical systems to investigate the dynamical properties of blood flow, and in particular to study under what conditions it has chaotic advection. We use simple models for the flow in blood vessels, and choose realistic physiological values for parameters such as the flow velocity and period. We focus on the case where the vessel has a localized anomaly in its diameter. This anomaly can be either a constriction — a stenosis, partial blocking of the vessel —, or a sudden enlargement — an aneurysm. This topic has great medical relevance, and such anomalies are directly related to the cause of serious and often fatal circulatory diseases.

Our main result is that under most conditions, sudden changes in the geometry of the vessel, such as constrictions or aneurysms, result in chaotic advection in the blood flow. This fact has a number of important consequences. Active processes can be greatly enhanced in chaotic flows, a fact which is not taken into account in the traditional study of physiological and biochemical phenomena taking place in blood. We argue that advective chaos must be properly taken into account for processes such as platelet activation and thrombus formation, which are associated with vessel constrictions and thus will be affected by the chaotic nature of the advection. We show that chaos induces a large increase in the average residence time of an advected platelet, which means it will have more time to become reactive and adhere to the vessel wall. We also show that the spatial distribution of particles with long residence times follows a fractal pattern, which has a strong effect on the dynamics of platelet activation and of other processes.

The paper is organized as follows. Chaotic advection in fluids is briefly reviewed in Section II. The models we use in our simulations and the choice of parameters, as well as numerical methods, are introduced in Section III. In Section IV we study the fractal spatial filamentary distribution of advected particles induced by chaos, and discuss on its importance for platelet activation and other active processes in the flow. In Section V we show how the residence time of particles behaves for the different anomalies. Then in Section VI we discuss the results and outline the possible applications resulting from the emerging fractal structures and their effects on the active processes in blood flows. Finally, we summarize our conclusions in Section VII.

II. CHAOTIC ADVECTION

Blood flow in blood vessels falls in the class of open flows. In open flows one usually defines a region of observation, which in our case is some appropriate region surrounding an anomaly in the vessel wall’s shape. The fluid transports particles into the region of observation, where it will spend some time, and then it will usually be washed out downstream. Before leaving this region, however, the particles may exhibit very complicated, chaotic behavior. In open flows, there is typically a set of advected particles that never leave the region of observation, and get trapped in the so-called chaotic set, which is the set of all particle orbits trapped permanently in the region of observation [1]. The chaotic set hence lies entirely in the region of observation.

The particle orbits of the chaotic set are exceptional (in the sense that the chaotic set has null measure), and unstable particles in their vicinity will almost always deviate from the chaotic set and leave the region of observation. Despite this, the chaotic set governs the long-time dynamics of the system; particles that happen to come close to the chaotic set wander in the vicinity of the trapped orbits for a long time, and eventually leave them (along the so-called unstable foliation). This mechanism gives rise to very large residence times for some particles. This will be discussed further in Sec. V.

One of the most visible effects of chaotic advection in open flows is that the particles with long residence times accumulate along a characteristic filamentary fractal structure. When the particles are chemically or biologically active, the effective rate equation governing the dynamics of the corresponding active process is determined by the fractal dimension of the filamentary set [4, 6, 8]. We will see that such filamentary structures are present in blood flow in many conditions, and argue that their presence has a major impact on the dynamics of important biochemical processes such as platelet activation.

A conspicuous feature of chaos is the exponential separation of nearby trajectories, which is caused by the combination of stretching and folding dynamics present in all chaotic systems. It is well established that the blood particles responsible for thrombus formation - platelets - are activated in regions of elevated shear stress [9, 10]. Chaos provides a natural mechanism for activating platelets in this way, since the stretching and folding in the dynamics deform them. This facilitates their activation and later deposition in low shear stress regions.

Next we introduce the models we use to investigate these issues.

III. FLUID MODEL

To investigate how anomalies in arterial wall shape affect chaotic mixing, we use simple models to mimic blood flow in two different pathological conditions: partly blocked vessels in coronary arteries and aneurysms in abdominal aortas.

In order to find the trajectories of particles transported by the blood, first the velocity field v(r, t) of the flow has to be computed. To achieve this, we use the finite volume solver Fluent [11].

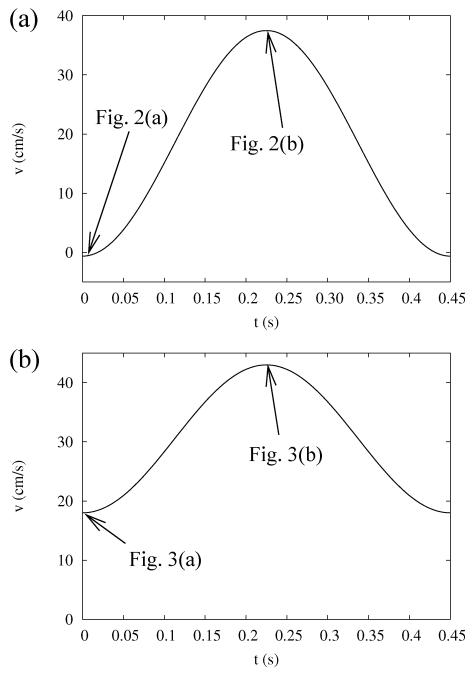
Blood is considered to be incompressible [12] and Newtonian [12, 22] with a constant dynamic viscosity μ = 0.04 g/cm·s [23]; the density of the blood is well approximated to be ρ = 1.06 g/cm³ [24]. Besides the no-slip boundary condition on the rigid surface of the vessel wall, the time-dependent inlet-velocity of the blood flow is specified both for the coronary artery and the aorta. The model used for the time-dependence of the inlet velocity is shown in Fig. 1(a) for the coronary artery and in Fig. 1(b) for the aorta during one heartbeat in exercise conditions [12]. In exercise, the resting stage of the cardiac cycle, witch is a characteristic for resting conditions, is missing, and the flow rate can be modelled by a rapid sequence of pumping stages. The flow rates shown in Fig. 1 are approximations of measured flow rates; they are simple enough for a mathematical treatment, while at the same time being reasonably close to the measured values.

FIG. 1.

Model of the inflow velocity in case of (a) coronary artery and (b) abdominal aorta during one heartbeat cycle. The arrows point to time instances when the streamlines are shown in Figs. 2 and 3.

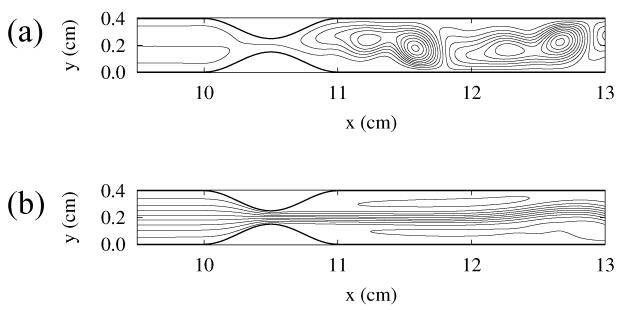
Figures 2 and 3 show snapshots of the streamlines, characterizing the flow patterns for the partly blocked artery and for the aneurysm, respectively.

FIG. 2.

Snapshots of the streamlines of the blood flow in the 2D model of an obstructed coronary artery. The time instances of snapshots are shown with arrows in Fig. 1(a).

FIG. 3.

Snapshots of the streamlines of the blood flow in the 2D model of an aorta with aneurysm. The time instances of snapshots are shown with arrows in Fig. 1(b).

We assume that it is only the fluid motion that governs the behavior of the transported particles, and the effects of diffusion are negligible. In fluid flows, the importance of the kinematic effects relative to the diffusion are characterized by the Péclet number [13]

$$\mathrm{Pe}=\frac{Rv}{D_{\mathrm{diff}}},$$ (1)

where R is the radius of the blood vessel, v is the average flow velocity, and D_diff is the diffusion coefficient. For large enough blood vessels, arteries and aortas, the radius is on the order of 1 cm, and v = 10 cm/s is a typical velocity. For platelets, the diffusion coefficient is D_diff = 10⁻⁷ cm²/s [14], which implies that the Péclet number is Pe = 10⁸. Even if we take processes on the length scale of platelets, R ≈ 10⁻⁴ cm, we have Pe = 10⁴. These very large values indicate that in blood vessels of large diameter the effects of diffusion are negligible.

Despite the relative simplicity of the flow field, the motion of particles transported by the blood is generally very complex. The finite size and inertia of the particles, the contact between particles of various shapes and sizes, the feedback of the particle motion to the flow renders it infeasible to follow the motion of too many particles. Also, many of these effects are not precisely known and are difficult to model. As a first step, we use a simple approximation, which assumes that the particles take on the velocity of the fluid instantaneously, without inertia, and provide no feedback to the flow. Then the particle at a position r(t) = (x(t), y(t)) takes on instantaneously the velocity v(r, t) of the fluid at that location:

$$\frac{d\mathbf{r}\left(t\right)}{dt}=\mathbf{v}(\mathbf{r},t).$$ (2)

Depending on the actual flow pattern, the fluid velocity on the right-hand side of this equation is usually a nonlinear function of the position r and time t, and the solutions of this equation are typically chaotic, even if the flow field v(r, t) is regular and non-turbulent.

IV. FRACTAL FILAMENTS

The sensitive dependence on the initial conditions implies that when we inject a blob of particles into a flow displaying chaotic advection, the initially close particles in the blob rapidly diverge from each other. As a consequence, the stretching and folding action of the chaotic dynamics generates a characteristic pattern of long, winding filaments with an intricate structure. The fractal filaments trace out the unstable foliation of the chaotic set responsible for the complicated behavior of the advected particles.

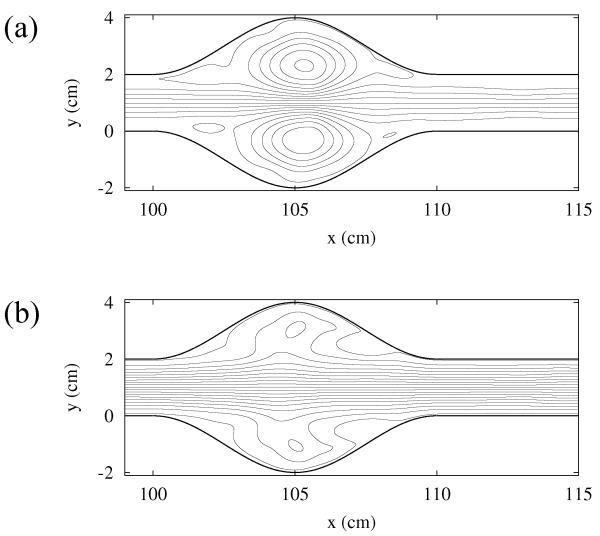
In order to see if blood flow near shape anomalies is chaotic, we use the blood flow model introduced in the previous section to follow the trajectories of many particles simultaneously, initially located evenly all over the observed region. The patterns traced by the initial blob are shown in Fig. 4 for the model of the coronary artery, and in Fig. 5 for the model of the aorta with aneurysm. Both figures show the location of the particles which have not been washed out after some given interval, chosen to be much longer than the flow’s period. The particles with long residence times either accumulate along the vessel wall, where the fluid is very slow, or along the evident filamentary structure. The visual appearance of Figures 4 and 5 strongly suggest that both cases have chaotic advection. To confirm this, we compute the fractal dimension D in both cases. We find the values D = 1.72±0.02 for the coronary artery, and D = 1.67 ± 0.02 for the aorta with aneurysm; this confirms that particle advection is indeed fractal due to the presence of chaos in the underlying dynamics.

FIG. 4.

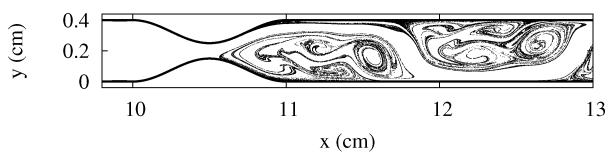
Snapshots of the unstable foliation of blood flow in the stenosed coronary artery.

FIG. 5.

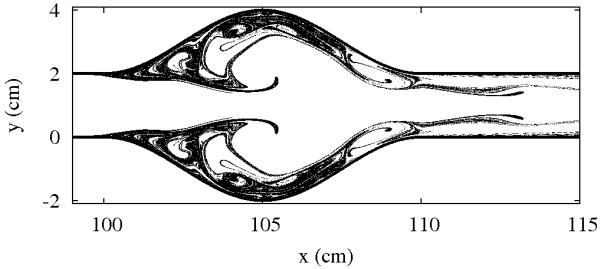
Snapshots of the unstable foliation of blood flow in the aorta with aneurysm.

We note that no fine tuning of parameters was necessary to find the chaotic regime; it is very typical, and present for a broad range of parameters. We also emphasize that the parameters where chaos is found are realistic, and fall well within the range of normal human physiology. Therefore, chaotic advection is a real property of blood flows in the presence of aneurysms and blocking vessel wall structures.

The fractal nature of the particle distribution implies that biochemically active particles such as platelets tend to concentrate along filaments like those in Figs. 4 and 5. Considering that these particles are strongly mixed and stretched along these filaments, the majority of the activity takes place along them.

Mathematically, the filaments are approximations of the unstable foliation of the chaotic set [17]. The unstable foliation is not a static structure, since the flow is time-dependent; but in a time-periodic flow, the set traced out by all these trapped particles repeats itself with the period of the flow. The periodic oscillation of the unstable foliation also means that parts of it can overlap with regions of the flow with high shear stress, for example at the throat of a stenosis, and other parts overlap with more stagnant regions. These regions correspond to the location of high activation rates of platelets (for example), or to the location of their deposition, respectively. The interplay of high mixing rates and increased perimeter due to fractality with the effects of high or low shear regions is expected to affect greatly biochemical processes in the blood.

V. RESIDENCE TIME

A very important property of the trapped blood particles is their residence time — the time the particles spend in the region of observation — in the vicinity of the vessel wall irregularities. For example, platelets need to spend a minimum amount of time in the high shear regions in order to be activated, and they also need time to attach to the wall and increase the blocking structure. The presence of chaos affects dramatically the residence time of advected particles in the blood: particles which get close to the unstable foliation stay in the region of observation for a very long time, while others are rapidly washed downstream.

Figure 6 gives a global image of the residence time as a function of the initial position of the particles in a coronary artery in exercise condition. The time the particles spend in the region of observation is shown with color coding. A lighter color indicates that the particle starting from that point stays longer before transported downstream, while darker colors correspond to shorter residence times.

FIG. 6.

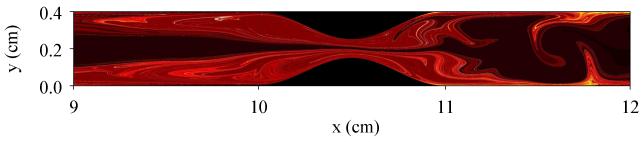
Time spent in the region of observation as a function of the initial position. Lighter colors indicate long residence time.

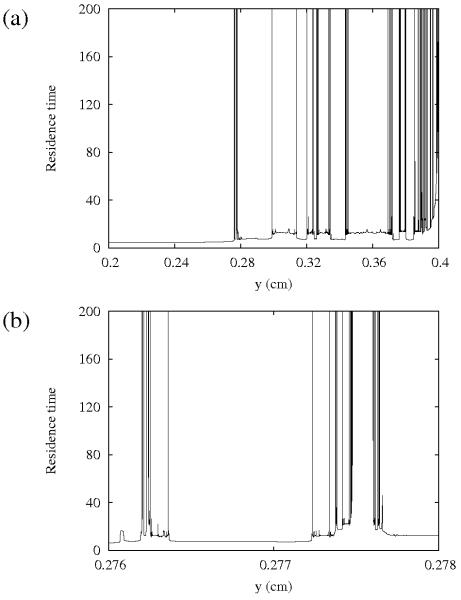
Figure 7(a) gives more insight into the intricate structure of the residence time function. The residence time is plotted as a function of the initial position of the particles starting from a straight line across the blood vessel. It is clear that the particles initially closer to the wall, in general, tend to spend more time in the region of observation. This is a consequence of the “stickiness” of the vessel wall, caused by the fact that the velocity of the flow is very small close to the wall. Besides this effect, there are also high peaks present in regions far away from the walls, and they have a quite intricate structure. These high peaks are the consequence of the chaotic particle trajectories. The distribution of the high peaks is quite irregular, but has a characteristic fractal structure: the peaks are at the intersection of the line of initial conditions with the unstable foliation. Therefore, the fractal dimension of the set of these high peaks is one less than that of the corresponding unstable foliation [16]. Subsequent magnifications reveal more of this intricate structure, as illustrated in Fig. 7(b), which shows a blow-up of a region of Fig. 7(a). The high peaks and the rapid, irregular changes between long and short residence times illustrate the stretching properties: initially close particles may follow completely different orbits as a result of high stretching and folding.

FIG. 7.

Residence time in the region of observation as a function of the initial position in resting conditions.

The same high stretching affects particles, like platelets or von Willebrand factor, which is a molecule associated with platelet activation in high shear regions [25] via a conformational change resulting in attachment. We argue that one must take into account not only the activation due to high shear, but also the exponential separation of nearby points in chaotic flow and the fractal distribution of particles with long residence times.

We found fractal structures and fractal residence times similar to the ones shown here for many conditions, including less severe vessel wall irregularities and under resting conditions, showing that chaotic advection is in fact a very common regime in blood flow.

In a non-attracting chaotic system such as open flows, it has been shown that the decay rate of particles obeys the following law [17]:

$$N\left(t\right)=N\left(0\right)\exp (-t∕\tau ),$$ (3)

as long as the dynamics is hyperbolic. Here N(t) is the number of particles in the region of observation at time t and τ is the mean residence time.

Although strictly speaking our problem is not hyperbolic because of the stickiness caused by the walls, we measure the mean residence time by obtaining the residence time for many particles starting from initial positions away from the walls, and then we fit the results to Eq.(3). The results obtained for the severely constricted artery in exercise conditions (c.f. Fig. 4) gives τ = 19.2 s, while the result for the aorta with the aneurysm (c.f. Fig. 5) is τ = 2.01 s. Note that these values are quite large compared with the period of the heartbeat, which is 0.45 s as shown in Fig. 1 for the exercise conditions. The severely constricted artery result especially shows dramatically that chaos can induce a very large residence time.

The residence time of any given particle depends sensitively on its initial position. There is a well-known relation connecting the residence time τ, the fractal dimension D and the Lyapunov exponent λ: [15]

$$\tau =\frac{1}{\lambda (2-D)}.$$ (4)

This relation is valid for hyperbolic open flows.

By using relation (4), we can compute the Lyapunov exponent λ from the measured fractal dimension D and mean residence time τ. The computed value for the coronary artery (Fig. 4) is λ = 0.187 s⁻¹, that for the aorta with the aneurysm (Fig. 5) is λ = 1.648 s⁻¹. Comparing this result with other simulations (not shown) carried out with smaller vessel wall irregularities and in resting conditions, we found that the more severe flow disturbance and the more strenuous exercise regimes lead to higher values of the Lyapunov exponent, and hence the particle motion becomes more chaotic. We also found consistently that chaotic advection makes an appearance in different sizes and shapes of anomalies.

VI. DISCUSSION

In this paper we draw attention to the effects of kinematic characteristics of mixing in the blood. The theory of nonlinear dynamics, and in particular chaotic advection, is the appropriate framework to address this issue. This, in parallel with our simple numerical studies, reveals the fractal filamentary distribution of particles with long residence times close to vessel wall irregularities. We emphasize that this feature is independent of the details of our model, and it is expected to hold for particles transported by blood under general conditions. Similar sensitive dependence of residence times and particle orbits on initial positions has been found in simulated blood flows in previous works [18], which is consistent with our results. This phenomenon has its origin in the chaotic nature of the advection dynamics of the flow.

In the blood, the advected particles can be active in a biochemical sense. Some previous models [18] have suggested to include the residence time of the particles in their activation and adherence model. However, they measured a time-averaged residence time over periods of the pulsating flow, which renders the filamentary structures invisible. We know, however, that the fractal nature of these filamentary structures are very important for the dynamics of active processes, including chemical reactions and biological activity. When activation takes place along fractal filaments the fractal dimension appears in the biological rate equations. This is the result of the very long fractal interface between different chemical substances or biological species; the consequence is the enhancement of the rate of activity. The fractal filaments serve as the skeleton and the dynamical catalyst of the activity. The production term of the biological activity has been shown to follow the scaling law [8]

$$P\sim c^{-\beta },$$ (5)

where c is the concentration of the chemically active material, and β = (D − 1)/(2 − D) depends uniquely on the fractal dimension of the unstable foliation. As the fractal dimension D is between 1 and 2, β is always positive, which implies that the production rate increases as the concentration decreases; as the concentration approaches zero, the production rate diverges. This seems at first a very counter-intuitive phenomenon, and it shows how dramatically chaos can affect the dynamics of active processes.

We have shown that these filamentary structures are present in blood flow in many situations, both in resting and exercise conditions. Their presence impacts greatly on the dynamics of active processes taking place in the blood. An important example is platelet activation and deposition, which plays a major role in blood clotting and thrombus formation. We suggest that the large stretching experienced along the unstable foliation enhances the activation of platelets. These effects — the fractality of the unstable foliation where residence time is long (τ is much longer than the heartbeat cycle) and stretching is high (λ > 0) — lead to an enhanced activation of the platelets along the unstable foliation. The activation of platelets is expected to follow the general production equation (5), hence the chaotic dynamics and the fractality of the distribution of the transported inactive platelets is expected to play a major role in their dynamics.

It has been observed that atherosclerotic plaques or thrombi [22, 26] usually build up downstream from the flow disturbances. This is in the recirculation region of the flow, where shear is quite low; but this is also the location of chaotic particle orbits and filaments of long residence times. All of these effects point in the direction of distal growth of plaques or thrombi: the long residence time and the filamentary structure promote attachment, while low shear helps attached particles to remain bounded to the vessel wall.

Similarly, the stagnating flow has an important role in aneurysms. In these regions of vessel wall dilation, blood particles spend a long time, as believed, trapped by vortices forming in the bulge of the vessel wall [27] reducing the amount of “fresh” particles, and hence oxygen, brought by the flow to the aneurysm. The long residence time in the aneurysm then leads to weakening of the wall and the increased potential of clotting and thrombus formation [18].

VII. CONCLUSIONS

Recent advances in the field of chaotic advection provide the impetus to revisit the dynamics of particles transported by blood flow in the presence of vessel wall irregularities. Each irregularity, being either a narrowing or expansion of the vessel, generates time-dependent flow patterns which can result in very complex motion by the advected particles. We have shown, using numeric models with realistic parameters, that the dynamics of particles transported by the blood flow in vessels with wall irregularities is typically chaotic. A consequence of this chaotic advection is the appearance of a characteristic filamentary distribution of advected particles. The particles transported by the blood which spend a long time around a disturbance either stick to the vessel wall or reside on fractal filaments. We argued that the non-trivial long-time distribution of transported particles has major effects on biochemical processes occurring in blood flow, including the activation and deposition of platelets. A clear future direction in this field is to take the enhancement of active processes by chaotic activation into account in a realistic model of platelet activation (and similar processes), and derive testable predictions from this.