Abstract
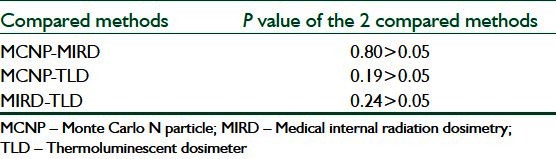
Radioiodine therapy is an effective method for treating thyroid cancer carcinoma, but it has some affects on normal tissues, hence dosimetry of vital organs is important to weigh the risks and benefits of this method. The aim of this study is to measure the absorbed doses of important organs by Monte Carlo N Particle (MCNP) simulation and comparing the results of different methods of dosimetry by performing a t-paired test. To calculate the absorbed dose of thyroid, sternum, and cervical vertebra using the MCNP code, *F8 tally was used. Organs were simulated by using a neck phantom and Medical Internal Radiation Dosimetry (MIRD) method. Finally, the results of MCNP, MIRD, and Thermoluminescent dosimeter (TLD) measurements were compared by SPSS software. The absorbed dose obtained by Monte Carlo simulations for 100, 150, and 175 mCi administered 131I was found to be 388.0, 427.9, and 444.8 cGy for thyroid, 208.7, 230.1, and 239.3 cGy for sternum and 272.1, 299.9, and 312.1 cGy for cervical vertebra. The results of paired t-test were 0.24 for comparing TLD dosimetry and MIRD calculation, 0.80 for MCNP simulation and MIRD, and 0.19 for TLD and MCNP. The results showed no significant differences among three methods of Monte Carlo simulations, MIRD calculation and direct experimental dosimetry using TLD.
Keywords: Absorbed dose, Monte Carlo N particle, medical internal radiation dosimetry, phantom, radioiodine therapy, thyroid cancer, thermoluminescent dosimeter
INTRODUCTION
Radioiodine ablation after total or near-total thyroidectomy is a standard procedure in patients with differentiated thyroid carcinoma.[1] In this therapy method, the prescription of sodium iodine is done orally.[2,3] After this procedure, the radioiodine concentrates in the functioning primary or secondary cancer of the thyroid destroying the tumor. The amount of activity of 131I is around 200 mCi for safety aspects. However, a higher administered activity is desired to achieve higher tumor doses.[4–7] treatment with 131I may result in abnormalities in other organs so it is important to estimate organ doses.[2] Protection against ionizing radiation requires information on the absorbed doses in organs of the human body. Implantation of many dosimeters in the human body is undesirable (or impossible), so the doses in organs are not measurable and some kind of dose calculation has to be applied.[5] The use of a well-supported radiation transport code such as MCNP with knowledge of patient anatomy will result in a significant improvement in the accuracy of dose calculations.[6–8]
Dosimetry is required by the clinician for several reasons. First, treatment is often limited by the dose delivered to critical organs, for example bone marrow. Second, dosimetry is required to prescribe the correct activity of radioiodine. Indeed, internal radiation dosimetry of radiopharmaceuticals is an important aspect of nuclear medicine to weigh risk versus benefit considerations. In MIRD method, the dose absorbed in the target organs are estimated by the activities accumulated in the source organ.[4]
The aim of this study was to obtain absorbed dose of organs using MCNP simulation method and the results were compared with MIRD method and experimental method (TLD) by performing a t-paired test.
MATERIALS AND METHODS
The mathematical phantom is a representation of the human body. In these phantoms, all organs are represented with geometrical bodies (like cylinders, ellipsoids, tori, etc.), which are described with suitable mathematical equations. A corresponding chemical constitution for various types of organ tissues is also defined. For the theoretical simulations of the thyroid, sternum and cervical vertebra MIRD mathematical phantom have been used.[9]
The first step is to prepare the input file. The MCNP input file describes the geometry problem, specifies the materials and the source, and defines the desired result from the calculation. Based on the Monte Carlo photon transport techniques, a computer program was developed. The program assumes a uniform distribution of 131I in the thyroid volume.
Both the F6 and *F8 tallies of MCNP code can be used for determination of absorbed dose by MCNP method, but since F6 tallies is not defined for electrons and since 131I emits beta particles, in this study *F8 was used to calculate absorbed dose. The *F8 tallies, which give results in MeV, were later converted to Gy by dividing MeV by the mass within the cell and multiplying by 1.602E-8 to convert the units from MeV g-1 to J kg-1 (Gy). The mass within the cell was determined by multiplying the density of material in the cell by the volume of the cell. The simulation was done for 1,000,000 particles and relative error was decreased by using variance reduction techniques to less than 0.001 for each organ.[10]
Since MCNP gives the results per disintegration, so to calculate the absorbed dose of administrated activity, it is necessary to multiply the result by accumulated activity in source organ (thyroid). The accumulated activity was estimated in previous work.[4] Briefly, a source of 10 mci of 131I was put on a head and neck phantom. Several TLDs were placed on the surface of thyroid on phantom for 24 h and then compared with the dose of phantom and patients followed by calculation of the activity in patient's thyroid.[4,8]
After calculating absorbed dose of organs using Monte Carlo simulation, comparing the results of three methods of MCNP simulation, MIRD calculation and direct dosimetry (TLDs) was done two by two, by performing the t-paired test via SPSS software. If the P value is more than 0.05, there is no significant difference between two compared methods. Here, the absorbed doses obtained through MIRD method and TLD direct dosimetry in previous work are used.[4]
RESULTS
Table 1 shows the amount of 131I in thyroid obtained by comparison of absorbed dose in patients and phantom which was estimated in previous study.[4] Table 2 shows the results of MCNP simulation. Table 3 also shows the result of calculating methods of MCNP simulation compared to MIRD method and experimental methods (TLD). The results of the t-paired test to compare the results of three methods are shown in Table 4.
Table 1.
Activity obtained, using the phantom
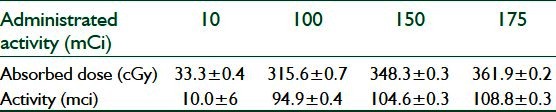
Table 2.
Results of MCNP simulations (cGy)
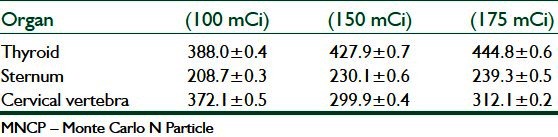
Table 3.
Results of MCNP simulation method
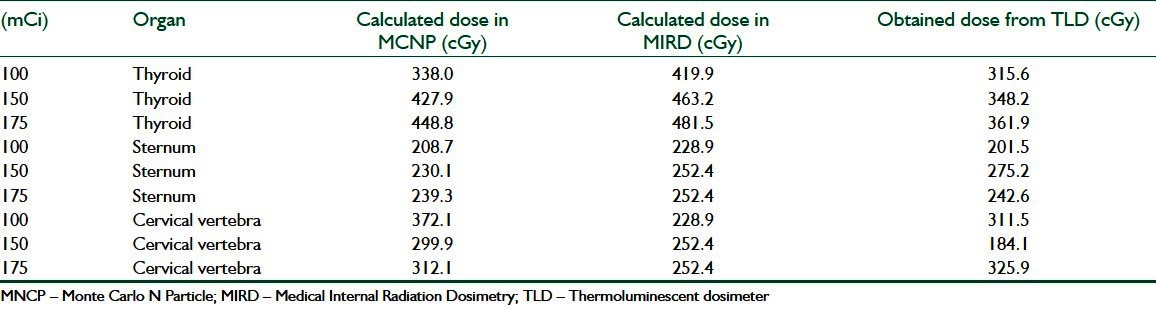
Table 4.
Results of t-paired test
DISCUSSION
Monte Carlo techniques have been used extensively in medical physics applications, and offer the most powerful tool for modeling radiation transport in different media. The availability of general purpose of MCNP codes combined with the ever-increasing computer speed and decreasing costs have lead to a boom in MCNP studies in recent years. MCNP techniques will dominate the field of radiation dosimetry and benchmark dose calculations in radiotherapy for many years to come.[10,11] In this study, the absorbed doses of sternum and cervical vertebra are lower than the absorbed dose of thyroid in all three mentioned (Monte Carlo simulation, MIRD, and the direct dosimtery using TLDs) methods.
Since the beta rays emitted from 131I travel a maximum distance of 3 mm in tissue, they do not contribute to measured dose by TLDs. As can be seen from Table 3, the absorbed doses measured by TLD dosimetry are slightly lower than other calculation methods such as MCNP and MIRD.
Calculation methods such as MIRD and Monte Carlo simulation also have some limitations. For example, The MIRD calculation and MCNP is based on reference human data and this could be a source of error in estimation of organ doses of every patient. It is possible to decrease this error by scanning the thyroid of each patient and estimate the mass of its thyroid.[9,12] Furthermore, to calculate dose in a target organ the source activity must be known. To calculate source activity a head and neck phantom was used and the source activity was estimated by using TLD dosimetry[4] which means that the source of error in TLD dosimetry affect the result of MIRD and MCNP. Results of three methods [Table 3] showed that thyroid of patients absorbed the amount of radiation and are in good agreement among three methods.
Table 4 shows that the P-values of any two compared method with each other is more than 0.05 which means that there is no significant differences among these three methods of dosimetry. In addition, as it is shown in Table 4, the P value of MCNP-MIRD is higher than the rest which means the results of these two methods are more in agreement as expected.
CONCLUSIONS
The results of this work showed that Monte Carlo simulation, MIRD calculations, and experimental dosimetry (TLDS) are in good agreement with each other and it is possible to use any of them by a clinician. Also, Monte Carlo simulation is a suitable and cost-effective method for dosimetry in radioiodine therapy.
BIOGRAPHIES

Daryoush Shahbazi-Gahrouei obtained his BSc from the Department of Science of Isfahan University in Iran in 1987, and his MSc from the School of Medical Sciences of Tarbiat Modarres University, Tehran, Iran, in 1991. He obtained his PhD in Medical Physics at the University of Western Sydney and St. George Cancer Care Centre, Sydney, Australia, in 2000. His PhD thesis entitled: “Development and application of new cancer-specific contrast agents for tumour detection by MR Imaging”. He holds the position of Professor of Medical Physics at the Department of Medical Physics and Medical Engineering in the School of Medicine of Isfahan University of Medical Sciences, Iran. He has authored many papers in the area of Medical Physics, including of novel nanoparticles as MR imaging contrast agents for cancer detection, natural radiation, nuclear medicine, medical imaging, effects of electromagnetic fields, radiation protection, advanced radiation therapy and radiation dosimetry.
E-mail: shahbazi@med.mui.ac.ir

Saba Ayat received her BSc in physics from Isfahan University of Technology, Iran in 2004 and her MSc in Medical Radiation Engineering from Science and Research Branch of Islamic Azad University of Tehran in 2011. Her current research interest includes Monte Carlo simulation of imaging systems, image reconstruction and shielding design.
E-mail: saba.ayat@gmail.com
Footnotes
Source of Support: Nil
Conflict of Interest: None declared
REFERENCES
- 1.Luster M, Clarke SE, Dietlein M, Lassmann M, Lind P, Oyen WJ, et al. Guidelines for radioiodine therapy of differentiated thyroid cancer. Eur J Nucl Med Mol Imaging, 2008;10:259–83. doi: 10.1007/s00259-008-0883-1. [DOI] [PubMed] [Google Scholar]
- 2.Hegedüs L, Bonnema SJ, Bennedbæk FN. Management of simple nodular goiter: Current status and future perspectives. Endocr Rev. 2003;24:102–32. doi: 10.1210/er.2002-0016. [DOI] [PubMed] [Google Scholar]
- 3.Hanscheid H, Lassmann M, Luster M, Thomas SR, Pacini F, Ceccarelli C, et al. Iodine biokinetics and dosimetry in radioiodine therapy of thyroid cancer: Procedures and results of a prospective international controlled study of ablation after rhTSH or hormone withdrawal. J Nucl Med. 2006;47:648–54. [PubMed] [Google Scholar]
- 4.Shahbazi-Gahrouei D, Nikzad S. Determination of organ doses in radioiodine therapy using medical internal radiation dosimetry (MIRD) method. Iran J Radiat Res. 2010;8:249–52. [Google Scholar]
- 5.Yuni KD, Scott JW, Michael L, Kenneth FK, Koral KZ, Mark SK. Accurate dosimetry in 131I radionuclide therapy using patient-specific, 3-dimensional methods for SPECT reconstruction and absorbed dose calculation. J Nucl Med. 2005;46:840–9. [PMC free article] [PubMed] [Google Scholar]
- 6.Yoriyaz H, Stabin MG, Dos Santos A. Monte Carlo MCNP-4B-based absorbed dose distribution estimates for patient-specific dosimetry. J Nucl Med. 2001;42:662–9. [PubMed] [Google Scholar]
- 7.Molavi A, Binesh A. Calculation of iodine-131 absorbed dose in thyroid by use of MCNP for two field of spiral and cylindrical. J Rafsanjan Univ Med Sci. 2006;5:7–10. [Google Scholar]
- 8.Shahbazi-Gahrouei D, Nikzad S, Shokrani P, Shahi Z, Monadi S. Determination of absorbed dose of organs (thyroid, sternum, cervical vertebra) in thyroid cancer patients following radioiodine therapy. Iran J Nucl Med. 2009;17:27–33. [Google Scholar]
- 9.Alberico B, de Carvalho AB, Jr, Hunt J, Silva AX, Garcia F. Use of a voxel phantom as a source and a second voxel phantom as a target to calculate effective doses in individuals exposed to patients treated with 131I. J Nucl Med Technol. 2009;37:53–6. doi: 10.2967/jnmt.108.058172. [DOI] [PubMed] [Google Scholar]
- 10.Capote R. IAEA workshop on Monte Carlo radiation transport and associated data needs for medical applications. 2011 [Google Scholar]
- 11.Fasso A, Ferrari A, Ranft J, Sala PR. FLUKA: Status and prospective for hadronic applications. In: Kling A, Barao F, Nakagawa M, Tavora L, Vaz P, editors. Advanced Monte Carlo for radiation physics, particle transport simulation and applications. Proceedings of the Monte Carlo 2000 Conference. Berlin, Germany: Springer–Verlag; 2000. pp. 955–60. [Google Scholar]
- 12.Lee C, Staton RJ, Hintenlang DE, Arreola MM, Williams JL, Bolch WE. Organ and effective doses in pediatric patients undergoing helical multislice computed tomography examination. Med Phys. 2007;34:1858–73. doi: 10.1118/1.2723885. [DOI] [PubMed] [Google Scholar]


