Resource and competitive dynamics shape the benefits of public goods cooperation in a plant pathogen

Abstract

Cooperative benefits depend on a variety of ecological factors. Many cooperative bacteria increase the population size of their groups by making a public good available. Increased local population size can alleviate the constraints of kin competition on the evolution of cooperation by enhancing the between-group fitness of cooperators. The cooperative pathogenesis of Agrobacterium tumefaciens causes infected plants to exude opines—resources that provide a nearly exclusive source of nutrient for the pathogen. We experimentally demonstrate that opines provide cooperative A. tumefaciens cells a within-group fitness advantage over saprophytic agrobacteria. Our results are congruent with a resource-consumer competition model, which predicts that cooperative, virulent agrobacteria are at a competitive disadvantage when opines are unavailable, but have an advantage when opines are available at sufficient levels. This model also predicts that freeloading agrobacteria that catabolize opines but cannot infect plants competitively displace the cooperative pathogen from all environments. However, we show that these cooperative public goods also promote increased local population size. A model built from the Price Equation shows that this effect on group size can contribute to the persistence of cooperative pathogenesis despite inherent kin competition for the benefits of pathogenesis.

Introduction

Cooperative individuals aid the reproduction of other individuals at the expense of their own reproduction. The evolutionary success of cooperative traits requires that such individuals indirectly benefit from the reproduction of the individuals that they aid and that these benefits outweigh the direct fitness costs of their cooperation, a result described by Hamilton’s rule (1964). Although the costs, benefits, and relatedness central to Hamilton’s rule are fundamentally influenced by a variety of ecological factors (van Baalen and Rand 1998; Aviles et al. 2002), these factors are often neglected in social evolution theory (reviewed by Platt and Bever 2009).

Kin competition is often regarded as a powerful antagonist of the indirect benefits of cooperation. This antagonism is particularly potent when density dependence is local such that kin are strictly competing for limited resources (Wade 1985; Taylor 1992a; Platt and Bever 2009). However when density dependence is determined at a scale broader than the scale over which individuals cooperate, local competition is a much less powerful antagonist of cooperative benefits as this facilitates cooperative groups being more productive than less cooperative groups (Wade 1985; Griffin et al. 2004). Even with local density dependence, the antagonizing effect of kin competition is diminished when the cooperative trait increases the local carrying capacity of cooperative groups, as this too eases the constraints on the degree to which cooperative groups contribute to future generations (Mitteldorf and Wilson 2000; Platt and Bever 2009).

Bacterial cooperation often involves actions that make a specific good available to neighboring individuals (West et al. 2007). Such behaviors are widespread and vary broadly, from the extracellular production of surfactants that aid motility, to the release of enzymes that digest nutrients and prey. Importantly, such public goods based cooperation can increase local carrying capacity thereby alleviating the constraint that kin competition imposes on the evolution of such cooperative acts (Platt and Bever 2009).

The cooperative pathogenesis of Agrobacterium tumefaciens, which leads to the plant disease known as crown gall, involves a particularly elaborate mechanism by which a public good is produced (Escobar and Dandekar 2003). Genes found on the Tumor-inducing (Ti) plasmid harbored by A. tumefaciens confer the ability to genetically transform dicotyledonous plant cells. A segment of the Ti plasmid (known as the T-DNA) is translocated into the plant’s cell via a type IV secretion (T4S) system and then directed to the plant’s nucleus by T4S system effector proteins, which carry nuclear localization signals. The T-DNA then randomly integrates into the plant’s genome and, through expression of T-DNA encoded functions, causes the plant to misregulate growth hormones. This leads to the growth of a plant tumor. Additional genes on the T-DNA result in production and release of opines into the rhizosphere. Opines are unique tumor metabolites that are rich in carbon, nitrogen, and in some cases phosphorus. These opines are a public good available to any cell harboring the genes necessary for the catabolism of opines. The capacity to degrade opines is relatively rare but usually encoded on the Ti plasmid. Thus the benefits of the cooperative pathogenesis of A. tumefaciens are directed toward genetically similar individuals without direct recognition. In this way, the Ti plasmid behaves like a multigene greenbeard with the benefits of its costly pathogenesis (Platt et al. 2011) intrinsically available primarily to individuals that also carry the genes for pathogenesis and opine catabolism due to their linkage on the Ti plasmid (Platt and Bever 2009; Gardner and West 2010).

The Ti plasmid is naturally polymorphic, and it is common for agrobacteria in the environment to lack the plasmid (Farrand 1998). Harboring the Ti plasmid involves a fitness tradeoff between being able to access the benefits of opine catabolism and a fitness burden under nutrient limiting conditions (Platt et al. 2011). In this study, we empirically demonstrate that opine availability increases the carrying capacity and fitness of A. tumefaciens cells bearing the Ti plasmid in competition with cells that lack the plasmid. This result confirms qualitative predictions of a consumer-resource competition model that the competitive dominance of cells harboring the Ti plasmid depends on a sufficiently high opine supply and is correlated with increased local carrying capacity. We also develop a novel derivation of Hamilton’s rule that incorporates effects on local carrying capacity. We find that cooperative traits that promote local carrying capacity can offset the antagonistic effects of kin competition and enjoy elevated between group fitness. These results suggest that the cooperative pathogenesis of A. tumefaciens is unlikely to be constrained by kin competition because, despite local density dependence the increased population size of cells in the presence of an infected, opine-producing plant allows cooperative groups to be more productive than less cooperative groups.

Path model of cooperative traits enhancing local population size

The success of any cooperative trait requires that the cooperative action increases the productivity of a group of individuals. Several ecological factors influence the degree to which this is or is not possible, including the scale of density dependence, population elasticity, and several demographic factors (Platt and Bever 2009). Population elasticity describes the potential of the population to expand in size in response to alleviation of some factor limiting its size. In elastic populations, cooperative individuals that affect local ecology to increase resource availability enjoy enhanced between-group fitness stemming from the resultant increase in group productivity. Following conventions for path models (Rice 2004), the fitness of such traits can be described by the following least squares regression:

$$W={\alpha }_1+{cg}_i+{bg}_g+dN+{\epsilon }_1$$

where, N is the local population size, g_i is the cooperative individual’s genic value, g_g is the average genic value of the individual’s group, while c, b, and d are partial regression coefficients describing the linear relationship between fitness (W) and g_i, g_g, and N, respectively (Queller 1992; Platt and Bever 2009). Table 1 describes all parameters used in the path model. For each least squares regression the intercept and residuals of the model are given by α and ε terms, respectively. The local population size (N) depends on the supply of resources that the group experiences (S) as well as the present genotypes (g_i and g_g). Consequently local group size (N) can also be described by the following least squares regression:

$$N={\alpha }_2+eS+p_i{g}_i+p_g{g}_g+{\epsilon }_2$$

wherein e, p_i, and p_g describe partial regression coefficients describing the linear relationship between local group size (N) and resource supply (S), focal genotype (g_i), and the average genotype of the group (g_g), respectively. When cooperative traits influence the resource supply, for example as with agrobacterial pathogenesis and certain types of iron acquisition, the resource supply experienced by the group (S) also depends on the present genotypes (g_i and g_g), giving the following least squares regression:

$$S={\alpha }_3+a_i{g}_i+a_g{g}_g+{\epsilon }_3$$

wherein a_i and a_g describe the relationship between the group’s resource supply (S) and the focal (g_i) and group (g_g) genotypes, respectively.

Table 1.

Path model notation summary. For terms that can be described as a partial regression coefficient, the corresponding partial regression coefficient is described in the form β_XY•ZV, which describes the linear relationship between variables X and Y, holding variables Z and V constant. See Queller (1992, 2011) for more details on this approach.

Symbol	Description	Regression coefficient
gi	Genic value of the focal individual
gg	Average genic value of the group
S	Supply of resources experienced by the group
N	Local population size
W	Fitness of the focal individual
c	Fitness changes as a function of focal individual’s genes	βWgi•ggN
b	Fitness changes as a function of group’s genes	βWgg•giN
r	Relatedness	βgggi
d	Fitness changes resulting from elasticity in population size	βWN•gigg
pi	Local population size changes resulting from the effects of the focal individual’s genes	βNgi•ggS
pg	Local population size changes resulting from the effects of the group’s genes	βNgg•giS
ai	Changes in resource supply resulting from the effects of focal individual’s genes	βSgi•gg
ag	Changes in resource supply resulting from the effects of the group’s genes	βSgg•gi
e	Elasticity of local population size in response to changes in resource supply	βNS•gigg

Using the approach described by Queller (1992, 2011), we apply the Price Equation to derive a version of Hamilton’s rule for cooperative traits that increase local population size by either enhancing the local supply of resources or alleviating some other factor regulating population size:

$$c+br+{dp}_i+{dp}_{g}r+{\mathit{dea}}_i+{\mathit{dea}}_{g}r>0$$ Equation 1

Each term in this inequality represents a path (Figure 1) through which the trait can influence fitness (Queller 1992; Rice 2004; Queller 2011).

Figure 1.

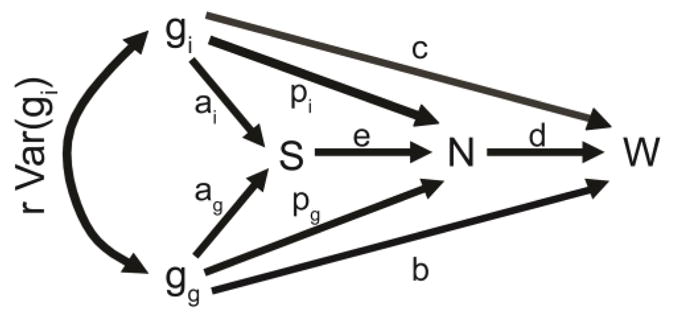
Path diagram of factors influencing the fitness of cooperative trait that affects the supply of resources. Terms over straight arrows describe the partial regression coefficients of the variables connected by the corresponding arrow, while the term over the curved arrow represents the covariance between the source variables. Changes in the supply of resources can affect population size in elastic populations, which in turn can influence fitness.

The first two terms parallel the cost (c) and benefit terms (br) found in Hamilton’s rule as re-derived by Queller (1992). Consequently when there are no paths to fitness (W) through local group size (N), this model simplifies to give the most basic form of Hamilton’s rule (c + br > 0 (Figure 1). The four additional terms in this equation describe the four paths to fitness (W) through the local group size (N) (Figure 1). The third and fourth terms describe the direct and indirect effects of focal and group genotype, respectively, on genetic change though group size. The direct effect depends on how fitness changes as a function of group size (d) and how group size changes as a function of the focal genotype (p_i). The indirect effect also depends on d but connotes how group size changes as a function of the average group genotype (g_g). Consequently this also must be weighted by relatedness (r), reflecting the degree to which g_i and g_g covary. The final two terms of this model describe the direct and indirect effects of cooperation mediated increases in resource supply, respectively. The direct effect of increased resource supply depends on the relationship between fitness and group size (d), the degree to which the resource supply influences local population size (e), and the degree to which the focal genotype influences resource supply (a_i). Similarly the indirect effect also depends on d and e, however now it is the degree to which the group’s genotype influences resource supply (a_g) that determines the magnitude of the effect. Thus the effect of this path on genetic change must be weighted by relatedness (r).

Cooperative individuals garner a benefit resulting from group elasticity whenever (dp_i + dp_gr + dea_i + dea_gr > 0). The first two terms of this inequality can reflect population size elasticity mediated by traits not associated with resources. Many behaviors or phenotypes are likely to promote increased group size without increasing the availability of resources. For example, alarm calling by meerkat sentries may alleviate predation mediated population regulation (Clutton-Brock et al. 1999). This may allow the group to expand to larger sizes, provided that population size is elastic. The second two terms of this inequality describe the influence of cooperation that enhances resource supply. In order for the effect of increased resource supply to be positive the population must be sufficiently elastic, such that the local population size is able to respond to the increased resource supply brought on by the cooperative action. A cooperative action that enhances resource availability will only provide benefit from this if the population’s growth is limited by resource availability. Further, the additional individuals resulting from growth on resources made available by cooperation must be able to stay in the population, either by dispersing to new sites or as additional residents in the new population.

Resource-consumer model of competition between virulent and avirulent agrobacteria

Our path model of a cooperative trait that enhances resource supply identifies novel pathways in which the benefits of cooperation can exceed the costs of cooperation and overcome the antagonistic effects of kin competition. However, like preceding models of kin competition (e.g. Taylor 1992a; Mitteldorf and Wilson 2000; Gardner and West 2006; Lehmann et al. 2008; Platt and Bever 2009), this model builds on simplifications of the ecological processes underlying competition, including assumptions of linearity of all relationships. We now present an explicit, rigorous model of the resource and population dynamics underlying the kin competition of an experimentally tractable system which exhibits this type of cooperative behavior. We construct this model around the biology of Agrobacterium tumefaciens and, building on the classic models of microbial dynamics of Monod (1949) and Tilman (1980; Tilman et al. 1982).

Neighboring A. tumefaciens lineages compete for a variety of resources. Cells that harbor the Ti plasmid (pTi⁺) are virulent, while pTi⁻ cells are avirulent. pTi⁺ cells also have Ti plasmid-encoded opine utilization genes that, when in the vicinity of a crown gall, provide access to opine resources that avirulent pTi⁻ cells are unable to metabolize. Opines can alleviate nitrogen, carbon, and in some cases phosphorous limitation. In this paper, we focus on octopine-type Ti plasmids which manipulate plants to produce octopine and other carbon and nitrogen based opines. To simplify discussion of the model we assume that nitrogen is limiting. Our model first describes the population dynamics of pTi⁺ and pTi⁻ cells competing for two potentially limiting but substitutable resources: nitrogen-containing opines and other nitrogen sources. When only pTi⁺ and pTi⁻ cells are present, opines are a private good, available only to the pTi⁺ cells that have the genes needed for opine catabolism. Our model explicitly tracks resource dynamics and assumes that all competitive interactions are mediated by limiting resources. The population growth rate of pTi⁺ cells is determined by the relative balance of the strain’s birth rate, which depends on the harvest and utilization of both opines (O) and non-opine nitrogen sources (A), and its death rate, which occurs at a constant per capita rate (m_pTi⁺; see Appendix A for more details on the model):

$$\frac{{dN}_{{\mathit{pTi}}^{+}}}{dt}=N_{{\mathit{pTi}}^{+}}[B_{{\mathit{pTi}}^{+}}(A,O)-m_{{\mathit{pTi}}^{+}}]$$

$$\frac{{dN}_{{\mathit{pTi}}^{-}}}{dt}=N_{{\mathit{pTi}}^{-}}[B_{{\mathit{pTi}}^{-}}(A)-m_{{\mathit{pTi}}^{-}}]$$

The available opine (O) and non-opine nitrogen source (N) levels depend on the balance of each nutrient’s supply rate (S_O and S_A, respectively) and the rate at which each is consumed. Non-opine nitrogen sources can be consumed by both pTi⁺ can pTi⁻ agrobacteria, with each strain’s consumption rate depending on the concentration of nutrients available for it to consume (Holling 1959; Vincent et al. 1996):

$$\frac{dO}{dt}=S_O-C_o(O,A)N_{{\mathit{pTi}}^{+}}$$

$$\frac{dA}{dt}=S_A-C_{A,{\mathit{pTi}}^{+}}(A,O)N_{{\mathit{pTi}}^{+}}-C_{A,{\mathit{pTi}}^{-}}(A)N_{{\mathit{pTi}}^{-}}$$

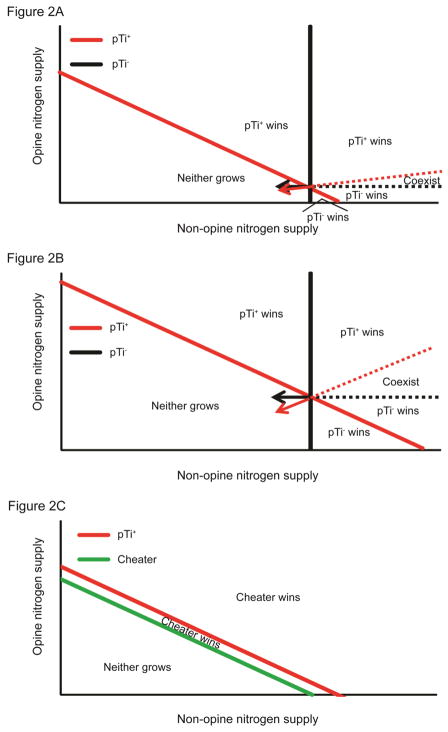
Analysis of this model reveals that when provided sufficient resources the avirulent pTi⁻ strain is competitively superior under low opine supply rates, but as opine supply increases, the competitive ability of the virulent pTi⁺ strain increases and becomes dominant at high opine supply rates. Equilibrial predictions of this model can be graphically represented on a 2-D plot of the resource supply rates (Figure 2). Bearing the Ti plasmid under resource-limiting conditions imposes significant fitness costs on pTi⁺ cells (Platt et al. 2011). Because of this pTi⁺ cells are likely to require a greater minimum supply of non-opine nitrogen sources than pTi⁻ cells. Consequently, pTi⁺ cells outcompete pTi⁻ cells provided that the octopine supply is sufficiently high but are outcompeted when octopine supply is too low (Figure 2). Not surprisingly, the model also predicts that the equilibrial population size of pTi⁺ cells increases with increasing octopine supply, while the equilibrial pTi⁻ population size does not depend on the octopine supply (Figure 3).

Figure 2.

Model predictions of resource competition for opines and a substitutable nitrogen resource between virulent pTi⁺ and either avirulent pTi⁻ (A and B) or cheater (C) strains. In (A) pTi⁺ experiences a low cost associated with bearing the plasmid; whereas in (B) this cost is higher. The solid lines represent the lowest amounts of resource supporting persistence of the indicated strains. Because, the pTi⁻ strain does not bear the cost of the plasmid (Appendix B, Platt et al. 2011) and therefore is able to persist at lower nitrogen concentrations, the pTi⁻ line crosses the x-axis at a lower value than that of the pTi⁺. Only pTi⁺ cells can persist on opine alone and so only its isocline intersects the opine axis. We assume that pTi⁺ cells utilize both opines and other nitrogen sources less efficiently than pTi⁻ cells utilize non-opine nitrogen sources ( $u_{{\mathit{pTi}}^{-}}^A>u_{{\mathit{pTi}}^{+}}^A=u_{{\mathit{pTi}}^{+}}^O$), reflecting the costs associated with harboring the Ti plasmid. (C) Agrobacterial cells bearing a cheater plasmid, which do not pay costs associated with virulence but can catabolize opines, are predicted to locally outcompete virulent agrobacteria regardless of the environment’s supply of opines and non-opine nitrogen resources. We assume that pTi⁺ cells utilize both resource types less efficiently than cheater cells ( $u_{\mathit{cheat}}^A>u_{{\mathit{pTi}}^{+}}^A$ and $u_{\mathit{cheat}}^O>u_{{\mathit{pTi}}^{+}}^O$), reflecting the costs associated with being able to infect host plants. The vectors represent the resource consumption of the indicated strains. Each point in the resource space represents environmentally determined supply rates of opine and other nitrogen sources. For simplicity, we assume that all strain types encounter with the same probability ( $e_{{\mathit{pTi}}^{+}}^A=e_{{\mathit{pTi}}^{-}}^A=e_{{\mathit{pTi}}^{+}}^O$) and have the same handling time for ( $h_{{\mathit{pTi}}^{+}}^A=h_{{\mathit{pTi}}^{-}}^A=h_{{\mathit{pTi}}^{+}}^O$) all resources that they catabolize. Similarly all strains experience the same mortality rate (m_pTi⁺ = m_pTi⁻), while both resources are assumed to be supplied at the same concentration (A₀ = O₀) and flow out of the competitive arena at the same rate (D_A = D_O).

Figure 3.

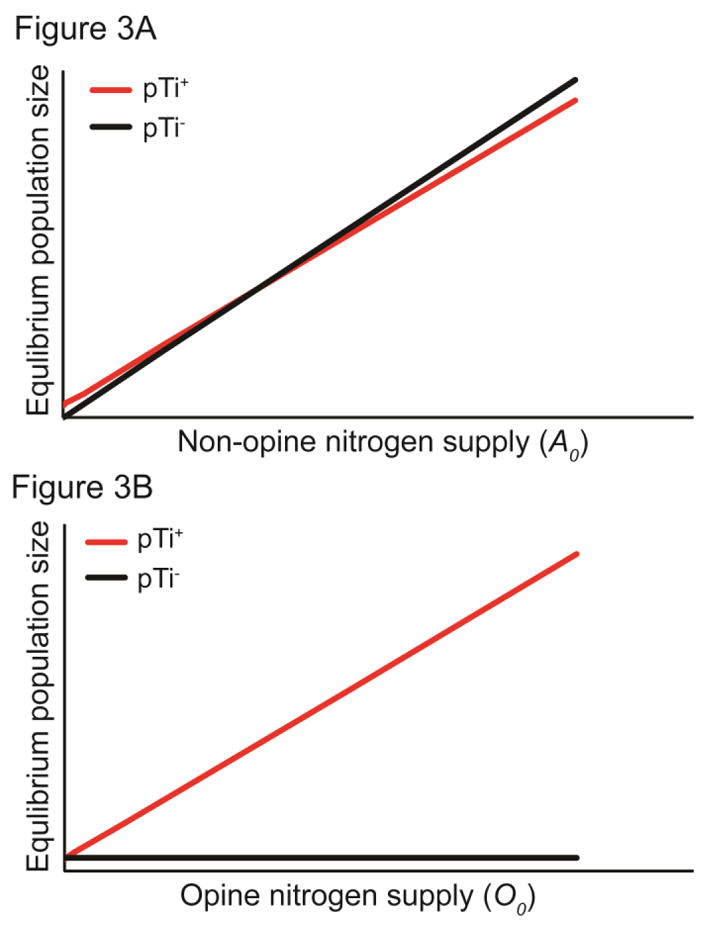
Model predictions for relationship between non-opine nitrogen supply (A) or opine nitrogen supply (B) and equilibrium population size for pTi⁺ and pTi⁻ cells. Parameter values are the same as in Figure 2A.

In addition to pTi⁺ and pTi⁻ agrobacteria, avirulent freeloaders that retain the ability to catabolize opines are also frequently observed among natural agrobacteria (Merlo and Nester 1977; Dessaux et al. 1986; Nautiyal and Dion 1990; Dessaux et al. 1998). These strains harbor opine catabolic plasmids, which confer the ability to freeload on the benefits of pathogenesis initiated by virulent agrobacteria by catabolizing opines but do not confer virulence functions. In contrast to competition between pTi⁺ and pTi⁻ cells, when pTi⁺ cells compete with these opine catabolic freeloaders opines are a public good—available for catabolism by both competitors (Frank 2010). Despite the competitive advantage that pTi⁺ cells have over pTi⁻ cells in the disease environment when opine supply is high, they are predicted to be competitively displaced from all environments by freeloading genotypes (Figure 2C). These genotypes do not pay the high costs of infecting plants, but can benefit from the catabolism of opines produced by plants infected by other agrobacteria strains. Freeloaders therefore require less resources for growth than do virulent, pTi⁺ cells and thus can always drive resource levels below the minimal needs of pTi⁺ cells (Figure 2C). Thus, pTi⁺ cells can only persist provided that they have at least transient exclusive access to the disease environments they initiate. Subsequent to this initial exclusive access, cheater genotypes may invade or arise via mutation. In order for the virulent type to have the opportunity of benefiting from the disease state it establishes, relatedness must be sufficiently high such that the neighboring genotypes tend also to be virulent, possibly simply due to the clonal growth of that genotype. Our path model reinforces this point, as the success of any genotype that enhances the availability of a public group resource requires sufficiently high genetic structure such that it is able to benefit from increased between group fitness, offsetting the within group fitness disadvantages of producing the public good.

Experimental methods

Our resource-consumer model predicts that under resource limiting conditions cells harboring the Ti plasmid have a competitive advantage over those lacking the plasmid in environments with high opine supply, but are at a disadvantage when opines are absent (Figure 2). The model also predicts that the population size of pTi⁺ cells, but not pTi⁻ cells, increases with increased opine availability (Figure 3B). We tested these predictions by first characterizing the growth of pTi⁺ populations on octopine and then competing pTi⁺ and pTi⁻ cells under environmental conditions supplied with varying amounts of octopine.

Strains and media

All bacterial strains used in this study are described in Table 3. For competition experiments, nalidixic acid resistant (Nal^R) derivatives were isolated as spontaneous mutants. Unless specified otherwise, bacteria were maintained on AT minimal medium supplemented with 0.5% glucose (wt/vol) and 15 mM (NH₄)₂SO₄ (ATGN; Tempe et al. 1977) and incubated at 28°C in a rotary aerator or on 1.5% agar plates. In contrast to the original formulation, FeSO₄•H₂O was omitted from our AT minimal media; ambient iron levels were sufficient to saturate the iron requirement (Merritt et al. 2007). We obtained reagents, antibiotics, and media components from Fisher Scientific (Pittsburgh, PA) and Sigma-Aldrich (St. Louis, MO).

Table 3.

Strains used in this study

A. tumefaciens strains	Relevant feature(s)	Reference
15955	Octopine type strain; contains pTi15955	Baek et al. (2003)
TGP101	Derivative of 15955 cured of pTi15955	Platt et al. (2011)
TGP110	Spontaneous nalidixic acid resistant mutant of 15955	Platt et al. (2011)
TGP114	Spontaneous nalidixic acid resistant mutant of TGP101	Platt et al. (2011)
R10	Octopine type strain; contains pTiR10 and pAtR10	S.K. Farrand
KYC55	Derivative of R10 cured of pTiR10, contains pAtR10 arcB::Tn5-gusA7, KmR	Cho et al. (1997)

Octopine catabolism and limitation

We synthesized and purified D-octopine using the method described by Taylor and Hall (1986). We confirmed that the final preparation was nearly pure D-octopine using C-13 NMR (data not shown). A. tumefaciens 15955 and TGP101 were grown to mid-log phase, cells harvested via centrifugation, and washed 7–8 times with 78.6 mM KH₂PO₄ buffer (pH 7.0) to remove sources of carbon and nitrogen from the inoculum. The washed cells were then diluted to an optical density at 600 nm (OD₆₀₀) of 0.005 in 2 ml of AT minimal media supplemented with either 1) octopine as the sole carbon and nitrogen source (3.25 mM octopine); 2) octopine as the sole source of carbon (3.25 mM octopine and 15 mM (NH₄)₂SO₄); 3) octopine as the sole source of nitrogen (3.25 mM octopine and 0.5% glucose (wt/vol)); 4) no source of carbon (15 mM (NH₄)₂SO₄); or 5) no source of nitrogen (0.5% glucose (wt/vol)).

We also characterized the dose response of A. tumefaciens strain 15955 population yield to increasing amounts of octopine as either a sole carbon or nitrogen source. To do this we prepared an inoculum of 15955 cells free of nitrogen and carbon sources as described above and inoculated the appropriate media to an OD₆₀₀ of 0.005. Octopine was the sole source of carbon in AT minimal media supplemented with 15 mM (NH₄)₂SO₄ and either 0, 1, 3, 6, 12, 18, 24 mM octopine or the sole source of nitrogen in AT minimal media supplemented with 0.5% glucose (wt/vol) and either 0, 0.25, 0.5, 0.75, 1, 3, or 4 mM octopine. We monitored the OD₆₀₀ of these cultures until their turbidity ceased to increase.

Fitness benefits of octopine

In order to assess the competitive fitness benefits associated with pTi⁺ cell catabolism of octopine, we grew pTi⁺ cells with pTi⁻ cells together under a variety of environmental conditions that differed in the amount of D-octopine present. pTi⁺ and pTi⁻ cells were competed in AT minimal medium (Tempe et al. 1977) supplemented with 0.5% glucose (wt/vol), 600 μM (NH₄)₂SO₄, and either 0, 9.4, 37.5, 150, or 600 μM D-octopine. Nitrogen limits the growth of A. tumefaciens strain 15955 when it is grown on 600 μM (NH₄)₂SO₄ (Platt et al. 2011), such that under these conditions octopine supplementation provides pTi⁺ cells with an additional, private source of nitrogen.

For each of the five environmental conditions, we performed six replicates. All competitions were inoculated with approximately 8 × 10⁶ cells, with roughly half being pTi⁺ and half being an otherwise isogenic pTicured derivative (Platt et al. 2011). Half of the competitions for each environmental condition set 15955 (Nal^S, pTi⁺) against TGP114 (Nal^R, pTi⁻), while the other half of the replicates competed TGP110 (Nal^R, pTi⁺) and TGP101 (Nal^S, pTi⁻), thereby controlling for possible effects of the label (Table 3). For each competition the appropriate pTi⁺ strain and pTi⁻ strain was grown to mid-log phase, harvested by centrifugation, and then washed 8 times with 78.6 mM KH₂PO₄ buffer (pH 7.0) to remove sources of nitrogen from the cell inoculum. These cells were then used to inoculate 2 ml of appropriate fresh media as described above. The competition cultures were then incubated at 28°C for 24 hours, after which all mixed cultures were sub-cultured 1:100 into another 2 ml of the same fresh media and incubated as before for 24 more hours. We plated dilution series of each mixed culture at 0 hours, 24 hours, and 48 hours from the start of the experiment onto ATGN and ATGN supplemented with 50 μg/ml nalidixic acid so that we could estimate the density and frequency of both strains present.

The relative fitness of pTi⁺ cells was estimated as the ratio of the number of doublings by pTi⁺ cells to that of pTi⁻ cells over the course of the competition experiment (Lenski 1988).

Analysis

Competition experiments were analyzed using Proc GLM based on SAS® software. The model included both the concentration of octopine and the nalidixic acid marker orientation as factors. Including the later factor allowed us to remove variance associated with the marker and thereby isolate the effect of varying levels of octopine availability by comparing least square means of the different octopine levels. We used a priori t-tests to evaluate whether relative fitness estimates and strain frequency changes differed from one or zero, respectively. The significance of differences between treatment means was evaluated using Tukey’s adjustment.

Results

Octopine catabolism by Ti plasmid bearing cells

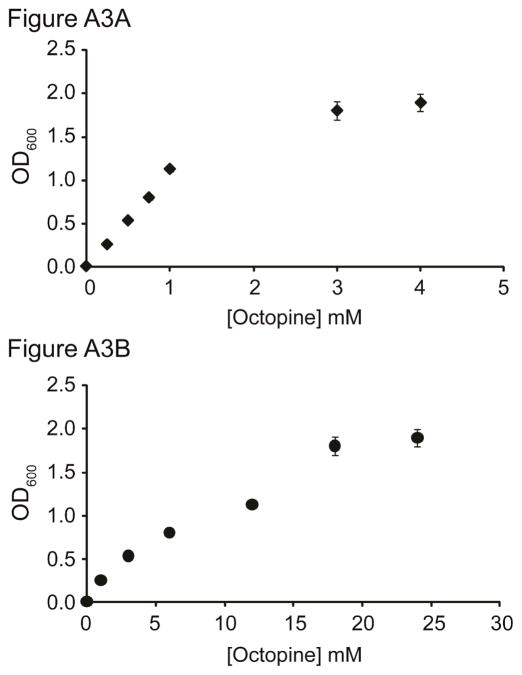
A. tumefaciens strain 15955 cells (pTi⁺) were able to grow on our synthesized D-octopine as the sole source of carbon, nitrogen, or carbon and nitrogen (Figure 4). Strain TGP101—a nearly isogenic pTi cured derivative of 15955—failed to grow under any of these conditions (Figure 4). We determined the range of octopine concentrations over which the growth yield of pTi⁺ populations increases in response to higher levels of octopine supplied as the sole nitrogen and carbon source (Figure A3).

Figure 4.

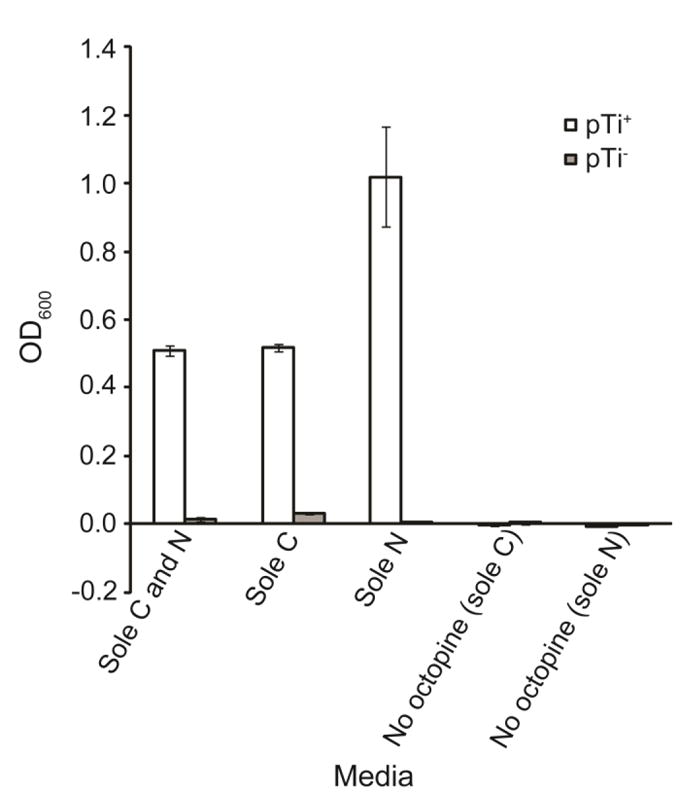
Strain 15955 (pTi⁺) can utilize octopine as the sole source of carbon, nitrogen, or both carbon and nitrogen supporting population growth. However strain TGP101 (pTi⁻) cannot grow on octopine. As a control showing that this population growth depends on octopine supplementation, neither strain grew when octopine was not provided as either the sole carbon or sole nitrogen source. Values represent mean ± standard error of three replicates.

Figure A3.

A. tumefaciens 15955 population size increases with octopine availability. Prior to octopine supplementation nitrogen (A) or carbon (B) limits population growth.

Relative fitness of Ti plasmid bearing cells

Octopine availability significantly influenced the relative fitness of Ti plasmid-bearing 15955 cells (F_{4, 29} =127.6, p < 0.0001; Figure 5). In contrast, pTi⁺ 15955 cells were at a significant competitive disadvantage to pTi⁻ TGP101 cells when no octopine was present and nitrogen was limiting (p < 0.01). The fitness of cells with and cells without the Ti plasmid (15955 and TGP101, respectively) did not significantly differ in the 9.4 μM (p = 0.06) and 37.5 μM octopine treatments (p = 0.31). However pTi⁺ cells had a significant competitive advantage over pTi⁻ cells when 150 μM (p < 0.0001) and 600 μM (p < 0.0001) of octopine was present. Cells bearing the Ti plasmid had highest fitness in populations that had higher final population size (F_{1, 29} =378.7, p < 0.0001; Figure 6A), which was dependent on the availability of octopine (Figures 6B and 6C). We observed similar results from competitions and clonal populations of A. tumefaciens R10 (pTi⁺) and KYC55 (pTi⁻) under carbon-limiting conditions (Appendix B, Figures A1 and A2). A. tumefaciens R10 is an octopine-type genotype genetically distinct from 15955, while KYC55 is a nearly isogenic pTi cured derivative of R10 (Table 3). Our relative fitness and population growth results for R10/KYC55 and 15955/TGP101 were consistent despite using different genotypes and a different limiting resource (i.e. carbon instead of nitrogen).

Figure 5.

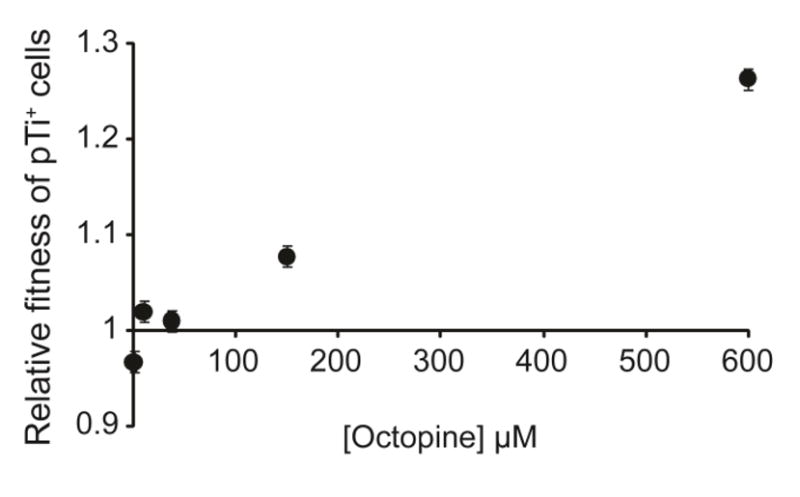
The fitness of cells harboring the Ti plasmid increased as the level of available octopine increased (t = 18.35, p < 0.0001). Utilizable nitrogen levels limited growth of the population and was available in the form of (NH₄)₂SO₄ and to varying levels octopine. Both pTi⁻ and pTi⁺ cells catabolized the (NH₄)₂SO₄, however only pTi⁺ cells could catabolize octopine. Values are LS mean ± standard error of six replicates.

Figure 6.

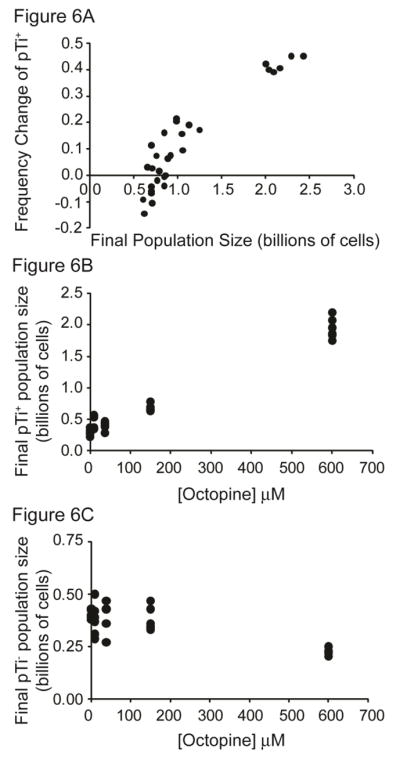
Fitness benefits of opine catabolism. Cells bearing the Ti plasmid became increasingly more common in populations that achieved the highest population density (A) (t = 19.46, p < 0.0001). The competitive advantage of cells bearing the Ti plasmid likely stems from their ability to grow to higher population numbers in response to increasing octopine availability (B) (t = 22.37, p < 0.0001). In contrast, cells lacking the Ti plasmid achieved their lowest population numbers when octopine availability was highest (C) (t = −4.53, p < 0.001). This result likely reflects the high numbers of pTi⁺ cells present driving the ammonium levels down such that the pTi⁻ cells effectively experienced lower nitrogen availability. Note that because pTi⁺ cells attained much larger population sizes than did pTi⁻ cells, the scale of the y-axes differs between panels B and C.

Figure A1.

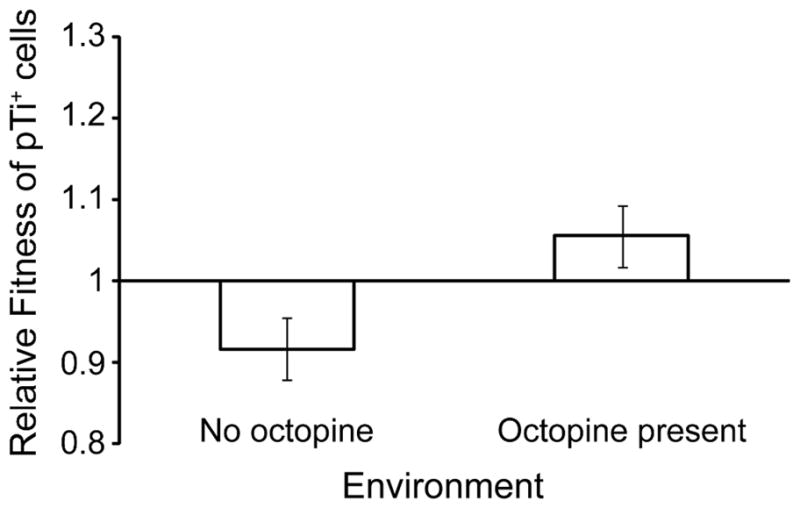
A. tumefaciens R10 cells harboring pTiR10 had a competitive advantage over pTi cured derivatives when octopine was present, but were at a competitive disadvantage when octopine was absent. Values represent mean ± standard error of eight replicates.

Figure A2.

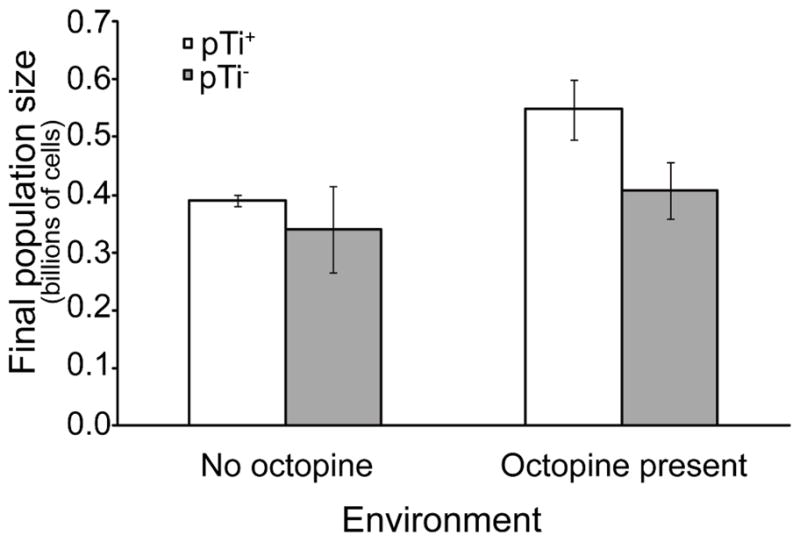
The carrying capacity of the pTi⁺ (R10), but not the pTi⁻ (KYC55), population increased with opine availability. Values represent mean ± standard error of eight replicates.

Octopine and population size

The level of octopine available significantly influenced the total population sizes (F_{4, 29} = 187.5, p < 0.0001), of pTi⁺ (F_{4, 29} = 289.9, p < 0.0001) and pTi⁻ lineages (F_{4, 29} =12.4, p < 0.0001) (Figure 6). The total and pTi⁺ population size was greatest in the 600 μM octopine treatment (Tukey post-hoc, p < 0.05), intermediate in the 150 μM octopine treatment (Tukey post-hoc, p < 0.05), and statistically equivalent in the 37.5 μM, 9.4 μM, and 0 μM octopine treatments (Tukey post-hoc, p < 0.05; Figure 6B). In contrast pTi⁻ cells achieved statistically equivalent population sizes in the 0 μM, 9.4 μM, 37.5 μM, and 150 μM octopine treatments, but attained a significantly lower population size in the 600 μM octopine treatment (Figure 6C). The carrying capacity of R10 (pTi⁺) populations also increased when octopine was available, while the carrying capacity of populations did not significantly change for its isogenic derivative KYC55 (pTi⁻) (Appendix B, Figure A2).

Discussion

Kin competition is frequently regarded as a powerful antagonist of the evolution of cooperation (reviewed by West et al. 2002). This conclusion stems from models that largely simplify the competitive process (Taylor 1992a, b; Wilson et al. 1992). However the degree to which kin competition limits the spread of cooperative traits depends on a variety of ecological factors that influence the costs and benefits associated with cooperation (Lion and van Baalen 2008; Platt and Bever 2009). Most research on the evolution of cooperation does not explicitly examine the potential importance of such ecological factors. In this study, we employed generalized and realistic theoretical models as well as empirical tests to examine how resource competition and population elasticity help shape the costs and benefits associated with the cooperative pathogenesis of A. tumefaciens.

Standard applications of Hamilton’s rule often neglect important ecological factors determining the costs and benefits associated with cooperation (Platt and Bever 2009). By incorporating effects on local population size, our path model derives a modified form of Hamilton’s rule which demonstrates that cooperative traits which increase local population size have an additional benefit to their between group fitness. Many cooperative behaviors have the potential to enhance local population size, provided that the population is elastic—that is, capable of expanding in response to the alleviation of some factor regulating population size (reviewed by Platt and Bever 2009). Examples of this could be easing predation pressure via cooperative anti-predator behaviors (Krams et al. 2010) as well as cooperative brood care allowing for increased group productivity (Peer and Taborsky 2007). Many other cooperative behaviors can affect local population size by increasing the supply of resources that the group experiences. Examples of this include aphid tending and farming by ants (Mueller et al. 2005; Stadler and Dixon 2005), iron acquisition via siderophore production by a variety of bacteria (West and Buckling 2003), mutualistic rhizobia promoting plant production of specialized metabolites called rhizopines and other exudates (Bever and Simms 2000), and the pathogenesis of agrobacteria in which infected plants produce opines (Dessaux et al. 1998).

As a case study for the consequences of increased local population size on public goods cooperation we examine the selective and population dynamics of agrobacterial cooperation using both explicit ecological models and empirical tests. Plants infected by pathogenic agrobacteria release resources into the rhizosphere that promote the growth of bacteria containing the Ti plasmid, but strains without the plasmid do not benefit (Figure 4). This pathogenesis is a cooperative endeavor—imposing large fitness costs on cells infecting plants while the benefits are publically available (Platt et al. 2011). Our resource-consumer model and experiments demonstrate that when octopine is available at a sufficiently high level, virulent cells harboring the Ti plasmid have a fitness advantage over those lacking the plasmid (Figures 2, 5, and A1). However, when opines are unavailable, pTi⁺ cells are at a fitness disadvantage to those without the plasmid because the plasmid imposes a fitness burden on the cell (Figures 5 and A1). Though the resource-consumer model predicts that in most environments one strain is able to competitively exclude the other, we did not observe this in our experiments. This likely reflects that insufficient time has passed for competitive exclusion to occur. Our results suggest that the disease environments in which opines are being produced by infected plants are likely to act as a source of virulent cells with the Ti plasmid, while non-disease environments in which opines are not produced should act as sinks for these bacteria (Sokurenko et al. 2006).

This within-group fitness advantage of pTi⁺ cells in environments supplied with opines partially reflects the ability of these cells to grow to much higher population sizes than that of cells lacking the plasmid (Figures 3B, 6, and A2, Appendix A). Notably, in the highest octopine treatment we saw that pTi⁻ agrobacteria achieved a significantly lower population size than they sustained when less octopine was present. This likely reflects the pTi⁺ cells growing to such a high population size that they consumed much of the ammonia that was already in low supply. Because of this, pTi⁻ cells experienced a much lower effective ammonia concentration, thus yielding a lower population size. This result is consistent with our resource-consumer model which predicts that the enhanced population size of pTi⁺ cells will have an adverse affect on the equilibrium population size of pTi⁻ cells (Appendix A).

Our resource-consumer model predicts that the local coexistence of virulent cells and avirulent, plasmidless cells is possible when neither strain can drive resource levels below the minimum needs of the other strain (Figure 2). The local coexistence of these strains stems from the increased opportunity of coexistence possible when resource partitioning effectively reduces competition between consumers (Tilman 1982; Chesson 2000). Only pTi⁺ cells can catabolize opines and thus use nitrogen source pools differently than do pTi⁻ cells. Cells lacking the Ti plasmid have higher fitness in opine-free environments with the tradeoff of not being able to catabolize opines when they are present. Thus, the monopoly that pTi⁺ cells have on opine resources facilitates the local coexistence of these strains under certain environmental conditions (Figure 2). The range of resource conditions in which coexistence occurs expands with increasing costs associated with the plasmid (compare Figures 2A and 2B). The concentration of opine at equilibrium is higher when the cost of the plasmid is higher because the population is less able to drive down opine levels. When more opine is available at equilibrium, pTi⁺ cells consume relatively more opine than other nitrogen sources, and thus are less likely to drive resource levels below the minimum needs of pTi⁻ cells. This result is analogous to an increased likelihood of coexistence stemming from an increased degree of resource partitioning, because when plasmid costs are larger, the pTi⁺ cells depend on non-opine sources of nitrogen to a lesser degree due to the higher equilibrium levels of octopine. However, greater plasmid costs also increase the minimum resource needs of pTi⁺ cells. Consequently, increasing costs associated with the plasmid also expand the range of environmental conditions over which the cells lacking the Ti plasmid are able to outcompete the cells with the plasmid (compare Figures 2A and 2B).

Importantly, the benefits of the cooperative pathogenesis of A. tumefaciens tend to be primarily available to other cooperative individuals. Due to linkage of the primary genetic determinants on the Ti plasmid, the pathogenesis genes needed to infect hosts are likely to be co-inherited with the opine catabolism genes required to access the benefits of pathogenesis. Consequently, pathogenesis-dependent opines tend to serve as private resources for virulent cells bearing the Ti plasmid and the Ti plasmid acts as a multigene greenbeard for which the cooperative benefits of the pathogenesis it encodes are inherently directed toward any cell that bears the plasmid (Platt and Bever 2009; Gardner and West 2010). As a result, cells with the plasmid locally outcompete avirulent, plasmidless agrobacteria when opines are present (Figures 2, 5, and A1). In contrast, public goods that are not intrinsically directed toward cooperative individuals would not be expected to provide a within-group advantage to these individuals since non-cooperative bacteria would be capable of freeloading (West et al. 2007; Frank 2010). Our model and data also suggest a possible basis for cooperative agrobacteria having a between-group fitness advantage over non-cooperative individuals. Under nitrogen-limiting conditions, the availability of opines increases the carrying capacity of virulent agrobacterial populations (Figures 3, 6, and A2; Appendix A). Because of this population elasticity, cooperative groups are more likely to contribute more to subsequent generations (Kummerli et al. 2009; Platt and Bever 2009).

This greenbeard system does not necessarily guarantee the competitive dominance of virulent strains within the disease environment. Once a host becomes infected that host becomes fertile habitat for freeloading strains that capitalize on diseased hosts while paying little cost (West et al. 2007; Platt and Bever 2009). The cooperative pathogenesis encoded by the Ti plasmid is vulnerable to cheating by mutant plasmids that lack or do not express genes required for pathogenesis but do confer opine catabolism to their host cells. Other factors being equal, cells with a cheater plasmid are predicted to locally outcompete virulent agrobacteria in all environments, regardless of the availability of opines (Figure 2C). This advantage stems from cheater cells being able to freeload, catabolizing opines without paying the costs associated with pathogenesis. Unlike with competition between pTi⁺ and pTi⁻ agrobacteria, in this case opines are a true public good promoting the growth of all individuals in the group (Frank 2010). As with cooperative agrobacteria, cheater strains also benefit from opine availability with increased local carrying capacity and therefore cheater strains would exclude cooperators in a well mixed system. However, spatial structure would increase the proportion of cooperators within diseased environments, in which case the population elasticity would provide cooperative agrobacteria a between-group fitness advantage over cheater genotypes (Eq. 1). Such spatial separation is likely given that the disease environment is generated by cooperating agrobacteria and there will be a lag before cheaters either emerge through mutation or arrive through dispersal. Avirulent, plasmidless cells are predicted to outcompete cheater cells in the absence of opines due to the costs associated with maintaining the genes necessary for opine catabolism. Thus, as with pTi⁺ cells, non-disease environments are likely to act as sinks, while disease environments should act as sources for cheater strains. Consequently, the persistence of cheaters depends on their either arising via mutation within or migrating into diseased plant environments.

The genes underlying bacterial public goods cooperation are disproportionately found on mobile genetic elements such as plasmids (Nogueira et al. 2009). One possible explanation for this pattern is that horizontal transmission promotes relatedness at these loci, thereby helping stabilize cooperation (Smith 2001; Rankin et al. 2011). A limitation of this explanation, however, is that it relies on the cooperative plasmid being able to horizontally transfer into potential freeloading strains, which may not be constrained when the ability to freeload depends on having a plasmid incompatible with the cooperative plasmid (Mc Ginty et al. 2011). This is likely to be the case with agrobacterial cooperative pathogenesis since freeloading plasmids can be derived from cooperative Ti plasmids. Such plasmids exist in nature and have been dubbed opine catabolic plasmids (Merlo and Nester 1977; Dessaux et al. 1986; Nautiyal and Dion 1990; Dessaux et al. 1998). The best characterized opine catabolic plasmid is the nopaline catabolic plasmid of A. radiobacter K84, a strain that has served for several decades as a powerful biocontrol agent of crown gall disease (Clare et al. 1990; Kim et al. 2006). Strain K84 is a strong antagonist of several virulent agrobacteria because it is able to access the resource benefits of opines without paying the costs associated with virulence and interferes with the growth of virulent strains via the production of a bacteriocin that specifically targets specific opine catabolic agrobacteria (Reader et al. 2005; Kim et al. 2006).

While the focus of our competition model and experiments is on local scale interactions, the long-term dynamics of the Ti plasmid will also depend upon possible biological feedbacks—such as the fact that the local prevalence of pathogenic agrobacteria likely influences whether or not pathogenesis-induced opines are present. While the path model identifies critical aspects of how biological feedbacks can influence this system, more explicit models linking the local dynamics of resource competition with dispersal out of these groups and infection of new hosts remains a challenge for future work.

Natural agrobacterial variation reflects at least two classes of genotypes that cooperative agrobacteria must contend with in order to persist. The population dynamics and maintenance of cooperative agrobacteria depend on how they fare in competition with both avirulent pTi⁻agrobacteria and cheater stains that are able to freeload upon the benefits of its pathogenesis on plants. We have presented a theoretical and empirical analysis of how resource competition and population elasticity shape the costs and benefits associated with the public and private goods of cooperative pathogenesis of A. tumefaciens. We demonstrate that the pathogenesis encoded by the Ti plasmid promotes the within-group fitness of cells with the plasmid over pTi⁻ cells due to opines serving as a private good for pTi⁺ cells. We also observe that the benefits of pathogenesis increase the local carrying capacity of virulent agrobacteria, thereby enhancing the between-group fitness of virulent agrobacteria over both pTi⁻ and freeloading agrobacteria. This effect is critically important to the persistence of cooperative pathogenesis in the face of competition from cheater bacterial strains which always have greater within-group fitness. Our path model demonstrates that this advantage associated with elasticity in population size requires that agrobacterial populations are genetically structured such that the pathogen can harness the benefits of opines, preventing freeloading genotypes from fully usurping disease environments. Thus the persistence of the cooperative agrobacterial pathogens in the face of intrinsic kin competition depends on how resource competition, population elasticity, and relatedness shape the pathogen’s cooperative benefits, illustrating the need to explicitly model each of these factors.

Appendix A Analysis of resource- consumer model

Model details

Neighboring A. tumefaciens lineages compete for a variety of resources. When in the vicinity of a crown gall, virulent pTi⁺ cells have access to opine resources that avirulent pTi⁻cells are unable to metabolize. The suite of opines released by infected plants varies considerably and depends on the type of Ti plasmid genetically transforming the plant (Dessaux et al. 1998). In this paper, we focus on octopine-type Ti plasmids whose T-DNA promotes plant production of several related opines (octopine-type opines and mannityl opines, that are derived from amino acids and simple organic acids or sugars) which can serve as nutritional sources of carbon and/or nitrogen for octopine-type pTi⁺ cells (Petit et al. 1983; Dessaux et al. 1998). Previous research suggests that for most octopine-type pTi⁺ cells, octopine is a more efficient source of nitrogen than it is for carbon (Bell 1990). To simplify discussion of the model we assume that nitrogen is limiting, though the presented model can alternatively be interpreted to represent opines acting as a source of carbon under carbon-limiting conditions.

Our model builds on the classic models of microbial dynamics of Monod (1949) and Tilman (1980; Tilman et al. 1982) to describe the population dynamics of pTi⁺ and pTi⁻ cells competing for two potentially limiting but substitutable resources: nitrogen-containing opines and other nitrogen sources. Our model explicitly tracks resource dynamics and assumes that all competitive interactions are mediated by limiting resources. The population growth rate of pTi⁺ cells is determined by the relative balance of the strain’s birth rate, which depends on the harvest and utilization of both opines (O) and non-opine nitrogen sources (A), and its death rate, which occurs at a constant per capita rate (m_pTi⁺; see Table 2 for complete description of parameters).

Table 2.

Resource- consumer model notation summary

Symbol	Description
Ni	Population size of the i-th consumer
A	Concentration of non-opine nitrogen sources at the site of competition
O	Concentration of opine nitrogen sources at the site of competition
Dj	Rate that j-th resource flows away from the site of competition
A0	Concentration at which non-opine nitrogen sources flow into the site of competition
O0	Concentration at which opine nitrogen sources flow into the site of competition
$e_i^j$	Probability of the i-th strain encountering and accepting a unit of the j-th resource
$u_i^j$	Utilization efficiency of the i-th strain for the j-th resource
$h_i^j$	Handling time of the i-th strain for the j-th resource
mi	Per capita mortality rate of the i-th strain

$$\frac{{dN}_{{\mathit{pTi}}^{+}}}{dt}=\left(\frac{u_{{\mathit{pTi}}^{+}}^A{e}_{{\mathit{pTi}}^{+}}^{A}A+u_{{\mathit{pTi}}^{+}}^O{e}_{{\mathit{pTi}}^{+}}^{O}O}{1+e_{{\mathit{pTi}}^{+}}^A{h}_{{\mathit{pTi}}^{+}}^{A}A+e_{{\mathit{pTi}}^{+}}^O{h}_{{\mathit{pTi}}^{+}}^{O}O}\right)N_{{\mathit{pTi}}^{+}}-m_{{\mathit{pTi}}^{+}}{N}_{{\mathit{pTi}}^{+}}$$

Similarly, the population growth rate of cells lacking the Ti plasmid also depends on its relative birth and death rates, however the birth rate of these cells depends only on the harvest and utilization of non-opine nitrogen sources since it cannot catabolize opines.

$$\frac{{dN}_{{\mathit{pTi}}^{-}}}{dt}=\left(\frac{u_{{\mathit{pTi}}^{-}}^A{e}_{{\mathit{pTi}}^{-}}^{A}A}{1+e_{{\mathit{pTi}}^{-}}^A{h}_{{\mathit{pTi}}^{-}}^{A}A}\right)N_{{\mathit{pTi}}^{-}}-m_{{\mathit{pTi}}^{-}}{N}_{{\mathit{pTi}}^{-}}$$

Plants manifesting crown gall disease typically release a suite of opine molecules (Dessaux et al. 1998) and there are likely to be several additional forms of non-opine nitrogen sources in the rhizosphere (Nannipieri and Eldor 2009). For simplicity and practicality, we describe the amount of these classes of resources by aggregate terms (e.g. O and A). More realistically, competition will depend on the resource dynamics of many more substrates that agrobacterial cells may utilize, encounter, or handle differently. The rate at which opine levels change is determined by the relative balance of the rate at which opines are supplied to the environment and the rate at which pTi⁺ cells catabolize them. We assume that both types of resources are flowing into and out of the environment at a fixed rate (D_O and D_A). Nutrients flow into the environment at a constant concentration (O₀ and A₀), flow out of the environment at their present concentration in the environment (O and A), and are not recycled following cell death.

$$\frac{dO}{dt}=D_O(O_0-O)-\left(\frac{e_{{\mathit{pTi}}^{+}}^{O}O}{1+e_{{\mathit{pTi}}^{+}}^A{h}_{{\mathit{pTi}}^{+}}^{A}A+e_{{\mathit{pTi}}^{+}}^O{h}_{{\mathit{pTi}}^{+}}^{O}O}\right)N_{{\mathit{pTi}}^{+}}$$

Changes in environmental levels of non-opine nitrogen sources also reflect the relative balance of the rate at which they are supplied to the environment and the rate at which they are consumed. However, unlike opines they can be consumed by both pTi⁺ and pTi⁻ cells.

$$\frac{dA}{dt}=D_A(A_0-A)-\left(\frac{e_{{\mathit{pTi}}^{+}}^{A}A}{1+e_{{\mathit{pTi}}^{+}}^A{h}_{{\mathit{pTi}}^{+}}^{A}A+e_{{\mathit{pTi}}^{+}}^O{h}_{{\mathit{pTi}}^{+}}^{O}O}\right)N_{{\mathit{pTi}}^{+}}-\left(\frac{e_{{\mathit{pTi}}^{-}}^{A}A}{1+e_{{\mathit{pTi}}^{-}}^A{h}_{{\mathit{pTi}}^{-}}^{A}A}\right)N_{{\mathit{pTi}}^{-}}$$

The model assumes that the substitutable resources co-occur such that the harvest rate of pTi⁺ cells can be described by the two-resource extension of Holling’s disc equation (Holling 1959; Vincent et al. 1996). In order to isolate the effects of resource competition on agrobacterial population dynamics, at present our model does not allow for the horizontal transmission of the Ti plasmid, however opines are known to stimulate conjugation of the plasmid (Kerr et al. 1977; Farrand 1998). The importance of conjugation to the fitness of the Ti plasmid in the disease environment is itself an interesting issue for future theoretical and empirical investigation.

Conditions for coexistence

Coexistence of pTi⁺ and pTi⁻ bacteria is possible if 1) the slope of the pTi⁺ consumption vector is greater than that of the pTi⁻ consumption vector; and 2) the minimum non-opine nitrogen source supply of pTi⁺ cells is greater than that of pTi⁻ cells ( $A_{{\mathit{pTi}}^{-}}^{\ast }<A_{{\mathit{pTi}}^{+}}^{\ast }$) (Vincent et al. 1996). This first condition is always true provided that opines are present in the environment, since pTi⁻ cells are not able to consume opines, while pTi⁺ cells can consume opines (Figure 2). pTi⁺ cells require more non-opine nitrogen supply than pTi⁻ cells when

$$\frac{m_{{\mathit{pTi}}^{-}}}{e_{{\mathit{pTi}}^{-}}^A(u_{{\mathit{pTi}}^{-}}^A-m_{{\mathit{pTi}}^{-}}{h}_{{\mathit{pTi}}^{-}}^A)}<\frac{m_{{\mathit{pTi}}^{+}}}{e_{{\mathit{pTi}}^{+}}^A(u_{{\mathit{pTi}}^{+}}^A-m_{{\mathit{pTi}}^{+}}{h}_{{\mathit{pTi}}^{+}}^A)}.$$ (Equation 2)

There are four ways this can happen: 1) pTi⁺ cells have a higher mortality rate (m_pTi⁺> m_pTi⁻); 2) pTi⁻ cells are more efficient at utilizing non-opine nitrogen sources ( $u_{{\mathit{pTi}}^{-}}^A>u_{{\mathit{pTi}}^{+}}^A$); 3) pTi⁺ cells have a greater handling time of non-opine nitrogen sources ( $h_{{\mathit{pTi}}^{+}}^A>h_{{\mathit{pTi}}^{-}}^A$); or 4) pTi⁻ cells are more likely to encounter and accept these nutrients ( $e_{{\mathit{pTi}}^{-}}^A>e_{{\mathit{pTi}}^{+}}^A$). We have experimentally demonstrated that there is a fitness cost associated with harboring the Ti plasmid under resource liming conditions (Platt et al. 2011), suggesting that the inequality in Equation 2 is true; however we do not currently know which parameter(s) underlies this cost. Most likely, the cost is a result of differences in mortality rates or utilization efficiencies; however this remains an issue for future work.

Resource competition and population size

A population of pTi⁺ cells growing in a nitrogen-limited environment supplied with both opine and non-opine nitrogen sustains an equilibrium population size that depends on the supply rates of both types of resources (Figure 3):

$$N_{{\mathit{pTi}}^{+}}^{\ast }=[D_A(A_0-A^{\ast }]+D_O(O_0-O^{\ast })]\ast \left[\frac{1+e_{{\mathit{pTi}}^{+}}^A{h}_{{\mathit{pTi}}^{+}}^A{A}^{\ast }+e_{{\mathit{pTi}}^{+}}^O{h}_{{\mathit{pTi}}^{+}}^O{O}^{\ast }}{e_{{\mathit{pTi}}^{+}}^A{A}^{\ast }+e_{{\mathit{pTi}}^{+}}^O{O}^{\ast }}\right]$$

In contrast, because pTi⁻ cells cannot catabolize opine nitrogen resources, the equilibrium population size of a pTi⁻ population depends only on the supply rate of non-opine nitrogen sources (Figure 3):

$$N_{{\mathit{pTi}}^{-}}^{\ast }=[D_A(A_0-A^{\ast }]\ast \left[\frac{1+e_{{\mathit{pTi}}^{-}}^A{h}_{{\mathit{pTi}}^{-}}^A{A}^{\ast }}{e_{{\mathit{pTi}}^{-}}^A{A}^{\ast }}\right]$$

When both consumers are present, the equilibrium population sizes of pTi⁺ and pTi⁻ cells partially depend on the degree to which the other strain consumes the resources making them effectively unavailable:

$$N_{{\mathit{pTi}}^{+}}^{\ast }=\left[D_A(A_0-A^{\ast })-N_{{\mathit{pTi}}^{-}}^{\ast }\left[\frac{e_{{\mathit{pTi}}^{-}}^A{A}^{\ast }}{1+e_{{\mathit{pTi}}^{-}}^A{h}_{{\mathit{pTi}}^{-}}^A{A}^{\ast }}\right]\right]\ast \left[1+e_{{\mathit{pTi}}^{+}}^A{h}_{{\mathit{pTi}}^{+}}^A{A}^{\ast }+e_{{\mathit{pTi}}^{+}}^O{h}_{{\mathit{pTi}}^{+}}^O{O}^{\ast }\right]\ast \left[\frac{1}{e_{{\mathit{pTi}}^{+}}^{A}A}\right]$$ Equation 3

$$N_{{\mathit{pTi}}^{-}}^{\ast }=\left[D_A(A_0-A^{\ast })-N_{{\mathit{pTi}}^{+}}^{\ast }\left[\frac{e_{{\mathit{pTi}}^{+}}^A{A}^{\ast }}{1+e_{{\mathit{pTi}}^{+}}^A{h}_{{\mathit{pTi}}^{+}}^A{A}^{\ast }+e_{{\mathit{pTi}}^{+}}^O{h}_{{\mathit{pTi}}^{+}}^O{O}^{\ast }}\right]\right]\ast \left[1+e_{{\mathit{pTi}}^{-}}^A{h}_{{\mathit{pTi}}^{-}}^A{A}^{\ast }\right]\ast \left[\frac{1}{e_{{\mathit{pTi}}^{-}}^A{A}^{\ast }}\right]$$ Equation 4

From Equation 3 we identified that the equilibrium population size of pTi⁺ cells increases linearly with increasing opine supply. In contrast, the equilibrium population size of pTi⁻ cells does not depend on opine supply, but is predicted to decline when opine availability is high due to resultant large populations of pTi⁺ cells removing non-opine nitrogen sources from the environmental supply (see Equation 4).

Appendix B Costs and benefits of pTiR10

In order to demonstrate that the costs and benefits associated with the Ti plasmid that we have demonstrated are not specific to the strain we are working with (A. tumefaciens 15955) we tested our model’s predictions using A. tumefaciens R10 which harbors an octopine-type Ti plasmid. We competed this strain against a kanamycin resistant derivative strain cured of pTiR10 (KYC55) under environmental conditions containing octopine (6.5 mM) or lacking this resource. KYC55 and R10 are demonstrably not as isogenic as the 15955/TP101 pair used for the bulk of our studies (Cho et al. 1997). Consistent with our previous results we observed that pTi⁺ R10 cells are at a competitive disadvantage to pTi⁻ KYC55 cells when octopine is absent from the environment (Figure A1, t = −2.2, 12 df, p < 0.05). Also as predicted, octopine availability significantly increased the fitness of cells harboring the virulence plasmid (Figure A1, F_1,12 = 6.71, p < 0.05). For this experiment the media contained AT minimal media salts, 500 μM phosphate, and was buffered by MES to pH 5.6. Under these conditions carbon availability limits population growth. We differentiated strains by plating onto media that either lacked or contained 150 μg/ml kanamycin.

We also observed that opine availability increased the carrying capacity of pTi⁺ R10 populations (t = −4.25, df = 7, p < 0.01), but did not affect the carrying capacity of pTi⁻ KYC55 populations (Figure A2, t = −2.16, df = 11, ns).

Appendix C Population elasticity in response to opine availability

A. tumefaciens strain 15955 achieved higher population density in response to being supplied with higher levels of octopine as the sole source of nitrogen (Figure A3A; β_[Oct] = 1.1, t = 13.8, p < 0.001) and as the sole source of carbon (Figure A3B; β_[Oct] = 0.1, t = 9.4, p < 0.001). In both cases, this effect eventually saturated, suggesting that other factors besides nitrogen or carbon availability, respectively, became limiting (when octopine was the sole nitrogen source: β_[Oct]²=−0.17, t = −8.6, p < 0.001; and when octopine was the sole carbon source: : β_[Oct]²=−0.002, t = −3.6, p < 0.01). OD₆₀₀ nm was measured 87 hours after inoculation. Values are mean ± standard error of three replicates.