Evaluation of Serum Creatinine Concentration–Based Glomerular Filtration Rate Equations in Pediatric Patients with Chronic Kidney Disease

Abstract

Study Objective

To evaluate the accuracy of four equations based on serum creatinine concentration—the original Schwartz equation and the Leger, Bedside Chronic Kidney Disease in Children (CKiD), and Counahan-Barratt equations—for determining glomerular filtration rate (GFR) in pediatric patients with chronic kidney disease.

Design

Retrospective, observational, cross-sectional study.

Setting

Single-center, academic, outpatient pediatric nephrology clinic.

Patients

Fifty-three pediatric patients with stages 2–5 chronic kidney disease who completed GFR assessment with ¹²⁵I-iothalamate between January 2002 and January 2005.

Measurement and Main Results

Data were collected from each patient’s medical record. Glomerular filtration rate data were analyzed using 59 evaluations from the 53 pediatric patients. ¹²⁵I-iothalamate clearance was used as the index GFR. The Bedside CKiD and Counahan-Barratt equations outperformed the Schwartz and Leger equations when the index GFR was less than 60 ml/minute/1.73 m²; the Schwartz and Counahan-Barratt equations performed best for index GFRs of 60 ml/minute/1.73 m² or greater. Overestimation was highest with the Schwartz and Leger equations (> 20% index GFR in 57.6% and 62.7% of patients, respectively). Underestimation was highest with the Bedside CKiD and Counahan-Barratt equations (> 20% index GFR in 30.5% and 28.8%, respectively).

Conclusion

The new Bedside CKiD equation performed well for pediatric patients with moderate-to-severe chronic kidney disease, but less well for pediatric patients with mild disease. Additional studies are needed to develop more precise GFR equations using serum creatinine concentration.

Glomerular filtration rate (GFR) provides an excellent measure of kidney filtering capacity and is a necessary clinical parameter for dosing drugs with significant renal elimination characteristics. Inulin clearance is widely regarded as the gold standard for determining GFR, but it is not used clinically because of its inconvenience and cost.¹ As a result, several alternative measures for estimating GFR have been devised. Urine-based measures of the renal clearance of exogenous radioactive markers, such as ¹²⁵I-iothalamate and ⁵⁴Cr-ethylenediaminetetraacetic acid, provide excellent measures of GFR but are not widely available in clinical practice.² Plasma clearance of exogenous substances, including iothalamate and iohexol, has been used as well.² Each of these methods has been used to assess GFR in children; however, the need to inject exogenous substances and the imprecision of urine collections and repeated blood sampling make these methods unfeasible for measuring GFR in children. The most widely used measures of GFR in clinical practice are based on endogenous serum creatinine concentration and 24-hour creatinine clearance. Estimating GFR from serum creatinine concentration in children is problematic. Serum creatinine concentrations vary with age, and normal ranges at any age are broad, as creatinine values are dependent on muscle mass as well as kidney function. In addition, elevation of serum creatinine concentrations can suggest more serious renal impairment in children than in adults, who have greater muscle mass.

Equations that estimate GFR based on serum creatinine concentration provide a substantial improvement over serum creatinine concentration alone in predicting GFR in children as well as in adults. Serum creatinine–based equations have advantages over 24-hour urine collections, as they produce faster results and do not require urine collection, which can be inaccurate. In fact, the National Kidney Foundation Kidney Disease Outcomes Quality Initiative (KDOQI) 2000 guidelines recommend the use of estimating GFR equations to establish the presence of chronic kidney disease (CKD). Many studies have examined the utility of different equations for predicting GFR and creatinine clearance from serum creatinine concentration. A KDOQI review suggested the Schwartz formula or Counahan-Barratt equation for children and the Modification of Diet in Renal Disease formula for adults.³ Since that time, several new GFR-estimating equations have been developed. In this study, we evaluated the accuracy of four glomerular filtration rate equations based on serum creatinine concentration—the original Schwartz equation and the Leger, Bedside Chronic Kidney Disease in Children (CKiD), and Counahan-Barratt equations— in pediatric patients with CKD by using the plasma disappearance of iothalamate as the index GFR measure.

Methods

Glomerular filtration rate data were analyzed from patients with CKD who were seen in a single-center, academic, outpatient, pediatric nephrology clinic between January 2002 and January 2005. The inclusion criteria were age of 2–22 years for patients who were evaluated, presence of stages 2–5 CKD at the time of GFR assessment, and GFR assessment completed with ¹²⁵I-iothalamate (Glofil-125; Iso-Tex Diagnostics, Friendswood, TX) clearance. Exclusion criteria were age younger than 2 years, receipt of chronic renal replacement therapy, and poor intravenous access. Data collected from each patient’s medical record included height, weight, age, sex, raceethnicity, and ¹²⁵I-iothalamate clearance. Institutional review board approval was obtained from the participating institutions.

¹²⁵I-Iothalamate Clearance Procedure

Patients were given an intravenous infusion of ¹²⁵I-iothalamate 0.357 µCi/kg at a rate of 0.5 ml/minute. Before ¹²⁵I-iothalamate administration, patients fasted for 6–8 hours and were orally hydrated with 10–15 ml/kg of water. Serum was collected at 5, 10, and 15 minutes and at 4 hours after ¹²⁵I-iothalamate infusion; if the patient’s serum creatinine concentration was greater than 2 mg/dl, one additional measurement was obtained at 5 or 6 hours after the end of infusion to ensure adequate sampling in the elimination phase for patients with reduced GFR. Clearance corrected for body surface area was calculated as described previously.⁴

Glomerular Filtration Rate Estimation Methods

The Schwartz, Leger, Bedside CkiD, and Counahan-Barratt equations were selected for this evaluation (Table 1).^5–8 The Bedside CKiD (updated Schwartz equation) and Counahan-Barratt equations are currently recommended for pediatric patients in the National Kidney Foundation GFR calculators for health care professionals. ³ The Leger equation was developed from nonlinear mixed-effects modeling.⁶ The newer Bedside CKiD and Counahan-Barratt equations are serum creatinine concentration–based formulas that can be used readily in the clinical setting.^{7, 8} The modified Jaffe method was used to determine serum creatinine concentrations.

Table 1.

Glomerular Filtration Rate Estimation Equations

Method	Equation	Patient Variables
Schwartz5	GFR (ml/min/1.73 m2) = k • HT ÷ Scr	k = 0.55 for children and female adolescents
		k = 0.7 for male adolescents
		HT = height (cm)
		Scr = serum creatinine concentration (mg/dl)
Leger6	GFR (ml/min) = [56.7 • WT + 0.142 • HT2] ÷ Scr	WT = weight (kg)
	GFR corrected for body surface area	HT = height (cm)
	(Mosteller method9 [WT • HT/3600]0.5)	Scr = serum creatinine concentration (µM); to convert to the value in µM, multiply value in mg/dl by 88.4
Bedside Chronic	GFR (ml/min/1.73 m2) = 0.413 • HT ÷ Scr	HT = height (cm)
Kidney Disease in Children (CKiD)7		Scr = serum creatinine concentration (mg/dl)
Counahan-Barratt8	GFR (ml/min/1.73 m2) = 0.43 • HT ÷ Scr	HT = height (cm)
		Scr = serum creatinine concentration (mg/dl)

GFR = glomerular filtration rate.

Comparison with ¹²⁵I-Iothalamate Clearance

¹²⁵I-iothalamate clearance was considered the patients’ actual or index GFR in this study. Calculated results of the GFR estimation methods were compared with the respective ¹²⁵I-iothalamate clearance for each patient by assessing the goodness of fit, bias and accuracy, and a Bland-Altman analysis to measure agreement.¹⁰ The goodness-of-fit plots were constructed by comparing the ¹²⁵I-iothalamate clearance with the corresponding calculated GFR method. Linear regression was performed to assess the linearity for agreement with each GFR method.

Bias was calculated as a percentage with the following formula:

$$\%\text{Bias}=\frac{[(\text{Calculated GFR method value})-({}^{125}\mathrm{I}-\text{iothalamate GFR value})]}{{}^{125}\mathrm{I}-\text{iothalamate GFR value}}\times 100$$

Accuracy was assessed by evaluating the ability of the respective calculated data set to be within ±10%, 20%, and 30% of the index ¹²⁵I-iothalamate GFR value. Precision was inferred from the standard deviations of the measurements. Bland-Altman plots were undertaken to measure agreement with the respective calculation methods.¹⁰

Results

During the study time frame, a total of 59 evaluations of GFR data from 53 patients were analyzed. Demographic and clinical characteristics of the patients are presented in Table 2. Patients ranged in age from 2–22 years and had a mean serum creatinine concentration of 1.94 mg/dl (range 0.3–6.8 mg/dl). Approximately 50% of the patients had a primary diagnosis of a congenital renal abnormality (e.g., obstructive uropathy, renal dysfunction, and hydronephrosis), and diagnoses for the remaining patients consisted of Alport syndrome, Henoch-Schonlein purpura, focal glomerulosclerosis, and post acute renal insufficiency. Eighteen (34%) of the 53 patients received kidney transplants. The mean ± SD index GFR for the 59 tests in the 53 patients was 50.3 ± 33.1 ml/minute/1.73 m²; the median index GFR was 41.6 ml/minute/1.73 m².

Table 2.

Demographic and Clinical Characteristics of the 53 Patients Receiving 59 Evaluations

Characteristic	Mean ± SD (range)	Median
Age (yrs)	12.0 ± 4.7 (2–22)	12
Weight (kg)	48.8 ± 26.5 (10.8–120)	46
Height (cm)	140.9 ± 23.9 (82.6–181)	144
aBody surface area (m2)	1.35 ± 0.47 (0.50–2.37)	1.38
Serum creatinine (mg/dl)	1.94 ± 1.37 (0.3–6.8)	1.4
bIndex GFR (ml/min/1.73 m2) (based on 59 evaluations)	50.3 ± 33.1 (7.5–142.3)	41.6
	No. of Patients
Sex
Male	33
Female	20
Race-ethnicity
Caucasian	24
Hispanic	14
African-American	10
Asian	3
Middle Eastern	2
Received kidney transplant	18

GFR = glomerular filtration rate.

^aUsing the Mosteller method⁹: (weight [in kg] • height [in cm]/3600)^0.5.

^bIndex GFR assessed by ¹²⁵I-iothalamate clearance.

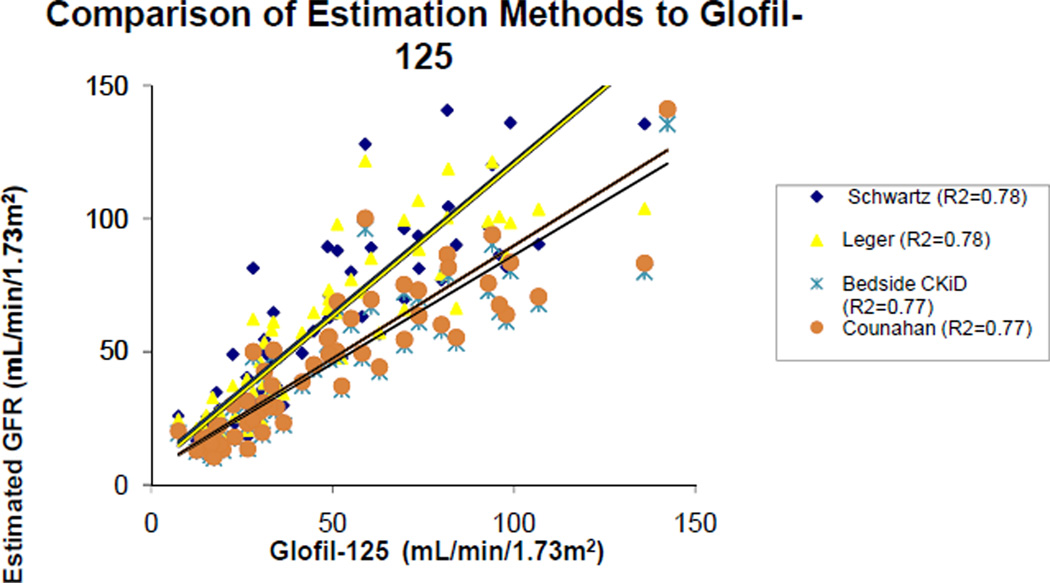
A comparison of GFR estimation methods with the index ¹²⁵I-iothalamate clearance method is presented in Figure 1. The correlation coefficients (r² values) for the Schwartz, Leger, Bedside CkiD, and Counahan-Barratt equations were 0.78, 0.78, 0.77, and 0.77, respectively. The percentages of index GFR measures that were overestimated by more than 20% were 57.6% for the Schwartz, 62.7% for the Leger, 13.6% for the Bedside CKiD, and 15.3% for the Counahan-Barratt equations. By contrast, the percentages of index GFR measures that were underestimated by more than 20% were 1.69% for the Schwartz, 8.47% for the Leger, 30.5% for the Bedside CKiD, and 28.8% for the Counahan-Barratt equations. The overall mean ± SD percent bias for the Schwartz, Leger, Bedside CkiD, and Counahan-Barratt equations was 36.9 ± 48.4%, 33.7 ± 43.1%, –3.56 ± 33.5%, and 0.41 ± 34.9%, respectively.

Figure 1.

Comparison of estimated glomerular filtration rate (GFR) using four equations with actual GFR determined by ¹²⁵I-iothalamate (Glofil-125) clearance. CKiD = Chronic Kidney Disease in Children.

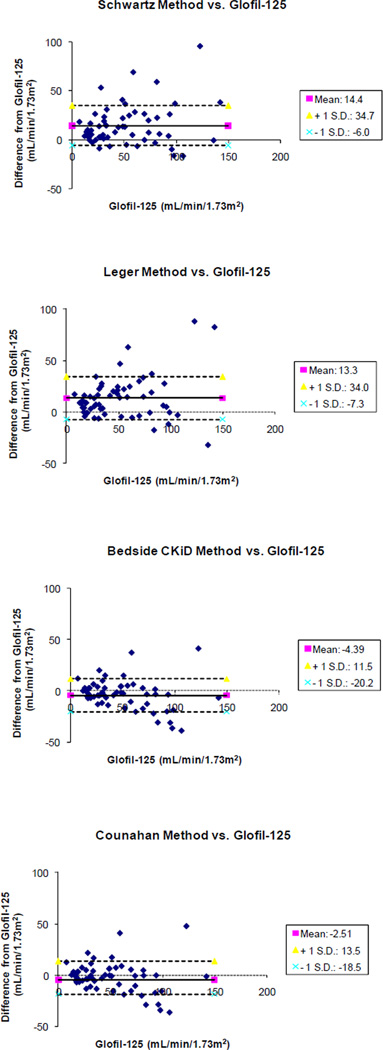
The overall percentages of patients with an estimated GFR within ± 30% of the index ¹²⁵I-iothalamate GFR were 54.2%, 52.5%, 67.8%, and 71.2% for the Schwartz, Leger, Bedside CkiD, and Counahan-Barratt equations, respectively. Bias and accuracy of each equation for the different stages of CKD are presented in Table 3. The Bland-Altman analysis for each estimating method is presented in Figure 2. The mean difference between the Schwartz estimated GFR and the index GFR was 14.4 ml/minute/ 1.73 m², and the mean difference between the Leger estimated GFR was 13.3 ml/minute/173 m², whereas the mean differences between the Bedside CKiD and Counahan-Barratt estimated GFRs and the index GFR were lower at –4.39 and –2.51 ml/minute/1.73 m², respectively, which indicates better unity than the Schwartz and Leger equations. In addition, the Bedside CKiD and Counahan-Barratt equations had smaller standard deviations, which indicate better precision than the Schwartz and Leger equations: 15.84 and 16.02 ml/minute/1.73 m² compared with 20.35 and 20.64 ml/minute/1.73 m², respectively.

Table 3.

Bias and Accuracy Analysis of the Four Equations for Estimating Glomerular Filtration Rate by Degree of Chronic Kidney Disease

	Schwartz Equation5		Leger Equation6		Bedside CKiD Equation7		Counahan-Barratt Equation8
125I-Iothalamate GFR (ml/min/1.73 m2)	Mean ± SD Percent Bias	Percentage Within ± 30%a	Mean ± SD Percent Bias	Percentage Within ± 30% a	Mean ± SD Percent Bias	Percentage Within ± 30%a	Mean ± SD Percent Bias	Percentage Within ± 30%a
≥ 60   (19 evaluations)	18.3 ± 27.8%	73.7%	16.3 ± 27.9%	63.2%	−15.5 ± 19.9%	63.2%	−12.0 ± 20.7%	73.7%
30–59   (20 evaluations)	39.8 ± 35.5%	50%	39.2 ± 34.2%	40%	0.14 ± 26.4%	70%	4.3 ± 27.5%	70%
≤ 29   (20 evaluations)	51.6 ± 67.6%	40%	44.7 ± 57.5%	50%	4.1 ± 46.2%	70%	8.27 ± 48.2%	70%

GFR= glomerular filtration rate; CKiD = Chronic Kidney Disease in Children.

^aPercentage of patients with estimated GFR within ± 30% of ¹²⁵I-iothalamate GFR.

Figure 2.

Bland-Altman plots for each glomerular filtration rate (GFR) estimation method versus actual GFR determined by ¹²⁵I-iothalamate (Glofil-125) clearance. CKiD = Chronic Kidney Disease in Children.

Discussion

In this study we evaluated the accuracy of four different serum creatinine concentration–based equations to estimate GFR in pediatric patients with CKD on the basis of equation bias and precision. In assessing accuracy, we determined how close the average of the estimating equations was to the true GFR value. We used the term accuracy to encompass both precision (how close the estimates are to each other) and bias (the systematic difference between the estimate and the measured value). We found that the Bedside CKiD and Counahan-Barratt equations outperformed the other two methods when the index GFR was less than 60 ml/minute/1.73 m². The Schwartz and Counahan-Barratt formulas performed the best for index GFRs of 60 ml/minute/1.73 m² or greater. The Schwartz and Leger equations performed similarly for estimating GFR in children with CKD, but with concerns of overestimation, whereas the very similar Bedside CKiD and Counahan-Barratt formulas performed similarly, with concerns of underestimation.

Bias expresses the systematic deviation from the index measure of GFR. In this study we used the plasma disappearance of iothalamate as the index GFR. The mean difference between the actual measured GFR with iothalamate and the estimated GFR based on an equation provides a valid measure of bias. In our study, the bias for the Schwartz and Leger formulas was 14.4 and 13.3 ml/minute/1.73 m², respectively, and the bias for the Bedside CKiD and Counahan-Barratt equations was –4.39 and –2.51 ml/ minute/1.73 m², respectively. Precision expresses the variability of the estimating equation’s GFR value around the index GFR measure. The standard deviation of the difference between the measured GFR and the estimated GFR based on an equation provides a valid measure of precision. The difference from the index GFR standard can also be expressed as a relative difference. We reported the percent difference from the measured GFR. This has the advantage of allowing for the decreased absolute precision in estimating higher values of GFR. At all three GFR levels (≥ 60, 30–59, and ≥ 29 ml/min/ 1.73 m²), the Bedside CKiD and Counahan-Barratt formulas consistently resulted in better precision than the Schwartz and Leger formulas.

The correlation for each of the equations was good. However, correlation measures relative rather than absolute agreement between methods. In addition, the correlation coefficient is highly dependent on the distribution of GFRs in the study population selected. The distribution of index GFRs in our patients ranged from 7.5–142.3 ml/minute/1.73 m².

One limitation of applying estimating equations to a broad sample of children in different centers is that the estimation of GFR is critically dependent on calibration of the serum creatinine assay, which varies across different laboratories. The difference in measurement of serum creatinine concentration among the laboratories in which all of the four formulas were originally developed may account for the differences in bias and precision seen in this study compared with the originally reported values. The modified Jaffe method used in the Counahan-Barratt equation and the enzymatic creatinine method for the Bedside CKiD equation are thought to be more accurate methods for determining serum creatinine concentration.¹¹

Body surface area is typically used to normalize index GFR values, but it has been shown not to correlate well when normalizing GFR with age and size.^{12, 13} Extracellular fluid volume (vECF) has been suggested as a better metric than body surface area for normalizing GFR; however, age-specific normal values for vECF-normalized GFR are currently not known.¹³ Issues surrounding GFR normalization with body surface area may have influenced the results of this analysis.

The Bedside CKiD and Counahan-Barratt formulas have been developed in children with median GFRs of 43 and 41.3 ml/minute/1.73 m², respectively, and performed very well in our population with a similar median GFR of 41.6 ml/minute/1.73 m². Performance in children with milder kidney disease (GFR ≥ 60 ml/ min/1.73 m²) in this study was weaker. This finding is consistent with two recent evaluations of the Bedside CKiD formula in children with better kidney function (mean ± SD index GFRs of 110.6 ± 28.7 and 101.3 ± 32 ml/min/ 1.73 m²), as better performance and accuracy were seen with GFRs less than 60 and less than 90 ml/minute/1.73 m².^{14, 15}

Measurement of progression of kidney disease is substantially more difficult than diagnosis of the presence of kidney disease, since progression of many forms of kidney disease is slow. Serum creatinine concentration–based estimates of GFR will allow for reliable detection of substantial progression (> 25–50% decline). However, substantial changes in individual creatinine metabolism can occur and will lead to false measures of progression. Therefore, in prospective research studies of kidney disease progression, more direct measures of GFR are still necessary.

For the evaluation of kidney function in children, we still need more precise measures of GFR than the current equations based on serum creatinine concentration. The value of these equations in terms of bias, precision, and practicality should be well tested in large samples of patients with and without kidney disease. In addition, these equations should be validated for the appropriate dosing of renally eliminated drugs in children with renal impairment.

Conclusion

The new Bedside CKiD equation performed well for pediatric patients with moderate-to-severe chronic kidney disease, but less well for children with mild disease. Additional studies are needed to develop more precise GFR equations using serum creatinine concentration.