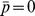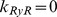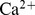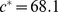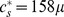Activation of Store-Operated Calcium Entry in Airway Smooth Muscle Cells: Insight from a Mathematical Model

Abstract

Intracellular 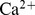dynamics of airway smooth muscle cells (ASMC) mediate ASMC contraction and proliferation, and thus play a key role in airway hyper-responsiveness (AHR) and remodelling in asthma. We evaluate the importance of store-operated 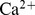entry (SOCE) in these 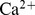dynamics by constructing a mathematical model of ASMC 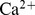signaling based on experimental data from lung slices. The model confirms that SOCE is elicited upon sufficient 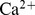depletion of the sarcoplasmic reticulum (SR), while receptor-operated 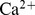entry (ROCE) is inhibited in such conditions. It also shows that SOCE can sustain agonist-induced 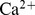oscillations in the absence of other 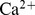influx. SOCE up-regulation may thus contribute to AHR by increasing the 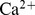oscillation frequency that in turn regulates ASMC contraction. The model also provides an explanation for the failure of the SERCA pump blocker CPA to clamp the cytosolic 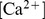of ASMC in lung slices, by showing that CPA is unable to maintain the SR empty of 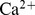. This prediction is confirmed by experimental data from mouse lung slices, and strongly suggests that CPA only partially inhibits SERCA in ASMC.

Introduction

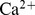is a ubiquitous cellular messenger, controlling a wide range of biological functions. These include ASMC contraction and proliferation, which are associated with airway hyper-responsiveness (enhanced contractility) and airway remodelling (structural changes) in asthma. The main trigger for cytoplasmic 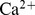(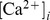) increase in ASMC is agonist stimulation at the cell membrane (e.g., by histamine released from mast cells or acethylcholine released from nerves). Binding of agonist to G-protein coupled receptors induces the production of 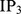, a second messenger which diffuses into the cytosol and binds to 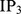receptor 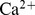channels (IPR) on the sarcoplasmic reticulum (SR) membrane (Fig. 1). This causes the IPR to open and release 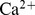from the SR into the cytosol (the SR being the main 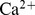store in ASMC). As 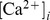exerts a positive feedback on IPR, this results in so-called 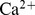-induced 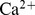release (CICR). The release is terminated by the inhibition of the IPR at large 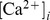, and 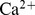is pumped back into the SR by 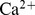ATP-ases (SERCA). Hence, for sufficient 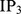concentration, cycling of 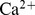through IPR can occur, and give rise to the repetitive propagation of 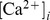waves through the cytosol. These appear as 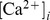oscillations at the whole-cell level. Importantly, airway contraction increases with the frequency of these 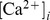oscillations [1], [2]. 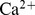dynamics are also involved in ASMC proliferation [3]–[5], and in the assembly of myosin thick filament and actin thin filament [6]–[8], which form the contractile machinery of ASMC. In addition, several 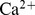channels and pumps in ASMC are regulated by inflammatory mediators present in asthma (e.g., [4], [9]–[12]). 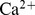dynamics therefore appear to be involved in multiple interrelated aspects of asthma at the cellular level. In the present work, we use mathematical modelling to investigate the important 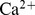pathways at play in 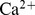dynamics of ASMC and thus improve our understanding of airway hyper-responsiveness and remodelling in asthma.

Figure 1. Schematic of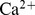 signalling in ASMC.

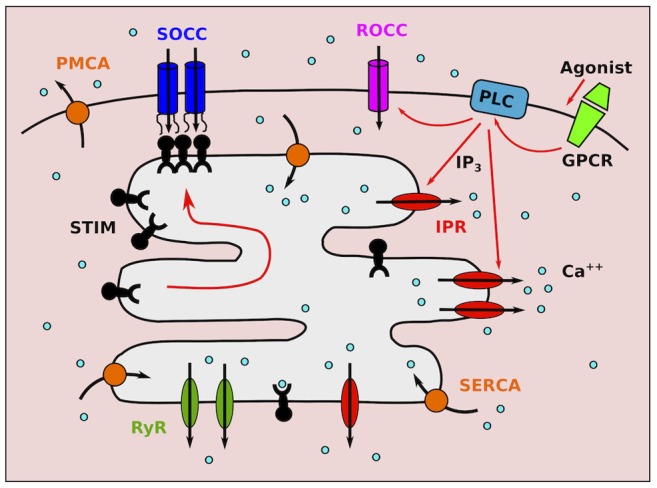
Agonist stimulation of G-protein coupled receptors (GPCR) induces PLC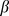 activation, giving rise to 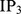production and 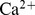entry through receptor-operated 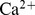channels (ROCC). 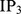triggers 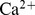release through IPR. Depletion of the SR from 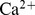causes STIM protein oligomerisation and migration toward the cell membrane, where they bind and activate store-operated 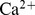channels (SOCC). 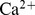ATP-ases pump 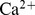back into the SR (SERCA) and out of the cell (PMCA).

Store-operated 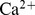entry (SOCE) is one important 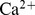entry mechanism, in which plasma membrane (PM) 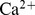channels open in response to 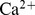store depletion. These are called store-operated 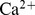channels (SOCC). Although the concept of SOCE was proposed 25 years ago [13], the mechanism of its activation has been identified only recently [14]. The process is mediated by stromal interaction molecules (STIM), proteins embedded in the SR membrane which are sensitive to SR 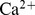. Upon dissociation of 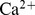from their SR binding site, they oligomerise and translocate within the SR membrane to the plasma membrane. Here, STIM proteins bind to Orai and/or TRP, the proteins forming the pore of SOCC, and trigger their opening (Fig. 1). Although SOCE has been identified in many cells, it is generally stimulated by artificial emptying of the 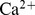store, as there is unfortunately no specific pharmacological SOCC blocker. Hence, the importance of store depletion, and therefore of SOCE, during physiological conditions such as 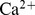oscillations, remains largely unknown. This may explain why SOCE has been included only in a few mathematical models of 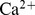dynamics [15]–[18]. In particular, no prior modelling work on 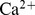dynamics in ASMC [19]–[23] has taken SOCE into account, even though there is evidence that SOCE is up-regulated by inflammatory mediators found in asthma (TNF- and IL-13) [9], [11], [24], and is associated with ASMC proliferation [3], [5].

In this paper, we develop a mathematical model to evaluate the importance of SOCE in 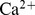dynamics of ASMC. While there is much evidence that SOCE occurs upon SR depletion in cultured ASMC in vitro (e.g., [3], [25]–[27]), these cultured cells often lose their contractile phenotype, and rarely display agonist-induced 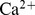oscillations. Hence, ASMC in lung slices, which retain most of their physiological and morphological characteristics, are a more reliable preparation to study ASMC 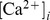dynamics. Moreover, the available data from lung slices reflect 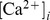dynamics in individual ASMC, while the majority of works with cultured cells provide only global imaging of 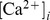over wells containing thousands of ASMC. Therefore, we base our model on data from lung slices. SOCE has not been studied directly in lung slices, but a treatment with ryanodine-caffeine (Rya-Caf) has previously been used to clamp the cytosolic 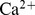of ASMC [2], [28], [29], which relies on emptying the SR from 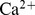. The results of these experiments therefore provide invaluable information about SOCE. Because agonist stimulation was systematically performed before Rya-Caf treatment to ensure that the lung slice is viable, i.e., that ASMC exhibit normal 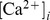oscillations and contraction, we can construct a mathematical model of 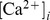dynamics informed by these data that accounts for both physiological and non-physiological conditions. The model is then used to i) evaluate the effect of SOCE up- and down-regulation on agonist-induced 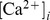oscillations, and (ii) explain the inability of the SERCA pump blocker CPA to clamp the 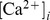, in contrast with Rya-Caf treatment.

Methods

Ethics Statement

The experimental study followed the recommendations in the Guide for the Care and Use of Laboratory Animals of the National Institutes of Health. The protocol was approved by the Institutional Animal Care Committee of the University of Massachusetts Medical School (Docket Number: A-836–12). Animals were euthanized with sodium pentobarbital before tissue collection.

Experimental data

Data consist of fluorescence recordings of 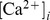dynamics in ASMC within intact lung slices. All the materials and methods have been previously described (e.g., [2], [28]). Essentially, 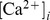imaging was performed from regions of about 4 within ASMC (Fig. 2), using two-photon laser scanning microscopy. The fluorescent indicator employed was Oregon Green BAPTA-1-AM, which has a high affinity for 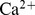(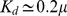M). We use published data [2] to develop the mathematical model, and new experimental results to test the model predictions (see Results). The latter data can be made freely available upon request for academic, non-commercial use.

Figure 2. Fluorescence image of part of a mouse airway wall obtained by two-photon laser scanning microscopy.

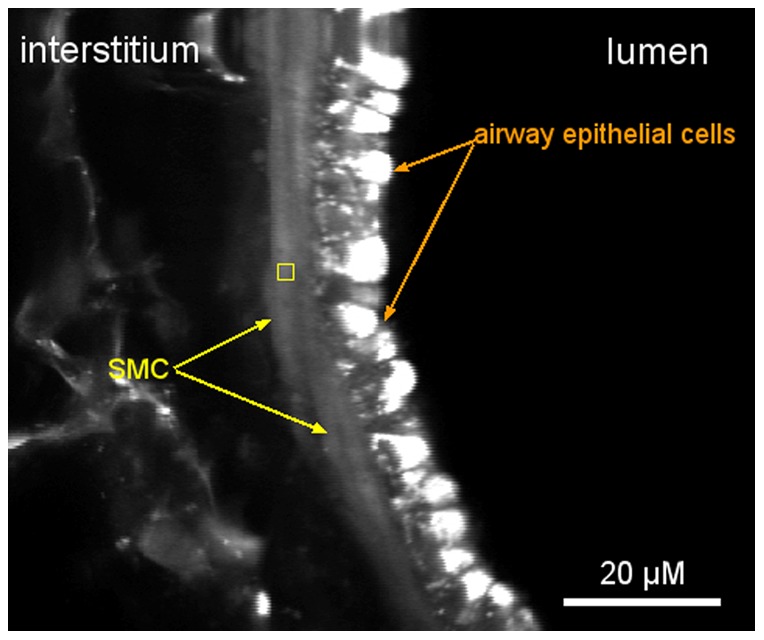
The yellow square shows a typical region, within an ASMC, from which 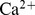dynamics is imaged.

Mathematical model

Intracellular dynamics are modelled at the whole-cell level, via the following system of ordinary differential equations (e.g., [30]):

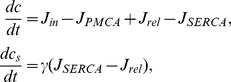(1)

where 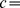is the free cytosolic 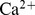concentration, and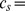 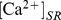is the free SR 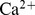concentration.

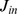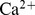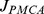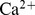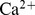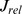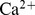The term represents the total influx of into the cytosol through PM channels; , the efflux through the PM ATP-ase pumps (PMCA); , the flux of 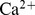from the SR into the cytosol, and 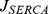, the flux of 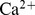from the cytosol into the SR through the SR/ER 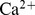ATP-ases (SERCA). The factor represents the ratio of cytoplasmic volume to SR volume, and implicitly incorporates the relative effect of fast, linear (e.g., low affinity) 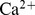buffers in the SR compared to the effect of similar buffers in the cytosol. Indeed, the effect of fast, linear buffers amounts to a global rescaling of the 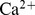fluxes in the corresponding compartment (e.g., [30]). The other buffers are assumed to have a negligible effect on 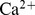dynamics at the whole-cell level (see also Discussion).

We assume that

(2)

where 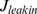is a constant 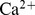leak through unspecified channels, 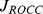is the 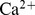influx through receptor-operated 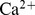channels (ROCC) and 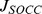the influx through SOCC. We neglect the 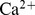influx through voltage-operated 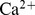channels (VOCC) because membrane depolarisation plays little role during agonist-induced 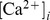signalling and contraction in ASMC (in contrast to other types of muscle cells, including vascular smooth muscle cells, where action potentials are crucial to contraction) [1], [31]. The 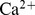influxes are modelled by:

(3a)

(3b)

(3c)

where 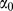and 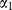are constants, 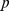is the agonist concentration, 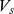is the maximum SOCC flux, and 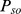represents the fraction of STIM proteins bound to Orai/TRP proteins, i.e. the fraction of activated SOCC. This fraction adapts slowly to changes in 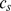, because the diffusion of STIM within the SR membrane is a slow process [32]. We model this phenomenologically by

(4a)

(4b)

The steady-state function 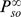can be interpreted as the fraction of STIM proteins dissociated from SR 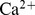(as a consequence of store depletion), and thus able to oligomerise and move toward the PM to bind with Orai and/or TRP (see also Discussion). 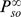is therefore a decreasing function of 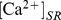, which we model by the reverse Hill function Eq. (0b), assuming affinity 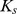for 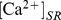and Hill coefficient 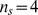[33].

The total 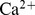flux from the SR into the cytosol is given by

(8)

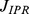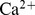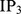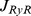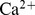where is the flux through receptors (IPR), the flux through ryanodine receptors (RyR), and 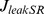an unspecified 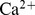leak out of the SR. We use the formulation (e.g., [30]):

(9)

where 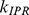(resp. 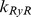) is the maximum rate of 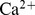flow through IPR (resp. RyR). Following [23], the IPR opening probability 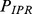is modelled using the Li-Rinzel/Tang et al. reduction of the De Young-Keizer (DYK) model [34]–[36]:

(10)

where 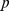is the 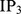concentration, and 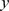is the fraction of inhibited IPR. The latter obeys

(11)

with

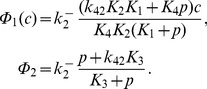(12)

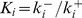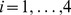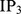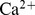The parameters () are equilibrium constants for and binding/unbinding to the IPR; we use the original values from the DYK model [34]. The value of 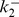is scaled so that the range of 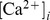oscillation frequencies matches the experimental range, with the ratio 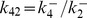kept constant to the value in ref. [30] (see also Table 1). In the experiments modelled in this work, either RyR play a negligible role, or they are locked open by Rya-Caf treatment (see Results). Hence, we neglect their dynamics and set the fraction of open RyR, 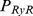, either to 0 or 1 depending on the experiment considered.

Table 1. Parameter values used in the model.

Parameter	symbol	value	units	reference
PMCA maximum flux	Vp	7.5	μM/s	this work
PMCA affinity	Kp	1.5	μM	0.1–1 [59]
SOCE maximum flux	Vs	1.57	μM/s	this work
STIM SR Ca2+ affinity	Ks	50	μM	c* s/2
SOCE Hill exponent	ns	4		[33]
SOCE timescale	τs	30	s	[32]
Constant leak influx	α0	0	μM/s	Vs
Cyt/SR vol. × buffer effects	γ	5.405		[34], [60]
ROCE rate	α1	0.00105	s−1	this work
SERCA maximum flux	Ve	5	μM/s	this work
SERCA affinity	Ke	0.1	μM	0.1–1 [61]
CPA effect timescale	τe	30	s	∼ min
IPR rate	kIPR	0.667	s−1	this work
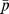
Agonist concentration		1	μM	this work
Agonist effect timescale	τp	30	s	∼ min
SR leak rate	JSR	0.01	s−1	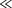kIPR
RyR leak rate (Rya-Caf effect)	KRYR	0.19	s−2	this work
Rya-Caf effect timescale	τSR	10	s	∼ min
IPR affinity for	K1	0.138	μM	[34]
IPR affinity for Ca2+ (inhib. site)	K2	1.05	μM	[34]
IPR affinity for IP3	K3	0.943	μM	[34]
IPR affinity for Ca2+ (inhib. site)	K4	0.144	μM	[34]
IPR affinity for Ca2+ (activ. site)	K5	0.082	μM	[34]
IPR Ca2+ dissoc. rate (inhib. site)	k−2	0.167	s−1	this work
IPR Ca2+ dissoc. rate (inhib. site)	k−4	0.138 k−2	s−1	[34]

For and , the equilibrium concentrations are nM and M, which are in the physiological ranges [40], [41].

The 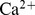ATP-ases are modelled using the usual expressions (e.g., [30]):

(13)

We do not model 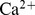pumping into mitochondria explicitly, but acknowledge that a portion of the extrusion process attributed to PMCA might actually be performed by mitochondria uniporters, as these might be activated at average 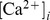as low as 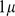M [20].

Gathering all expressions, the model is described by:

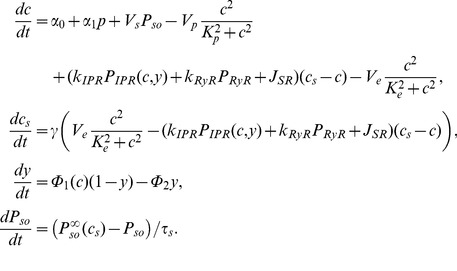(14)

In addition to Eq. (11), we use the following expressions to account for the time needed by drugs to reach full effect:

(15)

(16)

(17)

These equations describe respectively agonist stimulation, Rya-Caf treatment, and SERCA block by CPA (see Results).

Unless otherwise mentioned, parameter values were freely adapted (within physiological ranges when they are known) to account for the experimental results. The values retained are listed in Table 1. The fitting was performed “by hand” (i.e., no algorithmic method was used) within the Mathematica “Manipulate” environment (a useful framework for fitting an ODE model to several experimental results as it enables visualisation of the effect of a parameter change on several ODE integrations almost instantaneously). The code can be made freely available upon request for academic, non-commercial use.

All simulations were run from the same initial condition as in the experiment, which is usually the physiological equilibrium. Bifurcation diagrams were computed using the numerical continuation software AUTO [37], [38].

Results

Accounting for 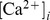dynamics of AMSC in lung slices

Fig. 3A-C shows representative 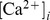dynamics of an ASMC in a human lung slice in response to a three-step experimental protocol [2]. This protocol was originally designed to clamp the 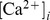of ASMC, in order to study independently the effects of agonist and 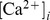on airway contraction [28]. The slice is first stimulated with agonist (histamine), to verify its viability (Fig. 3A). This induces 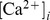oscillations. Agonist is then washed from the slice, and a Rya-Caf treatment is applied (Fig. 3B). This creates a permanent 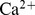leak through RyR, because caffeine opens RyR and ryanodine locks them open irreversibly. If this 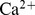leak is large enough, it keeps the SR empty and prevents any further change in 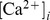, unless extracellular 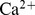is modified. The effectiveness of the treatment is confirmed by the second application of agonist (Fig. 3C): no further 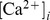increase is triggered, showing that 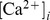is clamped. It is important to emphasise that these results are not specific to histamine stimulation of human lung slices: similar results have been obtained in mouse and rat lung slices with methylcholine (Fig. 6 in ref. [29], Figs. 5B and 6C-D in ref. [28]).

Figure 3. 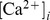dynamics in ASMC: experiment and model.

(A)–(C): Fluorescence imaging of 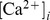dynamics in an ASMC within a human lung slice, during the following 3-step experiment: (A) Agonist stimulation, (B) Rya-Caf treatment, and (C) second agonist stimulation. Following the irreversible Rya-Caf treatment in (B), agonist stimulation (C) is no longer able to elicit 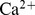oscillations, nor does it perturb the new elevated 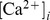equilibrium. Reprinted from [2] under a CC BY license, with permission of the American Thoracic Society, original copyright 2010. Cite: Ressmeyer et al. /2010/Am J Respir Cell Mol Biol/43/179–191. Official journal of the American Thoracic Society. This modified figure is based on the original figure available from www.atsjournals.org. (D)–(F): Simulations of the experiments in (A)–(C) using Eqs. 114–12 and the parameter values in Table 1. The evolution of 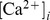, , 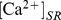and 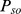(fraction of open SOCC) are shown (cf. legend in (E)).

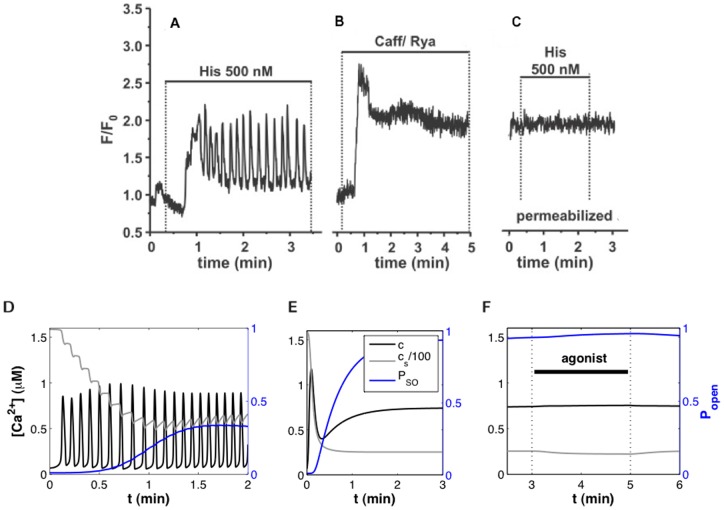
Figure 6. Effect of CPA on 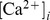dynamics.

(A) Fluorescence imaging of 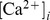in ASMC of a mouse lung slice treated with agonist and CPA. Agonist removal leads to 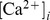decrease. (B–D) Model simulations of the experiments shown in (A), assuming that (B) CPA quickly blocks the SERCA, (C) CPA slowly blocks the SERCA, (D) CPA partially blocks the SERCA but reaches maximum strength rather quickly. Black solid and dashed curves (left y-axis) represent respectively 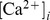and 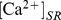; blue and red curves (right y-axis) show respectively the fraction of open SOCC and the fraction of operating SERCA (that is, 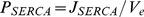, where 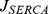is given by Eq. (0c)).

Figure 5. Influence of SOCE on agonist-induced oscillations.

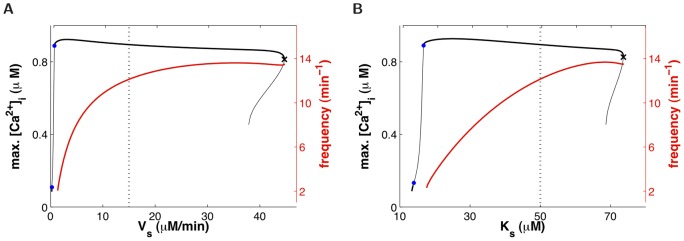
Amplitude (black) and frequency (red) of 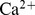oscillations as a function of (A) SOCE maximum rate, 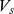, and (B) STIM affinity for SR 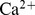, 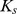. Dotted lines indicate the “normal” parameter values (Table 1, Figs. 3D–F). As in Fig. 4, only the frequency of the large-amplitude stable 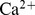oscillations is shown.

The mathematical model enables the deduction of valuable information from the experimental results. First, from Eq. (14), the new, elevated, 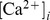equilbrium reached after Rya-Caf treatment satisfies:

(13a)

(13b)

where 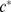and 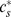are respectively the equilibrium 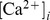and 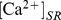. An important consequence of (18) is that, in the absence of SOCE, 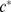depends only on the 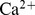fluxes through the PM. This may seem surprising, as any increase in 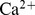flux out of the SR ( 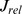in Eq. (1)) is expected to increase 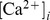. However, the equilibrium equation (18) tells us that such an increase would only be transient (because the PMCA pumping rate is an increasing function of 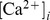), unless there is a concomitant permanent increase in 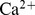influx through the PM. Hence, the persistence of an elevated 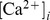means that a permanent SOCE has been elicited (as SOCE is the only 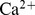influx capable of increase upon Rya-Caf treatment). Moreover, the model indicates that ROCE is negligible after Rya-Caf treatment. Indeed, if it was not, the addition of agonist would increase 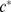via the increase in 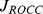. Hence, we assume that the ROCE rate 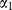is small (see Table 1 and Discussion).

Results of “hand-fitting” the model to the experimental results are shown in Figs. 3D–F and Fig. 4, with the corresponding parameter values listed in Table 1. The model reproduces (i) the agonist-induced 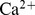oscillations, (ii) the similar magnitudes of the new equilibrium 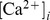in Fig. 3B and the amplitude of the oscillations in Fig. 3A, and (iii) the negligible effect of agonist stimulation after Rya-Caf treatment. Agonist-induced 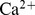oscillations were simulated with 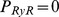because RyR appear to play a negligible role during agonist-induced 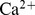oscillations [2], [39]. On the other hand, the response to Rya-Caf was simulated with 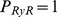since the treatment locks open the RyR. We did not attempt to reproduce the magnitude of the initial spike response to Rya-Caf treatment relative to that of the subsequent 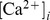plateau (Fig. 3B) because the fluorescent dye used in the experiments saturates rapidly with 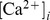. Parameter values were also adjusted to yield physiological 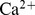equilibrium concentrations (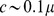M [40] and 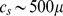M [41]), realistic 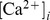oscillation amplitude (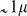M), and to reproduce the range of 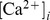oscillation frequencies observed in human lung slices as a function of agonist (0.5–11/min [2]). More detail on the parameter estimation procedure is given in Supporting Information S1.

Figure 4. 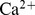dynamics as a function of agonist concentration.

Dashed curves represent steady-states (constant levels); solid curves, periodic solutions ( oscillations). The maximum 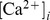(black) and the maximum fraction of open SOCC (blue) during one solution period are plotted as ordinates. The red curve (right y-axis) shows the frequency of the 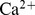oscillations on the main stable segment (from the upper blue dot to the black cross), which fits the experimental range in human [2]. The stable solutions are represented as thick lines and unstable solutions as thin lines. The green diamonds represent Hopf bifurcations, the black cross, a saddle-node bifurcation, and the blue dots, period-doubling points. Period-doubled branches are not shown because they extend only over a tiny range of 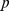values; moreover it is likely that the deterministic description of 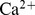oscillations fails at these low agonist concentrations (see Discussion). The vertical dotted line indicates the value of 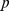used in Fig. 3 (Table 1).

Fig. 4 shows the bifurcation diagram of the model as a function of agonist concentration. Periodic solutions (i.e., 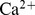oscillations) arise through a Hopf bifurcation, and disappear through a saddle-node bifurcation of limit cycles. A second Hopf bifurcation is present on the steady-state branch, and is associated with a region of bistability between the steady-state and the periodic solution at the right of the bifurcation diagram. It is not known whether such bistability occurs in reality. It should also be noted that the steady-state 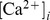increases with agonist concentration, as is expected (e.g., [30]). This increase is provided by SOCE in our model. Indeed, the 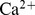flux through IPR increases with agonist, so that store depletion increases as well.

Effect of SOCE regulation on agonist-induced 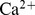oscillations

SOCE is the main 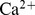influx in the model, as ROCE is negligible (see above) and the 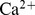leak influx is (by definition) small. Fig. 3D shows that while SOCE is almost zero at physiological equilibrium (initial condition), it substantially increases during agonist-induced 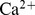oscillations (final condition; see also Fig. 4), due to significant SR 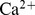depletion. Therefore, changes in SOCE can be expected to have a substantial effect on 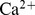oscillations. This is quantified in Fig. 5, where the amplitude and frequency of 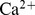oscillations are plotted as a function of (a) the maximum SOCE rate, 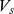, and (b) STIM affinity for 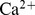, 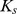(the 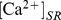at which half SOCC are open). It is found that the 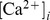oscillation frequency varies as much with 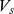and 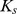at fixed agonist concentration (Fig. 5) as it varies with agonist concentration at fixed SOCE parameters (Fig. 4). Moreover, a too big departure from the “normal” values (dotted lines, Table 1) leads to the extinction of the 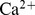oscillations (via a Hopf bifurcation to the left, and a saddle-node to the right, of the bifurcation diagrams in Figs. 5A–B). These results are not very surprising to the extent that 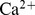oscillations are expected to depend crucially on 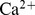influx (e.g., [42]). However, they suggest that SOCE could play a role in AHR since (i) 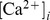oscillations mediate ASMC contraction, and (ii) SOCE up-regulation (which increases 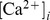oscillation frequency) can be triggered by inflammatory mediators commonly found in asthma [9], [11], [24].

Partial inhibition of SERCA by CPA

We now apply the model to experimental data from mouse lung slices showing an attempt to clamp 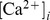with the SERCA blocker CPA, instead of Rya-Caf treatment (Fig. 6). After inducing 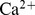oscillations with agonist, CPA is applied in the presence of agonist (for faster emptying of the SR than CPA alone) and causes a gradual damping of the 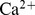oscillations, together with a rise of the 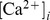baseline, until the oscillations become undistinguishable from fluctuations around an elevated steady 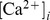mean. Because CPA is believed to inhibit SERCA, the assumption, at this stage of the experiment, is that the SR is empty and SOCE fully active. However, when agonist is removed (CPA remains), 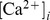falls. When agonist is reapplied, 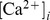increases. These 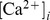responses to agonist addition and removal are not observed when SOCE is evoked by Rya-Caf treatment. According to our model (Eq.(18)), the decrease in 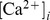upon agonist removal indicates that SOCE does not remain activated, i.e. that the SR refills with 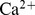. This suggests that the SERCA are not completely blocked by CPA, as illustrated by the simulations in Fig. 6B–D. If CPA was to fully block the SERCA (Fig. 6B), 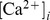would not decrease upon agonist removal. If 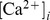falls, it must be because either CPA requires a longer time than that used in the experiment to fully block the SERCA (Fig. 6C), or CPA achieves only partial block of the SERCA (Fig. 6D).

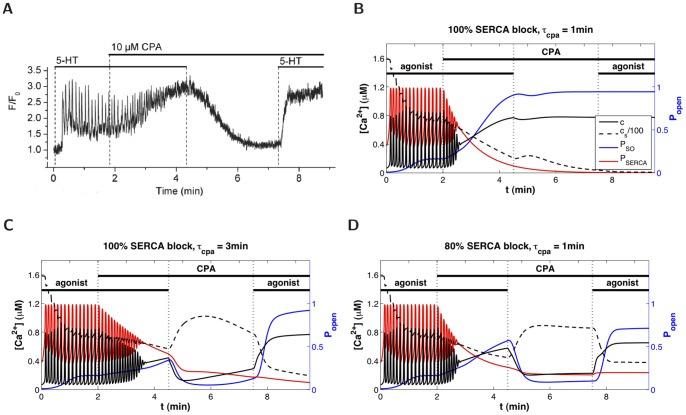
Experiments of longer duration were performed to test the model predictions. Fig. 7A shows that if CPA is applied in the presence of agonist for 5 minutes, followed by CPA only for a further 10 minutes, 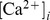still returns to the original equilibrium level when agonist is removed, and remains low until agonist is reintroduced. This suggests that the explanation in Fig. 6C can be rejected, otherwise the longer exposure to CPA should yield a result similar to Fig. 6B. The inability of CPA to fully empty the SR of 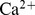is confirmed by Fig. 7B, where extracellular calcium is removed before agonist is applied a second time, to prevent any potential ROCE. The 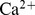response induced can thus be unambiguously attributed to 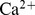release from the SR.

Figure 7. Experimental evidence that CPA does not fully empty the SR of ASMC.

Tests of the model predictions shown in Fig. 6B–D, performed with mouse lung slices. (A) Significantly longer exposure to agonist+CPA and to CPA than in Fig. 6A still fails to maintain SOCE. (B) Same experiment as in (A) except that extracellular 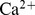is removed before agonist is applied a second time, confirming the residual presence of 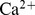in the SR and hence the partial efficacy of CPA to inhibit SERCA (scenario of Fig. 6D). (Insets show magnifications of selected time windows).

Hence, our combined modelling and experimental study indicates that CPA blocks only partially the SERCA of ASMC in lung slices (scenario simulated in Fig. 6D). This is a potentially important result given the wide use of CPA in cell biology to study SOCE. We note that Figs. 6A and 7 could also be explained by a model assuming that ROCE, instead of SOCE, is the main 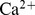influx (e.g., [23]). However, such a model would fail to explain the outcome of Rya-Caf treatment in human and mouse lung slices (both the persistent elevated 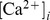in the absence of agonist, and the absence of effect of agonist on this elevated 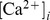). In contrast, our model, constructed to account for both agonist-induced oscillations and Rya-Caf treatment, explains the CPA results without requiring any modification. Its prediction holds provided CPA is not a 100% efficient SERCA blocker, and this hypothesis is supported by the experimental data in Fig. 7.

Discussion

Modelling SOCE

Our mathematical model accounts for the two main properties of SOCE: 1) SOCE is an increasing function of 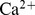store depletion, and 2) it activates slowly upon store depletion. While the mechanisms of SOCE activation are rather well understood [14], [32], the mechanisms of SOCE termination remain less clear [43], [44]. Hence, we do not explicitly distinguish between SOCE activation and inactivation in the model, and use a single parameter 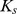for STIM affinity for SR 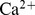and a single time constant 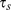for the slow adaptation to changes in 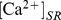. This is also justified by the fact that most experimental data available on SOCE come from a category of SOCC called CRACC ( 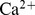-release-activated 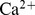channels), which are highly selective to 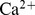, while there is evidence that SOCE in ASMC (and in other cells) occurs at least in part through non-selective 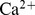channels (NSCC). It could be that the latter operate somewhat differently from CRACC in response to store depletion or refilling.

Our description of SOCE slow activation upon store depletion is continuous, which is easy to handle computationally, and compatible with experimental knowledge. Indeed, it is reasonable to assume that a small fraction of STIM proteins reside in close proximity to the PM, and may thus bind Orai quickly upon store depletion. Hence, a weak SOCE is likely to occur almost instantaneously upon store depletion, rendering unnecessary to introduce a finite activation delay in the model via a delay-differential equation.

We are aware of only few prior works on 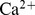dynamics that include a mathematical description of SOCE, all of which are ODE models [15]–[18]. The first two were published before the molecular basis for SOCE was established. The latter two works include more realistic descriptions of SOCE, but none of them accounts for the slow translocation of oligomerised STIM to the PM, while it is recognised as the rate-limiting event for SOCE activation [32]. Ong et al. however assume a slow diffusion of 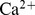between internal SR and superficial SR (modelled as distinct compartments exchanging 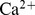), with SOCE being triggered by peripheral SR depletion [17]. Liu et al. explicitly model both SR 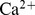dissociation from STIM and binding of STIM to Orai. Both models are used to study transient 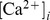responses only; 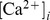oscillations are not considered. Prior models of 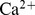dynamics specific to ASMC did not include SOCE, while we have shown that this is necessary to account for several experimental results obtained with lung slices. The work of Haberichter et al. [19] focused on the influence of the different IPR isoforms on 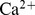signalling in ASMC. Brumen et al. studied the influence of the total 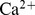content on the nature (damped or sustained) and frequency of agonist-induced 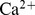oscillations [21]. Roux et al. did not model 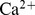oscillations, but transient 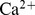responses to caffeine [20]. Finally, the model by Wang et al. [23] addressed the different contributions of IPR and RyR to agonist-induced and KCl-induced 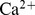oscillations in ASMC.

From the mathematical point of view, the fact that SOCE is an explicit function of store 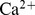renders the models of 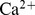dynamics including this influx qualitatively different from those which do not, as SOCE couples the homogenous steady-state 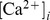to 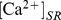(Eq. (18)). This property is essential for the predictions of our model (in particular, the persistence of an elevated 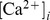upon sustained store depletion in the absence of agonist). On the other hand, whether SOCE is an instantaneous or delayed function of 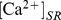appears to have little effect on our results.

SOCE vs. ROCE

While Fig. 3C (as well as Fig. 6 in ref. [29], Figs. 5B and 6C-D in ref. [28]) shows that no ROCE is elicited by agonist following Rya-Caf treatment, it does not imply that ROCE cannot play a substantial role during other, more physiological, conditions, such as agonist-induced 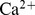oscillations. It could be that ROCE is inhibited at the large 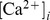levels induced by SOCE activation following Rya-Caf treatment. Instead of assuming the existence of an inactivation process at large 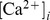, we assumed, for simplicity, that ROCE is negligible in the model. This approach enabled us to show that 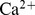influx through SOCC is sufficient to sustain agonist-induced 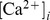oscillations, and to explain the experimental results obtained with CPA, although the latter could be interpreted as evidence for ROCE at first sight. The fact that there appears to be no selective blocker for SOCE and ROCE makes it difficult to evaluate experimentally the respective contributions of the two 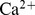influxes during physiological conditions. These magnitudes are probably also cell-type dependent. Such issues explain the persistence of the controversy regarding SOCE and ROCE [45]–[48]. An informative experiment would be to stimulate ASMC using flash photolysis of caged 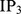instead of agonist stimulation. Indeed, as 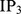does not induce ROCE, SOCE should be the essential 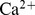influx left. By comparing the responses to 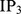stimulation in the presence and in the absence of extracellular calcium, one could then deduce the importance of SOCE in physiological conditions.

Efficacy of CPA

CPA is widely used as a SERCA blocker, having the advantage over Thapsigargin (Tg) of being reversible, and probably less toxic. Both have been used extensively to study SOCE in different cell types (e.g., [25]–[27], [43], [49]). Although our work indicates that CPA does not fully block the SERCA in intact tissue such as lung slices, it does not imply that CPA should not be used experimentally to induce SOCE. Indeed, CPA might still cause substantial SOCE activation in the presence of agonist. However, our results indicate that CPA is not a good mean to fully empty 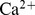stores, and care should be taken in interpreting the experimental results of its application. We suggest that a combined Rya-Caf treatment is a more reliable way to induce a permanent large SR depletion (Fig. 3B, C). There is evidence that Tg is an efficient SERCA blocker in cell lines such as Hela cells [43], but we have not addressed the effect of Tg on ASMC in lung slices in this study.

Modelling IPR

In this work, we followed the approach of Wang et al. [23], in that we have used one of the simplest models of IPR 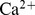release, namely the Li-Rinzel/Tang et al. reduction of the DYK ODE model [34]–[36]. This category of IPR model produces agonist-induced 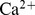oscillations characterised by significant SR 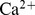depletion (Fig. 3D and [23]), hence the possibility of SOCE being activated during such 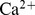oscillations. This property might be model-dependent, however there is evidence that the SR is actually depleted to some extent during agonist-induced 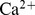oscillations in ASMC. Indeed, the absence of effect of ryanodine during agonist-induced oscillations can be explained by the average level of 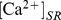being too low for RyR activation [1], [23]. However, the respective 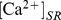“thresholds” for SOCE and RyR activation are experimentally unknown. In this work, the SOCE activation threshold was deduced from fitting the model simultaneously to Fig. 3A and Figs. 3B–C.

Finally, we note that our whole-cell 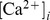model would likely not benefit from using a recent Markov model of an IPR (e.g., [50]–[52]), because these models are based on steady-state data only (i.e., single-channel opening and closing times in stationary 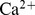and 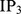) and typically miss the long inactivation timescale which was included “ad hoc” in the first IPR models to reproduce the observed behavior at the cell level (i.e., 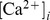oscillations upon agonist stimulation).

Limitations of the whole-cell model

As we are essentially interested in 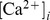responses of ASMC at the cell level, we have described 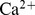dynamics via a deterministic ODE model. The scope of this model is, however, somewhat limited for the following reasons.

First, there is evidence that IPR are not homogeneously distributed on the SR membrane of cells, but are found as dense clusters. This channel clustering is especially patent upon stimulation by low agonist concentrations, for which local, stochastic 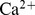releases may not propagate to neighboring clusters, resulting in spatially isolated, unsynchronised 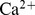releases, called “puffs”. At higher agonist concentrations, the frequency of these puffs increases, allowing 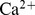releases from close sites to accumulate and propagate further away. This triggers, via CICR, the firing of more distant clusters, and results in 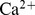waves propagating repeatedly throughout the cytosol. These waves usually appear as 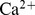oscillations at the whole-cell level. While 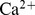waves are indeed associated with 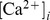oscillations in ASMC [1], it has, so far, been impossible to detect 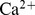puffs. This could arise from a less clustered distribution of IPR in ASMC, compared to the larger cells (ooycytes and Hela cells) where puffs have been characterised. On the other hand, 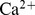“sparks”, the equivalent of 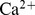puffs but mediated by RyR, have been detected in ASMC [1], which supports a clustered distribution of RyR. In this study, we did not attempt to consider these spatial/stochastic aspects of the 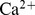signals. Our model is thus less reliable at low agonist concentrations.

Second, cytoplasmic microdomains often exist between cell organelles (e.g., between peripheral SR and the plasma membrane, between the SR and mitochondria), out of which 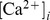cannot diffuse easily. These have consequences for SOCE dynamics. Indeed, it has been reported that upon store depletion, SERCA can colocalise with STIM proteins, in proximity to the PM [49], [53]. As a consequence, if SOCE is slow enough, the SR can refill with 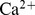without a concomitant increase in bulk 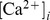[49]. Upon large SOCE, this is no longer the case; however, mitochondria prevent the local 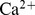increase to become too large by pumping 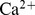from the subplasmalemmal space and releasing it deeper in the cytoplasm, where it can be absorbed by other SERCA [49]. These spatial effects cannot be accounted for by our current non-compartmentalised model.

Finally, 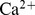dynamics are modified by 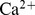buffers in the cytosol and SR, which bind 99% of the free 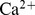. While the effect of fast, linear buffers can be taken into account by a global rescaling of 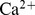fluxes (see Methods), this is not the case for high affinity buffers, in particular fluorescent dye indicators. Including such buffers in an ODE model of 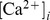dynamics leads to suppression of 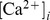oscillations, because the buffer affinity is close to the amplitude of whole-cell 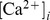oscillations. In reality, 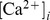reaches much higher levels locally upon IPR opening, so that the buffers become saturated and cannot prevent 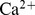oscillations. Again, this would have to be accounted for by a spatial model of 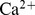dynamics.

Future work

Although RyR dynamics play a role only during the initial phases of agonist-induced 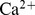oscillations and Rya-Caf treatment, the interaction between RyR and IPR may become important in other situations, such as drug-induced RyR sensitisation. We plan to extend our model to these dynamics.

Since our work is part of a broader effort to improve the understanding of airway hyper-responsiveness and remodelling via mathematical modelling [54]–[57], we also intent to model the interaction of ASMC 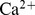signalling with other aspects of lung dynamics. Although mathematical models of ASM contraction have previously been developed [54], [55], [58], modelling of other signalling pathways, such as inflammation and proliferation, is, to our knowledge, still in its infancy.

Additionally, experimental studies of ASMC inflammation and proliferation in conjunction with 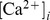imaging in lung slices would be desirable. While such studies have been carried out with cultured ASMC [3]–[5], [9]–[12], they do not provide individual 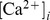dynamics; moreover, cultured ASMC often exhibit a different phenotype from ASMC in intact tissues.

Conclusions

The inclusion of SOCE in our mathematical model of 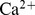dynamics in ASMC enables a better understanding of the experimental physiology of lung slices. It shows that the different abilities of CPA and Rya-Caf treatment to clamp the 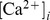of ASMC can be explained by their different ability to invoke SOCE. The model predicts that CPA, in contrast with Rya-Caf treatment, is unable to empty the SR because of its inefficiency to fully inhibit the SERCA. Furthermore, by accounting for both agonist-induced 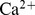oscillations and SOCE activation by SR 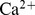depletion, the model shows that SOCE can be a major determinant of the frequency of agonist-induced 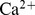oscillations. Because this frequency of the 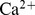oscillations regulates airway contraction, the model suggests a role for increased SOCE in AHR, a correlation consistent with SOCE up-regulation under inflammatory conditions typical of asthma. These predictions underscore the synergistic role for mathematical modeling in medical research.

Supporting Information

Supporting Information S1

Details of the parameter estimation procedure.

(PDF)

Funding Statement

BSB acknowledges funding from the Medical Research Council (http://www.mrc.ac.uk), New Investigator Grant: G0901174. MJS is supported by a grant from National Institutes of Health (http://www.nih.gov): HL103405. The funders had no role in study design, data collection and analysis, decision to publish, or preparation of the manuscript.