Three-dimensional distribution of polymorphs and magnesium in a calcified underwater attachment system by diffraction tomography

Abstract

Biological materials display complicated three-dimensional hierarchical structures. Determining these structures is essential in understanding the link between material design and properties. Herein, we show how diffraction tomography can be used to determine the relative placement of the calcium carbonate polymorphs calcite and aragonite in the highly mineralized holdfast system of the bivalve Anomia simplex. In addition to high fidelity and non-destructive mapping of polymorphs, we use detailed analysis of X-ray diffraction peak positions in reconstructed powder diffraction data to determine the local degree of Mg substitution in the calcite phase. These data show how diffraction tomography can provide detailed multi-length scale information on complex materials in general and of biomineralized tissues in particular.

1. Introduction

The performance of biological materials depends crucially on their hierarchical structure [1–3]. Understanding these structures is thus paramount to understanding how and why the materials work. Techniques used to probe these structures should preferably be able to probe the interior of the sample on several length scales without requiring destructive sample preparation. One of the few techniques fulfilling this requirement is micro-computed tomography (μCT). In standard μCT, three-dimensional images of the sample linear attenuation coefficients are reconstructed based on a series of projection images [4]. Higher contrast between materials with similar absorption power can be obtained by employing phase contrast, which is sensitive to the X-ray refractive index rather than only absorption [5,6]. Ptychography also provides high contrast refractive index maps [7]. However, none of these imaging modalities directly distinguish different crystalline materials with similar effective absorptivity and refractive index within a complex mixture. In CaCO₃ biominerals, for example, a mixture of the polymorphs aragonite and calcite are often observed in conjunction with an organic matrix. Until very recently, it has only been possible to map polymorph distributions by methods requiring destructive sample preparation such as position-resolved X-ray diffraction [8–10], confocal Raman scattering [10] or similar techniques.

Here, we employ an alternative tomographic technique to map polymorphs in a calcified biomineral structure. This is done using diffraction tomography that is based on collecting position-resolved diffraction data at many angular orientations of the specimen. This technique allows for reconstruction of the three-dimensional distribution of crystallographic phases [11–15]. This method is complementary to grain-resolved X-ray techniques, i.e. ‘high-energy diffraction microscopy’ [16] or ‘3DXRD’ [17,18], which require little or no diffraction spot overlap and thus are mainly applicable to larger (>μm) grained materials than those studied here. We show how diffraction tomography can be applied to highly complex CaCO₃ biomineral structures yielding not only maps of polymorphs but also of the local degree of Mg substitution, which can be determined from the local lattice constants because Mg substitution leads to known changes in the calcite lattice constants [19].

An important area where biological materials prove their mettle is in underwater adhesion with the mussel byssus [20,21], oysters [22] and barnacles [23] as prime examples. Contrary to the protein-based byssus of the Mytilidae [20,21], Anomia simplex uses a highly calcified (more than 90 wt%) byssus that extends through a notch in the right valve [24–30]. The byssus has a highly complex hierarchical structure [28,29,31], where both aragonite and calcite are present [24,26,28,29,31]. It consists of a lamellar part that forms the interface with the musculature of the animal and a porous part closer to the substrate as seen in figure 1. There is an array of large channels that extend roughly parallel to the substrate–byssus interface and branch out into many smaller pores that extend vertically down to the substrate, figure 1d. The large channels reach the substrate close to where the lamellar part reaches the substrate, figure 1b. Additional small perpendicular pores are also seen around edges of the byssus extending from the outer surface all the way down to the substrate [24,29], as can be seen in figure 1c.

Figure 1.

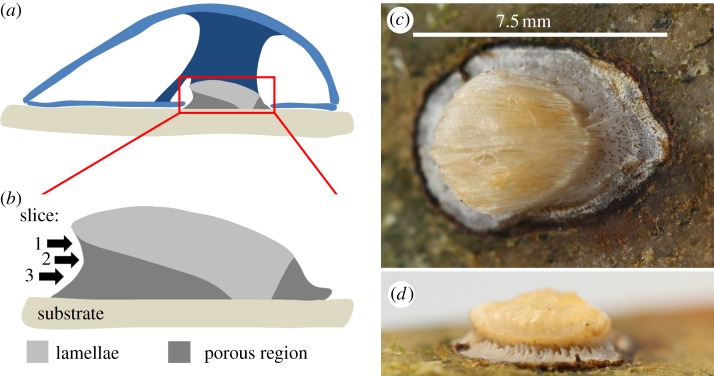
The Anomia byssus. (a,b) Sketches of a cross-section of the animal along its long axis. Schematic (a) shows the placement of the byssus in the animal. Light blue indicates the shell while the dark blue displays the byssus retractor muscle and the adductor muscle. Schematic (b) highlights the division of the byssus structure into a lamellar and a porous part and also indicates the positions of the three slices investigated by diffraction tomography in the present work. (c,d) Photographs of a byssus attached to a stone. Image (c) is recorded from above the substrate and shows the lamellar interface to the musculature. The pores extending from the top of the porous region to the substrate are visible as brown colorations on the surface of the porous part. (d) Byssus from behind towards the tip of the byssal notch. Channels approximately parallel to the substrate are seen extending into the byssus.

The lamellar part consists of sheets of organic material decorated with a herringbone arrangement of crystals [29]. The organic sheets penetrate into the musculature of the animal and maximize the contact area. The organic sheets also extend all the way through the byssus to the substrate, which highlights the structural importance of these sheets. Their role in the formation process of the byssus was studied by Yamaguchi in Anomia chinensis [24]. When the juvenile animal settles, the foot initially deposits a small lamellar organic plate that mineralizes during the juvenile stage of the animal (figure 2a). When the animal grows, the byssus also enlarges by addition of a larger lamellar pad above the previous byssus height within the animal. Near the substrate, this enlarged pad is supported by addition of the porous calcite (figure 2b). When the animal grows even more, an additional, even larger lamellar pad is added on top with corresponding calcitic support structures beneath (figure 2c). This leads to the complex morphology seen in figure 1.

Figure 2.

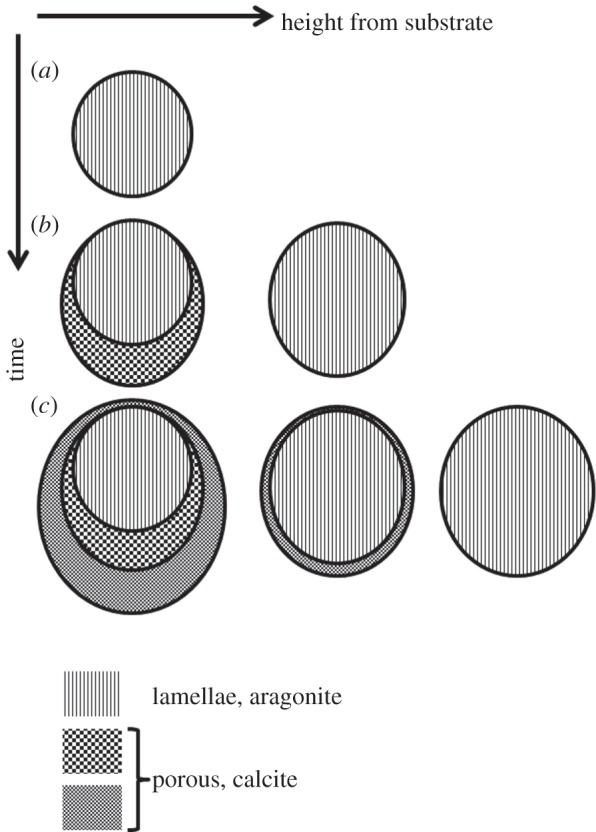
Sketch of the growth of Anomia byssi derived from the work of Yamaguchi [24]. Sketches of cross sections parallel to the substrate are shown at different stages of animal growth along the vertical axis and at different heights from the substrate along the horizontal axis. Vertically striated sections indicate aragonitic lamellae while the checkerboard patterns represent the calcitic porous part of the byssus.

2. Experimental

2.1. Animals

Live specimens of Anomia simplex were obtained from Woods Hole Marine Biological Laboratory (Woods Hole, MA, USA); they were sacrificed upon arrival by placing them in a −18°C freezer. The sample was extracted mechanically from an animal attached to a stone and briefly washed in 70 per cent ethanol. It measured about 9 × 6 mm² at the substrate interface and 7–8 × 4 mm² higher up, where diffraction tomography measurements were performed (see below).

2.2. Synchrotron X-ray absorption and diffraction tomography

Synchrotron radiation absorption and diffraction tomography data were measured at beamline 1-ID at the Advanced Photon Source (APS, Argonne National Laboratory, Argonne, IL, USA) using 70 keV X-rays and a 50 × 50 μm² beam. The sample was mounted so that the rotation axis of the goniometer was perpendicular to the substrate plane; hence, tomography slices were parallel to the substrate plane. For each angular orientation, diffraction data were collected at 160 positions with 50 μm intervals yielding an isotropic voxel size of 50 × 50 × 50 μm³ in the reconstructions. For each slice, 161 projections covering 0–360° in 2.25° intervals were measured. Diffraction patterns were detected using a General Electric Angio amorphous Si two-dimensional detector at a nominal sample-detector distance of 1118 mm, and calibrated to account for beam centre and tilting relative to the Debye ring using a CeO₂ standard and established procedures [11]. Sample absorption was measured by comparing the signal from a PIN diode mounted in the beamstop to that of an ion chamber before the specimen.

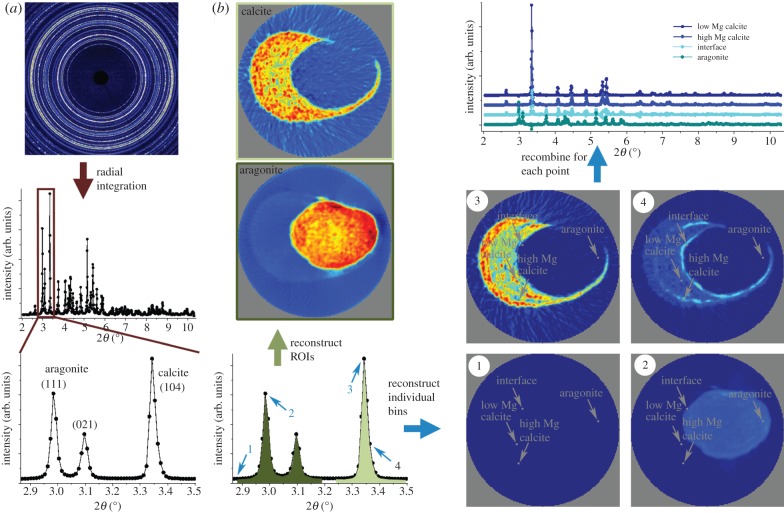
Two-dimensional diffraction patterns were obtained in a given spatial (y′, z′) and angular position (ϕ) of the sample as exemplified by the data shown in figure 3a. Small but systematic intensity variations around the Debye ring revealed a weak crystallographic texture. In the calibrated two-dimensional data, the intensity was a function of diffraction angle, position, sample angular position and azimuthal angle (2θ, y′, z′, ϕ, χ). The two-dimensional diffractograms were integrated over the full azimuthal angle (χ) to yield powder patterns depending on the position and sample angular position (2θ, y′, z′, ϕ) (figure 3a). By averaging over many orientations, this angular integration minimizes any effects of texture on the results obtained. Slices were reconstructed in two ways, both using a filtered backprojection. Software for azimuthal integration, tomographic reconstructions, data fitting and plotting was developed in-house in Matlab (MathWorks, Natick, MA)

Figure 3.

Schematic of the data treatment procedure used for the diffraction data; illustrated by using data from slice 2. (a) The raw data that have been radially integrated to give powder patterns. A zoom of the part of the powder pattern that is in focus in the rest of the analysis is shown in the lower image—this part of the powder pattern is shown again to the right in (b) highlighting the regions of interests (ROIs) used in the analysis. As a measure of the amount of the two polymorphs of CaCO₃ in the sample, the summed intensity across the aragonite (111) and (021) peaks (dark green ROI) and the calcite (104) peak (light green ROI) are used. These sums are reconstructed to give the polymorph maps shown at the top left of (b) and in figure 4 for all slices (the diameter of the field of view is 8 mm). Individual 2θ bins can also be used as ROIs and be reconstructed. A few examples of this (from the bins highlighted with blue arrows) are shown in the bottom right of (b). When all 2θ bins have been reconstructed, the intensity in each from one single voxel (x, y, z) can be recombined to give the entire diffractogram from this voxel. Examples from the voxels highlighted with the grey arrows are shown in the top of the right column in (b) and in figure 5.

In the first reconstruction approach, regions of interest (ROIs) in 2θ were defined. The intensities in the ROIs were integrated and reconstructed to yield maps of ROIs in real space (I(ROI(2θ), x, y, z)). Selecting two ROIs corresponding to the calcite (104) (light green in figure 3b) and the aragonite (111) and (021) (dark green in figure 3b) diffraction peaks results in maps of polymorph distributions as shown in figure 3b and the last two columns of figure 4. Note that the intensities in these maps are not on an absolute scale and hence not directly comparable for different ROIs. These reconstructions assume that the crystallites are randomly oriented within the material. If this is not fulfilled not all crystals will be equally ‘visible’ depending on their orientation with respect to the X-ray beam. Hence, a textured phase will give rise to variations in the apparent amount of this polymorph in the maps. This effect is especially obvious for the calcite phase, which displays a significantly larger degree of texture than the aragonite phase.

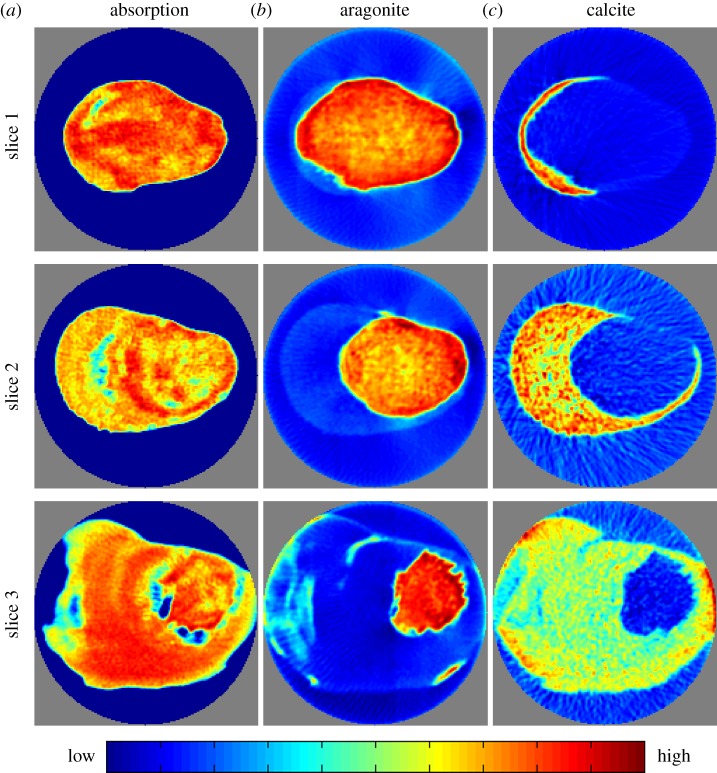
Figure 4.

Reconstructed slices based on X-ray absorption (a) and X-ray diffraction tomography (b,c). The diameter of the field of view is 8 mm. The latter show the distribution of aragonite and calcite, respectively. The slice numbers refer to the sketch in figure 1b. Grey voxels are the voxels outside of the field of view of the experiment.

In the second reconstruction strategy, fully reconstructed diffraction patterns were obtained by reconstructing each bin in 2θ space to yield fully reconstructed powder diffractograms in real space (I(2θ, x, y, z)). The procedure for this is sketched in figure 3b using blue arrows while selected diffractograms are shown in figure 3b and figure 5b,c.

Figure 5.

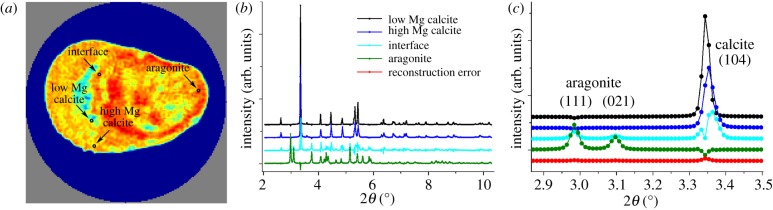
Reconstructed diffractograms from slice 2. Four one-dimensional diffractograms obtained in the voxels indicated in (a) are shown in (b,c) that show the full and a selected diffraction angle range, respectively. The image in (a) is the absorption-contrast reconstructed slice from figure 3.

It is worth commenting on the angular resolution of the diffractograms. The detector pixel size, 200 μm, leads to an angular resolution of 0.01° 2θ at the position of the calcite (104) peak, corresponding to a Δd/d of 0.003. However, since the sample is relatively large, motional averaging induced by rotation of the specimen during the measurements leads to additional broadening. Again referring to calcite (104), the maximal effect of this motional averaging comes from a contributing crystallite located at the sample surface. The present field of view of 8 mm then leads to a maximal motional averaging of 2.33 pixels. On average, the effect will be smaller. For the two main aragonite peaks, we obtain an average FWHM of ∼2.8 pixels. We note that the maximal motional averaging is smaller than the shifts used to determine the Mg substitution.

The local Mg content was obtained by analysis of the calcite (104) peak position from the reconstructed powder diffractograms. Mg substitution leads to a known change in the calcite lattice constants whereas Mg does not substitute into the aragonite lattice [19]. A 2θ-range 2.87–3.50° encompassing the calcite (104) and the aragonite (111) and (021) diffraction peaks was selected for analysis. The angular positions (d-spacings) of the aragonite diffraction peaks did not change with position within the sample. The calcite (104) peak, however, is shifted significantly as expected for the varying degree of Mg substitution, as seen from the patterns shown in figure 5c. These shifts are also observed when comparing different (ray integrated) diffractograms, and in powder diffractograms on pulverized byssi. In the latter case, a very clear shoulder toward high angles is observed [29,31]. Diffractograms reconstructed outside the sample should be featureless. However, due to reconstruction errors, they display non-zero values at the positions of the dominant diffraction peaks within the sample. Therefore, we used the average reconstructed diffractogram outside the sample (but within the field of view) as a measure of the precision of the data as a function of 2θ, i.e. their standard uncertainty (s.u.).

The major source of systematic error in the diffraction peak positions is imprecision in the determination of the average overall sample to detector distance. We compensated for this error by using the aragonite phase as an internal standard and assuming that its lattice parameters are the same as those found for biogenic aragonite from similar organisms. We calculated the expected peak positions of the aragonite (111) and (021) peaks from the lattice constants from biogenic aragonite from Bivalvia living in sea water [32]. Then an effective zero shift was determined by requiring that the observed peak positions in an average diffractogram of the entire sample matched the biogenic ones. This shift was then applied to the calcite peak positions; the zero point effectively corrects a sample to detector distance error because the high energy makes the diffraction peaks occur in a narrow range of small angles. The local Mg content was determined by weighted least squares against the distance-corrected data using the reconstruction errors as weights. As each voxel contains calcite with a range of Mg substitution, it was not possible to model the calcite (104) diffraction peak using a single peak and fitting its position. The distribution of Mg substitution was instead modelled using four Gaussian calcite (104) peaks with peak positions consistent with Mg contents of 0, 4, 8 and 12 mol% Mg, respectively. Their widths were constrained to be equal whereas their intensities were fitted independently. The effective Mg content was calculated from the relative intensities of the different Mg–calcite peaks. We have previously used this method on powdered byssus samples [31].

The s.u. of the Mg content was determined by error propagation of the s.u.'s of the intensities from the weighted least-squares fit. Besides the Mg–calcite (104) peaks, two Gaussian peaks fitting the aragonite (111) and (021) peaks and a background value were fitted.

3. Results

We performed diffraction tomography using high-energy X-rays (70 keV). These suffer little attenuation when traversing the specimen (up to 9 mm thick) and produce strong diffraction patterns; the measured transmission was in the range 28–45%. We collected and reconstructed data from three slices parallel to the byssus–substrate interface with isotropic voxel sizes of 50 μm as illustrated in figure 1 (see §2 for details). Both absorption and diffraction data were collected simultaneously. The first column of figure 4 displays the map of X-ray absorption in the three slices. Areas of low X-ray absorption correspond to pores and channels in the byssus. Besides these macroscopic features, the X-ray absorption images were almost featureless, at least at the current spatial resolution, and did not yield information on polymorph distribution as these have very similar linear attenuation coefficients.

The diffraction data on the other hand gave a wealth of information. The calcite and aragonite distributions were obtained by reconstructions using the azimuthally integrated intensities of selected isolated diffraction peaks. The resulting crystallographic phase maps are also shown in figure 4. It is immediately clear that the two polymorphs occupy distinct and disjoint parts of the byssus with aragonite being exclusively present in the lamellar part of the byssus while calcite makes up the porous part.

Full powder diffraction patterns could also be reconstructed for each voxel as shown in figure 5. Inspection of these reconstructed patterns (figure 5b,c) shows shifts in the calcite diffraction peaks at different positions within the byssus while the aragonite peaks stay at the same diffraction angle. It is well known that bio-calcites often are Mg substituted, and this is indeed also the case for the Anomia simplex byssus [29]. Mg substitution leads to a reduction in the calcite lattice constants thus resulting in a shift in diffraction peaks to higher 2θ angles. The peak shifts were used to create maps of the local degree of Mg substitution by fitting the calcite (104) peak (see §2 for details). The resulting maps and their associated errors are shown in figure 6 for the three slices through the byssus. The degree of Mg substitution thus determined was in most cases highly statistically significant, showing that the present diffraction tomography method is capable of yielding detailed insights into substitution chemistry in bio-calcite.

Figure 6.

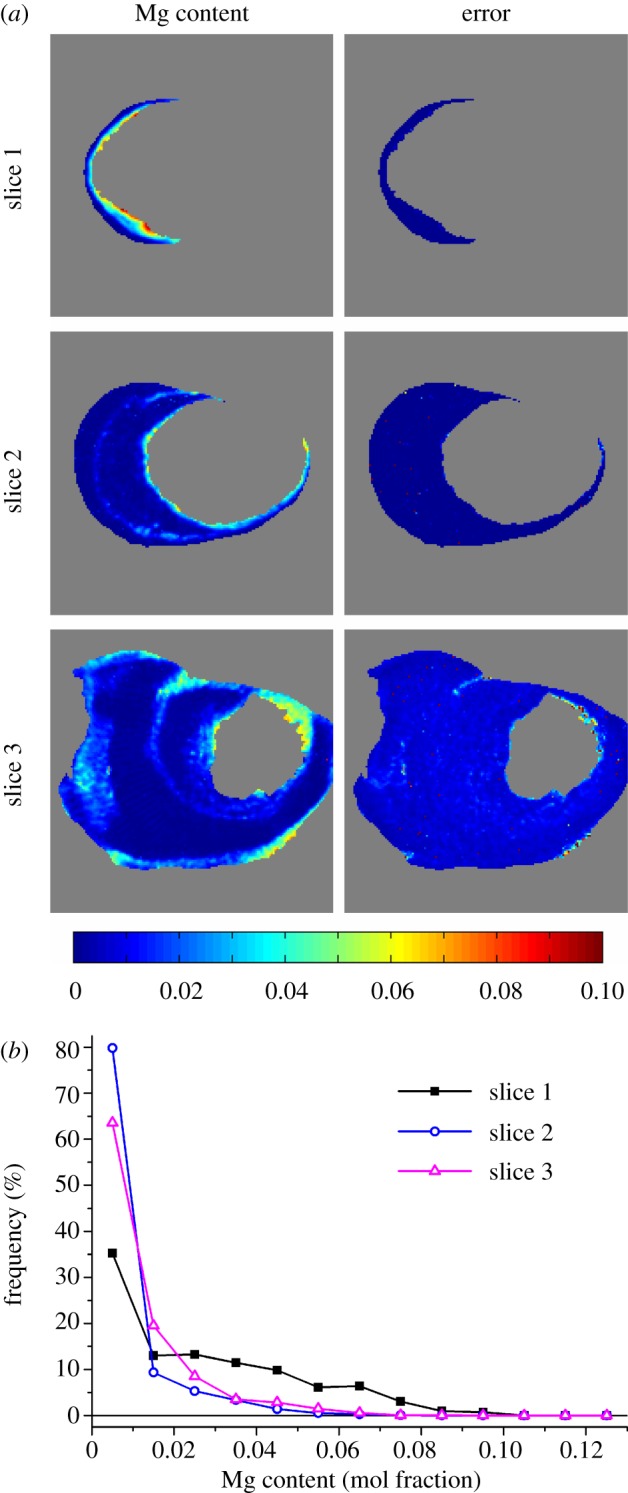
Magnesium substitution maps (a) determined by analysis of peak shifts of the calcite (104) peak in reconstructed diffractograms with the left column representing Mg content in mol% while the right column displays the associated standard uncertainty. Grey voxels are voxels where no calcite is present. (b) Mg content frequency distributions based on the maps in (a). The thin horizontal line represents zero frequency.

The Mg content in the byssus was found to vary from 0 to 10 per cent in the investigated sections. The experimental maps show that Mg substitution occurs in spatially distinct regions as was also previously observed by energy-dispersive X-ray spectroscopy (EDX) on polished sections [29]. Bands of higher Mg content are, for example, observed in calcite near the aragonite. Histograms of the Mg content for the three sections (figure 6b) show that the Mg contents of slices 2 and 3 are very similar, whereas slice 1 has significantly larger amounts of calcite containing a higher degree of Mg substitution. This is partially because of the small amount of calcite in slice 1 with only the high Mg-containing band at the interface to the aragonitic region, but also because the degree of Mg substitution in this slice is generally larger as compared with the others. The maximum degree of Mg substitution in slices 2 and 3 is approximately 6 per cent whereas it is 10 per cent in slice 1 corresponding to Ca_0.9Mg_0.1CO₃.

4. Discussion

The tomographic reconstruction of full diffractograms allows for extraction of the detailed information about the crystalline phases contained in these diffractograms. In the present case, this analysis determined the distribution of Mg substitution in biogenic calcite, but the methodology can easily be extended to other types of analyses.

We stress that the samples were not sectioned or cut in any way prior to measurements. Note also that it would not have been possible to determine the degree of Mg substitution without destructive preparation by other techniques such as EDX. Non-destructive synchrotron X-ray fluorescence tomography [33,34] would likewise have been impossible because the large size and high degree of mineralization of the sample would have led to complete absorption of emitted Mg X-ray photons, the Mg Kα attenuation length being only 1.5 μm for calcite. This effect would further be exacerbated by the high-air absorption of Mg X-rays that would require vacuum or helium environments around the sample [35].

The Mg distributions observed in the byssus may be caused by two distinct biological phenomena: an adaption of local structure to meet a mechanical demand or as a by product of byssus growth. Mg-substituted calcite is stiffer than Mg-free calcite [36], and such substitution may tune local mechanical properties to mechanical requirements. This has been suggested to be the case for sea urchin teeth that display very large, up to 40 per cent, degrees of Mg substitution.

Alternatively, Mg substitution can occur during calcite formation due to the presence of Mg in the precipitating medium. Sea water contains significant amounts of Mg. ‘Accidental’ substitution owing to direct precipitation of infiltrating sea water has been suggested to be the origin of Mg substitution in oyster cement that forms by crystallization of sea water in an organic matrix and displays degrees of Mg substitution of 7–10.4% [22] comparable to the ones observed in the high Mg regions of the Anomia byssus in the present work. The average Mg content determined in the present work is 1.13 per cent in the three slices but is much higher than the average near the interface with aragonite.

Recalling the byssus growth dynamics sketched in figure 2, it is clear from the data on slice 1 that when a new calcite portion is formed at a given height, Mg-rich calcite is deposited close to the lamellar/porous interface. We note that the micro-structure in this region is different from that further away from the interface in that the calcite crystal aggregates are smaller [29]. The higher Mg content close to the interface may result from rejection of Mg during aragonite precipitation and its incorporation into the calcite growing nearby or from control of the precipitating liquid. In either case, the Mg content falls off with distance from the interface. When a new growth process starts, a new line of high-Mg-content calcite would be put down as observed in slices 2 and 3. In this case, the Mg content would reflect the underlying animal growth dynamics. Under the reasonable assumption that animal growth is seasonal, the varying Mg content may additionally be a consequence of variations in average local water temperature. The Mg content in organic-matrix free precipitation of calcite also depends on the precipitation temperature. At low temperatures, calcite incorporates less Mg than at higher temperatures [37].

5. Conclusion

To summarize, we have used high-energy X-ray diffraction tomography to map the distribution of CaCO₃ polymorphs in the heavily calcified byssus of Anomia simplex. Because diffraction tomography allows for the reconstruction of full diffraction patterns, it was possible to determine the local degree of Mg substitution thus significantly enhancing the diffraction tomography methodology. All of this was achieved without cutting in the sample or destroying it in any way. Because of the very high X-ray energy used, the sample suffers minimal radiation damage and can hence be analysed using different techniques afterwards.

While the mechanism for the observed significant and systematic variations in the degree of Mg substitution remains unresolved, it clearly results in systematic variations in chemical composition and microstructure. The impact of these factors on byssus mechanical performance is under investigation.

Funding statement

S.R.S. acknowledges support from NICDR grant no. DE001374 (to Arthur Veis). Use of the Advanced Photon Source was supported by the US Department of Energy, Office of Science, Office of Basic Energy Sciences, under Contract no. DE-AC02-06CH11357.