Abstract
Microscopic MRI (μMRI) T1 experiments were carried out to investigate the strain dependency of the T1 change and glycosaminoglycans (GAG) quantification in articular cartilage at a spatial resolution of 17.6 μm. Both native and trypsin-degraded specimens were immersed in various concentrations of gadolinium (Gd) (up to 1 mM) and imaged at different strains (up to 50 % strains). Adjacent specimens were treated identically and analyzed biochemically by an Inductively Coupled Plasma Optical Emission Spectrometer (ICP-OES). The T1 profile in the native tissue was found to be both strain-dependent and depth-dependent, while there was no obvious depth-dependence in the degraded tissue. For the native tissue, compression reduced the tissue T1 when Gd in the solution was low (less than 0.4 mM) and increased the tissue T1 when Gd in the solution was high. A set of critical points, where the tissue T1 showed no change at a certain Gd concentration between two different loadings, was observed for the first time in the native tissue. It is concluded that the GAG quantification by MRI was accurate as long as the Gd concentration in the solution reached at least 0.2 mM (tissue not loaded) or 0.4 mM (tissue loaded).
Keywords: MRI, articular cartilage, dGEMRIC, strain, trypsin, GAG, ICP-OES, T1 relaxation
INTRODUCTION
The negatively charged glycosaminoglycans (GAG) in articular cartilage is responsible for the tissue stiffness; hence the reduction of GAG will weaken the mechanical properties of articular cartilage and can be regarded as an early sign of the tissue degradation (Maroudas et al 1969, Venn and Maroudas 1977). MRI relaxation measurements, which are sensitive to the motion of water molecules in tissue, have been used to detect the degradation of cartilage, including the use of T1 ρ (Duvvuri et al 2002, Li et al 2009, Nieminen et al 2002, Regatte et al 2002), T2 (Alhadlaq et al 2004, Dardzinski et al 1997, Gold et al 2004, Nieminen et al 2000, Xia 1998), and T1 with a gadolinium contrast agent (Bashir et al 1996, Bashir et al 1999, Nissi et al 2007, Taylor et al 2009, Tiderius et al 2003). In recent years, MRI has also been used to study the deformation of articular cartilage due to external compression (Alhadlaq and Xia 2004, Alhadlaq and Xia 2005, Juras et al 2009, Mayerhoefer et al 2010, Nishii et al 2010, Souza et al 2010, Xia et al 2011).
T1 in a native tissue is mostly uniform over the tissue depth (i.e., when comparing the shape of T1 with the shape of T2 in cartilage) and isotropic with respect to the tissue orientation (i.e., the same depth-dependent shape at both 0° and 55° (Xia 1998)), which are well documented in both high-resolution and clinical MRI studies (Hawezi et al 2011, Xia 1998). T1, however, can become sensitive to the GAG content in cartilage if a contrast agent (such as Gd(DTPA)2−) is administrated into the tissue (Bashir et al 1996). The paramagnetic gadolinium (Gd) ions are negatively charged and will distribute within articular cartilage in a spatially inversed relationship to the local GAG concentration, hence, influencing the tissue T1. In order to calculate a GAG image in cartilage quantitatively, two T1 maps are acquired before and after the Gd administration. The clinical version of this procedure has been termed as the dGEMRIC method in MRI literatures (Bashir et al 1996, Burstein et al 2001, Xia et al 2008, Zheng and Xia 2010).
The effect of loading on the tissue T1 by the dGEMRIC procedure was the subject of several recent MRI studies (Mayerhoefer et al 2010, Xia et al 2011). In one high-resolution study, both modest and heavy compressions increased T1 significantly when the tissue was soaked in the 1 mM Gd solution (Xia et al 2011). This trend of T1 increment in compressed tissue was, however, different from a human MRI study, where the mean T1 was found significantly decreased between the unloaded and loaded cartilage after the Gd administration (Mayerhoefer et al 2010). This inconsistency could be due to the complex nature of the significant depth-dependent properties in articular cartilage, which is originated by the depth-dependent collagen orientation in articular cartilage (i.e., SZ = the superficial zone, TZ = the transitional zone, RZ = the radial zone) (Xia et al 2001). Since the GAG concentration and the compressive modulus of articular cartilage are both depth-dependent (Chen et al 2001, Xia et al 2008), T1 in a compressed cartilage in the presence of gadolinium ions are both depth- and strain-dependent (Xia et al 2011).
The aim of this project was to resolve the above discussed inconsistency between two MRI studies of T1 in compressed cartilage (Mayerhoefer et al 2010, Xia et al 2011). Microscopic MRI (μMRI) was used to image both native and trypsin-degraded specimens that were immersed in various concentrations of gadolinium (up to 1 mM) and compressed at different strains (up to 50 % strains). The imaging resolution across the tissue depth was 17.6-μm. Although this microscopic resolution is currently not possible in clinical MRI, it is essential for the accurate determination of the depth-dependent changes over the thickness of articular cartilage. This is because each sub-tissue zone in articular cartilage has a different thickness; the first pixel in any low-resolution (clinical) MRI therefore likely contains both the superficial and transitional zones of cartilage. Hence, the detection of any early lesion (e.g., surface fibrillation, local GAG depletion) would be weakened or even masked by the volume averaging. In contrast, the high-resolution μMRI will be able to localize the focal defects of cartilage and study the progression of early/local diseases. Since μMRI shares the same physics principles and engineering architectures as in clinical MRI, the μMRI data from this type of high-resolution MRI studies could serve as the guideline for the future development of clinical MRI in cartilage lesion detection (Xia 2007).
METHODS
Specimen Preparation
Four humeral heads were harvested shortly (on three different occasions) after the sacrifice of four mature and healthy dogs that were used for an unrelated research, where the institutional review committee approved the animal handling. A total of twenty four cartilage specimens were harvested from the central load-bearing area (Xia et al 2008); each was about 3.5 × 2.5 × 6 mm. Eighteen specimens were divided equally into two groups (native, degraded) for μMRI, and the remaining six specimens were divided equally into two groups (native, degraded) for biochemical analysis. The identical MRI experiments were repeated three times; six samples were used in each time. Among the six samples, three specimens were immersed in physiological saline (154 mM NaCl in deionized water) with 1% protease inhibitor (Sigma, Missouri); the other three specimens were first degraded by soaking them in 10 μg/ml trypsin solution (Sigma, Missouri) for more than eight hours, then soaking in saline with 1% protease inhibitor to remove excess trypsin (Xia et al 2008, Xia et al 1995). The identical handling procedures and conditions were used for the two biochemical groups (native and degraded).
To ensure that the effect of compression was identical under different Gd concentrations, the tissue block was first compressed in a homemade, unconfined loading device (Alhadlaq and Xia 2004) and then soaked in the Gd solution. Each specimen was loaded under one particular strain: 0, 20, or 40% strain for native tissue, and 0, 28%, or 50% strain for degraded tissue respectively. The loaded specimens were then immersed in different concentrations of the Gd(DTPA)2− solution (Magnevist, Berlex, NJ) for at least six hours before the MRI experiments. The concentrations of the Gd solutions were 0, 0.05, 0.1, 0.2, 0.4, 0.7, and 1 mM. The volume of the soaking solution was at least 100 times the volume of the tissue to ensure a near constant concentration in the system.
MRI Methods
Microscopic MRI (μMRI) experiments were conducted at room temperature on a Bruker AVANCE II NMR spectrometer equipped with a 7-Tesla/89-mm magnet and micro-imaging accessory (Billerica, MA). The inversion-recovery T1 experiments followed the previously established protocols in μMRI (Xia et al 2008, Zheng and Xia 2010). Specifically, a homemade 5-mm coil was used for the imaging, where the orientation of the cartilage block with respect to B0 was set at 55° (the magic angle) to minimize the dipolar interaction to T2 (Xia 1998). The echo time (TE) of the imaging sequence was 7.2 ms; The number of dummy scans was two; the acquisition matrix was 256 × 128 (which image-reconstructed into the 256 × 256 matrix); the repetition time (TR) of the imaging experiment decreased steadily from 1.5 s without Gd to 0.5 s with 1 mM Gd immersion respectively; and the experiment time for each T1 experiment was about 2 – 4.5 h, depending upon the TR (which depended upon the Gd concentration). The 1-mm thick imaging slice was transversely located in the middle of the 6-mm long specimen. The 2D in-plane pixel size was 17.6 μm. The construction of 2D T1 images used the inversion-recovery pulse sequence with 5 inversion points (steadily reduced from 0, 0.4, 1.1, 2.2, 4.0 s for tissue without Gd to 0, 0.1, 0.3, 0.5, 1 s for tissue soaked in 1 mM Gd), which allowed the calculation of T1 relaxation by a single-component fitting procedure on a pixel-by-pixel basis. The total imaging time for any one sample was about 20 hours. The specimens were stored at 4°C when not used and imaged in the magnet at room temperature.
These T1 images enabled the calculation of the 2D GAG images in cartilage, based on the well documented Donnan equilibrium procedures (Bashir et al 1996). Since the relaxivity (the R-value) of the Gd ions in cartilage was a linear function of the solid concentration of the system (Stanisz and Henkelman 2000, Zheng and Xia 2010), a compressed tissue must have an increased solid concentration and, hence, an increased R-value. For this type of uncompressed and native cartilage, the R-value was found to be 5.77 (mM sec)−1 (~20% solid) (Zheng and Xia 2010). For the native cartilage with 20% and 40% strains, the R-values were determined to be 6.17 (mM sec)−1 and 6.84 (mM sec)−1 respectively. For the degraded tissues at three different strains, the R-values were 5.13 (mM sec)−1, 5.50 (mM sec)−1 and 6.09 (mM sec)−1 respectively, which corresponded to the solid concentrations of 12%, 17% and 24% respectively.
GAG Quantification using 23Na ICP-OES
Six specimens in the two biochemical groups were analyzed using a Perkin Elmer Inductively Coupled Plasma Optical Emission Spectrometer (ICP-OES) Optima 7000 DV (Waltham, MA), to determine the GAG concentration in tissue. To prepare for the ICP-OES experiments, the bone on each specimen was removed before weighing; the tissue was liquefied by concentrated 100 μl HNO3 overnight and subsequently diluted to 10 ml by deionized water for the 23Na ICP-OES analysis (Lesperance et al 1992, Shapiro et al 2000). In addition to the cartilage samples, three standard saline solutions (154 mM Na) were also analyzed for the calibration purpose. The calculation of the GAG in the ICP experiment followed the same the Donnan equilibrium equation (Bashir et al 1999, Zheng and Xia 2010), as the wet weight concentration with the unit of mg/ml, where an early work found an excellent agreement between MRI and ICP results (Zheng and Xia 2010). As a comparison, a conservation calculation based on the assumption that there should be little loss of GAG from the tissue to the solution during the specimen compression was also employed to calculate the GAG concentration of the compressed tissue according to the GAG concentration of the uncompressed tissue.
Division of the Histological Zones in Cartilage
Since the resolution of most MRI experiments could not reach tens of microns, as in this study, the trends of T1 as the functions of both tissue depth and mechanical strain were averaged over its sub-tissue zones (Chen et al 2001, Xia et al 2001). The division of the histological zones in the native cartilage was based on the μMRI and polarized light microscopy data of the uncompressed tissue (Xia et al 2001) and adapted from the confocal light microscopy data of the compression tissue by Chen et al (Chen et al 2001), as the following: at 0% strain: SZ = 8.0%, TZ = 16.0%, RZ = 76.0%; at 20% strain: SZ = 6.0%, TZ = 15.0%, RZ = 79.0%; and at 40% strain: SZ = 8.0%, TZ = 16.0%, RZ = 76.0%. To investigate the changes of relaxation time in the radial zone, RZ was further separated approximately equal to an upper part (RZ A) and a deeper part (RZ B). The division of the histological zones in all degraded tissues was: SZ = 8.0%, TZ = 16.0%, RZ = 76.0%, since there was no significant depth-dependence of T1 on strain or Gd concentration.
Image Analysis and Statistics
To facilitate the comparison among different T1 imaging results, the 1D profiles of cartilage over its entire depth were extracted from the 2D images. To enhance the signal-to-noise ratio of the 1D profile, 10 adjacent columns of the 1D profiles from a 2D image were averaged into one 1D profile (RMS average ± standard deviation) using KaleidaGraph (v4.0, Synergy Software, Reading, PA). Since the averaging occurred at every pixel depth, the depth resolution of the 1D profiles was still 17.6 μm. Kruskal-Walls test in KaleidaGraph was performed to compare the GAG concentration at different Gd(DTPA)2− concentrations. If the P-value is below 0.05, there is a significant difference among different groups.
RESULTS
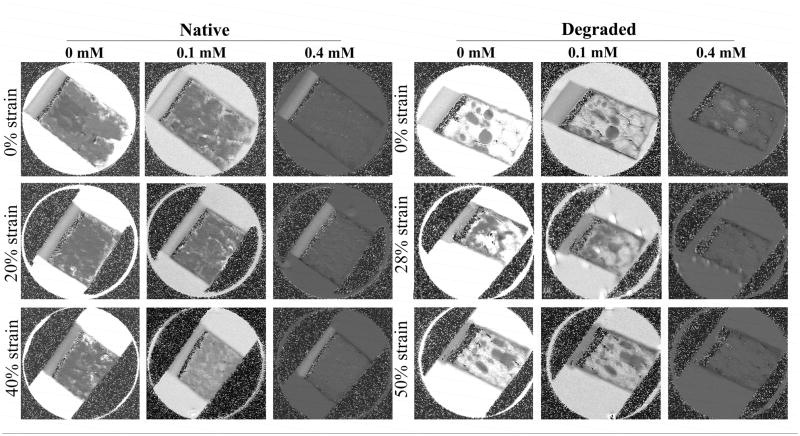
All six specimens in two imaging groups were imaged individually when the specimens were under different strains (0% strain (i.e., uncompressed), 20% ~ 28% strain (i.e., comparable to physiological loadings), 40% ~ 50% strain (i.e., sufficiently high to cause injury)). Since the tissue thickness was determined from the before- and after-compression images, the calculation of the strain was carried out after the imaging experiments and, therefore, highly accurate. Fig 1 showed the T1 images for the native (0% strain, ~ 20% strain, and ~ 40% strain) and degraded (0% strain, ~ 28% strain, and ~ 50% strain) specimens, both immersed in different concentrations of the Gd solution.
Fig 1.
The T1 maps of articular cartilage soaked in different concentrations of the Gd solution and under different strains. The min and max values in displaying all images were 0 and 2 s respectively. (The T1 images at higher concentrations (e.g., 0.7 mM and 1.0 mM) have the same features but darker in the image intensity when plotted at the same image display values.)
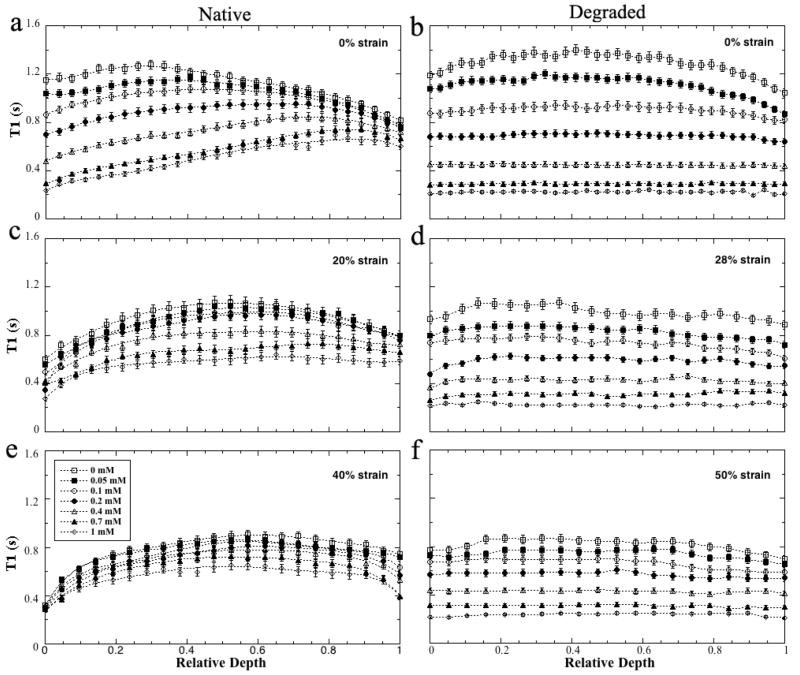
The depth-dependent profiles of all T1 images were plotted on the relative depth in Fig 2 (0 = articular surface, 1 = cartilage-bone interface). Several features were clearly visible in these T1 profiles. First, for any particular strain, T1 decreased steadily when the Gd concentration in the soaking solution increased; but the changes were smaller at higher strains (e.g., from 20% to 40%). Second, for the uncompressed native specimens (Fig 2a), T1 of the surface tissue (SZ and TZ) decreased more rapidly than in its deep tissue (RZ). The T1 of the surface tissue was higher than that of the deep tissue when the Gd concentration was less than 0.2 mM, but became lower than that of the deep tissue when the Gd concentration was above 0.4 mM. Third, T1 in the degraded tissue had no significant depth-dependency between any tissue zones (the right panel of Fig 2). The T1 of the soaking solutions in these quantitative images were 2.93±0.13 s, 1.60±0.04 s, 1.20±0.02 s, 0.77±0.02 s, 0.45±0.01 s, 0.27±0.01 s, and 0.20±0.01 s respectively for the seven concentrations of the Gd solution in an increasing concentration (averaging over a 4× 4 pixel area in the same location of all images).
Fig 2.
The T1 profiles of articular cartilage as the function of the Gd concentrations, plotted using the relative tissue depth (0 = articular surface, 1 = cartilage-bone interface). The native tissue at (a) 0% strain (not compressed), (c) 20% strain, (e) 40% strain. The degraded tissue at (b) 0% strain (not compressed), (d) 28% strain, (f) 50% strain. The error bars are the standard deviations.
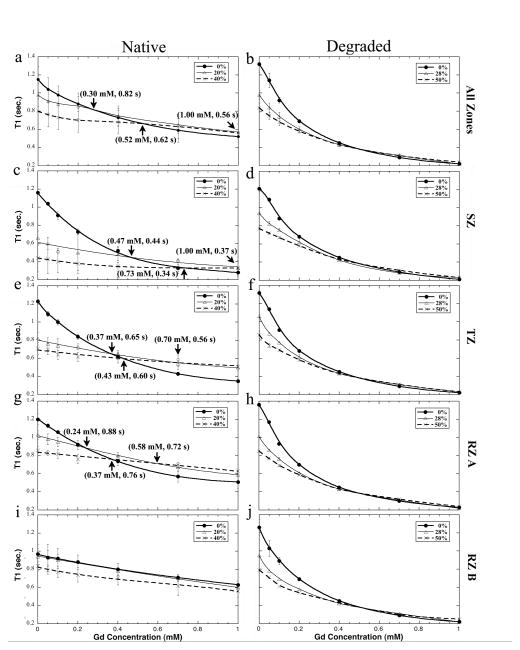
Fig 3 showed the averaged T1 in all zones using these division criteria. These zone-averaged T1 confirmed more clearly the previously stated three trends of T1 in cartilage under compression. A notable feature in the strain-dependent T1 plots (Fig 3) was the crossovers in native tissue (termed the critical points in this report, marked by the arrows). For the all-zone averaged native tissue (Fig 3a), the three T1 plots intersected at three particular Gd concentrations: at 0.30 mM between 0% and 20% strain, at 0.52 mM between 0% and 40% strain, and at 1.0 mM between 20% and 40% strain. In contrast, no critical point could be found for the degraded tissue (Fig 3b). Zonal-wise in the native tissue, as the depth became deeper from the surface (from Fig 3c, to Fig 3e, Fig 3g and Fig 3i), the locations of the critical points occurred at lower Gd concentrations, reflecting the non-uniform increment of GAG in the compressed tissue and, hence, the increasing difficulty of the tissue compression.
Fig 3.
The trends of the averaged T1 in the individual sub-tissue zones of the native (left) and degraded (right) cartilage as well as the whole tissue as the function of the Gd concentration in the soaking solution.
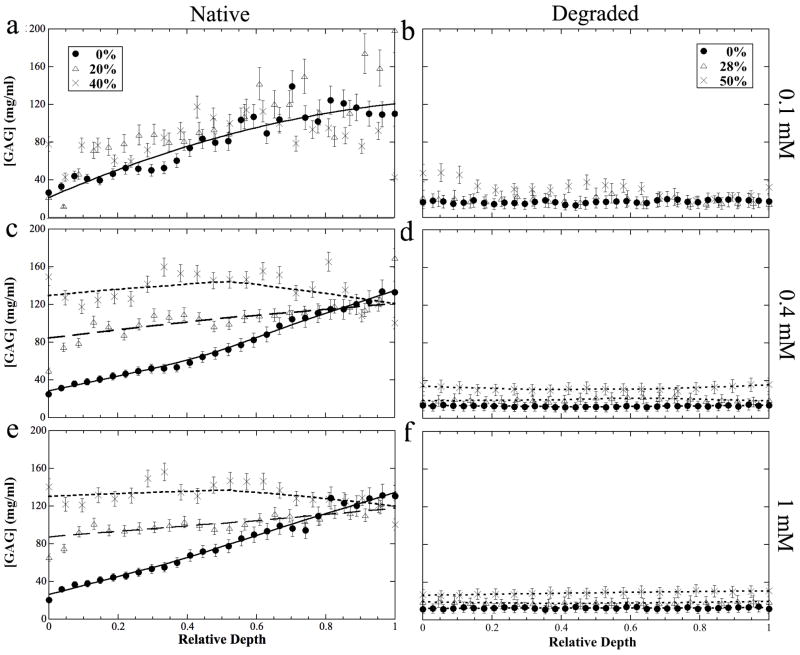
The depth-dependent profiles of the tissue GAG concentration at different concentrations of the soaking Gd solutions were calculated (without the empirical factor of 2) (Bashir et al 1996, Zheng and Xia 2010) and shown in Fig 4. These GAG profiles (Fig 4) had a number of notable features. First, the measured GAG concentration for the uncompressed cartilage was more accurate if the Gd concentration in the solution was high (which would produce a large difference between the before and after T1s). At low Gd concentration, the surface portion of the tissue had a better determination of its GAG content, because the surface tissue contained relatively more Gd ions even when the Gd concentration in the overall bath solution was low. At the concentration of 1 mM solution, the GAG profile in the uncompressed cartilage showed a well-defined linear function of the tissue depth. Second, the GAG concentration for the compressed cartilage lost its linear function as the tissue depth. Finally, there was no depth-dependency for GAG in the degraded tissue regardless of the amount of compression, reflecting the near completed depletion of GAG after the trypsin treatment.
Fig 4.
The profiles of the GAG concentration in the native (left) and degraded (right) articular cartilage at different Gd concentrations (0.1 mM, 0.4 mM, 1 mM).
The mean GAG concentrations in cartilage at various Gd concentrations were calculated from the GAG profiles and shown in Table 1. When the solution Gd concentration was above 0.2 mM, the averaged GAGs in cartilage were nearly constant, which were consistent with the results from the 23Na ICP-OES experiments in this project (shown in Table 1). As a comparison, the conservation calculation results were also shown in Table 1. The Kruskal-Wallis method showed that most of the mean concentrations above a minimum Gd concentration have no statistical difference among them.
Table 1.
The mean GAG concentrations in wet weight (mg/ml) in articular cartilage. The * in the table indicates that there was no statistic difference among the GAG concentrations for these groups of measurements at the same strain.
| Methods | GAG Concentration (mg/ml) | ||||||
|---|---|---|---|---|---|---|---|
| Native Tissue | Tissue Degraded by Trypsin | ||||||
| 0% strain | 20% strain | 40% strain | 0% strain | 28% strain | 50% strain | ||
| μMRI T1-Gd | Gd = 0.05mM | 71.4±5.5 | 112.4±17.9 | 114.1±19.9 | 27.5±8.3 | 13.4±7.3 | 34.8±9.4 |
| Gd = 0.1mM | 80.8±6.1 | 104.0±10.9 | 84.5±6.4 | 16.8±2.5 | 17.8±6.3 | 30.6±9.2 * | |
| Gd = 0.2mM | 78.1±6.3 * | 125.8±8.2 | 96.5±6.2 | 15.9±1.7 * | 19.5±2.3 * | 32.0±5.1 * | |
| Gd = 0.4mM | 76.4±6.4 * | 101.4±5.3 * | 130.6±5.9 * | 13.0±1.4 * | 19.5±1.7 * | 30.5±1.7 * | |
| Gd = 0.7mM | 74.6±7.0 * | 101.0±5.3 * | 131.7±5.8 * | 11.5±1.0 * | 21.5±1.4 * | 31.0±1.2 * | |
| Gd = 1.0mM | 78.5±6.5 * | 100.7±5.9 * | 130.8±5.9 * | 12.5±1.3 * | 18.0±1.1 * | 29.8±1.4 * | |
| Volume Conservation | 76.9±1.8+ | 96.1±2.3 | 128.2±3.0 | 13.2±1.6+ | 18.3±2.2 | 26.4±3.2 | |
| ICP-OES | 88.4±5.6 | 8.2±3.2 | |||||
Note that these two averaged numbers are the averages from Gd of 0.2 mM and above.
The Gd concentration profiles (not shown) in cartilage were averaged over the entire tissue depth and plotted in Fig 5, as the function of the Gd concentration in the solution. The dependency of the total Gd in both native tissue and degraded tissue was clearly linear with the solution concentration. The higher compression, the lower Gd concentration was in the tissue. The degraded tissue had more Gd ions than the native tissue.
Fig 5.
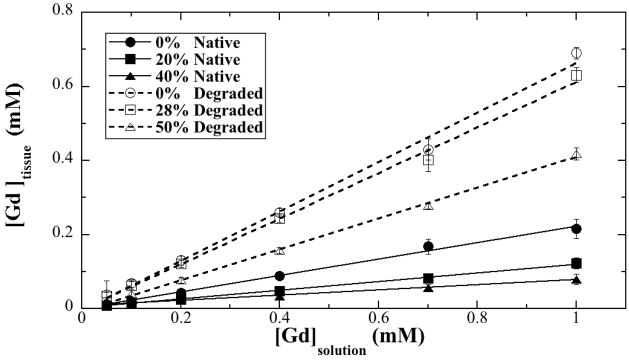
The concentrations of the mean Gd in the (a) native and (b) degraded tissue at different strains as the function of the Gd concentration in the soaking solution. The fitting coefficients in (a) were 0.9971 (0% strain), 0.9982 (20% strain) and 0.9949 (40% strain) respectively; and in (b) were 0.99697 (0% strain), 0.99787 (28% strain), and 0.9993 (50% strain) respectively.
DISCUSSION
The GAG quantification by the MRI dGEMRIC method relies on the equilibrium of the Gd ions diffusing into and out of the tissue. Since self-diffusion is a slow process, some regiments of physical exercises are used in the clinical dGEMRIC protocol to speed up the ion penetration into the tissue during the (short) waiting time (Burstein et al 2001, Salo et al 2012, Tiderius et al 2001). In this in vitro project, the contrast agent diffused into the small tissue block via its four ‘side-walls’, since the articular surface at the top and the bone at the bottom were covered by the plastic loading device. However, since the cartilage tissue in the images (Fig 1) was clearly uniform across the entire width of the tissue, a full equilibrium of the ion penetration into the center of the tissue block was unmistakable.
The depth-dependent effect of cartilage compression
For the native tissue (i.e., not-doped), static compression reduces the T1 value (Xia et al 2011) because of the reduction of the mobile water and the increase of the solid components in the compressed tissue. Since the compressive modulus of articular cartilage is depth-dependent (Chen et al 2001), a modest strain would reduce the surface tissue (SZ and TZ) much more than in the deeper tissue (RZ), hence reducing the surface T1 more than the deep T1. This non-linear reduction of T1 in a compressed cartilage signals a disproportional increase of the GAG concentration in the surface tissue. An increase of the surface GAG will result in an increase of the compressive modulus in the surface tissue. When the tissue is further compressed, its surface, which has already compressed the most and, hence, becomes harder to be compressed (Alhadlaq and Xia 2004, Alhadlaq and Xia 2005). For the degraded tissue, T1 value shows no obvious depth-dependence after loading because of the lost of the most GAG content in the tissue (Table 1).
The percentage tissue in our zone division at different strains leading to the data, shown in Fig 3, took into consideration several published data (Chen et al 2001, Xia et al 2001). It should be pointed out that the thickness of each cartilage zone that produced the average data in Fig 3 was not equal in percentage or absolute unit (μm). This type of zone-specific averaging and comparison is only possible when one has microscopic resolution where each pixel is smaller than the size of the thinnest zone. As comparison, each pixel dimension in clinical MRI typically has the same physical length, which exceeds the thickness of the thinnest cartilage zones. Hence, some pixels in clinical MRI data will contain several different structures of the tissue, which will cause inevitable errors in image analysis.
The critical points among the factors that influence the T1 values in compressed cartilage
T1 in cartilage reduces steadily as the increase of either the Gd concentration or strain (Fig 2), where the depth-dependent profiles were highly consistent with several previous reports (Xia 1998, Xia et al 2008, Xia et al 2011, Zheng and Xia 2010). Since the Gd contrast agent is negatively charged and distributes itself in a spatially inverse relationship to the local concentration of GAG within cartilage, more Gd ions will accumulate at SZ and TZ, which have a lower concentration of GAG (Xia et al 2008). This non-uniform distribution of Gd ions in articular cartilage results in a depth-dependent T1 profile, which is used in the GAG calculation. When the tissue is compressed, the non-uniform compression in articular cartilage has two competing effects. First, compression will cause the tissue to lose water and to gain the solid concentration, which should have an effect to decrease T1 (Xia et al 2011). Second, the tissue will also lose a portion of the Gd ions when the water is expelled, which should have an effect to increase T1. The measured T1 values in a Gd-doped and compressed cartilage reflect a balance between the two competing effects. The different trends around these critical points could explain the inconsistency between the human MRI study (Mayerhoefer et al 2010) and the μMRI study (Xia et al 2011). When the Gd concentration in the soaking solution (or body fluid) is low, T1 reduces when the tissue compression increases. In contrast, when the Gd concentration in the soaking solution (or body fluid) is high (above 0.4 mM), T1 increased when the tissue compression increases (Fig 3a). For degraded tissue, no obvious T1 change when the bulk Gd concentration is higher than 0.4 mM also suggested the balance between these two competing effects.
A novel observation of this study was the critical points of the zone-specific T1 plots in native tissues at different strains and different Gd concentrations (Fig 3), reflecting the complex consequence of the depth-dependent re-distributions of both the native molecules (collagen, GAG, water) and the externally infused ions (Gd) in cartilage when the tissue is compressed. Each critical point marks the particular Gd concentration that the T1 in a “GAG-poor” depth of the tissue (which drops faster at a higher Gd concentration) meets the T1 in a “GAG-rich” depth of the same block of tissue (which drops slower at the same Gd concentration). Zonal wise (Fig 3), each zone naturally has increasingly more GAG. Hence, the critical points meet at a lower Gd concentration for the same strain difference. For the degraded tissue, there was no critical point (Fig 3).
The influence of tissue loading to the GAG determination in any dGEMRIC-like protocol
Quantitatively, the GAG profiles and images in cartilage can be calculated based on a set of equations (Bashir et al 1996), where the T1 needs to be measured twice so the difference between the T1 relaxation rates can be calculated as (1/T1,Gd − 1/T1,before). The use of the dGEMRIC index would be a valid approximation, as long as T1,Gd is much smaller than T1,before and the profile of T1,before is mostly uniform. Even when both T1,Gd and T1,before are both used, it is clear in Fig 6a that the determination of the GAG could be inaccurate when the Gd concentration is low. This is due to the insufficient Gd ions in the tissue, which fails to cause a measurable difference between T1,before and T1,Gd. Since the use of any contrast agent should be limited to its necessity, this project showed that for the current experimental conditions (in vitro canine tissue at 7 Tesla magnet) a minimum Gd concentration should be about 0.2 mM for the native tissue without compression and 0.4 mM for compressed tissue. For the degraded tissue, a minimum Gd concentration should be about 0.2 mM for the GAG concentration. One important note, all experiments in this project were done at a steady state (i.e., at least 6 hours soaking in the Gd solution), which is different from the transient equilibrium situation clinically (Salo et al 2012). This steady state ensured the quantification of Gd ions and GAG concentrations in the tissue to be more accurate than the situation in clinical MRI (1.5 – 2 hours delay after injection) (Tiderius et al 2001). This μMRI result agreed with the findings in a recent clinical MRI report (Hawezi et al 2011), which showed that T1,before was lower in the deep region and an incomplete penetration of contrast medium into deep zone yielded a falsely too-long T1,Gd.
In conclusion, this study demonstrated that a compression of the native articular cartilage could cause a reduction of T1 values when the Gd concentration was low (less than 0.2 mM or 0.4 mM, depending upon the loading strain), or an increase of T1 values when the Gd concentration was high. It suggests that the 0.4 mM Gd concentration for the native tissue (0.2 mM for the degraded tissue) in the solution (or body fluid) to be a useful compromise, which is the minimum concentration of Gd that could provide an accurate determination of the GAG in cartilage without excess Gd ions. The critical point phenomenon of T1 suggests the quantification of GAG in articular cartilage by MRI dGEMRIC procedure depends upon the concentration of gadolinium contrast agent in the tissue.
Acknowledgments
Yang Xia is grateful to the National Institutes of Health for the R01 grants (AR 045172 and AR 052353). The authors are indebted to Drs. Cliff Les and Hani Sabbah (Henry Ford Hospital, Detroit) for providing the canine specimens, Mr. Farid Badar and Ms. Ji-hyun Lee (Dept of Physics, Oakland University) for helping with the tissue harvest and the experiments, Ms. Janelle Spann (Michigan Resonance Imaging, Rochester Hills, MI) for providing the contrast agent, and Ms. Carol Searight (Dept of Physics, Oakland University) for editorial comments.
References
- Alhadlaq H, Xia Y, Moody JB, Matyas J. Detecting Structural Changes in Early Experimental Osteoarthritis of Tibial Cartilage by Microscopic MRI and Polarized Light Microscopy. Ann Rheum Dis. 2004;63:709–717. doi: 10.1136/ard.2003.011783. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Alhadlaq HA, Xia Y. The structural adaptations in compressed articular cartilage by microscopic MRI (μMRI) T2 anisotropy. Osteoarthritis Cartilage. 2004;12:887–894. doi: 10.1016/j.joca.2004.07.006. [DOI] [PubMed] [Google Scholar]
- Alhadlaq HA, Xia Y. Modifications of orientational dependence of microscopic magnetic resonance imaging T2 anisotropy in compressed articular cartilage. J Magn Reson Imaging. 2005;22:665–673. doi: 10.1002/jmri.20418. [DOI] [PubMed] [Google Scholar]
- Bashir A, Gray ML, Burstein D. Gd-DTPA2- as a measure of cartilage degradation. Magn Reson Med. 1996;36:665–673. doi: 10.1002/mrm.1910360504. [DOI] [PubMed] [Google Scholar]
- Bashir A, Gray M, Hartke J, Burstein D. Nondestructive imaging of human cartilage glycosaminoglycan concentration by MRI. Magn Reson Med. 1999;41:857–865. doi: 10.1002/(sici)1522-2594(199905)41:5<857::aid-mrm1>3.0.co;2-e. [DOI] [PubMed] [Google Scholar]
- Burstein D, Velyvis J, Scott KT, Stock KW, Kim YJ, Jaramillo D, Boutin RD, Gray ML. Protocol issues for delayed Gd-DTPA2- enhanced MRI (dGEMRIC) for clinical evaluation of articular cartilage. Magn Reson Med. 2001;45:36–41. doi: 10.1002/1522-2594(200101)45:1<36::aid-mrm1006>3.0.co;2-w. [DOI] [PubMed] [Google Scholar]
- Chen S, Falcovitz Y, Schneiderman R, Maroudas A, Sah R. Depth-dependent compressive properties of normal aged human femoral head articular cartilage: relationship to fixed charge density. Osteoarthritis Cartilage. 2001;9:561–569. doi: 10.1053/joca.2001.0424. [DOI] [PubMed] [Google Scholar]
- Dardzinski BJ, Mosher TJ, Li S, Van Slyke MA, Smith MB. Spatial variation of T2 in human articular cartilage. Radiology. 1997;205:546–550. doi: 10.1148/radiology.205.2.9356643. [DOI] [PubMed] [Google Scholar]
- Duvvuri U, Kudchodkar S, Reddy R, Leigh JS. T1ρ relaxation can assess longitudinal proteoglycan loss from articular cartilage in vitro. Osteoarthritis Cartilage. 2002;10:838–844. doi: 10.1053/joca.2002.0826. [DOI] [PubMed] [Google Scholar]
- Gold GE, Han E, Stainsby J, Wright G, Brittain J, Beaulieu C. Musculoskeletal MRI at 3.0 T: relaxation times and image contrast. Am J Roentgenol. 2004;183:343–51. doi: 10.2214/ajr.183.2.1830343. [DOI] [PubMed] [Google Scholar]
- Hawezi ZK, Lammentausta E, Svensson J, Dahlberg LE, Tiderius CJ. In vivo transport of Gd-DTPA2- in human knee cartilage assessed by depth-wise dGEMRIC analysis. J Magn Reson Imaging. 2011;34:1352–8. doi: 10.1002/jmri.22750. [DOI] [PubMed] [Google Scholar]
- Juras V, Bittsansky M, Majdisova Z, Szomolanyi P, Sulzbacher I, Gäbler S, Stampfl J, Schüller G, Trattnig S. In vitro determination of biomechanical properties of human articular cartilage in osteoarthritis using multi-parametric MRI. J Magn Reson. 2009;197:40–47. doi: 10.1016/j.jmr.2008.11.019. [DOI] [PubMed] [Google Scholar]
- Lesperance LM, Gray ML, Burstein D. Determination of fixed charge density in cartilage using nuclear magnetic resonance. J Orthopaedic Research. 1992;10:1–13. doi: 10.1002/jor.1100100102. [DOI] [PubMed] [Google Scholar]
- Li X, Pai A, Blumenkrantz G, Carballido-Gamio J, Link T, Ma B, Ries M, Majumdar S. Spatial distribution and relationship of T1ρ and T2 relaxation times in knee cartilage with osteoarthritis. Magn Reson Med. 2009;61:1310–1318. doi: 10.1002/mrm.21877. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Maroudas A, Muir H, Wingham J. The correlation of fixed negative charge with glycosaminoglycan content of human articular cartilage. Biochim Biophys Acta. 1969;177:492–500. doi: 10.1016/0304-4165(69)90311-0. [DOI] [PubMed] [Google Scholar]
- Mayerhoefer ME, Welsch GH, Mamisch TC, Kainberger F, Weber M, Nemec S, Friedrich KM, Dirisamer A, Trattnig S. The in vivo effects of unloading and compression on T1-Gd (dGEMRIC) relaxation times in healthy articular knee cartilage at 3.0 Tesla. Eur Radiology. 2010;20:443–449. doi: 10.1007/s00330-009-1559-3. [DOI] [PubMed] [Google Scholar]
- Nieminen MT, Toyras J, Rieppo J, Hakumaki JM, Silvennoinen J, Helminen HJ, Jurvelin JS. Quantitative MR microscopy of enzymatically degraded articular cartilage. Magn Reson Med. 2000;43:676–81. doi: 10.1002/(sici)1522-2594(200005)43:5<676::aid-mrm9>3.0.co;2-x. [DOI] [PubMed] [Google Scholar]
- Nieminen MT, Rieppo J, Silvennoinen J, Toyras J, Hakumaki JM, Hyttinen MM, Helminen HJ, Jurvelin JS. Spatial assessment of articular cartilage proteoglycans with Gd-DTPA-enhanced T1 imaging. Magn Reson Med. 2002;48:640–8. doi: 10.1002/mrm.10273. [DOI] [PubMed] [Google Scholar]
- Nishii T, Shiomi T, Tanaka H, Yamazaki Y, Murase K, Sugano N. Loaded cartilage T2 mapping in patients with hip dysplasia. Radiology. 2010;256:955–65. doi: 10.1148/radiol.10091928. [DOI] [PubMed] [Google Scholar]
- Nissi M, Rieppo J, Töyräs J, Laasanen M, Kiviranta I, Nieminen M, Jurvelin J. Estimation of mechanical properties of articular cartilage with MRI-dGEMRIC, T2 and T1 imaging in different species with variable stages of maturation. Osteoarthritis Cartilage. 2007;15:1141–1148. doi: 10.1016/j.joca.2007.03.018. [DOI] [PubMed] [Google Scholar]
- Regatte RR, Akella SVS, Borthakur A, Kneeland JB, Reddy R. Proteoglycan Depletion-Induced Changes in Transverse Relaxation Maps of Cartilage: Comparison of T2 and T1ρ. Acad Radiology. 2002;9:1388–1394. doi: 10.1016/s1076-6332(03)80666-9. [DOI] [PubMed] [Google Scholar]
- Salo EN, Nissi MJ, Kulmala KA, Tiitu V, Toyras J, Nieminen MT. Diffusion of Gd- DTPA2 into articular cartilage. Osteoarthritis Cartilage. 2012;20:117–26. doi: 10.1016/j.joca.2011.11.016. [DOI] [PubMed] [Google Scholar]
- Shapiro EM, Borthakur A, Dandora R, Kriss A, Leigh JS, Reddy R. Sodium visibility and quantitation in intact bovine articular cartilage using high field 23Na MRI and MRS. J Magn Reson. 2000;142:24–31. doi: 10.1006/jmre.1999.1932. [DOI] [PubMed] [Google Scholar]
- Souza RB, Stehling C, Wyman BT, Hellio Le Graverand MP, Li X, Link TM, Majumdar S. The Effects of Acute Loading on T1ρ and T2 Relaxation Times of Tibiofemoral Articular Cartilage. Osteoarthritis Cartilage. 2010;18:1557–1563. doi: 10.1016/j.joca.2010.10.001. [DOI] [PubMed] [Google Scholar]
- Stanisz GJ, Henkelman RM. Gd-DTPA relaxivity depends on macromolecular content. Magn Reson Med. 2000;44:665–667. doi: 10.1002/1522-2594(200011)44:5<665::aid-mrm1>3.0.co;2-m. [DOI] [PubMed] [Google Scholar]
- Taylor C, Carballido-Gamio J, Majumdar S, Li X. Comparison of quantitative imaging of cartilage for osteoarthritis: T2, T1ρ, dGEMRIC and contrast-enhanced computed tomography. Magn Reson Imaging. 2009;27:779–784. doi: 10.1016/j.mri.2009.01.016. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Tiderius CJ, Olsson LE, Leander P, Ekberg O, Dahlberg L. Delayed gadolinium-enhanced MRI of cartilage (dGEMRIC) in early knee osteoarthritis. Magn Reson Med. 2003;49:488–492. doi: 10.1002/mrm.10389. [DOI] [PubMed] [Google Scholar]
- Tiderius CJ, Olsson LE, de Verdier H, Leander P, Ekberg O, Dahlberg L. Gd-DTPA2)-enhanced MRI of femoral knee cartilage: a dose-response study in healthy volunteers. Magn Reson Med. 2001;46:1067–71. doi: 10.1002/mrm.1300. [DOI] [PubMed] [Google Scholar]
- Venn M, Maroudas A. Chemical composition and swelling of normal and osteoarthrotic femoral head cartilage. I. Chemical composition. Ann Rheum Dis. 1977;36:121–129. doi: 10.1136/ard.36.2.121. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Xia Y. Relaxation anisotropy in cartilage by NMR microscopy (μMRI) at 14-μm resolution. Magn Reson Med. 1998;39:941–949. doi: 10.1002/mrm.1910390612. [DOI] [PubMed] [Google Scholar]
- Xia Y. Resolution ‘scaling law’ in MRI of articular cartilage. Osteoarthritis Cartilage. 2007;15:363–365. doi: 10.1016/j.joca.2006.12.004. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Xia Y, Zheng SK, Bidthanapally A. Depth dependent profiles of glycosaminoglycans in articular cartilage by MRI and histochemistry. J Magn Reson Imaging. 2008;28:151–157. doi: 10.1002/jmri.21392. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Xia Y, Moody J, Burton-Wurster N, Lust G. Quantitative In Situ Correlation Between Microscopic MRI and Polarized Light Microscopy Studies of Articular Cartilage. Osteoarthritis Cartilage. 2001;9:393–406. doi: 10.1053/joca.2000.0405. [DOI] [PubMed] [Google Scholar]
- Xia Y, Wang N, Lee J, Badar F. Strain-dependent T1 Relaxation Profiles in Articular Cartilage by MRI at Microscopic Resolutions. Magn Reson Med. 2011;65:1733–1737. doi: 10.1002/mrm.22933. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Xia Y, Farquhar T, Burton-Wurster N, Vernier-Singer M, Lust G, Jelinski L. Self-diffusion monitors degraded cartilage. Arch Biochem Biophys. 1995;323:323–328. doi: 10.1006/abbi.1995.9958. [DOI] [PubMed] [Google Scholar]
- Zheng SK, Xia Y. The impact of the relaxivity definition on the quantitative measurement of glycosaminoglycans in cartilage by the MRI dGEMRIC method. Magn Reson Med. 2010;63:25–32. doi: 10.1002/mrm.22169. [DOI] [PMC free article] [PubMed] [Google Scholar]