Pollinator effectiveness varies with experimental shifts in flowering time

Abstract

The earlier flowering times exhibited by many plant species are a conspicuous sign of climate change. Altered phenologies have caused concern that species could suffer population declines if they flower at times when effective pollinators are unavailable. For two perennial wildflowers, Tradescantia ohiensis and Asclepias incarnata, we used an experimental approach to explore how changing phenology affects the taxonomic composition of the pollinator assemblage and the effectiveness of individual pollinator taxa. After finding in the previous year that fruit set varied with flowering time, we manipulated flowering onset in greenhouses, placed plants in the field over the span of five weeks, and measured pollinator effectiveness as the number of seeds produced after a single visit to a flower. The average effectiveness of pollinators and the expected rates of pollination success were lower for plants of both species flowering earlier than for plants flowering at historical times, suggesting there could be reproductive costs to earlier flowering. Whereas for A. incarnata, differences in average seed set among weeks were due primarily to changes in the composition of the pollinator assemblage, the differences for T. ohiensis were driven by the combined effects of compositional changes and increases over time in the effectiveness of some pollinator taxa. Both species face the possibility of temporal mismatch between the availability of the most effective pollinators and the onset of flowering, and changes in the effectiveness of individual pollinator taxa through time may add an unexpected element to the reproductive consequences of such mismatches.

Introduction

Global climate change appears to be affecting the flowering times of many temperate plant species. Long-term phenological records from the Northern Hemisphere commonly show advances in flowering onset ranging from a few days to more than a month, although dates of first bloom for some plants remain unchanged or are even delayed (Bradley et al. 1999, Abu-Asab et al. 2001, Fitter and Fitter 2002, Miller-Rushing and Primack 2008). Annual plants, particularly those that flower in spring and are pollinated by insects, tend to show the greatest advances (Fitter and Fitter 2002).

Because the reproductive success of most flowering plants depends at least in part on pollination by insects (Ollerton et al. 2011), changing phenologies have caused concern that species could suffer population declines if they flower when pollinators are unavailable (Hegland et al. 2009). Although temperature and photoperiod may trigger both flowering onset (Rathcke and Lacey 1985) and insect activity (Tauber and Tauber 1976), asynchrony may ensue if interacting species are sensitive to those cues at different times or to different degrees (Visser and Both 2005, Doi et al. 2008, Forrest and Thomson 2011). Furthermore, natural selection may not suffice to maintain synchrony if there is insufficient heritable variation governing the response to changing cues (Memmott et al. 2007). Interactions with other species also may reduce the likelihood that plant and pollinator phenologies will coevolve in a changing climate (Gilman et al., in press).

Data on flowering time and pollinator activity in response to both longer-term (Gordo and Sanz 2005, Doi et al. 2008) and shorter-term (Wall et al. 2003, Kudo et al. 2004) temperature changes, along with community-level simulations (Memmott et al. 2007), support the idea that mismatches will grow larger in the future. Even in the absence of complete mismatches, plants may suffer from reduced pollination because the composition of pollinator assemblages fluctuates temporally (Alarcón et al. 2008, Olesen et al. 2008), and not all pollinators are equally effective (Ivey et al. 2003, Sahli and Conner 2007). Even the effectiveness of a given pollinator species can change over time (Fishbein and Venable 1996), for example, as a function of the identities of co-flowering species (Mitchell et al. 2009). On the other hand, there are reasons to suspect that fitness consequences of mismatches might not be as great as feared. Reciprocal specialization between plants and pollinators is rare (Waser et al. 1996, Bascompte et al. 2003, Vázquez and Aizen 2004), and the diffuse nature of pollination webs suggests that species may be buffered from the loss of some interactions (Memmott et al. 2004). An experimental manipulation (Rafferty and Ives 2011) showed that most species in a wildflower community that had experienced historical shifts to earlier flowering received more pollinator visits when induced to flower earlier than their normal phenology.

These complications suggest the need to explore how the timing of flowering influences pollination success through changes in the identities of pollinators that may vary in their effectiveness due to morphological and behavioral differences. Here, we report on such an exploration with two plant species, Tradescantia ohiensis Raf. (Commelinaceae) and Asclepias incarnata L. (Apocynaceae). We experimentally manipulated the flowering onset of these species so that we could expose flowers to pollinators in the field up to two weeks before and after natural flowering would have begun. Thus, our goal was to assess pollination success for plants at the beginning of their flowering curves by advancing and delaying flowering relative to the current timing of first flowering. We characterized the community of visitors to plants with different phenologies, and measured the pollination effectiveness of different insect species and of the same species through time.

Methods

Study system

We studied T. ohiensis (common spiderwort) and A. incarnata (swamp milkweed), two perennial species in a long-term census of dates of first bloom of Wisconsin wildflowers carried out from 1935 to 1945 and from 1977 to 2007 (Bradley et al. 1999; N. Bradley, C. Bradley, and A. Leopold, unpublished data). We found that both species flowered significantly earlier in 1977–2007 than in 1935–1945, with flowering onset advanced by 6 and 9 days for T. ohiensis and A. incarnata, respectively (Rafferty and Ives 2011). Comparable data on insect phenology are unavailable in our study system.

We chose to focus on these species for two reasons. First, data from an experiment in the previous year (2009) indicated that overall visitation rates to these species showed contrasting patterns. Of six species that showed historically advanced flowering, T. ohiensis was among the five that experienced greater pollinator visitation rates when placed earlier into the field; A. incarnata was the exception that showed lower visitation rates earlier in the season (Rafferty and Ives 2011). Second, the temporal pattern of fruit set varied with flowering onset for both species, suggesting changes in the aggregate effectiveness of the pollinators visiting each of the two plant species that do not necessarily correspond to overall visitation rates (Appendix A).

T. ohiensis is a self-incompatible (Owens and McGrath 1984) species that flowers from late May until early August in our study area. Individual plants flower for several weeks, grow to be about 0.5 m tall, and typically produce one to several blue flowers in a terminal cyme that last for a single day and do not possess nectaries (Grundel et al. 2000). The actinomorphic flowers are three petaled and possess six stamens. T. ohiensis produces capsules that ripen in about 60 days and contain a maximum of six seeds (N. Rafferty, unpublished data).

Although A. incarnata populations vary in levels of self incompatibility (Ivey et al. 1999), they are nonautogamous and therefore dependent on insects, with paired umbels of small pink flowers that produce nectar as the sole reward. Plants flower from late June to mid-August in our study area. Individual plants flower for several weeks, grow to be 1.5 m tall, and can produce hundreds of flowers that last for several days. The flowers are complex, with five pollinaria and five stigmatic chambers surrounded by hoods. The fruits are follicles that ripen in about 60 days and contain as many as 95 wind-dispersed seeds (N. Rafferty, unpublished data).

Manipulation of flowering onset

We aimed to manipulate the flowering onset of both species such that some plants were in flower one and two weeks in advance of the current date of first bloom (averaged across 1977–2007), and one and two weeks after that date, for a total of five phenological treatments. Between 1977 and 2007, the date of first bloom ranged from 7 May to 11 June with a mean of 28 May for T. ohiensis, and from 20 June to 8 July with a mean of 29 June for A. incarnata (Bradley et al. 1999; N. Bradley, C. Bradley, and A. Leopold, unpublished data). Assuming a linear relationship between year and date of first bloom, the one-week shift between each phenological treatment represents about 60 and 50 years for T. ohiensis and A. incarnata, respectively. Seedlings of local genotypes were obtained from a nursery in April 2010 and maintained in greenhouses at the University of Wisconsin-Madison. Plants were raised in 2.8-L pots in Sun Gro Metro-Mix 300 growing medium (Sun Gro, Vancouver, British Columbia, Canada) and fertilized every three months. When necessary, plants were sprayed with Ultra Pure Oil (Prescription Treatment, St. Louis, Missouri, USA) to control thrips, after which we waited at least one week before using them in the field. Otherwise, plants were treated with pesticides only after they had been exposed to pollinators.

To manipulate flowering time, subsets of each species were placed in two greenhouses: a warmer greenhouse (24–28°C day, 21°C night) and a cooler greenhouse (18–21°C day, 16°C night). In the warmer greenhouse, half of the plants were exposed to supplemental lighting and half were not. Supplemental lights turned on automatically during the day when ambient light levels were less than 500 μmol·m⁻²·s⁻¹ and did not alter photoperiod. Plants were rotated among the three greenhouse treatments, such that all plants began in the cooler greenhouse, were moved to the warmer greenhouse but did not receive supplemental light, and then were exposed to both warmer conditions and supplemental light. The first group of plants placed under the lights began flowering earliest; these were then replaced by plants that had not yet been exposed to supplemental light to yield plants with different flowering phenologies. All plants were at least one year old when they began flowering. Plants were haphazardly assigned to one of three groups: focal plants that were used to measure pollinator effectiveness, hand-pollinated field controls that served as pollen donors, or hand-pollinated greenhouse controls.

While our phenological design was realized for T. ohiensis, we were unable to force A. incarnata to flower in advance of the current mean date of first bloom. However, the first two plant arrays of A. incarnata do represent advanced flowering relative to the historical mean date of first bloom (1935–1945). Thus, the five weeks in which plant arrays were placed in the field differed in their timing relative to the current or historical mean dates of first bloom for the two species.

Study site

The experiment was performed in a 40-m² study area (43.04° N, 89.43° W) in the Curtis Prairie, a 24-ha, 76-year-old restored tallgrass prairie in the University of Wisconsin Arboretum, Madison, Wisconsin, USA, in 2010. Although only T. ohiensis occurs in the study area, A. incarnata is found within 450 m elsewhere in the Curtis Prairie. The dates of flowering onset of all forbs in the study area were documented and the numbers of T. ohiensis plants and flowers within 5 m of focal plants were counted each day.

Plant arrays

The experiment was designed to simulate the initiation of the flowering curve at different points in time. In the field, 10 plants that served as pollen donors were arranged in a 2 m diameter circle, spaced 0.5 m apart and 1 m from the focal plant, which was placed in the center of the circle (Sahli and Conner 2007). Although we do not have comparable data for A. incarnata, we noted 10 T. ohiensis plants flowering in the study area on 27 May, with no plants flowering the previous two days; thus, 10 donor plants should realistically mimic floral density at the start of the flowering curve. Prior to focal observation, visits to focal flowers were prevented by enclosing plants or flowers in fine mesh.

Once a focal plant was placed in the center of the array, it was observed continuously. For T. ohiensis, individual flowers were monitored for visits, whereas individual inflorescences were monitored for A. incarnata. Focal flowers/inflorescences (hereafter referred to as flowers) were allowed only a single visit from a single pollinator. Visits were timed, with visit duration defined as the total time the visitor contacted reproductive structures. Each visit to a focal flower was assigned a unique code, and the identity of the pollinator, the time the visit began, and the visit duration were recorded. Visited focal flowers were bagged with fine mesh to prevent further visits and tagged with the visit code. When possible, visits to pollen donors were recorded, and it was noted whether pollinators that visited the focal plant had previously visited pollen donors.

After each visit to a focal flower, a different plant was used so that we cycled through focal plants, of which there were 10 each day. Thus, multiple flowers on the same focal plants were sometimes used. In general, focal plants were exposed to pollinators from 0930 to 1400, and each phenological treatment consisted of three days of field observations, for a total of 30 focal plants and 30 pollen donor plants. Based on observations made in 2010 and weekly census data from 2009, three days represents the first 7.5% of the flowering season for T. ohiensis in the study area. Although we did not document the end of the flowering curve for A. incarnata in the Curtis Prairie, we estimate that three days represents the first 5% of the flowering season based on personal observations.

Controls

Two groups of plants were hand pollinated to serve as controls. The hand-pollinated field control plants served two purposes. First, they served as pollen donors for the focal plants; thus, pollinators were allowed unrestricted access to these flowers. Second, they acted as controls for any effects that daily weather conditions may have had on seed set, with the idea that hand-pollinated flowers should have set full seed if conditions permitted. The hand-pollinated greenhouse control plants were exposed to the same greenhouse conditions as the focal plants and served to control for any effects that the flowering manipulations alone may have had on seed set due, for example, to fewer ovules or available resources. The mean dates of first bloom for both hand-pollinated field and greenhouse control plants were always within one to six days of those of focal plants of each phenological treatment for both species (Appendix B). Pollen from multiple donor plants was sometimes used to hand-pollinate a single control plant, but individual flowers received pollen from only one donor plant.

For T. ohiensis, all open flowers on field control plants were hand-pollinated on the morning they were placed in the field by brushing the stigmas with anthers from other plants until pollen was deposited. For A. incarnata, two flowers per field control plant were hand pollinated the evening before they were used in the field following the methods of Wyatt (1976). For both species, 5–10 greenhouse control plants were hand pollinated each week.

Because plants with different phenologies differed in the time available for resource acquisition, we recorded the number of flowers and height for each plant on the day it was used as a focal or control. The manipulations might also have affected aspects of flower quality, and, in particular, nectar production in A. incarnata (Rafferty and Ives 2011). We therefore measured nectar quantity and sugar content for each A. incarnata plant that was used as a greenhouse control. Nectar was extracted with microcapillary pipettes (Drummond Microcaps; Broomall, Pennsylvania, USA), the length of the nectar column was measured to give nectar volume, and the nectar was then discharged onto a refractometer (Bellingham and Stanley Eclipse 45-82; Tunbridge Wells, Kent, UK) to give sugar content (Corbet 2003).

Measurement of pollinator effectiveness

Pollinator effectiveness was defined as the number of seeds resulting from a single visit to a flower (Wiggam and Ferguson 2005, Sahli and Conner 2007) and thus provides a measure of female reproductive success. After exposure in the field, all plants were returned to the cooler greenhouse until fruits ripened to measure visitor effectiveness using seed set in controlled environmental conditions. Seeds were then counted. For focal and hand-pollinated flowers that did not set fruit, seed set was recorded as zero. Voucher specimens of visitors were deposited in the Insect Research Collection of the University of Wisconsin, Madison. Because not all pollinators were identified to species, we refer to them as different pollinator taxa. With the exception of the taxon Bombus, we believe that each of our taxon classifications reflect a single species.

Statistical analyses

We calculated the mean effectiveness of each pollinator taxon that visited at least five focal flowers and the mean seed set per flower for focal and control plants of each phenological treatment (week). In all of these calculations, we included values of zero for flowers that did not set fruit, except in the case of A. incarnata hand-pollinated controls, for which we included only flowers that produced fruits or were known to have aborted fruits, because we suspect that many of our hand pollinations were unsuccessful. Although fruit set from hand pollinations under ideal greenhouse conditions is typically low (<50%) in this species (Ivey et al. 1999), our hand pollinations were successful for only 2, 3, 7, 5, and 18 flowers in each phenological treatment; thus, fruit set was as low as 3% for some of our treatment groups (data not shown). We used the seed set of one open-pollinated flower per field control plant that produced at least one mature fruit, including zero values for each field control plant that did not produce fruit, as an additional gauge of the maximum pollination success of A. incarnata on any given field day.

We investigated variation in the seed set of focal flowers using generalized linear mixed models (GLMM; Gelman and Hill 2007) with the number of seeds as the response variable, week (continuous) and pollinator taxon (factor with six and eight levels for T. ohiensis and A. incarnata, respectively) as fixed effects, and individual plant (factor with 97 and 111 levels for T. ohiensis and A. incarnata, respectively) as a random effect. We introduced additional predictor variables to these baseline models, including the number of T. ohiensis flowers within 5 m of the focal plant, the number of co-flowering species in the study area, visit duration, the mean seed set of hand-pollinated control plants, the height of the focal plant, and the number of flowers on the focal plant. To gauge the importance of visits to pollen donor plants, we ran separate baseline models that included whether or not a pollinator was known to have visited a field control plant before visiting the focal plant (factor with two levels) as a fixed effect for each pollinator taxon for which we had at least five samples in each category. For T. ohiensis, we assumed that seed set was Poisson distributed due to the small numbers of seeds per fruit, whereas, for A. incarnata, we assumed that seed set was normally distributed; diagnostics of residuals from the analyses supported both of these assumptions. To assess simultaneously multiple coefficients in the models, we used likelihood ratio (LR) tests and retained only those predictor variables that were significant when introduced to the baseline models.

In the analyses just described, we included hand-pollinated plants as predictor variables to control for possible differences in weekly seed set caused by time-dependent variation among plants. For a direct comparison between seed set for experimental and control flowers, we used additional GLMMs that included both experimental and control flowers as response variables, with a categorical predictor variable “pollinator type” to distinguish controls (hand-pollinated for T. ohiensis and open-pollinated for A. incarnata) from experimental flowers. Thus, the baseline model included seed set of both focal and hand/open-pollinated flowers as the response variable, week (continuous), pollinator type (factor with six and nine levels for T. ohiensis and A. incarnata, respectively), and all pollinator taxa that met the criteria above as fixed effects. Day-of-sample (factor with 18 and 15 levels for T. ohiensis and A. incarnata, respectively) and individual plant (factor with 283 and 231 levels for T. ohiensis and A. incarnata, respectively) were included as random effects. Hand-pollinated flowers of A. incarnata were not included in these analyses because sample sizes in some weeks were very small (e.g., N = 2 for field and greenhouse hand-pollinated controls combined in the week of current date of first bloom). To determine whether the effectiveness of pollinators changed in a taxon-specific way, we tested for an interaction between week and pollinator. Because we were interested in this interaction and in comparing the model with vs. without the interaction, we excluded one taxon that occurred in only one week for T. ohiensis.

We assessed whether the analyses of individual pollinator taxa could combine to explain overall pollination success by summing for each week the product of each pollinator’s effectiveness and the proportion of visits each pollinator made (i.e., pollinator importance; Olsen 1997, Sahli and Conner 2007). This calculation should predict seed set because each flower was restricted to have only a single pollinator visit, so that seed set is given by the average proportion of pollinator taxa visits weighted by effectiveness. For this analysis, we calculated pollinator effectiveness using a GLMM in which hand-pollinated controls were excluded. To test whether the composition of the visitor assemblage varied with flowering onset, we used a chisquare test. We also examined visitation rates of focal plants by calculating the time between exposure and visitation (visit latency) and then taking the inverse to give the expected number of pollinators per minute. In this way, we were able to compare the visitation rates for 2010 with those from 2009 (Rafferty and Ives 2011). To account for the fact that higher visitation rates by less-effective pollinators could result in the same reproductive output as lower visitation rates by more-effective pollinators, we used the product of the weekly visitation rates for 2010 and the mean effectiveness of all visitors to plants of a given phenological treatment to estimate the expected rate of successful pollination leading to seed set.

Finally, to determine whether nectar volume or sugar content of A. incarnata increased or decreased over the five weeks, we used linear mixed models (LMM). Week (continuous), number of inflorescences (continuous), and time of day (continuous) were included as fixed effects, and individual plant (factor with 40 levels) and flower replicate (factor with four levels) were included as random effects. All analyses were performed in R 2.12.1 (R Core Development Team 2010), using the lmer function in the lme4 package (available online).²

Results

In total, we recorded 235 visits to focal flowers of T. ohiensis by 10 pollinator taxa and 257 visits to focal inflorescences of A. incarnata by 15 pollinator taxa. Limiting our sample to only those pollinator taxa that visited on at least five occasions yielded 228 visits to focal flowers of T. ohiensis by six pollinator taxa from two orders (Diptera and Hymenoptera) and three families (Syrphidae, Halictidae, and Apidae), and 248 visits to focal inflorescences of A. incarnata by eight pollinator taxa, all hymenopterans from four families (Halictidae, Apidae, Megachilidae, and Vespidae). Three of the pollinator taxa were shared by the plant species.

Tradescantia ohiensis

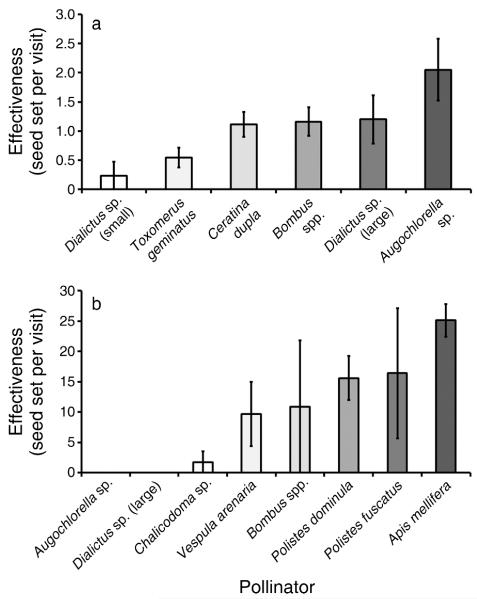
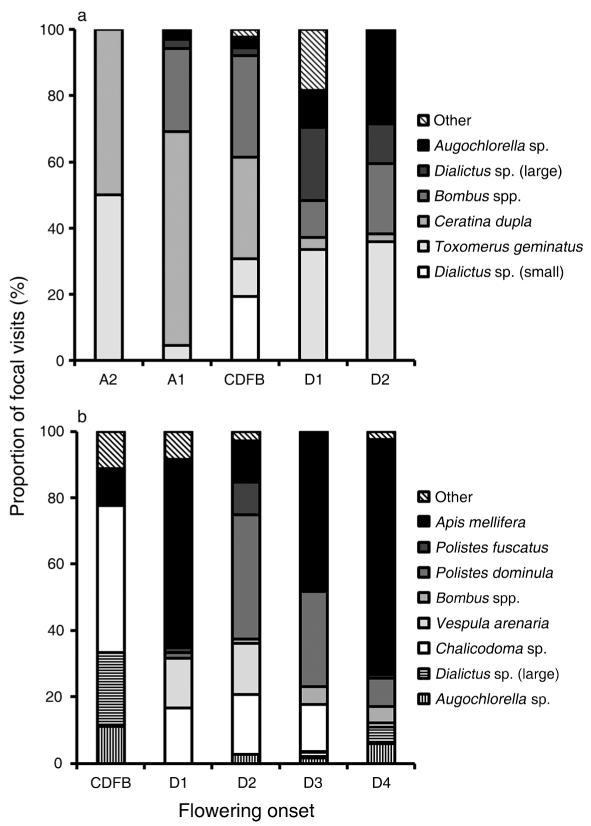
There were significant differences among taxa in their effectiveness as pollinators of T. ohiensis (Fig. 1a); the model that included pollinator taxa (Table 1) fit significantly better than the model without taxon-specific pollinator effects (LR test, ${\chi }_6^2$ = 39.2, P < 0.0001). In addition, the composition of the pollinator taxa that visited focal plants differed significantly among weeks (${\chi }_{24}^2$ = 169.4, P < 0.0001; Fig. 2a).

Fig. 1.

Effectiveness (mean ± SE), as measured by seed set from a single visit to a single flower, by visitors to (a) Tradescantia ohiensis and (b) Asclepias incarnata. The SE values are presented for descriptive purposes only.

Table 1.

Results for a generalized linear mixed model (GLMM) analysis with seed set of focal flowers of Tradescantia ohiensis as the response variable and pollinator taxa and week as predictor variables.

Effects	Estimate	SE/SD†	z	P	N
Fixed
Week	0.41	0.15	2.76	0.006	5
Bombus spp.	−1.79	0.57	−3.14	0.002	56
Dialictus sp. small	−3.37	0.74	−4.58	<0.0001	17
Augochlorella sp.	−1.74	0.62	−2.81	0.005	20
Ceratina dupla	−1.85	0.51	−3.65	0.0003	78
Toxomerus geminatus	−2.80	0.64	−4.38	<0.0001	42
Dialictus sp. large	−2.07	0.65	−3.20	0.001	15
Random
Individual plant		1.26		<0.0001	97

Note: Coefficients are on a log scale because seed set was assumed to be Poisson distributed.

^†SE for fixed effects, SD for random effects.

Fig. 2.

Proportion of visits to plants with different weeks of flowering onset relative to the current date of first bloom (CDFB) for (a) Tradescantia ohiensis and (b) Asclepias incarnata. “Other” are taxa that made fewer than five visits. Flowering onset is described as A2, advanced by 2 weeks; A1, advanced by 1 week; D1, delayed by 1 week; D2, delayed by 2 weeks; D3, delayed by 3 weeks; and D4, delayed by 4 weeks.

Week had a positive effect on seed set, and the log number of T. ohiensis (non-experimental) flowers within 5 m of the focal plant had a negative effect (−0.008 ± 0.004 [estimated model coefficient, mean ± SE], z = −2.0, P < 0.04). Nonsignificant effects included the log number of co-flowering species in the study area (0.1 ± 0.1, z = 0.9, P > 0.4), the log visit duration (−0.0007 ± 0.001, z = −0.7, P > 0.5), the log mean seed set of hand-pollinated field controls (0.6 ± 0.6, z = 1.0, P > 0.3), the log mean seed set of hand-pollinated greenhouse controls (0.4 ± 0.3, z = 1.7, P > 0.1), log plant height (−0.006 ± 0.06, z = −0.1, P > 0.9), and the log number of flowers on plants (−0.1 ± 0.2, z = −0.6, P > 0.5).

Bombus spp., which was the only taxon for which sample sizes were adequate, were significantly more effective (1.5 vs. 0.86 seeds) when they were known to have visited a field control before visiting a focal flower (z = 2.96, P < 0.003), but there was no significant interaction with week.

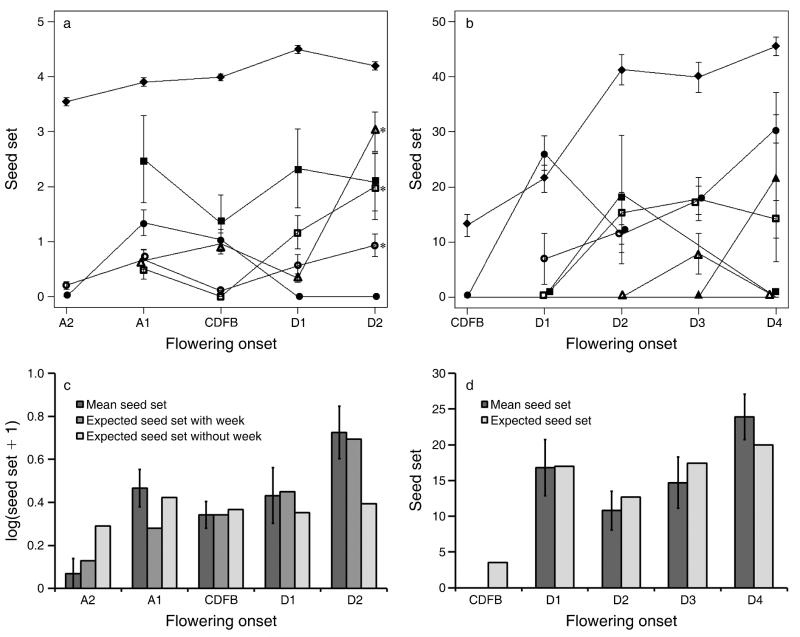
To investigate in more detail changes in the effectiveness of pollinators through time (week), we performed analyses including the seed set of hand-pollinated controls in the response variable; there was no significant difference between the hand-pollinated field and greenhouse controls (LR test, ${\chi }_2^2$ = 2.4, P > 0.3), so we pooled these data. There was heterogeneity in the changes in effectiveness with week; the changes in seed set with week for three of the pollinator taxa were of greater magnitude than the change in seed set for the hand-pollinated controls (Table 2; Fig. 3a). Furthermore, the model containing pollinator-specific changes in effectiveness (interactions between pollinator/hand pollination and week) fit better than the model without interactions (LR test, ${\chi }_5^2$ = 20.6, P < 0.001). To compare natural pollinators with hand-pollination controls, we aggregated all pollinators; as a group, the pollinators showed a stronger increase in effectiveness with week (estimated model coefficients of 0.33 ± 0.081 vs. 0.049 ± 0.025). Furthermore, this model aggregating pollinator taxa fit the data marginally less well than the model assuming pollinator-specific changes in effectiveness (LR test, ${\chi }_4^2$ = 8.8, P < 0.066). A difficulty in this analysis is that we included day of sample as a random effect, which could absorb some of the variation in effectiveness with week. In an analysis excluding hand-pollinated plants in which the day-of-sample random variable is removed, there is statistically significant variation among pollinators in their change in effectiveness with week (LR test, ${\chi }_1^2$ = 4.6, P < 0.032). Thus, there is some statistical evidence that pollinator taxa differed in how their effectiveness changed.

Table 2.

Results for a GLMM with seed set of focal and hand-pollinated field and greenhouse flowers of Tradescantia ohiensis as the response variable and pollinator taxa and hand-pollination as predictor variables.

Effects	Estimate	SE/SD†	z	P	N
Fixed
Hand pollination	1.21	0.083			440
Bombus spp.	−1.54	0.46			56
Augochlorella sp.	0.17	0.71			20
Ceratina dupla	0.0028	0.44			78
Toxomerus geminatus	−2.51	0.92			42
Dialictus sp. large	−2.80	1.48			15
Week × hand pollination	0.049	0.024	2.03	0.043
Week × Bombus spp.	0.44	0.12	3.49	0.0005
Week × Augochlorella sp.	0.056	0.16	0.35	0.73
Week × Ceratina dupla	−0.048	0.18	−0.27	0.79
Week × Toxomerus geminatus	0.42	0.21	1.98	0.047
Week × Dialictus sp. large	0.65	0.33	1.96	0.050
Random
Individual plant		0.30		<0.0001	283
Date		0		1	18

Notes: The interaction between week and hand pollination gives the slope for hand pollinations, and the interactions between pollinator taxa and week test whether the slope for each pollinator differs from the slope for hand pollinations. The z scores and P values are not given for the main effects because we are not interested in hypotheses regarding these terms. Coefficients are on a log scale because seed set was assumed to be Poisson distributed.

^†SE for fixed effects, SD for random effects.

Fig. 3.

(a, b) Seed set (mean ± SE) from a single visit by visitors and hand or open pollinations over different weeks of flowering onset relative to the current date of first bloom (CDFB) for (a) Tradescantia ohiensis (diamonds, hand pollinations; solid squares, Augochlorella sp.; open squares, Dialictus sp. large; open triangles, Bombus spp.; solid circles, Ceratina dupla; open circles, Toxomerus geminatus) (asterisks indicate taxa that differ significantly [P < 0.05] from the hand-pollinated controls) and (b) Asclepias incarnata (diamonds, open pollinations; solid circles, Apis mellifera; solid squares, Polistes fuscatus; open squares, Polistes dominula; solid triangles, Bombus spp.; open circles, Vespula arenaria; open triangles, Chalicodoma sp., line at 0, ineffective visitors: Augochlorella sp. and Dialictus sp. large). (c, d) Observed and expected seed set of focal flowers on plants with different weeks of flowering onset relative to the CDFB for (c) T. ohiensis and (d) A. incarnata. Expected seed-set values are based on the summed product of each pollinator taxon’s effectiveness and the proportion of visits each pollinator taxon made each week (pollinator importance) and, for T. ohiensis, pollinator importance plus the effect of week. The SE values are presented for descriptive purposes only. Flowering onset is as described in Fig. 2.

For T. ohiensis, two factors affected the increase in the effectiveness per visitation of pollinators through time, leading to an increase in the average seed set (Fig. 3c; Appendix C). First, the composition of pollinator taxa varied among the five phenological treatments (Fig. 2a), with the proportion of visits from more-effective pollinators (i.e., aggregated pollinator importance) increasing through time. Second, the effectiveness of some individual pollinator taxa increased over time. If increases in pollinator effectiveness through time are ignored, only 29% of the variation in seed set among phenological treatments was explained by aggregated pollinator importance (Fig. 3c). Adding the effect of week to this model (in an analysis similar to Table 2 but excluding hand-pollinated controls in the response variable) explained 84% of the variation in seed set among phenological treatments (Fig. 3c), underscoring the importance of increasing effectiveness exhibited by pollinators as a group through time. However, taxon-specific changes in effectiveness were less important, as 81% of the variation in seed set was explained by the interaction between week and pollinator effectiveness.

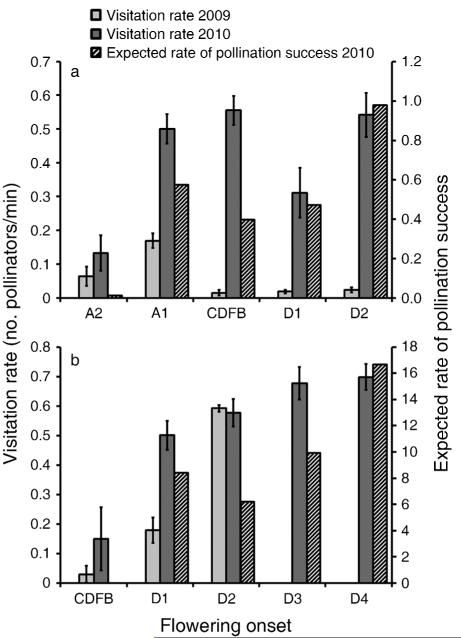
Unlike in 2009, when visitation rates decreased over time (Rafferty and Ives 2011), plants placed in the field two weeks early had the lowest visitation rates, and visitation rates did not decline for plants around the current date of first bloom (Fig. 4a). Accounting for weekly variation in visitation rates in 2010, the expected rate of successful pollination was lowest for plants flowering two weeks before, and highest for plants flowering two weeks after, the current date of first bloom (Fig. 4a).

Fig. 4.

Visitation rate (mean ± SE) of pollinators that visited focal flowers and expected rate of pollination success (mean effectiveness of all visitors × number of visits per minute) for plants with different weeks of flowering onset relative to the current date of first bloom (CDFB) for (a) Tradescantia ohiensis and (b) Asclepias incarnata. The SE values are presented for descriptive purposes only. Flowering onset is as described in Fig. 2.

Asclepias incarnata

Of the eight pollinators that visited A. incarnata most frequently, two were ineffective (Fig. 1b). As was true for T. ohiensis, there were significant differences among pollinators in effectiveness (LR test, ${\chi }_8^2$ = 22, P < 0.005; Table 3), and the composition of pollinator taxa varied among weeks (chi square test, ${\chi }_{32}^2$ = 156.0, P < 0.0001; Fig. 2b).

Table 3.

Results for a GLMM with seed set of focal flowers of Asclepias incarnata as the response variable and pollinator taxa and plant height as predictor variables.

Effects	Estimate	SE/SD†	t	P	N
Fixed
Chalicodoma sp.	−53.9	16.4	−3.28	0.001	36
Bombus spp.	−0.93	9.38	−0.10	0.9	8
Augochlorella sp.	−9.58	9.11	−1.05	0.3	9
Apis mellifera	15.6	4.55	3.43	0.0007	129
Vespula arenaria	8.22	6.71	1.23	0.2	20
Polistes fuscatus	12.8	8.82	1.45	0.2	9
Polistes dominula	8.19	5.28	1.55	0.1	51
Dialictus sp. large	−0.42	9.74	−0.04	0.97	7
Plant height	1.33	0.36	3.64	0.0003	111
Random
Individual plant		12.8		<0.0001	111

^†SE for fixed effects, SD for random effects.

Week was not important in explaining seed set (estimated model coefficient 2.2 ± 1.6, t₂₅₈ = 1.4, P > 0.21), implying that effectiveness did not increase for the aggregate pollinator community. The model including the interaction between pollinator taxa and week did not explain significantly more variation in seed set than the model without this interaction (LR test, ${\chi }_7^2$ = 2, P > 0.96), implying that pollinators did not show different patterns of effectiveness through time. When introduced as fixed effects to the baseline model (Table 3), plant height had a significant positive effect on seed set (1.4 ± 0.4, t₂₅₅ = 3.5, P < 0.0006), whereas the number of co-flowering species (0.4 ± 1.7, t₂₅₅ = 0.2, P > 0.8), visit duration (−0.03 ± 0.04, t₂₅₅ = −1.0, P > 0.3), mean seed set of hand-pollinated control plants (0.2 ± 0.2, t₂₅₅ = 0.9, P > 0.4), and the number of inflorescences on plants (0.4 ± 0.7, t₂₅₅ = 0.6, P > 0.6) did not explain a significant amount of the variation in seed set.

Although seed set was greater when Apis mellifera (29.9 vs. 19.4), Polistes dominula (20.4 vs. 13.7), or Vespula arenaria (13.7 vs. 6.5) had visited a hand-pollinated field control flower before visiting a focal flower, these differences were not statistically significant (A. mellifera, t₁₂₄ = 1.26, P > 0.2; P. dominula, t₄₆ = 0.52, P > 0.6; V. arenaria, t₁₅ = 0, P = 1).

To compare between focal and open-pollinated field control flowers, we treated seed set from both as the response variable, with a categorical variable to distinguish between the two types of pollination, in an analysis analogous to T. ohiensis (Table 2). There was no significant interaction between pollinator taxa and week, or between type of pollination and week (LR test, ${\chi }_8^2$ = 10, P > 0.3), indicating no differences among pollinator taxa, or between pollinators and open pollination, in effectiveness through time (Fig. 3b; Appendix C); therefore, we do not present a table of results for this analysis. Analyzing the open-pollinated flowers separately, there was an increase in effectiveness through time (t₁₁₇ = 2.12, P < 0.04). An analysis of the pollinators in aggregate, without including a random effect for date, also showed an increase through time (t₂₆₆ = 2.10, P < 0.04).

Plants placed in the field around the current mean date of first bloom produced no seeds, whereas those placed in the field four weeks later had the highest seed set per visit (Fig. 3d; Appendix C). In contrast to T. ohiensis, 80% of the variation in seed set among weeks was explained just by aggregated pollinator importance (Fig. 3d), assuming that no pollinator taxon increased in effectiveness through time. After adding temporal changes in pollinator effectiveness, the model explained 90% of the variation in seed set among weeks.

For the weeks that overlap, visitation rates in 2010 were similar to those documented in 2009 (Rafferty and Ives 2011). Both years showed a pattern of increasing pollinator visitation rates over time (Fig. 4b). The expected pollination rate leading to successful seed set calculated from visitation rates, pollinator composition, and taxon-specific pollinator effectiveness was lowest for plants flowering around the current date of first bloom and highest for plants flowering two weeks after (Fig. 4b). Nectar volume and sugar content of greenhouse control plants increased significantly over time, although neither the time of day nor the number of inflorescences had a significant effect on either response variable (Appendix D).

Discussion

The results of this study demonstrate that pollinator effectiveness and thus seed set can vary with flowering time not only as a result of changes in pollinator taxonomic composition but also through changes in effectiveness within pollinator taxa. In the case of T. ohiensis, pollinator taxa differed in effectiveness by an order of magnitude, and three taxa increased in effectiveness over time. For A. incarnata, the among-taxa differences in effectiveness were even greater, yet their effectiveness changed little through time. Due to changes in pollinator composition and, for T. ohiensis, changes in pollinator effectiveness, seed set per visit increased with later flowering onset in both species, suggesting that there could be reproductive output costs to earlier flowering.

While our experimental manipulation does not realistically mimic the effects of changing climatic cues (Price and Waser 1998), particularly for pollinators, we gained useful information about the responses of pollinators to the availability of floral resources at different times. The lower seed set we documented in earlier-flowering plants could represent the worst-case scenario, in that pollinators did not experience the cues that, under natural conditions, would have caused plants to start flowering at different times. In this light, our results suggest that complete mismatches are unlikely, at least for plants with fairly generalized pollination systems, even if more-effective pollinators are unavailable at first flowering. In general, differences in pollinator effectiveness among species are related to morphological features, such as body size and tongue length, and/or behavioral aspects, such as visit duration and the probability of visiting conspecific flowers (Beattie 1971, Ivey et al. 2003, Sahli and Conner 2007, Theiss et al. 2007). We did not detect an effect of visit duration on pollinator effectiveness, and we did not measure body size, but on the whole smaller pollinators were less effective for both plant species.

Although we cannot rule out the possibility that the increased effectiveness of some visitors to T. ohiensis was due to limited conspecific pollen availability of earlier-flowering plants, we did not find evidence for this. First, the increased seed set with later flowering that we documented was driven in part by compositional changes in the pollinator assemblage. It is possible that the increasing density of T. ohiensis attracted new pollinator taxa into the study area; however, it seems likely that concurrent changes in the composition of the co-flowering community as a whole, which reflect the natural pattern that would be expected with different dates of T. ohiensis flowering onset, would have a much larger effect on pollinator composition. Second, the number of conspecific flowers within 5 m of the focal plant had a significant but negative influence on seed set, whereas a positive effect would be expected if the abundance of T. ohiensis flowers limited pollen availability. Third, Bombus spp. individuals that visited a pollen donor before visiting focal flowers were more effective; however, this effect was no more important for plants in the field early than for plants flowering later and so could not explain the increase in effectiveness through time. Furthermore, only some of the pollinator taxa increased in effectiveness; neither the most-nor the least-effective pollinator taxa varied in effectiveness over time, implying that pollen availability was certainly not a factor for these species. Finally, because the seed set for insect-pollinated plants increased much more than for hand-pollinated plants, the increased effectiveness of pollinators cannot be explained entirely by increased seed set potential. Thus, the changes in effectiveness within taxa are probably not a product of the experimental design or a peculiarity of the study site, and instead likely reflect true changes that are driven by pollinator behavior.

Among studies that have measured pollinator effectiveness (e.g., Ivey et al. 2003, Wiggam and Ferguson 2005, Sahli and Conner 2007), we are not aware of any that have examined changes in effectiveness on such a fine temporal scale. We can only speculate about the reasons for our results. Part of the change in effectiveness may be the result of changes in plants, since seed set of hand-pollinated T. ohiensis flowers increased through time. Also, the increasing floral quality of A. incarnata plants, as reflected in nectar volume and sugar content, may have led the pollinator taxa that visited later-flowering plants to behave in a way that resulted in more-frequent pollinaria insertion. Other plant traits that we did not isolate, such as floral odor or pollen production, may have been altered by the phenological manipulations, as well. For T. ohiensis, intraspecific changes in effectiveness may have also been caused by changes in pollinator behavior. With greater availability of pollen resources in the flowering community as a whole as the season progressed, some pollinator taxa may have groomed less pollen from their bodies (Beattie 1971), resulting in greater pollen deposition. The effectiveness of some pollinators of A. tuberosa differed between years (Fishbein and Venable 1996), and within-species differences in pollinator performance have been documented among sites, suggesting that interactions with other community members are important (Jürgens et al. 2009). Regardless of the underlying reasons, within-species changes in effectiveness indicate that pooling estimates of pollinator performance over temporal scales or treating them as static traits may obscure important variation and could lead to inaccurate predictions of the plant reproductive consequences of phenological shifts (Fig. 3a and c).

That pollinators differed so greatly in their effectiveness and that the effectiveness of some pollinators changed over the five weeks that each plant species was in the field suggest that even small shifts in flowering time could have consequences for plant reproductive success. For both T. ohiensis and A. incarnata, plants that began flowering around the current mean date of first bloom in 2010 were likely to be visited by less-effective pollinators than plants that began flowering around the historical mean date of first bloom. Whether these differences translate into net differences in seed set depends, however, on whether plants are able to compensate for lower pollination success of flowers produced early by investing more in flowers produced later. There is evidence that at least one early flowering wildflower possesses such a flexible resource allocation strategy (Forrest and Thomson 2010), although flowers produced later can have lower fecundity (Stephenson 1981, Thomson 1989). Additionally, we allowed the focal flowers only a single visit; thus, it is possible that multiple visits by less-effective pollinators could negate any differences in seed set. That the open-pollinated flowers of the A. incarnata field control plants had similar levels of seed set as focal flowers in the first two weeks indicates this is not necessarily the case, however. Similarly, although high visitation rates by an assemblage of less-effective pollinators could result in the same overall seed set as low visitation rates by more-effective pollinators, we found that visitation rates and effectiveness generally varied together.

Whatever the possible reproductive costs, the potential for temporal mismatches in the availability of the most effective pollinators and the onset of flowering exists for both T. ohiensis and A. incarnata. Whereas visitation rates were greater for T. ohiensis when flowering was advanced in 2009 (Rafferty and Ives 2011), they did not vary as drastically or as directionally in 2010. Interestingly, the greatest numbers of visits by the most effective pollinator (Augochlorella sp.) occurred in the two weeks of advanced flowering in 2009, suggesting that overall visitation rates were a reliable predictor of the potential for phenological synchrony. Interannual variation in visitation rates and the timing of visits by more-effective pollinators could oppose directional selection for earlier flowering in T. ohiensis and perhaps other species that flower early in the season. The flowering onset of A. incarnata seemed to be mismatched in terms of both overall pollinator visitation rates in 2010 and 2009 (Rafferty and Ives 2011) and visitation by the most effective pollinators, both of which increased with later flowering.

The findings of this study demonstrate the importance of measuring pollinator effectiveness over time for understanding the plant reproductive consequences of shifts in flowering onset associated with climate change. For the two plant species studied here, mismatches between current flowering onset and visitation by the most effective pollinators occurred in the study year, but several additional pieces of information are needed to understand the ultimate significance of those mismatches for plant reproductive success, especially given that these species are relatively long-lived perennials.