Abstract
The purpose of this study was to test if substituting a regular maximum strength-oriented training regimen by a power-oriented one at the optimal power load in the first phase of a traditional periodization produces similar performance improvements later on into the training period. Forty five soldiers of the Brazilian brigade of special operations with at least one year of army training experience were divided into a control group (CG - n = 15, 20.18 ± 0.72 yrs, 1.74 ± 0.06 m, 66.7 ± 9.8 kg, and 1RM/weight ratio = 1.14 ± 0.12), a traditional periodization group (TG - n = 15, 20.11 ± 0.7 yrs, 1.72 ± 0.045 m, 63.1 ± 3.6 kg, and 1RM/weight ratio = 1.21 ± 0.16); and a maximum-power group (MPG - n = 15, 20.5 ± 0.6 yrs, 1.73 ± 0.049m, 67.3 ± 9.8 kg, 1RM/weight ratio = 1.20 ± 0.14). Maximum strength (26.2% and 24.6%), CMJ height (30.8% and 39.1%) and sprint speed (11.6% and 14.5%) increased significantly (p < 0.05) and similarly for the MPG and TG, respectively, from pre- to post-assessments. Our data suggests that a power training regimen may be used in the initial phase of the training cycle without impairing performance later on into the training period.
Key points.
Training at the optimal power zone during two mesocycles of a traditional periodization did not hamper strength, speed and power performance improvements.
Additional research is required in order to find out if longer periods of training at optimal power zone are capable of producing similar performance improvements to traditional strength training regimen.
Key words: Maximum-power zone, maximum strength, mean propulsive power, mean power
Introduction
Training periodization has been considered as an important strategy to improve the performance of athletes in several sports (Issurin, 2008; 2010). The traditional periodization theory advocates that training loads with distinct orientations should be distributed along a macrocycle in order to allow athletes to achieve peak performance in the most important competition of the period (Fleck, 1999; Kraemer et al., 2003). Accordingly, the initial training phase should build a strength foundation for subsequent power development in periods closer to the competition, in power- and speed-based sports. Furthermore, the traditional periodization theory also suggests that training load should vary on a weekly basis to maximize bodily adaptations and performance improvements.
Even though the concept of developing a strength foundation before power is widely accepted among coaches and sport scientists, empirical evidence supporting such a training scheme is equivocal. In fact, there are reports of similar improvements in maximum strength and power production when comparing strength and power training regimens (Harris et al., 2000; Jones et al., 2001; Lamas et al., 2010; McBride et al., 2002). Thus, if a power training regimen produces equivalent improvements in maximum strength than those of a regular strength training regimen, it is plausible to suggest that the former could be used in the initial phase of the periodization without impairing performance improvements later into the macrocycle. Moreover, power training requires a lower total training volume when compared to a regular strength training regimen, which could be beneficial to athletes as it may reduce the risk of injuries.
Additionally, power training has a relative intensity (i.e. percentage of an exercise 1 RM), usually defined as the optimal power load (Cormie et al., 2011), in which both components of the power equation are optimized (i.e. force and velocity). This intensity produces the highest mechanical power, being considered the maximum point of a parabolic function.
Two corollary hypotheses may be obtained from the previous statement. First, the optimal power load should be the most effective training load to increase mechanical power and performance in power-dependent activities (Cormie et al., 2011; McBride et al., 2002). Second, this load would produce faster increments in performance when compared to the traditional training model as its effects may be readily transferred to performance. However, there is paucity of data supporting such a suggestion in short-term periodization models.
Therefore, the purpose of this study was to test if training at the optimal power load produces similar performance improvements than a traditional training model in a short-term periodization. We hypothesized that training at the optimal power load would produce similar improvements in maximum strength and faster initial gains in functional tests.
Methods
Experimental design
We used two experimental groups and one control group (CG) to test if training at a fixed relative intensity (i.e. optimal power load) in the first two mesocycles (i.e. 3-wk duration) of a 9-wk macrocycle would hamper performance improvements. In the first mesocycle, subjects from the maximum power group (MPG) performed high-velocity back squats at the optimal power load (i.e. 65% of 1RM load). In the second mesocycle individuals performed jump-squats also at the optimal load for this specific exercise (i.e. 45% of the 1RM load). The traditional periodization group (TG) performed a regular back squat strength-training program in which the intensity increased and the volume decreased over the first 3-wk period. In the second 3-wk period, they performed jump-squats with increasing intensity and decreasing volume. In the third 3-wk mesocycle, individuals from both groups performed counter movement jumps. The following tests were performed at the beginning and at the end of each three-week training period (i.e. 0-wk, 3-wk, 6-wk, and 9-wk): back squat 1RM test, 20-m sprint test, and countermovement jumping height. Mean power (MP) and mean propulsive power (MPP) in the high-velocity back squat exercise, and in the jump squat were also evaluated pre- and post-training (i.e. 0-wk and 9-wk).
Subjects
Forty-five soldiers of the Brazilian brigade of special operations with at least one year of army training experience (i.e. aerobic exercise, calisthenics, and strength-endurance circuit training) volunteered for this study. The subjects were balanced and randomly assigned to the following groups: control group (CG - n = 15, 20.18 ± 0.72 yrs, 1.74 ± 0.06 m, and 66.7 ± 9.8 kg); TG (n =15, 20.11 ± 0.7 yrs, 1.72 ± 0.05 m, and 63.1 ± 3.6 kg); and MPG (n =15, 20.5 ± 0.6 yrs, 1.73 ± 0.05 m, and 67.3 ± 9.8 kg). We assumed no differences between groups for nutritional status, macronutrients ingestion, and training time schedule as subjects were from the same company following a 5-day on and 2-day off in the army unit and performed all of the daily tasks together. Subjects were informed of the experimental risks and signed an informed consent form prior to the investigation. The investigation was approved by an Institutional Review Board for use of human subjects.
Back squat 1RM test
Initially, participants ran for five minutes on a treadmill at 9 km·h-1, followed by five minutes of lower limb stretching exercises. Then, they performed two back squat exercise warm-up sets. In the first one, participants performed eight repetitions with 50% of the estimated 1RM load and in the second set performed three repetitions with 70% of the estimated 1RM load. A 3-min resting interval was given between sets (Brown and Weir, 2001). Three minutes after the warm up, the actual test started with a load of approximately 90% of the subject’s body mass. Participants had up to five attempts to achieve the squat 1RM value. A 3-min interval was also allowed between attempts. Each repetition was performed from full extension up to the point in which the thighs were parallel to the floor. Strong verbal support was provided during the attempts (within-subject coefficient of variability CV < 5%).
Mean power (MP) and mean propulsive power (MPP) in the high-velocity back squat exercise
Subjects were instructed to perform two sets of three repetitions of the back squat exercise with maximal speed at 65% of the 1RM load in a Smith machine. A linear transducer (T-force, Dynamic Measurement System, Ergotech Consulting S.L., Murcia, Spain) was attached to the Smith machine bar. Bar position data was sampled at a frequency of 1000 Hz and recorded into a computer and used to determine lower. Finite differentiation technique was used to estimate the bar velocity and acceleration. Mean power and MPP on each repetition of the back squat exercise were obtained by the product of the average force and average speed, over the entire concentric phase (MP) and the positive acceleration region of the concentric phase (MPP) (Sanchez-Medina et al., 2010) (within-subject CV < 10%).
MP and MPP in the jump squat
This test was performed following the same basic procedures (i.e. number of sets and repetitions) described for the previous tests. In addition, subjects were instructed to start from a static squat position (i.e. ~90° of knee flexion) and jump as high as possible without losing contact with the bar, using a load corresponding to 45% of the squat 1RM. Mean power (MP) and MPP were calculated as previously described (Sanchez-Medina et al., 2010) (within-subject CV< 10 %).
We opted for using MP and MPP rather than peak power in both the high-velocity back squat and the jump squat as Sanchez-Medina et al., 2010 have demonstrated that referring the mean mechanical values during the propulsive phase better reflects the differences in the neuromuscular potential between two given individuals. This approach avoids underestimation of true strength potential as the higher the mean velocity is (and lower the relative load), the greater is the relative contribution of the braking phase to the entire concentric time.
Counter movement jumping height
Subjects were instructed to maintain their hands on their waist and freely determine the amplitude of the countermovement in order to avoid changes in jumping coordination. They performed five jumps with a 15-sec interval between attempts (within-subject CV < 10%). The jumps were performed on a contact platform (Winlaborat, Buenos Aires, Argentine), which measures flight time. The obtained flight time (t) was used to estimate the height of the rise of the body’s center of gravity (h) during the vertical jump (i.e., h = gt2/8, where g = 9.81 m/s2). A specific jump was considered for further analysis only the take-off and landing positions were visually similar. The best and the worst jumps were discarded and the average jumping height of the remaining jumps was used for data analysis purpose.
20-m sprint test
Two pairs of photocells were used to mark a 20m distance. Participants accelerated as much as possible for 5m before crossing the first pair of photocells and were instructed to maintain acceleration for the following 10m after crossing the second pair of photocells (within-subject CV < 10%). They had two attempts and the best one was considered for statistical analysis.
Training protocols
The training protocols were composed of regular parallel back squat exercises, JS (starting from ~90° knee flexion), and CMJ (hands on the waist and auto- adjusted countermovement amplitude). A 3-minute resting interval was allowed between exercise sets. Table 1 displays the training protocol for each group.
Table 1.
Training protocols for the traditional periodization group (TG) and the maximum power group (MPG) over the 9-week training period.
| Traditional Group | ||||||||
|---|---|---|---|---|---|---|---|---|
| wk-1 | wk-2 | wk-3 | wk-4 | wk-5 | wk-6 | wk-7 | wk-8 | wk-9 |
|
Squat (3 Sessions) |
Squat (3 Sessions) |
Squat (3 Sessions) |
JS (3 Sessions) |
JS (3 Sessions) |
JS (3 Sessions) |
CMJ (3 Sessions) |
CMJ (3 Sessions) |
CMJ (3 Sessions) |
| Set 1 (3x8/50%RM) |
Set 1 (3x6/60%RM) |
Set 1 (3x5/70%RM) |
Set 1 (3x6/30%RM) |
Set 1 (3x5/45%RM) |
Set 1 (3x4/60%RM) |
Set 1 (3x4/40CM) |
Session 1 (3x6/40CM) |
Session 1 (3x8/40CM) |
| Set 2 (3x6/55%RM) |
Set 2 (3x5/65%RM) |
Set 2 (3x4/75%RM) |
Set 2 (3x6/30%RM) |
Set 2 (3x5/45%RM) |
Set 2 (3x4/60%RM) |
Set 2 (3x4/40CM) |
Session 2 (3x6/40CM) |
Session 2 (3x8/40CM) |
| Set 3 (3x5/60%RM) |
Set 3 (3x4/70%RM) |
Set 3 (3x3/80%RM) |
Set 3 (3x6/30%RM) |
Set 3 (3x5/45%RM) |
Set 3 (3x4/60%RM) |
Set 3 (3x4/40CM) |
Set 3 (3x6/40CM) |
Session 3 (3x8/40CM) |
| Maximun Power Group | ||||||||
| wk-1 | wk-2 | wk-3 | wk-4 | wk-5 | wk-6 | wk-7 | wk-8 | wk-9 |
|
Squat (3 Sessions) |
Squat (3 Sessions) |
Squat (3 Sessions) |
JS (3 Sessions) |
JS (3 Sessions) |
JS (3 Sessions) |
CMJ (3 Sessions) |
CMJ (3 Sessions) |
CMJ (3 Sessions) |
| Set 1 (3X4/65%RM) |
Set 1 (3X5/65%RM) |
Set 1 (3X6/65%RM) |
Set 1 (3X4/45%RM) |
Set 1 (3X5/45%RM) |
Set 1 (3X6/45%RM) |
Set 1 (3X4/40cCM) |
Set 1 (3X6/40CM) |
Set 1 (3X8/40CM) |
| Set 2 (3X4/65%RM) |
Set 2 (3X5/65%RM) |
Set 2 (3X6/65%RM) |
Set 2 (3X4/45%RM) |
Set 2 (3X5/45%RM) |
Set 2 (3X6/45%RM) |
Set 2 (3X4/40CM) |
Set 2 (3X6/40CM) |
Set 2 (3X8/40CM) |
| Set 3 (3X4/65%RM) |
Set 3 (3X5/65%RM) |
Set 3 (3X6/65%RM) |
Set 3 (3X4/45%RM) |
Set 3 (3X5/45%RM) |
Set 3 (3X6/45%RM) |
Set 3 (3X4/40CM) |
Set 3 (3X6/40CM) |
Set 3 (3X8/40CM) |
Squat, JS and CMJ represent the training exercise.
Statistical analysis
It was assured the normal distribution of the data (Shapiro-Wilk test) and the absence of extreme observations (visual analysis) in each group. As the experimental groups were balanced and randomized based on squat 1 RM values, a number of one-way ANOVA were used to test for differences in the initial values between groups for all dependent variables. There were no differences in the initial values for the back squat 1 RM, CMJ height, and average speed in the 20-m sprint test. However, mean power and mean propulsive power in the back squat and jump squat exercises presented significant differences between groups.
Mixed models having group (CG, MPG, and TG) and time (0 wk, 3 wk, 6 wk, and 9 wk) as fixed factors, and participants as random factor were used for the variables that did not present significant differences between groups (back squat 1 RM, CMJ height, and average speed in the 20m sprint test) (Ugrinowitsch et al., 2004).
For the variables that presented significant differences in the initial values, a number of mixed models having groups as fixed factor, pre-test mean power and mean propulsive power in the 65% 1 RM high velocity back squat and 45% 1 RM jump squat as covariates, and participants as a random factor were used for covariance analysis. In case of significant F-values a Tukey adjustment was used for multiple comparison purposes. Significance level was set at p ≤ 0.05. Data are presented as mean ± SD.
Results
MPG and TG presented significant increments in maximum strength (26.2% and 24.6%, respectively), CMJ height (30.8% and 39.1%, respectively) and 20- m sprint speed (11.6% and 14.5%, respectively) from the pre- to the post-training assessment (p ≤ 0. 05). There were no differences between the training groups in the rate of increment of these variables (Figure 1) (p ≥ 0.05). The CG did not present significant changes in these variables from the pre- to the post-test (p ≥ 0.05).
Figure 1.

Maximum strength (squat 1 RM, kg – panel A), counter movement jump height (cm – panel B) and 20 m sprint speed (m·s-1 - panel C) pre- and post-training for the control (CG), maximal power (MPG), and traditional periodization (TG) groups, at the instants 0-wk (pre-training), 3-wk, 6-wk, and 9-wk (post-training) (Mean ± SD). *, # and † - p≤0.05 compared to the control group at the same time point.
The training groups (MPG and TG) had significantly higher mean power and mean propulsive power at both the high velocity back squat and the jump squat tests (p ≤ 0.05) than the CG, after the training period (Figure 2 A, B, C AND D). Overall, these variables were consistently higher for both training groups when compared to the CG in the post-training (24. 98%) assessment.
Figure 2.
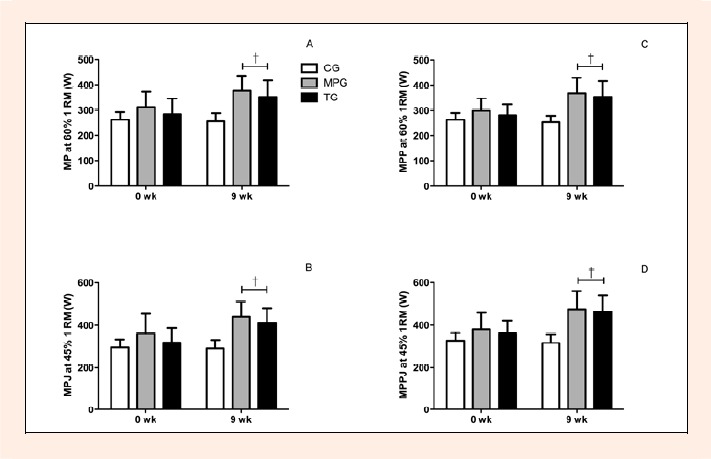
Mean power (MP - W) and mean propulsive power (MPP - W) in the squat exercise with 60% of the squat 1 RM (panels A and C), mean power (MPJ - W) and mean propulsive power (MPPJ - W) in the jump-squat exercise with 45% of the squat 1 RM (panels B and D), pre- and post-training for the control (CG), maximal power (MPG), and traditional periodization (TG) groups, at the instants 0-wk (pre-test) and 9-wk (post-test) (Mean ± SD). † - p≤0.05 compared to the control group at the same time point
Figure 3 depicts individual data over the 9-week training period for the MPG and TG groups. Besides two subjects in the TG group that had a very steep increment in CMJ height, the individual responses were very similar between groups.
Figure 3.
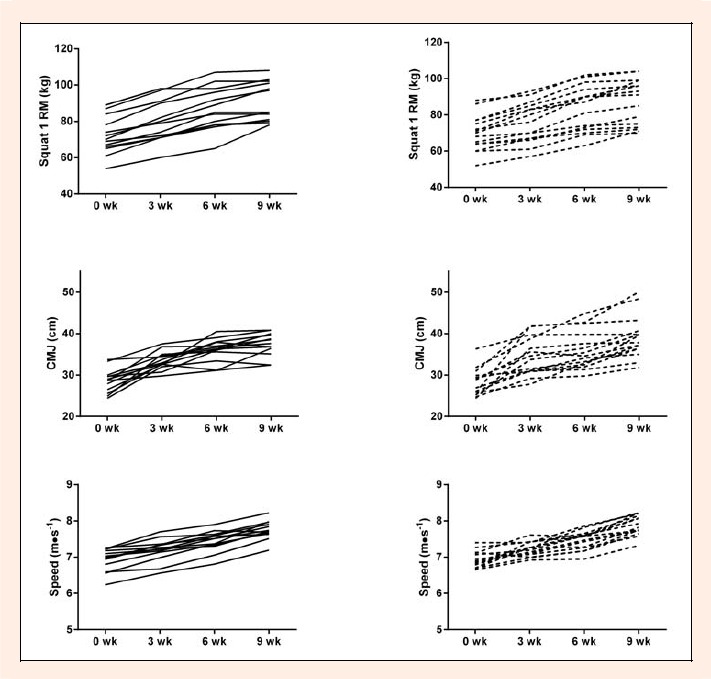
Individual responses of the squat 1RM (upper panels), countermovement jump height (middle panels), and 20-m sprint speed (lower panels) for the maximum power group (MPG – left column) and traditional periodization group (TG – right column) at 0-wk (pre-test), 3-wk, 6-wk, and 9-wk (post-test) time points.
Discussion
The purpose of this study was to test if a short-term periodization model at the optimal power load would be as effective as a traditional periodization model to increase performance. It was hypothesized that the MPG would present greater and similar functional adaptations at the initial and at the later phases of a short-term periodization model, respectively, than the TG. The main findings of the present study were: a) the MPG showed similar increments in maximum strength than the TG over the 9-wk training period (i.e. at the 3-wk, 6-wk, and 9-wk tests); b) training at the optimal power load did not produce faster performance improvements in power-related tests; and c) there were no differences in functional tests after the 9-week training period between the MPG and TG.
Regarding maximum strength improvements, Jones et al., 2001 presented gains of 16.3% and 11.0% for the strength training and power training groups, respectively, after a 10-wk training period. Similarly, Lamas et al., 2010 described increments of 22.8% and 16.6% after 8 weeks of a maximum strength and power training programs, respectively. Neither study reported significant differences in 1 RM values between the strength and power training groups. In the present study, the MPG and the TG increased maximum strength by 26.2% and 24.6%, respectively. Taken together, these findings support the concept that training at the optimal power load does not hamper muscle force production capacity, at least during short-term macrocycles.
Both the MPG and TG groups had significant and similar strength increments from the pre-training assessment up to the sixth week of training (20.8% and 19.6%, respectively). Furthermore, the largest increase in strength occurred from the third week to the sixth week of training for both the MPG and the TG (9.2% and 10.4%, respectively). As both training groups performed jump squats during the second 3-wk cycle (i.e. from week 4 to 6), it may also be suggested that this exercise is also effective to increase maximum strength. However, caution should be exercised in generalizing this suggestion. For instance, McBride et al., 2002 used light (30% of the squat 1 RM) and heavy (80% of the squat 1 RM) loads in an 8-week jump-squat training program for trained individuals. These authors reported increments in maximum strength of 8.2% and 10.2% for the light- and heavy-load groups, respectively. These increments are smaller than those reported herein, especially when taking into consideration that the present study only used three weeks of jump-squat training. Thus, it seems that participants’ training background may modulate the magnitude of performance changes. Our subjects may be considered weaker (squat 1RM ~around 1.5 body weight) when compared to McBride et al.’s (2002) study (squat 1RM ~around 2.0 body weight). Another possible explanation for the great increments in strength reported in the present study may be the usage of cycles in which different training exercises were employed. It is feasible that both the strength and the power training regimens used in the first 3-wk cycle allowed maximization of the strength gains at the end of the second 3-wk cycle, when jump squats were employed.
Countermovement jumping height also presented significant increments (MPG-30.8% and TG-39.1%) from pre- to post-training tests. Other studies reported smaller increments in CMJ height compared to ours. For example, Tricoli et al., 2005 reported increments in CMJ height of 6.3% and 5.7% after an 8-wk training intervention combining heavy squats and Olympic lifts, and heavy squats and plyometrics, respectively, in physically active individuals. Similarly, Harris et al., 2000 reported smaller increments in jumping height (i.e. 3.8%) after 9 weeks of high-power training in college football players. The reasons for such a discrepancy in jumping height increments are hard to reconcile. However, a low reliability in the jump data presented herein must be ruled out as the CG group presented a coefficient of variation lower than 2% among the four assessments (0wk, 3wk, 6wk, and 9wk).
Significant increases in sprint capacity were observed in both training groups (MPG and TG, ~5%). Ronnestad et al., 2008 also reported increments in the 40-m sprint time (~1.1%) after a 7-wk training program which combined heavy strength exercises and plyometrics. Nevertheless, there were no increases in the 40- m sprint time in the heavy strength exercise group. Harris et al., 2000 reported no increments in sprint ability after a 7-wk training program at either 80% of the 1 RM or at the optimal power load (using jump squats). Thus, it seems that either combining or changing the characteristics of the training load along the training cycle may be important to change the sprint ability.
Several aspects should be emphasized regarding the distribution of training loads with distinct orientations along a short-term macrocycle. As previously mentioned, training at the optimal power load produced similar strength increments than regular strength training. Thus, it can be suggested that power training is as effective as strength training regimens in developing a strength foundation during a macrocycle.
The absence of faster initial performance improvements in the MPG is somewhat puzzling, as the MPG did not present a more rapidly improvement in performance compared to the TG (Cormie, et al., 2011; McBride, et al., 2002). A possible reason for such findings is the occurrence of a large braking phase during the high-velocity back squat to prevent from taking-off at the end of each repetition (Sanchez-Medina, et al., 2010). Several motor skills, such as vertical jump and sprinting, require the maximization of the propulsive forces throughout the range of motion. Thus, it may be speculated that the large braking phase during the high velocity back squat may have hampered a faster increment in performance in the TG. The significant 1-RM increments presented by both groups during the second 3-wk phase of our training cycle (i.e. the jump squat phase) suggests that jump squats may be more effective for the purpose of rapidly increasing maximum strength and power production capacity (McBride, et al., 2002) in the first phase of a traditional periodization. However, as mentioned before, using jump-squats as the only strength-exercise may produce lower strength gains which may impair performance improvements later into the macrocycle.
It should be emphasized that reports regarding the optimal power load present different results. Interestingly, Cormie et al., 2008 reported that maximum power is achieved during unloaded jump squats and that mechanical power decreases as a function of the jump squat load. However, caution should be taken when analyzing such findings as the optimal load seems to be a function of the subject’s training experience. For instance, the participants in Cormie’s study presented lower amplitude of the jump squat concentric phase as the load increased. These results may indicate that individuals might have anticipated take-off. In our experience, trained individuals are capable of accelerating the trunk throughout the squat range of motion. On the other hand, novice lifters reduce the range of motion of the concentric phase, decreasing the time of force application in the bar and, consequently, its peak velocity. Unfortunately, Cormie’s study and the present one have no kinematic data to support such a hypothesis. Furthermore, it has been demonstrated that power production is inversely related to the exercise load for weaker individuals, while stronger ones presented peak power with exercise loads greater than body weight only (i.e. 60% 1RM) (Alcaraz, Romero-Arenas, Vila, & Ferragut, 2011; Turner, Unholz, Potts, & Coleman, 2012).
Conclusion
In summary, the data presented herein is in accordance with previous findings and suggest that performance increments are associated with strength increases (Stone et al., 2003; Lamas et al., 2012; Cormie et el. 2010). However, the characteristics of the training regimen do not seem to be critical as long as it increases maximum strength, which is supported by the fact that training at the optimal power zone during two mesocycles of a traditional periodization did not hamper performance improvements.
Acknowledgements
The results of the present study do not constitute endorsement of the product by the authors or the journal. The authors declare that they have no conflict of interest. C.U. (470207/2008-6) and VT (304814/2010-5) are supported by CNPq.
Biographies
Irineu Loturco
Employment
Pão de Açúcar Group – Nucleus of High Performance in Sports, São Paulo, SP - Brazil
Degree
MSc
Research interests
Training periodization, power and strength training for physical performance in high-level sports.
E-mail: irineu.loturco@terra.com.br
Carlos Ugrinowitsch
Employment
School of Physical Education and Sport, University of São Paulo, SP - Brazil
Degree
PhD
Research interests
Neuromuscular adaptations due to resistance training, training periodization.
E-mail: ugrinowi@usp.br
Hamilton Roschel
Employment
School of Physical Education and Sport, University of São Paulo, SP - Brazil
Degree
PhD
Research interests
Neuromuscular adaptations to strength training, training periodization and therapeutical effects on muscle diseases.
E-mail: hars@usp.br
Valmor Tricoli
Employment
School of Physical Education and Sport, University of São Paulo, SP - Brazil
Degree
PhD
Research interests
Neuromuscular adaptations to strength training
E-mail: vtricoli@usp.br
Juan José González-Badillo
Employment
Faculty of Sport, Pablo de Olavide University, Seville, Spain
Degree
PhD
Research interests
Strength training and conditioning; identification of determinant factors responsible for physical performance in high-level sports.
E-mail: jjgonbad@upo.es
References
- Alcaraz P.E., Romero-Arenas S., Vila H., Ferragut C. (2011) Power-load curve in trained sprinters. Journal of Strength and Conditioning Research 25(11), 3045-3050 [DOI] [PubMed] [Google Scholar]
- Brown L.E., Weir J.P. (2001) ASEP procedures recommendation I: accurate assessment of muscular strength and power. Journal of Exercise Physiology Online 4(3), 1-21 [Google Scholar]
- Cormie P., McBride J.M., McCaulley G.O. (2008) Power-time, force-time, and velocity-time curve analysis during the jump squat: impact of load. Journal of Applied Biomechanics 24(2), 112-120 [DOI] [PubMed] [Google Scholar]
- Cormie P., McGuigan M.R., Newton R.U. (2010) Adaptations in athletic performance after ballistic power versus strength training. Medicine and Science in Sports and Exercise 42(8), 1582-1598 [DOI] [PubMed] [Google Scholar]
- Cormie P., McGuigan M.R., Newton R.U. (2011) Developing maximal neuromuscular power: part 2 - training considerations for improving maximal power production. Sports Medicine, 41(2), 125-146 [DOI] [PubMed] [Google Scholar]
- Fleck S.J. (1999) Periodized strength training: a critical review. Journal of Strength and Conditioning Research, 13(1), 82-89 [Google Scholar]
- Harris G.R., Stone M.H., O’Bryant H.S., Proulx C.M., Johnson R.L. (2000) Short-term performance effects of high power, high force, or combined weight-training methods. Journal of Strength and Conditioning Research 14(1), 14-20 [Google Scholar]
- Issurin V.B. (2008) Block periodization versus traditional training theory: a review. The Journal of Sports Medicine and Physical Fitness 48(1), 65-75 [PubMed] [Google Scholar]
- Issurin V.B. (2010) New horizons for the methodology and physiology of training periodization. Sports Medicine 40(3), 189-206 [DOI] [PubMed] [Google Scholar]
- Jones K., Bishop P., Hunter G., Fleisig G. (2001) The effects of varying resistance-training loads on intermediate- and high-velocity-specific adaptations. Journal of Strength and Conditioning Research 15(3), 349-356 [PubMed] [Google Scholar]
- Kraemer W.J., Hakkinen K., Triplett-McBride N.T., Fry A.C., Koziris L.P., Ratamess N.A., Bauer J.E., Volek J.S., McConnel T., Newton R.U., Gordon S.E., Cummings D., Hauth J., Pullo F., Lynch J.M., Fleck S.J., Mazzetti S.A., Knuttgen H.G. (2003) Physiological changes with periodized resistance training in women tennis players. Medicine and Science in Sports and Exercise 35(1), 157-168 [DOI] [PubMed] [Google Scholar]
- Lamas L., Ugrinowitsch C., Rodacki A., Pereira G., Mattos E.C., Kohn A.F., Tricoli V. (2012) Effects of strength and power training on neuromuscular adaptations and jumping movement pattern and performance. Journal of Strength and Conditioning Research 26(12), 3335-3344 [DOI] [PubMed] [Google Scholar]
- Lamas L., Aoki M.S., Ugrinowitsch C., Campos G.E., Regazzini M., Moriscot A.S., Tricoli V. (2010) Expression of genes related to muscle plasticity after strength and power training regimens. Scandinavian Journal of Medicine & Science in Sports 20(2), 216-225 [DOI] [PubMed] [Google Scholar]
- McBride J.M., Triplett-McBride T., Davie A., Newton R.U. (2002) The effect of heavy- vs. light-load jump squats on the development of strength, power, and speed. Journal of Strength and Conditioning Research 16(1), 75-82 [PubMed] [Google Scholar]
- Ronnestad B.R., Kvamme N.H., Sunde A., Raastad T. (2008) Short-term effects of strength and plyometric training on sprint and jump performance in professional soccer players. Journal of Strength and Conditioning Research 22(3), 773-780 [DOI] [PubMed] [Google Scholar]
- Sanchez-Medina L., Perez C.E., Gonzalez-Badillo J.J. (2010) Importance of the propulsive phase in strength assessment. International Journal of Sports Medicine 31(2), 123-129 [DOI] [PubMed] [Google Scholar]
- Stone M.H., O’Bryant H.S., McCoy L., Coglianese R., Lehmkuhl M., Schilling B. (2003) Power and maximum strength relationships during performance of dynamic and static weighted jumps. Journal of Strength and Conditioning Research 17(1), 140-147 [DOI] [PubMed] [Google Scholar]
- Tricoli V., Lamas L., Carnevale R., Ugrinowitsch C. (2005) Short-term effects on lower-body functional power development: weightlifting vs. vertical jump training programs. Journal of Strength and Conditioning Research 19(2), 433-437 [DOI] [PubMed] [Google Scholar]
- Turner A.P., Unholz C.N., Potts N., Coleman S.G. (2012) Peak power, force, and velocity during jump squats in professional rugby players. Journal of Strength and Conditioning Research 26(6), 1594-1600 [DOI] [PubMed] [Google Scholar]
- Ugrinowitsch C., Fellingham G.W., Ricard M.D. (2004) Limitations of ordinary least squares models in analyzing repeated measures data. Medicine and Science in Sports and Exercise 36(12), 2144-2148 [DOI] [PubMed] [Google Scholar]


